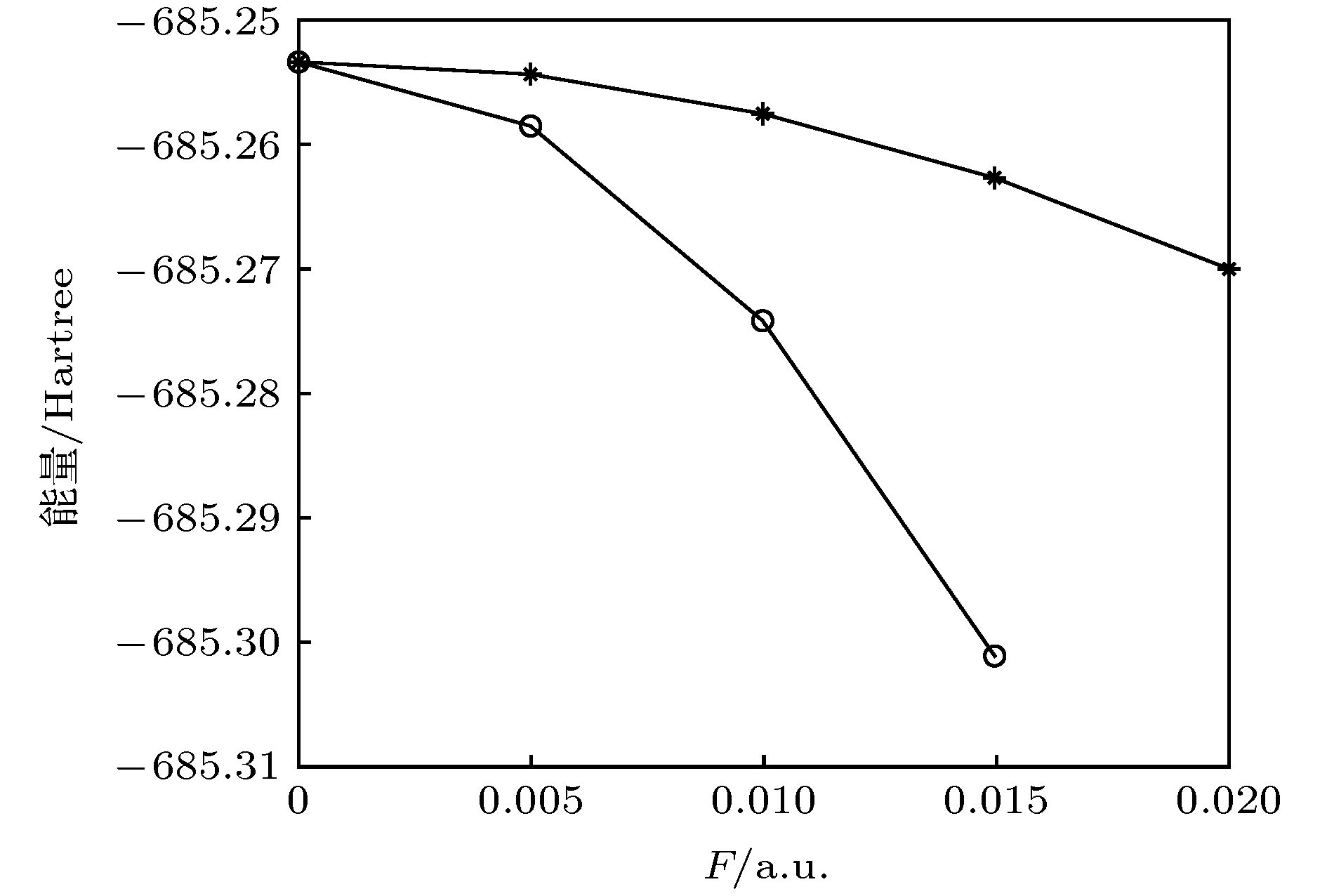
-
采用密度泛函理论方法(ωB97XD/def2-TZVP)研究了沿不同方向(x, y, z)加电场对环形C18的基态几何结构、能量、电子结构、芳香性、红外及拉曼光谱特性的影响; 继而采用含时的TD-ωB97XD方法研究了C18在外电场下的激发特性. 研究结果表明: 外电场导致分子对称性降低, 偶极矩随外电场的增加逐渐增加, 体系总能量和LUMO-HOMO能隙随着外电场的增加一直减小. 外电场将改变环上π电子的离域特征以及分子芳香性, 如分子z方向加入电场将减弱π电子离域性及分子芳香性, 分子x或y方向加入电场可以增强π电子离域性及分子芳香性. 外电场将改变红外光谱特征, 如谐振频率的移动以及红外峰的增强或减弱. 外电场对环形C18的激发特性影响较大, 如当分子y方向加电场时, 激发波长发生红移; 同时对振子强度有很大影响, 原来振子强度很强的激发态变弱或成为禁阻跃迁, 而原来振子强度很弱或禁阻的激发态变强. 可以通过改变外电场来控制C18的基态性质和光谱特性, 促进C18在分子器件等纳米领域的应用.In this work, density functional theory method with the ωB97XD/def2-TZVP level is carried out to investigate the ground state structures, energy, electronic structures, aromaticity, infrared and Raman spectra of cyclo[18]carbon under different external electric field in the x, y and z direction of cyclo[18]carbon molecule. The excitation properties (the first 48 excited states containing excited energies, excited wavelengths and oscillator strengths) of cyclo[18]carbon are calculated by the time-dependent density functional theory method (TD-ωB97XD) with the def2-TZVP basis set under the same external electric field. The results show that cyclo[18]carbon can be elongated in the x or y direction under the electric field, and some C-C bond lengths can be elongated or shortened under the electric field. Meanwhile, the calculated results show that electric dipole moment is proved to be increasing with the increase of the external field intensity, but the total energy and LUMO-HOMO gap are proved to decrease with the increase of external field intensity. Moreover, addition of electric field can modify the electron delocalization and molecular aromaticity, such as external electric field in z direction can lower the electron delocalization and molecular aromaticity and external electric field in x or y direction can enhance the electron delocalization and molecular aromaticity. The addition of electric field can modify the infrared spectra, such as shift of vibrational frequencies and strengthening of infrared peaks. Furthermore, the calculated results indicate that the external electric field has significant effects on the excitation properties of cyclo[18]carbon. The increase of the electric field intensity can lead to the redshift of transition wavelengths (such as the first excited state). With the change of the electric field intensity, the stronger excited state (with the bigger oscillator strength) can become weak (with the small oscillator strength) or optically inactive (with the oscillator strength of zero). Meanwhile, the weak or optically inactive excited state can become stronger excited state by the external field. The ground state properties and excitation properties of cyclo[18]carbon can be modified by the external electric field. Our works can provide theoretical guidance for the application of cyclo[18]carbon in the nanotechnology such as molecular device.
-
Keywords:
- C18 /
- external electric field /
- ground state /
- excitation properties
[1] Kroto H W, Heath J R, O’Brien S C, Curl R F, Smalley R E 1985 Nature 318 162
Google Scholar
[2] Iijima S 1991 Nature 354 56
Google Scholar
[3] Novoselov K S, Geim A K, Morozov S V, Jiang D, Zhang Y, Dubonos S V, Grigorieva I V, Firsov A A 2004 Science 306 666
Google Scholar
[4] Parasuk V, Almlof J, Feyereisen M W 1991 J. Am. Chem. Soc. 113 1049
Google Scholar
[5] Torelli T, Mitas L 2000 Phys. Rev. Lett. 85 1702
Google Scholar
[6] Arulmozhiraja S, Ohno T 2008 J. Chem. Phys. 128 114301
Google Scholar
[7] Fowler P W, Mizoguchi N, Bean D E, Havenith R W 2009 Chemistry 15 6964
Google Scholar
[8] Kaiser K, Scriven L M, Schulz F, Gawel P, Gross L, Anderson H L 2019 Science 365 1299
Google Scholar
[9] Nandi A, Solel E, Kozuch S 2020 Chemistry 26 625
Google Scholar
[10] Pereira Z S, da Silva E Z 2020 J. Phys. Chem. A 124 1152
Google Scholar
[11] Pichierri F 2020 Chem. Phys. Lett. 738 136860
Google Scholar
[12] Shi B, Yuan L, Tang T, Yuan Y, Tang Y 2020 Chem. Phys. Lett. 741 136975
Google Scholar
[13] Stasyuk A J, Stasyuk O A, Solà M, Voityuk A A 2020 Chem. Commun. 56 352
Google Scholar
[14] 史丹丹, 张西沙, 张德清 2018 化学进展 30 658
Google Scholar
Shi D D, Zhang X S, Zhang D Q 2018 Progress in Chemistry 30 658
Google Scholar
[15] Yamaguchi Y, Takubo M, Ogawa K, Nakayama K, Koganezawa T, Katagiri H 2016 J. Am. Chem. Soc. 138 11335
Google Scholar
[16] Sluysmans D, Stoddart J F 2019 Trends in Chemistry 1 185
Google Scholar
[17] Perez E M 2017 Chemistry 23 12681
Google Scholar
[18] Castelvecchi D 2019 Nature 572 426
Google Scholar
[19] 杨涛, 刘代俊, 陈建钧 2016 65 053101
Google Scholar
Yang T, Liu D J, Chen J J 2016 Acta Phys. Sin. 65 053101
Google Scholar
[20] 李世雄, 张正平, 隆正文, 秦水介 2017 66 103102
Google Scholar
Li S X, Zhang Z P, Long Z W, Qin S J 2017 Acta Phys. Sin. 66 103102
Google Scholar
[21] 杜建宾, 冯志芳, 张倩, 韩丽君, 唐延林, 李奇峰 2019 68 173101
Google Scholar
Du J B, Feng Z F, Zhang Q, Han L J, Tang Y L, Li Q F 2019 Acta Phys. Sin. 68 173101
Google Scholar
[22] 李亚莎, 孙林翔, 周筱, 陈凯, 汪辉耀 2020 69 013101
Google Scholar
Li Y S, Sun L X, Zhou X, Chen K, Wang H Y 2020 Acta Phys. Sin. 69 013101
Google Scholar
[23] Shaik S, Mandal D, Ramanan R 2016 Nat. Chem. 8 1091
Google Scholar
[24] English N J, Waldron C J 2015 Phys. Chem. Chem. Phys. 17 12407
Google Scholar
[25] Foroutan-Nejad C, Andrushchenko V, Straka M 2016 Phys. Chem. Chem. Phys. 18 32673
Google Scholar
[26] Weigend F, Ahlrichs R 2005 Phys. Chem. Chem. Phys. 7 3297
Google Scholar
[27] Chai J D, Head-Gordon M 2008 Phys. Chem. Chem. Phys. 10 6615
Google Scholar
[28] Frisch M J et al. 2009 Gaussian 09 (Revision A.02) (Gaussian Inc., Wallingford, CT)
[29] Lu T, Chen F 2012 J. Comput. Chem. 33 580
Google Scholar
[30] Mayer I 1983 Chem. Phys. Lett. 97 270
Google Scholar
[31] Schmider H L, Becke A D 2000 J. Mol. Struct.THEOCHEM 527 51
Google Scholar
[32] Becke A D, Edgecombe K E 1990 J. Chem. Phys. 92 5397
Google Scholar
[33] Matito E 2016 Phys. Chem. Chem. Phys. 18 11839
Google Scholar
-
图 1 分子结构与轨道图 (a) 沿z方向观察, z正方向垂直纸面向里; (b) HOMO; (c) LUMO
Fig. 1. Optimized structure and molecular orbitals: (a) Structure observed along the z direction; (b) HOMO; (c) LUMO.
图 2 π轨道的定域化轨道函数, 等值面数值为0.45 (a) 环平面上/下π轨道的LOL; (b) 环内/外π轨道的LOL
Fig. 2. Localized orbital locator calculated based on π orbitals, the isovalue is set to 0.45: (a) LOL of out-plane π orbitals; (b) LOL of in-plane π orbitals.
图 3 不同电场下C18的能量, 圆圈标记y方向加电场, 星号标记z方向加电场
Fig. 3. The energies of C18 under different external electric fields. Circle and asterisk represent the external electric fields in y and z direction, respectively.
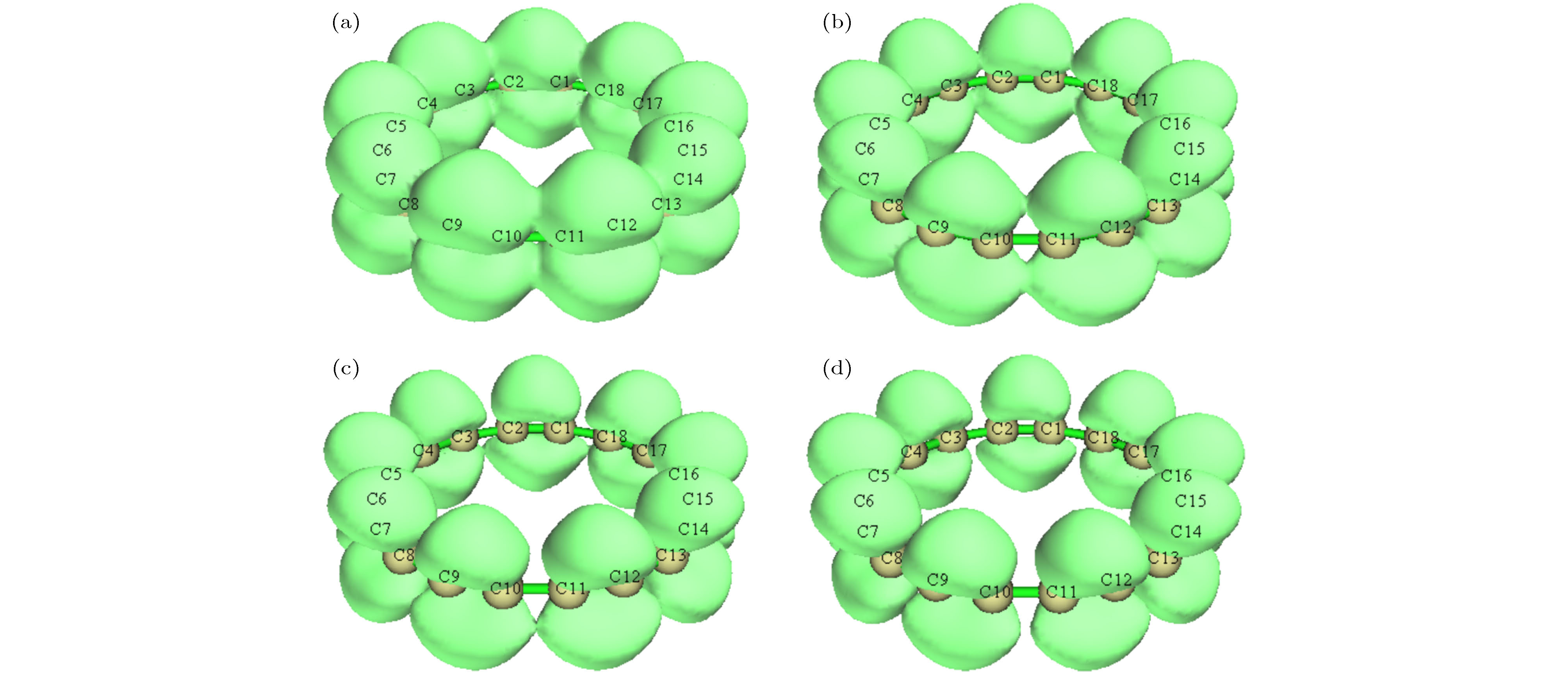
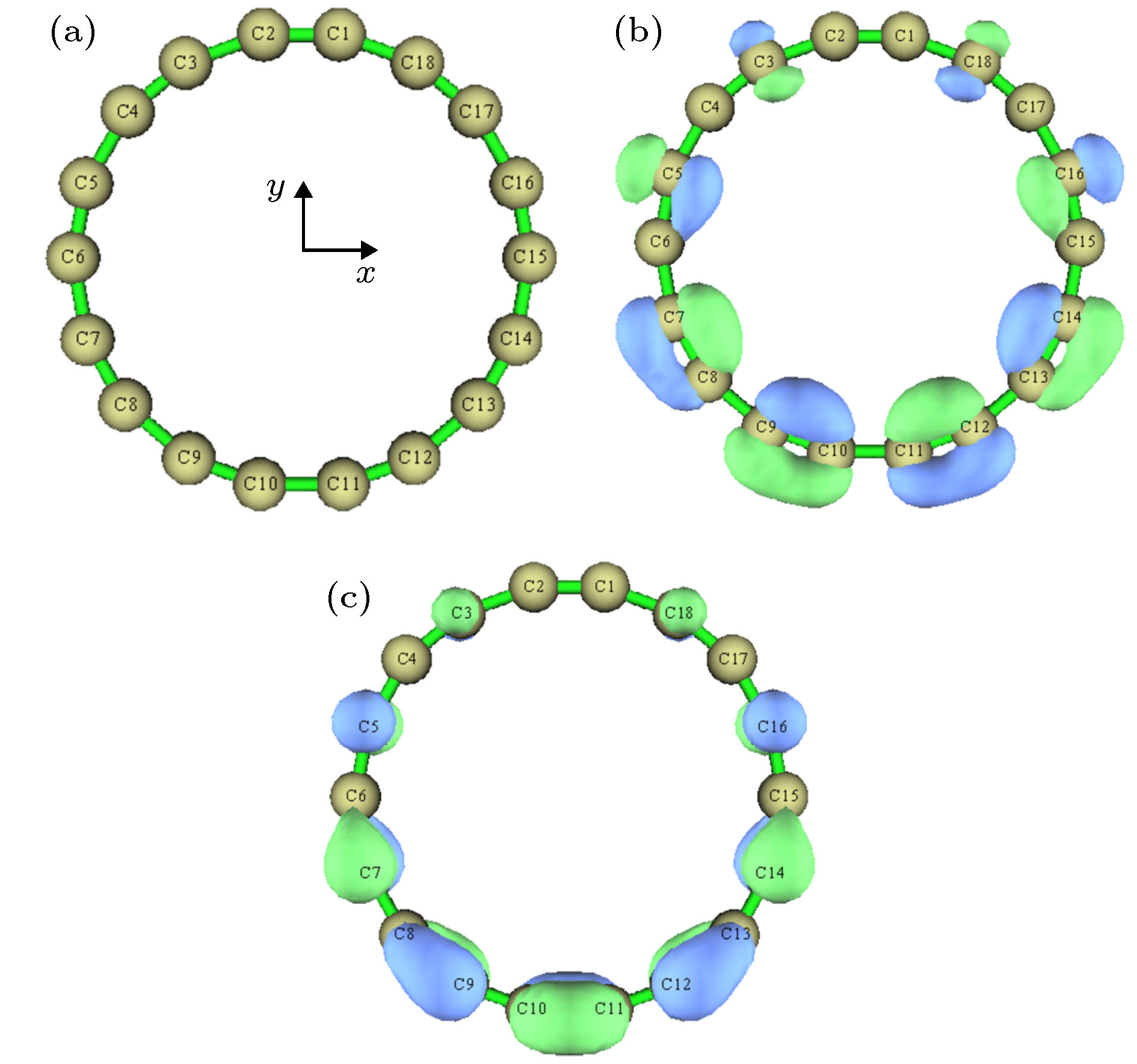
图 4 z方向外电场下环平面上/下(out-plane)π轨道的LOL, 等值面数值为0.38 (a) 0 a.u.; (b) 0.005 a.u.; (c) 0.01 a.u.; (d) 0.015 a.u.
Fig. 4. Localized orbital locator calculated based on out-plane π orbitals under different external electric fields in the z direction, the isovalue is set to 0.38: (a) 0 a.u.; (b) 0.005 a.u.; (c) 0.01 a.u.; (d) 0.015 a.u..
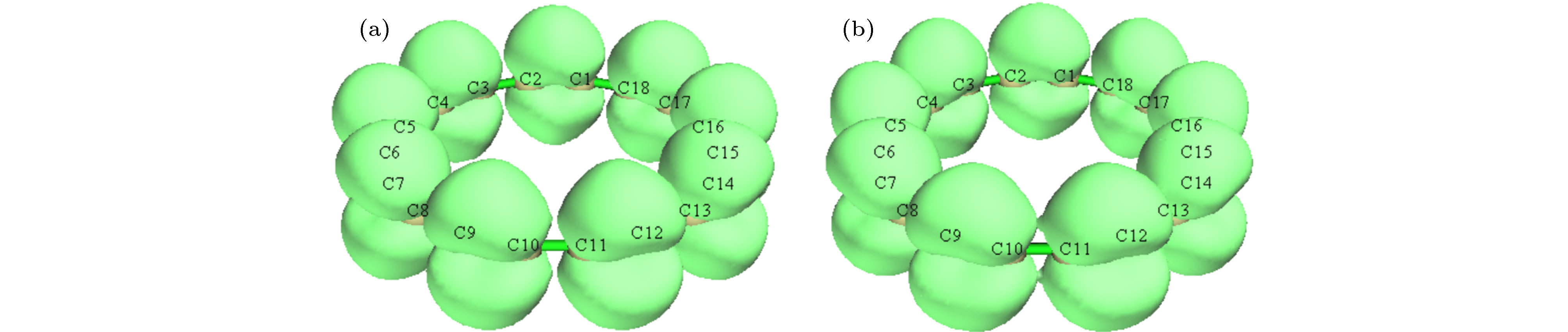
图 5 z方向外电场下环平面上/下(out-plane)π轨道的ELF, 等值面数值为0.38 (a) 0 a.u.; (b) 0.005 a.u.; (c) 0.01 a.u.; (d) 0.015 a.u.
Fig. 5. Electron localization function calculated based on out-plane π orbitals under different external electric fields in the z direction, the isovalue is set to 0.38: (a) 0 a.u.; (b) 0.005 a.u.; (c) 0.01 a.u.; (d) 0.015 a.u..
图 6 y方向外电场下环平面上/下π轨道的LOL, 等值面数值为0.52 (a) 0 a.u.; (b) 0.015 a.u.
Fig. 6. LOL calculated based on out-plane π orbitals under different external electric fields in the y direction, the isovalue is set to 0.52: (a) 0 a.u.; (b) 0.015 a.u..
图 7 y方向外电场下环平面上/下π轨道的ELF, 等值面数值为0.61 (a) 0 a.u.; (b) 0.015 a.u.
Fig. 7. ELF calculated based on out-plane π orbitals under different external electric fields in the y direction, the isovalue is set to 0.61: (a) 0 a.u.; (b) 0.015 a.u..
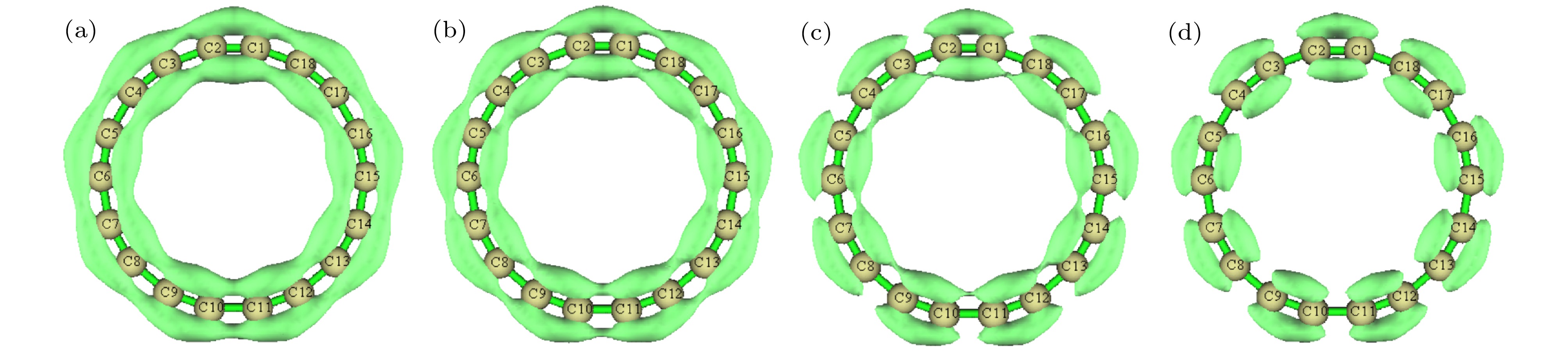
图 8 z方向外电场下环内/外(in-plane)π轨道的LOL, 等值面数值为0.37 (a) 0 a.u.; (b) 0.005 a.u.; (c) 0.01 a.u.; (d) 0.015 a.u.
Fig. 8. LOL calculated based on in-plane π orbitals under different external electric fields in the z direction, the isovalue is set to 0.37: (a) 0 a.u.; (b) 0.005 a.u.; (c) 0.01 a.u.; (d) 0.015 a.u..
图 9 y方向外电场下环内/外(in-plane)π轨道的ELF, 等值面数值为0.62 (a) 0 a.u.; (b) 0.015 a.u.
Fig. 9. ELF calculated based on in-plane π orbitals under different external electric fields in the y direction, the isovalue is set to 0.62: (a) 0 a.u.; (b) 0.015 a.u..
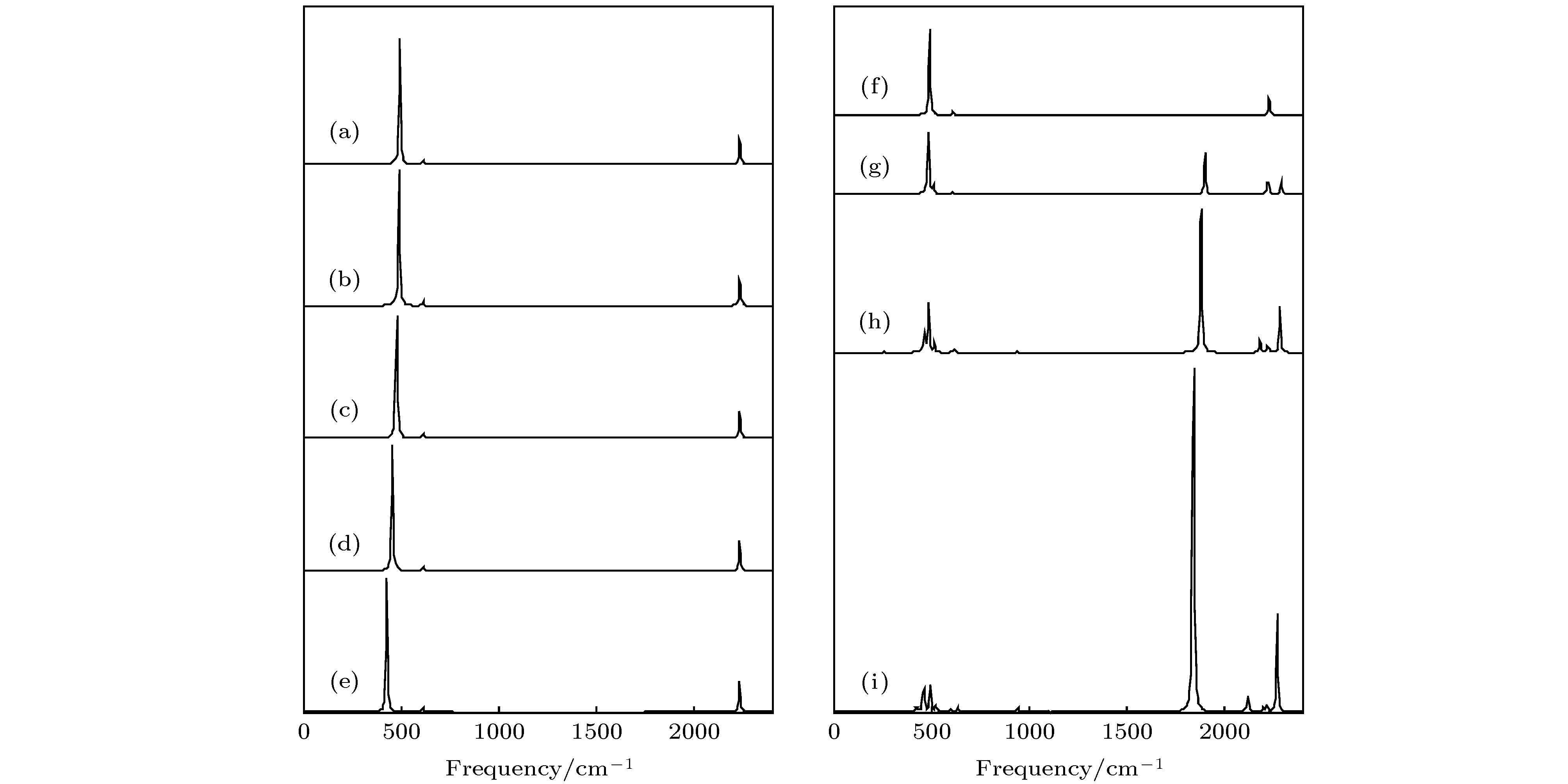
图 10 红外光谱随外电场的变化, 左边为随z方向电场变化, 右边是随y方向电场变化 (a) 0 a.u.; (b) 0.005 a.u.; (c) 0.01 a.u.; (d) 0.015 a.u.; (e) 0.02 a.u.; (f) 0 a.u.; (g) 0.005 a.u.; (h) 0.01 a.u.; (i) 0.015 a.u.
Fig. 10. Calculated infrared spectra based on different external electric fields in the z direction (left side) and y direction (right side): (a) 0 a.u.; (b) 0.005 a.u.; (c) 0.01 a.u.; (d) 0.015 a.u.; (e) 0.02 a.u.; (f) 0 a.u.; (g) 0.005 a.u.;(h) 0.01 a.u.; (i) 0.015 a.u..
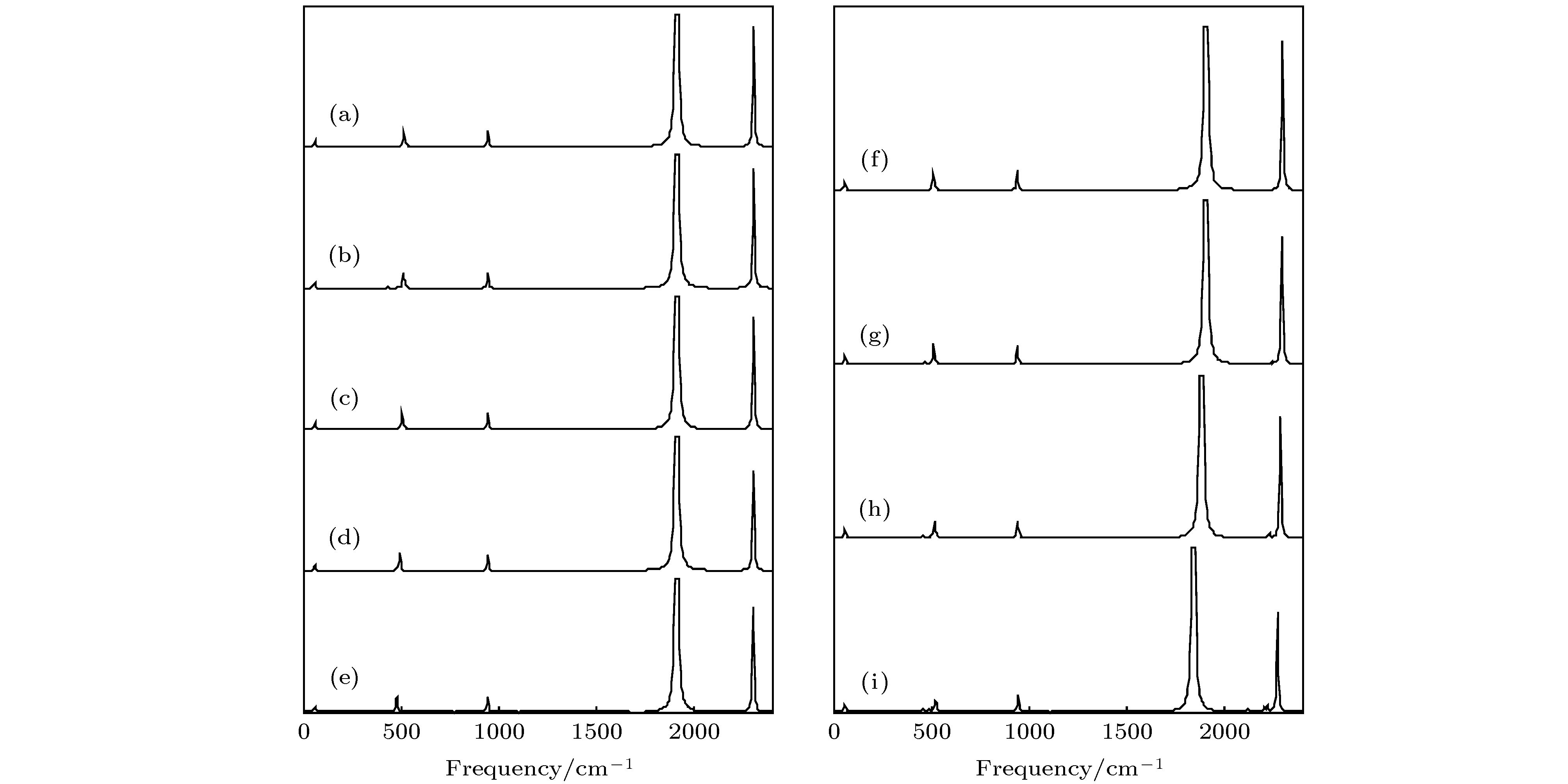
图 11 拉曼光谱随外电场的变化, 左边为z方向加电场, 右边是y方向加电场 (a) 0 a.u.; (b) 0.005 a.u.; (c) 0.01 a.u.; (d) 0.015 a.u.; (e) 0.02 a.u.; (f) 0 a.u.; (g) 0.005 a.u.; (h) 0.010 a.u.; (i) 0.015 a.u.
Fig. 11. Calculated Raman spectra based on different external electric fields in the z direction (left side) and y direction (right side): (a) 0 a.u.; (b) 0.005 a.u.; (c) 0.01 a.u.; (d) 0.015 a.u.; (e) 0.02 a.u.; (f) 0 a.u.; (g) 0.005 a.u.;(h) 0.01 a.u.; (i) 0.015 a.u..
表 1 不同电场下的轨道能量EH, EL, EH–1, EH–2, EH–3, EL+1, EL+2, EL+3以及Eg. 轨道能量的单位是Hartree (1 Hartree = 2625.5 kJ/mol), 上标x, y, z分别表示x, y, z方向加电场
Table 1. The orbital energies EH, EL, EH–1, EH–2, EH–3, EL+1, EL+2, EL+3 and Eg of C18 under different external electric fields. The unit of orbital energy is hartree, the superscripts x, y and z denote thex, y and z direction, respectively.
F/a.u. EH – 3 EH – 2 EH – 1 EH EL EL + 1 EL + 2 EL + 3 Eg/eV 0 –8.5508 –8.5508 –8.4485 –8.4485 –1.7005 –1.7005 –1.6754 1.6754 6.7479 0.005z –8.5544 –8.5544 –8.4486 –8.4486 –1.7141 –1.7141 –1.6666 1.6666 6.7345 0.010z –8.5650 –8.5650 –8.4495 –8.4495 –1.7392 –1.7392 1.6547 1.6547 6.7102 0.015z –8.5819 –8.5819 –8.4518 –8.4518 –1.7700 –1.7700 –1.6461 –1.6461 6.6818 0.020z –8.6044 –8.6044 –8.4562 –8.4562 –1.8056 –1.8056 –1.6415 –1.6415 6.6505 0.005x –8.6369 –8.5205 –8.4629 –8.3831 –1.8002 –1.7217 –1.6612 –1.6317 6.5829 0.010x –8.7048 –8.5881 –8.3700 –8.3153 –1.9358 –1.8075 –1.6986 –1.6119 6.3794 0.015x –8.7516 –8.6519 –8.2846 –8.2518 –2.0999 –1.9295 –1.8027 –1.6493 6.1518 0.050y –8.6367 –8.5204 –8.4630 –8.3831 –1.8005 –1.7222 –1.6609 –1.6315 6.5825 0.010y –8.7046 –8.5879 –8.3700 –8.3153 –1.9359 –1.8075 –1.6988 –1.6120 6.3793 0.015y –8.7527 –8.6529 –8.2847 –8.2516 –2.0992 –1.9293 –1.8012 –1.6482 6.1524 
下载: 导出CSV
表 2 不同电场下的AV1245指数
Table 2. The AV1245 of C18 under different external electric fields.
F/a.u. z x y 0 4.253 4.253 4.253 0.005 4.248 4.256 4.257 0.010 4.232 4.398 4.399 0.015 4.205 4.682 4.706 0.020 4.170
下载: 导出CSV
表 3 不同外电场(y方向)下C18分子部分激发态的激发能
Table 3. Excitation energy of C18 at different electric field in y direction.
F/a.u. E/eV n = 1 2 6 7 14 21 22 23 35 36 0 2.5076 2.6372 3.1285 3.1285 3.8301 5.6519 5.6519 5.7890 6.4794 6.4794 0.005 2.4993 2.6264 3.0978 3.1234 3.8130 5.6339 5.6558 5.7753 6.4573 6.4644 0.010 2.4560 2.5759 2.9933 3.0229 3.7358 5.5725 5.6564 5.7195 6.3833 6.4070 0.015 2.3747 2.4684 2.8621 2.9017 3.6094 5.4638 5.6232 5.6320 6.2782 6.3067
下载: 导出CSV
表 5 不同外电场(y方向)下C18分子部分激发态的振子强度
Table 5. Oscillator strength of C18 at different electric field in y direction.
F/a.u. f n = 1 2 6 7 14 21 22 23 35 36 0 0.0000 0.0000 0.0030 0.0030 0.0000 3.0216 3.0216 0.0000 0.3695 0.3695 0.005 0.0000 0.0003 0.0025 0.0028 0.0132 2.9676 3.0222 0.0000 0.3831 0.3838 0.010 0.0000 0.0011 0.0014 0.0002 0.0463 2.8009 2.9973 0.0000 0.4379 0.4337 0.015 0.0000 0.0000 0.0003 0.0000 0.0842 2.4917 0.0000 2.4509 0.5197 0.5373
下载: 导出CSV
表 4 不同外电场(y方向)下C18分子部分激发态的波长
Table 4. Excitation wavelength of C18 at different electric field in y direction.
F/a.u. $\lambda $/nm n = 1 2 6 7 14 21 22 23 35 36 0 494.43 470.14 396.30 396.30 323.71 219.37 219.37 214.17 191.35 191.35 0.005 496.08 472.07 400.24 396.96 325.17 220.07 219.21 214.68 192.01 191.80 0.010 504.82 481.32 414.20 410.15 331.88 222.49 219.19 216.77 194.23 193.51 0.015 522.11 502.28 433.20 427.29 343.51 226.92 220.49 220.14 197.48 196.59
下载: 导出CSV
-
[1] Kroto H W, Heath J R, O’Brien S C, Curl R F, Smalley R E 1985 Nature 318 162
Google Scholar
[2] Iijima S 1991 Nature 354 56
Google Scholar
[3] Novoselov K S, Geim A K, Morozov S V, Jiang D, Zhang Y, Dubonos S V, Grigorieva I V, Firsov A A 2004 Science 306 666
Google Scholar
[4] Parasuk V, Almlof J, Feyereisen M W 1991 J. Am. Chem. Soc. 113 1049
Google Scholar
[5] Torelli T, Mitas L 2000 Phys. Rev. Lett. 85 1702
Google Scholar
[6] Arulmozhiraja S, Ohno T 2008 J. Chem. Phys. 128 114301
Google Scholar
[7] Fowler P W, Mizoguchi N, Bean D E, Havenith R W 2009 Chemistry 15 6964
Google Scholar
[8] Kaiser K, Scriven L M, Schulz F, Gawel P, Gross L, Anderson H L 2019 Science 365 1299
Google Scholar
[9] Nandi A, Solel E, Kozuch S 2020 Chemistry 26 625
Google Scholar
[10] Pereira Z S, da Silva E Z 2020 J. Phys. Chem. A 124 1152
Google Scholar
[11] Pichierri F 2020 Chem. Phys. Lett. 738 136860
Google Scholar
[12] Shi B, Yuan L, Tang T, Yuan Y, Tang Y 2020 Chem. Phys. Lett. 741 136975
Google Scholar
[13] Stasyuk A J, Stasyuk O A, Solà M, Voityuk A A 2020 Chem. Commun. 56 352
Google Scholar
[14] 史丹丹, 张西沙, 张德清 2018 化学进展 30 658
Google Scholar
Shi D D, Zhang X S, Zhang D Q 2018 Progress in Chemistry 30 658
Google Scholar
[15] Yamaguchi Y, Takubo M, Ogawa K, Nakayama K, Koganezawa T, Katagiri H 2016 J. Am. Chem. Soc. 138 11335
Google Scholar
[16] Sluysmans D, Stoddart J F 2019 Trends in Chemistry 1 185
Google Scholar
[17] Perez E M 2017 Chemistry 23 12681
Google Scholar
[18] Castelvecchi D 2019 Nature 572 426
Google Scholar
[19] 杨涛, 刘代俊, 陈建钧 2016 65 053101
Google Scholar
Yang T, Liu D J, Chen J J 2016 Acta Phys. Sin. 65 053101
Google Scholar
[20] 李世雄, 张正平, 隆正文, 秦水介 2017 66 103102
Google Scholar
Li S X, Zhang Z P, Long Z W, Qin S J 2017 Acta Phys. Sin. 66 103102
Google Scholar
[21] 杜建宾, 冯志芳, 张倩, 韩丽君, 唐延林, 李奇峰 2019 68 173101
Google Scholar
Du J B, Feng Z F, Zhang Q, Han L J, Tang Y L, Li Q F 2019 Acta Phys. Sin. 68 173101
Google Scholar
[22] 李亚莎, 孙林翔, 周筱, 陈凯, 汪辉耀 2020 69 013101
Google Scholar
Li Y S, Sun L X, Zhou X, Chen K, Wang H Y 2020 Acta Phys. Sin. 69 013101
Google Scholar
[23] Shaik S, Mandal D, Ramanan R 2016 Nat. Chem. 8 1091
Google Scholar
[24] English N J, Waldron C J 2015 Phys. Chem. Chem. Phys. 17 12407
Google Scholar
[25] Foroutan-Nejad C, Andrushchenko V, Straka M 2016 Phys. Chem. Chem. Phys. 18 32673
Google Scholar
[26] Weigend F, Ahlrichs R 2005 Phys. Chem. Chem. Phys. 7 3297
Google Scholar
[27] Chai J D, Head-Gordon M 2008 Phys. Chem. Chem. Phys. 10 6615
Google Scholar
[28] Frisch M J et al. 2009 Gaussian 09 (Revision A.02) (Gaussian Inc., Wallingford, CT)
[29] Lu T, Chen F 2012 J. Comput. Chem. 33 580
Google Scholar
[30] Mayer I 1983 Chem. Phys. Lett. 97 270
Google Scholar
[31] Schmider H L, Becke A D 2000 J. Mol. Struct.THEOCHEM 527 51
Google Scholar
[32] Becke A D, Edgecombe K E 1990 J. Chem. Phys. 92 5397
Google Scholar
[33] Matito E 2016 Phys. Chem. Chem. Phys. 18 11839
Google Scholar
下载:
计量
- 文章访问数: 7435
- PDF下载量: 158
- 被引次数: 0
