-
在有对称性保护的条件下, 拓扑能带绝缘体等自由费米子体系的拓扑不变量可以在能带结构计算中得到. 但是, 为了得到强关联拓扑物质态的拓扑不变量, 我们需要全新的理论思路. 最典型的例子就是分数量子霍尔效应: 其低能有效物理一般可以用Chern-Simons拓扑规范场论来计算得到; 霍尔电导的量子化平台蕴含着十分丰富的强关联物理. 本文将讨论存在于玻色和自旋模型中的三大类强关联拓扑物质态: 本征拓扑序、对称保护拓扑态和对称富化拓扑态. 第一类无需考虑对称性, 后两者需要考虑对称性. 理论上, 规范场论是一种非常有效的研究方法. 本文将简要回顾用规范场论来研究强关联拓扑物质态的一些研究进展. 具体内容集中在“投影构造理论”、“低能有效理论”、“拓扑响应理论”三个方面.In the presence of symmetry-protection, topological invariants of topological phases of matter in free fermion systems, e.g., topological band insulators, can be directly computed via the properties of band structure. Nevertheless, it is usually difficult to extract topological invariants in strongly-correlated topological phases of matter in which band structure is not well-defined. One typical example is the fractional quantum Hall effect whose low-energy physics is governed by Chern-Simons topological gauge theory and Hall conductivity plateaus involve extremely fruitful physics of strong correlation. In this article, we focus on intrinsic topological order (iTO), symmetry-protected topological phases (SPT), and symmetry-enriched topological phases (SET) in boson and spin systems. Through gauge field-theoretical approach, we review some research progress on these topological phases of matter from the aspects of projective construction, low-energy effective theory and topological response theory.
-
Keywords:
- strongly-correlated system /
- topological order /
- symmetry-protected topological state /
- topological quantum field theory
[1] Chaikin P M, Lubensky T C 2000 Principles of Condensed Matter Physics (Vol. 1) (Cambridge: Cambridge University Press)
[2] Wen X G 1989 Phys. Rev. B 40 7387
[3] Wen X G 1991 International Journal of Modern Physics B 5 1641
Google Scholar
[4] Zhang S C, Hansson T H, Kivelson S 1989 Phys. Rev. Lett. 62 82
Google Scholar
[5] Lopez A, Fradkin E 1991 Phys. Rev. B 44 5246
Google Scholar
[6] Jain J K 2007 Composite Fermions (Cambridge: Cambridge University Press)
[7] Jain J K 1989 Phys. Rev. B 40 8079
Google Scholar
[8] Jain J K 1989 Phys. Rev. Lett. 63 199
Google Scholar
[9] Wen X G, 1990 International Journal of Modern Physics B 4 239
Google Scholar
[10] Wen X G 2016 Natl. Sci. Rev. 3 68
[11] Wen X G 2004 Quantum Field Theory of Many-body Systems: from the Origin of Sound to An Origin of Light and Electrons. (Oxford: Oxford University Press)
[12] Haldane F 1983 Physics Letters A 93 464
Google Scholar
[13] Chen X, Gu Z C, Wen X G 2010 Phys. Rev. B 82 155138
Google Scholar
[14] Verstraete F, Cirac J I, Latorre J I, Rico E, Wolf M M 2005 Phys. Rev. Lett. 94 140601
Google Scholar
[15] Vidal G 2007 Phys. Rev. Lett. 99 220405
Google Scholar
[16] Affleck I, Kennedy T, Lieb E H, Tasaki H 1987 Phys. Rev. Lett. 59 799
Google Scholar
[17] Gu Z C, Wen X G 2009 Phys. Rev. B 80 155131
Google Scholar
[18] Pollmann F, Berg E, Turner A M, Oshikawa M 2012 Phys. Rev. B 85 075125
Google Scholar
[19] Pollmann F, Turner A M, Berg E, Oshikawa M 2010 Phys. Rev. B 81 064439
Google Scholar
[20] Wang Q R, Ye P 2014 Phys. Rev. B 90 045106
[21] Chen X, Liu Z X, Wen X G 2011 Phys. Rev. B 84 235141
Google Scholar
[22] He Y C, Bhattacharjee S, Moessner R, Pollmann F 2015 Phys. Rev. Lett. 115 116803
Google Scholar
[23] Senthil T, Levin M 2013 Phys. Rev. Lett. 110 046801
Google Scholar
[24] Regnault N, Senthil T 2013 Phys. Rev. B 88 161106
Google Scholar
[25] Levin M, Gu Z C 2012 Phys. Rev. B 86 115109
Google Scholar
[26] Liu Z X, Wen X G 2013 Phys. Rev. Lett. 110 067205
Google Scholar
[27] Wang C, Nahum A, Senthil T 2015 Phys. Rev. B 91 195131
Google Scholar
[28] Vishwanath A, Senthil T 2013 Phys. Rev. X 3 011016
[29] Chen X, Gu Z C, Liu Z X, Wen X G 2013 Phys. Rev. B 87 155114
Google Scholar
[30] Chen X, Gu Z C, Liu Z X, Wen X G 2012 Science 338 1604
Google Scholar
[31] Kapustin A 2014 arXiv: 1404.6659
[32] Wang Z 2010 Topological Quantum Computation (American Mathematical Society)
[33] Nayak C, Simon S H, Stern A, Freedman M, Das Sarma S 2008 Rev. Mod. Phys. 80 1083
Google Scholar
[34] Lan T, Kong L, Wen X G 2018 Phys. Rev. X 8 021074
[35] Lan T, Wen X G 2019 Phys. Rev. X 9 021005
[36] Kitaev A, Laumann C 2009 Les Houches Summer School Exact Methods in Low-dimensional Physics and Quantum Computing 89 101
[37] Levin M A, Wen X G 2005 Phys. Rev. B 71 045110
Google Scholar
[38] Levin M, Wen X G 2005 Rev. Mod. Phys. 77 871
Google Scholar
[39] Dijkgraaf R, Witten E 1990 Commun. Math. Phys. 129 393
Google Scholar
[40] Kapustin A, Seiberg N 2014 J. High Energ. Phys. 2014 1
[41] Savary L, Balents L 2016 Reports on Progress in Physics 80 016502
[42] Zhou Y, Kanoda K, Ng T K 2017 Rev. Mod. Phys. 89 025003
Google Scholar
[43] Barkeshli M, Bonderson P, Cheng M, Wang Z 2019 Phys. Rev. B 100 115147
Google Scholar
[44] Lan T, Kong L, Wen X G 2017 Phys. Rev. B 95 235140
Google Scholar
[45] Kong L, Wen X G 2014 arXiv: 1405.5858
[46] Lan T, Kong L, Wen X G 2017 Communications in Mathematical Physics 351 709
Google Scholar
[47] Etingof P, Nikshych D, Ostrik V 2010 Quantum Topology 1 209
[48] Ning S Q, Liu Z X, Ye P 2016 Phys. Rev. B 94 245120
Google Scholar
[49] Ye P 2018 Phys. Rev. B 97 125127
Google Scholar
[50] Ning S Q, Liu Z X, Ye P 2018 arXiv: 1801.01638
[51] Ye P, Wang J 2013 Phys. Rev. B 88 235109
Google Scholar
[52] Mesaros A, Ran Y 2013 Phys. Rev. B 87 155115
Google Scholar
[53] Xu C 2013 Phys. Rev. B 88 205137
Google Scholar
[54] Chen X, Hermele M 2016 Phys. Rev. B 94 195120
Google Scholar
[55] Chen G 2017 Phys. Rev. B 96 195127
Google Scholar
[56] Chen G 2017 Phys. Rev. B 96 085136
Google Scholar
[57] Cheng M 2015 arXiv: 1511.02563
[58] Zou L, Wang C, Senthil T 2018 Phys. Rev. B 97 195126
Google Scholar
[59] Ning S Q, Zou L, Cheng M 2019 arXiv: 1905.03276
[60] Wang C, Senthil T 2016 Phys. Rev. X 6 011034
[61] Lee P A, Nagaosa N, Wen X G, 2006 Rev. Mod. Phys. 78 17
Google Scholar
[62] Nagaosa N, 1999 Quantum field theory in strongly correlated electronic systems. Springer Science & Business Media
[63] Fradkin E 2013 Field Theories of Condensed Matter Physics (Cambridge: Cambridge University Press)
[64] Baskaran G, Zou Z, Anderson P 1987 Solid State Commun. 63 973
Google Scholar
[65] Baskaran G, Anderson P W 1988 Phys. Rev. B 37 580
Google Scholar
[66] Affleck I, Marston J B 1988 Phys. Rev. B 37 3774
Google Scholar
[67] Kotliar G, Liu J 1988 Phys. Rev. B 38 5142
Google Scholar
[68] Suzumura Y, Hasegawa Y, Fukuyama H 1988 J. Phys. Soc. Jpn. 57 2768
Google Scholar
[69] Affleck I, Zou Z, Hsu T, Anderson P 1988 Phys. Rev. B 38 745
Google Scholar
[70] Dagotto E, Fradkin E, Moreo A 1988 Phys. Rev. B 38 2926
Google Scholar
[71] Wen X G, Wilczek F, Zee A 1989 Phys. Rev. B 39 11413
Google Scholar
[72] Wen X G 1991 Phys. Rev. B 44 2664
[73] Lee P A, Nagaosa N 1992 Phys. Rev. B 46 5621
Google Scholar
[74] Mudry C, Fradkin E 1994 Phys. Rev. B 49 5200
Google Scholar
[75] Wen X G, Lee P A 1996 Phys. Rev. Lett. 76 503
Google Scholar
[76] Weng Z Y, Sheng D N, Chen Y C, Ting C S 1997 Phys. Rev. B 55 3894
Google Scholar
[77] Ye P, Tian C S, Qi X L, Weng Z Y 2011 Phys. Rev. Lett. 106 147002
Google Scholar
[78] Ye P, Tian C S, Qi X L, Weng Z Y 2012 Nucl. Phys. B 854 815
Google Scholar
[79] Wen X G 1991 Mod. Phys. Lett. B 05 39
Google Scholar
[80] Wen X G 1999 Phys. Rev. B 60 8827
Google Scholar
[81] Barkeshli M, Wen X G 2010 Phys. Rev. B 81 155302
Google Scholar
[82] Lu Y M, Lee D H 2014 Phys. Rev. B 89 184417
Google Scholar
[83] Ye P, Wen X G 2013 Phys. Rev. B 87 195128
Google Scholar
[84] Ye P, Wen X G 2014 Phys. Rev. B 89 045127
Google Scholar
[85] Ye P, Hughes T L, Maciejko J, Fradkin E 2016 Phys. Rev. B 94 115104
Google Scholar
[86] Liu Z X, Mei J W, Ye P, Wen X G 2014 Phys. Rev. B 90 235146
Google Scholar
[87] Deser S, Jackiw R, Templeton S 1982 Ann. Phys. 140 372
Google Scholar
[88] Laughlin R B 1981 Phys. Rev. B 23 5632
Google Scholar
[89] Wen X G, Zee A 1992 Phys. Rev. B 46 2290
Google Scholar
[90] Polyakov A M 1975 Phys. Lett. B 59 82
Google Scholar
[91] Polyakov A M 1977 Nucl. Phys. B 120 429
Google Scholar
[92] Polyakov A M 1978 Phys. Lett. B 72 477
Google Scholar
[93] Wen X G 2014 Phys. Rev. B 89 035147
Google Scholar
[94] Hohenadler M, Meng Z Y, Lang T C, Wessel S, Muramatsu A, Assaad F F 2012 Phys. Rev. B 85 115132
Google Scholar
[95] Griset C, Xu C 2012 Phys. Rev. B 85 045123
Google Scholar
[96] Lee D H 2011 Phys. Rev. Lett. 107 166806
Google Scholar
[97] Kitaev A, 2006 Ann. Phys. 321 2
Google Scholar
[98] Bombin H 2010 Phys. Rev. Lett. 105 030403
Google Scholar
[99] You Y Z, Wen X G 2012 Phys. Rev. B 86 161107
Google Scholar
[100] Teo J C, Hughes T L, Fradkin E 2015 Ann. Phys. 360 349
Google Scholar
[101] Khan M N, Teo J C Y, Hughes T L 2014 Phys. Rev. B 90 235149
Google Scholar
[102] Mesaros A, Kim Y B, Ran Y 2013 Phys. Rev. B 88 035141
Google Scholar
[103] Barkeshli M, Wen X G 2010 Phys. Rev. B 81 045323
Google Scholar
[104] Teo J C Y, Roy A, Chen X 2014 Phys. Rev. B 90 155111
Google Scholar
[105] Barkeshli M, Qi X L 2012 Phys. Rev. X 2 031013
[106] Barkeshli M, Jian C M, Qi X L 2013 Phys. Rev. B 87 045130
Google Scholar
[107] Barkeshli M, Qi X L 2014 Phys. Rev. X 4 041035
[108] Barkeshli M, Jiang H C, Thomale R, Qi X L 2015 Phys. Rev. Lett. 114 026401
Google Scholar
[109] 't Hooft G 1981 Nucl. Phys. B 190 455
[110] Mandelstam S 1980 Phys. Rep. 67 109
Google Scholar
[111] Peskin M E 1978 Ann. Phys. 113 122
Google Scholar
[112] 't Hooft G 1978 Nucl. Phys. B 138 1
[113] 't Hooft G 1979 Nucl. Phys. B 153 141
[114] Susskind L 1979 Phys. Rev. D 20 2610
[115] Fradkin E Susskind L 1978 Phys. Rev. D 17 2637
Google Scholar
[116] van Baal P 2002 Confinement, Duality, and Nonperturbative Aspects of QCD. (Kluwer: Kluwer Academic Publishers)
[117] Seiberg N, Witten E 1994 Nucl. Phys. B 431 484
Google Scholar
[118] Witten E 1979 Phys. Lett. B 86 283
Google Scholar
[119] Qi X L, Hughes T L, Zhang S C 2008 Phys. Rev. B 78 195424
Google Scholar
[120] Rosenberg G, Franz M 2010 Phys. Rev. B 82 035105
Google Scholar
[121] Wen X G 2003 Phys. Rev. Lett. 90 016803
Google Scholar
[122] Horowitz G T 1989 Commun. Math. Phys. 125 417
Google Scholar
[123] Baez J C, Huerta J 2011 General Relativity and Gravitation 43 2335
Google Scholar
[124] Blau M, Thompson G 1991 Ann. Phys. 205 130
Google Scholar
[125] Bergeron M, Semenoff G W, Szabo R J 1995 Nucl. Phys. B 437 695
Google Scholar
[126] Szabo R J 1998 Nucl. Phys. B 531 525
Google Scholar
[127] Lu Y M, Vishwanath A 2012 Phys. Rev. B 86 125119
Google Scholar
[128] Gu Z C, Wang J C, Wen X G 2016 Phys. Rev. B 93 115136
Google Scholar
[129] Ye P, Gu Z C 2016 Phys. Rev. B 93 205157
Google Scholar
[130] Ye P, Gu Z C 2015 Phys. Rev. X 5 021029
[131] v Bodecker H, Hornig G, 2004 Phys. Rev. Lett. 92 030406
Google Scholar
[132] Wen X, Wen X G 2014 arXiv: 1908.10381
[133] Wilczek F 1990 Fractional Statistics and Anyon Superconductivity (Vol. 5) (Singapore: World Scientific)
[134] Wu Y S 1984 Phys. Rev. Lett. 52 2103
Google Scholar
[135] Bonderson P, Shtengel K, Slingerland J 2008 Annals of Physics 323 2709
Google Scholar
[136] Witten E 1989 Commun. Math. Phys. 121 351
Google Scholar
[137] Leinaas J M, Myrheim J, 1977 Il Nuovo Cimento B 37 1
[138] Wen X, He H, Tiwari A, Zheng Y, Ye P 2018 Phys. Rev. B 97 085147
Google Scholar
[139] Hansson T H, Oganesyan V, Sondhi S L 2004 Annals of Physics 313 497
Google Scholar
[140] Wang C, Levin M 2014 Phys. Rev. Lett. 113 080403
Google Scholar
[141] Wang J C, Wen X G 2015 Phys. Rev. B 91 035134
Google Scholar
[142] Tiwari A, Chen X, Ryu S 2017 Phys. Rev. B 95 245124
Google Scholar
[143] Putrov P, Wang J, Yau S T 2017 Annals of Physics 384 254
Google Scholar
[144] Jian C M, Qi X L 2014 Phys. Rev. X 4 041043
[145] Wang C, Lin C H, Levin M 2016 Phys. Rev. X 6 021015
[146] Wan Y, Wang J C, He H 2015 Phys. Rev. B 92 045101
Google Scholar
[147] Kapustin A, Thorngren R 2014 arXiv: 1404.3230
[148] Wang J C, Gu Z C, Wen X G 2015 Phys. Rev. Lett. 114 031601
Google Scholar
[149] Chen X, Tiwari A, Ryu S 2016 Phys. Rev. B 94 045113
Google Scholar
[150] Wang J, Wen X G, Yau S T 2016 arXiv: 1602.05951
[151] Jiang S, Mesaros A, Ran Y 2014 Phys. Rev. X 4 031048
[152] Ye P, 2020 unpublished
[153] von Keyserlingk C W, Burnell F J, Simon S H 2013 Phys. Rev. B 87 045107
Google Scholar
[154] Walker K, Wang Z 2012 Frontiers of Physics 7 150
Google Scholar
[155] Wang Q R, Cheng M, Wang C, Gu Z C 2019 Phys. Rev. B 99 235137
Google Scholar
[156] Wang Z, Chen X 2017 Phys. Rev. B 95 115142
Google Scholar
[157] Chan A P O, Ye P, Ryu S 2018 Phys. Rev. Lett. 121 061601
Google Scholar
[158] Milnor J 1954 Ann. Math. 59 177
Google Scholar
[159] Hatcher A 2002 Algebraic Topology. (Cambridge: Cambridge University Press)
[160] Han B, Wang H, Ye P 2019 Phys. Rev. B 99 205120
Google Scholar
[161] Hasan M Z, Kane C L 2010 Rev. Mod. Phys. 82 3045
Google Scholar
[162] Qi X L, Zhang S C 2011 Rev. Mod. Phys. 83 1057
Google Scholar
[163] Lapa M F, Jian C M, Ye P, Hughes T L 2017 Phys. Rev. B 95 035149
Google Scholar
[164] Witten E 2016 Rev. Mod. Phys. 88 035001
Google Scholar
[165] Witten E 1995 Selecta Mathematica 1 383
Google Scholar
[166] Vafa C, Witten E 1994 Nuclear Physics B 431 3
Google Scholar
[167] Montonen C, Olive D 1977 Physics Letters B 72 117
Google Scholar
[168] Son D T 2015 Phys. Rev. X 5 031027
[169] Metlitski M A, Vishwanath A 2016 Phys. Rev. B 93 245151
Google Scholar
[170] Wang C, Senthil T 2016 Phys. Rev. B 93 085110
Google Scholar
[171] Mross D F, Alicea J, Motrunich O I 2016 Phys. Rev. Lett. 117 016802
Google Scholar
[172] Kachru S, Mulligan M, Torroba G, Wang H 2015 Phys. Rev. B 92 235105
Google Scholar
[173] Karch A, Tong D 2016 Phys. Rev. X 6 031043
[174] Seiberg N, Senthil T, Wang C, Witten E 2016 Annals of Physics 374 395
Google Scholar
[175] Fradkin E, Kivelson S 1996 Nuclear Physics B 474 543
Google Scholar
[176] Witten E 2016 arXiv: hep-th/0307041
[177] Gaiotto D, Witten E 2009 Adv. Theor. Math. Phys. 13 721
Google Scholar
[178] Chen X, Fidkowski L, Vishwanath A 2014 Phys. Rev. B 89 165132
Google Scholar
[179] Wang C, Potter A C, Senthil T 2014 Science 343 629
Google Scholar
[180] Bonderson P, Nayak C, Qi X L 2013 J. Stat. Mech. 201 3
[181] Metlitski M A, Kane C L, Fisher M P A 2015 Phys. Rev. B 92 125111
Google Scholar
[182] Maciejko J, Qi X L, Karch A, Zhang S C 2010 Phys. Rev. Lett. 105 246809
Google Scholar
[183] Maciejko J, Qi X L, Karch A, Zhang S C 2012 Phys. Rev. B 86 235128
Google Scholar
[184] Maciejko J, Fiete G A 2015 Nat. Phys. 11 385
Google Scholar
[185] Swingle B, Barkeshli M, McGreevy J, Senthil T 2011 Phys. Rev. B 83 195139
Google Scholar
[186] Sahoo S, Sirota A, Cho G Y, Teo J C Y 2017 Phys. Rev. B 96 161108
Google Scholar
[187] Levin M, Burnell F J, Koch-Janusz M, Stern A 2011 Phys. Rev. B 84 235145
Google Scholar
[188] Swingle B 2012 Phys. Rev. B 86 245111
Google Scholar
[189] Ye P, Cheng M, Fradkin E 2017 Phys. Rev. B 96 085125
Google Scholar
[190] Hirzebruch F, Berger T, Jung R, Landweber P S 1992 Manifolds and Modular Forms (Vol. 20) (Springer, 1992)
[191] Chan A P O, Kvorning T, Ryu S, Fradkin E 2016 Phys. Rev. B 93 155122
Google Scholar
[192] Liu C X, Ye P, Qi X L 2013 Phys. Rev. B 87 235306
Google Scholar
[193] Liu C X, Ye P, Qi X L 2015 Phys. Rev. B 92 119904
Google Scholar
[194] Liu C X, Ye P, Qi X L 2017 Phys. Rev. B 96 247302
Google Scholar
[195] Ye P, Zhang L, Weng Z Y 2012 Phys. Rev. B 85 205142
Google Scholar
[196] Ma Y, Ye P, Weng Z Y 2014 New Journal of Physics 16 083039
Google Scholar
[197] Xu C, Sachdev S 2009 Phys. Rev. B 79 064405
Google Scholar
[198] Cheng M, Gu Z C 2014 Phys. Rev. Lett. 112 141602
Google Scholar
[199] Hung L Y, Wen X G 2013 Phys. Rev. B 87 165107
Google Scholar
[200] Goldhaber A S, MacKenzie R, Wilczek F 1989 Mod. Phys. Lett. A 4 21
Google Scholar
[201] Fu L 2011 Phys. Rev. Lett. 106 106802
Google Scholar
[202] Chiu C K, Teo J C Y, Schnyder A P, Ryu S 2016 Rev. Mod. Phys. 88 035005
Google Scholar
[203] Song H, Huang S J, Fu L, Hermele M 2017 Phys. Rev. X 7 011020
[204] Huang S J, Song H, Huang Y P, Hermele M 2017 Phys. Rev. B 96 205106
Google Scholar
[205] Huang S J, Hermele M 2018 Phys. Rev. B 97 075145
Google Scholar
[206] Song Z, Huang S J, Qi Y, Fang C, Hermele M 2019 Science Advances 5 eaax2007
[207] Thorngren R, Else D V 2018 Phys. Rev. X 8 011040
[208] Jiang S, Ran Y 2017 Phys. Rev. B 95 125107
Google Scholar
[209] Wen X G, Zee A 1992 Phys. Rev. Lett. 69 953
Google Scholar
[210] Gaiotto D, Kapustin A, Seiberg N 2015 Journal of High Energy Physics 2015 172
[211] Katanaev M, Volovich I 1992 Annals of Physics 216 1
Google Scholar
[212] Landau L D, Lifshitz E M 1986 Theory of Elasticity of Course of Theoretical Physics (Vol. 7) (New York: Elsevier Butterworth-Heinemann)
[213] Hehl F W, Obukhov Y N 2007 Annales Fond. Broglie 0711 1535
[214] Nakahara M 2003 Geometry, Topology and Physics (2nd Ed.) (Graduate Student Series in Physics) (Taylor & Francis, 2003)
[215] Xu C, Senthil T 2013 Phys. Rev. B 87 174412
Google Scholar
[216] Bi Z, Rasmussen A, Slagle K, Xu C 2015 Phys. Rev. B 91 134404
Google Scholar
[217] You Y Z, Xu C 2014 Phys. Rev. B 90 245120
Google Scholar
[218] Bi Z, You Y Z, Xu C 2014 Phys. Rev. B 90 081110
Google Scholar
[219] Bi Z, Rasmussen A, Xu C 2014 Phys. Rev. B 89 184424
Google Scholar
[220] Xu C 2013 Phys. Rev. B 87 144421
Google Scholar
[221] Wang Q R, Gu Z C 2018 Phys. Rev. X 8 011055
[222] Wen X G 2019 Phys. Rev. B 99 205139
Google Scholar
-
图 1 蒙特卡洛验证投影后得到的SPT波函数的拓扑纠缠熵为零, 摘自文献[86]
Fig. 1. Monte Carlo verification of vanishing topological entanglement entropy of the SPT wave function obtained from the projective construction
图 2 一种将电子分成三个部分子的投影构造(即
$ n=1 $ ), 摘自文献[85]Fig. 2. Parton decomposition of electron operators
图 3 “Twist缺陷和拓扑激发”的融合规则示意图 (a) 二维iTO的任意子和点缺陷的融合. (b) 三维iTO的点激发与线缺陷的融合. (c) 三维iTO的圈激发与线缺陷的融合. 摘自[85]
Fig. 3. Diagrammatic illustration of fusion rules among twist defects and topological excitations. (a) Fusions between an anyon (quasiparticle) and a point-defect in a two-dimensional iTO. (b) Fusions between a particle excitation and a line defect in a three-dimensional iTO; (c) Fusions between a loop excitation and a line defect in a three-dimensional iTO.[85]
图 5 三维iTO中的点激发和圈激发示意图[138]
Fig. 5. Illustration of point-like excitations and loop excitations in three-dimensional iTO
图 6 (a) 点粒子-圈之间的编织: 点粒子激发
$ e_i $ (携带单位$ \mathbb{Z}_{N_i} $ 规范荷) 绕着圈激发$ m_i $ (携带单位$ \mathbb{Z}_{N_i} $ 规范磁通)转一圈.$ e_i $ 的轨迹$ \gamma_{e_i} $ 与静止的圈$ m_i $ 形成一个Hopf环链. (b) 博罗梅安编织(点粒子-圈-圈编织): 点粒子$ e_k $ 绕着两个互相未链接的圈激发$ m_i, m_j $ 转一圈.$ e_k $ 的轨迹$ \gamma_{e_k} $ 与$ m_i $ ,$ m_j $ 一起形成博罗梅安环(Borromean Rings, 或更一般的Brunnian link)Fig. 6. (a) Particle-loop braiding: a particle
$ e_i $ travels around a loop$ m_i $ such that the braiding trajectory$ \gamma_{e_i} $ and$ m_i $ form a Hopf link. (b) Borromean-Rings braiding: a particle$ e_k $ moves around two unlinked loops$ m_i, m_j $ such that$ m_i $ ,$ m_j $ and the trajectory$ \gamma_{e_k} $ form the Borromean rings (or generally the Brunnian link)
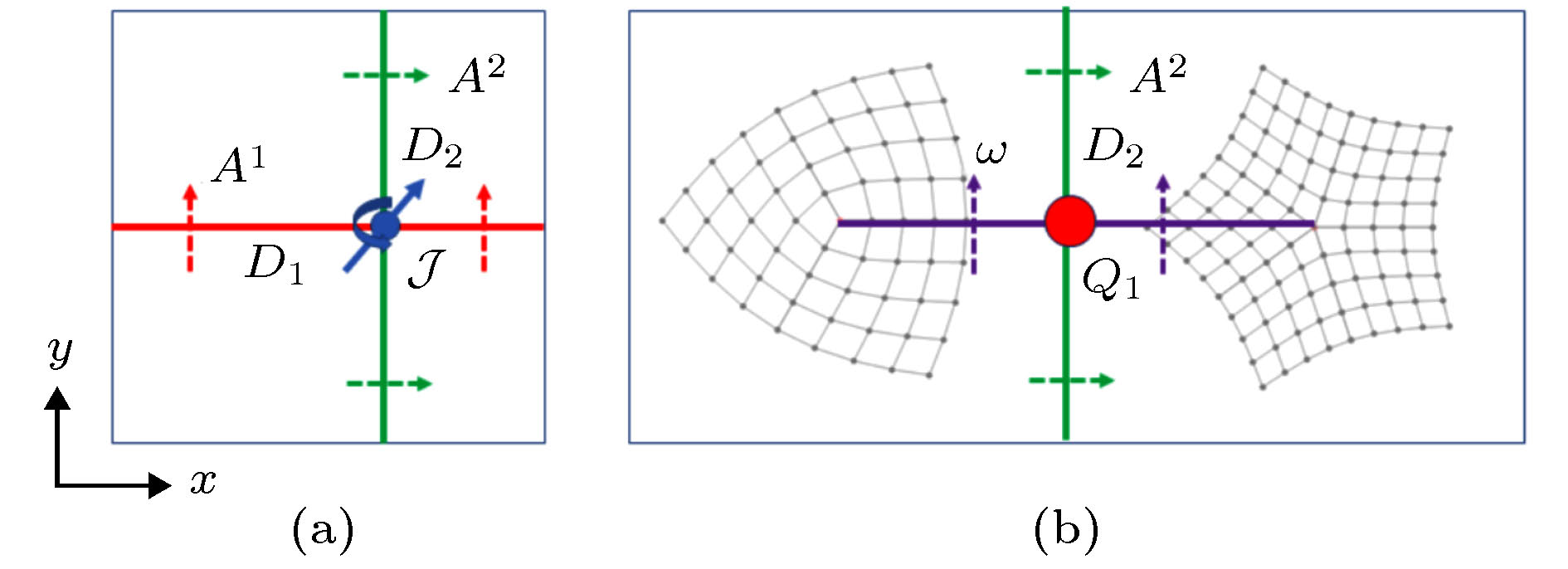
图 8 (a) 公式(42)代表的拓扑响应现象的示意图.
$ \mathbb{Z}_{N_1} $ 的对称性畴壁$ D_1 $ 和$ \mathbb{Z}_{N_2} $ 的对称性畴壁$ D_2 $ 的交点携带分数角动量$ \mathcal{J} $ .$ A^1 $ 和$ A^2 $ 分别是垂直于畴壁$ D_1 $ 和$ D_2 $ 的规范联络. (b) 公式(44)代表的拓扑响应现象的示意图. 旋错线与$ \mathbb{Z}_{N_2} $ 对称性畴壁$ D_2 $ 的交点携带$ A^1 $ 规范场的分数规范荷$ \mathcal{Q}_1 $ .$ \omega $ 和$ A^2 $ 分别是垂直于旋错线和畴壁的规范联络[160]Fig. 8. (a). Topological response for Eq. (42). The intersection of
$ \mathbb{Z}_{N_1} $ and$ \mathbb{Z}_{N_2} $ symmetry domain walls$ D_1 $ and$ D_2 $ carries the angular momentum$ \mathcal{J} $ .$ A^1 $ and$ A^2 $ are the gauge connections normal to the domain walls. (b). Topological response of Eq. (44). The intersection of disclination line and$ \mathbb{Z}_{N_2} $ symmetry domain walls$ D_2 $ carries the$ A^1 $ charge$ \mathcal{Q}_1 $ .$ \omega $ and$ A^2 $ are the gauge connections normal to the disclination line and domain wall, respectively
图 9 两个三维SPT拓扑响应现象示意图. 摘自文献[160]
Fig. 9. Illustration of two examples of SPT topological response phenomena in three dimensions
表 1 二维投影构造中的部分子的拟设.
$ A1, A2,\cdots, A4 $ 代表四种拟设. 每条完全填充的能带由箭头和正负号标记. 箭头表示自旋方向, 正负号代表陈数为1或–1. A1一共有8条填满的陈-能带. A2和A3都有4条填满的陈-能带. A4只用到了f1, 一共有两条陈-能带被填满. 括号里成对的数字表示单个元胞里的费米子f1或f2的填充数: (自旋向上的费米子数目, 自旋向下的费米子数目).Table 1. Parton ansatzes in the two-dimensional projective construction.
$ A1,A2, \cdots, A4 $ stand for four different ansatzes respectively. Each fully occupied band is labeled by a pair of arrow and plus/minus sign. The arrow represents the spin eigenvalue of$ S^z $ , and$ \pm $ represents Chern number$ \pm1 $ . In A1, there are 8 fully occupied Chern bands; There are 4 fully occupied Chern bands in each of A2 and A3. In A4, flavor index is not involved, so only one flavor, say,$ f_1 $ is taken into account. And there are two filled Chern bands. A pair of integers denote the filling number of either$ f_1 $ and$ f_2 $ in each unit cell: (fermion number with up spin, fermion number with down spin).拟设 完全被$f_1$填充的陈-能带 完全被$f_2$填充的陈-能带 自旋矢量$q^T_s$ 电荷矢量$q^T_c$ $A1$ $\uparrow+, \downarrow+, \uparrow-, \downarrow-$ $(2, 2)$ $\uparrow+, \downarrow+, \uparrow-, \downarrow-$ $(2, 2)$ $\left(\dfrac{1}{2}~~-\dfrac{1}{2}~~\dfrac{1}{2}~~-\dfrac{1}{2}~~\dfrac{1}{2}~~-\dfrac{1}{2}~~\dfrac{1}{2}~~-\dfrac{1}{2}\right)$ $(1~~1~~1~~1~~1~~1~~1~~1)$ $A2$ $\uparrow+, \downarrow-$ $(1, 1)$ $\uparrow+, \downarrow-$ $(1, 1)$ $\left( {1}/{2}~~- {1}/{2}~~ {1}/{2}~~ -{1}/{2}\right)$ $(1~~1~~1~~1)$ $A3$ $\uparrow+, \downarrow-$ $(1, 1)$ $\downarrow+, \uparrow-$ $(1, 1)$ $\left(1/{2}~~- {1}/{2}~~- {1}/{2}~~ {1}/{2}\right)$ $(1~~1~~1~~1)$ $A4$ $\uparrow+, \downarrow-$ $(1, 1)$ 无 $\left( {1}/{2}~~- {1}/{2}\right)$ $(1~~1)$ 
下载: 导出CSV
表 2 在大U极限下, 实空间每个格点上的不消耗U能量的占据状态形成了物理希尔伯特空间. 我们需要对费米子的总的填充数做限制. 限制之后, 所有格点都能够同时处于物理希尔伯特空间.
Table 2. At large U limit, the physical Hilbert space is formed by those occupancy bases without energy cost. We should restrict the total particle number of each flavor properly such that Hilbert space of every site is always in the physical Hilbert space
U 任意一个格点上的物理希尔伯特空间基矢$ [f_1]n_{i, 1 \uparrow}, n_{i, 1 \downarrow}, n_{i, 2 \uparrow}, n_{i, 2 \downarrow}[f_2] $ 费米子填充总数要求 $U_1$ $(0, 0, 0, 0)$, $(0, 1, 0, 1)$, $(0, 1, 1, 0)$, $(1, 0, 0, 1)$, $(1, 0, 1, 0)$, $(1, 1, 1, 1)\, $ $N^{f1}=N^{f2} $ $U_2$ $(0, 0, 0, 0)$, $(0, 1, 1, 0)$, $(1, 0, 0, 1)$, $(1, 1, 1, 1)\, $ $N^{f1}_{\uparrow} = N^{f2}_{\downarrow}, $ $N^{f1}_{\downarrow}=N^{f2}_{\uparrow}$ $U_3$ $(0, 0, 0, 0)$, $(0, 1, 0, 1)$, $(1, 0, 1, 0)$, $(1, 1, 1, 1)\, $ $N^{f1}_{ \uparrow}=N^{f2}_{ \uparrow}, $ $N^{f1}_{ \downarrow}=N^{f2}_{\downarrow}$ $U_4$ $(0, 0, 1, 1)$, $(0, 1, 0, 1)$, $(1, 0, 1, 0)$, $(1, 1, 0, 0)$ $N^{f1}_{\uparrow}+N^{f2}_{\downarrow}=N_{\rm latt}$, $N^{f2}_{\uparrow}+ N^{f1}_{\downarrow}=N_{\rm att}$ $U_5$ $(1, 0, 0, 0)$, $(0, 1, 0, 0)$, $(0, 0, 1, 0)$, $(0, 0, 0, 1)$ $ N^{f1} + N^{f2}=N_{\rm latt}$ $U_6$ $(1, 0)$, $(0, 1)$ $ N^{f1} =N_{\rm latt}$ $U_7$ $(0, 0)$, $(1, 1)$ $N^{f1}_{\uparrow} = N^{f1}_{\downarrow }$
下载: 导出CSV
表 3 受到幺正阿贝尔群保护的“不可约”的三维SPT态的低能有效理论及其分类.
$ a^I $ 和$ b^I $ 分别是1-形式 和2-形式$ {U(1)} $ 规范场. 系数p、$ p_1 $ 、$ p_2 $ 的取值满足一定的量子化条件和周期性. 系数的周期给出分类的结果.“($ {\mathbb{Z}}_{N_{12}} ) \cdots $ ”表示相应的分类. 其中, 符号$ N_{IJ\cdots} $ 表示$ N_I, N_J, \cdots $ 等整数的最大公约数. 受到$ {\mathbb{Z}}_N $ 或$ {U(1)}^k $ 或$ {\mathbb{Z}}_N\times {U(1)}^k $ 保护的SPT态都是平凡的, 因而没有列入表中.“不可约”是指对称群的所有子群都起着保护SPT的作用. 其他SPT都可以通过表格里的结果构造出来. 具体摘自[129].Table 3. A brief summary of irreducible 3D SPT phases with unitary Abelian symmetry.
$ a^I $ and$ b^I $ are 1-form and 2-form$ {U(1)} $ gauge fields, respectively. “($ {\mathbb{Z}}_{N_{12}} $ )$ \cdots $ ”denote the corresponding classifications, where$ N_{IJ\cdots} $ are greatest common divisors of$ N_I, N_J, \cdots $ . SPT phases with either$ {\mathbb{Z}}_N $ or${U(1)}^k $ or$ {\mathbb{Z}}_N\times {U(1)}^k $ are trivial and not included below. By “irreducible”, we means that all subgroups of symmetry group play nontrivial roles in protecting the nontrivial SPT phases. All other SPT's with unitary Abelian group symmetries can be obtained directly by using this table[129].对称群G 拓扑规范场论与分类 $\mathbb{Z}_{N_1}\times\mathbb{Z}_{N_2}$ $\dfrac{1}{2{\text{π}}}\displaystyle\int \sum^2_Ib^I\wedge \, {\rm d}a^I+ p_1\displaystyle\int a^1\wedge a^2\wedge \, {\rm d}a^2~~(\mathbb{Z}_{N_{12}} )$ ; $\dfrac{1}{2{\text{π}}}\displaystyle\int \sum^2_Ib^I\wedge \, {\rm d}a^I+ p_2\displaystyle\int a^2\wedge a^1\wedge \, {\rm d}a^1 ~(\mathbb{Z}_{N_{12}}) $ $\mathbb{Z}_{N_1}\times\mathbb{Z}_{N_2}\times\mathbb{Z}_{N_3} $ $\dfrac{1}{2{\text{π}}}\displaystyle\int \sum^3_Ib^I\wedge \, {\rm d}a^I+ p_1 \displaystyle\int a^1\wedge a^2\wedge \, {\rm d}a^3~~(\mathbb{Z}_{N_{123}})$ ; $\dfrac{1}{2{\text{π}}}\displaystyle\int \sum^3_Ib^I\wedge \, {\rm d}a^I+ p_2 \displaystyle\int a^2\wedge a^3\wedge \, {\rm d}a^1~~(\mathbb{Z}_{N_{123}}) $ $\prod^4_I\mathbb{Z}_{N_I} $ $\dfrac{1}{2{\text{π}}}\displaystyle\int \sum^4_Ib^I\wedge \, {\rm d}a^I+p \displaystyle\int a^1\wedge a^2\wedge a^3\wedge a^4~~ ( \mathbb{Z}_{N_{1234}} )$ $\mathbb{Z}_{N_1}\times\mathbb{Z}_{N_2}\times {U}(1)$ $\dfrac{1}{2{\text{π}}}\displaystyle\int \sum^3_Ib^I\wedge \, {\rm d}a^I+p\displaystyle\int a^1\wedge a^2\wedge \, {\rm d}a^3~~ (\mathbb{Z}_{N_{12}})$
下载: 导出CSV
表 4 部分三维SET的分类, 摘自[50].
Table 4. Classification of SET examples.
规范群$G_g$ twisted拓扑项 对称群$G_s$ SET分类 ${\mathbb{Z}}_2$ – ${\mathbb{Z}}_{2 n + 1}$ ${{\mathbb{Z}}_1}$ ${\mathbb{Z}}_2$ – ${\mathbb{Z}}_{2 n}$ $ {({\mathbb{Z}}_2)^2}\oplus {\mathbb{Z}}_1$ ${\mathbb{Z}}_3$ – ${\mathbb{Z}}_{3 n}$ $({\mathbb{Z}}_3)^2\oplus {\mathbb{Z}}_1 \oplus {\mathbb{Z}}_1$ ${\mathbb{Z}}_3$ – ${\mathbb{Z}}_{3 n + 1}$ ${\mathbb{Z}}_1$ ${\mathbb{Z}}_4$ – ${\mathbb{Z}}_{2 n + 1}$ ${\mathbb{Z}}_1$ ${\mathbb{Z}}_4$ – ${\mathbb{Z}}_{4 n+2}$ $ ({\mathbb{Z}}_2)^2\oplus {\mathbb{Z}}_1$ ${\mathbb{Z}}_4$ – ${\mathbb{Z}}_{4 n}$ $ ({\mathbb{Z}}_4)^2\oplus ({\mathbb{Z}}_2)^2\oplus {\mathbb{Z}}_1 \oplus {\mathbb{Z}}_1 $ ${\mathbb{Z}}_2\times {\mathbb{Z}}_2$ (0, 0) ${\mathbb{Z}}_{2 n}$ $({\mathbb{Z}}_2)^6\oplus ({\mathbb{Z}}_2)^2 \oplus ({\mathbb{Z}}_2)^2 \oplus ({\mathbb{Z}}_2)^2$ ${\mathbb{Z}}_2\times {\mathbb{Z}}_2$ (0, 0) ${\mathbb{Z}}_{2 n + 1}$ ${\mathbb{Z}}_1$ ${\mathbb{Z}}_2\times {\mathbb{Z}}_2$ (2, 0) ${\mathbb{Z}}_{2 n}$ $({\mathbb{Z}}_2)^6$ ${\mathbb{Z}}_2\times {\mathbb{Z}}_2$ (2, 0) ${\mathbb{Z}}_{2 n + 1}$ ${\mathbb{Z}}_1$ ${\mathbb{Z}}_2\times {\mathbb{Z}}_2$ (2, 2) ${\mathbb{Z}}_{2 n + 1}$ ${\mathbb{Z}}_1$ ${\mathbb{Z}}_2\times {\mathbb{Z}}_4$ (0, 0) ${\mathbb{Z}}_{2 n + 1}$ ${\mathbb{Z}}_1$ ${\mathbb{Z}}_2\times {\mathbb{Z}}_4$ (4, 0) ${\mathbb{Z}}_{2 n + 1}$ ${\mathbb{Z}}_1$ ${\mathbb{Z}}_2\times {\mathbb{Z}}_4$ (4, 4) ${\mathbb{Z}}_{2 n + 1}$ ${\mathbb{Z}}_1$ ${\mathbb{Z}}_2\times {\mathbb{Z}}_4$ (0, 0) ${\mathbb{Z}}_{4 n}$ $({\mathbb{Z}}_2)^4\times ({\mathbb{Z}}_4)^2\oplus 2({\mathbb{Z}}_4)^2\oplus4({\mathbb{Z}}_2)^2 \oplus ({\mathbb{Z}}_2)^6$ ${\mathbb{Z}}_2\times {\mathbb{Z}}_4$ (4, 0) ${\mathbb{Z}}_{4 n}$ $({\mathbb{Z}}_2)^4\times ({\mathbb{Z}}_4)^2$ ${\mathbb{Z}}_2\times {\mathbb{Z}}_4$ (4, 4) ${\mathbb{Z}}_{4 n}$ $({\mathbb{Z}}_2)^4\times ({\mathbb{Z}}_4)^2$ ${\mathbb{Z}}_2$ – ${\mathbb{Z}}_{2 m + 1} \times {\mathbb{Z}}_{2 n + 1}$ $({\mathbb{Z}}_{2\gcd(2 m + 1, 2 n + 1)})^2$ ${\mathbb{Z}}_2$ – ${\mathbb{Z}}_{2 m + 1} \times {\mathbb{Z}}_{2 n}$ $({\mathbb{Z}}_{\gcd(2 m + 1, 2 n)})^2 \oplus ({\mathbb{Z}}_{2\gcd(2 m + 1, 2 n)})^2 $ ${\mathbb{Z}}_2$ – ${\mathbb{Z}}_{2 m} \times {\mathbb{Z}}_{2 n}$ $({\mathbb{Z}}_2)^6\times({\mathbb{Z}}_{2\gcd(m, n)})^2\oplus ({\mathbb{Z}}_{2\gcd(2 m, n)})^2 \oplus ({\mathbb{Z}}_{2\gcd(m, 2 n)})^2 \oplus ({\mathbb{Z}}_{2\gcd(m, n)})^2$ ${\mathbb{Z}}_4$ – ${\mathbb{Z}}_{2 n + 1} \times {\mathbb{Z}}_{2 n + 1}$ $16({\mathbb{Z}}_{2 n + 1})^2$ ${\mathbb{Z}}_4$ – ${\mathbb{Z}}_{2(2 n + 1)} \times {\mathbb{Z}}_{2(2 n + 1)}$ $4({\mathbb{Z}}_2)^6 \times ({\mathbb{Z}}_{2(2 n + 1)})^2\oplus 12({\mathbb{Z}}_{2(2 n + 1)})^2$ ${\mathbb{Z}}_4$ – ${\mathbb{Z}}_{4 n} \times {\mathbb{Z}}_{4 n}$ $({\mathbb{Z}}_4)^6 \times ({\mathbb{Z}}_{4 n})^2\oplus 12({\mathbb{Z}}_{4 n})^2 \oplus 3[ ({\mathbb{Z}}_{4 n})^2\times ({\mathbb{Z}}_2)^6]$ ${\mathbb{Z}}_2\times {\mathbb{Z}}_2$ (0, 0) ${\mathbb{Z}}_{2}\times {\mathbb{Z}}_2$ $({\mathbb{Z}}_2)^{18}\oplus 6({\mathbb{Z}}_2)^8 \oplus 3({\mathbb{Z}}_2)^6 \oplus 6({\mathbb{Z}}_2)^4$ ${\mathbb{Z}}_2\times {\mathbb{Z}}_2$ (2, 0) ${\mathbb{Z}}_{2}\times {\mathbb{Z}}_2$ $({\mathbb{Z}}_2)^{18}$ ${\mathbb{Z}}_2\times {\mathbb{Z}}_2$ (2, 2) ${\mathbb{Z}}_{2}\times {\mathbb{Z}}_2$ $({\mathbb{Z}}_2)^{18}$
下载: 导出CSV
表 5 部分含有反幺正对称群(时间反演)的SET的体内理论与边界理论, 摘自[51].
Table 5. Bulk and boundary theories of SET with anti-unitary symmetry (e.g., time-reversal symmetry).
投影对称群(PSG) 规范群$G_g$ 对称群$G_s$ 三维体内($\varSigma^3$)
的规范理论表面($\partial\varSigma^3$)的反常
玻色理论二维平面($\varSigma^2$)的正常Chern-Simons理论的$K_G$-矩阵 ${\mathbb{Z}}_N \rtimes{\mathbb{Z}}^T_2$ ${\mathbb{Z}}_N$ ${\mathbb{Z}}^T_2$ $\dfrac{N}{4{\text{π}}} \epsilon^{\mu\nu\lambda\rho} B^c_{\mu\nu}\partial_\lambda A_\rho^c+$
$\dfrac{\theta_c}{8{\text{π}}^2} \epsilon^{\mu\nu\lambda\rho}\partial_\mu A^c_\nu \partial_\lambda A^c_\rho$${\mathbb{Z}}^T_2$破缺的 $\partial\varSigma^3$:
$\dfrac{N}{2{\text{π}}}\partial_0 \phi^c \epsilon^{ij}\partial_i \lambda^c_j$${\mathbb{Z}}^T_2$破缺的$\varSigma^2$:
$\left(\begin{array}{*{20}{c}} {2 p}&N\\ N&0 \end{array}\right)$${\mathbb{Z}}_N\!\times\!{\mathbb{Z}}^T_2$ ${\mathbb{Z}}_N$ ${\mathbb{Z}}^T_2$ $\dfrac{N}{4{\text{π}}} \epsilon^{\mu\nu\lambda\rho} B^s_{\mu\nu}\partial_\lambda A_\rho^s+$
$\dfrac{\theta_s}{8{\text{π}}^2} \epsilon^{\mu\nu\lambda\rho}\partial_\mu A^s_\nu \partial_\lambda A^s_\rho$${\mathbb{Z}}^T_2$破缺的$\partial\varSigma^3$:
$\dfrac{N}{2{\text{π}}}\partial_0 \phi^s \epsilon^{ij}\partial_i \lambda^s_j$${\mathbb{Z}}^T_2$破缺的$\varSigma^2$:
$ \left({\begin{array}{*{20}{c}} 2 p &N \\ N & 0 \end{array}} \right)$${\mathbb{Z}}_N \!\times\! [U(1)_{S^z}\rtimes{\mathbb{Z}}_2]$ ${\mathbb{Z}}_N\!\times\! U(1)_{S^z}$ ${\mathbb{Z}}_2$ $\dfrac{N}{4{\text{π}}} \epsilon^{\mu\nu\lambda\rho} B^c_{\mu\nu}\partial_\lambda A_\rho^c +$
$\dfrac{\theta_0}{4{\text{π}}^2} \epsilon^{\mu\nu\lambda\rho}\partial_\mu A^s_\nu \partial_\lambda A^c_\rho$${\mathbb{Z}}_2$破缺的$\partial\varSigma^3$:
$\dfrac{N}{2{\text{π}}}\partial_0 \phi^c \epsilon^{ij}\partial_i \lambda^c_j$${\mathbb{Z}}_2$破缺的$\varSigma^2$:
$ \left({\begin{array}{*{20}{c}} 2 p_1 &N & p_{12}& 0\\ N & 0 &0 & 0\\ p_{12} & 0 &2 p_2 & 0\\ 0 & 0 &0 & 0 \end{array}} \right)$$U(1)_C \!\times\! [{\mathbb{Z}}_N \rtimes{\mathbb{Z}}_2]$ $U(1)_C\!\times\!{\mathbb{Z}}_N$ ${\mathbb{Z}}_2$ $\dfrac{N}{4{\text{π}}} \epsilon^{\mu\nu\lambda\rho} B^s_{\mu\nu}\partial_\lambda A_\rho^s+$
$\dfrac{\theta_0}{4{\text{π}}^2} \epsilon^{\mu\nu\lambda\rho}\partial_\mu A^s_\nu \partial_\lambda A^c_\rho$${\mathbb{Z}}_2$破缺的$\partial\varSigma^3$:
$\dfrac{N}{2{\text{π}}}\partial_0 \phi^s \epsilon^{ij}\partial_i \lambda^s_j$${\mathbb{Z}}_2$破缺的$\varSigma^2$:
$ \left({\begin{array}{*{20}{c}} 2 p_1 &0 & p_{12}& 0\\ 0 & 0 &0 & 0\\ p_{12} & 0 &2 p_2 & N\\ 0 & 0 &N & 0 \end{array}} \right)$${\mathbb{Z}}_{N_1} \!\times\! [{\mathbb{Z}}_{N_2}\rtimes{\mathbb{Z}}_2]$ ${\mathbb{Z}}_{N_1}\!\times\! {\mathbb{Z}}_{N_2}$ ${\mathbb{Z}}_2$ $\dfrac{N_1}{4{\text{π}}} \epsilon^{\mu\nu\lambda\rho} B^c_{\mu\nu}\partial_\lambda A_\rho^c+$
$\dfrac{N_2}{4{\text{π}}} \epsilon^{\mu\nu\lambda\rho} B^s_{\mu\nu}\partial_\lambda A_\rho^s+$
$\dfrac{\theta_0}{4{\text{π}}^2} \epsilon^{\mu\nu\lambda\rho}\partial_\mu A^s_\nu \partial_\lambda A^c_\rho$${\mathbb{Z}}_2$破缺的$\partial\varSigma^3$:
$\dfrac{N_1}{2{\text{π}}}\partial_0 \phi^c \epsilon^{ij}\partial_i \lambda^c_j+$
$\dfrac{N_2}{2{\text{π}}}\partial_0 \phi^s \epsilon^{ij}\partial_i \lambda^s_j$${\mathbb{Z}}_2$破缺的$\varSigma^2$:
$\begin{aligned} & {}\\ & \left({\begin{array}{*{20}{c}} 2 p_1 &N_1 & p_{12}& 0\\ N_1 & 0 &0 & 0\\ p_{12} & 0 &2 p_2 & N_2\\ 0 & 0 &N_2 & 0 \end{array}} \right)\end{aligned}$
下载: 导出CSV
表 6 带整数自旋和电荷的玻色SPT的电荷和自旋响应理论[51].
Table 6. Charge and spin response of spin-1 and charge-1 boson systems.
轴子角 对称群 三维体内($\varSigma^3$)的响应 二维表面($\partial\varSigma^3$)的反常响应 二维平面($\varSigma^2$)的响应 $ \theta_c=2{\text{π}}+4{\text{π}} k$
(带电玻色系统)$U(1)_C\rtimes{\mathbb{Z}}^{\rm T}_2$ 电荷-威腾效应:
$N^c=n^c+N^c_m$量子电荷霍尔效应
(${\mathbb{Z}}^{\rm T}_2$破缺的$\partial\varSigma^3$):
$\widetilde{\sigma}^{c}=(1+2 k)\dfrac{1}{2{\text{π}}}$量子电荷霍尔效应
(${\mathbb{Z}}^{\rm T}_2$破缺的$\varSigma^2$)
$\sigma^c=2 k\dfrac{1}{2{\text{π}}}$$ \theta_s=2{\text{π}}+4{\text{π}} k$
(整数自旋的
玻色系统)$U(1)_{S^z} \times {\mathbb{Z}}^{\rm T}_2$ 自旋-威腾效应:
$N^s=\displaystyle \sum_i q_in_i^s+N^s_m\sum_{i}q_i^2$量子自旋霍尔效应
(${\mathbb{Z}}^{\rm T}_2$破缺的$\partial\varSigma^3$):
$\widetilde{\sigma}^{s}=(1+2 k)\dfrac{1}{2{\text{π} } }\displaystyle\sum_i{q_i^2}$量子自旋霍尔效应
(${\mathbb{Z}}^{\rm T}_2$破缺的$\varSigma^2$)
$\sigma^s=2 k\dfrac{1}{2{\text{π} } }\displaystyle\sum_i{q_i^2}$$ \theta_0={\text{π}}+2{\text{π}} k$
(带电和整数自旋
的玻色系统)$U(1)_C \!\times\! [U(1)_{S^z} \!\rtimes\! {\mathbb{Z}}_2]$ 交互-威腾效应: $N^c=n^c+\dfrac{1}{2}N^s_m$;
$N^s=n^s_{+}-n^s_{-}+\dfrac{1}{2}N^c_m$量子电荷-自旋/
自旋-电荷效应
(${\mathbb{Z}}_2$破缺的 $\partial\varSigma^3$):
$\widetilde{\sigma}^{cs}=\widetilde{\sigma}^{sc}=\left(\dfrac 1 2+k\right)\dfrac{1}{2{\text{π}}}$量子电荷-自旋/
效应 自旋-电荷
(${\mathbb{Z}}_2$破缺的$\varSigma^2$):
$\sigma^{cs}=\sigma^{sc}=k\dfrac{1}{2{\text{π}}}$
下载: 导出CSV
表 7 推广的Wen-Zee拓扑项, 摘自[160].
Table 7. Generalized Wen-Zee terms.
时空维度 空间对称群$G_s$ 内部对称群$G_i$ 不可约的Wen-Zee拓扑项$S$ 角动量/自旋${\cal{J}}$ $(2 + 1)$维 $SO(2)$ $U(1)$ $\dfrac{k}{2{\text{π}}} \displaystyle\int \omega \wedge {\rm d}A$, $k \in \mathbb{Z}$ $\dfrac{k}{2{\text{π}}} \displaystyle\int_{M^2} {\rm d}A$ $(2 + 1)$维 $C_{N_0}$ $\mathbb{Z}_{N_1}$ $\dfrac{k}{2{\text{π}}} \displaystyle\int \omega \wedge {\rm d}A$, $k \in \mathbb{Z}_{N_{01}}$, $\dfrac{k}{2{\text{π}}} \displaystyle\int_{M^2} {\rm d}A$ $(2 + 1)$维 $C_{N_0}$ $\mathbb{Z}_{N_1} \times \mathbb{Z}_{N_2} $ $k \dfrac{ N_1 N_2}{(2{\text{π}})^2 N_{012}} \displaystyle\int \omega \wedge A^1 \wedge A^2$, $k \in \mathbb{Z}_{N_{012}}$ $k \dfrac{ N_1 N_2}{(2{\text{π}})^2 N_{012}} \displaystyle\int_{M^2} A^1 \wedge A^2$ $(3 + 1)$维 $C_{N_0}$ $\mathbb{Z}_{N_1}$ $k \dfrac{N_0 N_1}{ (2{\text{π}})^2 N_{01}} \displaystyle\int \omega \wedge A \wedge {\rm d}A$, $k \in \mathbb{Z}_{N_{01}}$ $k \dfrac{ N_1}{ (2{\text{π}})^2 N_{01}} \displaystyle\int _{M^3} A \wedge {\rm d}A$ $(3 + 1)$维 $C_{N_0}$ $\mathbb{Z}_{N_1}$ $k \dfrac{N_0 N_1}{(2{\text{π}})^2 N_{01}} \displaystyle\int A \wedge \omega \wedge {\rm d} \omega$, $k \in \mathbb{Z}_{N_{01}}$ $k \dfrac{ N_1}{2{\text{π}}^2 N_{01}} \displaystyle\int_{M^3} A \wedge {\rm d}\omega$ $(3 + 1)$维 $C_{N_0}$ $\mathbb{Z}_{N_1} \times U(1)$ $k \dfrac{N_0 N_1}{(2{\text{π}})^2 N_{01}} \displaystyle\int \omega \wedge A^1 \wedge {\rm d}A^2$, $k \in \mathbb{Z}_{N_{01}}$ $k \dfrac{N_1}{(2{\text{π}})^2 N_{01}} \displaystyle\int_{M^3} A^1 \wedge {\rm d}A^2$ $(3 + 1)$维 $SO(2)$ $\mathbb{Z}_{N_1} \times \mathbb{Z}_{N_2}$ $k \dfrac{N_1 N_2}{(2{\text{π}})^2 N_{12}} \displaystyle\int A^1 \wedge A^2 \wedge {\rm d} \omega$, $k \in \mathbb{Z}_{N_{12}}$ $k \dfrac{N_1 N_2}{(2{\text{π}})^2 N_{12}} \displaystyle\int_{M^3} {\rm d} (A^1 \wedge A^2)$ $(3 + 1)$维 $C_{N_0}$ $\mathbb{Z}_{N_1} \times \mathbb{Z}_{N_2}$ $k \dfrac{N_0 N_1}{(2{\text{π}})^2 N_{01}} \displaystyle\int \omega \wedge A^1 \wedge {\rm d}A^2$, $k \in \mathbb{Z}_{N_{012}}$ $k \dfrac{N_1}{(2{\text{π}})^2 N_{01}} \displaystyle\int_{M^3} A^1 \wedge {\rm d}A^2$ $(3 + 1)$维 $C_{N_0}$ $\mathbb{Z}_{N_1} \times \mathbb{Z}_{N_2}$ $k \dfrac{N_0 N_2}{(2{\text{π}})^2 N_{02}} \displaystyle\int \omega \wedge A^2 \wedge {\rm d}A^1$, $k \in \mathbb{Z}_{N_{012}}$ $k \dfrac{ N_2}{(2{\text{π}})^2 N_{02}} \displaystyle\int_{M^3} A^2 \wedge {\rm d}A^1$ $(3 + 1)$维 $C_{N_0}$ $\mathbb{Z}_{N_1} \times \mathbb{Z}_{N_2} \times \mathbb{Z}_{N_3}$ $k \dfrac{N_0 N_1 N_2 N_3}{(2{\text{π}})^3 N_{0123}} \displaystyle\int \omega \wedge A^1 \wedge A^2 \wedge A^3$,
$k \in \mathbb{Z}_{N_{0123}}$$k \dfrac{ N_1 N_2 N_3}{(2{\text{π}})^3 N_{0123}} \displaystyle\int_{M^3} A^1 \wedge A^2 \wedge A^3$ $(3 + 1)$维($*$) $SO(2)$ $U(1)$ $\dfrac{k}{2{\text{π}}} \displaystyle\int \omega \wedge {\rm d}B$, $k \in \mathbb{Z}$ $\dfrac{k}{2{\text{π}}} \displaystyle\int_{M^3} {\rm d}B$ $(3 + 1)$维($*$) $C_{N_0}$ ${\mathbb{Z}}_{N_1}$ $\dfrac{k}{2{\text{π}}} \displaystyle\int \omega \wedge {\rm d}B$, $k \in \mathbb{Z}_{N_{01}}$ $\dfrac{k}{2{\text{π}}} \displaystyle\int_{M^3} {\rm d}B$ $(3 + 1)$维($*$) $C_{N_0}$ $\mathbb{Z}_{N_1} \times \mathbb{Z}_{N_2}$ $k \dfrac{N_0 N_1 N_2}{(2{\text{π}})^2 N_{012}} \displaystyle\int \omega \wedge A \wedge B$, $k \in \mathbb{Z}_{N_{012}}$ $k \dfrac{N_1 N_2}{(2{\text{π}})^2 N_{012}} \displaystyle\int_{M^3} A \wedge B$
下载: 导出CSV
-
[1] Chaikin P M, Lubensky T C 2000 Principles of Condensed Matter Physics (Vol. 1) (Cambridge: Cambridge University Press)
[2] Wen X G 1989 Phys. Rev. B 40 7387
[3] Wen X G 1991 International Journal of Modern Physics B 5 1641
Google Scholar
[4] Zhang S C, Hansson T H, Kivelson S 1989 Phys. Rev. Lett. 62 82
Google Scholar
[5] Lopez A, Fradkin E 1991 Phys. Rev. B 44 5246
Google Scholar
[6] Jain J K 2007 Composite Fermions (Cambridge: Cambridge University Press)
[7] Jain J K 1989 Phys. Rev. B 40 8079
Google Scholar
[8] Jain J K 1989 Phys. Rev. Lett. 63 199
Google Scholar
[9] Wen X G, 1990 International Journal of Modern Physics B 4 239
Google Scholar
[10] Wen X G 2016 Natl. Sci. Rev. 3 68
[11] Wen X G 2004 Quantum Field Theory of Many-body Systems: from the Origin of Sound to An Origin of Light and Electrons. (Oxford: Oxford University Press)
[12] Haldane F 1983 Physics Letters A 93 464
Google Scholar
[13] Chen X, Gu Z C, Wen X G 2010 Phys. Rev. B 82 155138
Google Scholar
[14] Verstraete F, Cirac J I, Latorre J I, Rico E, Wolf M M 2005 Phys. Rev. Lett. 94 140601
Google Scholar
[15] Vidal G 2007 Phys. Rev. Lett. 99 220405
Google Scholar
[16] Affleck I, Kennedy T, Lieb E H, Tasaki H 1987 Phys. Rev. Lett. 59 799
Google Scholar
[17] Gu Z C, Wen X G 2009 Phys. Rev. B 80 155131
Google Scholar
[18] Pollmann F, Berg E, Turner A M, Oshikawa M 2012 Phys. Rev. B 85 075125
Google Scholar
[19] Pollmann F, Turner A M, Berg E, Oshikawa M 2010 Phys. Rev. B 81 064439
Google Scholar
[20] Wang Q R, Ye P 2014 Phys. Rev. B 90 045106
[21] Chen X, Liu Z X, Wen X G 2011 Phys. Rev. B 84 235141
Google Scholar
[22] He Y C, Bhattacharjee S, Moessner R, Pollmann F 2015 Phys. Rev. Lett. 115 116803
Google Scholar
[23] Senthil T, Levin M 2013 Phys. Rev. Lett. 110 046801
Google Scholar
[24] Regnault N, Senthil T 2013 Phys. Rev. B 88 161106
Google Scholar
[25] Levin M, Gu Z C 2012 Phys. Rev. B 86 115109
Google Scholar
[26] Liu Z X, Wen X G 2013 Phys. Rev. Lett. 110 067205
Google Scholar
[27] Wang C, Nahum A, Senthil T 2015 Phys. Rev. B 91 195131
Google Scholar
[28] Vishwanath A, Senthil T 2013 Phys. Rev. X 3 011016
[29] Chen X, Gu Z C, Liu Z X, Wen X G 2013 Phys. Rev. B 87 155114
Google Scholar
[30] Chen X, Gu Z C, Liu Z X, Wen X G 2012 Science 338 1604
Google Scholar
[31] Kapustin A 2014 arXiv: 1404.6659
[32] Wang Z 2010 Topological Quantum Computation (American Mathematical Society)
[33] Nayak C, Simon S H, Stern A, Freedman M, Das Sarma S 2008 Rev. Mod. Phys. 80 1083
Google Scholar
[34] Lan T, Kong L, Wen X G 2018 Phys. Rev. X 8 021074
[35] Lan T, Wen X G 2019 Phys. Rev. X 9 021005
[36] Kitaev A, Laumann C 2009 Les Houches Summer School Exact Methods in Low-dimensional Physics and Quantum Computing 89 101
[37] Levin M A, Wen X G 2005 Phys. Rev. B 71 045110
Google Scholar
[38] Levin M, Wen X G 2005 Rev. Mod. Phys. 77 871
Google Scholar
[39] Dijkgraaf R, Witten E 1990 Commun. Math. Phys. 129 393
Google Scholar
[40] Kapustin A, Seiberg N 2014 J. High Energ. Phys. 2014 1
[41] Savary L, Balents L 2016 Reports on Progress in Physics 80 016502
[42] Zhou Y, Kanoda K, Ng T K 2017 Rev. Mod. Phys. 89 025003
Google Scholar
[43] Barkeshli M, Bonderson P, Cheng M, Wang Z 2019 Phys. Rev. B 100 115147
Google Scholar
[44] Lan T, Kong L, Wen X G 2017 Phys. Rev. B 95 235140
Google Scholar
[45] Kong L, Wen X G 2014 arXiv: 1405.5858
[46] Lan T, Kong L, Wen X G 2017 Communications in Mathematical Physics 351 709
Google Scholar
[47] Etingof P, Nikshych D, Ostrik V 2010 Quantum Topology 1 209
[48] Ning S Q, Liu Z X, Ye P 2016 Phys. Rev. B 94 245120
Google Scholar
[49] Ye P 2018 Phys. Rev. B 97 125127
Google Scholar
[50] Ning S Q, Liu Z X, Ye P 2018 arXiv: 1801.01638
[51] Ye P, Wang J 2013 Phys. Rev. B 88 235109
Google Scholar
[52] Mesaros A, Ran Y 2013 Phys. Rev. B 87 155115
Google Scholar
[53] Xu C 2013 Phys. Rev. B 88 205137
Google Scholar
[54] Chen X, Hermele M 2016 Phys. Rev. B 94 195120
Google Scholar
[55] Chen G 2017 Phys. Rev. B 96 195127
Google Scholar
[56] Chen G 2017 Phys. Rev. B 96 085136
Google Scholar
[57] Cheng M 2015 arXiv: 1511.02563
[58] Zou L, Wang C, Senthil T 2018 Phys. Rev. B 97 195126
Google Scholar
[59] Ning S Q, Zou L, Cheng M 2019 arXiv: 1905.03276
[60] Wang C, Senthil T 2016 Phys. Rev. X 6 011034
[61] Lee P A, Nagaosa N, Wen X G, 2006 Rev. Mod. Phys. 78 17
Google Scholar
[62] Nagaosa N, 1999 Quantum field theory in strongly correlated electronic systems. Springer Science & Business Media
[63] Fradkin E 2013 Field Theories of Condensed Matter Physics (Cambridge: Cambridge University Press)
[64] Baskaran G, Zou Z, Anderson P 1987 Solid State Commun. 63 973
Google Scholar
[65] Baskaran G, Anderson P W 1988 Phys. Rev. B 37 580
Google Scholar
[66] Affleck I, Marston J B 1988 Phys. Rev. B 37 3774
Google Scholar
[67] Kotliar G, Liu J 1988 Phys. Rev. B 38 5142
Google Scholar
[68] Suzumura Y, Hasegawa Y, Fukuyama H 1988 J. Phys. Soc. Jpn. 57 2768
Google Scholar
[69] Affleck I, Zou Z, Hsu T, Anderson P 1988 Phys. Rev. B 38 745
Google Scholar
[70] Dagotto E, Fradkin E, Moreo A 1988 Phys. Rev. B 38 2926
Google Scholar
[71] Wen X G, Wilczek F, Zee A 1989 Phys. Rev. B 39 11413
Google Scholar
[72] Wen X G 1991 Phys. Rev. B 44 2664
[73] Lee P A, Nagaosa N 1992 Phys. Rev. B 46 5621
Google Scholar
[74] Mudry C, Fradkin E 1994 Phys. Rev. B 49 5200
Google Scholar
[75] Wen X G, Lee P A 1996 Phys. Rev. Lett. 76 503
Google Scholar
[76] Weng Z Y, Sheng D N, Chen Y C, Ting C S 1997 Phys. Rev. B 55 3894
Google Scholar
[77] Ye P, Tian C S, Qi X L, Weng Z Y 2011 Phys. Rev. Lett. 106 147002
Google Scholar
[78] Ye P, Tian C S, Qi X L, Weng Z Y 2012 Nucl. Phys. B 854 815
Google Scholar
[79] Wen X G 1991 Mod. Phys. Lett. B 05 39
Google Scholar
[80] Wen X G 1999 Phys. Rev. B 60 8827
Google Scholar
[81] Barkeshli M, Wen X G 2010 Phys. Rev. B 81 155302
Google Scholar
[82] Lu Y M, Lee D H 2014 Phys. Rev. B 89 184417
Google Scholar
[83] Ye P, Wen X G 2013 Phys. Rev. B 87 195128
Google Scholar
[84] Ye P, Wen X G 2014 Phys. Rev. B 89 045127
Google Scholar
[85] Ye P, Hughes T L, Maciejko J, Fradkin E 2016 Phys. Rev. B 94 115104
Google Scholar
[86] Liu Z X, Mei J W, Ye P, Wen X G 2014 Phys. Rev. B 90 235146
Google Scholar
[87] Deser S, Jackiw R, Templeton S 1982 Ann. Phys. 140 372
Google Scholar
[88] Laughlin R B 1981 Phys. Rev. B 23 5632
Google Scholar
[89] Wen X G, Zee A 1992 Phys. Rev. B 46 2290
Google Scholar
[90] Polyakov A M 1975 Phys. Lett. B 59 82
Google Scholar
[91] Polyakov A M 1977 Nucl. Phys. B 120 429
Google Scholar
[92] Polyakov A M 1978 Phys. Lett. B 72 477
Google Scholar
[93] Wen X G 2014 Phys. Rev. B 89 035147
Google Scholar
[94] Hohenadler M, Meng Z Y, Lang T C, Wessel S, Muramatsu A, Assaad F F 2012 Phys. Rev. B 85 115132
Google Scholar
[95] Griset C, Xu C 2012 Phys. Rev. B 85 045123
Google Scholar
[96] Lee D H 2011 Phys. Rev. Lett. 107 166806
Google Scholar
[97] Kitaev A, 2006 Ann. Phys. 321 2
Google Scholar
[98] Bombin H 2010 Phys. Rev. Lett. 105 030403
Google Scholar
[99] You Y Z, Wen X G 2012 Phys. Rev. B 86 161107
Google Scholar
[100] Teo J C, Hughes T L, Fradkin E 2015 Ann. Phys. 360 349
Google Scholar
[101] Khan M N, Teo J C Y, Hughes T L 2014 Phys. Rev. B 90 235149
Google Scholar
[102] Mesaros A, Kim Y B, Ran Y 2013 Phys. Rev. B 88 035141
Google Scholar
[103] Barkeshli M, Wen X G 2010 Phys. Rev. B 81 045323
Google Scholar
[104] Teo J C Y, Roy A, Chen X 2014 Phys. Rev. B 90 155111
Google Scholar
[105] Barkeshli M, Qi X L 2012 Phys. Rev. X 2 031013
[106] Barkeshli M, Jian C M, Qi X L 2013 Phys. Rev. B 87 045130
Google Scholar
[107] Barkeshli M, Qi X L 2014 Phys. Rev. X 4 041035
[108] Barkeshli M, Jiang H C, Thomale R, Qi X L 2015 Phys. Rev. Lett. 114 026401
Google Scholar
[109] 't Hooft G 1981 Nucl. Phys. B 190 455
[110] Mandelstam S 1980 Phys. Rep. 67 109
Google Scholar
[111] Peskin M E 1978 Ann. Phys. 113 122
Google Scholar
[112] 't Hooft G 1978 Nucl. Phys. B 138 1
[113] 't Hooft G 1979 Nucl. Phys. B 153 141
[114] Susskind L 1979 Phys. Rev. D 20 2610
[115] Fradkin E Susskind L 1978 Phys. Rev. D 17 2637
Google Scholar
[116] van Baal P 2002 Confinement, Duality, and Nonperturbative Aspects of QCD. (Kluwer: Kluwer Academic Publishers)
[117] Seiberg N, Witten E 1994 Nucl. Phys. B 431 484
Google Scholar
[118] Witten E 1979 Phys. Lett. B 86 283
Google Scholar
[119] Qi X L, Hughes T L, Zhang S C 2008 Phys. Rev. B 78 195424
Google Scholar
[120] Rosenberg G, Franz M 2010 Phys. Rev. B 82 035105
Google Scholar
[121] Wen X G 2003 Phys. Rev. Lett. 90 016803
Google Scholar
[122] Horowitz G T 1989 Commun. Math. Phys. 125 417
Google Scholar
[123] Baez J C, Huerta J 2011 General Relativity and Gravitation 43 2335
Google Scholar
[124] Blau M, Thompson G 1991 Ann. Phys. 205 130
Google Scholar
[125] Bergeron M, Semenoff G W, Szabo R J 1995 Nucl. Phys. B 437 695
Google Scholar
[126] Szabo R J 1998 Nucl. Phys. B 531 525
Google Scholar
[127] Lu Y M, Vishwanath A 2012 Phys. Rev. B 86 125119
Google Scholar
[128] Gu Z C, Wang J C, Wen X G 2016 Phys. Rev. B 93 115136
Google Scholar
[129] Ye P, Gu Z C 2016 Phys. Rev. B 93 205157
Google Scholar
[130] Ye P, Gu Z C 2015 Phys. Rev. X 5 021029
[131] v Bodecker H, Hornig G, 2004 Phys. Rev. Lett. 92 030406
Google Scholar
[132] Wen X, Wen X G 2014 arXiv: 1908.10381
[133] Wilczek F 1990 Fractional Statistics and Anyon Superconductivity (Vol. 5) (Singapore: World Scientific)
[134] Wu Y S 1984 Phys. Rev. Lett. 52 2103
Google Scholar
[135] Bonderson P, Shtengel K, Slingerland J 2008 Annals of Physics 323 2709
Google Scholar
[136] Witten E 1989 Commun. Math. Phys. 121 351
Google Scholar
[137] Leinaas J M, Myrheim J, 1977 Il Nuovo Cimento B 37 1
[138] Wen X, He H, Tiwari A, Zheng Y, Ye P 2018 Phys. Rev. B 97 085147
Google Scholar
[139] Hansson T H, Oganesyan V, Sondhi S L 2004 Annals of Physics 313 497
Google Scholar
[140] Wang C, Levin M 2014 Phys. Rev. Lett. 113 080403
Google Scholar
[141] Wang J C, Wen X G 2015 Phys. Rev. B 91 035134
Google Scholar
[142] Tiwari A, Chen X, Ryu S 2017 Phys. Rev. B 95 245124
Google Scholar
[143] Putrov P, Wang J, Yau S T 2017 Annals of Physics 384 254
Google Scholar
[144] Jian C M, Qi X L 2014 Phys. Rev. X 4 041043
[145] Wang C, Lin C H, Levin M 2016 Phys. Rev. X 6 021015
[146] Wan Y, Wang J C, He H 2015 Phys. Rev. B 92 045101
Google Scholar
[147] Kapustin A, Thorngren R 2014 arXiv: 1404.3230
[148] Wang J C, Gu Z C, Wen X G 2015 Phys. Rev. Lett. 114 031601
Google Scholar
[149] Chen X, Tiwari A, Ryu S 2016 Phys. Rev. B 94 045113
Google Scholar
[150] Wang J, Wen X G, Yau S T 2016 arXiv: 1602.05951
[151] Jiang S, Mesaros A, Ran Y 2014 Phys. Rev. X 4 031048
[152] Ye P, 2020 unpublished
[153] von Keyserlingk C W, Burnell F J, Simon S H 2013 Phys. Rev. B 87 045107
Google Scholar
[154] Walker K, Wang Z 2012 Frontiers of Physics 7 150
Google Scholar
[155] Wang Q R, Cheng M, Wang C, Gu Z C 2019 Phys. Rev. B 99 235137
Google Scholar
[156] Wang Z, Chen X 2017 Phys. Rev. B 95 115142
Google Scholar
[157] Chan A P O, Ye P, Ryu S 2018 Phys. Rev. Lett. 121 061601
Google Scholar
[158] Milnor J 1954 Ann. Math. 59 177
Google Scholar
[159] Hatcher A 2002 Algebraic Topology. (Cambridge: Cambridge University Press)
[160] Han B, Wang H, Ye P 2019 Phys. Rev. B 99 205120
Google Scholar
[161] Hasan M Z, Kane C L 2010 Rev. Mod. Phys. 82 3045
Google Scholar
[162] Qi X L, Zhang S C 2011 Rev. Mod. Phys. 83 1057
Google Scholar
[163] Lapa M F, Jian C M, Ye P, Hughes T L 2017 Phys. Rev. B 95 035149
Google Scholar
[164] Witten E 2016 Rev. Mod. Phys. 88 035001
Google Scholar
[165] Witten E 1995 Selecta Mathematica 1 383
Google Scholar
[166] Vafa C, Witten E 1994 Nuclear Physics B 431 3
Google Scholar
[167] Montonen C, Olive D 1977 Physics Letters B 72 117
Google Scholar
[168] Son D T 2015 Phys. Rev. X 5 031027
[169] Metlitski M A, Vishwanath A 2016 Phys. Rev. B 93 245151
Google Scholar
[170] Wang C, Senthil T 2016 Phys. Rev. B 93 085110
Google Scholar
[171] Mross D F, Alicea J, Motrunich O I 2016 Phys. Rev. Lett. 117 016802
Google Scholar
[172] Kachru S, Mulligan M, Torroba G, Wang H 2015 Phys. Rev. B 92 235105
Google Scholar
[173] Karch A, Tong D 2016 Phys. Rev. X 6 031043
[174] Seiberg N, Senthil T, Wang C, Witten E 2016 Annals of Physics 374 395
Google Scholar
[175] Fradkin E, Kivelson S 1996 Nuclear Physics B 474 543
Google Scholar
[176] Witten E 2016 arXiv: hep-th/0307041
[177] Gaiotto D, Witten E 2009 Adv. Theor. Math. Phys. 13 721
Google Scholar
[178] Chen X, Fidkowski L, Vishwanath A 2014 Phys. Rev. B 89 165132
Google Scholar
[179] Wang C, Potter A C, Senthil T 2014 Science 343 629
Google Scholar
[180] Bonderson P, Nayak C, Qi X L 2013 J. Stat. Mech. 201 3
[181] Metlitski M A, Kane C L, Fisher M P A 2015 Phys. Rev. B 92 125111
Google Scholar
[182] Maciejko J, Qi X L, Karch A, Zhang S C 2010 Phys. Rev. Lett. 105 246809
Google Scholar
[183] Maciejko J, Qi X L, Karch A, Zhang S C 2012 Phys. Rev. B 86 235128
Google Scholar
[184] Maciejko J, Fiete G A 2015 Nat. Phys. 11 385
Google Scholar
[185] Swingle B, Barkeshli M, McGreevy J, Senthil T 2011 Phys. Rev. B 83 195139
Google Scholar
[186] Sahoo S, Sirota A, Cho G Y, Teo J C Y 2017 Phys. Rev. B 96 161108
Google Scholar
[187] Levin M, Burnell F J, Koch-Janusz M, Stern A 2011 Phys. Rev. B 84 235145
Google Scholar
[188] Swingle B 2012 Phys. Rev. B 86 245111
Google Scholar
[189] Ye P, Cheng M, Fradkin E 2017 Phys. Rev. B 96 085125
Google Scholar
[190] Hirzebruch F, Berger T, Jung R, Landweber P S 1992 Manifolds and Modular Forms (Vol. 20) (Springer, 1992)
[191] Chan A P O, Kvorning T, Ryu S, Fradkin E 2016 Phys. Rev. B 93 155122
Google Scholar
[192] Liu C X, Ye P, Qi X L 2013 Phys. Rev. B 87 235306
Google Scholar
[193] Liu C X, Ye P, Qi X L 2015 Phys. Rev. B 92 119904
Google Scholar
[194] Liu C X, Ye P, Qi X L 2017 Phys. Rev. B 96 247302
Google Scholar
[195] Ye P, Zhang L, Weng Z Y 2012 Phys. Rev. B 85 205142
Google Scholar
[196] Ma Y, Ye P, Weng Z Y 2014 New Journal of Physics 16 083039
Google Scholar
[197] Xu C, Sachdev S 2009 Phys. Rev. B 79 064405
Google Scholar
[198] Cheng M, Gu Z C 2014 Phys. Rev. Lett. 112 141602
Google Scholar
[199] Hung L Y, Wen X G 2013 Phys. Rev. B 87 165107
Google Scholar
[200] Goldhaber A S, MacKenzie R, Wilczek F 1989 Mod. Phys. Lett. A 4 21
Google Scholar
[201] Fu L 2011 Phys. Rev. Lett. 106 106802
Google Scholar
[202] Chiu C K, Teo J C Y, Schnyder A P, Ryu S 2016 Rev. Mod. Phys. 88 035005
Google Scholar
[203] Song H, Huang S J, Fu L, Hermele M 2017 Phys. Rev. X 7 011020
[204] Huang S J, Song H, Huang Y P, Hermele M 2017 Phys. Rev. B 96 205106
Google Scholar
[205] Huang S J, Hermele M 2018 Phys. Rev. B 97 075145
Google Scholar
[206] Song Z, Huang S J, Qi Y, Fang C, Hermele M 2019 Science Advances 5 eaax2007
[207] Thorngren R, Else D V 2018 Phys. Rev. X 8 011040
[208] Jiang S, Ran Y 2017 Phys. Rev. B 95 125107
Google Scholar
[209] Wen X G, Zee A 1992 Phys. Rev. Lett. 69 953
Google Scholar
[210] Gaiotto D, Kapustin A, Seiberg N 2015 Journal of High Energy Physics 2015 172
[211] Katanaev M, Volovich I 1992 Annals of Physics 216 1
Google Scholar
[212] Landau L D, Lifshitz E M 1986 Theory of Elasticity of Course of Theoretical Physics (Vol. 7) (New York: Elsevier Butterworth-Heinemann)
[213] Hehl F W, Obukhov Y N 2007 Annales Fond. Broglie 0711 1535
[214] Nakahara M 2003 Geometry, Topology and Physics (2nd Ed.) (Graduate Student Series in Physics) (Taylor & Francis, 2003)
[215] Xu C, Senthil T 2013 Phys. Rev. B 87 174412
Google Scholar
[216] Bi Z, Rasmussen A, Slagle K, Xu C 2015 Phys. Rev. B 91 134404
Google Scholar
[217] You Y Z, Xu C 2014 Phys. Rev. B 90 245120
Google Scholar
[218] Bi Z, You Y Z, Xu C 2014 Phys. Rev. B 90 081110
Google Scholar
[219] Bi Z, Rasmussen A, Xu C 2014 Phys. Rev. B 89 184424
Google Scholar
[220] Xu C 2013 Phys. Rev. B 87 144421
Google Scholar
[221] Wang Q R, Gu Z C 2018 Phys. Rev. X 8 011055
[222] Wen X G 2019 Phys. Rev. B 99 205139
Google Scholar
下载:
计量
- 文章访问数: 20398
- PDF下载量: 822
- 被引次数: 0
