AlGaN/GaN高电子迁移率器件外部边缘电容的物理模型

刘乃漳; 张雪冰; 姚若河

doi:10.7498/aps.69.20191931

摘要

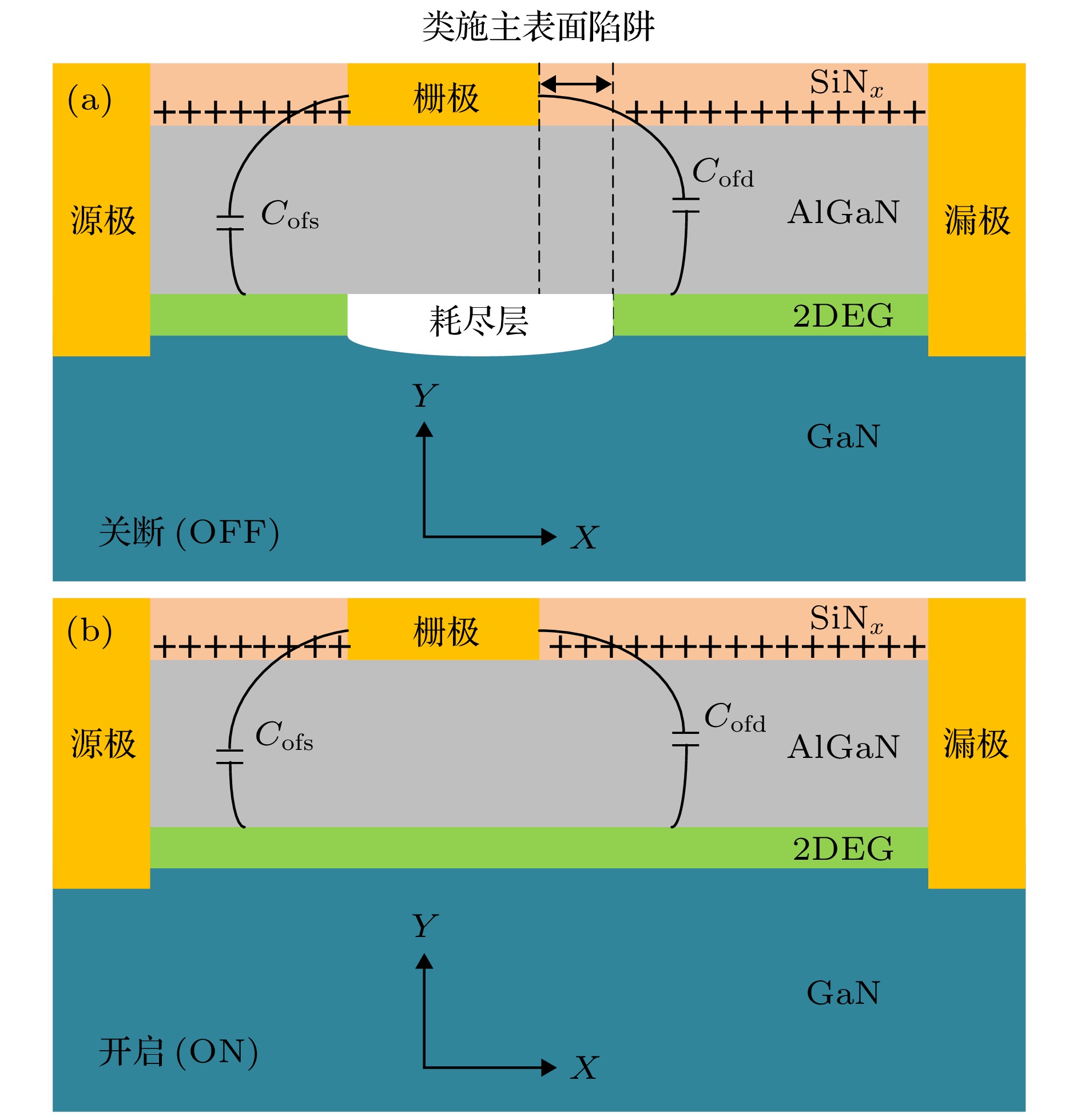
AlGaN/GaN HEMT外部边缘电容C_ofd是由栅极垂直侧壁与二维电子气水平壁之间的电场构成的等效电容. 本文基于保角映射法对C_ofd进行物理建模, 考虑沟道长度调制效应, 研究外部偏置、阈值电压漂移和温度变化对C_ofd的影响: 随着漏源偏压从零开始增加, C_ofd先保持不变再开始衰减, 其衰减速率随栅源偏压的增加而减缓; AlGaN势垒层中施主杂质浓度的减小和Al组分的减小都可引起阈值电压的正向漂移, 正向阈值漂移会加强沟道长度调制效应对C_ofd的影响, 导致C_ofd呈线性衰减. 在大漏极偏压工作情况下, C_ofd对器件工作温度的变化更加敏感.

关键词:

Abstract

With the development of the application of AlGaN/GaN high electron mobility transistors in the radio frequency field, a capacitance model that can accurately describe the C-V characteristics of the device has become an important research topic. The gate capacitance of GaN HEMT can be divided into two parts: intrinsic capacitance and fringing capacitance related to two-dimensional electronic gas (2DEG) electrode. The fringing capacitance plays an important part in the switching device. The outer fringing capacitance C_ofs/d dominates the fringing capacitance and is affected by the bias applied, especially the drain outer fringing capacitance C_ofd. In order to establish the C_ofd model which is related to the bias condition, the physics-based model of C_ofd is established based on the conformal mapping, including the drain channel length variable. Since the drain channel length is related to the bias applied, the channel length modulation effect can be used to study how bias apllied effect the channel, and the relationship between C_ofd and the bias condition is obtained. In addition, the threshold voltage variable is introduced when the channel length modulation effect is considered, and the threshold voltage drift caused by changes in the internal parameters and temperature of the device is studied using the threshold voltage variable in the model, and the relationship between C_ofd and threshold voltage and temperature under different bias was obtained. It is found from the results of the study that as drain bias increases from zero, the channel length modulation effect keeps C_ofd unchanged at lower drain bias. When the drain bias continues to increase, C_ofd begins to decay again, and its decay rate slows down with the increase of gate bias. The decrease of donor impurity concentration and Al component in AlGaN barrier layer may increase the threshold voltage, which will strengthen the channel length modulation effect on C_ofd, resulting in linear attenuation of C_ofd. With the increasing of drain bias, the influence of threshold voltage shift on C_ofd is enhanced, and the change of device operating temperature will enhance the threshold voltage shift and cause the deviation of C_ofd. Moreover, with the continuous increase of drain bias, C_ofd becomes more sensitive to the temperature variation.

Keywords:

作者及机构信息

华南理工大学电子与信息学院, 广州　510640

通信作者: 姚若河, phrhyao@scut.edu.cn

基金项目: 国家级-国家重点研究计划(2018YFB1802100)

Authors and contacts

School of Electronics and Information Technology, South China University of Technology, Guangzhou 510640, China

Corresponding author: Yao Ruo-He, phrhyao@scut.edu.cn

文章全文 : translate this paragraph

参考文献

[1]	Jones E A, Wang F F, Costinett D 2016 IEEE J. Emerg. Sel. Top. Power Electron. 4 707 Google Scholar
[2]	王林, 胡伟达, 陈效双, 陆卫 2010 59 5730 Google Scholar Wang L, Hu W D, Chen X S, Lu W 2010 Acta Phys. Sin. 59 5730 Google Scholar
[3]	Zhang A, Zhang L, Tang Z, Cheng X, Wang Y, Chen K J, Chan M 2014 IEEE Trans. Electron Devices 61 755 Google Scholar
[4]	Pregaldiny F, Lallement C, Mathiot D 2002 Solid-State Electron. 46 2191 Google Scholar
[5]	Bansal A, Paul B C, Roy K 2005 IEEE Trans. Electron Devices 52 256 Google Scholar
[6]	Li K, Rakheja S 2018 Device Research Conference-Conference Digest, DRC the University of California, Santa Barbara, June 24–27, 2018 p1
[7]	Jia Y, Xu Y, Wen Z, Wu Y, Guo Y 2019 IEEE Trans. Electron Devices 66 357 Google Scholar
[8]	Vetury R, Zhang N Q, Keller S, Mishra U K 2001 IEEE Trans. Electron Devices 48 560 Google Scholar
[9]	郭伟玲, 陈艳芳, 李松宇, 雷亮, 柏常青 2017 发光学报 38 1000 Guo Y L, Chen Y F, Li S Y, Lei L, Bai C Q 2017 Chin. J. Lumin. 38 1000
[10]	Cheng X, Wang Y 2011 IEEE Trans. Electron Devices 58 448 Google Scholar
[11]	Dasgupta N, DasGupta A 1993 Solid-State Electron. 36 201 Google Scholar
[12]	Huque M A, Eliza S A, Ragman T, Huq H F, Islam S K 2009 Solid-State Electron. 53 341
[13]	Ahsan S A, Ghosh S, Sharma K, Dasgupta A, Khandelwal S, Chauhan Y S 2016 IEEE Trans. Electron Devices 63 565
[14]	Rashmi, Kranti A, Haldar S, Gupta R S 2002 Solid-State Electron. 46 621 Google Scholar
[15]	Ambacher O, Smart J, Shealy J R, Weimann N G, Chu K, Murphy M, Schaff W J, Eastman L F, Dimitrov R, Wittmer L, Stutzmann M, Rieger W, Hilsenbeck J 1999 J. Appl. Phys 85 3222 Google Scholar
[16]	范隆, 郝跃 2007 56 3393 Google Scholar Fan L, Hao Y 2007 Acta Phys. Sin. 56 3393 Google Scholar
[17]	Ambacher O, Majewski J, Miskys C, Link A, Hermann M, Eickhoff M, Stutzmann M, Bernardini F, Fiorentini V, Tilak V, Schaff B, Eastman L F 2002 J. Phys.-Condes. Matter 14 3399 Google Scholar
[18]	Khandelwal S, Chauhan Y S, Fjeldly T A 2012 IEEE Trans. Electron Devices 59 2856 Google Scholar
[19]	He X G, Zhao D G, Jiang D S 2015 Chin. Phys. B 24 067301 Google Scholar
[20]	Li M, Wang Y 2008 IEEE Trans. Electron Devices 55 261 Google Scholar
[21]	Alim M A, Rezazadeh A A, Gaquiere C 2016 Semicond. Sci. Technol. 31 125016 Google Scholar

施引文献

图 1 GaN HEMT不同工作状态下外部边缘电容示意图 (a)处于关断状态; (b)处于开启状态

Fig. 1. Schematic of GaN HEMT outer fringing capacitances in different state: (a) In the OFF-state; (b) in the ON-state.

下载: 全尺寸图片幻灯片

图 2 栅极侧壁与2DEG之间的电场示意图

Fig. 2. Schematic of normal electric field between the side wall of the gate and the 2DEG.

下载: 全尺寸图片幻灯片

图 3 (a)共焦后的电场示意图; (b) L_cd = L_d时的共焦电场

Fig. 3. (a) Electric field lines after transforming the nonconfocal elliptical system to the confocal system; (b) the confocal system with L_cd = L_d.

下载: 全尺寸图片幻灯片

图 4 L_cd = L_d所引入的误差

Fig. 4. Error in the confocal system with L_cd = L_d.

下载: 全尺寸图片幻灯片

图 5 2DEG沟道被类施主表面陷阱耗尽的长度对C_ofd的影响关系图

Fig. 5. C_ofd versus the extended depletion length induced by donor-like surface traps.

下载: 全尺寸图片幻灯片

图 6 V_g与2DEG浓度n_s和E_f的关系曲线

Fig. 6. The curve of the density n_s of 2DEG and E_f versus V_g

下载: 全尺寸图片幻灯片

图 7 V_g与V_dsat的关系曲线

Fig. 7. The curve of V_dsat versus V_g.

下载: 全尺寸图片幻灯片

图 8 传统模型和本文模型得到的V_ds与C_ofd的关系曲线

Fig. 8. The curve of C_ofd versus V_ds obtained from the traditional model and the model in this paper.

下载: 全尺寸图片幻灯片

图 9 V_th对C_ofd的影响关系曲线(插图为V_th与Al组分x和掺杂浓度N_D的关系曲线)

Fig. 9. The curve ofC_ofd versus V_th(The illustration show the curve of V_th with Al component and doped concentration).

下载: 全尺寸图片幻灯片

图 10 温度T对C_ofd的影响关系曲线

Fig. 10. The curve of C_ofd versus T

下载: 全尺寸图片幻灯片

图 11 不同漏极偏压下C_ofd对温度敏感程度的关系曲线

Fig. 11. The curve oftemperature sensitivity of C_ofd under different drain bias.

下载: 全尺寸图片幻灯片

表 1 模型仿真的器件参数值

Table 1. Model parameters in this paper.

参数	定义	数值
ε_x	有效介电常数	7.65ε₀
E_sat/V·μm^–1	饱和电场	15
L_d/μm	漏端沟道长度	1
T_g/μm	栅极厚度	0.3
T_AlGaN/nm	AlGaN层厚度	22
$ E_{\rm g}^{\rm AIN} $/eV	AIN禁带宽度	6.13
$ E_{\rm g}^{\rm GaN} $/eV	GaN禁带宽度	3.42
V_temp	V_th的依赖系数温度	0.1689
T_NOM/K	器件温标	300
ξ₁	拟合参数	1.1
ξ₂	拟合参数	0.24
m	拟合参数	1.2
p	拟合参数	0.307
τ	拟合参数	3.2
a	拟合参数	1.497
b	拟合参数	1.9
c	拟合参数	0.31

下载: 导出CSV

map

[1]	Jones E A, Wang F F, Costinett D 2016 IEEE J. Emerg. Sel. Top. Power Electron. 4 707 Google Scholar
[2]	王林, 胡伟达, 陈效双, 陆卫 2010 59 5730 Google Scholar Wang L, Hu W D, Chen X S, Lu W 2010 Acta Phys. Sin. 59 5730 Google Scholar
[3]	Zhang A, Zhang L, Tang Z, Cheng X, Wang Y, Chen K J, Chan M 2014 IEEE Trans. Electron Devices 61 755 Google Scholar
[4]	Pregaldiny F, Lallement C, Mathiot D 2002 Solid-State Electron. 46 2191 Google Scholar
[5]	Bansal A, Paul B C, Roy K 2005 IEEE Trans. Electron Devices 52 256 Google Scholar
[6]	Li K, Rakheja S 2018 Device Research Conference-Conference Digest, DRC the University of California, Santa Barbara, June 24–27, 2018 p1
[7]	Jia Y, Xu Y, Wen Z, Wu Y, Guo Y 2019 IEEE Trans. Electron Devices 66 357 Google Scholar
[8]	Vetury R, Zhang N Q, Keller S, Mishra U K 2001 IEEE Trans. Electron Devices 48 560 Google Scholar
[9]	郭伟玲, 陈艳芳, 李松宇, 雷亮, 柏常青 2017 发光学报 38 1000 Guo Y L, Chen Y F, Li S Y, Lei L, Bai C Q 2017 Chin. J. Lumin. 38 1000
[10]	Cheng X, Wang Y 2011 IEEE Trans. Electron Devices 58 448 Google Scholar
[11]	Dasgupta N, DasGupta A 1993 Solid-State Electron. 36 201 Google Scholar
[12]	Huque M A, Eliza S A, Ragman T, Huq H F, Islam S K 2009 Solid-State Electron. 53 341
[13]	Ahsan S A, Ghosh S, Sharma K, Dasgupta A, Khandelwal S, Chauhan Y S 2016 IEEE Trans. Electron Devices 63 565
[14]	Rashmi, Kranti A, Haldar S, Gupta R S 2002 Solid-State Electron. 46 621 Google Scholar
[15]	Ambacher O, Smart J, Shealy J R, Weimann N G, Chu K, Murphy M, Schaff W J, Eastman L F, Dimitrov R, Wittmer L, Stutzmann M, Rieger W, Hilsenbeck J 1999 J. Appl. Phys 85 3222 Google Scholar
[16]	范隆, 郝跃 2007 56 3393 Google Scholar Fan L, Hao Y 2007 Acta Phys. Sin. 56 3393 Google Scholar
[17]	Ambacher O, Majewski J, Miskys C, Link A, Hermann M, Eickhoff M, Stutzmann M, Bernardini F, Fiorentini V, Tilak V, Schaff B, Eastman L F 2002 J. Phys.-Condes. Matter 14 3399 Google Scholar
[18]	Khandelwal S, Chauhan Y S, Fjeldly T A 2012 IEEE Trans. Electron Devices 59 2856 Google Scholar
[19]	He X G, Zhao D G, Jiang D S 2015 Chin. Phys. B 24 067301 Google Scholar
[20]	Li M, Wang Y 2008 IEEE Trans. Electron Devices 55 261 Google Scholar
[21]	Alim M A, Rezazadeh A A, Gaquiere C 2016 Semicond. Sci. Technol. 31 125016 Google Scholar

[1]	王帅, 葛晨, 徐祖银, 成爱强, 陈敦军. 微波GaN器件温度效应建模. , 2024, 73(17): 177101. doi: 10.7498/aps.73.20240765
[2]	张林, 马林东, 杜林, 李艳波, 徐先峰, 黄鑫蓉. 不同栅压下Si-n型金属氧化物半导体场效应管总剂量效应的瞬态特性仿真. , 2023, 72(13): 138501. doi: 10.7498/aps.72.20230207
[3]	谢天赐, 张彬, 贺泊, 李昊鹏, 秦壮, 钱金钱, 石锲铭, LewisElfed, 孙伟民. 放疗绝对剂量的数学算法模型. , 2021, 70(1): 018701. doi: 10.7498/aps.70.20200986
[4]	刘佳文, 姚若河, 刘玉荣, 耿魁伟. 一个圆柱形双栅场效应晶体管的物理模型. , 2021, 70(15): 157302. doi: 10.7498/aps.70.20202156
[5]	刘乃漳, 姚若河, 耿魁伟. AlGaN/GaN高电子迁移率晶体管的栅极电容模型. , 2021, 70(21): 217301. doi: 10.7498/aps.70.20210700
[6]	马骥刚, 马晓华, 张会龙, 曹梦逸, 张凯, 李文雯, 郭星, 廖雪阳, 陈伟伟, 郝跃. AlGaN/GaN高电子迁移率晶体管中kink效应的半经验模型. , 2012, 61(4): 047301. doi: 10.7498/aps.61.047301
[7]	段宝兴, 杨银堂, 陈敬. F离子注入新型Al0.25Ga0.75 N/GaN HEMT 器件耐压分析. , 2012, 61(22): 227302. doi: 10.7498/aps.61.227302
[8]	姜志宏, 王晖, 高超. 一种基于随机行走和策略连接的网络演化模型. , 2011, 60(5): 058903. doi: 10.7498/aps.60.058903
[9]	吴华英, 张鹤鸣, 宋建军, 胡辉勇. 单轴应变硅nMOSFET栅隧穿电流模型. , 2011, 60(9): 097302. doi: 10.7498/aps.60.097302
[10]	李斌, 刘红侠, 袁博, 李劲, 卢凤铭. 应变Si/Si1-xGex n型金属氧化物半导体场效应晶体管反型层中的电子迁移率模型. , 2011, 60(1): 017202. doi: 10.7498/aps.60.017202
[11]	王鑫华, 赵妙, 刘新宇, 蒲颜, 郑英奎, 魏珂. AlGaN/AlN/GaN高电子迁移率器件的电容电压特性的经验拟合. , 2011, 60(4): 047101. doi: 10.7498/aps.60.047101
[12]	王鑫华, 庞磊, 陈晓娟, 袁婷婷, 罗卫军, 郑英奎, 魏珂, 刘新宇. GaN HEMT栅边缘电容用于缺陷的研究. , 2011, 60(9): 097101. doi: 10.7498/aps.60.097101
[13]	李琦, 张波, 李肇基. 漂移区表面阶梯掺杂LDMOS的击穿电压模型. , 2008, 57(3): 1891-1896. doi: 10.7498/aps.57.1891
[14]	张鹤鸣, 崔晓英, 胡辉勇, 戴显英, 宣荣喜. 应变SiGe SOI量子阱沟道PMOSFET阈值电压模型研究. , 2007, 56(6): 3504-3508. doi: 10.7498/aps.56.3504
[15]	郭亮良, 冯倩, 郝跃, 杨燕. 高击穿电压的AlGaN/GaN FP-HEMT研究与分析. , 2007, 56(5): 2895-2899. doi: 10.7498/aps.56.2895
[16]	范隆, 郝跃. 辐射感生应力弛豫对AlmGa1－mN/GaN HEMT电学特性的影响. , 2007, 56(6): 3393-3399. doi: 10.7498/aps.56.3393
[17]	郝跃, 韩新伟, 张进城, 张金凤. AlGaN/GaN HEMT器件直流扫描电流崩塌机理及其物理模型. , 2006, 55(7): 3622-3628. doi: 10.7498/aps.55.3622
[18]	李东临, 曾一平. InP基HEMT器件中二维电子气浓度及分布与沟道层厚度关系的理论分析. , 2006, 55(7): 3677-3682. doi: 10.7498/aps.55.3677
[19]	马仲发, 庄奕琪, 杜磊, 包军林, 李伟华. 栅氧化层介质经时击穿的逾渗模型. , 2003, 52(8): 2046-2051. doi: 10.7498/aps.52.2046
[20]	吕红亮, 张义门, 张玉明. 4H-SiC pn结型二极管击穿特性中隧穿效应影响的模拟研究. , 2003, 52(10): 2541-2546. doi: 10.7498/aps.52.2541

计量

文章访问数: 8470
PDF下载量: 178
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板