色散和杂质对双参量非对称高斯势量子点量子比特的影响

乌云其木格; 韩超; 额尔敦朝鲁

doi:10.7498/aps.68.20190960

摘要

选取双参量非对称高斯势描写量子点中电子的受限效应, 采用LLP-Pekar变换变分法研究了色散和杂质对量子点量子比特性质的影响. 结果表明, 量子点量子比特中电子的概率密度随非对称高斯势阱宽的减小而呈现显著振荡, 并随介电常数比的增加而减小; 量子比特振动周期随高斯势阱深的增加或介电常数比的增加而减小; 退相干时间随介电常数比的增加或色散系数的增加而增大; 相位旋转品质因子随介电常数比的增加或色散系数的增加而增大.

关键词:

Abstract

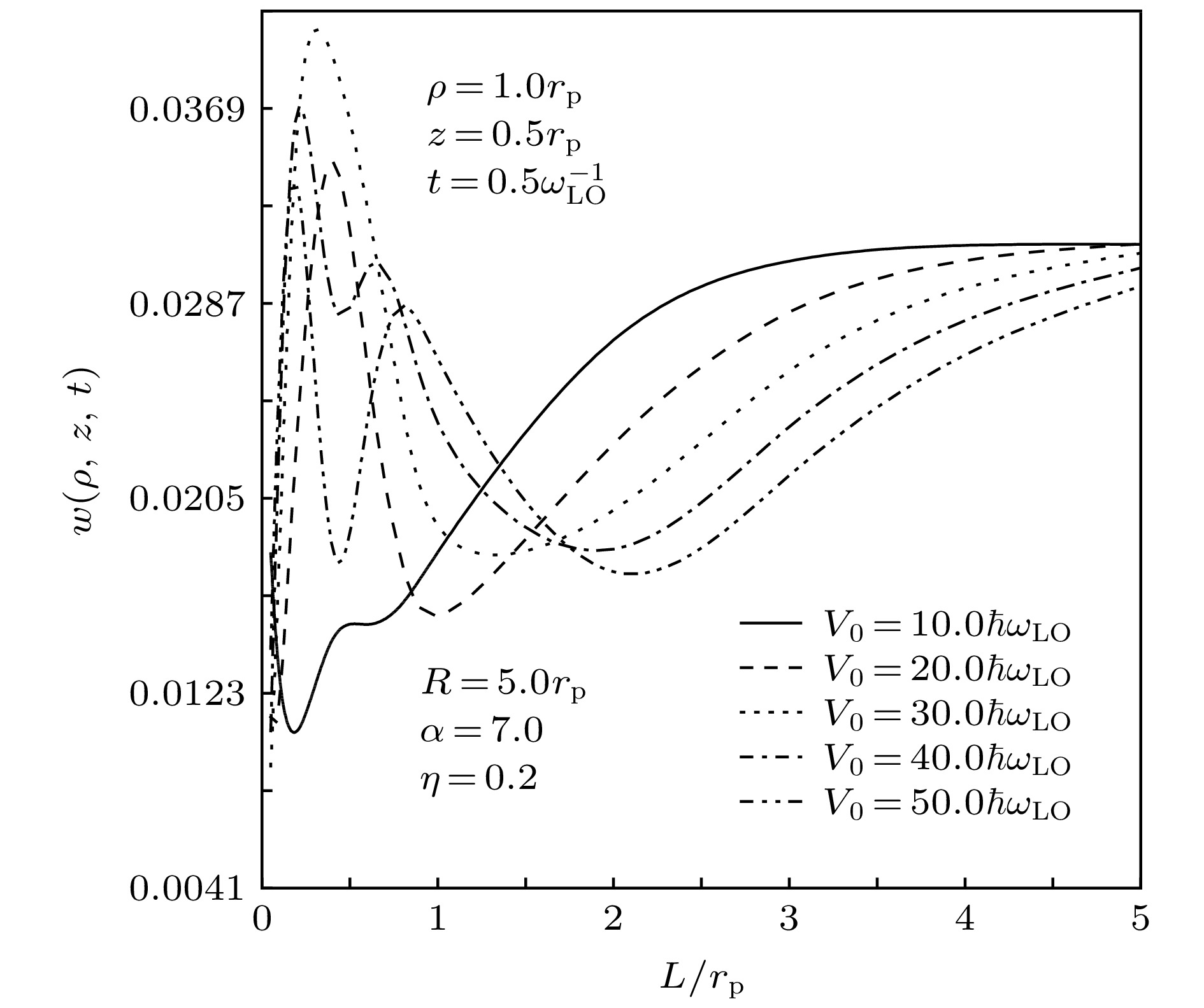
Selecting the double-parameter asymmetric Gaussian (AG) potential to describe the confinement effect of electrons in a quantum dot, the ground state and the first excited state energy eigenvalues and eigenfunctions of the three-body interaction system that are composed of the electrons, the impurity and the longitudinal optical phonon are derived by using the Lee-Low-Pines unitary transformation and the Pekar-type variational method, and the two-level structure required for a qubit is constructed. The influences of material parameters such as the dispersion coefficient, dielectric constant (DC) ratio, and electron-phonon coupling (EPC) constant on the probability density and the oscillation period of electron in the AG potential qubit are investigated. Based on the Fermi gold rule and the even-order approximation, the effects of the DC ratio, the dispersion coefficient and the EPC constant on the qubit decoherence are studied. And then the influences of the dispersion coefficient, the DC ratio and the EPC constant on the phase rotation manipulation of the qubit sphere are discussed. Numerical results show that the dispersion coefficient, the DC ratio and EPC constant of the medium have both advantages and disadvantages for the formation and information storage of qubits. The probability density of electrons in quantum dot qubits decreases with DC ratio increasing and exhibits significant oscillations as the well width of the AG potential decreases; the oscillation period of the qubit decreases with the well depth of the AG potential or the DC ratio increasing; the decoherence time increases with DC ratio or dispersion coefficient increasing; the phase rotation quality factor increases with DC ratio or dispersion coefficient increasing. Using the double-parameter AG potential to describe the confinement of electrons in quantum dot will better reflect the quantization properties of qubit. Increasing the dispersion coefficient or the DC ratio of the material is beneficial to not only the phase rotation manipulation of the qubit sphere, but also improving the coherence of the quantum dot qubit. The results of this paper can be used for reference in the experimental work on the constructing and manipulating of the quantum dot qubits.

Keywords:

作者及机构信息

1.
内蒙古民族大学物理与电子信息学院, 通辽　028043

2.
河北科技师范学院凝聚态物理研究所, 秦皇岛　066004

通信作者: 韩超, hc_206@126.com

基金项目: 国家自然科学基金(批准号: 51902085)和内蒙古自治区自然科学基金(批准号: 2019MS01011)资助的课题

Authors and contacts

Han Chao²,

1.
College of Physics and Electronic Information, Inner Mongolia University for Nationalities, Tongliao 028043, China

2.
Institute of Condensed Matter Physics, Hebei Normal University of Science and Technology, Qinhuangdao 066004, China

Corresponding author: Han Chao, hc_206@126.com

Funds: Supported by the National Natural Science Foundation of China (Grant No. 51902085) and the Nature Science Foundation of Inner Mongolia Autonomous Region, China (Grant No. 2019MS01011)

文章全文 : translate this paragraph

参考文献

[1]	Li S S, Long G L, Bai F S, Feng S L, Zheng H Z 2001 Proc. Nat. Acad. Sci. USA 98 11847 Google Scholar
[2]	Zhang X, Li H O, Wang K, Cao G, Xiao X, Guo G P 2018 Chin. Phys. B 27 020305 Google Scholar
[3]	Tang J, Xu X L 2018 Chin. Phys. B 27 027804 Google Scholar
[4]	Wang K, Li H O, Xiao M, Cao G, Guo G P 2018 Chin. Phys. B 27 090308 Google Scholar
[5]	Xiao W, Xiao J L 2016 Int. J. Theor. Phys. 55 2936 Google Scholar
[6]	Chen Y J, Xiao J L 2017 J. Low Temp. Phys. 186 241 Google Scholar
[7]	Sun Y, Ding Z H, Xiao J L 2017 J. Electron. Mater. 46 439 Google Scholar
[8]	Wang X Q, Xiao J L 2017 Iran. J. Sci. Technol. Trans. Sci. 41 273 Google Scholar
[9]	Khordad R, Goudarzi S, Bahramiyan H 2016 Indian J. Phys. 90 659 Google Scholar
[10]	Xie W F 2003 Solid State Commun. 127 401 Google Scholar
[11]	谷娟, 梁九卿 2005 54 5335 Google Scholar Gu J, Liang J J 2005 Acta Phys. Sin. 54 5335 Google Scholar
[12]	Xiao W, Qi B, Xiao J L 2015 J. Low Temp. Phys. 179 166 Google Scholar
[13]	Xiao J L 2016 Int. J. Theor. Phys. 55 147 Google Scholar
[14]	Wuyunqimuge, Xin W, Wang G S, Eerdunchaolu 2018 J. Low Temp. Phys. 193 48 Google Scholar
[15]	Bai X F, Zhao Y W, Xin W, Yin H W, Eerdunchaolu 2019 Opt. Quant. Electron. 51 114 Google Scholar
[16]	Lee T D, Low F M, Pines D 1953 Phys. Rev. 90 297 Google Scholar
[17]	Pekar S I, Deigen M F 1948 Zh. Eksp. Teor. Fiz. 18 481
[18]	Landau L D, Lifshitz E M 1987 Quantum Mechanics: Nonrelativistic Theory (London: Pergamen) p532
[19]	Sun Y, Ding Z H, Xiao J L 2014 J. Low Temp. Phys. 177 151 Google Scholar
[20]	Liang Z H, Cai C Y, Xiao J L 2019 Int. J. Theor. Phys. 58 2320 Google Scholar

施引文献

图 1 能隙$\Delta E$在非对称高斯势不同阱深${V_0}$下随阱宽L的变化

Fig. 1. Energy gap $\Delta E$ versus the well width L under different the well depth ${V_0}$ of the asymmetric Gaussian (AG) potential.

下载: 全尺寸图片幻灯片

图 10 相位品质因子Q在不同色散系数$\varsigma $下随高斯势阱宽L的变化

Fig. 10. Quality factors of phase rotation Q as a function of the well width L of the AG potential at different dispersion coefficient $\varsigma $.

下载: 全尺寸图片幻灯片

图 2 能隙$\Delta E$在不同介电常数比$\eta $下随高斯势阱宽L的变化

Fig. 2. Energy gap $\Delta E$ versus the well width L of the AG potential under different the dielectric constant (DC) ratio $\eta $

下载: 全尺寸图片幻灯片

图 3 概率密度w在高斯势不同阱深${V_0}$下随阱宽L的变化

Fig. 3. Probability density w versus the well width L under different the well depth ${V_0}$ of the AG potential.

下载: 全尺寸图片幻灯片

图 4 概率密度w在不同介电常数比$\eta $下随高斯势不同阱宽L的变化

Fig. 4. Probability density w as a function of the well width L of the AG potential at different the DC ratio $\eta $.

下载: 全尺寸图片幻灯片

图 5 振动周期T在高斯势不同阱深${V_0}$下随其阱宽L的变化

Fig. 5. Variations of oscillation period T as a function of the well width L at different well width L of the AG potential.

下载: 全尺寸图片幻灯片

图 6 振动周期T在不同介电常数比$\eta $下随高斯势阱宽L的变化

Fig. 6. Variations of oscillation period T as a function of the well width L of the AG potential at different the DC ratio $\eta $

下载: 全尺寸图片幻灯片

图 7 自发辐射率${\tau ^{ - 1}}$在不同介电常数比$\eta $下随高斯势阱宽L的变化

Fig. 7. Spontaneous emission rate ${\tau ^{ - 1}}$ as a function of the well width L of the AG potential at different the DC ratio $\eta $

下载: 全尺寸图片幻灯片

图 8 自发辐射率${\tau ^{ - 1}}$在不同色散系数$\varsigma $下随高斯势阱宽L的变化

Fig. 8. Spontaneous emission rate ${\tau ^{ - 1}}$ as a function of the well width L of the AG potential at different dispersion coefficient $\varsigma $.

下载: 全尺寸图片幻灯片

图 9 相位品质因子Q在不同介电常数比$\eta $下随高斯势阱宽L的变化

Fig. 9. Quality factors of phase rotation Q as a function of the well width L of the AG potential at different the DC ratio $\eta $.

下载: 全尺寸图片幻灯片

map

[1]	Li S S, Long G L, Bai F S, Feng S L, Zheng H Z 2001 Proc. Nat. Acad. Sci. USA 98 11847 Google Scholar
[2]	Zhang X, Li H O, Wang K, Cao G, Xiao X, Guo G P 2018 Chin. Phys. B 27 020305 Google Scholar
[3]	Tang J, Xu X L 2018 Chin. Phys. B 27 027804 Google Scholar
[4]	Wang K, Li H O, Xiao M, Cao G, Guo G P 2018 Chin. Phys. B 27 090308 Google Scholar
[5]	Xiao W, Xiao J L 2016 Int. J. Theor. Phys. 55 2936 Google Scholar
[6]	Chen Y J, Xiao J L 2017 J. Low Temp. Phys. 186 241 Google Scholar
[7]	Sun Y, Ding Z H, Xiao J L 2017 J. Electron. Mater. 46 439 Google Scholar
[8]	Wang X Q, Xiao J L 2017 Iran. J. Sci. Technol. Trans. Sci. 41 273 Google Scholar
[9]	Khordad R, Goudarzi S, Bahramiyan H 2016 Indian J. Phys. 90 659 Google Scholar
[10]	Xie W F 2003 Solid State Commun. 127 401 Google Scholar
[11]	谷娟, 梁九卿 2005 54 5335 Google Scholar Gu J, Liang J J 2005 Acta Phys. Sin. 54 5335 Google Scholar
[12]	Xiao W, Qi B, Xiao J L 2015 J. Low Temp. Phys. 179 166 Google Scholar
[13]	Xiao J L 2016 Int. J. Theor. Phys. 55 147 Google Scholar
[14]	Wuyunqimuge, Xin W, Wang G S, Eerdunchaolu 2018 J. Low Temp. Phys. 193 48 Google Scholar
[15]	Bai X F, Zhao Y W, Xin W, Yin H W, Eerdunchaolu 2019 Opt. Quant. Electron. 51 114 Google Scholar
[16]	Lee T D, Low F M, Pines D 1953 Phys. Rev. 90 297 Google Scholar
[17]	Pekar S I, Deigen M F 1948 Zh. Eksp. Teor. Fiz. 18 481
[18]	Landau L D, Lifshitz E M 1987 Quantum Mechanics: Nonrelativistic Theory (London: Pergamen) p532
[19]	Sun Y, Ding Z H, Xiao J L 2014 J. Low Temp. Phys. 177 151 Google Scholar
[20]	Liang Z H, Cai C Y, Xiao J L 2019 Int. J. Theor. Phys. 58 2320 Google Scholar

[1]	吴宇恺, 段路明. 离子阱量子计算规模化的研究进展. , 2023, 72(23): 230302. doi: 10.7498/aps.72.20231128
[2]	熊凡, 陈永聪, 敖平. 热噪声环境下偶极场驱动的量子比特动力学. , 2023, 72(17): 170302. doi: 10.7498/aps.72.20230625
[3]	高飞, 冯琦, 王霆, 张建军. 硅(001)图形衬底上锗硅纳米线的定位生长. , 2020, 69(2): 028102. doi: 10.7498/aps.69.20191407
[4]	白旭芳, 陈磊, 额尔敦朝鲁. 电磁场中施主中心量子点内磁极化子态寿命与qubit退相干. , 2020, 69(14): 147802. doi: 10.7498/aps.69.20200242
[5]	廖庆洪, 邓伟灿, 文健, 周南润, 刘念华. 纳米机械谐振器耦合量子比特非厄米哈密顿量诱导的声子阻塞. , 2019, 68(11): 114203. doi: 10.7498/aps.68.20182263
[6]	宋洪胜, 庄桥, 刘桂媛, 秦希峰, 程传福. 菲涅耳深区散斑强度统计特性及演化. , 2014, 63(9): 094201. doi: 10.7498/aps.63.094201
[7]	宋庆庆, 王新波, 崔万照, 王志宇, 冉立新. 多载波微放电中二次电子横向扩散的概率分析. , 2014, 63(22): 220205. doi: 10.7498/aps.63.220205
[8]	戈阳祯, 米建春. 圆柱热尾流中温度的概率密度函数. , 2013, 62(2): 024702. doi: 10.7498/aps.62.024702
[9]	谢文贤, 蔡力, 岳晓乐, 雷佑铭, 徐伟. 两种群随机动力系统的信息熵和动力学研究. , 2012, 61(17): 170509. doi: 10.7498/aps.61.170509
[10]	赵翠兰, 丛银川. 球壳量子点中极化子和量子比特的声子效应. , 2012, 61(18): 186301. doi: 10.7498/aps.61.186301
[11]	宋洪胜, 程传福, 滕树云, 刘曼, 刘桂媛, 张宁玉. 参考光干涉提取复振幅的散斑统计函数的实验研究. , 2009, 58(11): 7654-7661. doi: 10.7498/aps.58.7654
[12]	姜福仕, 赵翠兰. 量子环中量子比特的声子效应. , 2009, 58(10): 6786-6790. doi: 10.7498/aps.58.6786
[13]	刘云飞, 肖景林. LA声子对激子qubit纯退相干的影响. , 2008, 57(6): 3324-3327. doi: 10.7498/aps.57.3324
[14]	高宽云, 赵翠兰. 量子环中量子比特的性质. , 2008, 57(7): 4446-4449. doi: 10.7498/aps.57.4446
[15]	陈英杰, 肖景林. 抛物线性限制势二能级系统量子点量子比特的温度效应. , 2008, 57(11): 6758-6762. doi: 10.7498/aps.57.6758
[16]	尹辑文, 肖景林, 于毅夫, 王子武. 库仑势对抛物量子点量子比特消相干的影响. , 2008, 57(5): 2695-2698. doi: 10.7498/aps.57.2695
[17]	董庆瑞. 磁场方向调制的InAs量子点分子量子比特. , 2007, 56(9): 5436-5440. doi: 10.7498/aps.56.5436
[18]	李艳玲, 冯健, 孟祥国, 梁宝龙. 量子比特的普适远程翻转和克隆. , 2007, 56(10): 5591-5596. doi: 10.7498/aps.56.5591
[19]	王子武, 肖景林. 抛物线性限制势量子点量子比特及其光学声子效应. , 2007, 56(2): 678-682. doi: 10.7498/aps.56.678
[20]	胡学宁, 李新奇. 量子点接触对单电子量子态的量子测量. , 2006, 55(7): 3259-3264. doi: 10.7498/aps.55.3259

计量

文章访问数: 6046
PDF下载量: 39
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

色散和杂质对双参量非对称高斯势量子点量子比特的影响