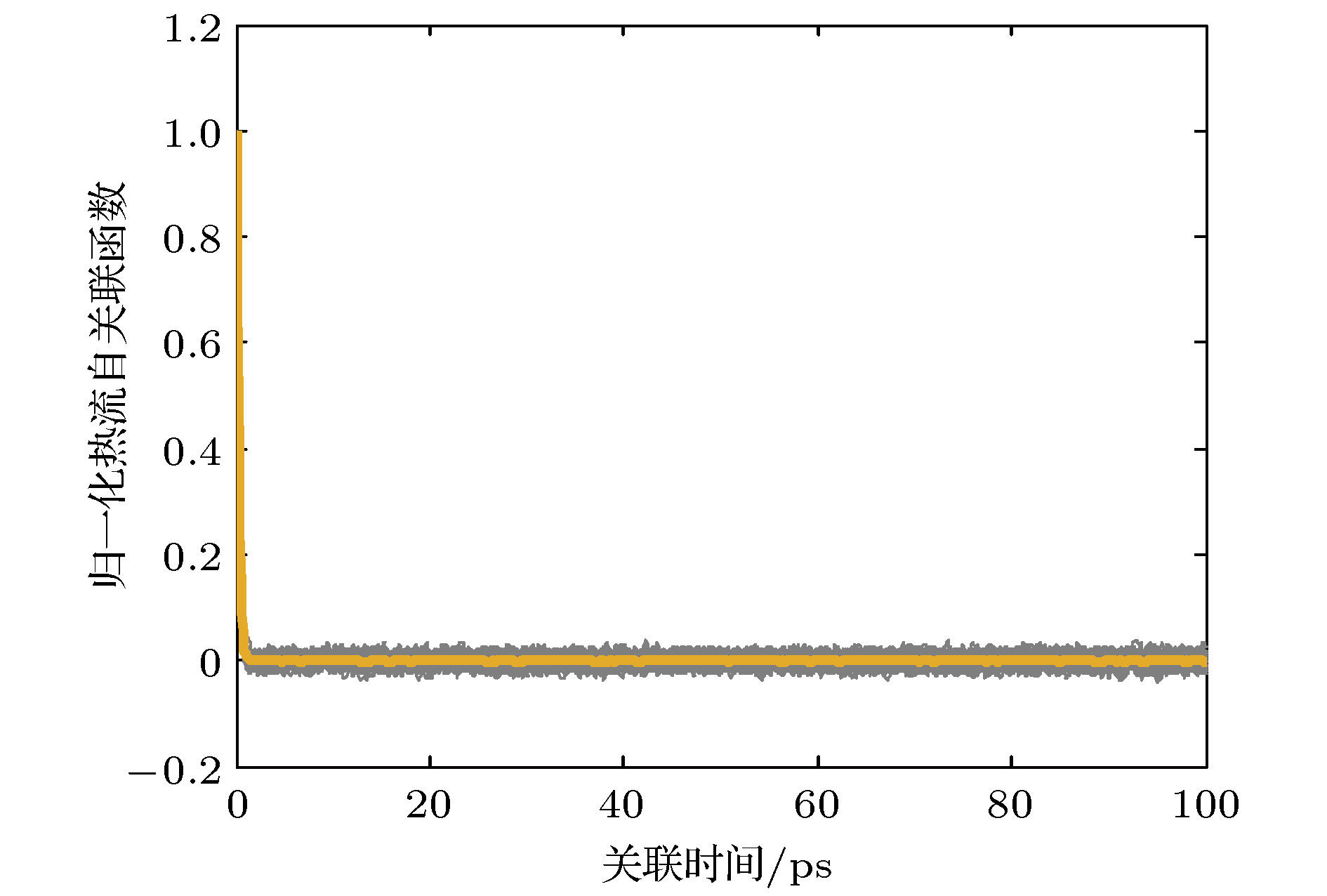
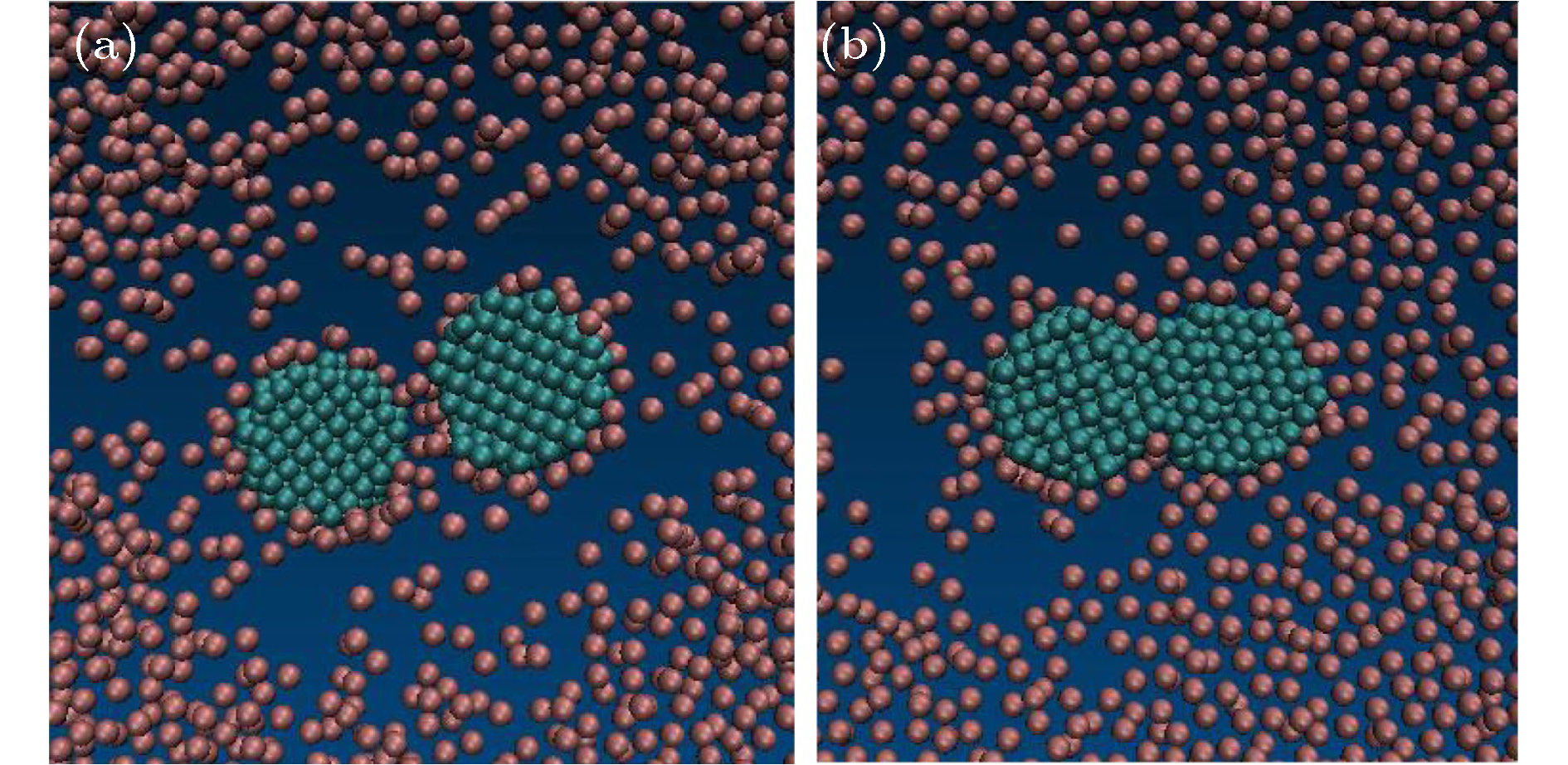
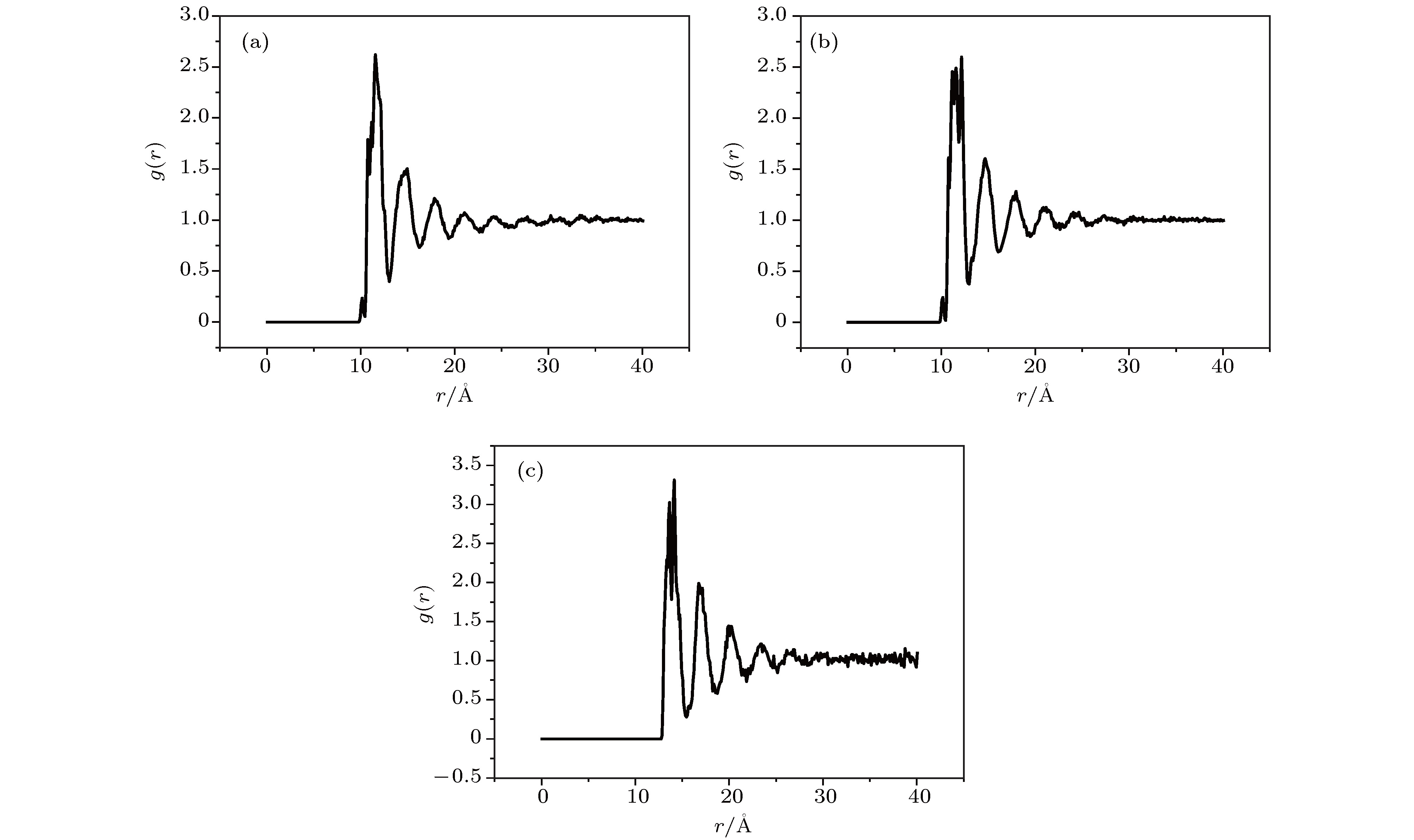
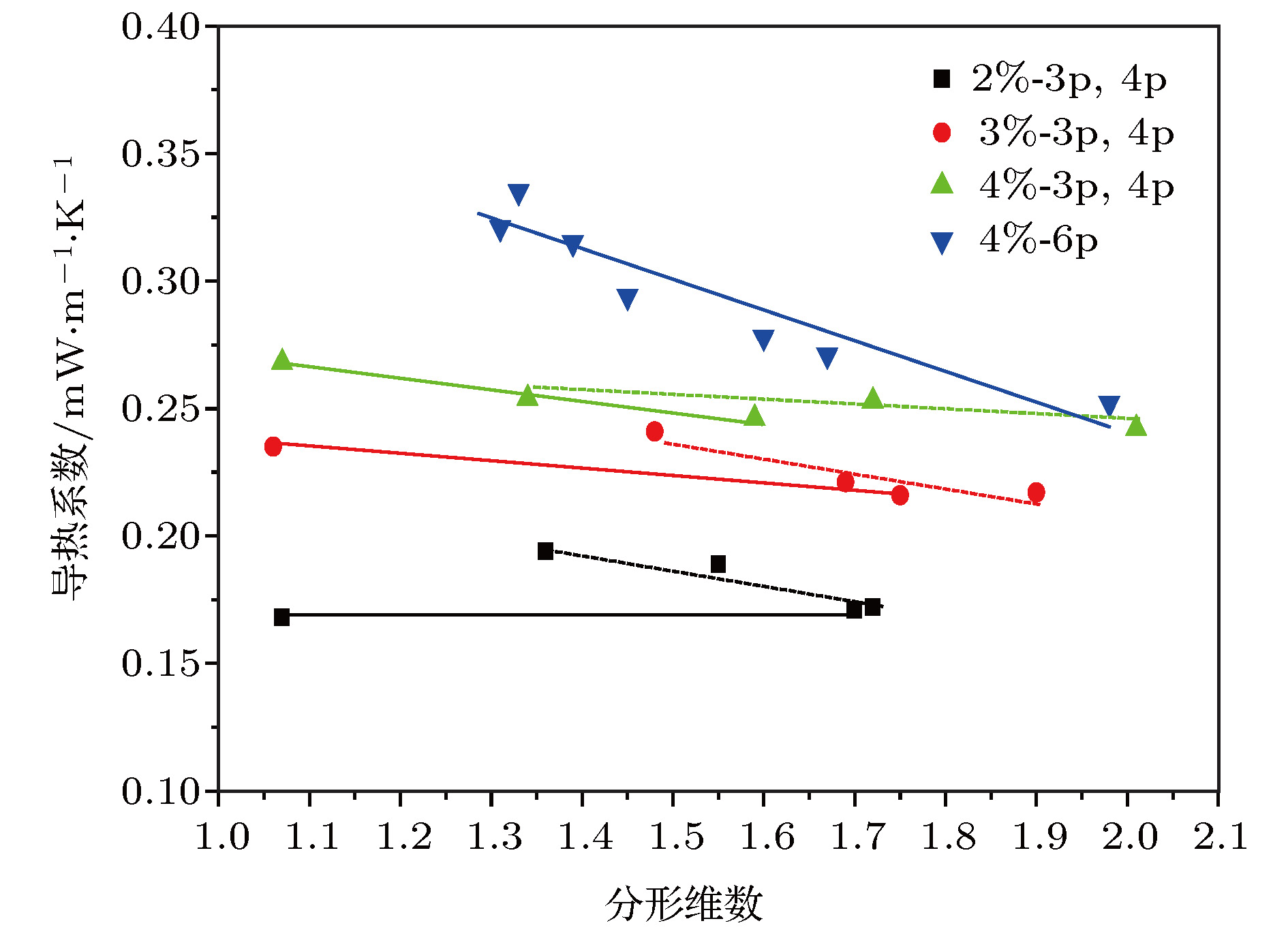
-
纳米流体中悬浮的纳米颗粒可以增强其导热性能已经得到广泛认可, 然而纳米流体颗粒增强传热的机理目前尚不清楚. 研究表明, 纳米颗粒的聚集是纳米流体导热系数增大的重要机制, 而且纳米颗粒聚集的形态对纳米流体的导热系数有重要影响, 但是目前的导热系数模型大多是建立在Maxwell有效介质理论的“静态”和“均匀分散”假设基础上. 本文用平衡分子动力学模拟Cu-Ar纳米流体, 采用Green-Kubo公式计算导热系数, 采用Schmidt-Ott关系式计算不同聚集形态下的分形维数. 对比导热系数与分形维数可以发现: 在相同体积分数下, 较低的分形维数会有更高的导热系数, 分析了分形维数与导热系数的定量关系. 此外, 通过径向分布函数可以看出纳米颗粒紧密聚集与松散聚集的差异, 基液分子在纳米颗粒附近的纳米薄层中处于动态平衡状态. 研究结果有助于理解纳米颗粒聚集形态对导热系数的影响机理.The great interest of many researchers has been aroused in recent two decades due to the great heat transfer enhancement of nanofluid as a heat transfer medium. The reason why the nanofluid can enhance heat transfer is that a number of nanoparticles are suspended in the carry fluid. Most of researchers believe that the microconvection induced by Brownian motion of nanoparticle, the nanolayer around the nanoparticle, the aggregation of nanoparticles and near-field radiation are the underlying mechanisms for heat transfer enhancement by nanofluid. However, contradictories and inconsistencies among experimental results, theoretical results and numerical results are existent commonly because the mechanism of heat transportation by nanoparticles remains unclear so far. Quite a few researches have proven that the aggregation of nanoparticles is one of the important mechanisms for elevating the effective thermal conductivity (ETC) of nanofluid. However, the aggregation morphology (AM) of nanoparticles evaluated by fractal dimension (FD) will greatly influence the thermal conductivity of nanofluid. Unfortunately, all of the existing ETC models are based on the effective medium theory under the assumption of " static state” and " homo-dispersion”. In the present work, equilibrium molecular dynamics (EMD) simulations are carried out to calculate the thermal conductivity of Cu-Ar nanofluid via Green-Kubo formula. In existing researches, fractal dimensions of the aggregations with various morphologies are obtained by Schmidt-Ott equation. Comparisons between the ETC and FD of the nanofluid with same volume fraction show that lower FD can possess greater ETC. It is the first time that the quantitative relationship between ETC and FD has been analyzed. In addition, the difference between loose and compact aggregation can be read out of the pair correlation function near nanoparticles. And the solvent atoms in nanolayer are mobilized and dynamically balanced. The results obtained in the present research are conducible to understanding the influence of AM of nanoparticles on the ETC of nanofluid.
[1] Choi S U S 1995 ASME International Mechanical Engineering Congress and Exposition San Francisco, California, November 12–17, 1995 p99
[2] Choi S U S, Zhang Z G, Yu W 2001 Appl. Phys. Lett. 79 52
[3] Maxwell J C 1873 A Treatise on Electricity and Magnetism (Oxford: Clarendon Press) p68
[4] Hamilton R L, Crosser O K 1962 Ind. Eng. Chem. Fundam. 1 187
Google Scholar
[5] Xuan Y M, Li Q, Zhang X, Fujii M 2006 J. Appl. Phys. 100 043507
Google Scholar
[6] 王补宣, 颜文盛 2007 自然科学进展 17 984
Google Scholar
Wang B X, Yan W S 2007 Prog. Nat. Sci. 17 984
Google Scholar
[7] Prasher R, Phelan P E, Bhattacharya P 2006 Nano Lett. 6 1529
Google Scholar
[8] Lin J Z, Xia Y, Ku X K 2016 Int. J. Heat Mass Transf. 93 57
Google Scholar
[9] 李屹同, 沈谅平, 王浩, 汪汉斌 2013 62 124401
Google Scholar
Li Y T, Shen L P, Wang H, Wang H B 2013 Acta Phys. Sin. 62 124401
Google Scholar
[10] Xue L, Keblinski P, Phillpot S R, Choi S U S, Eastman J A 2004 Int. J. Heat Mass Transf. 47 4277
Google Scholar
[11] Keblinski P, Prasher R, Eapen J 2008 J. Nanopart. Res. 10 1089
Google Scholar
[12] Hu Y Q, Zhao Y P, Yu T X 2008 Int. J. Nonlin. Sci. Num. Sim. 9 315
[13] Hu Y Q, Zhao Y P, Yu T X 2008 Mater. Sci. Eng. A 483−484 611
[14] Sedighi M, Mohebbi A 2014 J. Mol. Liq. 197 14
Google Scholar
[15] Hong J, Kim D 2012 Thermochim. Acta 542 28
Google Scholar
[16] Philip J, Shima P D, Raj B 2007 Appl. Phys. Lett. 91 203103
Google Scholar
[17] Wu C, Cho T J, Xu J, Lee D G, Yang B, Zachariah M R 2010 Phys. Rev. E 81 011406
Google Scholar
[18] Thaseem T, Christopher J H 2011 J. Nanopart. Res. 13 7099
Google Scholar
[19] Xuan Y M, Li Q, Hu W 2003 AIChE J. 49 1038
Google Scholar
[20] Feng Y, Yu B, Xu P, Zou M 2007 J. Phys. D 40 3164
Google Scholar
[21] Wang B X, Zhou L P, Peng X F 2003 Int. J. Heat Mass Transf. 46 2665
Google Scholar
[22] Evans W, Prasher R, Fish J, Meakin P, Phelan P, Keblinski P 2008 Int. J. Heat Mass Transf. 51 1431
Google Scholar
[23] Prasher R, Evans W, Meakin P, Fish J, Phelan P, Keblinski P 2006 Appl. Phys. Lett. 89 143119
Google Scholar
[24] Xiao B Q, Yang Y, Chen L X 2013 Powder Technol. 239 409
Google Scholar
[25] Cai J C, Hua X Y, Xiao B Q, Zhou Y F, Wei W 2017 Int. J. Heat Mass Transf. 105 623
Google Scholar
[26] Atmuri A K, Henson M A, Bhatia S R 2013 Colloids and Surfaces A 436 325
Google Scholar
[27] Gaganpreet S S 2012 Appl. Nanosci. 2 325
Google Scholar
[28] Kang H, Zhang Y, Yang M, Li L 2012 J. Nanotechnol. Eng. Med. 3 021001
Google Scholar
[29] Duan F, Kwek D, Crivol A 2011 Nanoscale Res. Lett. 6 248
Google Scholar
[30] Lin J Z, Xia Y, Ku X K 2014 J. Heat Transf. T. ASME 136 111701
Google Scholar
[31] Kang H, Zhang Y, Yang M 2011 Appl. Phys. A 103 1001
[32] Lee S L, Saidur R, Sabri M F M, Min T K 2015 Num. Heat Transf. A 8 432
[33] 李凌, 郭丽, 杨茉, 卢玫, 余敏 2010 工程热 31 1933
Li L, Guo L, Yang M, Lu M, Yu M 2010 J. Eng. Thermophys. 31 1933
[34] Gross D, Hauger W, Schröder J, Wall W A 2014 Engineering Mechanics (Berlin: Springer) p104
[35] Daw M S, Baskes M I 1984 Phys. Rev. B 29 6443
Google Scholar
[36] Jones R E, Mandadapu K K 2012 J. Chem. Phys. 136 154102
Google Scholar
[37] McQuarrie D A 2000 Statistical Mechanics (Sausalito: University Science Books) p55
[38] Jullien R, Botet R 1987 Aggregation and Fractal Aggregates (Singapore: World Scientific Publishing Co.) p138
[39] Schmidt-Ott A, Wüstenberg J 1995 J. Aerosol. Sci. 26 S923
Google Scholar
[40] Agresti F, Barison S, Battiston S 2013 Nanotechnology 24 365601
Google Scholar
[41] Rapaport D C 2004 The Art of Molecular Dynamics Simulations (New York: Cambridge University Press) p35
[42] Allen M P, Tildesley D J 1987 Computer Simulation of Liquids (New York: Oxford Press) p236
[43] Sarkar S, Selvam R P 2007 J. Appl. Phys. 102 074302
Google Scholar
[44] Fang X P, Xuan Y M, Li Q 2009 Appl. Phys. Lett. 95 203108
Google Scholar
[45] Gharagozloo P E, Goodson K E 2010 J. Appl. Phys. 108 074309
Google Scholar
[46] Sadeghi R, Haghshenasfard M, Etemad S, Keshavarzi E 2016 Int. Com. Heat Mass Transf. 72 57
Google Scholar
[47] Xie H, Wang J, Xi T, Liu Y, Ai F 2002 J. Appl. Phys. 91 4568
Google Scholar
-
图 7 铜纳米颗粒周围的Ar原子初始分布(a)和2000步时的分布(b)
Fig. 7. Initial distribution (a) and at 2000th time-step (b) of Ar-atoms around Cu-nanoparticle.
图 8 纳米颗粒聚集 (a)紧密聚集; (b)松散聚集
Fig. 8. Aggregation of nanoparticles: (a) Compact aggregation; (b) loose aggregation.
图 9 对分布函数 (a)液氩体系; (b) Cu-Ar纳米流体
Fig. 9. Pair correlation function of fluid Ar (a) and nanofluid Cu/Ar (b).
图 10 两颗纳米颗粒下纳米流体的对分布函数 (a)分散; (b)松聚集; (c)紧聚集
Fig. 10. Pair correlation function of nanofluid with two nanoparticles: (a) Dispersed nanoparticle; (b) loose aggregation; (c) compact aggregation.
图 11 分形维数与导热系数对比(3颗为实线, 4颗为虚线)
Fig. 11. Thermal conductivity at various fractal dimensions (3-p solid line, 4-p dash line).
表 1 势函数参数
Table 1. Parameters of potential functions.
原子 $\varepsilon $/10–2 eV $\sigma $/nm Ar-Ar 1.0437 0.3405 Cu-Cu 41.0156 0.2338 Ar-Cu 6.5429 0.2871 
下载: 导出CSV
表 2 3—4颗纳米颗粒下的导热系数与分形维数
Table 2. Thermal conductivity for various aggregation morphologies with 3−4 particles.
体积分数 2% 3% 4% 颗粒数目 3 4 3 4 3 4 聚集形态 分形维数 1.07 1.72 1.36 1.55 1.70 1.06 1.75 1.48 1.69 1.90 1.06 1.72 1.34 1.59 2.01 导热系数/W·m–1·K–1) 0.168 0.172 0.194 0.189 0.171 0.235 0.216 0.241 0.221 0.217 0.268 0.253 0.254 0.246 0.242
下载: 导出CSV
表 3 6颗粒4%体积分数时导热系数与分形维数
Table 3. Thermal conductivity for various aggregation morphologies with 6 particles.
聚集方式 分形维数 1.31 1.33 1.39 1.45 1.60 1.67 1.98 导热系数/W·m–1·K–1) 0.321 0.335 0.315 0.294 0.278 0.271 0.252
下载: 导出CSV
-
[1] Choi S U S 1995 ASME International Mechanical Engineering Congress and Exposition San Francisco, California, November 12–17, 1995 p99
[2] Choi S U S, Zhang Z G, Yu W 2001 Appl. Phys. Lett. 79 52
[3] Maxwell J C 1873 A Treatise on Electricity and Magnetism (Oxford: Clarendon Press) p68
[4] Hamilton R L, Crosser O K 1962 Ind. Eng. Chem. Fundam. 1 187
Google Scholar
[5] Xuan Y M, Li Q, Zhang X, Fujii M 2006 J. Appl. Phys. 100 043507
Google Scholar
[6] 王补宣, 颜文盛 2007 自然科学进展 17 984
Google Scholar
Wang B X, Yan W S 2007 Prog. Nat. Sci. 17 984
Google Scholar
[7] Prasher R, Phelan P E, Bhattacharya P 2006 Nano Lett. 6 1529
Google Scholar
[8] Lin J Z, Xia Y, Ku X K 2016 Int. J. Heat Mass Transf. 93 57
Google Scholar
[9] 李屹同, 沈谅平, 王浩, 汪汉斌 2013 62 124401
Google Scholar
Li Y T, Shen L P, Wang H, Wang H B 2013 Acta Phys. Sin. 62 124401
Google Scholar
[10] Xue L, Keblinski P, Phillpot S R, Choi S U S, Eastman J A 2004 Int. J. Heat Mass Transf. 47 4277
Google Scholar
[11] Keblinski P, Prasher R, Eapen J 2008 J. Nanopart. Res. 10 1089
Google Scholar
[12] Hu Y Q, Zhao Y P, Yu T X 2008 Int. J. Nonlin. Sci. Num. Sim. 9 315
[13] Hu Y Q, Zhao Y P, Yu T X 2008 Mater. Sci. Eng. A 483−484 611
[14] Sedighi M, Mohebbi A 2014 J. Mol. Liq. 197 14
Google Scholar
[15] Hong J, Kim D 2012 Thermochim. Acta 542 28
Google Scholar
[16] Philip J, Shima P D, Raj B 2007 Appl. Phys. Lett. 91 203103
Google Scholar
[17] Wu C, Cho T J, Xu J, Lee D G, Yang B, Zachariah M R 2010 Phys. Rev. E 81 011406
Google Scholar
[18] Thaseem T, Christopher J H 2011 J. Nanopart. Res. 13 7099
Google Scholar
[19] Xuan Y M, Li Q, Hu W 2003 AIChE J. 49 1038
Google Scholar
[20] Feng Y, Yu B, Xu P, Zou M 2007 J. Phys. D 40 3164
Google Scholar
[21] Wang B X, Zhou L P, Peng X F 2003 Int. J. Heat Mass Transf. 46 2665
Google Scholar
[22] Evans W, Prasher R, Fish J, Meakin P, Phelan P, Keblinski P 2008 Int. J. Heat Mass Transf. 51 1431
Google Scholar
[23] Prasher R, Evans W, Meakin P, Fish J, Phelan P, Keblinski P 2006 Appl. Phys. Lett. 89 143119
Google Scholar
[24] Xiao B Q, Yang Y, Chen L X 2013 Powder Technol. 239 409
Google Scholar
[25] Cai J C, Hua X Y, Xiao B Q, Zhou Y F, Wei W 2017 Int. J. Heat Mass Transf. 105 623
Google Scholar
[26] Atmuri A K, Henson M A, Bhatia S R 2013 Colloids and Surfaces A 436 325
Google Scholar
[27] Gaganpreet S S 2012 Appl. Nanosci. 2 325
Google Scholar
[28] Kang H, Zhang Y, Yang M, Li L 2012 J. Nanotechnol. Eng. Med. 3 021001
Google Scholar
[29] Duan F, Kwek D, Crivol A 2011 Nanoscale Res. Lett. 6 248
Google Scholar
[30] Lin J Z, Xia Y, Ku X K 2014 J. Heat Transf. T. ASME 136 111701
Google Scholar
[31] Kang H, Zhang Y, Yang M 2011 Appl. Phys. A 103 1001
[32] Lee S L, Saidur R, Sabri M F M, Min T K 2015 Num. Heat Transf. A 8 432
[33] 李凌, 郭丽, 杨茉, 卢玫, 余敏 2010 工程热 31 1933
Li L, Guo L, Yang M, Lu M, Yu M 2010 J. Eng. Thermophys. 31 1933
[34] Gross D, Hauger W, Schröder J, Wall W A 2014 Engineering Mechanics (Berlin: Springer) p104
[35] Daw M S, Baskes M I 1984 Phys. Rev. B 29 6443
Google Scholar
[36] Jones R E, Mandadapu K K 2012 J. Chem. Phys. 136 154102
Google Scholar
[37] McQuarrie D A 2000 Statistical Mechanics (Sausalito: University Science Books) p55
[38] Jullien R, Botet R 1987 Aggregation and Fractal Aggregates (Singapore: World Scientific Publishing Co.) p138
[39] Schmidt-Ott A, Wüstenberg J 1995 J. Aerosol. Sci. 26 S923
Google Scholar
[40] Agresti F, Barison S, Battiston S 2013 Nanotechnology 24 365601
Google Scholar
[41] Rapaport D C 2004 The Art of Molecular Dynamics Simulations (New York: Cambridge University Press) p35
[42] Allen M P, Tildesley D J 1987 Computer Simulation of Liquids (New York: Oxford Press) p236
[43] Sarkar S, Selvam R P 2007 J. Appl. Phys. 102 074302
Google Scholar
[44] Fang X P, Xuan Y M, Li Q 2009 Appl. Phys. Lett. 95 203108
Google Scholar
[45] Gharagozloo P E, Goodson K E 2010 J. Appl. Phys. 108 074309
Google Scholar
[46] Sadeghi R, Haghshenasfard M, Etemad S, Keshavarzi E 2016 Int. Com. Heat Mass Transf. 72 57
Google Scholar
[47] Xie H, Wang J, Xi T, Liu Y, Ai F 2002 J. Appl. Phys. 91 4568
Google Scholar
下载:
计量
- 文章访问数: 12256
- PDF下载量: 155
- 被引次数: 0
