-
High-altitude nuclear explosions can inject large amounts of relativistic electrons into the inner magnetosphere, resulting in the formation of artificial radiation belts. These high-energy electrons pose a potential threat to spacecraft due to their long-term stability and influence on space weather. The investigation of the formation and evolution of artificial radiation belts is of great significance for the safety of spacecraft and human space activities. In this study, the comprehensive inner magnetosphere-ionosphere(CIMI) model is used to simulate the transition of electrons from a locally concentrated distribution to an azimuthally uniform distribution, which reveals the spiral encircling, azimuthal expansion, and diffusion behaviors exhibited by the electron cloud during the formation of artificial radiation belts. The CIMI model is a four-dimensional model based on the Fokker-Planck equation. It simulates the evolution of particles across four degrees of freedom: radial, azimuthal, energy, and equatorial pitch angle. Unlike previous studies that mainly focus on the long-term evolution of artificial radiation belts already reaching azimuthal uniformity, this work specifically ascertains the azimuthal evolution process of the injected electrons and how they form the artificial radiation belts. Numerical simulations are conducted on the captured nuclear explosion electrons initially concentrated at $L=1.1\sim2.2 $ and covering approximately one time zone azimuthally. The results show that the injected electrons primarily evolve into an azimuthally uniform distribution through a spiral encircling process, with diffusion playing a smaller role. In this process, the electrons undergo eastward drift, and those at higher altitudes exhibit faster drift velocities. The velocity shear leads to the formation of a helical structure around the Earth. Additionally, the formation of this spiral structure is accompanied by azimuthal expansion, driven mainly by energy and pitch angle dispersion during the drift. Electrons with different energy values and equatorial pitch angles exhibit varying drift speeds, contributing to the azimuthal expansion of electron clusters during the drift. The expansion process can fill the gaps in the helical structure. Ultimately, the electron distribution achieves azimuthal uniformity through energy-pitch angle diffusion.
-
Keywords:
- high-altitude nuclear explosions /
- artificial radiation belt /
- Fokker-Planck equations /
- electrons diffusion
-
图 1 注入电子的初始分布. (a)初始注入电子在不同L值上的总通量分布; (b)初始注入电子中不同能量的占比
Figure 1. Initial distribution of the injected electrons: (a) Total flux distribution of initially injected electrons at different L values; (b) Proportions of different energies of the initially injected electrons
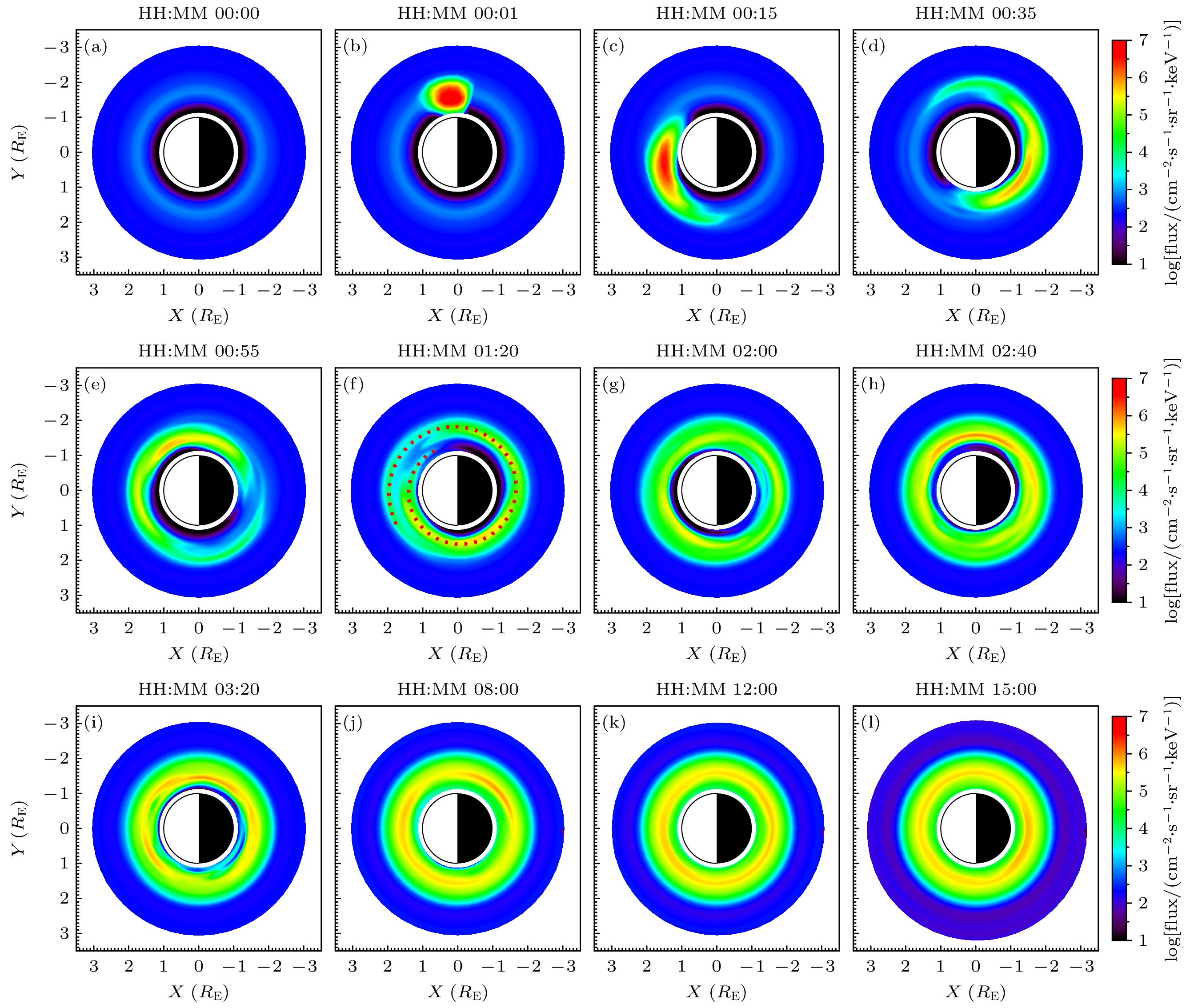
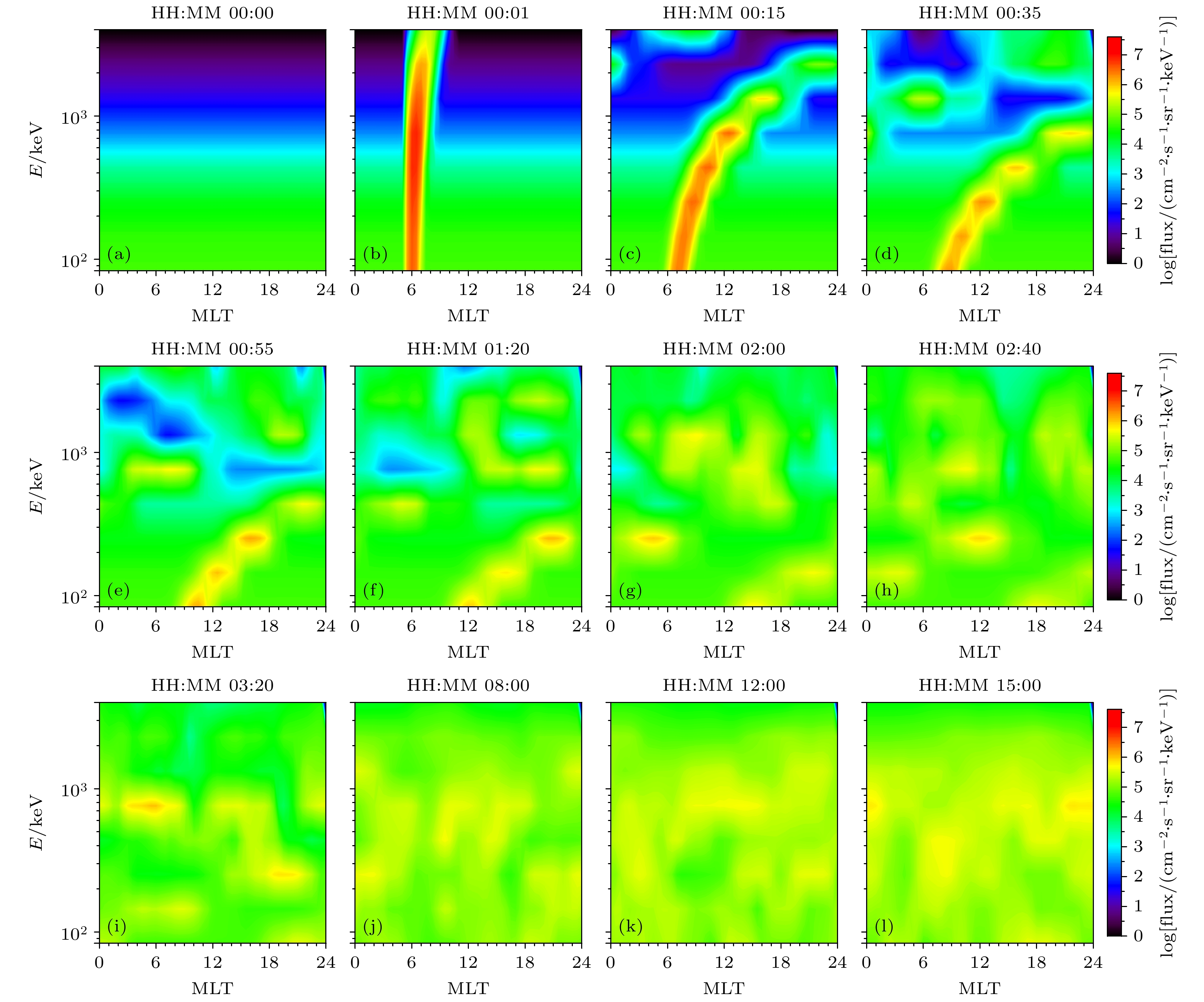
图 2 $ 0.761\;\rm MeV $电子通量的空间分布随时间的演化过程. (a)—(l)不同时刻电子微分通量在赤道面上的分布, 图中XY平面为从地球北侧看向磁赤道面的俯视图, 太阳位于图片左侧. 图(f)中红色虚线表示注入电子在漂移过程中形成的螺旋线结构
Figure 2. Temporal evolution of the spatial distribution of 0.761 MeV electrons’ flux. (a)–(l) show the differential flux distribution of electrons in the equatorial plane at different times. The XY plane in the figures represents a top-down view of the magnetic equatorial plane from the Earth's northern side, with the Sun located to the left side. The red dashed line in (f) indicates the spiral structure formed by the injected electrons during their drift process.
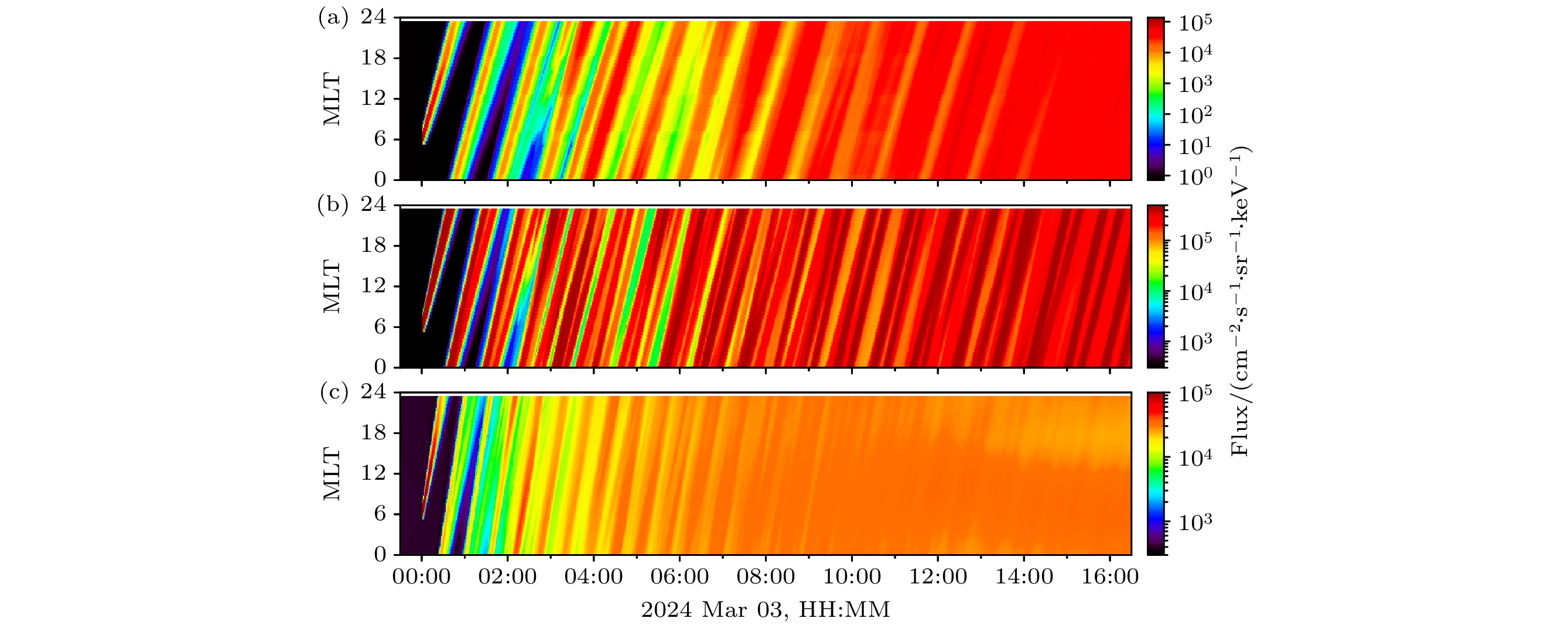
图 3 不同L处电子通量的MLT分布随时间的演化规律. (a)—(c)依次为$ L=1.23 $、$ L=1.45 $、$ L=1.98 $处$ 0.761\;\rm MeV $电子的通量环向分布
Figure 3. Temporal evolution of the MLT distribution of electron flux at different L values. (a)–(c) show the azimuthal distribution of 0.761 MeV electron flux at $ L=1.23 $, $ L=1.45 $, and $ L=1.98 $, respectively.
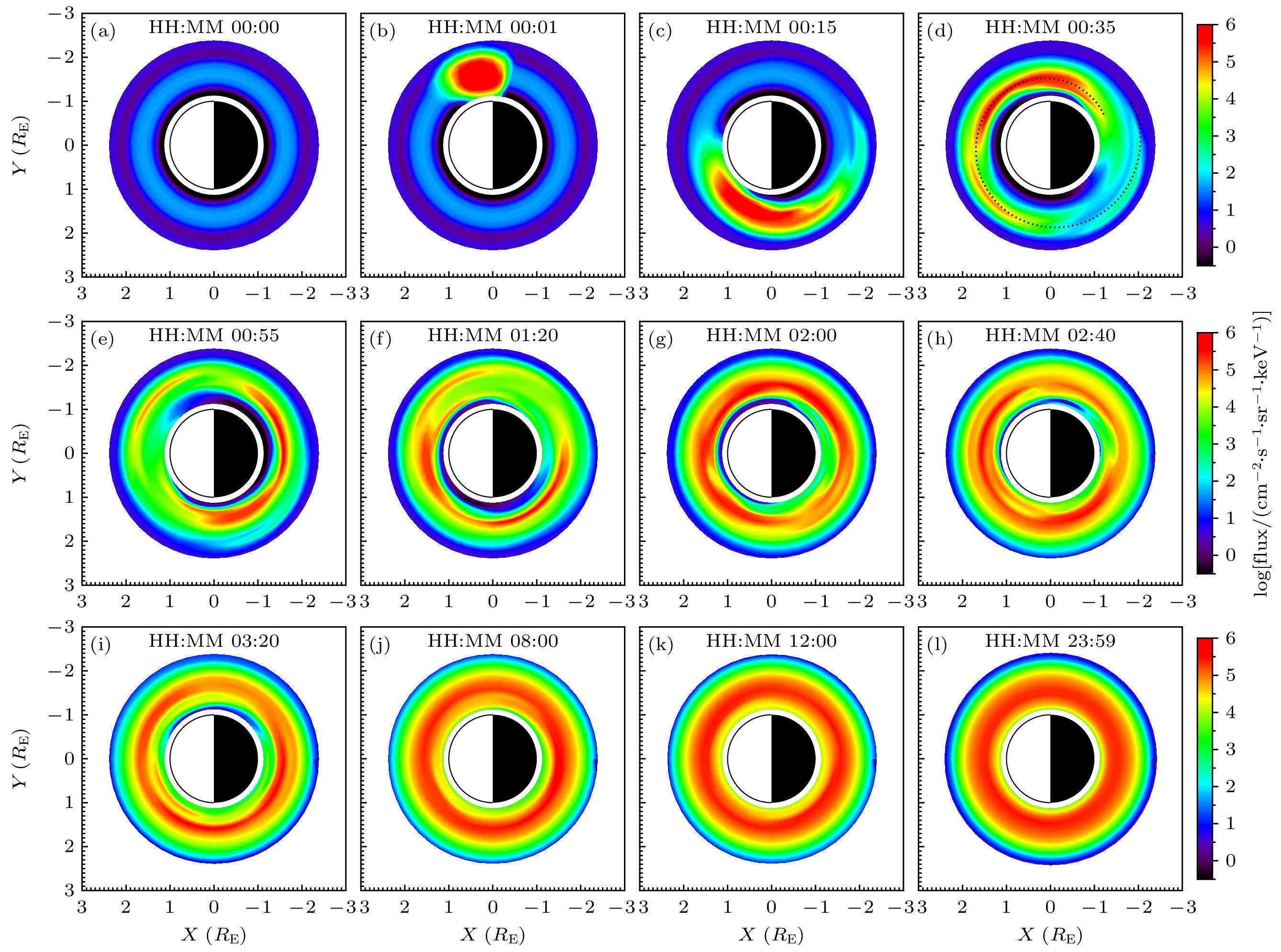
图 4 $ 1.323\;\rm MeV $电子通量的空间分布随时间的演化过程. (a)—(l)不同时刻电子微分通量在赤道面上的分布. 图(d)中黑色虚线表示注入电子在漂移过程中形成的螺旋线结构
Figure 4. Temporal evolution of the spatial distribution of 1.323 MeV electrons' flux. (a)–(l) show the differential flux distribution of electrons in the equatorial plane at different times. The black dashed line in (d) indicates the spiral structure formed by the injected electrons during their drift process.
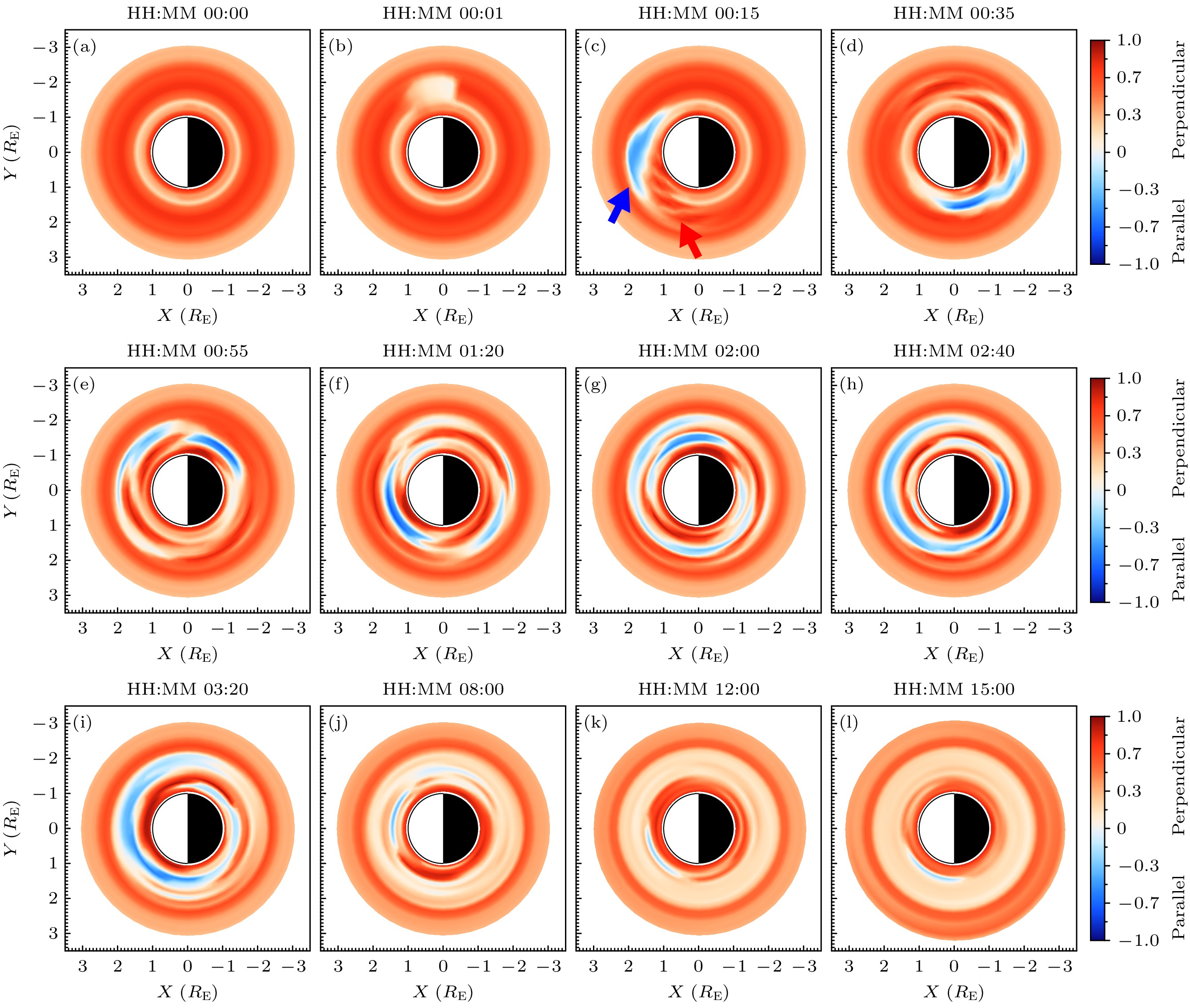
图 5 $ 0.761\;\rm MeV $电子各向异性指数的空间分布随时间的演化过程. (a)—(l)分别为不同时刻电子各向异性指数在磁赤道面中的分布. 图(c)中红色箭头指示注入电子团前端各向异性指数升高的区域, 蓝色箭头指示后端各向异性指数降低的区域
Figure 5. Temporal evolution of the spatial distribution of the anisotropy index for 0.761 MeV electrons. (a)–(l) show the distribution of the anisotropy index in the magnetic equatorial plane at different times. In figure (c), the red arrow indicates the region where the anisotropy index increases at the front of the injected electron cluster, while the blue arrow indicates the region where the anisotropy index decreases at the rear.
图 6 $ L=1.45 $处电子的能量-MLT分布随时间的演化过程. (a)—(l)分别为不同时刻电子通量在能量-MLT空间中的分布
Figure 6. Temporal evolution of the energy-MLT distribution of electrons at $ L=1.45 $. (a)–(l) show the electron flux distribution in the energy-MLT space at different times.
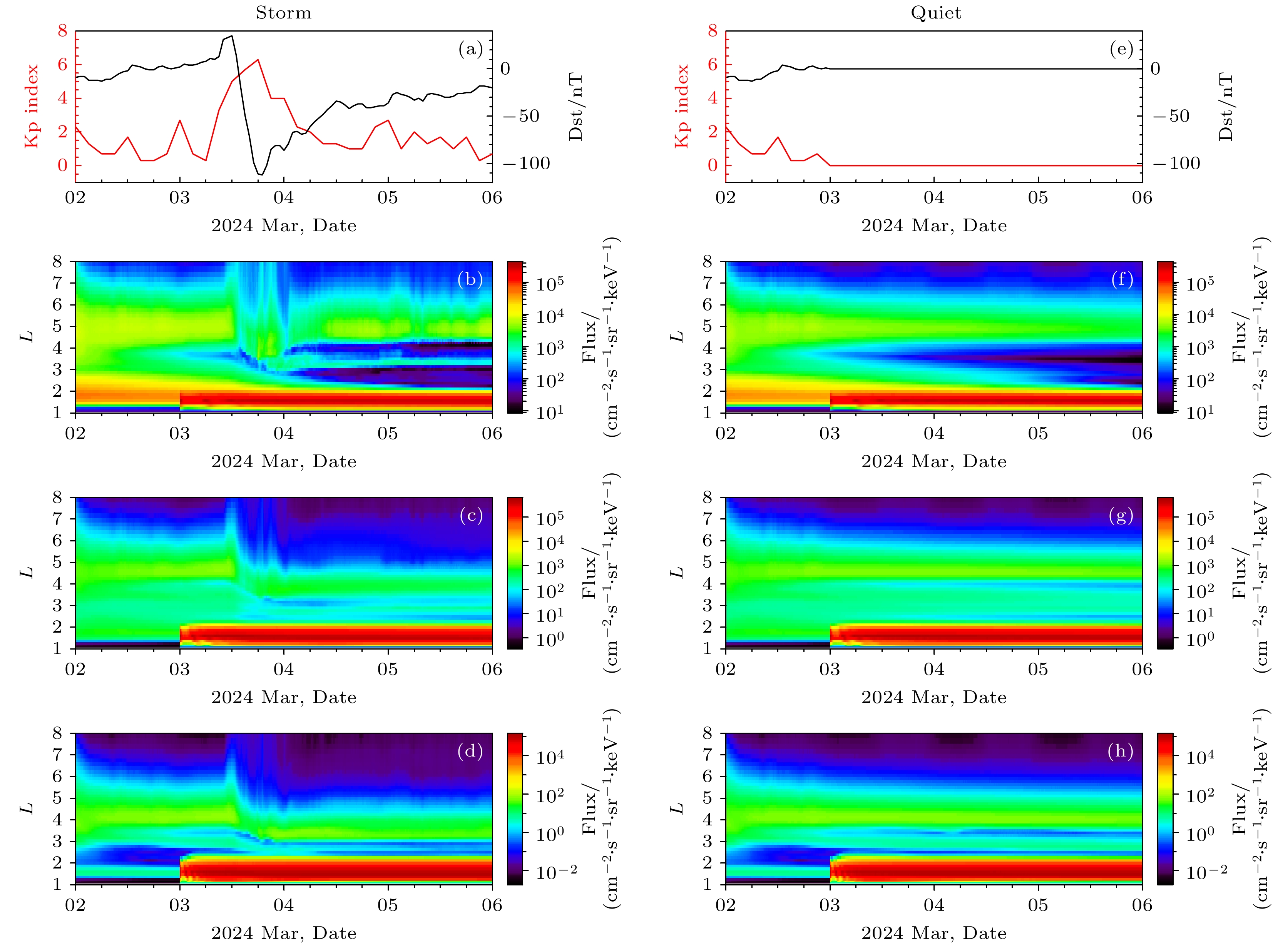
图 7 人工辐射带的长时间演化过程 (a)为模拟期间的地磁指数, 红线表示Kp指数, 黑线表示Dst指数(b)—(d)分别为$ 0.252\;\rm MeV $、$ 0.761\;\rm MeV $、$ 2.301\;\rm MeV $电子通量的L分布随时间的演化过程, 图中通量为对应L上的环向平均通量(e)—(h)为地磁平静条件下左图相应内容
Figure 7. Long-term evolution of the artificial radiation belt. (a) shows the geomagnetic indices during the simulation period, with the red line representing the Kp index and the black line representing the Dst index. (b)–(d) illustrate the temporal evolution of the L distribution of electron flux for $ 0.252\;\rm MeV $, $ 0.761\;\rm MeV $, and $ 2.301\;\rm MeV $, respectively, where the flux represents the azimuthally averaged flux at the corresponding L. (e)–(h) show the corresponding content under geomagnetically quiet conditions.
-
[1] Lyons L R 1973 Journal of Geophysical Research 78 6793
Google Scholar
[2] Zheng Y, Ganushkina N Y, Jiggens P, Jun I, Meier M, Minow J I, O’Brien T P, Pitchford D, Shprits Y, Tobiska W K, Xapsos M A, Guild T B, Mazur J E, Kuznetsova M M 2019 Space Weather 17 1384
Google Scholar
[3] Christofilos N C 1959 Journal of Geophysical Research (1896-1977) 64 869
[4] Beall D S, Bostrom C O, Williams D J 1967 Journal of Geophysical Research 72 3403
Google Scholar
[5] 王建国, 刘利, 牛胜利, 左应红, 高银军, 朱金辉, 张相华, 李桠, 李夏至 2023 现代应用物理 14 3
Google Scholar
[6] 朱金辉, 左应红, 刘利, 牛胜利, 商鹏, 李夏至, 王学栋 2023 现代应用物理 14 44
[7] 顾旭东, 赵正予, 倪彬彬, 汪枫 2009 58 5871
Google Scholar
Gu X D, Zhao Z Y, Ni B B, Wang F 2009 Acta Phys. Sin 58 5871
Google Scholar
[8] Tu W, Cunningham G S, Chen Y, Morley S K, Reeves G D, Blake J B, Baker D N, Spence H 2014 Geophysical Research Letters 41 1359
Google Scholar
[9] Fok M C, Buzulukova N Y, Chen S H, Glocer A, Nagai T, Valek P, Perez J D 2014 Journal of Geophysical Research: Space Physics 119 7522
Google Scholar
[10] Fok M C, Wolf R A, Spiro R W, Moore T E 2001 Journal of Geophysical Research: Space Physics 106 8417
Google Scholar
[11] Fok M ff, Kang S ff, Ferradas C P, Buzulukova N Y, Glocer A, Komar C M 2021 Journal of Geophysical Research: Space Physics 126 e2020JA028987
Google Scholar
[12] Albert J M 2005 Journal of Geophysical Research: Space Physics 110 A03218
[13] Albert J M 2008 Journal of Geophysical Research: Space Physics 113 A06208
[14] Albert J M, Meredith N P, Horne R B 2009 Journal of Geophysical Research: Space Physics 114 1DD
[15] Weimer D R 2001 Journal of Geophysical Research: Space Physics 106 407
Google Scholar
[16] Tsyganenko N A 2012 Journal of Geophysical Research: Space Physics 100 5599
[17] Tsyganenko N A, Stern D P 1996 Journal of Geophysical Research: Space Physics 101 27187
Google Scholar
[18] Tsyganenko N A, Mukai T 2003 Journal of Geophysical Research: Space Physics 108 1136
[19] Tsyganenko N A, Sitnov M I 2005 Journal of Geophysical Research: Space Physics 110 A03208
[20] Meredith N P, Horne R B, Thorne R M, Summers D, Anderson R R 2004 Journal of Geophysical Research: Space Physics 109 A06209
[21] Meredith N P, Horne R B, Sicard‐Piet A, Boscher D, Yearby K H, Li W, Thorne R M 2012 Journal of Geophysical Research: Space Physics 117 A10225
[22] Rairden R L, Frank L A, Craven J D 2012 Journal of Geophysical Research: Space Physics 91 13613
[23] Gombosi T I, Baker D N, Balogh A, Erickson P J, Huba J D, Lanzerotti L J 2017 Space Science Reviews 212 985
Google Scholar
[24] Cowee M, Winske D 2012 (Report) Update on the Electron Source Model, DOI: 10.2172/1046529
[25] Dyal P 2006 Journal of Geophysical Research: Space Physics 111 A12211
[26] 王建国, 牛胜利, 张殿辉 2010 高空核爆炸效应参数手册 (北京: 原子能出版社), pp 259–260
[27] Vette J I 1991 The AE-8 trapped electron model environment, vol. 91 (Greenbelt, Md.: NSSDC/WDCA-R&S 91–24, NASA Goddard Space Flight Center), pp 3–1 – 3–9
[28] Hamlin D A, Karplus R, Vik R C, Watson K M 1961 Journal of Geophysical Research 66 1
Google Scholar
[29] Southwood D J, Kivelson M G 1981 Journal of Geophysical Research: Space Physics 86 5643
Google Scholar
[30] Southwood D J, Kivelson M G 1982 Journal of Geophysical Research: Space Physics 87 1707
Google Scholar
[31] Fei Y, Chan A A, Elkington S R, Wiltberger M J 2006 Journal of Geophysical Research: Space Physics 111 A12209
[32] Lejosne S, Albert J M 2023 Frontiers in Astronomy and Space Sciences 10 1200485
Google Scholar
[33] Lejosne S, Albert J M, Walton S D 2023 Frontiers in Astronomy and Space Sciences 10 1232512
Google Scholar
[34] Sandhu J K, Rae I J, Wygant J R, Breneman A W, Tian S, Watt C E J, Horne R B, Ozeke L G, Georgiou M, Walach M T 2021 Journal of Geophysical Research: Space Physics 126 e2020JA029024
Google Scholar
[35] Aryan H, Bortnik J, Meredith N P, Horne R B, Sibeck D G, Balikhin M A 2020 Journal of Geophysical Research: Space Physics 126 e2020JA028403
[36] Malaspina D M, Zhu H, Drozdov A Y 2020 Journal of Geophysical Research: Space Physics 125 e2019JA027415
Google Scholar
DownLoad:
Metrics
- Abstract views: 253
- PDF Downloads: 9
- Cited By: 0
