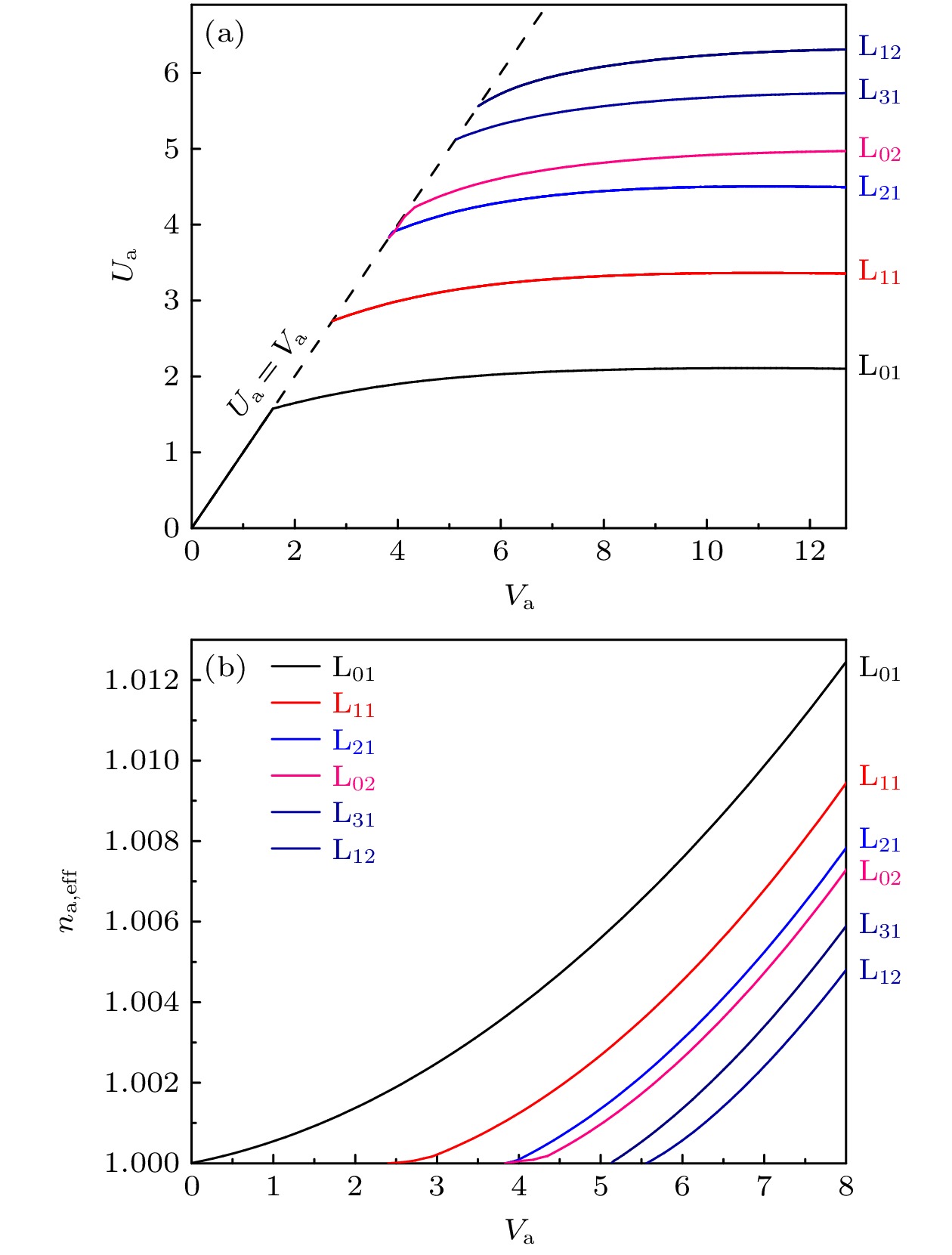
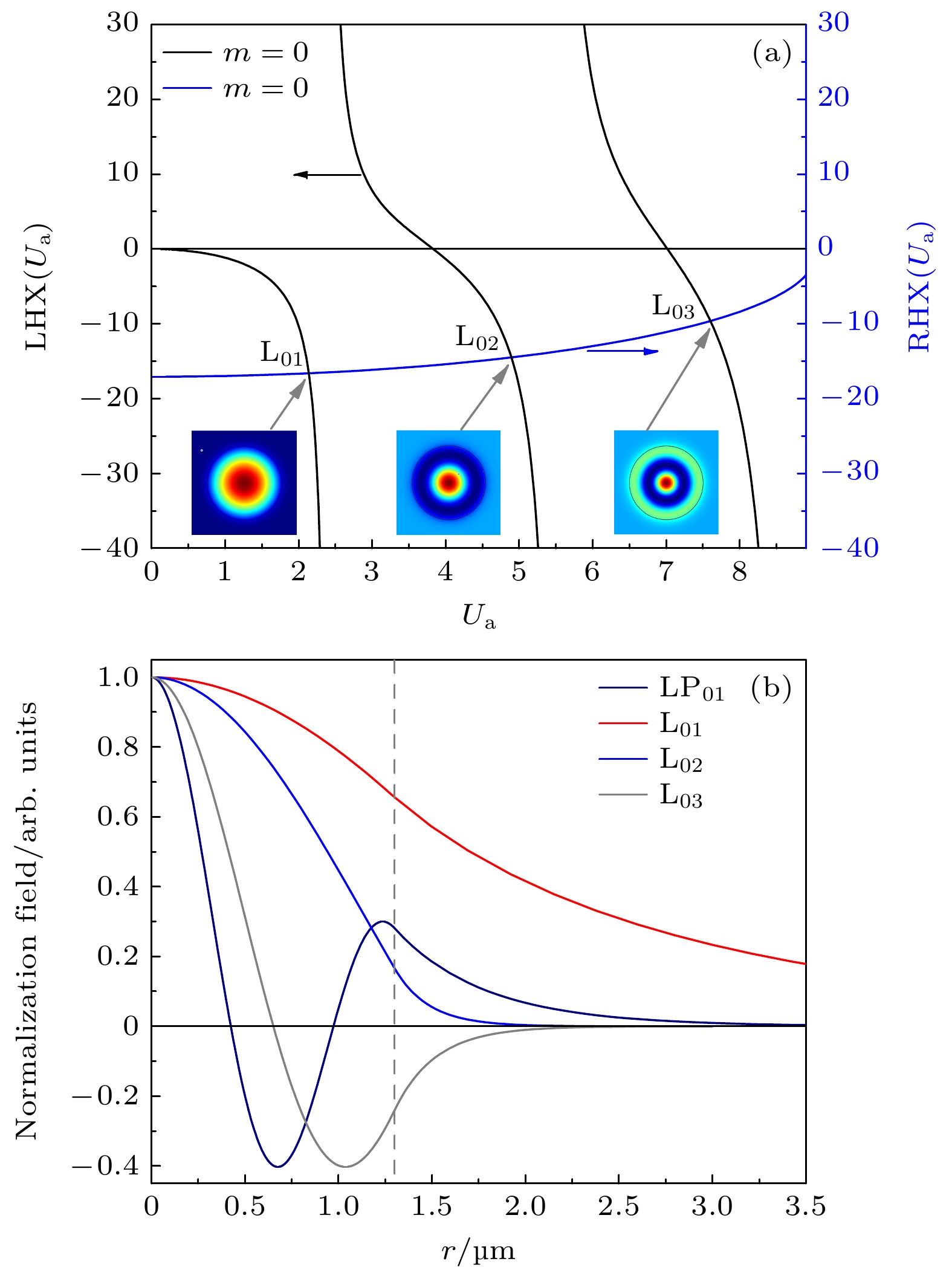
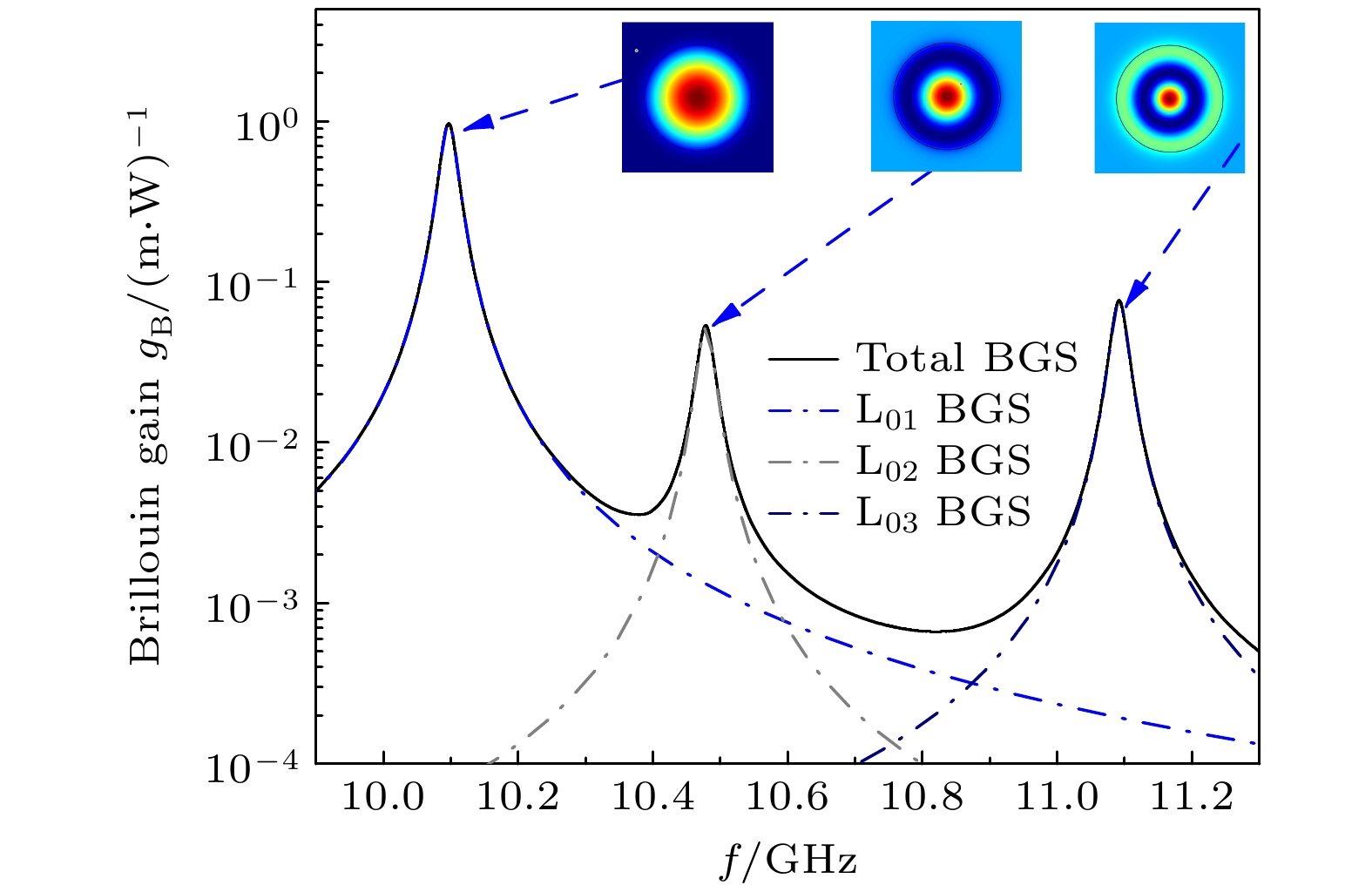
-
In this work, the acoustic Helmholtz equation is derived, and its analytical solution and the characteristic equation of the uniform guide mode in single mode fibers are obtained by the method of separation of variables. The normalized frequency of the acoustic mode is defined. By combining the argument approximation of the Bessel function are analyzed the eigenvalue range of the acoustic mode, the cut-off frequency, far from the cut-off state of the acoustic mode induced by backward stimulated Brillouin scattering, the dispersion and the multi-peak Brillouin gain spectrum. The research results indicate that the longitudinal acoustic fundamental mode L01 cannot be cut-off and is mainly confined in the fiber core, which is coupled with the optical fundamental mode LP01 to form the main peak of the Brillouin gain spectrum. The other higher-order acoustic modes all have low cut-off frequencies and are distributed more in the fiber cladding than mode L01 which couples with the optical fundamental mode LP01 to form the subpeaks of the Brillouin gain spectrum. The transverse normalized phase constant and effective refractive index of the acoustic mode increase with normalized frequency increasing. Only longitudinal acoustic modes L0n contribute to backward Brillouin gain spectrum in single mode fiber. When the GeO2 concentration is less than 4% and core radius is 4.5 μm, the single mode characteristics of the fiber remain unchanged, but the maximum number of acoustic L0n modes is 4. With the increase of GeO2 concentration in the fiber core, the Brillouin gain spectrum is red-shifted and the number of acoustic modes increases, the Brillouin gain peak value of L01 mode gradually increases, and the contributions of higher-order modes decrease. The single-mode fiber with a core’s germanium doped concentration of 3.65% and core radius of 4.3 μm has 4 L0n modes and 16 Lmn (m>0) modes at a wavelength of 1.55 μm, with one main peak and two subpeaks in the Brillouin gain spectrum appearing due to the acousto-optic coupling of the acoustic L01, L03, and L04 modes with the optical LP01 mode. The single-mode fiber with a core’s germanium doped concentration of 15% and core radius of 1.3 μm has 3 L0n modes and 7 Lmn (m>0) modes, with the Brillouin gain spectrum having 3 main peaks due to the acousto-optic coupling of the L01, L02, and L03 modes with the LP01 mode. These conclusions are well consistent with the reported experimental phenomena and provide theoretical references for studying and utilizing the SBS acoustic waveguide in optical fibers.
[1] Shimizu K, Horiguchi T, Koyamada Y, Kurashima T 1993 Opt. Lett. 18 185
Google Scholar
[2] Bao X Y, Chen L 2012 Sensors-Basel 12 8601
Google Scholar
[3] Li M J, Xing C, Wang J, Gray S, Liu A P, Demeritt J A, Ruffin A B, Crowley A M, Walton D T, Zenteno L A 2007 Opt. Express 15 8290
Google Scholar
[4] Gonzalez H M, Song K Y, Thévenaz L 2005 Appl. Phys. Lett. 87 081113
Google Scholar
[5] Hon D T 1980 Opt. Lett. 5 516
Google Scholar
[6] Jen C K, Neron C, Shang A, Abe K, Bonnell L, Kushibiki J 1993 J. Am. Ceram. Soc. 76 712
Google Scholar
[7] Waldron R 1969 IEEE Trans. Microwave Theory Tech. 17 893
Google Scholar
[8] Shelby R, Levenson M, Bayer P 1985 Phys. Rev. B 31 5244
Google Scholar
[9] Shibata N, Okamoto K, Azuma Y 1989 J. Opt. Soc. Am. B 6 1167
Google Scholar
[10] Tartara L, Codemard C, Maran J N, Cherif R, Zghal M 2009 Opt. Commun. 282 2431
Google Scholar
[11] Poulton C G, Pant R, Eggleton B J 2013 J. Opt. Soc. Am. B 30 2657
Google Scholar
[12] Xing C, Ke C J, Guo Z, Yang K J, Wang H Y, Zhong Y B, Liu D M 2018 Opt. Express 26 28793
Google Scholar
[13] Tsvetkov S V, Likhachev M E 2023 B. Lebedev. Phys. Inst+. 50 291
Google Scholar
[14] Huang B, Wang J Q, Shao X P 2023 Photonics-Basel 10 282
Google Scholar
[15] He D Y, Liao M S, Hu L L, Yu C L, Qi Y F, Shen H, Chen L, Yang Q B, Liu M Z, Wang M, Zhou Q L, Gao W Q, Wang T X 2023 Opt. Express 31 1888
Google Scholar
[16] Kobyakov A, Kumar S, Chowdhury D Q, Ruffin A B, Sauer M, Bickham S R, Mishra R 2005 Opt. Express 13 5338
Google Scholar
[17] Dong Y, Ren G B, Xiao H, Gao Y X, Li H S, Xiao S Y, Jian S S 2017 Ieee Photonic Tech. L. 29 1955
Google Scholar
[18] 吴重庆 2000光波导理论(北京: 清华大学出版社) 第41—43页
Wu C Q 2000 Optical Waveguide Theory (Beijing: Tsinghua University Press) pp41–43
[19] Zou W W, He Z Y, Hotate K 2008 Opt. Express 16 10006
Google Scholar
[20] Jia D F, Ge C F 2004 Nonlinear Fiber Optics (Beijing: Electronic Industry Press) 第246—247页
贾东方, 葛春风 2014非线性光纤光学(北京: 电子工业出版社) pp246–247
[21] Ruffin A B, Li M J, Chen X, Kobyakov A, Annunziata F 2005 Opt. Lett. 30 3123
Google Scholar
[22] Zou W W, He Z Y, Hotate K 2006 Ieee Photonic Tech. L. 18 2487
Google Scholar
[23] Dasgupta S, Poletti F, Liu S, Petropoulos P, Richardson D J, Grüner-Nielsen L, Herstrøm S 2011 J. Lightwave Techno. 29 22
Google Scholar
[24] Koyamada Y, Sato S, Nakamura S, Sotobayashi H, Chujo W 2004 J. Lightwave Techno. 22 631
Google Scholar
[25] Kobyakov A, Sauer M, Chowdhury D 2010 Adv. Opt. Photonics 2 1
Google Scholar
-
图 1 (a) L0n模; (b) L1n模的Ua取值范围
Figure 1. The Ua value range of (a) L0n mode and (b) L1n mode
图 2 声模色散曲线 (a) Ua与Va的关系; (b) na, eff与Va的关系
Figure 2. Dispersion curve of acoustic mode: (a) $U_{{\mathrm{a}}}=f(V_{{\mathrm{a}}}) $; (b) $n_{{\mathrm{a,eff}}}=f(V_{\mathrm{a}}) $.
图 3 Va和Vo随掺锗浓度的变化
Figure 3. Variation of Va and Vo with germanium doped concentration.
图 4 布里渊增益谱随掺锗浓度变化曲线
Figure 4. BGS variation curves with germanium doped concentration.
图 5 Fiber 1的(a) L0n模特征方程和(b)归一化场分布
Figure 5. (a) L0n mode characteristic equation and (b) normalized field distribution of Fiber 1.
图 6 Fiber 1的布里渊增益谱
Figure 6. The BGS of Fiber 1.
图 7 Fiber 2的(a) L0n模特征方程和(b)归一化场分布
Figure 7. (a) L0n mode characteristic equation and (b) normalized field distribution of Fiber 2.
图 8 Fiber 2的布里渊增益谱
Figure 8. The BGS of Fiber 2.
表 2 Fiber 1和Fiber 2的Lmn模BFS (GHz)
Table 2. BFS (GHz) of the Lmn modes in Fiber 1 and Fiber 2.
Fiber type m n 1 2 3 4 Fiber 1 0 10.9244 10.9694 11.0488 11.1576 1 10.9407 11.0032 11.0985 — 2 10.9620 11.0418 11.1576 — 3 10.9880 11.0848 11.2052 — 4 11.0185 11.1318 — — 5 11.0532 11.1812 — — 6 11.0921 — — — 7 11.1349 — — — 8 11.1814 — — — Fiber 2 0 10.0902 10.4721 11.0857 — 1 10.2306 10.7466 — — 2 10.4117 11.0438 — — 3 10.6287 — — — 4 10.8779 — — — 5 11.1511 — — — 
DownLoad: CSV
表 3 两种单模光纤的理论计算与实验结果比较
Table 3. Comparison of experimental and theoretical calculation results of two single-mode optical fibers.
Fiber type Aeff/μm2 Aao/μm2 I BFS/GHz Relative error/% Reference This paper Fiber 1 76.32 78.87 0.9668 10.9170[22] 10.9244 0.0678 15612.35 0.0049 10.9630[22] 10.9694 0.0584 14173.60 0.0054 11.0430[22] 11.0488 0.0525 11249.48 0.0068 11.1540[22] 11.1576 0.0323 Fiber 2 21.02 26.06 0.8068 10.0000[23] 10.0902 0.8087 482.47 0.0436 10.5000[23] 10.4721 0.2657 328.89 0.0639 11.1100[23] 11.0857 0.2187
DownLoad: CSV
-
[1] Shimizu K, Horiguchi T, Koyamada Y, Kurashima T 1993 Opt. Lett. 18 185
Google Scholar
[2] Bao X Y, Chen L 2012 Sensors-Basel 12 8601
Google Scholar
[3] Li M J, Xing C, Wang J, Gray S, Liu A P, Demeritt J A, Ruffin A B, Crowley A M, Walton D T, Zenteno L A 2007 Opt. Express 15 8290
Google Scholar
[4] Gonzalez H M, Song K Y, Thévenaz L 2005 Appl. Phys. Lett. 87 081113
Google Scholar
[5] Hon D T 1980 Opt. Lett. 5 516
Google Scholar
[6] Jen C K, Neron C, Shang A, Abe K, Bonnell L, Kushibiki J 1993 J. Am. Ceram. Soc. 76 712
Google Scholar
[7] Waldron R 1969 IEEE Trans. Microwave Theory Tech. 17 893
Google Scholar
[8] Shelby R, Levenson M, Bayer P 1985 Phys. Rev. B 31 5244
Google Scholar
[9] Shibata N, Okamoto K, Azuma Y 1989 J. Opt. Soc. Am. B 6 1167
Google Scholar
[10] Tartara L, Codemard C, Maran J N, Cherif R, Zghal M 2009 Opt. Commun. 282 2431
Google Scholar
[11] Poulton C G, Pant R, Eggleton B J 2013 J. Opt. Soc. Am. B 30 2657
Google Scholar
[12] Xing C, Ke C J, Guo Z, Yang K J, Wang H Y, Zhong Y B, Liu D M 2018 Opt. Express 26 28793
Google Scholar
[13] Tsvetkov S V, Likhachev M E 2023 B. Lebedev. Phys. Inst+. 50 291
Google Scholar
[14] Huang B, Wang J Q, Shao X P 2023 Photonics-Basel 10 282
Google Scholar
[15] He D Y, Liao M S, Hu L L, Yu C L, Qi Y F, Shen H, Chen L, Yang Q B, Liu M Z, Wang M, Zhou Q L, Gao W Q, Wang T X 2023 Opt. Express 31 1888
Google Scholar
[16] Kobyakov A, Kumar S, Chowdhury D Q, Ruffin A B, Sauer M, Bickham S R, Mishra R 2005 Opt. Express 13 5338
Google Scholar
[17] Dong Y, Ren G B, Xiao H, Gao Y X, Li H S, Xiao S Y, Jian S S 2017 Ieee Photonic Tech. L. 29 1955
Google Scholar
[18] 吴重庆 2000光波导理论(北京: 清华大学出版社) 第41—43页
Wu C Q 2000 Optical Waveguide Theory (Beijing: Tsinghua University Press) pp41–43
[19] Zou W W, He Z Y, Hotate K 2008 Opt. Express 16 10006
Google Scholar
[20] Jia D F, Ge C F 2004 Nonlinear Fiber Optics (Beijing: Electronic Industry Press) 第246—247页
贾东方, 葛春风 2014非线性光纤光学(北京: 电子工业出版社) pp246–247
[21] Ruffin A B, Li M J, Chen X, Kobyakov A, Annunziata F 2005 Opt. Lett. 30 3123
Google Scholar
[22] Zou W W, He Z Y, Hotate K 2006 Ieee Photonic Tech. L. 18 2487
Google Scholar
[23] Dasgupta S, Poletti F, Liu S, Petropoulos P, Richardson D J, Grüner-Nielsen L, Herstrøm S 2011 J. Lightwave Techno. 29 22
Google Scholar
[24] Koyamada Y, Sato S, Nakamura S, Sotobayashi H, Chujo W 2004 J. Lightwave Techno. 22 631
Google Scholar
[25] Kobyakov A, Sauer M, Chowdhury D 2010 Adv. Opt. Photonics 2 1
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 2395
- PDF Downloads: 209
- Cited By: 0
