-
Underwater sound propagation models are generally established from the extrinsic perspective, that is, embedding acoustic channels in Euclidean space with a fixed coordinate system. Riemannian geometry is intrinsic for curved space, which can describe the essential properties of background manifolds. The underwater acoustic Gaussian beam is originally adopted from seismology. Till now it has been the most important method used in acoustic ray based modeling and applications. Owing to the advantages of Gaussian beam method over the traditional ray counterpart, it is the mainstream technology of ray propagation computational software such as the famous Bellhop. With the assumption of Euclidean space, it is hard to grasp the naturally curved characteristics of the Gaussian beam. In this work, we propose the Riemannian geometry theory of underwater acoustic ray propagation, and obtain the following results. 1) The Riemannian geometric intrinsic forms of the eikonal equation, paraxial ray equation and the Gaussian beam under radially symmetric acoustic propagation environments are established, which provide a Riemannian geometric interpretation of the Gaussian beam. In fact, the underwater acoustic eikonal equation is equivalent to the geodesic equation in Riemannian manifolds, and the intrinsic geometric spreading of the Gaussian beam corresponds to the lateral deviation of geodesic curve along the Jacobian field. 2) Some geometric and topological properties of acoustic ray about conjugate points and section curvature are acquired by the Jacobi field theory, indicating that the convergence of ray beam corresponds to the intersection of geodesics at the conjugate point with positive section curvature. 3) The specific modeling method under horizontal stratified and distance-related environment is presented by using the above theory. And we point out that the method proposed here is also applicable to other radially symmetric acoustic propagation environments. 4) Simulations and comparative analyses of three typical underwater acoustic propagation examples, confirm the feasibility of the Riemannian geometric model for underwater acoustic propagation, and show that the Riemannian geometric model has exact mathematical physics meaning over the Euclidean space method adopted by the Bellhop model. The basic theory given in this paper can be extended to the curved surface, three-dimensional and other complex propagation cases. And especially it lays a theoretical foundation for the further research of long-range acoustic propagation considering curvature of the earth.
-
Keywords:
- Gaussian beam /
- underwater acoustic modeling /
- geodesic /
- geometric spread /
- Jacobi field /
- conjugate point
[1] Kravtsov Y A, Berczynski P 2007 Stud. Geophys. Geod. 51 1
Google Scholar
[2] Babich V M, Lazutkin V F 1968 Commun. Math. Phys. 2 9
Google Scholar
[3] Červený V, Popov M M, Pšenčík I 1982 Geophys. J. R. astr. Soc. 70 109
Google Scholar
[4] Porter M B, Bucker H P 1987 J. Acoust. Soc. Am. 82 1349
Google Scholar
[5] Červený V, 2001 Seismic Ray Theory (Cambridge: Cambridge University Press) p237
[6] Červený V, Pšenčík I 2010 Geophys. J. Int. 180 798
[7] Smith S T 2014 SIAM J. Appl. Math. 74 1637
Google Scholar
[8] Jensen F B, Kuperman W A, Porter M B 2011 Schmidt H Computational Ocean Acoustics ( NewYork: Springer-Verlag) p125
[9] 梁灿彬, 周彬 2006 微分几何入门与广义相对论 (北京: 科学出版社) 第190页
Liang C B, Zhou B 2006 Introduction to Differential Geometry and General Relativity (Beijing: Science Press) p190 (in Chinese)
[10] Gordon W 1923 Ann. Phys. 72 421
[11] 郭弘, 邓锡铭 1995 中国科学: A辑 25 7
Guo H, Deng X M 1995 Sci. China (Series A) 25 7 (in Chinese)
[12] 邓锡铭, 郭弘, 曹清 1995 中国激光 22 523
Google Scholar
Deng X M, Guo H, Cao Q 1995 Chin. J. Lasers 22 523
Google Scholar
[13] 杨孔庆, 罗明秋, 李幼铭 2018 石油物探 57 5
Google Scholar
Yang K Q, Luo M Q, Li Y M 2018 Geophys. Prospect. Petrol. 57 5
Google Scholar
[14] White R 1973 J. Acoust. Soc. Am. 53 1700
Google Scholar
[15] Meyer R, Schroeter G 1981 Acta Acust united Ac 47 105
[16] Bergman D R 2005 Technical Report NRL/MR/7140-05-8835 Naval Research Laboratory
[17] Bergman D R 2005 Waves Random Complex 15 417
Google Scholar
[18] Bergman D R 2006 Wave Motion 43 508
Google Scholar
[19] Bergman D R 2021 Int. J. Acoust. Vib. 26 2
Google Scholar
[20] Bergman D R 2015 Physics 120 5
Google Scholar
[21] 陈维恒, 李兴校 2002 黎曼几何引论 (北京: 北京大学出版社) 第171页
Chen W H, Li X X 2002 Introduction to Riemannian Geometry (Beijing: Peking University Press) p267 (in Chinese)
[22] 侯伯元, 侯伯宇 2004 物理学家用微分几何 (北京: 科学出版社) 第143页
Hou B Y, Hou B Y 2004 Differential Geometry in Physics (Beijing: Science Press) p143 (in Chinese)
[23] Do Carmo M P 1992 Riemannian Geometry (Boston: Birkhauser) p110
[24] Cheeger J, D G Ebin 2008 Comparison Theorems in Riemannian Geometry (Providence, Rhode Island: American Mathematical Society) p35
[25] Kobayashi S, Nomizu K 1963 Foundations of Differential Geometry (Vol. 2) (New York: John Wiley & Sons) p73
[26] Eisner L, Pšenčík I 1996 Pageoph 148 227
Google Scholar
[27] Davis H, Fleming H, Hardy WAR, Miningham R, Rosenbaum S 1968 Technical Report the Hudson Laboratories Ray Tracing Program (Beijing: University of Science and Technology of China Press)
[28] Foreman T 1988 Ph. D. Dissertation (Austin: The University of Texas at Austin)
-
图 1 傍轴射线示意图,
${\boldsymbol{T}} = \dfrac{{{{\rm{d}}} {\boldsymbol{x}}}}{{{{\rm{d}}} s}}$ 是射线的切向量, N是射线的法向量Figure 1. Paraxial ray tube,
${\boldsymbol{T}} = \dfrac{{{{\rm{d}}} {\boldsymbol{x}}}}{{{{\rm{d}}} s}}$ is the tangent vector, N is the normal vector.
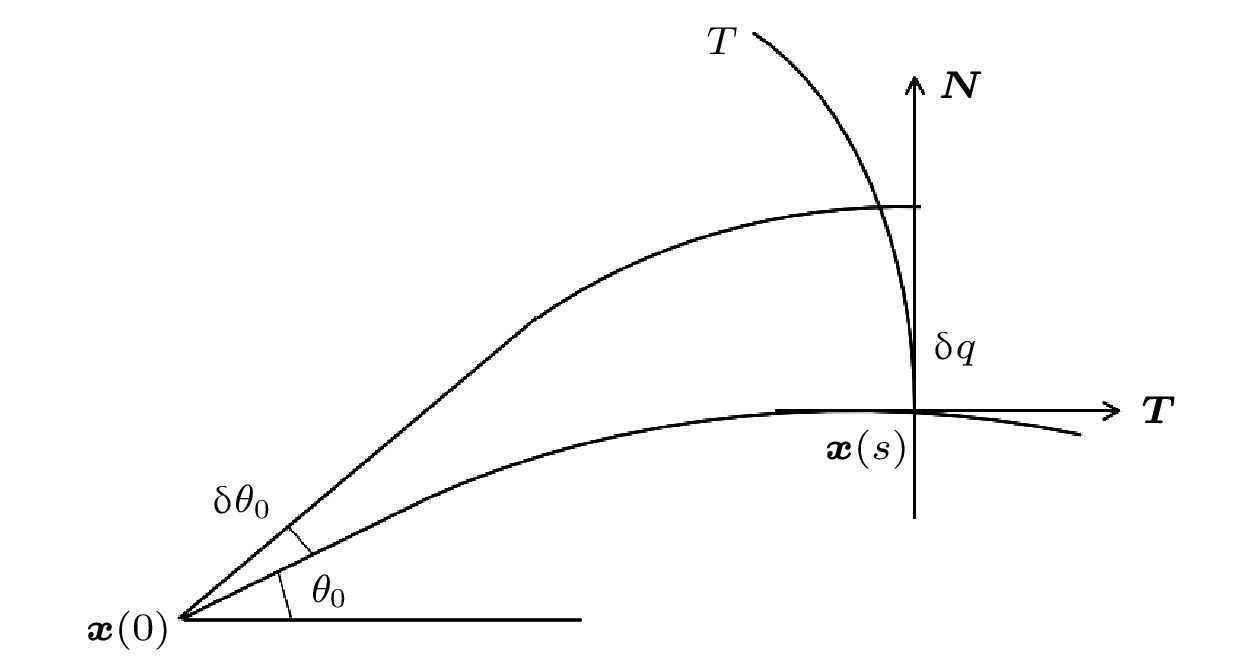
图 2 双曲余弦声速剖面的声线和焦散点, 参数为
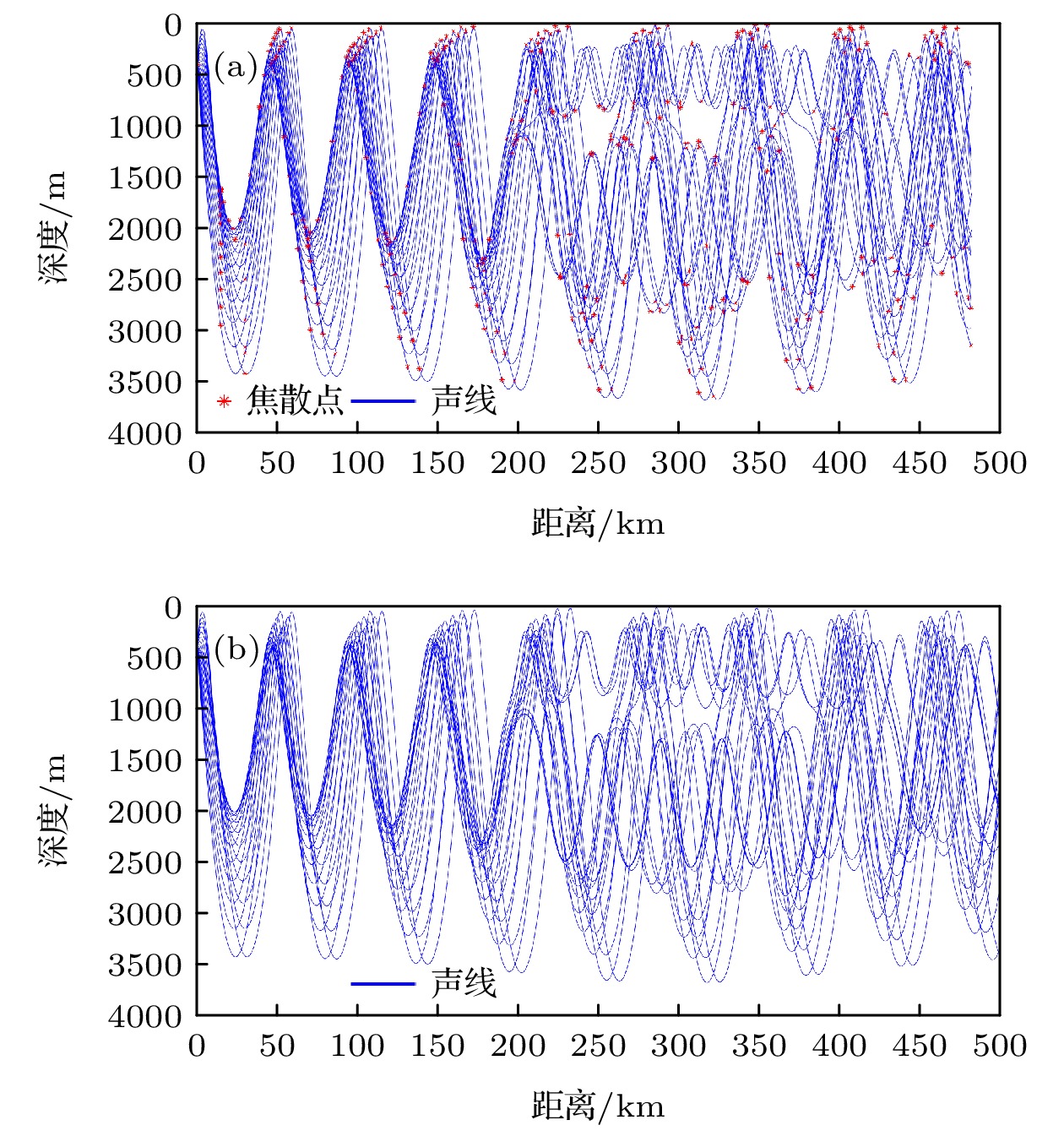
$ {c_1} = 1500 $ m/s,$ {z_1} = 1.5 $ km,$ E = 5 $ km, 声源深度为$ {z_0} = 1 $ km, 初始掠射角$ {\theta }_{0}\in (-{10}^{\circ}, {12}^{\circ}) $ ,$t \in [0, 130]~ \text{s}$ (a)共轭点理论仿真结果; (b) Bellhop仿真结果Figure 2. Rays and caustics for the hyperbolic cosine sound speed profile with parameters
$ {c_1} = 1500 $ m/s,$ {z_1} = 1.5 $ km,$ E = 5 $ km,$ {z_0} = 1 $ km,$ {\theta }_{0}\in (-{10}^{\circ}, {12}^{\circ}) $ ,$t \in [0, 130]~\text{s}$ : (a) Conjugate point theory simulation results; (b) Bellhop simulation results.
图 3 线性声速剖面的声线求解结果, 参数为
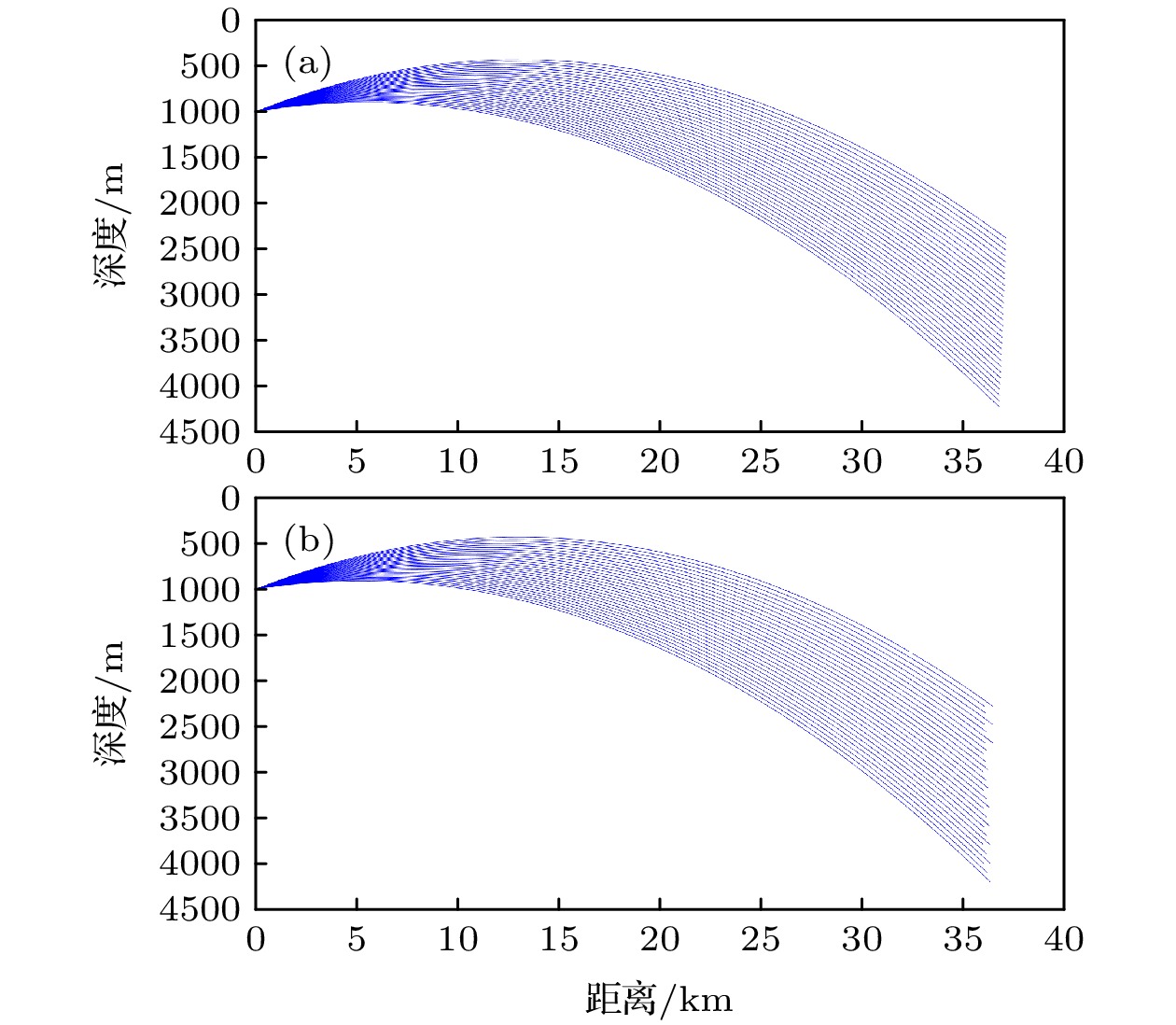
${c_0} = 1500$ m/s,$b = - 0.01$ , 声源深度为${z_0} = 1$ km, 初始掠射角$ {\theta }_{0} \in (-{5}^{\circ }, -{2}^{\circ }) $ ,$t \in [0, 25]~ \text{s}$ (a)共轭点理论仿真结果; (b) Bellhop仿真结果Figure 3. Rays for the linear sound speed profile with parameters
$ {c_0} = 1500 $ m/s,$ b = - 0.01 $ ,$ {z_0} = 1 $ km,$ {\theta }_{0}\in (-{5}^{\circ }, -{2}^{\circ }) $ ,$t \in [0, 25]~ \text{s}$ : (a) Conjugate point theory simulation results; (b) Bellhop simulation results.
图 4 声速剖面(38) 的声速等值线, 参数为
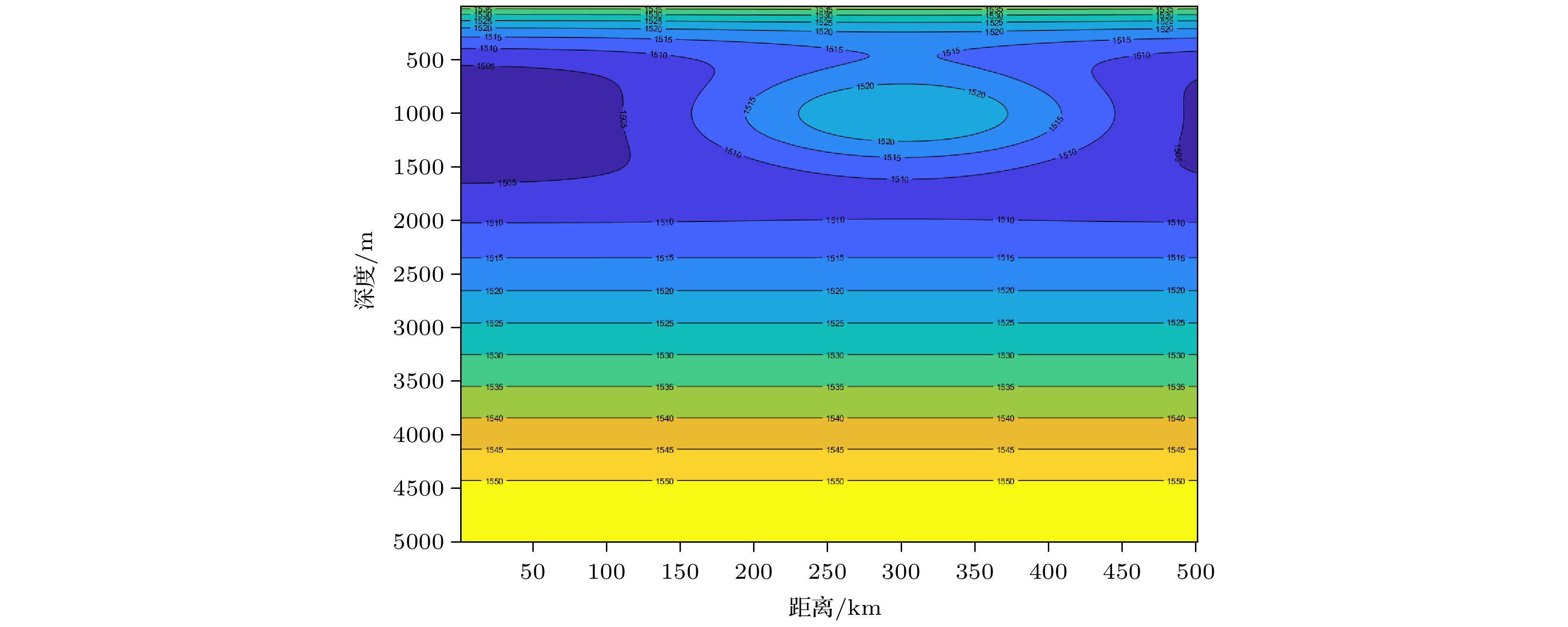
${\text{DR = 150}}{\kern 1 pt} {\kern 1 pt} {\kern 1 pt} {\kern 1 pt} {\text{km, }}\;{\text{DZ = 500}}{\kern 1 pt} {\kern 1 pt} {\kern 1 pt} {\kern 1 pt} {\text{km, }}\;{\text{Ze = 1000}}{\kern 1 pt} {\kern 1 pt} {\kern 1 pt} {\kern 1 pt} {\text{km, }}\;{\text{Re = 300}}{\kern 1 pt} {\kern 1 pt} {\kern 1 pt} {\kern 1 pt} {\text{km}}$ Figure 4. Sound speed contours for the sound speed profile (38) with parameters
${\text{DR = 150}}~{\text{km, }} \;{\rm DZ =} $ $ 500 {\text{km, }} \;{\text{Ze = 1000}}{\kern 1 pt} {\kern 1 pt} {\kern 1 pt} {\kern 1 pt} {\text{km, }} \;{\text{Re = 300}}{\kern 1 pt} {\kern 1 pt} {\kern 1 pt} {\kern 1 pt} {\text{km}}$ .
-
[1] Kravtsov Y A, Berczynski P 2007 Stud. Geophys. Geod. 51 1
Google Scholar
[2] Babich V M, Lazutkin V F 1968 Commun. Math. Phys. 2 9
Google Scholar
[3] Červený V, Popov M M, Pšenčík I 1982 Geophys. J. R. astr. Soc. 70 109
Google Scholar
[4] Porter M B, Bucker H P 1987 J. Acoust. Soc. Am. 82 1349
Google Scholar
[5] Červený V, 2001 Seismic Ray Theory (Cambridge: Cambridge University Press) p237
[6] Červený V, Pšenčík I 2010 Geophys. J. Int. 180 798
[7] Smith S T 2014 SIAM J. Appl. Math. 74 1637
Google Scholar
[8] Jensen F B, Kuperman W A, Porter M B 2011 Schmidt H Computational Ocean Acoustics ( NewYork: Springer-Verlag) p125
[9] 梁灿彬, 周彬 2006 微分几何入门与广义相对论 (北京: 科学出版社) 第190页
Liang C B, Zhou B 2006 Introduction to Differential Geometry and General Relativity (Beijing: Science Press) p190 (in Chinese)
[10] Gordon W 1923 Ann. Phys. 72 421
[11] 郭弘, 邓锡铭 1995 中国科学: A辑 25 7
Guo H, Deng X M 1995 Sci. China (Series A) 25 7 (in Chinese)
[12] 邓锡铭, 郭弘, 曹清 1995 中国激光 22 523
Google Scholar
Deng X M, Guo H, Cao Q 1995 Chin. J. Lasers 22 523
Google Scholar
[13] 杨孔庆, 罗明秋, 李幼铭 2018 石油物探 57 5
Google Scholar
Yang K Q, Luo M Q, Li Y M 2018 Geophys. Prospect. Petrol. 57 5
Google Scholar
[14] White R 1973 J. Acoust. Soc. Am. 53 1700
Google Scholar
[15] Meyer R, Schroeter G 1981 Acta Acust united Ac 47 105
[16] Bergman D R 2005 Technical Report NRL/MR/7140-05-8835 Naval Research Laboratory
[17] Bergman D R 2005 Waves Random Complex 15 417
Google Scholar
[18] Bergman D R 2006 Wave Motion 43 508
Google Scholar
[19] Bergman D R 2021 Int. J. Acoust. Vib. 26 2
Google Scholar
[20] Bergman D R 2015 Physics 120 5
Google Scholar
[21] 陈维恒, 李兴校 2002 黎曼几何引论 (北京: 北京大学出版社) 第171页
Chen W H, Li X X 2002 Introduction to Riemannian Geometry (Beijing: Peking University Press) p267 (in Chinese)
[22] 侯伯元, 侯伯宇 2004 物理学家用微分几何 (北京: 科学出版社) 第143页
Hou B Y, Hou B Y 2004 Differential Geometry in Physics (Beijing: Science Press) p143 (in Chinese)
[23] Do Carmo M P 1992 Riemannian Geometry (Boston: Birkhauser) p110
[24] Cheeger J, D G Ebin 2008 Comparison Theorems in Riemannian Geometry (Providence, Rhode Island: American Mathematical Society) p35
[25] Kobayashi S, Nomizu K 1963 Foundations of Differential Geometry (Vol. 2) (New York: John Wiley & Sons) p73
[26] Eisner L, Pšenčík I 1996 Pageoph 148 227
Google Scholar
[27] Davis H, Fleming H, Hardy WAR, Miningham R, Rosenbaum S 1968 Technical Report the Hudson Laboratories Ray Tracing Program (Beijing: University of Science and Technology of China Press)
[28] Foreman T 1988 Ph. D. Dissertation (Austin: The University of Texas at Austin)
DownLoad:
Catalog
Metrics
- Abstract views: 4129
- PDF Downloads: 106
- Cited By: 0
