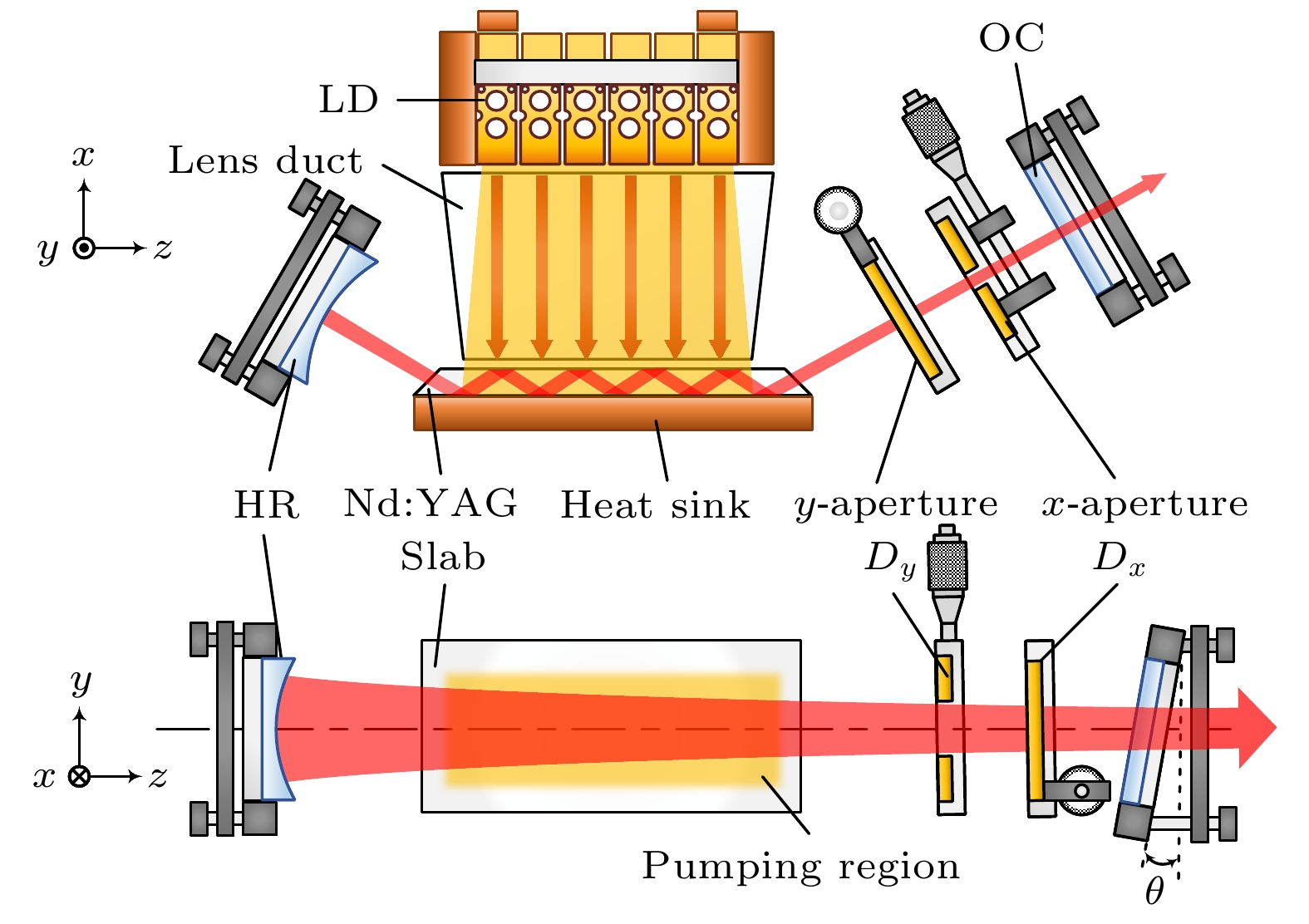
-
Hermite-Gaussian (HG) beams have many important applications in the optical frontier, and the limited output power of the high-purity HG beams is partly due to the small gain volume of the mode. The commonly used off-axis end-pumped scheme offers a narrow gain volume whose diameter is about a hundred microns. In this work, a new method of generating the HG beams based on a slab resonator that has a large mode volume is proposed and experimentally demonstrated. According to the optical resonator theory, the intra-cavity modes in thickness and width direction of the slab resonator are restricted by inserting two size-adjustable apertures, respectively. The one-dimensional HG beam generation is mainly guaranteed by the size of the aperture along the thickness direction of the slab, which matches the diameter of the fundamental mode. The different order one-dimensional HG beams are obtained by refined intra-cavity mode modulation. Since the higher-order modes are less sensitive to the misalignment of the cavity mirror than the lower-order modes, and the manipulation of the modes-loss at different orders is achieved by combining the tilt control of the coupled output mirror and the size control of intra-cavity apertures. By adjusting the optical gain and loss in the resonant cavity, the single mode wins the competition of laser modes. Therefore, high-purity one-dimensional HG beams with 0 to 9 orders (HG00 to HG09) are generated. The pump module is comprised of a two-dimensional laser diode array which offers face-pumping to the large surface of the slab, therefore the width of the mode volume is extended to several millimeters. By further incorporating the 100mm-level long slab, the total gain volume is much larger than the counterpart in the off-axis pumping scheme. In this work, the output power of the highest order HG09 mode increases up to 244 mW. Owing to the large gain volume and uniform gain distribution caused by the face-pumped slab, the purity of high order HG modes is quite good. The correlation coefficient
$ \rho $ between the measured intensity distribution and the theoretical value is larger than 0.95. The beam quality factor$ {M}^{2} $ is also in good agreement with the theoretical one. Finally, a conversion from Hermite-Gaussian beams to the donut-shaped Laguerre-Gaussian beams is realized by using an astigmatic mode converter. Hopefully, power scaling of the HG beam output is also expected by employing cascaded slab amplifiers, and the approach in this paper provides a novel solution for generation of high power HG beams.-
Keywords:
- Hermite-Gaussian beam /
- slab laser /
- large mode volume /
- tilt control /
- power scaling
[1] Kogelnik H, Li T 1996 Appl. Opt. 5 1550
Google Scholar
[2] Sayan Ö F, Gerçekcioğlu H, Baykal Y 2020 Opt. Commun. 458 124735
Google Scholar
[3] Meyrath T P, Schreck F, Hanssen J L, Chuu C S, Raizen M G 2005 Opt. Express 13 2843
Google Scholar
[4] Wadhwa J, Singh A 2019 Phys. Plasmas 26 062118
Google Scholar
[5] Ghotra H S, Jaroszynski D, Ersfeld B, Saini N S, Yoffe S, Kant N 2018 Laser Part. Beams 36 154
Google Scholar
[6] Beijersbergen M W, Allen L, van der Veen H E L O, Woerdman J P 1993 Opt. Commun. 96 123
Google Scholar
[7] Chu S C, Ohtomo T, Otsuka K 2008 Appl. Opt. 47 2583
Google Scholar
[8] Ohtomo T, Chu S C, Otsuka K 2008 Opt. Express 16 5082
Google Scholar
[9] Shen Y, Meng Y, Fu X, Gong M 2018 Opt. Lett. 43 291
Google Scholar
[10] Coullet P, Gil L, Rocca F 1989 Opt. Commun. 73 403
Google Scholar
[11] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Phys. Rev. A 45 8185
Google Scholar
[12] Romero J, Leach J, Jack B, Dennis M R, Franke-Arnold S, Barnett S M, Padgett M J 2011 Phys. Rev. Lett. 106 100407
Google Scholar
[13] Gecevičius M, Drevinskas R, Beresna M, Kazansky P G 2014 Appl. Phys. Lett. 104 231110
Google Scholar
[14] Hamazaki J, Morita R, Chujo K, Kobayashi Y, Tanda S, Omatsu T 2010 Opt. Express 18 2144
Google Scholar
[15] Gu Y, Gbur G 2010 Opt. Commun. 283 1209
Google Scholar
[16] Ohtomo T, Kamikariya K, Otsuka K, Chu S C 2007 Opt. Express 15 10705
Google Scholar
[17] 连天虹, 王石语, 寇科, 刘芸 2020 69 114202
Google Scholar
Lian T H, Wang S Y, Kou K, Liu Y 2020 Acta Phys. Sin. 69 114202
Google Scholar
[18] Chu S C, Chen Y T, Tsai K F, Otsuka K 2012 Opt. Express 20 7128
Google Scholar
[19] Delaubert V, Shaddock D A, Lam P K, Buchler B C, Bachor H A, McClelland D E 2002 J. Opt. A: Pure Appl. Opt. 4 393
Google Scholar
[20] Ma L, Guo H, Sun H, Liu K, Su B, Gao J 2020 Photonics Res. 8 1422
Google Scholar
[21] Hu A, Lei J, Chen P, Wang Y, Li S 2014 Appl. Opt. 53 7845
Google Scholar
[22] Li S, Guo Y D, Chen Z Z, Zhang L, Gong K L, Zhang Z F, Xu Z Y 2019 Chin. Phys. Lett. 36 044204
Google Scholar
[23] Boyd G D, Gordon J P 1961 Bell Syst. Tech. J. 40 489
Google Scholar
[24] Carter W H 1980 Appl. Opt. 19 1027
Google Scholar
[25] Zhang L, Guo Y D, Chen Z Z, Gong K L, Xu J L, Yuan L, Lin Y Y, Meng S, Li Y, Shao C F, Li S, Zhang Z F, Bo Y, Peng Q J, Cui D F, Xu Z Y 2019 IEEE Photonics Technol. Lett. 31 405
Google Scholar
[26] McCumber D E 1965 Bell Syst. Tech. J. 44 333
Google Scholar
[27] Freiberg R J, Halsted A S 1969 Appl. Opt. 8 355
Google Scholar
[28] Benesty J, Chen J, Huang Y, Cohen I 2009 Noise Reduction in Speech Processing (Berlin: Springer) pp1–4
[29] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Physical Review A 45 8185
[30] 丁攀峰, 蒲继雄 2011 60 094204
Google Scholar
Ding P F, Pu J X 2011 Acta Phys. Sin. 60 094204
Google Scholar
-
图 1 大面泵浦Nd:YAG板条激光器产生一维厄米-高斯模式输出装置示意图
Figure 1. Experimental setup for the generation of one-dimensional Hermite-Gaussian modes based on face-pumped Nd:YAG slab laser.
图 2 测量的高阶HG模式光斑强度分布及其沿中心轴线强度与理论强度的比较,
$ \rho $ 是各阶模式实验值与理论值的相关系数Figure 2. Measured intensity distributions of different high order HG mode, corresponding intensity distribution curves along the central axis and the calculation curves.
$ \rho $ is the correlation coefficient between the experimental and theoretical values of each mode.
图 3 (a)—(c) 测量的典型 HG 模式光斑直径与传输距离的关系; (d) 不同HG模式光束质量
$ {M}^{2} $ 因子测量值与理论计算值对比Figure 3. (a)–(c) Beam diameter of typical HG modes versus the transmission distance; (d) the comparison of experimental and theoretical values of the beam quality factor
$ {M}^{2} $ for different HG modes.
图 4 输入厄米-高斯模式产生拉盖尔-高斯模式输出装置的构造
Figure 4. Experimental setup for generating Laguerre-Gaussian mode by inputting Hermite - Gaussian modes.
图 5 测量的各阶LG模式的光斑,
$ {r}_{n}/{r}_{1} $ 是 LG0n 与 LG01 模式光斑半径比值Figure 5. Measured beam spots of different LG modes,
$ {r}_{n}/{r}_{1} $ is the spot radius ratio between LG0n and LG01 mode.表 1 各阶HG模式半径的计算理论值(
${w}_{ {n}\rm{s}}$ ), 对应的光阑实际宽度($ {D}_{x} $ ,$ {D}_{y} $ ), OC的俯仰角($ \theta $ )和功率(P)Table 1. Calculated radius (
$ {w}_{n\rm{s}} $ ) of different order HG mode, the corresponding width of the aperture ($ {D}_{x}, {D}_{y} $ ), pitch angle (θ) of OC and power (P).Mode HG00 HG01 HG02 HG03 HG04 HG05 HG06 HG07 HG08 HG09 Dx/mm 1.5 wns/mm 0.52 0.90 1.16 1.38 1.56 1.72 1.87 2.01 2.14 2.27 Dy/mm 1.5 2.0 2.4 2.7 3.2 3.5 4.0 4.2 4.5 5.0 θ/μrad 0 101.5 23.6 18.2 96.1 32.7 96.1 76.2 58.1 142.7 P/mW 213 215 223 232 239 235 241 237 242 244 
DownLoad: CSV
-
[1] Kogelnik H, Li T 1996 Appl. Opt. 5 1550
Google Scholar
[2] Sayan Ö F, Gerçekcioğlu H, Baykal Y 2020 Opt. Commun. 458 124735
Google Scholar
[3] Meyrath T P, Schreck F, Hanssen J L, Chuu C S, Raizen M G 2005 Opt. Express 13 2843
Google Scholar
[4] Wadhwa J, Singh A 2019 Phys. Plasmas 26 062118
Google Scholar
[5] Ghotra H S, Jaroszynski D, Ersfeld B, Saini N S, Yoffe S, Kant N 2018 Laser Part. Beams 36 154
Google Scholar
[6] Beijersbergen M W, Allen L, van der Veen H E L O, Woerdman J P 1993 Opt. Commun. 96 123
Google Scholar
[7] Chu S C, Ohtomo T, Otsuka K 2008 Appl. Opt. 47 2583
Google Scholar
[8] Ohtomo T, Chu S C, Otsuka K 2008 Opt. Express 16 5082
Google Scholar
[9] Shen Y, Meng Y, Fu X, Gong M 2018 Opt. Lett. 43 291
Google Scholar
[10] Coullet P, Gil L, Rocca F 1989 Opt. Commun. 73 403
Google Scholar
[11] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Phys. Rev. A 45 8185
Google Scholar
[12] Romero J, Leach J, Jack B, Dennis M R, Franke-Arnold S, Barnett S M, Padgett M J 2011 Phys. Rev. Lett. 106 100407
Google Scholar
[13] Gecevičius M, Drevinskas R, Beresna M, Kazansky P G 2014 Appl. Phys. Lett. 104 231110
Google Scholar
[14] Hamazaki J, Morita R, Chujo K, Kobayashi Y, Tanda S, Omatsu T 2010 Opt. Express 18 2144
Google Scholar
[15] Gu Y, Gbur G 2010 Opt. Commun. 283 1209
Google Scholar
[16] Ohtomo T, Kamikariya K, Otsuka K, Chu S C 2007 Opt. Express 15 10705
Google Scholar
[17] 连天虹, 王石语, 寇科, 刘芸 2020 69 114202
Google Scholar
Lian T H, Wang S Y, Kou K, Liu Y 2020 Acta Phys. Sin. 69 114202
Google Scholar
[18] Chu S C, Chen Y T, Tsai K F, Otsuka K 2012 Opt. Express 20 7128
Google Scholar
[19] Delaubert V, Shaddock D A, Lam P K, Buchler B C, Bachor H A, McClelland D E 2002 J. Opt. A: Pure Appl. Opt. 4 393
Google Scholar
[20] Ma L, Guo H, Sun H, Liu K, Su B, Gao J 2020 Photonics Res. 8 1422
Google Scholar
[21] Hu A, Lei J, Chen P, Wang Y, Li S 2014 Appl. Opt. 53 7845
Google Scholar
[22] Li S, Guo Y D, Chen Z Z, Zhang L, Gong K L, Zhang Z F, Xu Z Y 2019 Chin. Phys. Lett. 36 044204
Google Scholar
[23] Boyd G D, Gordon J P 1961 Bell Syst. Tech. J. 40 489
Google Scholar
[24] Carter W H 1980 Appl. Opt. 19 1027
Google Scholar
[25] Zhang L, Guo Y D, Chen Z Z, Gong K L, Xu J L, Yuan L, Lin Y Y, Meng S, Li Y, Shao C F, Li S, Zhang Z F, Bo Y, Peng Q J, Cui D F, Xu Z Y 2019 IEEE Photonics Technol. Lett. 31 405
Google Scholar
[26] McCumber D E 1965 Bell Syst. Tech. J. 44 333
Google Scholar
[27] Freiberg R J, Halsted A S 1969 Appl. Opt. 8 355
Google Scholar
[28] Benesty J, Chen J, Huang Y, Cohen I 2009 Noise Reduction in Speech Processing (Berlin: Springer) pp1–4
[29] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Physical Review A 45 8185
[30] 丁攀峰, 蒲继雄 2011 60 094204
Google Scholar
Ding P F, Pu J X 2011 Acta Phys. Sin. 60 094204
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4153
- PDF Downloads: 104
- Cited By: 0
