-
In the process of laser propagation in atmosphere, its coherence characteristics are reduced by turbulence, which further affects the efficiency and performance of coherent detection process. In this paper, atmospheric coherence time is defined to describe the fluctuation speed of laser field after atmospheric transmission. The relative size of atmospheric coherence time and coherence process duration time determines the performance of coherent detection process. According to the infinitely long phase screen technology, we simulate laser atmospheric transmission, and systematically study the influences of atmospheric parameters, transceiver parameters and wavelength on atmospheric coherence time. It is found that the atmospheric coherence time is positively correlated with wavelength, receiving aperture and atmospheric coherence length, and inversely proportional to wind speed, which shows that the atmospheric coherence time can be effectively improved by improving the optical design and changing the laser band, thus effectively reducing the disturbance caused by turbulence and improving the stability of the received light field. The atmospheric coherence time defined in this paper is an important parameter to measure the influence of turbulence on coherent detection. This study can provide a powerful reference for evaluating the influence of atmosphere on coherent detection process.
-
Keywords:
- coherent detection /
- atmospheric turbulence /
- atmospheric coherence time /
- infinitely long phase screen
[1] Zhao Y Y, Zhu D S, Tu Y R, Pi L L, Li H T, Xu L, Hu Zh J, Shen Y C, Yu B L, Lu L 2021 Opt. Lett. 46 1229
 Google Scholar
Google Scholar
[2] Redding B, Choma M A, Cao H 2012 Nat. Photon. 6 355
 Google Scholar
Google Scholar
[3] 黄龙, 冯国英, 廖宇 2015 红外与激光工程 44 3530
 Google Scholar
Google Scholar
Huang L, Feng G Y, Liao Y 2015 Infrared Laser Eng. 44 3530
 Google Scholar
Google Scholar
[4] Semjon S, Mark G, Werner R 2016 Opt. Eng. 55 111614
[5] 曾素娟, 蓝银涛, 高伟健, 黄文燕, 舒焱, 葛立宏, 张建 2021 激光生物学报 30 131
 Google Scholar
Google Scholar
Zeng S J, Lan Y T, Gao W J, Huang W Y, Shu Y, Ge L H, Zhang J 2021 Acta. Laser Bio. Sinc. 30 131
 Google Scholar
Google Scholar
[6] Ali R 2021 Ultrasonic Imaging. 43 282
 Google Scholar
Google Scholar
[7] 张羽, 罗秀娟, 刘辉, 陈明徕 2018 67 044201
 Google Scholar
Google Scholar
Zhang Y, Luo X J, Liu H, Chen M L 2018 Acta Phys. Sin. 67 044201
 Google Scholar
Google Scholar
[8] 陈晓文, 汤明玥, 季小玲 2008 4 2607
 Google Scholar
Google Scholar
Chen X W, Tang M Y, Ji X L 2008 Acta Phys. Sin. 4 2607
 Google Scholar
Google Scholar
[9] 陈晓文, 季小玲 2009 58 2435
 Google Scholar
Google Scholar
Chen X W, Ji X L 2009 Acta Phys. Sin. 58 2435
 Google Scholar
Google Scholar
[10] 季小玲, 肖希, 吕百达 2004 53 3996
 Google Scholar
Google Scholar
Ji X L, Xiao X, Lü B D 2004 Acta Phys. Sin. 53 3996
 Google Scholar
Google Scholar
[11] 王华, 王向朝, 曾爱军, 杨坤 2008 57 634
 Google Scholar
Google Scholar
Wang H, Wang X C, Zeng A J, Yang K 2008 Acta Phys. Sin. 57 634
 Google Scholar
Google Scholar
[12] 任建迎, 孙华燕, 赵延仲, 张来线 2020 中国光学 13 728
 Google Scholar
Google Scholar
Ren J Y, Sun H Y, Zhao T Z, Zhang L X 2020 Chin. Opt. 13 728
 Google Scholar
Google Scholar
[13] 王华, 王向朝, 曾爱军 2007 光学学报 27 1548
 Google Scholar
Google Scholar
Wang H, Wang X C, Zeng A J 2007 Acta Optic Sin. 27 1548
 Google Scholar
Google Scholar
[14] 饶瑞中 2005 光在湍流大气中的传播 (合肥: 安徽科学技术出版社) 第95页
Rao R Z 2005 Light Propagation in the Turbulent Atmosphere (Hefei: Anhui Science & Technology Press) p95 (in Chinese)
[15] McGlamery B L 1976 Proc. SPIE. 0074 954724
 Google Scholar
Google Scholar
[16] Sedmak G 2004 Appl. Opt. 38 2161
 Google Scholar
Google Scholar
[17] 吴晗玲, 严海星, 李新阳 2009 光学学报 129 114
Wu H L, Yan H X, Li X Y 2009 Acta. Opt. Sinc. 129 114
[18] Roddier N A 1990 Opt. Eng. 29 1174
 Google Scholar
Google Scholar
[19] Harding C M, Johnston R A, Lane R G 1999 Appl. Opt. 38 2161
 Google Scholar
Google Scholar
[20] Assémat F, Wilson R W, Gendron E 2006 Opt. Express. 14 988
 Google Scholar
Google Scholar
[21] Roddier F 1981 Prog. Optics 19 281
 Google Scholar
Google Scholar
[22] Ziad A, Borgnino J, Martin F, Maire J, Mourad D 2010 Proc. SPIE. 7733 857259
 Google Scholar
Google Scholar
[23] Fried D L 1965 J. Opt. Soc. Am. 55 1427
 Google Scholar
Google Scholar
[24] Press W H, Teukolsky S A, Vetterling W T, Flannery B P 2003 Eur. J. Phys. 24 329
 Google Scholar
Google Scholar
[25] Andrews L C, Phillips R L 2005 SPIE Press. 201 250
 Google Scholar
Google Scholar
[26] Wolf E 1954 Nuovo. Cimento. 12 884
 Google Scholar
Google Scholar
[27] Longuet-Higgins H C, Roberts M De V 1955 Pro. Roy. Soc. A 230 110
 Google Scholar
Google Scholar
[28] Beckers J M 1993 Annu. Rev. Astron. Astr. 10 1146
 Google Scholar
Google Scholar
-
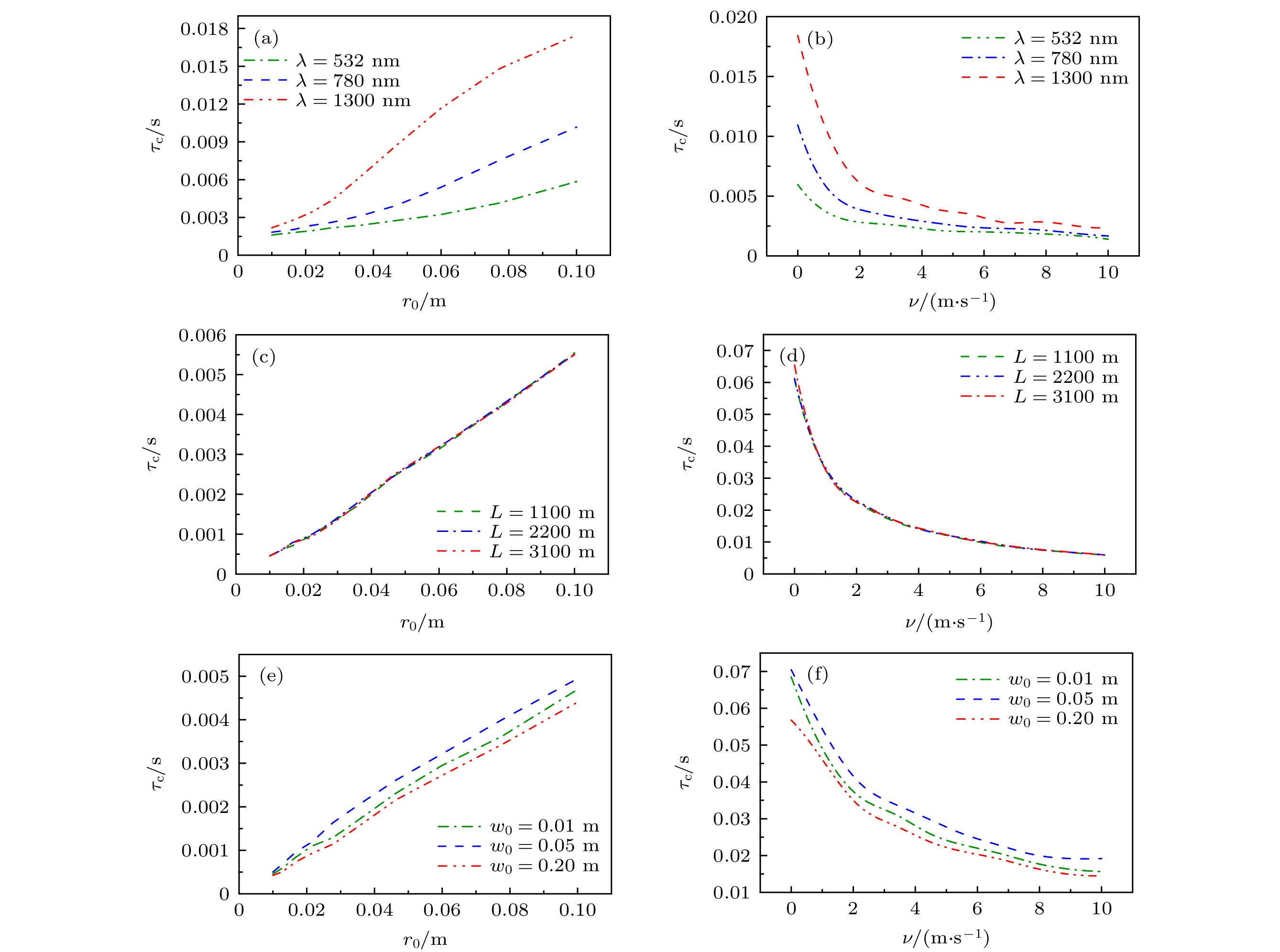
图 3 大气相干时间随大气相干长度与风速的变化 (a) L = 1100 m,
${w_0} = 0.1\;{\text{ m}}$ ,$\nu = 10 \;{\rm{m/s}}$ ; (b)$L = 1100\;{\text{ m}}$ ,${w_0} = 0.1\;{\text{ m}}$ ,${r_0} = 0.1\;{\text{ m}}$ ; (c)$\lambda = 532\;{\text{ nm}}$ ,${w_0} = 0.1\;{\text{ m}}$ ,$\nu = 10 \;{\rm{m/s}}$ ; (d)$\lambda = 532\;{\text{ nm}}$ ,${w_0} = 0.1\;{\text{ m}}$ ,${r_0} = 0.1\;{\text{ m}}$ ; (e)$L = 1100\;{\text{ m}}$ ,$\lambda = 532\;{\text{ nm}}$ ,$\nu = 10\;{\text{ }}{{\text{m}} \mathord{\left/ {\vphantom {{\text{m}} {\text{s}}}} \right. } {\text{s}}}$ ; (f)$L = 1100\;{\text{ m}}$ ,$\lambda = 532\;{\text{ nm}}$ ,${r_0} = 0.1\;{\text{ m}}$ Figure 3. Atmospheric coherent time varies with atmospheric coherence length and wind speeds: (a)
$L = 1100\;{\text{ m}}$ ,${w_0} = 0.1\;{\text{ m}}$ ,$\nu = 10 \;{\rm{m/s}}$ ; (b)$L = 1100\;{\text{ m}}$ ,${w_0} = 0.1\;{\text{ m}}$ ,${r_0} = 0.1\;{\text{ m}}$ ; (c)$\lambda = 532\;{\text{ nm}}$ ,${w_0} = 0.1\;{\text{ m}}$ ,$\nu = 10 \;{\rm{m/s}}$ ; (d)$\lambda = 532\;{\text{ nm}}$ ,${w_0} = 0.1\;{\text{ m}}$ ,${r_0} = 0.1\;{\text{ m}}$ ; (e)$L = 1100\;{\text{ m}}$ ,$\lambda = 532\;{\text{ nm}}$ ,$\nu = 10\;{\text{ }}{{\text{m}} \mathord{\left/ {\vphantom {{\text{m}} {\text{s}}}} \right. } {\text{s}}}$ ; (f)$L = 1100\;{\text{ m}}$ ,$\lambda = 532\;{\text{ nm}}$ ,${r_0} = 0.1\;{\text{ m}}$ .图 4 大气相干时间随束腰半径变化 (a)
$L = 1100\;{\text{ m}}$ ,$\nu = 10 \;{\rm{m/s}}$ ,$\lambda = 532\;{\text{ nm}}$ ,$D = 0.5\;{\text{ m}}$ ; (b)$\nu = 10 \;{\rm{m/s}}$ ,${r_0} = 0.1\;{\text{ m}}$ ,$\lambda = 532\;{\text{ nm}}$ ,$D = 0.5\;{\text{ m}}$ ; (c)$L = 1100\;{\text{ m}}$ ,$\nu = 10 \;{\rm{m/s}}$ ,${r_0} = 0.1\;{\text{ m}}$ ,$\lambda = 532\;{\text{ nm}}$ ; (d)$\nu = 10 \;{\rm{m/s}}$ ,${r_0} = 0.1\;{\text{ m}}$ ,$L = 1100\;{\text{ m}}$ ,$\lambda = 532\;{\text{ nm}}$ Figure 4. Atmospheric coherent time varies with beam waist radius: (a)
$L = 1100\;{\text{ m}}$ ,$\nu = 10 \;{\rm{m/s}}$ ,$\lambda = 532\;{\text{ nm}}$ ,$D = 0.5\;{\text{ m}}$ ; (b)$\nu = 10 \;{\rm{m/s}}$ ,${r_0} = 0.1\;{\text{ m}}$ ,$\lambda = 532\;{\text{ nm}}$ ,$D = 0.5\;{\text{ m}}$ ; (c)$L = 1100\;{\text{ m}}$ ,$\nu = 10 \;{\rm{m/s}}$ ,${r_0} = 0.1\;{\text{ m}}$ ,$\lambda = 532\;{\text{ nm}}$ ; (d)$\nu = 10 \;{\rm{m/s}}$ ,${r_0} = 0.1\;{\text{ m}}$ ,$L = 1100\;{\text{ m}}$ ,$\lambda = 532\;{\text{ nm}}$ 图 5
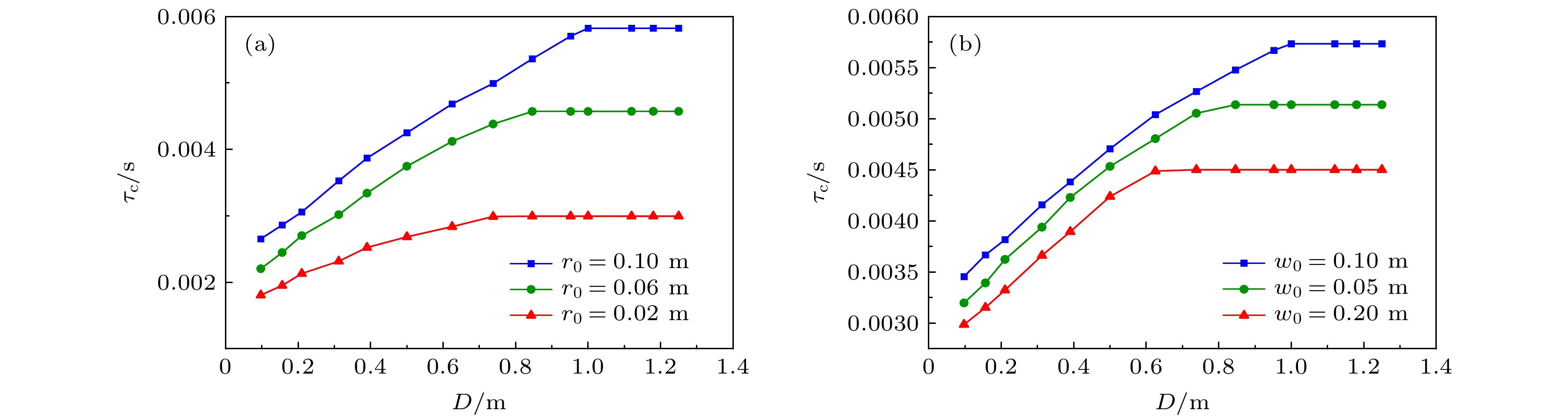
$\nu = 10 \;{\rm{m/s}} , L=1100\;\text{ m }, \lambda =532\;\text{ nm}$ , 大气相干时间随接收口径的变化 (a)${w_0} = 0.1\;{\text{ m}}$ ; (b)${r_0} = 0.1\;{\text{ m }}$ Figure 5. Atmospheric coherent time varies with receiving aperture at
$\nu = 10 \;{\rm{m/s}} , L=1100\;\text{ m }, \lambda =532\;\text{ nm}$ : (a)${w_0} = 0.1\;{\text{ m}}$ ; (b)${r_0} = 0.1\;{\text{ m }}$ -
[1] Zhao Y Y, Zhu D S, Tu Y R, Pi L L, Li H T, Xu L, Hu Zh J, Shen Y C, Yu B L, Lu L 2021 Opt. Lett. 46 1229
 Google Scholar
Google Scholar
[2] Redding B, Choma M A, Cao H 2012 Nat. Photon. 6 355
 Google Scholar
Google Scholar
[3] 黄龙, 冯国英, 廖宇 2015 红外与激光工程 44 3530
 Google Scholar
Google Scholar
Huang L, Feng G Y, Liao Y 2015 Infrared Laser Eng. 44 3530
 Google Scholar
Google Scholar
[4] Semjon S, Mark G, Werner R 2016 Opt. Eng. 55 111614
[5] 曾素娟, 蓝银涛, 高伟健, 黄文燕, 舒焱, 葛立宏, 张建 2021 激光生物学报 30 131
 Google Scholar
Google Scholar
Zeng S J, Lan Y T, Gao W J, Huang W Y, Shu Y, Ge L H, Zhang J 2021 Acta. Laser Bio. Sinc. 30 131
 Google Scholar
Google Scholar
[6] Ali R 2021 Ultrasonic Imaging. 43 282
 Google Scholar
Google Scholar
[7] 张羽, 罗秀娟, 刘辉, 陈明徕 2018 67 044201
 Google Scholar
Google Scholar
Zhang Y, Luo X J, Liu H, Chen M L 2018 Acta Phys. Sin. 67 044201
 Google Scholar
Google Scholar
[8] 陈晓文, 汤明玥, 季小玲 2008 4 2607
 Google Scholar
Google Scholar
Chen X W, Tang M Y, Ji X L 2008 Acta Phys. Sin. 4 2607
 Google Scholar
Google Scholar
[9] 陈晓文, 季小玲 2009 58 2435
 Google Scholar
Google Scholar
Chen X W, Ji X L 2009 Acta Phys. Sin. 58 2435
 Google Scholar
Google Scholar
[10] 季小玲, 肖希, 吕百达 2004 53 3996
 Google Scholar
Google Scholar
Ji X L, Xiao X, Lü B D 2004 Acta Phys. Sin. 53 3996
 Google Scholar
Google Scholar
[11] 王华, 王向朝, 曾爱军, 杨坤 2008 57 634
 Google Scholar
Google Scholar
Wang H, Wang X C, Zeng A J, Yang K 2008 Acta Phys. Sin. 57 634
 Google Scholar
Google Scholar
[12] 任建迎, 孙华燕, 赵延仲, 张来线 2020 中国光学 13 728
 Google Scholar
Google Scholar
Ren J Y, Sun H Y, Zhao T Z, Zhang L X 2020 Chin. Opt. 13 728
 Google Scholar
Google Scholar
[13] 王华, 王向朝, 曾爱军 2007 光学学报 27 1548
 Google Scholar
Google Scholar
Wang H, Wang X C, Zeng A J 2007 Acta Optic Sin. 27 1548
 Google Scholar
Google Scholar
[14] 饶瑞中 2005 光在湍流大气中的传播 (合肥: 安徽科学技术出版社) 第95页
Rao R Z 2005 Light Propagation in the Turbulent Atmosphere (Hefei: Anhui Science & Technology Press) p95 (in Chinese)
[15] McGlamery B L 1976 Proc. SPIE. 0074 954724
 Google Scholar
Google Scholar
[16] Sedmak G 2004 Appl. Opt. 38 2161
 Google Scholar
Google Scholar
[17] 吴晗玲, 严海星, 李新阳 2009 光学学报 129 114
Wu H L, Yan H X, Li X Y 2009 Acta. Opt. Sinc. 129 114
[18] Roddier N A 1990 Opt. Eng. 29 1174
 Google Scholar
Google Scholar
[19] Harding C M, Johnston R A, Lane R G 1999 Appl. Opt. 38 2161
 Google Scholar
Google Scholar
[20] Assémat F, Wilson R W, Gendron E 2006 Opt. Express. 14 988
 Google Scholar
Google Scholar
[21] Roddier F 1981 Prog. Optics 19 281
 Google Scholar
Google Scholar
[22] Ziad A, Borgnino J, Martin F, Maire J, Mourad D 2010 Proc. SPIE. 7733 857259
 Google Scholar
Google Scholar
[23] Fried D L 1965 J. Opt. Soc. Am. 55 1427
 Google Scholar
Google Scholar
[24] Press W H, Teukolsky S A, Vetterling W T, Flannery B P 2003 Eur. J. Phys. 24 329
 Google Scholar
Google Scholar
[25] Andrews L C, Phillips R L 2005 SPIE Press. 201 250
 Google Scholar
Google Scholar
[26] Wolf E 1954 Nuovo. Cimento. 12 884
 Google Scholar
Google Scholar
[27] Longuet-Higgins H C, Roberts M De V 1955 Pro. Roy. Soc. A 230 110
 Google Scholar
Google Scholar
[28] Beckers J M 1993 Annu. Rev. Astron. Astr. 10 1146
 Google Scholar
Google Scholar
Catalog
Metrics
- Abstract views: 6339
- PDF Downloads: 159
- Cited By: 0














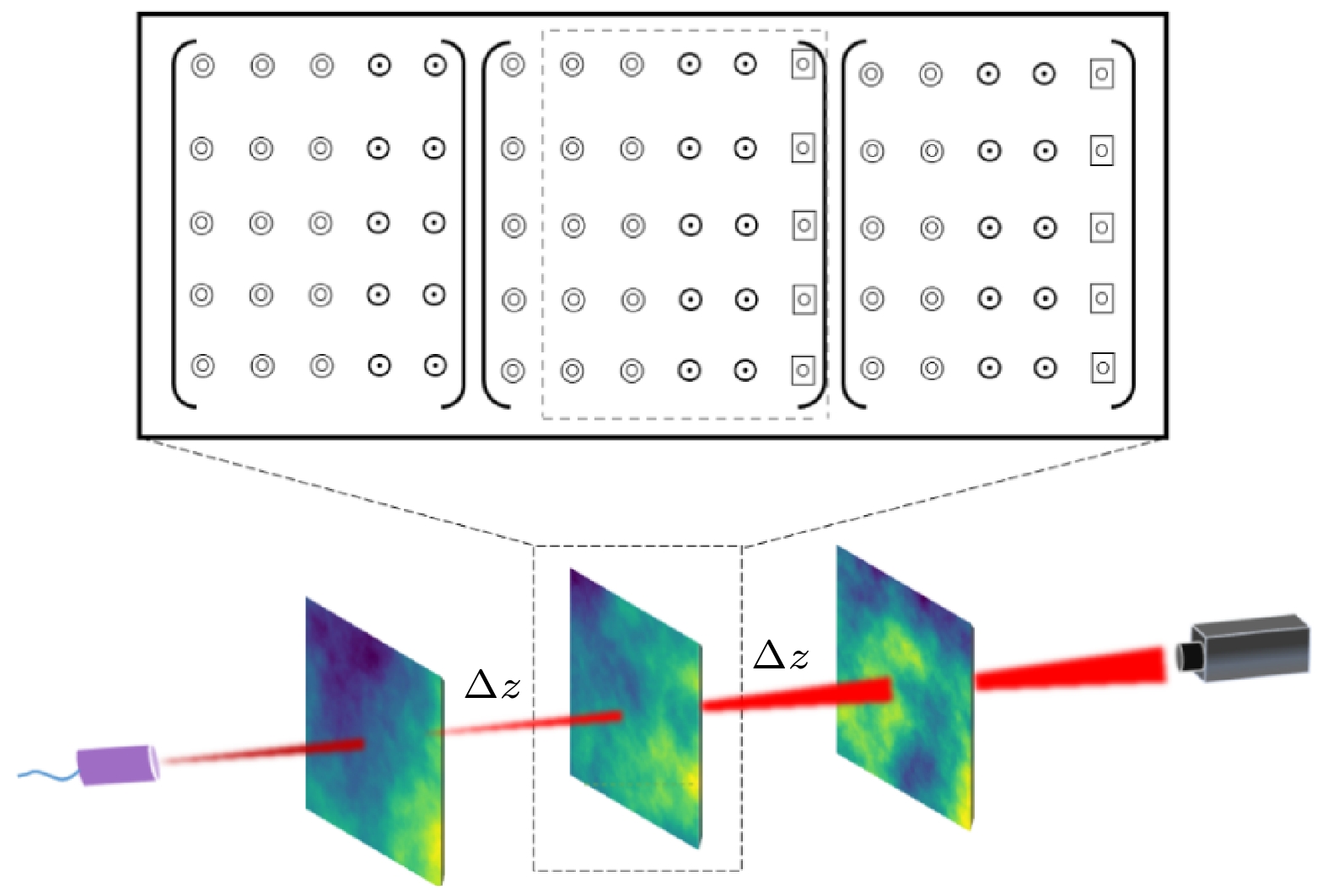
 DownLoad:
DownLoad: