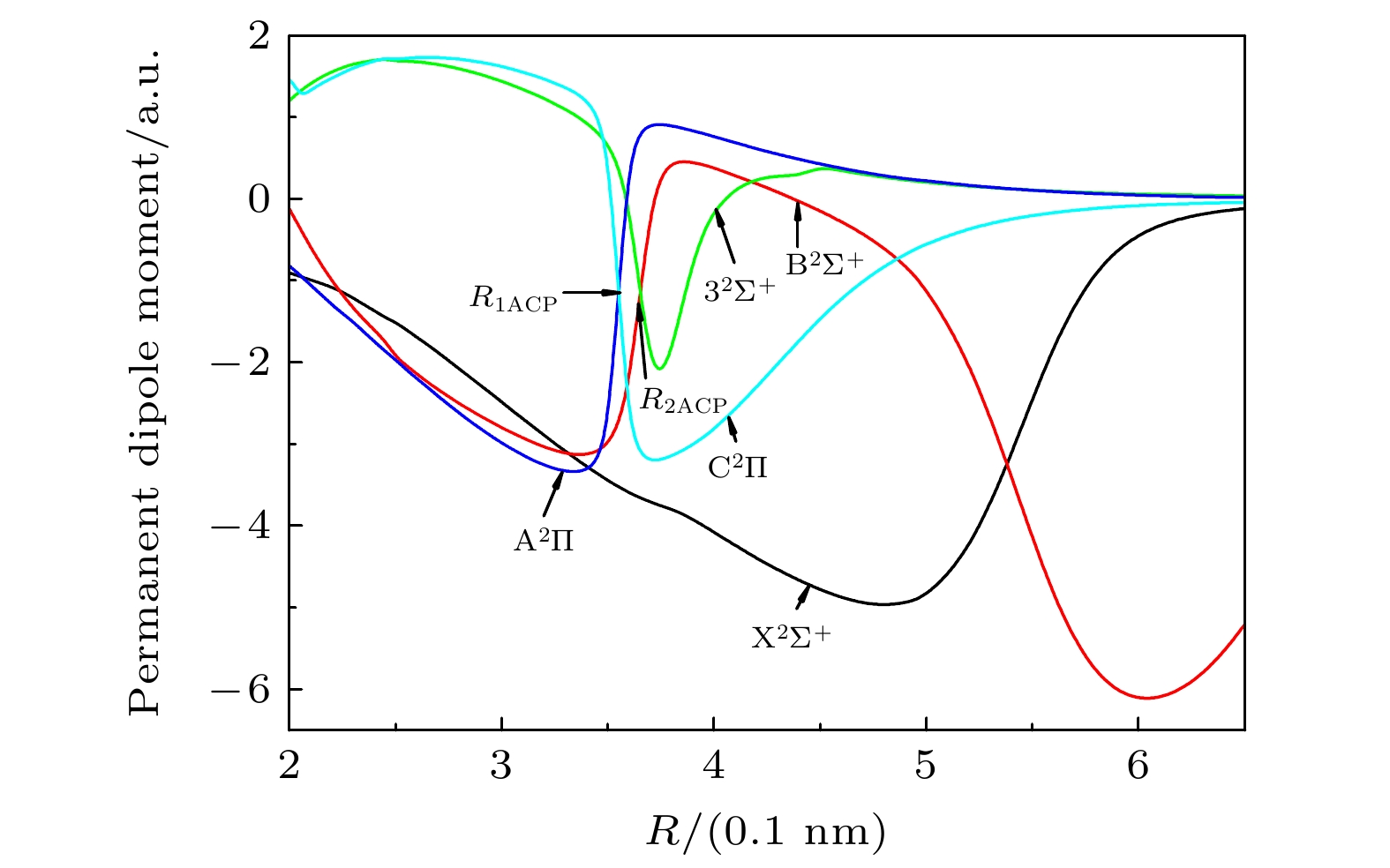
-
The electronic structures and single point energy of 14 lowest electronic states of 88Sr79Br molecule are optimized by using the internal contraction multi-reference configuration interaction method and relativistic effective core pseudo-potential basis. Because 88Sr79Br molecule belongs to heavy element system, the single point energy must be corrected to obtain more accurate spectral parameters. Therefore, Davidson is introduced to correct the energy inconsistency, nuclear valence correlation is used to correct the electron correlation effect of inner shell and valence shell, and the relativistic scalar effect is corrected by calculating the third-order Douglas-Kroll-Hess Hamilton single electron integral. According to the single point energy calculated by the modified optimization, the potential energy curves, electric dipole moments, and transition dipole moments of 14 lowest electronic states are obtained. Using the latest LEVEL8.0 program to fit the modified potential energy curve, the spectral constants, molecular constants and vibration energy levels of 5 lowest bound states of 88Sr79Br molecule are given. In order to explain the changing trend of spectral constants of homologous compounds, the spectral parameters of each compound are compared and analyzed in this paper. At the same time, the vibration energy levels and molecular constants of 88Sr81Br molecule are also fitted and calculated for analyzing the influence of isotopes. The comparative analysis shows that the results of 88Sr79Br molecule are in better agreement with the experimental values. Finally, the Franck-Condon factors are gained by fitting the optimized single point energy and transition dipole moment of 88Sr79Br molecule. The transition band with the largest factor and obvious diagonalization is selected by analyzing the Franck-Condon factor of each transition band, and whether it meets the conditions for selecting laser cooling molecular system is judged. The radiation lifetimes of the transitions from the lowest two excited states to the ground state are calculated by combining the transition dipole moment, Franck-Condon factor, single point energy and vibration energy level of each electronic state. The results of this paper are in good agreement with the experimental values, which shows that the method in this paper is reliable. These spectral characteristic parameters provide theoretical support for further experimental measurement and construction of molecular laser cooling scheme of 88Sr79Br molecule.
-
Keywords:
- 88Sr79Br molecule /
- ic-MRCI /
- spectroscopic and molecular constant /
- vibration levels
[1] Yang C L, Zhang X Y, Gao F, Ren T Q 2007 J. Mol. Struct. THEOCHEM 807 147
 Google Scholar
Google Scholar
[2] Wang C, Li N, Xia Y, Zhang X, Ge M, Liu Y, Li Q 2011 Comput. Theor. Chem. 963 319
 Google Scholar
Google Scholar
[3] Short C I, Hauschildt P H 2006 Astrophys. J. 641 494
 Google Scholar
Google Scholar
[4] Carlson K D, Claydon C R 1967 Adv. High Temp. Chem. 1 43
 Google Scholar
Google Scholar
[5] Hansen C J, Bergemann M, Cescutti G, Francois P, Arcones A, Karakas A I, Lind K, Chiappini C 2013 Astron. Astrophys. 551 1
 Google Scholar
Google Scholar
[6] Bergemann M, Hansen C J, Bautista M, Ruchti G 2012 Astron. Astrophys. 546 1
 Google Scholar
Google Scholar
[7] Caffau E, Andrievsky S, Korotin S, Origlia L, Oliva E, Sanna N, Ludwig H G, Bonifacio P 2016 Astron. Astrophys. 585 44
 Google Scholar
Google Scholar
[8] Törring T, Doebl K, Weiler G 1985 Chem. Phys. Lett. 117 539
 Google Scholar
Google Scholar
[9] Ernst W E, Schröder J O 1986 Z. Phys. D:At. Mol. Clusters 1 103
 Google Scholar
Google Scholar
[10] Keijzer F, Teule J M, Bulthuis J, de Graaff G J, Hilgeman M H, Janssen M H M, van Kleef E H, van Leuken J J, Stolte S 1996 Chem. Phys. 207 261
 Google Scholar
Google Scholar
[11] Coxon J A, Dickinson C S 1998 J. Mol. Spectrosc. 190 150
 Google Scholar
Google Scholar
[12] Gurvich L V, Ryabova V G, Khitrov A N 1973 Faraday Symp. Chem. Soc. 8 83
 Google Scholar
Google Scholar
[13] Hildenbrand D L 1977 J. Chem. Phys. 66 3526
 Google Scholar
Google Scholar
[14] Ernst W E, Schröder J O 1986 J. Mol. Spectrosc. 117 444
 Google Scholar
Google Scholar
[15] Dickinson C S, Coxon J A 2003 J. Mol. Spectrosc. 221 269
 Google Scholar
Google Scholar
[16] Schröder J O, Ernst W E 1985 J. Mol. Spectrosc. 112 413
 Google Scholar
Google Scholar
[17] Castano F, Sanchez Rayo M N, Pereira R, Adams J W, Husain D, Schifino J 1994 J. Photochem. Photobiol. , A 83 79
 Google Scholar
Google Scholar
[18] Gunduz S, Akman S 2014 Microchem. J. 116 1
 Google Scholar
Google Scholar
[19] Werner H J, Knowles P J, Knizia G, et al. 2012 MOLPRO, version 2012.1, a package of ab initio Programs
[20] Peterson K A, Figgen D, Goll E, Stoll H, Dolg M 2003 J. Chem. Phys. 119 11099
 Google Scholar
Google Scholar
[21] Werner H-J, Knowles P J 1985 J. Chem. Phys. 82 5053
 Google Scholar
Google Scholar
[22] Knowles P J, Werner H-J 1985 Chem. Phys. Lett. 115 259
 Google Scholar
Google Scholar
[23] Werner H-J, Knowles P J 1988 J. Chem. Phys. 89 5803
 Google Scholar
Google Scholar
[24] Knowles P J, Werner H-J 1988 Chem. Phys. Lett. 145 514
 Google Scholar
Google Scholar
[25] Le Roy R J 2007 LEVEL 8.0: A Computer Program for Solving the Radial Schrödinger Equation for Bound and Quasi-bound Levels (Waterloo: University of Waterloo) Chemical Physics Research Report CP-663
[26] Wu D L, Tan B, Wen Y F, Zeng X F, Xie A D, Yan B 2016 Spectrochim. Acta, Part A 161 101
 Google Scholar
Google Scholar
[27] Fu M K, Ma H T, Cao J W, Bian W S 2017 J. Chem. Phys. 146 134309
 Google Scholar
Google Scholar
[28] Adema Z, Makhlouf S, Taher F 2016 Comput. Theor. Chem. 1093 48
 Google Scholar
Google Scholar
[29] Liu L, Yang C L, Wang M S, Ma X G, Sun Z P 2019 Spectrochim. Acta, Part A 164 162
 Google Scholar
Google Scholar
[30] Huber K P, Herzberg G 1979 Constants of Diatonic Molecules, Molecular spectra molecular structure (Vol. IV) (NewYork: Van Nostrand Reinhold)
[31] Wu D L, Lin C Q, Wen Y F, Xie A D, Yan B 2017 Chin. Phys. B 594 083101
 Google Scholar
Google Scholar
[32] 魏长立, 梁桂颖, 刘晓婷, 颜培源, 闫冰 2016 65 163101
 Google Scholar
Google Scholar
Wei C L, Liang G Y, Liu X T, Yan P Y, Yan B 2016 Acta. Phys. Sin. 65 163101
 Google Scholar
Google Scholar
[33] Zhang X M, Liang G Y, Li R, Shi D D, Liu Y C, Liu X S, Xu H F, Yan B 2014 Chem. Phys. 443 142
 Google Scholar
Google Scholar
[34] Okabe H 1978 Photochemistry of Small Molecules (New York: Wiley-Interscience)
[35] Zou W L, Liu W J 2005 J. Comput. Chem. 26 106
 Google Scholar
Google Scholar
[36] Bahrini C, Augé-Rochereau F, Rostas J, Taïeb G 2006 Chem. Phys. 330 130
 Google Scholar
Google Scholar
-
表 1 5个束缚态的光谱常数
Table 1. The spectroscopic constants of the 5 lowest electronic states.
Λ-S 态 Te/cm–1 Re/nm ωe/cm–1 ωeχe/cm–1 Be/cm–1 αe/(10–4 cm–1) De/eV Re附近主要电子组态/% X2Σ+ 0.0 0.2740 216.17 0.499 0.0538 1.731 3.310 11σ212σα13σ06π47π2(80.2)
11σ212σ013σα6π47π2(6.9)理论[28] 0.0 0.2746 212.78 0.509 0.0535 — — 理论[29] 0.0 0.2799 205.6 0.53 0.051 1.742 — 实验[30] 0.0 0.2735 216 0.51 0.0541 — — A2Π 14679.348 0.2701 222.38 0.5346 0.0549 1.213 1.334 11σ212σ013σ06π47π3(86.1) 理论[28] 14657 0.272 220 0.57 0.0544 — — 理论[29] 14269.9 0.275 215.1 0.54 0.052 1.241 — 实验[30] 14850 0.2717 222 0.53 0.0545 — — B2Σ+ 15376.803 0.2702 223.03 0.5272 0.0552 1.799 1.597 11σ212σ013σα6π47π2(79.2)
11σ212σα13σ06π47π2(6.1)理论[28] 15208 0.2710 220.5 0.52 0.0547 — — 理论[29] 15222.8 0.2749 214.5 0.56 0.053 1.831 — 实验[30] 15352 0.2701 222 0.53 0.0552 — — C2Π 24947.818 0.3373 201.07 0.5012 0.0515 2.369 1.500
11σ212σ213σ06π37π3(80.0)
11σ212σ013σ06π47π3(2.2)
11σ212σ013σ06π37π4(2.8)
11σα12σα13σ06π47π3(2.7)理论[28] 25491 0.2810 197 0.49 0.0509 — — 理论[29] 25323.2 0.285 191.2 0.46 0.049 2.477 — 实验[30] 24665 — 205 0.49 — — — 32Σ+ 29079.756 0.3548 238.98 0.4556 0.0542 1.653 1.109 11σ212σα13σ06π47π2(1.3)
11σα12σ213σ06π47π2(69.4)
11σ212σ013σα6π47π2(5.3)
11σ212σα13σ06π37π3(2.9)
11σ212σ013σα6π47π1(.9)理论[28] 28117 0.266 242 0.54 0.0567 — — 理论[29] 27228.9 0.27 235.8 0.54 0.055 1.660 — 实验[30] 28958 — 247 0.55 — — — 表 2 88Sr79Br分子5个束缚态的Gν, Bν和Dν值
Table 2. The values of Gν, Bν and Dν of 5 lowest electronic states for 88Sr79Br molecule.
ν 0 1 2 3 4 5 6 7 8 9 X2Σ+ Gν/cm–1 0 217.21 422.31 648.92 863.52 1077.13 1289.84 1501.60 1712.39 1922.24 Bν/cm–1 0.054170 0.054003 0.053836 0.053663 0.053494 0.053330 0.053165 0.052997 0.052827 0.052659 Dν/(10–8 cm–1) 1.299301 1.303053 1.284638 1.297713 1.299115 1.295311 1.296435 1.298790 1.296092 1.292529 A2Π Gν/cm–1 14691.87 14934.23 15175.25 15415.96 15656.46 15896.12 16134.07 16369.73 16603.1 16834.89 Bν/cm–1 0.055780 0.055620 0.055462 0.055295 0.055124 0.054976 0.054861 0.054772 0.054687 0.054578 Dν/(10–8 cm–1) 1.347703 1.355108 1.331605 1.313371 1.331860 1.390480 1.454430 1.476056 1.452901 1.372534 B2Σ+ Gν/cm–1 15376.52 15507.84 15738.33 15967.89 16196.74 16425.06 16652.61 16878.97 17103.8 17327.04 Bν/cm–1 0.055152 0.054998 0.054842 0.054679 0.054501 0.054326 0.054170 0.054020 0.053860 0.053722 Dν/(10–8 cm–1) 1.383536 1.380925 1.382206 1.370045 1.352258 1.361286 1.370500 1.430538 1.454700 1.453644 C2Π Gν/cm–1 25067.11 25478.29 25768.5 25995.01 26202.12 26394.03 26575.33 26748.69 26915.65 27077.76 Bν/cm–1 0.051613 0.051929 0.052694 0.053118 0.0536173 0.053991 0.054389 0.054762 0.055104 0.055412 Dν/(10–8 cm–1) 7.342555 2.052993 4.137982 4.629906 5.880088 6.862856 7.6697626 8.6153136 9.298999 9.430722 32Σ+ Gν/cm–1 31178.79 31534.74 31825.03 32085.24 32324.03 32546.51 32756.77 32958.15 33153 33342.76 Bν/cm–1 0.052457 0.052986 0.053498 0.053999 0.054484 0.054959 0.055414 0.055843 0.056246 0.056634 Dν/(10–8 cm–1) 8.487942 1.681584 2.237826 2.904620 3.611947 4.250499 4.782100 5.233730 5.676720 6.069434 表 3 88Sr79Br分子A2Π–X2Σ+和B2Σ+–X2Σ+跃迁的Franck-Condon因子
Table 3. The Franck-Condon factors of the transitions A2Π–X2Σ+和B2Σ+–X2Σ+ of 88Sr79Br.
ν'' = 0 1 2 3 4 5 6 7 8 9 A2Π–X2Σ+ ν' = 0 0.645022 0.336688 0.098008 0.017772 0.002264 0.000225 0.000019 0.000001 0.000000 0.000000 1 0.436688 0.083076 0.303189 0.190372 0.661533 0.165378 0.003316 0.000568 0.000087 0.000011 2 0.098008 0.434973 0.000835 0.180970 0.223953 0.113214 0.036827 0.009054 0.001812 0.000305 3 0.017772 0.293991 0.318827 0.055875 0.070256 0.256239 0.170741 0.062531 0.018499 0.004301 4 0.002263 0.053921 0.247678 0.100225 0.125148 0.010981 0.177146 0.190350 0.089728 0.031718 5 0.000225 0.009392 0.099735 0.271659 0.024898 0.160484 0.001473 0.098532 0.170079 0.114744 6 0.000019 0.001159 0.022742 0.145408 0.236122 0.000076 0.155129 0.024834 0.046417 0.151117 7 0.000000 0.000110 0.003473 0.042832 0.181875 0.178132 0.014614 0.150355 0.062132 0.011646 8 0.000000 0.000008 0.000391 0.008050 0.068736 0.202633 0.095379 0.050504 0.073401 0.096387 9 0.000000 0.000000 0.000034 0.001075 0.015763 0.098088 0.204697 0.042685 0.089124 0.031198 B2Σ+–X2Σ+ ν' = 0 0.825605 0.238234 0.033347 0.002666 0.000142 0.000006 0.000000 0.000000 0.000000 0.000000 1 0.388234 0.634880 0.110814 0.094271 0.088490 0.062873 0.040384 0.000047 0.000006 0.000000 2 0.033347 0.414395 0.520552 0.090304 0.084878 0.077853 0.067309 0.051175 0.000165 0.000020 3 0.002666 0.083449 0.366773 0.423286 0.082800 0.072301 0.061320 0.014268 0.002657 0.000419 4 0.000142 0.009678 0.107691 0.317402 0.350042 0.097638 0.0602219 0.055584 0.023573 0.005001 5 0.000006 0.000654 0.052162 0.087692 0.280018 0.317641 0.083112 0.07925 0.068340 0.044978 6 0.000000 0.000000 0.000117 0.006341 0.098918 0.277382 0.309402 0.081296 0.063711 0.051334 7 0.000000 0.000000 0.000093 0.003861 0.057313 0.055610 0.245025 0.292709 0.036511 0.075513 8 0.000000 0.000000 0.000004 0.000245 0.007248 0.080579 0.069675 0.186559 0.227752 0.010265 9 0.000000 0.000000 0.000000 0.000012 0.000552 0.012263 0.036322 0.069989 0.121499 0.152774 表 4 88Sr79Br分子A2Π–X2Σ+和B2Σ+–X2Σ+跃迁的辐射寿命
Table 4. The radiative lifetimes of the transitions A2Π–X2Σ+ and B2Σ+–X2Σ+ of 88Sr79Br.
Transition Radiative lifetimes/ns ν′ = 0 ν′ = 1 ν′ = 2 A2Π–X2Σ+ 32.23 32.35 32.56 B2Σ+–X2Σ+ 40.93 40.95 41.22 -
[1] Yang C L, Zhang X Y, Gao F, Ren T Q 2007 J. Mol. Struct. THEOCHEM 807 147
 Google Scholar
Google Scholar
[2] Wang C, Li N, Xia Y, Zhang X, Ge M, Liu Y, Li Q 2011 Comput. Theor. Chem. 963 319
 Google Scholar
Google Scholar
[3] Short C I, Hauschildt P H 2006 Astrophys. J. 641 494
 Google Scholar
Google Scholar
[4] Carlson K D, Claydon C R 1967 Adv. High Temp. Chem. 1 43
 Google Scholar
Google Scholar
[5] Hansen C J, Bergemann M, Cescutti G, Francois P, Arcones A, Karakas A I, Lind K, Chiappini C 2013 Astron. Astrophys. 551 1
 Google Scholar
Google Scholar
[6] Bergemann M, Hansen C J, Bautista M, Ruchti G 2012 Astron. Astrophys. 546 1
 Google Scholar
Google Scholar
[7] Caffau E, Andrievsky S, Korotin S, Origlia L, Oliva E, Sanna N, Ludwig H G, Bonifacio P 2016 Astron. Astrophys. 585 44
 Google Scholar
Google Scholar
[8] Törring T, Doebl K, Weiler G 1985 Chem. Phys. Lett. 117 539
 Google Scholar
Google Scholar
[9] Ernst W E, Schröder J O 1986 Z. Phys. D:At. Mol. Clusters 1 103
 Google Scholar
Google Scholar
[10] Keijzer F, Teule J M, Bulthuis J, de Graaff G J, Hilgeman M H, Janssen M H M, van Kleef E H, van Leuken J J, Stolte S 1996 Chem. Phys. 207 261
 Google Scholar
Google Scholar
[11] Coxon J A, Dickinson C S 1998 J. Mol. Spectrosc. 190 150
 Google Scholar
Google Scholar
[12] Gurvich L V, Ryabova V G, Khitrov A N 1973 Faraday Symp. Chem. Soc. 8 83
 Google Scholar
Google Scholar
[13] Hildenbrand D L 1977 J. Chem. Phys. 66 3526
 Google Scholar
Google Scholar
[14] Ernst W E, Schröder J O 1986 J. Mol. Spectrosc. 117 444
 Google Scholar
Google Scholar
[15] Dickinson C S, Coxon J A 2003 J. Mol. Spectrosc. 221 269
 Google Scholar
Google Scholar
[16] Schröder J O, Ernst W E 1985 J. Mol. Spectrosc. 112 413
 Google Scholar
Google Scholar
[17] Castano F, Sanchez Rayo M N, Pereira R, Adams J W, Husain D, Schifino J 1994 J. Photochem. Photobiol. , A 83 79
 Google Scholar
Google Scholar
[18] Gunduz S, Akman S 2014 Microchem. J. 116 1
 Google Scholar
Google Scholar
[19] Werner H J, Knowles P J, Knizia G, et al. 2012 MOLPRO, version 2012.1, a package of ab initio Programs
[20] Peterson K A, Figgen D, Goll E, Stoll H, Dolg M 2003 J. Chem. Phys. 119 11099
 Google Scholar
Google Scholar
[21] Werner H-J, Knowles P J 1985 J. Chem. Phys. 82 5053
 Google Scholar
Google Scholar
[22] Knowles P J, Werner H-J 1985 Chem. Phys. Lett. 115 259
 Google Scholar
Google Scholar
[23] Werner H-J, Knowles P J 1988 J. Chem. Phys. 89 5803
 Google Scholar
Google Scholar
[24] Knowles P J, Werner H-J 1988 Chem. Phys. Lett. 145 514
 Google Scholar
Google Scholar
[25] Le Roy R J 2007 LEVEL 8.0: A Computer Program for Solving the Radial Schrödinger Equation for Bound and Quasi-bound Levels (Waterloo: University of Waterloo) Chemical Physics Research Report CP-663
[26] Wu D L, Tan B, Wen Y F, Zeng X F, Xie A D, Yan B 2016 Spectrochim. Acta, Part A 161 101
 Google Scholar
Google Scholar
[27] Fu M K, Ma H T, Cao J W, Bian W S 2017 J. Chem. Phys. 146 134309
 Google Scholar
Google Scholar
[28] Adema Z, Makhlouf S, Taher F 2016 Comput. Theor. Chem. 1093 48
 Google Scholar
Google Scholar
[29] Liu L, Yang C L, Wang M S, Ma X G, Sun Z P 2019 Spectrochim. Acta, Part A 164 162
 Google Scholar
Google Scholar
[30] Huber K P, Herzberg G 1979 Constants of Diatonic Molecules, Molecular spectra molecular structure (Vol. IV) (NewYork: Van Nostrand Reinhold)
[31] Wu D L, Lin C Q, Wen Y F, Xie A D, Yan B 2017 Chin. Phys. B 594 083101
 Google Scholar
Google Scholar
[32] 魏长立, 梁桂颖, 刘晓婷, 颜培源, 闫冰 2016 65 163101
 Google Scholar
Google Scholar
Wei C L, Liang G Y, Liu X T, Yan P Y, Yan B 2016 Acta. Phys. Sin. 65 163101
 Google Scholar
Google Scholar
[33] Zhang X M, Liang G Y, Li R, Shi D D, Liu Y C, Liu X S, Xu H F, Yan B 2014 Chem. Phys. 443 142
 Google Scholar
Google Scholar
[34] Okabe H 1978 Photochemistry of Small Molecules (New York: Wiley-Interscience)
[35] Zou W L, Liu W J 2005 J. Comput. Chem. 26 106
 Google Scholar
Google Scholar
[36] Bahrini C, Augé-Rochereau F, Rostas J, Taïeb G 2006 Chem. Phys. 330 130
 Google Scholar
Google Scholar
Catalog
Metrics
- Abstract views: 5327
- PDF Downloads: 62
- Cited By: 0














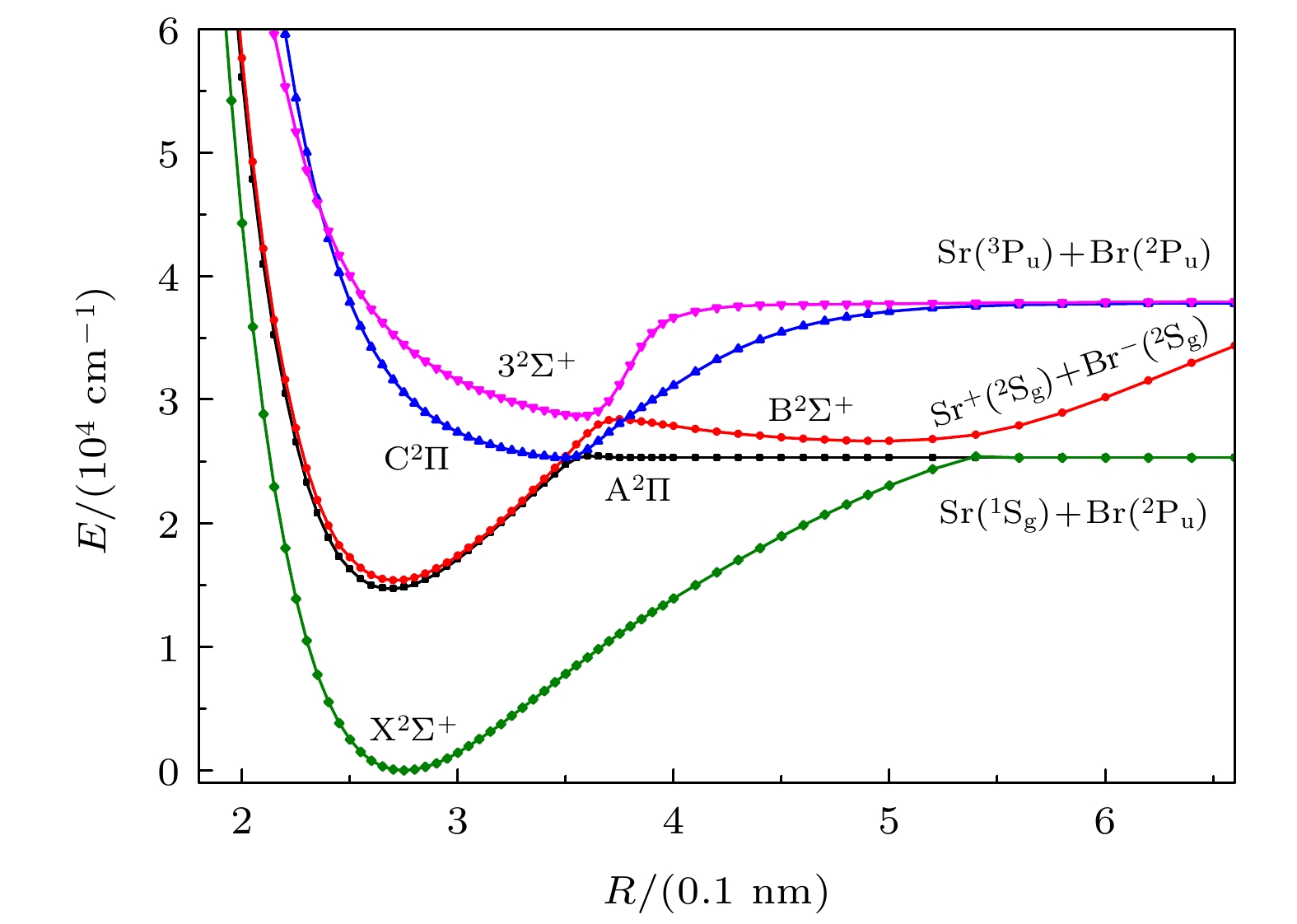
 DownLoad:
DownLoad: