-
In this paper, we theoretically study the influence of cubic nonlinearity effect on quadratic solitons in the boundary-constrained self-focusing oscillatory response function system. Based on the Newton iteration approach, we numerically solve the nonlinear coupled-wave equations with both quadratic and cubic nonlinearity. Moreover, a family of quadratic solitons is obtained. By comparing the quadratic solitons with both quadratic and cubic nonlinearity with those with only quadratic nonlinearity, we find that the cubic nonlinearity changes the transverse distribution of the soliton profiles only slightly. However, because of the existence of the cubic nonlinearity, quadratic solitons can be found only in the strongly nonlocal case and general nonlocal case, and they cannot be found in the weakly nonlocal case, in which the quadratic solitons with only quadratic nonlinearity can be found. In addition, the existence of cubic nonlinearity reduces the number of extended half-periods of the quadratic solitons. Through the linear stability analysis of the obtained soliton solutions, it is found that the stability intervals of solitons are also shrunk due to the existence of the cubic nonlinearity. The results of the linear stability analysis are verified by the numerical simulations of soliton propagations through using the split-step Fourier method.
-
Keywords:
- quadratic solitons /
- cubic nonlinearity /
- existence interval /
- stability
[1] 陈险峰 2014 非线性光学研究前沿 (上海: 上海交通大学出版社) 第257—333页
Chen X F 2014 Advances in Nonlinear Optics (Shanghai: Shanghai Jiao Tong University Press) pp257–333 (in Chinese)
[2] Kivshar Y S, Agrawal G P 2003 Optical Solitons: from Fibers to Photonic Crystals (New York: Elsevier) pp1–15
[3] Mitchell M, Segev M, Christodoulides D N 1998 Phys. Rev. Lett. 80 4657
Google Scholar
[4] Hu W, Zhang T, Guo Q, Xuan L, Lan S 2006 Appl. Phys. Lett. 89 071111
Google Scholar
[5] Conti C, Fratalocchi A, Peccianti M, Ruocco G, Trillo S 2009 Phys. Rev. Lett. 102 083902
Google Scholar
[6] Skupin S, Saffman M, Królikowski W 2007 Phys. Rev. Lett. 98 263902
Google Scholar
[7] Pedri P, Santos L 2005 Phys. Rev. Lett. 95 200404
Google Scholar
[8] Liang G, Liu J L, Hu W, Guo Q 2022 Appl. Sci. 12 2386
Google Scholar
[9] Królikowski W, Bang O, Rasmussen J J, Wyller J 2001 Phys. Rev. E 64 016612
Google Scholar
[10] Bang O, Królikowski W, Wyller J, Rasmussen J J 2002 Phys. Rev. E 66 046619
Google Scholar
[11] Conti C, Peccianti M, Assanto G 2004 Phys. Rev. Lett. 92 113902
Google Scholar
[12] Piccardi A, Alberucci A, Buchnev O, Kaczmarek M, Khoo I C, Assanto G 2012 Appl. Phys. Lett. 101 081112
Google Scholar
[13] Laudyn U A, Kwasny M, Karpierz M A, Assanto G 2019 Opt. Lett. 44 167
Google Scholar
[14] Rotschild C, Cohen O, Manela O, Segev M, Carmon T 2005 Phys. Rev. Lett. 95 213904
Google Scholar
[15] Shou Q, Zhang X, Hu W, Guo Q 2011 Opt. Lett. 36 4194
Google Scholar
[16] Nikolov N I, Neshev D, Bang O, Królikowski W Z 2003 Phys. Rev. E 68 036614
Google Scholar
[17] Torruellas W E, Wang Z, Hagan D J, VanStryland E W, Stegeman G I, Torner L, Menyuk C R 1995 Phys. Rev. Lett. 74 5036
Google Scholar
[18] Torner L, Menyuk C R, Torruellas W E, Stegeman G I 1995 Opt. Lett. 20 13
Google Scholar
[19] Chen J, Ge J W, Lu D Q, Hu W 2020 Appl. Math. Lett. 102 106108
Google Scholar
[20] Karamzin Y N, Sukhorukov A P 1974 JETP Lett. 20 339
[21] Karamzin Y N, Sukhorukov A P 1975 Sov. Phys. JETP 41 414
[22] Buryak A V, Kivshar Y S 1995 Phys. Lett. A 197 407
Google Scholar
[23] Buryak A V, Di Trapani P, Skryabin D V, Trillo S 2002 Phys. Rep. 370 63
Google Scholar
[24] Torner L, Mihalache D, Mazilu D, Akhmediev N N 1995 Opt. Lett. 20 2183
Google Scholar
[25] Schiek R, Baek Y, Stegeman G I 1996 Phys. Rev. E 53 1138
Google Scholar
[26] Esbensen B K, Bache M, Królikowski W, Bang O 2012 Phys. Rev. A 86 023849
Google Scholar
[27] Wang J, Li Y H, Guo Q, Hu W 2014 Opt. Lett. 39 405
Google Scholar
[28] Wang J, Ma Z L, Li Y H, Lu D Q, Guo Q, Hu W 2015 Phys. Rev. A 91 033801
Google Scholar
[29] Zheng Y Z, Gao Y, Wang J, Lv F, Lu D Q, Hu W 2017 Phys. Rev. A 95 013808
Google Scholar
[30] Chen M N, Ping X R, Liang G, Guo Q, Lu D Q, Hu W 2018 Phys. Rev. A 97 013829
Google Scholar
[31] Agrawal G P 2007 Nonlinear Fiber Optics (4th Ed.) (New York: Elsevier) pp25–50
-
图 1 强非局域和一般非局域情况下二阶和三阶非线性共同作用时的孤子波形(红色: 基频波; 绿色: 二次谐波) (a), (b)强非局域情况
$ \alpha=0.04 $ , 其中图(b)的波形是在图(a)的基础上向两边各拓展了半个周期; (c), (d)一般非局域情况$ \alpha=10 $ , 其中图(c)中$ l=18.0974 $ , 图(d)中$ l=18.7214 $ . 图中虚线为二阶和三阶非线性共同作用时的孤子波形; 实线为只有二阶非线性作用时的二次孤子波形;$ |d_2/d_1 |=1 $ Figure 1. Soliton profiles (red: FW; green: SH) with quadratic and cubic nonlinearities for strongly nonlocal case and general nonlocal case: (a), (b) Strongly nonlocal case
$ \alpha=0.04 $ ; the soliton in panel (b) is an extension of that in panel (a) toward the right and left sides; (c), (d) general nonlocal case$ \alpha=10 $ , where (c)$ l=18.0974 $ , (d)$ l=18.7214 $ . Dotted line: soliton profiles with quadratic and cubic nonlinearities; Solid line: the profiles of quadratic solitons with only quadratic nonlinearity.$ |d_2/d_1 |=1 $ 
图 2 基频波的束宽(蓝色)和功率(红色)随样品宽度l 的变化规律 (a)强非局域情况
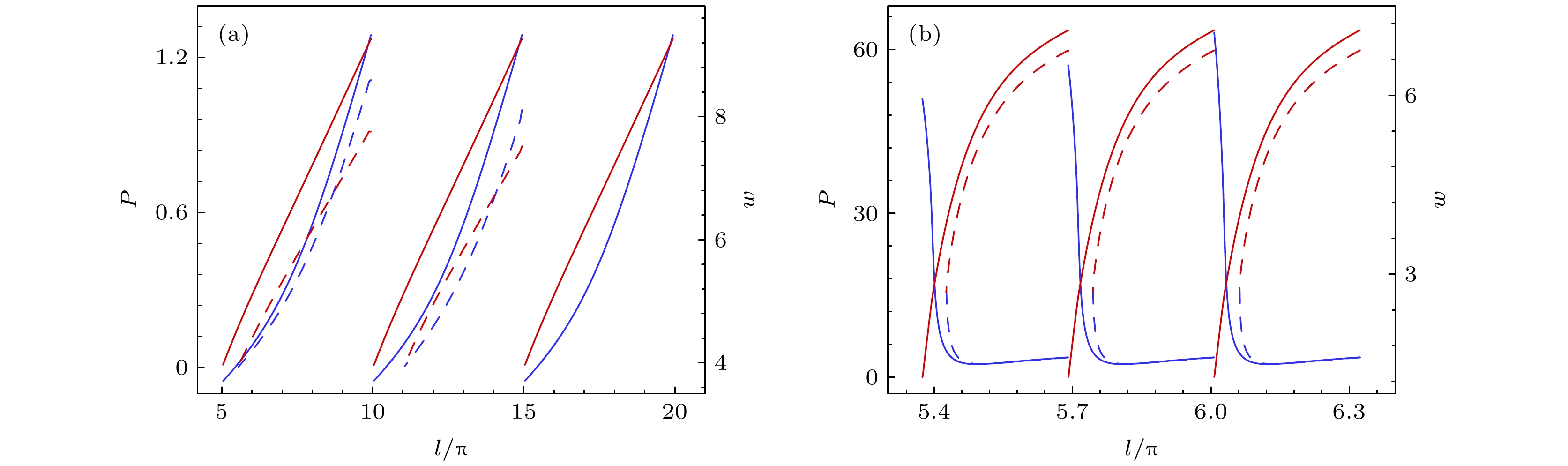
$ \alpha=0.04 $ ; (b)一般非局域情况$ \alpha=10 $ . 虚线为二阶和三阶非线性共同作用时的结果; 实线为只有二阶非线性作用时的结果.$ |d_2/d_1 |=0.8 $ Figure 2. Soliton width (blue line, right ordinate) and power (red line, left ordinate) of the FW versus the sample size l: (a) Strongly nonlocal case
$ \alpha=0.04 $ ; (b) general nonlocal case$ \alpha=10 $ . Dotted line: results with quadratic and cubic nonlinearities; Solid line: results with only quadratic nonlinearity.$ |d_2/d_1 |=0.8 $ 
图 3 (a), (b)对应图2的孤子不稳定增长率曲线; (c)孤子的不稳定增长率随非局域程度α的变化规律,
$ l=18 $ ,$ |d_2/d_1 |=2 $ ; (d)孤子的不稳定增长率随$ d_2/d_1 $ 的变化规律,$ \alpha=0.11 $ ,$ l=18 $ . 虚线为二阶和三阶非线性共同作用时的结果; 实线为只有二阶非线性作用时的结果Figure 3. (a), (b) Curves of unstable growth rate corresponding to Fig. 2; (c) unstable growth rate versus the nonlocal degree α.
$ l=18 $ ,$ |d_2/d_1 |=2 $ ; (d) unstable growth rate versus$ d_2/d_1 $ .$ \alpha=0.11 $ ,$ l=18 $ . Dotted line: results with quadratic and cubic nonlinearities; Solid line: results with only quadratic nonlinearity
-
[1] 陈险峰 2014 非线性光学研究前沿 (上海: 上海交通大学出版社) 第257—333页
Chen X F 2014 Advances in Nonlinear Optics (Shanghai: Shanghai Jiao Tong University Press) pp257–333 (in Chinese)
[2] Kivshar Y S, Agrawal G P 2003 Optical Solitons: from Fibers to Photonic Crystals (New York: Elsevier) pp1–15
[3] Mitchell M, Segev M, Christodoulides D N 1998 Phys. Rev. Lett. 80 4657
Google Scholar
[4] Hu W, Zhang T, Guo Q, Xuan L, Lan S 2006 Appl. Phys. Lett. 89 071111
Google Scholar
[5] Conti C, Fratalocchi A, Peccianti M, Ruocco G, Trillo S 2009 Phys. Rev. Lett. 102 083902
Google Scholar
[6] Skupin S, Saffman M, Królikowski W 2007 Phys. Rev. Lett. 98 263902
Google Scholar
[7] Pedri P, Santos L 2005 Phys. Rev. Lett. 95 200404
Google Scholar
[8] Liang G, Liu J L, Hu W, Guo Q 2022 Appl. Sci. 12 2386
Google Scholar
[9] Królikowski W, Bang O, Rasmussen J J, Wyller J 2001 Phys. Rev. E 64 016612
Google Scholar
[10] Bang O, Królikowski W, Wyller J, Rasmussen J J 2002 Phys. Rev. E 66 046619
Google Scholar
[11] Conti C, Peccianti M, Assanto G 2004 Phys. Rev. Lett. 92 113902
Google Scholar
[12] Piccardi A, Alberucci A, Buchnev O, Kaczmarek M, Khoo I C, Assanto G 2012 Appl. Phys. Lett. 101 081112
Google Scholar
[13] Laudyn U A, Kwasny M, Karpierz M A, Assanto G 2019 Opt. Lett. 44 167
Google Scholar
[14] Rotschild C, Cohen O, Manela O, Segev M, Carmon T 2005 Phys. Rev. Lett. 95 213904
Google Scholar
[15] Shou Q, Zhang X, Hu W, Guo Q 2011 Opt. Lett. 36 4194
Google Scholar
[16] Nikolov N I, Neshev D, Bang O, Królikowski W Z 2003 Phys. Rev. E 68 036614
Google Scholar
[17] Torruellas W E, Wang Z, Hagan D J, VanStryland E W, Stegeman G I, Torner L, Menyuk C R 1995 Phys. Rev. Lett. 74 5036
Google Scholar
[18] Torner L, Menyuk C R, Torruellas W E, Stegeman G I 1995 Opt. Lett. 20 13
Google Scholar
[19] Chen J, Ge J W, Lu D Q, Hu W 2020 Appl. Math. Lett. 102 106108
Google Scholar
[20] Karamzin Y N, Sukhorukov A P 1974 JETP Lett. 20 339
[21] Karamzin Y N, Sukhorukov A P 1975 Sov. Phys. JETP 41 414
[22] Buryak A V, Kivshar Y S 1995 Phys. Lett. A 197 407
Google Scholar
[23] Buryak A V, Di Trapani P, Skryabin D V, Trillo S 2002 Phys. Rep. 370 63
Google Scholar
[24] Torner L, Mihalache D, Mazilu D, Akhmediev N N 1995 Opt. Lett. 20 2183
Google Scholar
[25] Schiek R, Baek Y, Stegeman G I 1996 Phys. Rev. E 53 1138
Google Scholar
[26] Esbensen B K, Bache M, Królikowski W, Bang O 2012 Phys. Rev. A 86 023849
Google Scholar
[27] Wang J, Li Y H, Guo Q, Hu W 2014 Opt. Lett. 39 405
Google Scholar
[28] Wang J, Ma Z L, Li Y H, Lu D Q, Guo Q, Hu W 2015 Phys. Rev. A 91 033801
Google Scholar
[29] Zheng Y Z, Gao Y, Wang J, Lv F, Lu D Q, Hu W 2017 Phys. Rev. A 95 013808
Google Scholar
[30] Chen M N, Ping X R, Liang G, Guo Q, Lu D Q, Hu W 2018 Phys. Rev. A 97 013829
Google Scholar
[31] Agrawal G P 2007 Nonlinear Fiber Optics (4th Ed.) (New York: Elsevier) pp25–50
DownLoad:
Catalog
Metrics
- Abstract views: 3464
- PDF Downloads: 68
- Cited By: 0
