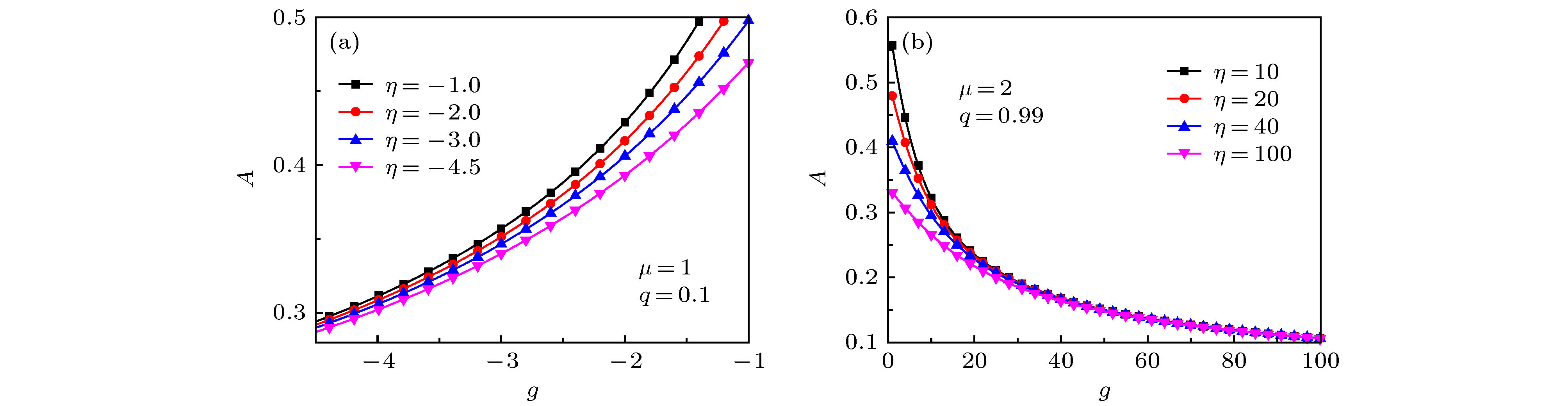
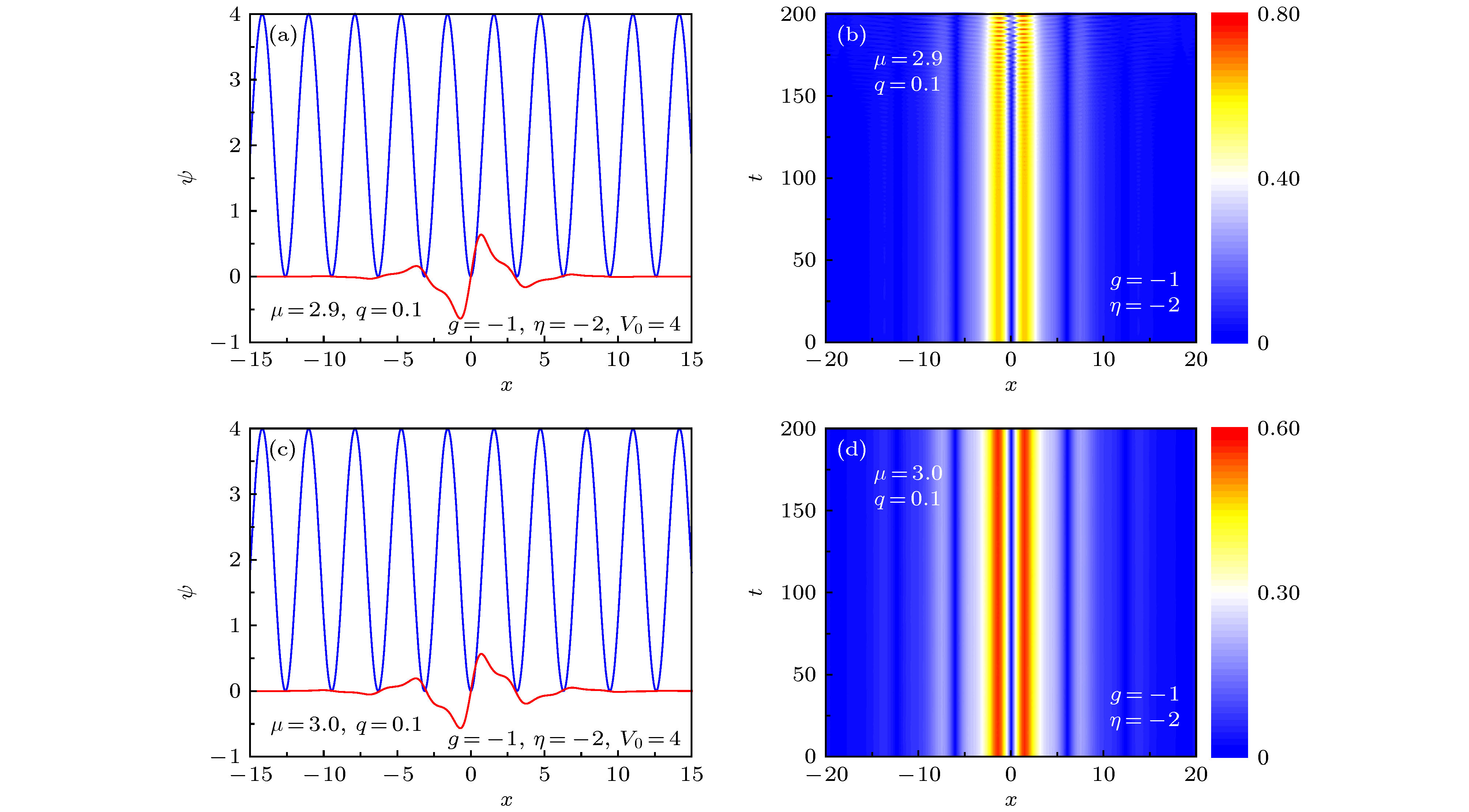
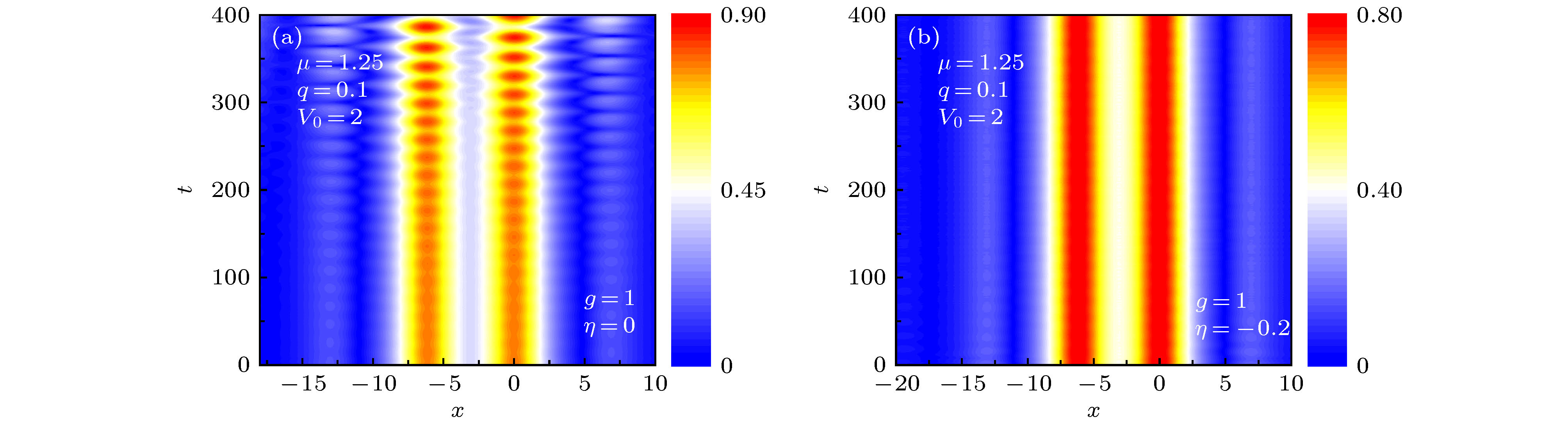
-
具有三体相互作用的玻色-爱因斯坦凝聚体(Bose-Einstein Condensate, BEC)束缚于雅可比椭圆周期势中, 在平均场近似下可用3—5次Gross-Pitaevskii方程(GPE)描述. 首先利用多重尺度法对该系统进行了理论分析, 将GPE化为一定态非线性薛定谔方程(Nonlinear Schrödinger Equation, NLSE), 并给出了一类带隙孤子的解析表达式. 然后采用牛顿共轭梯度法数值得到了该系统中存在的两类带隙孤子, 发现孤子的振幅随着三体相互作用的增强而减小, 这与多重尺度法分析所得结论一致. 最后用时间劈裂傅里叶谱方法对GPE进行长时间动力学演化以考察孤子的稳定性, 发现系统中既存在稳定的带隙孤子, 也存在不稳定的带隙孤子, 且外势的模数会对孤子的结构和稳定性产生明显影响.We study the gap solitons and their stability properties in a Bose-Einstein condensation (BEC) under three-body interaction loaded in a Jacobian elliptic sine potential, which can be described by a cubic-quintic Gross-Pitaevskii equation (GPE) in the mean-field approximation. Firstly, the GPE is transformed into a stationary cubic-quintic nonlinear Schrödinger equation (NLSE) by the multi-scale method. A class of analytical solution of the NLSE is presented to describe the gap solitons. It is shown analytically that the amplitude of the gap soliton decreases as the two-body or three-body interaction strength increases. Secondly, many kinds of gap solitons, including the fundamental soliton and the sub-fundamental soliton, are obtained numerically by the Newton-Conjugate-Gradient (NCG) method. There are two families of fundamental solitons: one is the on-site soliton and the other is the off-site soliton. All of them are bifurcated from the Bloch band. Both in-phase and out-phase dipole solitons for off-site solitons do exist in such a nonlinear system. The numerical results also indicate that the amplitude of the gap soliton decreases as the nonlinear interaction strength increases, which accords well with the analytical prediction. Finally, long-time dynamical evolution for the GPE is performed by the time-splitting Fourier spectrum method to investigate the dynamical stability of gap solitons. It is shown that the on-site solitons are always dynamically stable, while the off-site solitons are always unstable. However, both stable and unstable in-phase or out-phase dipole solitons, which are not bifurcated from the Bloch band, indeed exist. For a type of out-phase soliton, there is a critical value
$ q_c$ when the chemical potential μ is fixed. The solitons are linearly stable as$ q>q_c$ , while they are linearly unstable for$ q<q_c$ . Therefore, the modulus q plays an important role in the stability of gap solitons. One can change the dynamical behavior of gap solitons by adjusting the modulus of external potential in experiment. We also find that there exists a kind of gap soliton, in which the soliton is dynamically unstable if only the two-body interaction is considered, but it becomes stable when the three-body interaction is taken into account. This indicates that the three-body interaction has influence on the stability of gap solitons.-
Keywords:
- Bose-Einstein condensate /
- gap soliton /
- three-body interactions /
- stability
[1] Bose S N 1924 Z. Phy. 26 178
[2] Anderson M H, Ensher J R, Matthews M R, Wieman C E, Cornell E A 1995 Science 269 198
Google Scholar
[3] Tsurumi T 2000 Int. J. Mod. Phys. B 14 655
Google Scholar
[4] Malomed B A 2006 Soltion Management in Periodic Systems (Vol. 1) (Berlin: Springer) pp 1—6
[5] Wang D S, Hu X H, Hu J, Liu W M 2010 Phys. Rev. A 81 025604
Google Scholar
[6] Burger S, Bongs K, Dettmer S, Ertmer W, Sengstock K, Sanpera A, Shlyapnikov G V, Lewenstein M 1999 Phys. Rev. Lett. 83 5198
Google Scholar
[7] Khaykovich L, Schreck F, Ferrari G, Bourdel T, Cubizolles J, Carr L D, Castin Y, Salomon C 2002 Science 296 1290
Google Scholar
[8] Ku M J H, Ji W, Mukherjee B, Guardado S E, Cheuk L W, Yefash T, Zwierlein M W 2014 Phys. Rev. Lett. 113 065301
Google Scholar
[9] Yu H Y, Yan J R, Xie Q T 2004 Chin. Phys. Lett. 21 1881
Google Scholar
[10] Wang Z X, Zhang X H, Shen K 2008 Chin. Phys. B 17 3270
Google Scholar
[11] Li J H, Kuang L M 2003 Commun. Theor. Phys. 39 44
Google Scholar
[12] EtienneW B, Alidou M D, Timoléon C K 2008 Phys. Rev. E 77 046216
Google Scholar
[13] Zhang W, Wright E M, Pu H, Meystre P 2003 Phys. Rev. A 68 023605
Google Scholar
[14] Abdullaev F Kh, Gammal A, Tomio L, Frederico T 2001 Phys. Rev. A 63 043604
Google Scholar
[15] Chang N N, Yu Z F, Zhang A X, Xue J K 2017 Chin. Phys. B 26 115202
Google Scholar
[16] Etienne W, Porsezian K, Alidou M, Timoléon C K 2013 Phys. Lett. A 377 262
Google Scholar
[17] Chin C, Kraemer T, Mark M, Herbig J, Waldburger P, Nagerl H C, Grimm R 2005 Phys. Rev. Lett. 94 123201
Google Scholar
[18] Peng P, Li G Q 2009 Chin. Phys. B 18 3221
Google Scholar
[19] 阮航宇, 陈一新 2001 4 0586
Ruan H Y, Chen Y X 2001 Acta. Phys. Sin. 4 0586
[20] Saito H, Ueda M 2001 Phys. Rev. Lett. 86 1406
Google Scholar
[21] Fiho V S, Gammal A, Frederico T, Tomio L 2000 Phys. Rev. A 62 033605
Google Scholar
[22] Meng D X, Li K Z 2019 Phys. Rev. Lett. 33 19150123
[23] Wahlquist H D, Estabreok F B 1973 Phys. Rev. Lett. 31 1386
Google Scholar
[24] Radhakrishnan R, Sahadcvan R, Lakshmanan M 1995 Chaos, Solitons Fractals 5 2315
Google Scholar
[25] Weiss J, Tabor M, Carneval G 1983 J. Math. Phys. 24 6
[26] Wang D S, Liu J 2018 Appl. Math. Lett. 79 211
Google Scholar
[27] 文林, 梁毅, 周晶, 余鹏, 夏雷, 牛连斌, 张晓斐 2019 68 080301
Google Scholar
Wen L, Liang Y, Zhou J, Yu P, Xia L, Niu L B, Zhang X F 2019 Acta Phys. Sin. 68 080301
Google Scholar
[28] Dmitry E P, Andrey A S, Yuri S K 2004 Phys. Rev. E 70 036618
Google Scholar
[29] Tang N, Yang X Y, Feng W X, Song L, Li X L, Xi Z H, Wang D S, Shi Y R 2019 Physica A 528 1
[30] Akozbek N, John S 1998 Phys. Rev. E 57 2287
Google Scholar
[31] Mingaleev S F, Kivshar Yu S 2001 Phys. Rev. Lett. 86 5474
Google Scholar
[32] Louis P J Y, Ostrovskaya E A, Kivshar Yu S 2005 Phys. Rev. A 71 023612
Google Scholar
[33] Eiermann B, Anker Th, Albiez M, Taglieber M, Treutlein M, Marzlin K P, Oberthaler M K 2004 Phys. Rev. Lett. 92 230401
Google Scholar
[34] Zhu J, Bian C L, Wang H C 2019 Chin. Phys. B 28 093701
Google Scholar
[35] Li Y, Hai W H 2005 Commun. Theor. Phys. 44 840
Google Scholar
[36] Eddy T, Paolo T, Mahir H, Arthur K 1999 Phys. Rep. 315 199
Google Scholar
[37] Bao W 2007 Dynamics In Models Of Coarsening, Coagulation, Condensation And Quantization. (Vol. 1) (Singpore: World Scientific) p147—149
[38] Yang J K 2010 Nonlinear Waves in Integrable and Nonintegrable Systems (Vol. 1) (Philadelphia: SIAM) p269−283
[39] Kostov N A, Enolśkii V Z, Gerdjikov V S, Konotop V V and Salerno M 2004 Phys. Rev. E 70 056617
Google Scholar
[40] Catarina C, Vitor Nistor, Yu Q 2017 Operator Theory: Advances and Applications (Vol. 1) (Berlin: Springer) p20−24
[41] Yang J K 2009 J. Comput. Phys. 228 7007
Google Scholar
[42] 唐恒永 1985 北京工业大学学报 11 69
Tang H Y 1985 J. BJUT. Tech. 11 69
[43] Royer C W, Neill M O’, Wright S J 2018 Math. Program. 1-38
[44] Zhang Q, Li S, Lei Y, Zhang X D 2012 J. Control Theory Appl. 10 223
Google Scholar
[45] Allen A J, Zuccher S, Caliari M, Proukakis N, Parker N G, Barenghi C F 2014 Phys. Rev. A 90 013601
Google Scholar
[46] Adhikari S F, Malomed B A 2007 Europhys. Lett. 79 50003
Google Scholar
-
图 1 3−5次GPE的单峰带隙孤子(
$V_0=4$ ) (a), (b)$q=0.1$ ; (c), (d)$q=0.99$ . 阴影部分表示外势$V(x)$ 低处Fig. 1. Profiles of singl-hump gap solitons of the cubic-quintic GPE (
$V_0=4$ ): (a), (b)$q$ = 0.1; (c), (d)$q$ = 0.99. Shaded regions represent lattice sites, i.e., regions of low potential values$V(x)$ .
图 2 单峰带隙孤子的振幅随相互作用强度的变化(
$V_0=4$ )Fig. 2. Amplitudes of single-hump gap solitons v.s. nonlinear interaction strength.
图 3 on-site孤子三体相互作用能量和两体相互作用能量的比
Fig. 3. The ratio of three-body energy to two-body energy for on-site solitons with different interaction strength.
图 4 3−5次GPE的同相偶极孤子和异相偶极孤子(
$V_0=4$ ) (a), (b)$q=0.1; $ (c), (d)$q=0.99$ . 阴影部分表示外势$V(x)$ 低处Fig. 4. Profiles of double-hump gap solitons of the cubic-quintic GPE (
$V_0=4$ ) (a), (b)$q$ = 0.1; (c), (d)$q$ = 0.99. Shaded regions represent lattice sites, i.e., regions of low potential values$V(x)$ .
图 5 不同类型带隙孤子的动力学演化 (a) on-site孤子; (b) 同相偶极孤子; (c), (d) 异相偶极孤子
Fig. 5. Contour plots of
$|{\varPsi(x, t)}|$ for perturbed gap solitons: (a) On-site soliton; (b) in-phase dipole soliton; (c), (d) out-phase dipole soliton.
图 6 (a), (c) 第一带隙中的亚基本带隙孤子(红色). 蓝线表示外势; (b), (d) 亚基本带隙孤子的动力学演化
Fig. 6. (a), (c) Profiles of sub-fundamental gap solitons (red lines) lie in the first bandgap. The solid blue lines denote the external potential; (b), (d) contour plots of
$|{\varPsi(x, t)}|$ for perturbed gap solitons.
图 7 第一带隙中同相偶极孤子 (a)
$\eta=0$ 和(b)$\eta=-0.2$ 时的动力学演化Fig. 7. Contour plots of
$|{\varPsi(x, t)}|$ for in-phase dipole solitons lie in the first bandgap with (a)$\eta=0$ and (b)$\eta=-0.2$ . -
[1] Bose S N 1924 Z. Phy. 26 178
[2] Anderson M H, Ensher J R, Matthews M R, Wieman C E, Cornell E A 1995 Science 269 198
Google Scholar
[3] Tsurumi T 2000 Int. J. Mod. Phys. B 14 655
Google Scholar
[4] Malomed B A 2006 Soltion Management in Periodic Systems (Vol. 1) (Berlin: Springer) pp 1—6
[5] Wang D S, Hu X H, Hu J, Liu W M 2010 Phys. Rev. A 81 025604
Google Scholar
[6] Burger S, Bongs K, Dettmer S, Ertmer W, Sengstock K, Sanpera A, Shlyapnikov G V, Lewenstein M 1999 Phys. Rev. Lett. 83 5198
Google Scholar
[7] Khaykovich L, Schreck F, Ferrari G, Bourdel T, Cubizolles J, Carr L D, Castin Y, Salomon C 2002 Science 296 1290
Google Scholar
[8] Ku M J H, Ji W, Mukherjee B, Guardado S E, Cheuk L W, Yefash T, Zwierlein M W 2014 Phys. Rev. Lett. 113 065301
Google Scholar
[9] Yu H Y, Yan J R, Xie Q T 2004 Chin. Phys. Lett. 21 1881
Google Scholar
[10] Wang Z X, Zhang X H, Shen K 2008 Chin. Phys. B 17 3270
Google Scholar
[11] Li J H, Kuang L M 2003 Commun. Theor. Phys. 39 44
Google Scholar
[12] EtienneW B, Alidou M D, Timoléon C K 2008 Phys. Rev. E 77 046216
Google Scholar
[13] Zhang W, Wright E M, Pu H, Meystre P 2003 Phys. Rev. A 68 023605
Google Scholar
[14] Abdullaev F Kh, Gammal A, Tomio L, Frederico T 2001 Phys. Rev. A 63 043604
Google Scholar
[15] Chang N N, Yu Z F, Zhang A X, Xue J K 2017 Chin. Phys. B 26 115202
Google Scholar
[16] Etienne W, Porsezian K, Alidou M, Timoléon C K 2013 Phys. Lett. A 377 262
Google Scholar
[17] Chin C, Kraemer T, Mark M, Herbig J, Waldburger P, Nagerl H C, Grimm R 2005 Phys. Rev. Lett. 94 123201
Google Scholar
[18] Peng P, Li G Q 2009 Chin. Phys. B 18 3221
Google Scholar
[19] 阮航宇, 陈一新 2001 4 0586
Ruan H Y, Chen Y X 2001 Acta. Phys. Sin. 4 0586
[20] Saito H, Ueda M 2001 Phys. Rev. Lett. 86 1406
Google Scholar
[21] Fiho V S, Gammal A, Frederico T, Tomio L 2000 Phys. Rev. A 62 033605
Google Scholar
[22] Meng D X, Li K Z 2019 Phys. Rev. Lett. 33 19150123
[23] Wahlquist H D, Estabreok F B 1973 Phys. Rev. Lett. 31 1386
Google Scholar
[24] Radhakrishnan R, Sahadcvan R, Lakshmanan M 1995 Chaos, Solitons Fractals 5 2315
Google Scholar
[25] Weiss J, Tabor M, Carneval G 1983 J. Math. Phys. 24 6
[26] Wang D S, Liu J 2018 Appl. Math. Lett. 79 211
Google Scholar
[27] 文林, 梁毅, 周晶, 余鹏, 夏雷, 牛连斌, 张晓斐 2019 68 080301
Google Scholar
Wen L, Liang Y, Zhou J, Yu P, Xia L, Niu L B, Zhang X F 2019 Acta Phys. Sin. 68 080301
Google Scholar
[28] Dmitry E P, Andrey A S, Yuri S K 2004 Phys. Rev. E 70 036618
Google Scholar
[29] Tang N, Yang X Y, Feng W X, Song L, Li X L, Xi Z H, Wang D S, Shi Y R 2019 Physica A 528 1
[30] Akozbek N, John S 1998 Phys. Rev. E 57 2287
Google Scholar
[31] Mingaleev S F, Kivshar Yu S 2001 Phys. Rev. Lett. 86 5474
Google Scholar
[32] Louis P J Y, Ostrovskaya E A, Kivshar Yu S 2005 Phys. Rev. A 71 023612
Google Scholar
[33] Eiermann B, Anker Th, Albiez M, Taglieber M, Treutlein M, Marzlin K P, Oberthaler M K 2004 Phys. Rev. Lett. 92 230401
Google Scholar
[34] Zhu J, Bian C L, Wang H C 2019 Chin. Phys. B 28 093701
Google Scholar
[35] Li Y, Hai W H 2005 Commun. Theor. Phys. 44 840
Google Scholar
[36] Eddy T, Paolo T, Mahir H, Arthur K 1999 Phys. Rep. 315 199
Google Scholar
[37] Bao W 2007 Dynamics In Models Of Coarsening, Coagulation, Condensation And Quantization. (Vol. 1) (Singpore: World Scientific) p147—149
[38] Yang J K 2010 Nonlinear Waves in Integrable and Nonintegrable Systems (Vol. 1) (Philadelphia: SIAM) p269−283
[39] Kostov N A, Enolśkii V Z, Gerdjikov V S, Konotop V V and Salerno M 2004 Phys. Rev. E 70 056617
Google Scholar
[40] Catarina C, Vitor Nistor, Yu Q 2017 Operator Theory: Advances and Applications (Vol. 1) (Berlin: Springer) p20−24
[41] Yang J K 2009 J. Comput. Phys. 228 7007
Google Scholar
[42] 唐恒永 1985 北京工业大学学报 11 69
Tang H Y 1985 J. BJUT. Tech. 11 69
[43] Royer C W, Neill M O’, Wright S J 2018 Math. Program. 1-38
[44] Zhang Q, Li S, Lei Y, Zhang X D 2012 J. Control Theory Appl. 10 223
Google Scholar
[45] Allen A J, Zuccher S, Caliari M, Proukakis N, Parker N G, Barenghi C F 2014 Phys. Rev. A 90 013601
Google Scholar
[46] Adhikari S F, Malomed B A 2007 Europhys. Lett. 79 50003
Google Scholar
下载:
计量
- 文章访问数: 9744
- PDF下载量: 211
- 被引次数: 0
