-
As a crucial quantum resource for quantum computing and quantum information processing, cluster state has attracted extensive attention due to its unique entanglement properties and rich structures. In this work, we theoretically propose a scheme for generating four-mode entangled states based on cascaded four-wave mixing (FWM) process. The internal entanglement characteristics are studied by using the positivity under partial transposition criterion and eigenmode decomposition. In addition, the output entangled states are reconstructed and optimized by adjusting the relative phase of balanced homodyne detection and postprocessing the signal noise, and finally three four-mode cluster states with different structures are generated. Such a method can effectively reduce the excess noise induced by finite squeezing. Our theoretical results provide a reliable way of generating scalable continuous variable cluster states based on FWM process in atomic ensemble.
-
Keywords:
- four-wave mixing /
- eigenmode /
- cluster states
[1] Fabre C, Treps N 2020 Rev. Mod. Phys. 92 035005
Google Scholar
[2] Briegel H J, Browne D E, Dür W, Raussendorf R, Van den Nest M 2009 Nat. Phys. 5 19
Google Scholar
[3] Menicucci N C, Loock P V, Gu M, Weedbrook C, Ralph T C, Nielsen M A 2006 Phys. Rev. Lett. 97 110501
Google Scholar
[4] Su X L, Hao S H, Deng X W, Ma L Y, Wang M H, Jia X J, Xie C D, Peng K C 2013 Nat. Commun. 4 2828
Google Scholar
[5] Su X L, Zhao Y P, Hao S H, Jia X J, Xie C D, Peng K C 2012 Opt. Lett. 37 5178
Google Scholar
[6] Armstrong S, Morizur J F, Janousek J, Hage B, Treps N, Lam P K, Bachor H A 2012 Nat. Commun. 3 1026
Google Scholar
[7] Asavanant W, Shiozawa Y, Yokoyama S, et al. 2019 Science 366 373
Google Scholar
[8] Larsen M V, Guo X S, Breum C R, Neergaard-Nielsen J S, Andersen U L 2019 Science 366 369
Google Scholar
[9] Larsen M V, Guo X S, Breum C R, Neergaard-Nielsen J S, Andersen U L 2021 Nat. Phys. 17 1018
Google Scholar
[10] Cai Y, Roslund J, Ferrini G, Arzani F, Xu X, Fabre C, Treps N 2017 Nat. Commun. 8 15645
Google Scholar
[11] Barbosa F A S, Coelho A S, Muñoz-Martínez L F, Ortiz-Gutiérrez L, Villar A S, Nussenzveig P, Martinelli M 2018 Phys. Rev. Lett. 121 073601
Google Scholar
[12] Marino A M, Pooser R C, Boyer V, Lett P D 2009 Nature 457 859
Google Scholar
[13] Lawrie B, Evans P, Pooser R 2013 Phys. Rev. Lett. 110 156802
Google Scholar
[14] Qin Z Z, Cao L M, Wang H L, Marion A M, Zhang W P, Jing J T 2014 Phys. Rev. Lett. 113 023602
Google Scholar
[15] Liu S S, Lou Y B, Jing J T 2019 Phys. Rev. Lett. 123 113602
Google Scholar
[16] Pooser R C, Savino N, Batson E, Beckey J L, Garcia J, Lawrie B J 2020 Phys. Rev. Lett. 124 230504
Google Scholar
[17] McCormick C F, Boyer V, Arimondo E, Lett P D 2007 Opt. Lett. 32 178
Google Scholar
[18] Boyer V, Marino A M, Pooser P C, Lett P D 2008 Science 321 5888
[19] Cao L M, Du J J, Feng J L, Qin Z Z, Marino A M, Kolobov M I, Jing J T 2017 Opt. Lett. 42 7
[20] Pan X Z, Yu S, Zhou Y F, Zhang K, Zhang K, Lv S C, Li S J, Wang W, Jing J T 2019 Phys. Rev. Lett. 123 070506
Google Scholar
[21] Li S J, Pan X Z, Ren Y, Liu H Z, Yu S, Jing J T 2020 Phys. Rev. Lett. 124 083605
Google Scholar
[22] Wang W, Zhang K, Jing J T 2020 Phys. Rev. Lett. 125 140501
Google Scholar
[23] Liu S S, Lou Y B, Jing J T 2020 Nat. Commun. 11 3875
Google Scholar
[24] Marino A M, Pooser R C, Boyer V, Lett P D 2009 Nature 457 859
[25] Chen Y X, Liu S S, Lou Y B, Jing J T 2021 Phys. Rev. Lett. 127 093601
Google Scholar
[26] Liu S S, Lou Y B, Chen Y X 2021 Phys. Rev. Lett. 126 060503
Google Scholar
[27] Medeiros de Araújo R, Roslund J, Cai Y, Ferrini G, Fabre C, Treps N 2014 Phys. Rev. A 89 053828
Google Scholar
[28] Roslund J, Medeiros de Araújo R, Jing S F, Fabre C, Treps N 2014 Nat. Photonics 8 109
Google Scholar
[29] Ferrini G, Gazeau J P, Coudreau T, Fabre C, Treps N 2013 New J. Phys. 15 093015
Google Scholar
[30] Zhang K, Wang W, Liu S S, Pan X Z, Du J J, Lou Y B, Yu S, Lü S C, Treps N, Fabre C 2020 Phys. Rev. Lett. 124 090501
Google Scholar
[31] Simon R 2000 Phys. Rev. Lett. 84 2726
Google Scholar
[32] Werner R F, Wolf M M 2001 Phys. Rev. Lett. 86 3658
Google Scholar
[33] Braunstein S L 2005 Phys. Rev. A 71 055801
Google Scholar
[34] Hensen B, Bernien H 2005 Nature 526 682
[35] Lloyd S, Braunstein S L 1999 Phys. Rev. Lett. 82 1784
Google Scholar
[36] Braunstein S L, van Loock P 2005 Rev. Mod. Phys. 77 513
Google Scholar
[37] Weedbrook C, Pirandola S 2012 Rev. Mod. Phys. 84 621
Google Scholar
[38] Kimble H J 2008 Nature 453 1023
Google Scholar
[39] Guo X, Breum C R 2020 Nat. Phys. 16 281
Google Scholar
[40] Ferrini G, Roslund J, Arzani F, Fabre C, Treps N 2016 Phys. Rev. A 94 062332
Google Scholar
[41] Ferrini G, Roslund J, Arzani F, Cai Y, Fabre C, Treps N 2015 Phys. Rev. A 91 032314
Google Scholar
[42] Gu M, Weedbrook C, Menicucci N C, Ralph T C, Look P V 2009 Phys. Rev. A 79 062318
Google Scholar
[43] Menicucci N C, Flammia S T, Look P V 2011 Phys. Rev. A 83 042335
Google Scholar
[44] 郝树宏 2016 博士学位论文 (山西: 山西大学)
Hao S H 2016 Ph. D. Dissertation (Shanxi: Shanxi University) (in Chinese)
[45] van Loock P, Weedbrook C, Gu M 2007 Phys. Rev. A 76 032321
Google Scholar
[46] Cai Y, Feng J L, Wang H L, Ferrini G, Xu X Y, Jing J T, Treps N 2015 Phys. Rev. A 91 013843
Google Scholar
[47] van Loock P, Furusawa A 2003 Phys. Rev. A 67 052315
Google Scholar
-
图 1 通过非对称结构级联三个四波混频过程产生四模纠缠态的示意图.
$ {\widehat{a}}_{\mathrm{P}\mathrm{r}1} $ ,$ {\widehat{a}}_{\mathrm{P}\mathrm{r}2} $ 和$ {\widehat{a}}_{\mathrm{P}\mathrm{r}3} $ 是四波混频过程FWM1, FWM2和FWM3的信号光;$ {\widehat{a}}_{\mathrm{v}1} $ ,$ {\widehat{a}}_{\mathrm{v}2} $ 和$ {\widehat{a}}_{\mathrm{v}3} $ 是真空输入模式;$ {\widehat{a}}_{\mathrm{C}1} $ ,$ {\widehat{a}}_{\mathrm{C}2} $ ,$ {\widehat{a}}_{\mathrm{C}3} $ 和$ {\widehat{a}}_{\mathrm{P}\mathrm{r}4} $ 是最终输出的四个模式; Pump1, Pump2和Pump3表示三个四波混频过程的泵浦光; LO表示用于平衡零拍探测(HD)的本振光场Figure 1. Schematic diagram of four-mode entangled states generated by cascading three four-wave mixing processes with asymmetric structure.
$ {\widehat{a}}_{\mathrm{P}\mathrm{r}1} $ ,$ {\widehat{a}}_{\mathrm{P}\mathrm{r}2} $ and$ {\widehat{a}}_{\mathrm{P}\mathrm{r}3} $ are seed beams of FWM1, FWM2 and FWM3, respectively.$ {\widehat{a}}_{\mathrm{v}1} $ ,$ {\widehat{a}}_{\mathrm{v}2} $ and$ {\widehat{a}}_{\mathrm{v}3} $ are vacuum input modes.$ {\widehat{a}}_{\mathrm{C}1} $ ,$ {\widehat{a}}_{\mathrm{C}2} $ ,$ {\widehat{a}}_{\mathrm{C}3} $ and$ {\widehat{a}}_{\mathrm{P}\mathrm{r}4} $ are the final four output modes. Pump1, pump2 and pump3 denote the pump light of three four-wave mixing processes. LO denotes the local oscillator for balanced homodyne detection (HD).
图 2 当
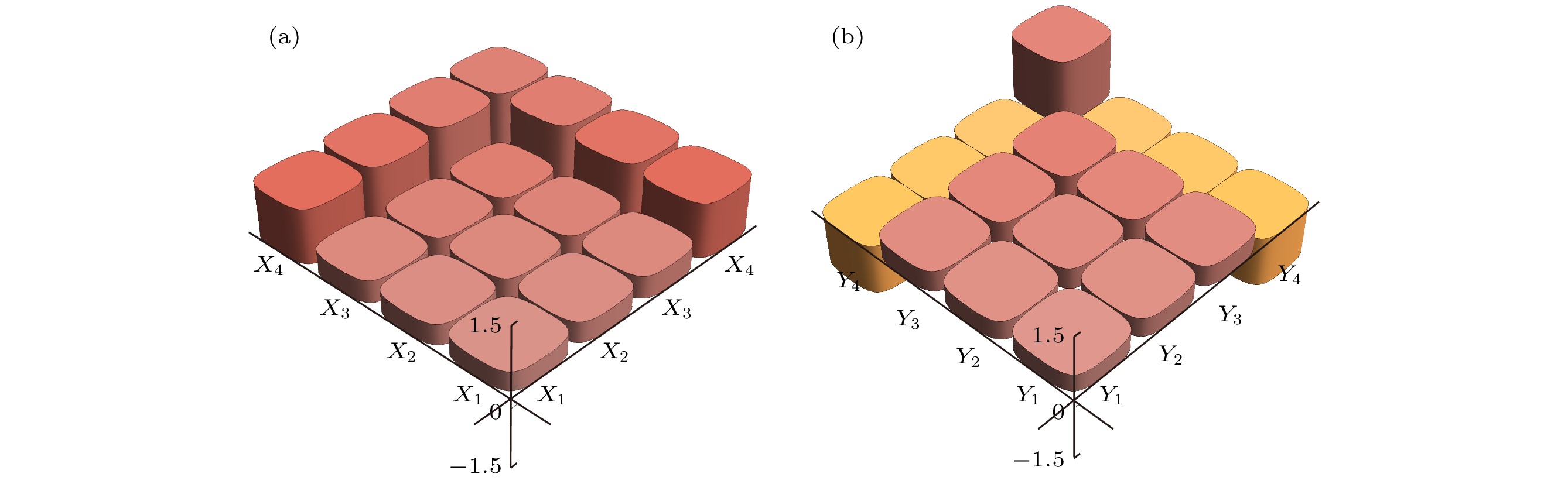
$ {G}_{1}={G}_{2}={G}_{3}=1.2 $ 时四模态系统的协方差矩阵 (a) 正交振幅的协方差矩阵$\langle{{\widehat{X}}_{i}{\widehat{X}}_{j}}\rangle$ ; (b) 正交相位的协方差矩阵$\langle{{\widehat{Y}}_{i}{\widehat{Y}}_{j}}\rangle$ Figure 2. The covariance matrix of the four-mode state in the case of
$ {G}_{1}={G}_{2}={G}_{3}=1.2 $ : (a) The covariance of amplitude quadratures$\langle{{\widehat{X}}_{i}{\widehat{X}}_{j}}\rangle$ ; (b) the covariance of phase quadratures$\langle{{\widehat{Y}}_{i}{\widehat{Y}}_{j}}\rangle$ .
图 3 不同强度增益情况下, 四模态的最小辛本征值. 不同颜色的条形图分别表示强度增益为1.2, 1.5和1.8时的最小辛本征值
Figure 3. The smallest symplectic eigenvalues of four-mode state for different gains. Bar chart with different colors represent the smallest symplectic eigenvalues at gains of 1.2, 1.5 and 1.8, respectively.
图 4 对于不同强度增益
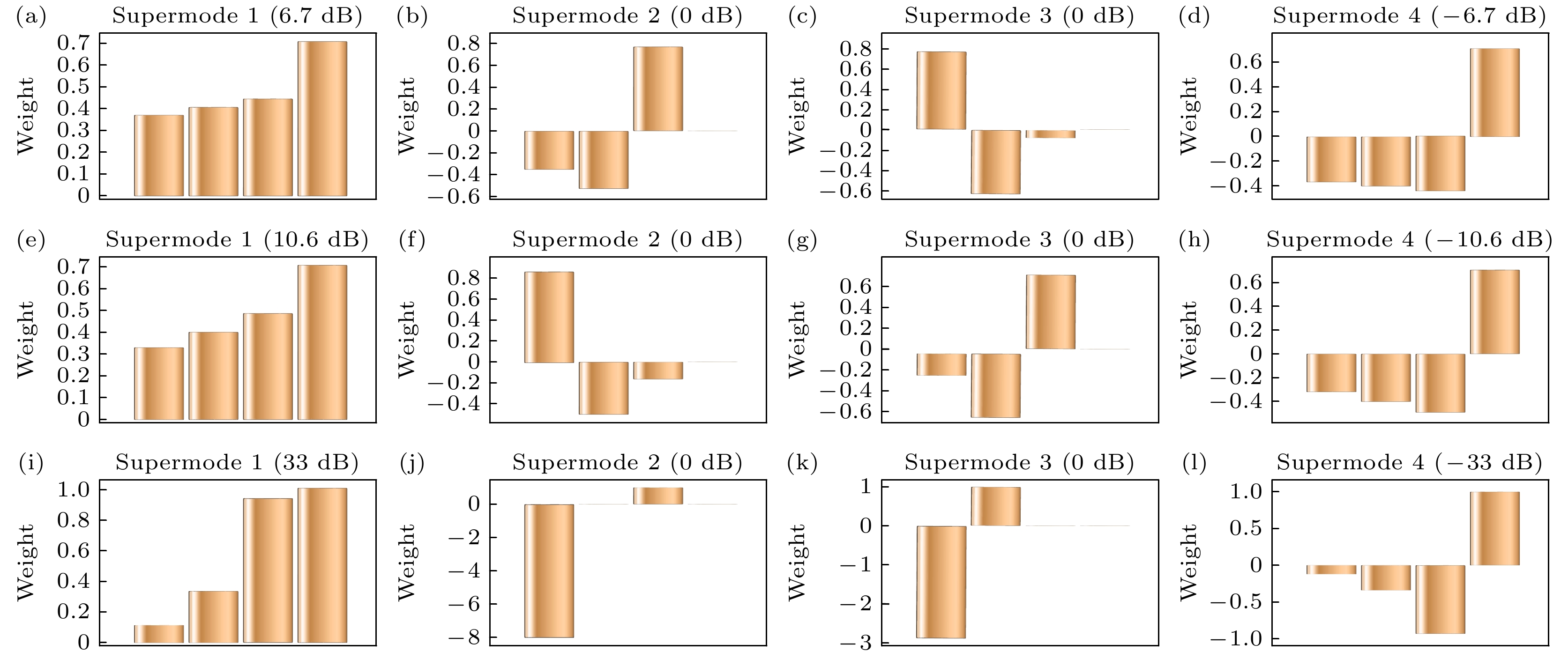
$ G $ , 基于级联四波混频过程生成的四模纠缠态的本征模及其相应的压缩值. 每幅图中的条形柱分别表示模$ {\widehat{a}}_{\mathrm{C}1}, {\widehat{a}}_{\mathrm{C}2}, {\widehat{a}}_{\mathrm{C}3} $ 和$ {\widehat{a}}_{\mathrm{P}\mathrm{r}4} $ 的相对权重, 图上面的数字代表压缩值. 图(a)—(d), 图(e)—(h)和图(i)—(l)分别对应强度增益$ G $ 为1.2, 1.5和8时的本征模分解情况Figure 4. The supermodes of the four-mode entangled state generated based on the cascade four-wave mixing process and their corresponding squeezing levels for different gain values G. The bars represent the relative weight of the modes
$ {\widehat{a}}_{\mathrm{C}1}, {\widehat{a}}_{\mathrm{C}2}, {\widehat{a}}_{\mathrm{C}3} $ and$ {\widehat{a}}_{\mathrm{P}\mathrm{r}4} $ , respectively. The number above the figure represents the squeezing levels. Figures (a)–(d), Figures (e)–(h) and Figures (i)–(l) correspond to the eigenmode decomposition when the gain values G are 1.2, 1.5 and 8, respectively.
图 5 四模线型、T-型和方型簇态的结构图和对应的邻接矩阵
Figure 5. The structure diagram and corresponding adjacency matrix of four-mode linear, T-type, and square cluster states.
表 1 对于不同的强度增益, 四模线型簇态Nullifier的归一化方差
Table 1. Normalized variances of the four-mode linear cluster state nullifiers for different intensity gains.
强度增益 Nullifier 1 Nullifier 2 Nullifier 3 Nullifier 4 $ {G}_{1}={G}_{2}={G}_{3}=1.2 $ 0.59 0.62 0.59 0.69 $ {G}_{1}={G}_{2}={G}_{3}=1.5 $ 0.68 0.56 0.48 0.49 $ {G}_{1}={G}_{2}={G}_{3}=1.8 $ 0.51 0.52 0.47 0.34 $ {G}_{1}={G}_{2}={G}_{3}=3 $ 0.59 2.14 0.68 5.6 
DownLoad: CSV
表 2 对于不同的强度增益, 四模T-型簇态Nullifier的归一化方差
Table 2. Normalized variances of the four-mode T-type cluster state nullifiers for different intensity gains.
强度增益 Nullifier 1 Nullifier 2 Nullifier 3 Nullifier 4 $ {G}_{1}={G}_{2}={G}_{3}=1.2 $ 0.47 0.37 0.51 0.47 $ {G}_{1}={G}_{2}={G}_{3}=1.5 $ 0.36 0.26 0.4 0.4 $ {G}_{1}={G}_{2}={G}_{3}=1.8 $ 0.34 0.21 0.34 0.38 $ {G}_{1}={G}_{2}={G}_{3}=3 $ 2.63 0.56 0.56 3.79
DownLoad: CSV
表 3 对于不同的强度增益, 四模方型簇态Nullifier的归一化方差
Table 3. Normalized variances of the four-mode square cluster state nullifiers for different intensity gains.
强度增益 Nullifier 1 Nullifier 2 Nullifier 3 Nullifier 4 $ {G}_{1}={G}_{2}={G}_{3}=1.2 $ 0.36 0.54 0.36 0.34 $ {G}_{1}={G}_{2}={G}_{3}=1.5 $ 0.24 0.31 0.24 0.38 $ {G}_{1}={G}_{2}={G}_{3}=1.8 $ 0.58 0.20 0.38 0.64 $ {G}_{1}={G}_{2}={G}_{3}=3 $ 0.87 0.51 4.67 4.38
DownLoad: CSV
-
[1] Fabre C, Treps N 2020 Rev. Mod. Phys. 92 035005
Google Scholar
[2] Briegel H J, Browne D E, Dür W, Raussendorf R, Van den Nest M 2009 Nat. Phys. 5 19
Google Scholar
[3] Menicucci N C, Loock P V, Gu M, Weedbrook C, Ralph T C, Nielsen M A 2006 Phys. Rev. Lett. 97 110501
Google Scholar
[4] Su X L, Hao S H, Deng X W, Ma L Y, Wang M H, Jia X J, Xie C D, Peng K C 2013 Nat. Commun. 4 2828
Google Scholar
[5] Su X L, Zhao Y P, Hao S H, Jia X J, Xie C D, Peng K C 2012 Opt. Lett. 37 5178
Google Scholar
[6] Armstrong S, Morizur J F, Janousek J, Hage B, Treps N, Lam P K, Bachor H A 2012 Nat. Commun. 3 1026
Google Scholar
[7] Asavanant W, Shiozawa Y, Yokoyama S, et al. 2019 Science 366 373
Google Scholar
[8] Larsen M V, Guo X S, Breum C R, Neergaard-Nielsen J S, Andersen U L 2019 Science 366 369
Google Scholar
[9] Larsen M V, Guo X S, Breum C R, Neergaard-Nielsen J S, Andersen U L 2021 Nat. Phys. 17 1018
Google Scholar
[10] Cai Y, Roslund J, Ferrini G, Arzani F, Xu X, Fabre C, Treps N 2017 Nat. Commun. 8 15645
Google Scholar
[11] Barbosa F A S, Coelho A S, Muñoz-Martínez L F, Ortiz-Gutiérrez L, Villar A S, Nussenzveig P, Martinelli M 2018 Phys. Rev. Lett. 121 073601
Google Scholar
[12] Marino A M, Pooser R C, Boyer V, Lett P D 2009 Nature 457 859
Google Scholar
[13] Lawrie B, Evans P, Pooser R 2013 Phys. Rev. Lett. 110 156802
Google Scholar
[14] Qin Z Z, Cao L M, Wang H L, Marion A M, Zhang W P, Jing J T 2014 Phys. Rev. Lett. 113 023602
Google Scholar
[15] Liu S S, Lou Y B, Jing J T 2019 Phys. Rev. Lett. 123 113602
Google Scholar
[16] Pooser R C, Savino N, Batson E, Beckey J L, Garcia J, Lawrie B J 2020 Phys. Rev. Lett. 124 230504
Google Scholar
[17] McCormick C F, Boyer V, Arimondo E, Lett P D 2007 Opt. Lett. 32 178
Google Scholar
[18] Boyer V, Marino A M, Pooser P C, Lett P D 2008 Science 321 5888
[19] Cao L M, Du J J, Feng J L, Qin Z Z, Marino A M, Kolobov M I, Jing J T 2017 Opt. Lett. 42 7
[20] Pan X Z, Yu S, Zhou Y F, Zhang K, Zhang K, Lv S C, Li S J, Wang W, Jing J T 2019 Phys. Rev. Lett. 123 070506
Google Scholar
[21] Li S J, Pan X Z, Ren Y, Liu H Z, Yu S, Jing J T 2020 Phys. Rev. Lett. 124 083605
Google Scholar
[22] Wang W, Zhang K, Jing J T 2020 Phys. Rev. Lett. 125 140501
Google Scholar
[23] Liu S S, Lou Y B, Jing J T 2020 Nat. Commun. 11 3875
Google Scholar
[24] Marino A M, Pooser R C, Boyer V, Lett P D 2009 Nature 457 859
[25] Chen Y X, Liu S S, Lou Y B, Jing J T 2021 Phys. Rev. Lett. 127 093601
Google Scholar
[26] Liu S S, Lou Y B, Chen Y X 2021 Phys. Rev. Lett. 126 060503
Google Scholar
[27] Medeiros de Araújo R, Roslund J, Cai Y, Ferrini G, Fabre C, Treps N 2014 Phys. Rev. A 89 053828
Google Scholar
[28] Roslund J, Medeiros de Araújo R, Jing S F, Fabre C, Treps N 2014 Nat. Photonics 8 109
Google Scholar
[29] Ferrini G, Gazeau J P, Coudreau T, Fabre C, Treps N 2013 New J. Phys. 15 093015
Google Scholar
[30] Zhang K, Wang W, Liu S S, Pan X Z, Du J J, Lou Y B, Yu S, Lü S C, Treps N, Fabre C 2020 Phys. Rev. Lett. 124 090501
Google Scholar
[31] Simon R 2000 Phys. Rev. Lett. 84 2726
Google Scholar
[32] Werner R F, Wolf M M 2001 Phys. Rev. Lett. 86 3658
Google Scholar
[33] Braunstein S L 2005 Phys. Rev. A 71 055801
Google Scholar
[34] Hensen B, Bernien H 2005 Nature 526 682
[35] Lloyd S, Braunstein S L 1999 Phys. Rev. Lett. 82 1784
Google Scholar
[36] Braunstein S L, van Loock P 2005 Rev. Mod. Phys. 77 513
Google Scholar
[37] Weedbrook C, Pirandola S 2012 Rev. Mod. Phys. 84 621
Google Scholar
[38] Kimble H J 2008 Nature 453 1023
Google Scholar
[39] Guo X, Breum C R 2020 Nat. Phys. 16 281
Google Scholar
[40] Ferrini G, Roslund J, Arzani F, Fabre C, Treps N 2016 Phys. Rev. A 94 062332
Google Scholar
[41] Ferrini G, Roslund J, Arzani F, Cai Y, Fabre C, Treps N 2015 Phys. Rev. A 91 032314
Google Scholar
[42] Gu M, Weedbrook C, Menicucci N C, Ralph T C, Look P V 2009 Phys. Rev. A 79 062318
Google Scholar
[43] Menicucci N C, Flammia S T, Look P V 2011 Phys. Rev. A 83 042335
Google Scholar
[44] 郝树宏 2016 博士学位论文 (山西: 山西大学)
Hao S H 2016 Ph. D. Dissertation (Shanxi: Shanxi University) (in Chinese)
[45] van Loock P, Weedbrook C, Gu M 2007 Phys. Rev. A 76 032321
Google Scholar
[46] Cai Y, Feng J L, Wang H L, Ferrini G, Xu X Y, Jing J T, Treps N 2015 Phys. Rev. A 91 013843
Google Scholar
[47] van Loock P, Furusawa A 2003 Phys. Rev. A 67 052315
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4579
- PDF Downloads: 159
- Cited By: 0
