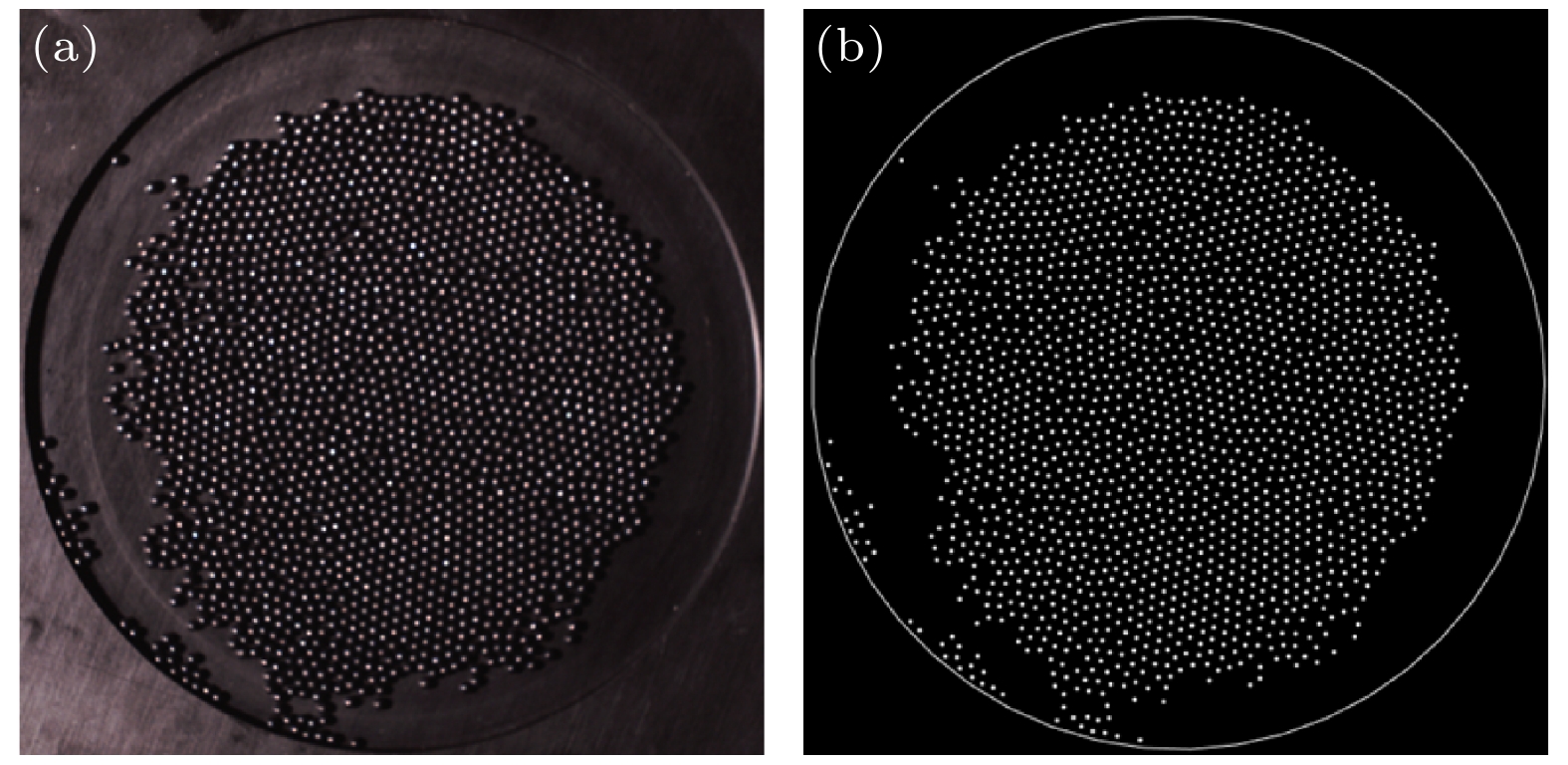
-
The study of structural defects in particle systems is of great value for studying solid-liquid melting. The volume fraction is a key parameter that can be used to accurately quantify the phase-transition process. The collective behavior and interaction form in a wet particle system are much more complex than that of a dry particle material because of the existence of liquid bridge force between the wet particles. In this paper, the structural defects and the critical value of solid-liquid transformation in the monolayer wet particles during solid-liquid melting under vertical vibration are experimentally studied. The contact model of the wet particle system is constructed according to experimental and theoretical analysis, and the structural changes of the particles in the melting process of the quasi-two-dimensional wet particle system are quantified. The Voronoi tessellation is established to study the phase transition of the particle system, and the local volume fraction is adopted to determine the state of structural defect change during melting. The experimental results indicate that the phase-transition process is caused by structural defects in the solid. The defects appear from the edge of the particle system, and the chain defect pairs spread to the center. The reason for structural defects at the edge of the cluster is that the particles at the edge of the cluster are subjected to less liquid bridge force, and the kinetic energy brought by the collision between the particles and the bottom wall makes the particles become active and begin to explore the available space. The chain defects are caused by the force chain generated by the fluid bridge force, which makes the particles tend to move together in rows. In addition, the local volume fraction of seven-phase defective particles decreases significantly and is much smaller than that of five-phase defective particles and six-phase defective particles when defects occur. Therefore, the evolution and the critical state of the structural defects can be quantified by measuring the change in the minimum local volume fraction (the local volume fraction of particles with 7-fold defects) in the particle system. The local volume fraction of the analysis shows that when the minimum local volume fraction ϕ ≤ 0.6652 defects occur, and when ϕ ≤ 0.4872 particle system transforms from solid to liquid.
-
Keywords:
- solid-liquid melting /
- defect /
- wet particles /
- local volume fraction
[1] Herminghaus S 2013 Wet Granular Matter: A Truly Complex Fluid (Vol. 5) (New York: World Scientific) pp1−14
[2] May C, Wild M, Rehberg I, Huang K 2013 Phys. Rev. E 6 062201
Google Scholar
[3] Gao Y, Yan W, Gao T, Chen Q, Li L 2020 Mat. Sci. Semicon. Proc. 116 105155
Google Scholar
[4] 李洋 2013 硕士毕业论文 (江苏: 苏州大学)
Li Y 2013 M. S. Dissertation (Jiangshu: Soochow University) (in Chinese)
[5] Ros V, Müller M, Scardicchio A 2015 Nucl. Phys. B 891 420
Google Scholar
[6] Andrea F, Kai H 2015 Phys. Rev. E 91 032206
Google Scholar
[7] Eisenmann C, Gasser U, Keim P, Maret G 2004 Phys. Rev. Lett. 93 105702
Google Scholar
[8] Sun X, Sakai M 2018 Chem. Eng. Sci. 182 28
Google Scholar
[9] Chen J, Williams K, Guo J 2019 13th International Conference on Bulk Materials Storage Gold Coast, Australia, July 17, 2019 pp716−720
[10] Bossler F, Koos E 2016 Langmuir. 32 1489
Google Scholar
[11] Ramming P, Huang K 2017 Cond-mat. Soft. 10 1051
Google Scholar
[12] Kosterlitz J M, Thouless D J 1973 J. Phys. C 6 1181
Google Scholar
[13] Nelson D R, Halperin B I 1979 Phys. Rev. B 19 2457
Google Scholar
[14] 席航波 2014 硕士毕业论文 (江苏: 苏州大学)
Xi H B 2014 M. S. Dissertation (Jiangshu: Soochow University) (in Chinese)
[15] Rafael, Jungmann, Paulo, Cesar, Nascimento 2018 J. Phys-Condens. Mat. 30 465402
Google Scholar
[16] Strauch S, Herminghaus S 2012 Soft Matter 8 8271
Google Scholar
[17] Schindler T, Kapfer S C 2019 Phys. Rev. E 99 022902
Google Scholar
[18] Olafsen J S, Urbach J S 2005 Phys. Rev. Lett. 95 098002
Google Scholar
[19] 孙晓燕 2014 博士毕业论文 (江苏: 苏州大学)
Sun X Y 2014 Ph. D. Dissertation (Jiangshu: Soochow University) (in Chinese)
[20] Levashov V A, Ryltsev R, Chtchelkatchev N 2019 Soft Matter 15 8840
Google Scholar
[21] Lei C, Ruan J 2010 Biodata. Min. 3 9
Google Scholar
[22] Scheel M, Seemann R, Brinkmann M 2008 Nat. Mater. 7 189
Google Scholar
[23] Huang K 2015 J. Mater. Sci. Lett. 12 p1690
Google Scholar
[24] Agosta L, Metere A, Dzugutov M 2018 Phys. Rev. E 97 052702
Google Scholar
[25] Bertola V, Haw M D 2015 Powder Technol. 270 412
Google Scholar
[26] Dino R, Rodrigo S, Guzmán Marcelo 2018 Phys. Rev. E 98 022901
Google Scholar
[27] Luu L H, Castillo G, Mujica N, Rodrigo S 2013 Phys. Rev. E 87 040202
Google Scholar
[28] Du D, Doxastakis M, Hilou E, Biswal L 2017 Soft Matter 13 1548
Google Scholar
[29] Devroye L, László Györfi, Gábor Lugosi 2015 J. Appl. Probab. 54 2
Google Scholar
[30] Williamson J J, Evans R M L 2014 J. Chem. Phys. 141 148101
Google Scholar
-
图 1 实验装置示意图
Figure 1. Schematic of experimental setup.
图 2 (a)原始图像; (b)颗粒的定位
Figure 2. (a) The original image; (b) particle localization.
图 3 颗粒的局部结构识别示意图
Figure 3. Schematic diagram of local structure identification of particles.
图 4 接触模型的结构形式图, 蓝色表示自由颗粒, 在颗粒系统中没有接触颗粒; 红色表示具有六个接触颗粒的六角相颗粒.
Figure 4. Diagram of the structure of the contact model. The blue color represents free particles, and there are no particles in contact with the particle system. Hexagonal phase particles with six contact particles in red.
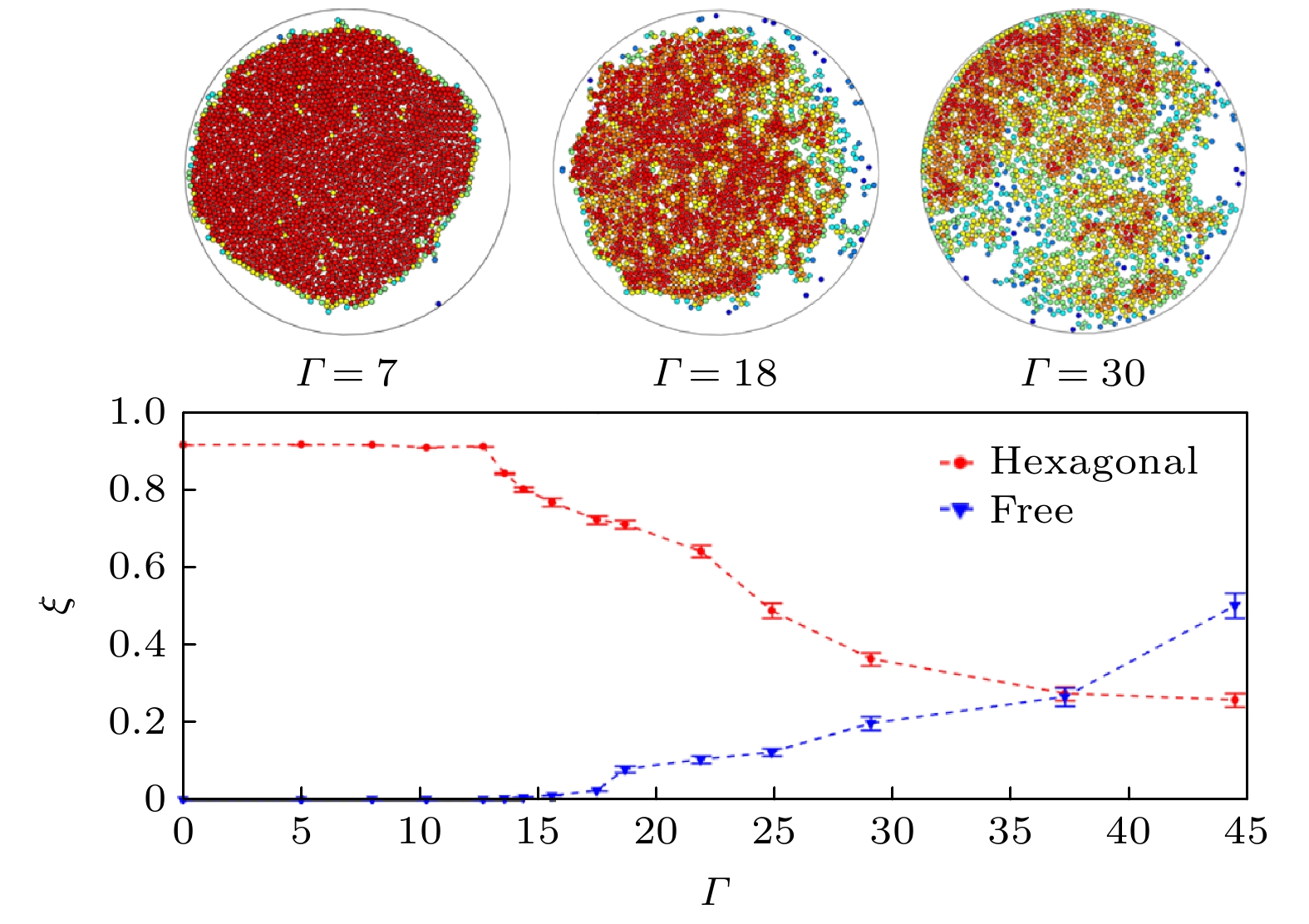
图 5 不同加速度下单层湿颗粒在团聚后颗粒系统的结构变化图. 颗粒的结构是采用BOO参数识别并颜色表征后的结果, 曲线图表示了随着加速度的增加, 六角相和自由颗粒所占全局颗粒的百分比
$ \xi $ 的变化.Figure 5. The structure change diagram of single layer wet particle system after agglomeration under different accelerations. The particle structure is the result of BOO parameter identification and color characterization. The curve shows the change of the proportion of hexagonal phase and free particles in the system.
图 6 (a), (b), (c)分别为在
$ \varGamma =14, 14.4, 15 $ 时颗粒在Voronoi cell中的结构形式; (d), (e), (f) 的柱状图分别展示了图6(a), (b), (c)加速度下颗粒的局部体积分数, 红色柱子表示结构为7-fold颗粒的局部体积分数, 蓝色柱子表示结构为5-fold颗粒的局部体积分数, 黑色柱子为发生缺陷前结构为六角相颗粒的局部体积分数Figure 6. (a), (b), (c), respectively in when Γ = 14, 14.4, 15 particles in the Voronoi cell structure of the form; the histograms of (d), (e), (f) respectively show the local volume fraction of particles under the acceleration of Figs. 6 (a), (b) and (c). The red column represents the local volume fraction of 7-fold particles, the blue column represents the local volume fraction of 5-fold particles, and the black column represents the local volume fraction of hexagonal phase particles before the occurrence of defects.
图 7 不同振动强度下颗粒系统中局部体积分数的变化曲线. 其中红色曲线表示系统中最小的局部体积分数变化, 蓝色表示系统中所有颗粒的平均局部体积分数变化. 图中Voronoi cell表示在该加速度下颗粒系统的“相”位图, 其中Voronoi cell右边缘处于颗粒系统中团簇的边缘位置
Figure 7. The change curve of local volume fraction in particle system under different vibration intensity. The red curve represents the minimum local volume fraction change in the system, while the blue curve represents the average local volume fraction change of all particles in the system. Voronoi cell in the figure represents the phase diagram of the particle system under this acceleration, where the right edge of Voronoi cell is located at the edge of the cluster in the particle system.
图 8 最小局部体积分数
$ \varphi $ 的增减变化趋势, 红色曲线表示加速度$ \varGamma $ 从0依次增加到44时颗粒系统中最小局部体积分数的变化, 黑色曲线表示加速度$ \varGamma $ 从44依次减小到0时颗粒系统中最小局部体积分数的变化Figure 8. The trend of the increase and decrease of the minimum local volume fraction
$ \varphi $ . The red curve shows the change of the minimum local volume fraction in the particle system as the acceleration gradually increases from 0 to 44, and the black curve shows the change of the minimum local volume fraction in the particle system as the acceleration decreases from 44 to 0.表 1 颗粒参数
Table 1. Particle parameters.
参数 值 颗粒直径d/mm $ 2.0\pm 0.02 $ 初始含水量W/% $ 3{\%} $ 颗粒数N $ 2355 $ 颗粒粗糙度${C}_{\rm{d} }/$μm $ 5 $ 弹性模数/GPa $ 63 $ 全局颗粒面积分数$ \varphi $/% $ 55.7 $ 
DownLoad: CSV
-
[1] Herminghaus S 2013 Wet Granular Matter: A Truly Complex Fluid (Vol. 5) (New York: World Scientific) pp1−14
[2] May C, Wild M, Rehberg I, Huang K 2013 Phys. Rev. E 6 062201
Google Scholar
[3] Gao Y, Yan W, Gao T, Chen Q, Li L 2020 Mat. Sci. Semicon. Proc. 116 105155
Google Scholar
[4] 李洋 2013 硕士毕业论文 (江苏: 苏州大学)
Li Y 2013 M. S. Dissertation (Jiangshu: Soochow University) (in Chinese)
[5] Ros V, Müller M, Scardicchio A 2015 Nucl. Phys. B 891 420
Google Scholar
[6] Andrea F, Kai H 2015 Phys. Rev. E 91 032206
Google Scholar
[7] Eisenmann C, Gasser U, Keim P, Maret G 2004 Phys. Rev. Lett. 93 105702
Google Scholar
[8] Sun X, Sakai M 2018 Chem. Eng. Sci. 182 28
Google Scholar
[9] Chen J, Williams K, Guo J 2019 13th International Conference on Bulk Materials Storage Gold Coast, Australia, July 17, 2019 pp716−720
[10] Bossler F, Koos E 2016 Langmuir. 32 1489
Google Scholar
[11] Ramming P, Huang K 2017 Cond-mat. Soft. 10 1051
Google Scholar
[12] Kosterlitz J M, Thouless D J 1973 J. Phys. C 6 1181
Google Scholar
[13] Nelson D R, Halperin B I 1979 Phys. Rev. B 19 2457
Google Scholar
[14] 席航波 2014 硕士毕业论文 (江苏: 苏州大学)
Xi H B 2014 M. S. Dissertation (Jiangshu: Soochow University) (in Chinese)
[15] Rafael, Jungmann, Paulo, Cesar, Nascimento 2018 J. Phys-Condens. Mat. 30 465402
Google Scholar
[16] Strauch S, Herminghaus S 2012 Soft Matter 8 8271
Google Scholar
[17] Schindler T, Kapfer S C 2019 Phys. Rev. E 99 022902
Google Scholar
[18] Olafsen J S, Urbach J S 2005 Phys. Rev. Lett. 95 098002
Google Scholar
[19] 孙晓燕 2014 博士毕业论文 (江苏: 苏州大学)
Sun X Y 2014 Ph. D. Dissertation (Jiangshu: Soochow University) (in Chinese)
[20] Levashov V A, Ryltsev R, Chtchelkatchev N 2019 Soft Matter 15 8840
Google Scholar
[21] Lei C, Ruan J 2010 Biodata. Min. 3 9
Google Scholar
[22] Scheel M, Seemann R, Brinkmann M 2008 Nat. Mater. 7 189
Google Scholar
[23] Huang K 2015 J. Mater. Sci. Lett. 12 p1690
Google Scholar
[24] Agosta L, Metere A, Dzugutov M 2018 Phys. Rev. E 97 052702
Google Scholar
[25] Bertola V, Haw M D 2015 Powder Technol. 270 412
Google Scholar
[26] Dino R, Rodrigo S, Guzmán Marcelo 2018 Phys. Rev. E 98 022901
Google Scholar
[27] Luu L H, Castillo G, Mujica N, Rodrigo S 2013 Phys. Rev. E 87 040202
Google Scholar
[28] Du D, Doxastakis M, Hilou E, Biswal L 2017 Soft Matter 13 1548
Google Scholar
[29] Devroye L, László Györfi, Gábor Lugosi 2015 J. Appl. Probab. 54 2
Google Scholar
[30] Williamson J J, Evans R M L 2014 J. Chem. Phys. 141 148101
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4150
- PDF Downloads: 58
- Cited By: 0
