Dark soliton molecules in nonlinear optics

Xu Dan-Hong; Lou Sen-Yue

doi:10.7498/aps.69.20191347

Abstract

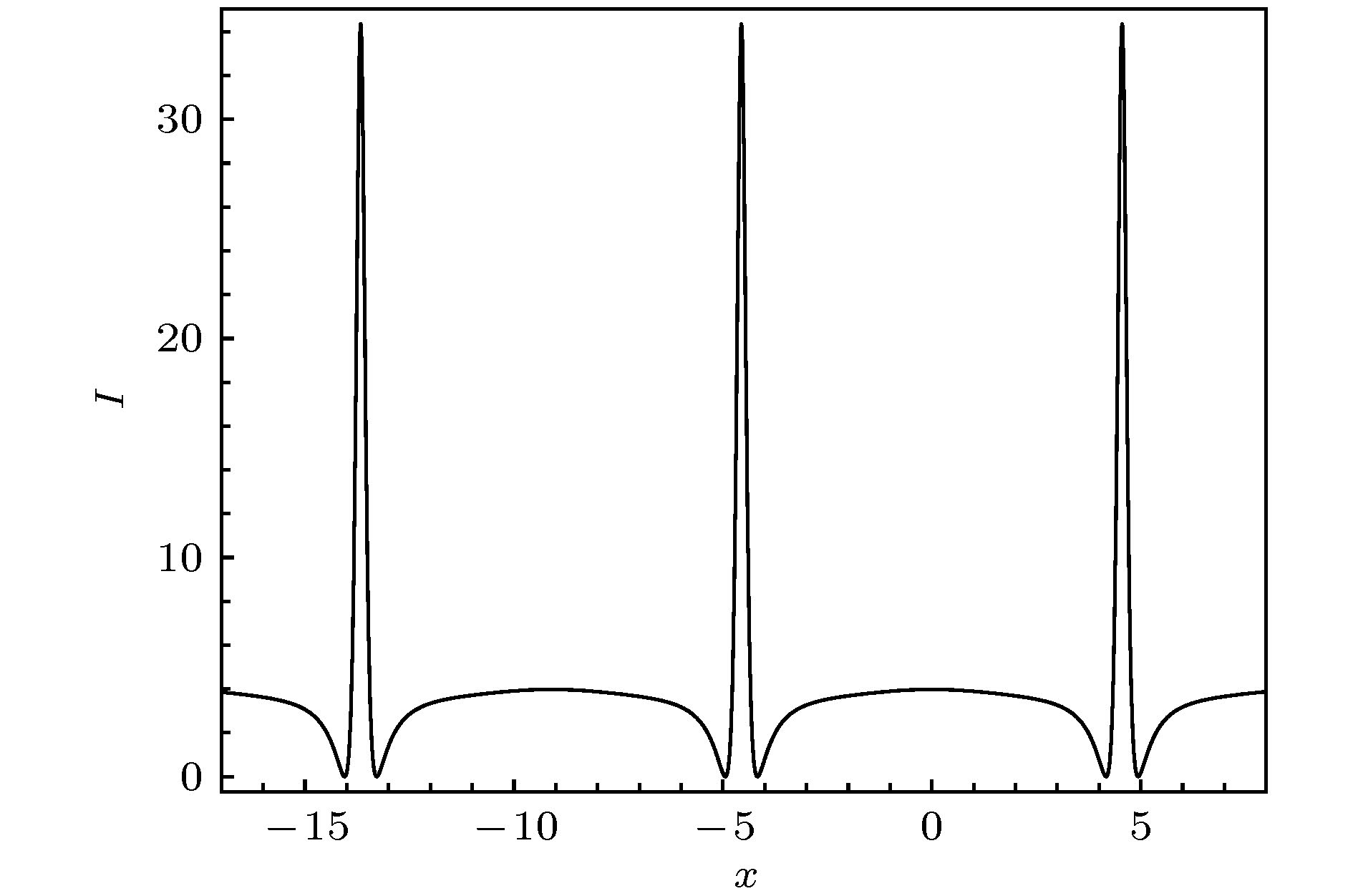
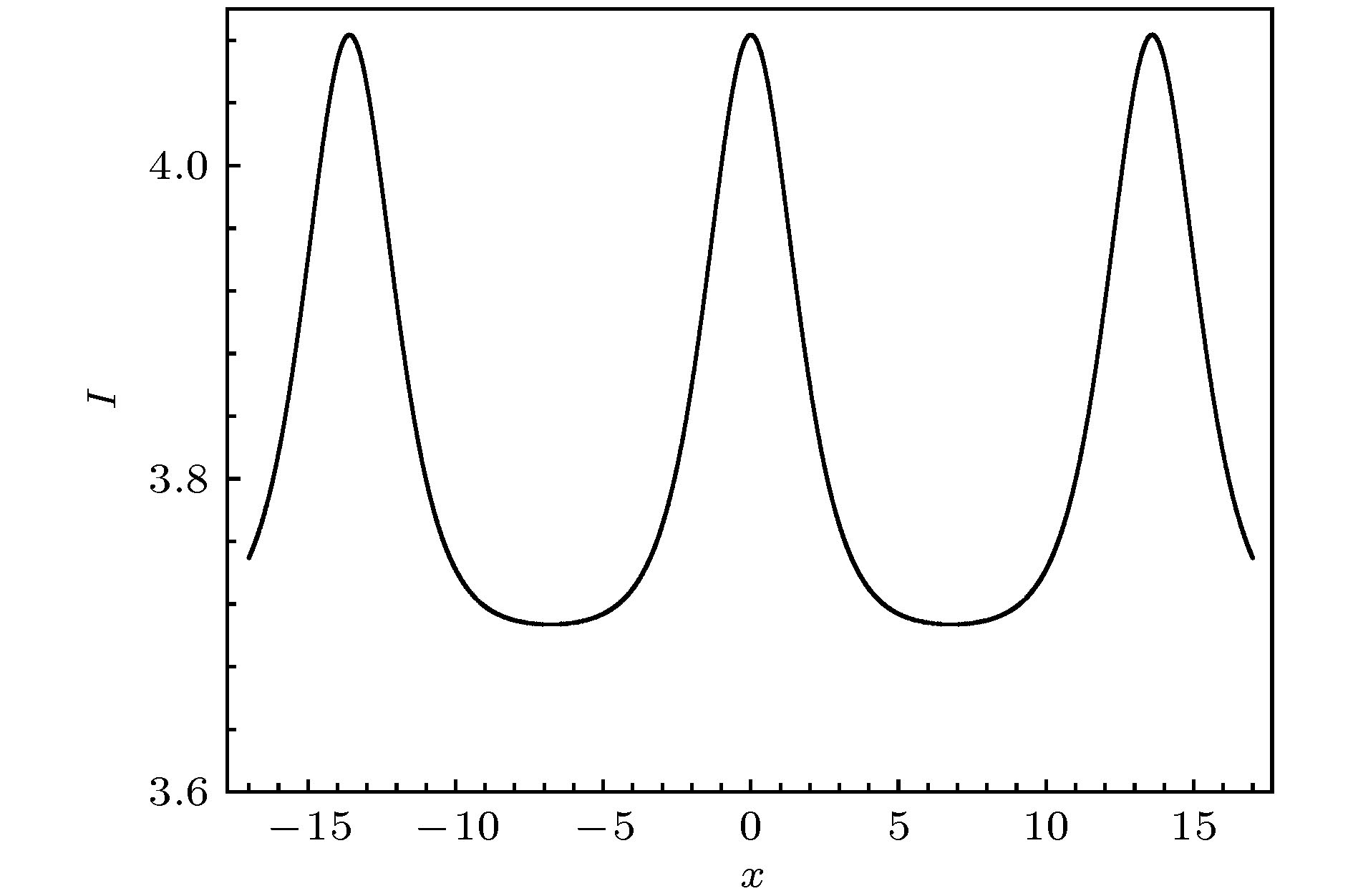
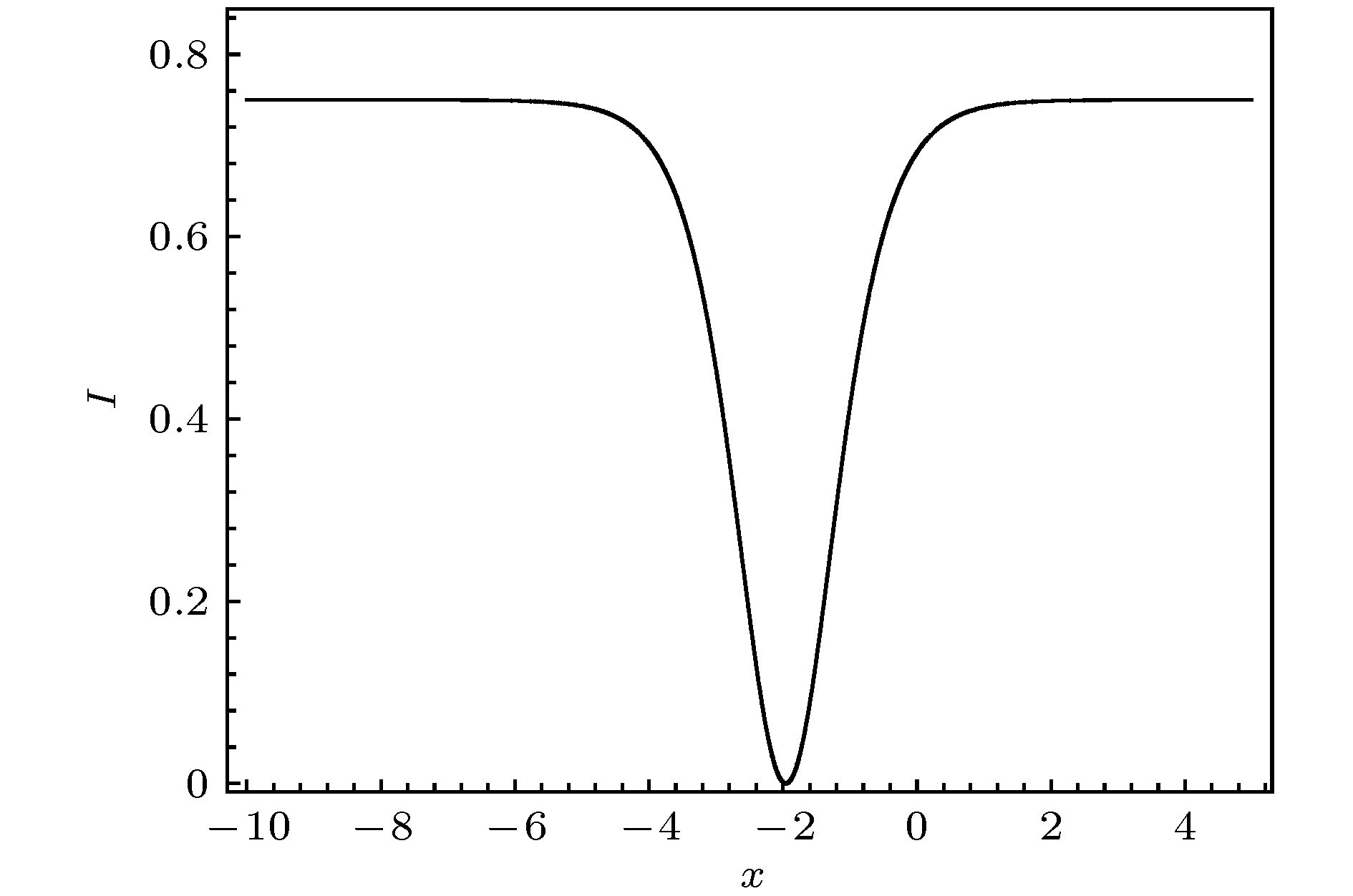
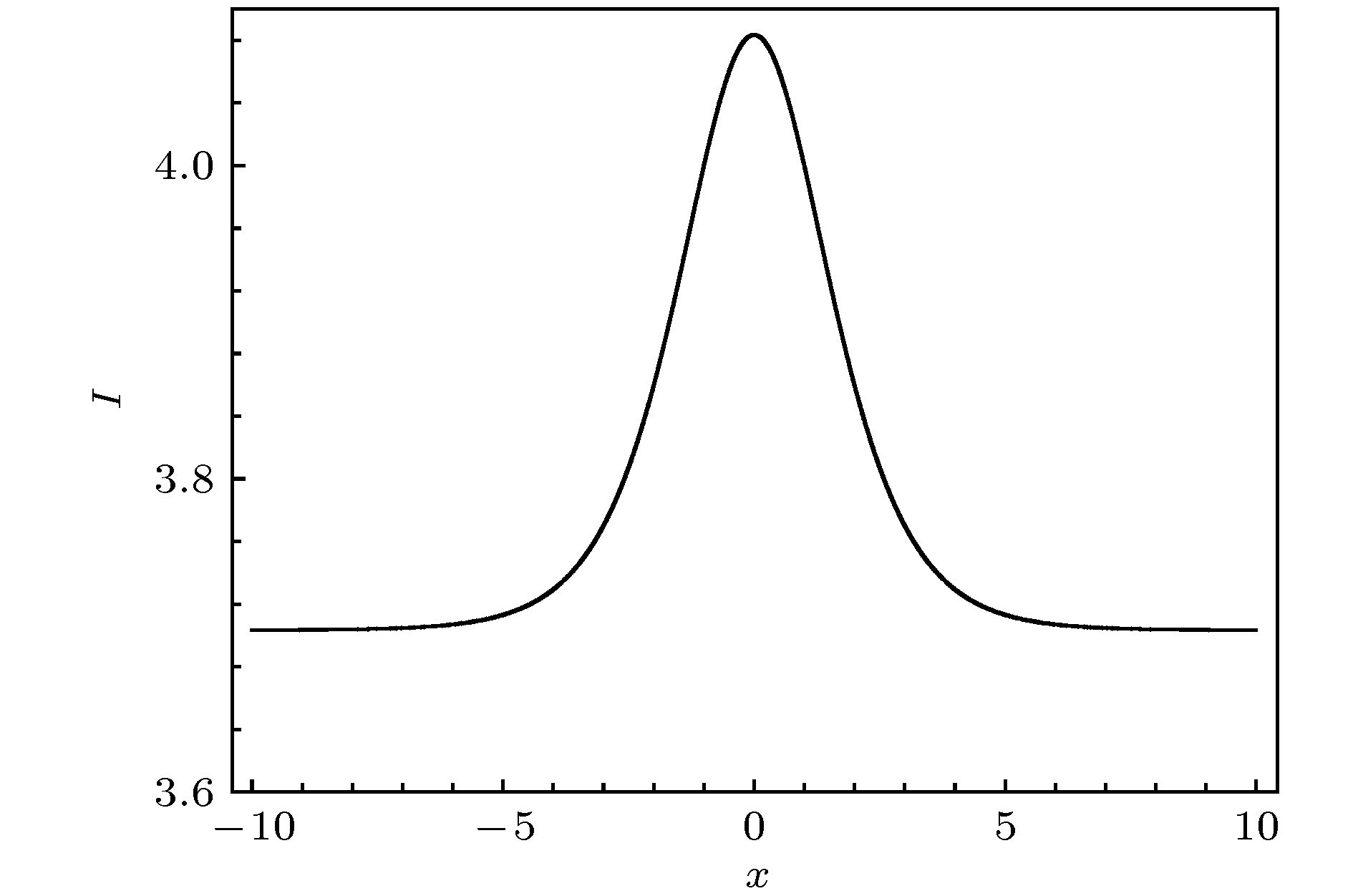
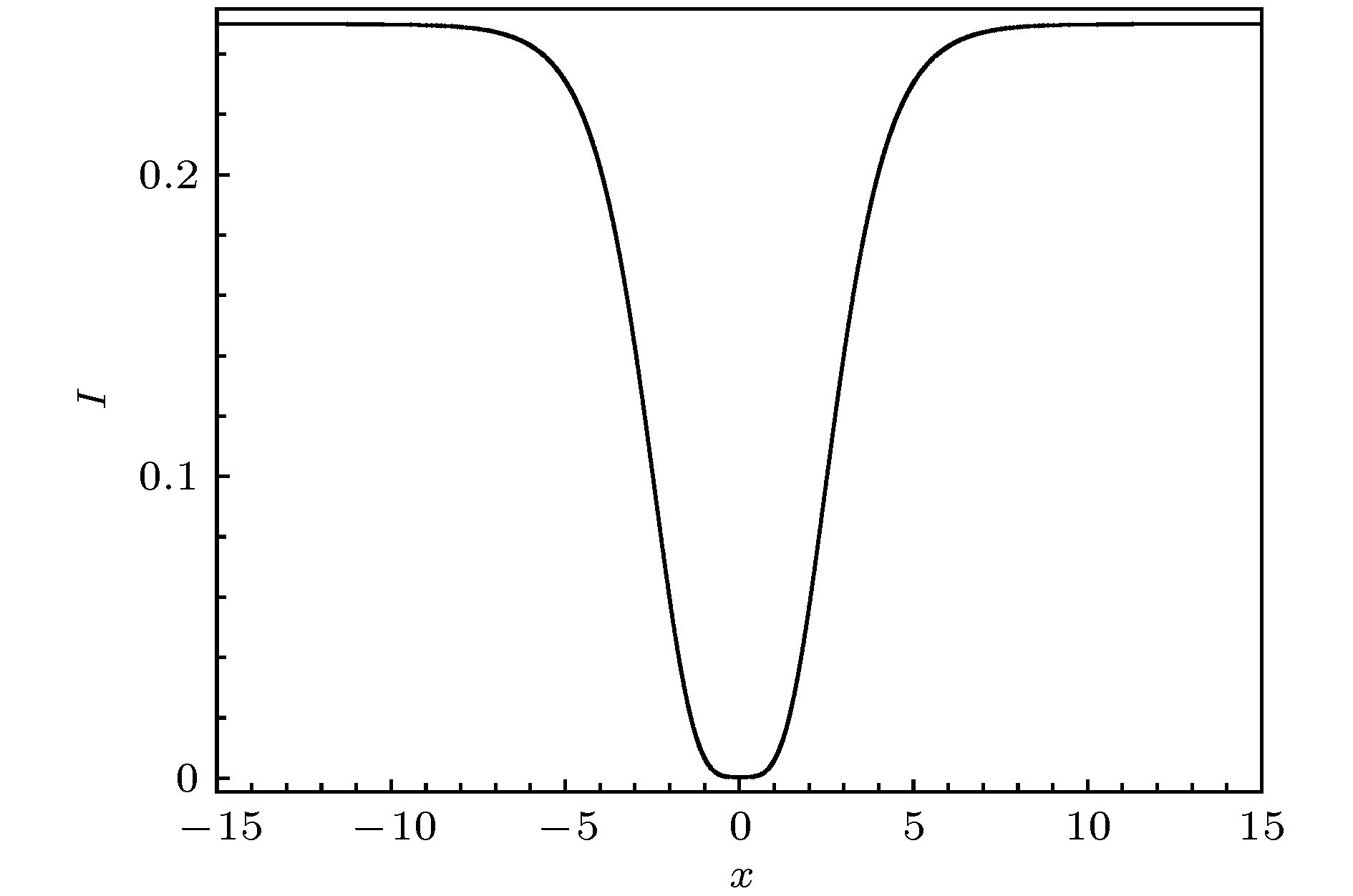
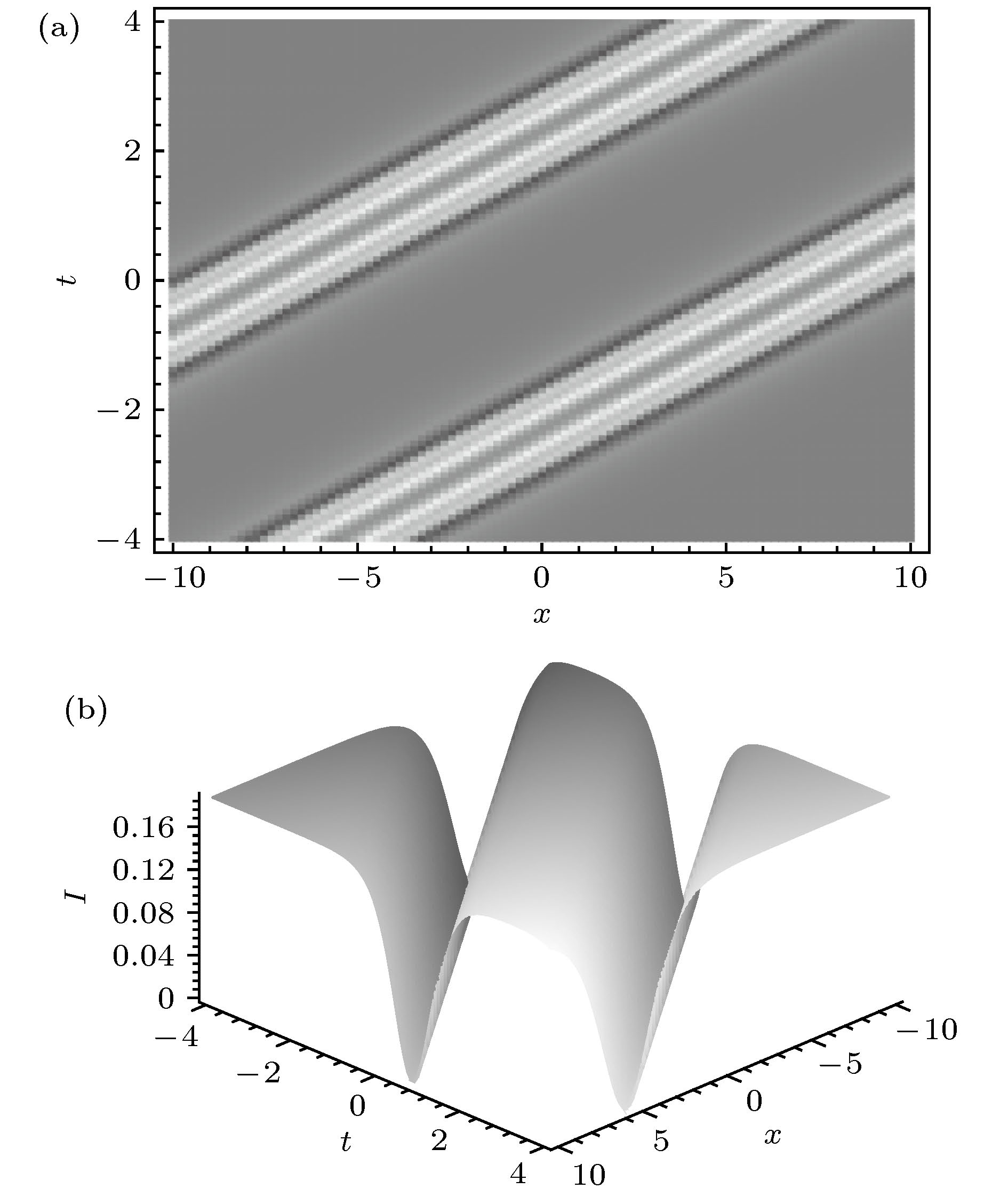
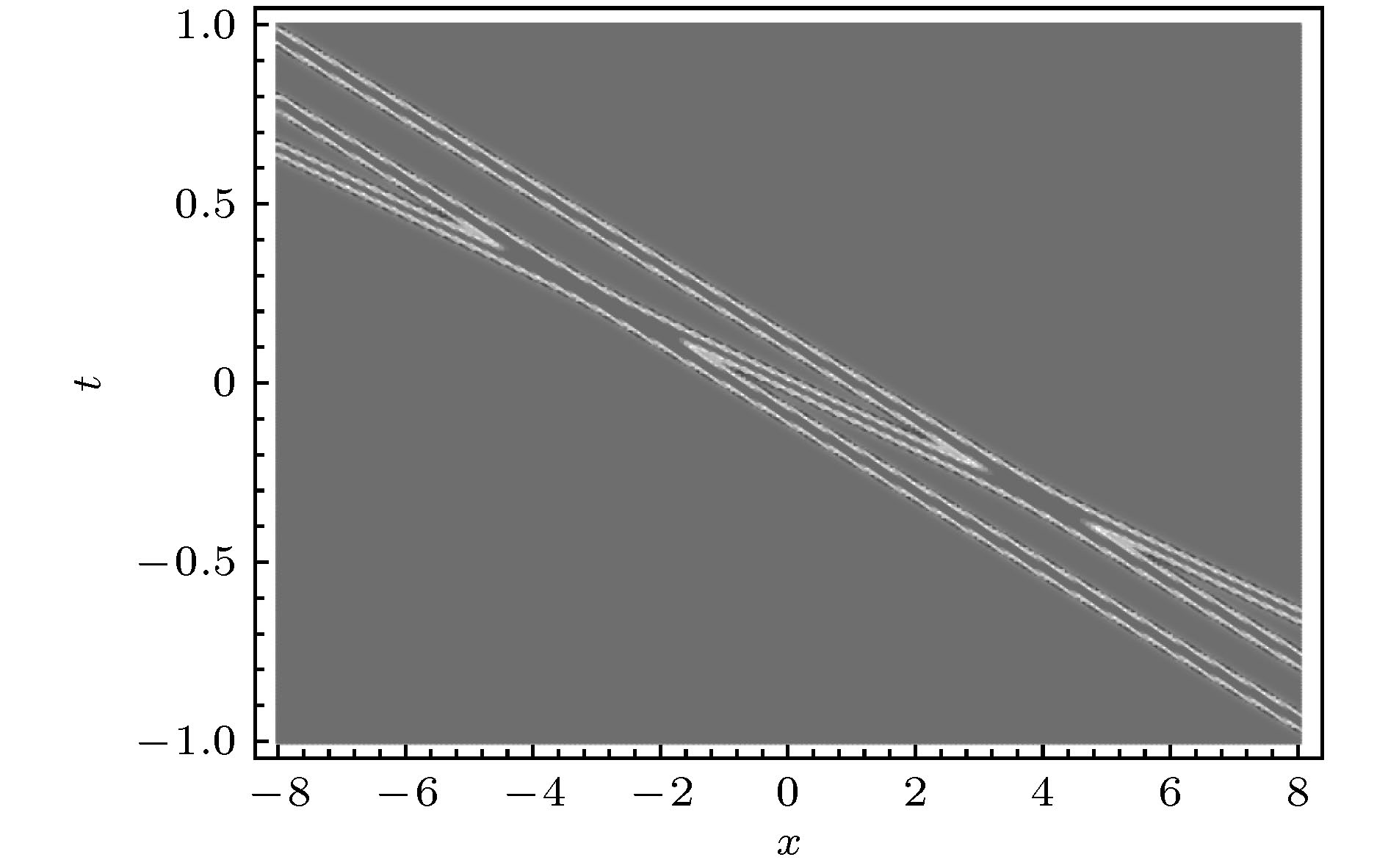
The study on soliton molecules is one of the important topics in nonlinear science especially in nonlinear optics. The bright soliton molecules have been experimentally observed in optics, however, the dark soliton molecules have not yet been experimentally observed. Theoretically, the soliton molecules have been found for some coupled nonlinear systems. Nevertheless, the soliton molecules have not been obtained for non-coupled single component nonlinear models. In this paper, we first study the exact periodic waves (soliton lattices) and solitary waves for a nonlinear nonintegrable optical model with second and third order dispersions and high order nonlinear effects including self-steeping, Raman scattering and nonlinear dispersion. Two types of dark soliton lattice and three types of soliton lattice are explicitly exhibited for general nonintegrable system. Five types of bright (with and without gray background), dark and gray solitons can be obtained from the limit cases of the modules of the soliton lattices. For an integrable case, using a novel generalized bilinear form of a single component nonlinear system, the multi-soliton solutions are obtained and expressed by a completely new form which are invariant under the full reversal transformations such as the parity, the time reversal, the charge conjugate and the field reversal. To find soliton molecules, a novel mechanism, the velocity resonant, is proposed. Starting from the multi-soliton solutions and using the velocity resonance mechanism, the analytical expression of the dark soliton molecules can be readily obtained. For the model given in this paper, the integrable higher order nonlinear Schrodinger equation, one can proved that the interactions among the dark soliton molecules and the usually solitons are elastic. It is worth pointing out that soliton molecules can also exist in the case of nonintegrable systems.

Keywords:

Authors and contacts

School of Physical Science and Technology, Ningbo University, Ningbo 315211, China

Corresponding author: Lou Sen-Yue, lousenyue@nbu.edu.cn

Funds: National Natural Science Foundation of China (Nos. 11975131, 11435005)

FullText HTML

References

[1]	Kivshar Y S, Malomed B A 1989 Rev. Mod. Phys. 61 763 Google Scholar
[2]	Köttig F, Tani T, Travers J C, Russell P St J 2017 Phys. Rev. Lett. 118 263902 Google Scholar
[3]	Strogatz S 2001 Nature (London) 410 268 Google Scholar
[4]	Forte S 1992 Rev. Mod. Phys. 64 193 Google Scholar
[5]	Hertog T, Horowitz G T 2005 Phys. Rev. Lett. 94 221301 Google Scholar
[6]	Drummond P D, Kheruntsyan K V, He H 1998 Phys. Rev. Lett. 81 3055 Google Scholar
[7]	Lou S Y, Huang F 2017 Sci. Rep. 7 869 Google Scholar
[8]	Wright L G, Christodoulides D N, Wise F W 2017 Science 358 94 Google Scholar
[9]	Dudley J M, Dias F, Erkintalo M, Genty G 2014 Nat. Photonics 8 755 Google Scholar
[10]	Stratmann M, Pagel T, Mitschke F 2005 Phys. Rev. Lett. 95 143902 Google Scholar
[11]	Herink G, Kurtz F, Jalali B, Solli D R, Ropers C 2017 Science 356 50 Google Scholar
[12]	Liu X M, Yao X K, Cui Y D 2018 Phys. Rev. Lett. 121 023905 Google Scholar
[13]	徐中巍, 张祖兴 2013 62 104210 Google Scholar Xu Z W, Zhang Z X, 2013 Acta Phys. Sin. 62 104210 Google Scholar
[14]	Sheppard A P, Kivshar Y S 1997 Phys. Rev. E 55 4773 Google Scholar
[15]	Lakomy K, Nath R, Santos L 2012 Phys. Rev. A 86 013610 Google Scholar
[16]	Lou S Y 2019 arxiv: 1909.03399 v1[nlin.SI]
[17]	Hirota R 1971 Phys. Rev. Lett., 27 1192 Google Scholar
[18]	Liu S J, Tang X Y, Lou S Y 2018 Chin. Phys. B 27 060201 Google Scholar
[19]	Ablowitz M J, Clarkson P A 1991 Solitons, Nonlinear Evolution Equations and Inverse Scattering (Cambridge: Cambridge University Press)
[20]	Li Y Q, Chen J C, Chen Y, Lou S Y 2014 Chin. Phys. Lett. 31 010201 Google Scholar
[21]	陈登远 2006 孤子引论 (北京: 科学出版社) 第14−42页 Chen D Y 2006 Introduction on Solitons (Beijing: China Science Publishing and Media Ltd) pp14−42
[22]	Lou S Y 2018 J. Math. Phys. 59 083507 Google Scholar
[23]	Sasa N, Satsuma J 1991 J. Phys. Soc. Jpn. 60 409 Google Scholar
[24]	Hirota R 1973 J. Math. Phys. 14 805 Google Scholar
[25]	Kaup D J, Newell A C 1978 J. Math. Phys. 19 798
[26]	楼森岳 2020 69 010503 Google Scholar Lou S Y 2020 Acta Phys. Sin. 69 010503 Google Scholar

Cited By

图 1 由(15) 式描述的亮孤子晶格, 其中参数由(16)式给定

Figure 1. Bright soliton lattice described by Eq.(15) with the parameter selected from Eq. (16)

DownLoad: Full-Size Img PowerPoint

图 2 由(15)式描述的暗孤子晶格, 其中参数由 (17)式给定

Figure 2. Dark soliton lattice described by Eq. (15) with the parameter selected from Eq. (17)

DownLoad: Full-Size Img PowerPoint

图 3 由(18)式描述第二类亮孤子晶格, 其中参数由 (20)式给定

Figure 3. Second type of bright soliton lattice described by Eq. (18) with the parameter selected from Eq. (20)

DownLoad: Full-Size Img PowerPoint

图 4 第三类亮孤子晶格. 由(21)式描述, 其中参数由 (23)式给定

Figure 4. Third type of bright soliton lattice described by Eq. (21) with the parameter selected from Eq. (23)

DownLoad: Full-Size Img PowerPoint

图 5 第二类暗孤子晶格由(21)式描述, 其中参数由 (24)式给定

Figure 5. Second type of dark soliton lattice described by Eq. (21) with the parameter selected from Eq. (24)

DownLoad: Full-Size Img PowerPoint

图 6 由(25)式描述的暗孤子, 其中参数由 (26)式给定

Figure 6. Dark soliton described by Eq. (25) with the parameter selected from Eq. (26)

DownLoad: Full-Size Img PowerPoint

图 7 由(27)式描述的具有灰背景的亮孤子, 其中参数由 (29)式给定

Figure 7. Bright soliton (with gray background) described by Eq. (27) with the parameter selected from Eq. (29)

DownLoad: Full-Size Img PowerPoint

图 8 由(27)式描述的暗孤子, 其中参数由 (30)式给定

Figure 8. Dark soliton described by Eq. (27) with the parameter selected from Eq. (30)

DownLoad: Full-Size Img PowerPoint

图 9 (a)由(27)式描述的暗孤子分子的密度图; (b) 与(a)对应的立体图, 图中参数由 (31)式给定

Figure 9. (a)Density plot of the dark soliton molecule described by Eq. (27) with the parameter selected from Eq. (31); (b) three dimensional plot related to Fig.(a)

DownLoad: Full-Size Img PowerPoint

图 10 由(42)−(43)式描述的二暗孤子相互作用的密度图, 图中参数由 (44)式给定

Figure 10. Density plot of the interaction between two dark solitons described by Eq. (42)and Eq. (43) with the parameter selected from Eq. (44)

DownLoad: Full-Size Img PowerPoint

图 11 (a) 由(42)式和(43)式描述的暗孤子分子密度图, 图中参数由 (47)式给定; (b) 与图(a)对应的三维立体图

Figure 11. (a) Density plot of the dark soliton molecule described by Eq. (42) and Eq.(43) with the parameter selected from Eq. (47); (b) three dimensional plot related to Fig. (a)

DownLoad: Full-Size Img PowerPoint

图 12 由(34)式，(37)式和(38)式描述的暗孤子分子和暗孤子的弹性相互作用的密度图, 图中参数由 (49)式给定

Figure 12. Density plot of the interaction between a dark soliton molecule and a dark soliton described by Eq. (34), Eq. (37) and Eq. (38) with the parameter selected from Eq. (49)

DownLoad: Full-Size Img PowerPoint

图 13 由(34)式，(37)式和(38)式描述的二暗孤子分子的弹性相互作用的密度图, 图中参数由 (50)式给定

Figure 13. Density plot of the interaction between two dark soliton molecules described by Eq. (34), Eq. (37) and Eq. (38) with the parameter selected from Eq. (50)

DownLoad: Full-Size Img PowerPoint

map

[1]	Kivshar Y S, Malomed B A 1989 Rev. Mod. Phys. 61 763 Google Scholar
[2]	Köttig F, Tani T, Travers J C, Russell P St J 2017 Phys. Rev. Lett. 118 263902 Google Scholar
[3]	Strogatz S 2001 Nature (London) 410 268 Google Scholar
[4]	Forte S 1992 Rev. Mod. Phys. 64 193 Google Scholar
[5]	Hertog T, Horowitz G T 2005 Phys. Rev. Lett. 94 221301 Google Scholar
[6]	Drummond P D, Kheruntsyan K V, He H 1998 Phys. Rev. Lett. 81 3055 Google Scholar
[7]	Lou S Y, Huang F 2017 Sci. Rep. 7 869 Google Scholar
[8]	Wright L G, Christodoulides D N, Wise F W 2017 Science 358 94 Google Scholar
[9]	Dudley J M, Dias F, Erkintalo M, Genty G 2014 Nat. Photonics 8 755 Google Scholar
[10]	Stratmann M, Pagel T, Mitschke F 2005 Phys. Rev. Lett. 95 143902 Google Scholar
[11]	Herink G, Kurtz F, Jalali B, Solli D R, Ropers C 2017 Science 356 50 Google Scholar
[12]	Liu X M, Yao X K, Cui Y D 2018 Phys. Rev. Lett. 121 023905 Google Scholar
[13]	徐中巍, 张祖兴 2013 62 104210 Google Scholar Xu Z W, Zhang Z X, 2013 Acta Phys. Sin. 62 104210 Google Scholar
[14]	Sheppard A P, Kivshar Y S 1997 Phys. Rev. E 55 4773 Google Scholar
[15]	Lakomy K, Nath R, Santos L 2012 Phys. Rev. A 86 013610 Google Scholar
[16]	Lou S Y 2019 arxiv: 1909.03399 v1[nlin.SI]
[17]	Hirota R 1971 Phys. Rev. Lett., 27 1192 Google Scholar
[18]	Liu S J, Tang X Y, Lou S Y 2018 Chin. Phys. B 27 060201 Google Scholar
[19]	Ablowitz M J, Clarkson P A 1991 Solitons, Nonlinear Evolution Equations and Inverse Scattering (Cambridge: Cambridge University Press)
[20]	Li Y Q, Chen J C, Chen Y, Lou S Y 2014 Chin. Phys. Lett. 31 010201 Google Scholar
[21]	陈登远 2006 孤子引论 (北京: 科学出版社) 第14−42页 Chen D Y 2006 Introduction on Solitons (Beijing: China Science Publishing and Media Ltd) pp14−42
[22]	Lou S Y 2018 J. Math. Phys. 59 083507 Google Scholar
[23]	Sasa N, Satsuma J 1991 J. Phys. Soc. Jpn. 60 409 Google Scholar
[24]	Hirota R 1973 J. Math. Phys. 14 805 Google Scholar
[25]	Kaup D J, Newell A C 1978 J. Math. Phys. 19 798
[26]	楼森岳 2020 69 010503 Google Scholar Lou S Y 2020 Acta Phys. Sin. 69 010503 Google Scholar

Catalog

Vol. 69, Issue 1 - 2020-01-05

Metrics

Abstract views: 15088
PDF Downloads: 494
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板