-
Topological sound has enriched the way of implementing the sound manipulation, which can effectively suppress the backscattering due to topological protection. As an inherent longitudinal wave, sound wave has no " spin” and only supports longitudinal vibration. Creating the " pseudospin” degree of freedom is crucial to topological state for acoustic wave. In previous studies, a circulating fluid flow in the background field is introduced to break the reciprocity of wave propagation in an acoustic system, which still faces technically a challenge. On the other hand, acoustic analogues of quantum spin Hall state and valley Hall state are realized by relying on the Kramers doublet in the lattices with C6 symmetry and the broken mirror symmetry or inversion symmetry, respectively. In these cases, the distributions of acoustic energy flux in the unit cells emulate the pseudospins. Based on the band inversion, the topological sound carrying pseudospin is implemented at the interface between topologically trivial and non-trivial sonic crystal. Because of the close relevance to the lattice symmetry, these pseudospin-based topological state in the time-reversal invariant system is sensitive to structural defects. In this work, we investigate the topological sound in chiral sonic crystal consisting of resonant air tubes. The counterclockwise and clockwise length variation of air tube correspond to different topological phases. A defect meta-molecule is created at the symmetric interface, which supports resonant state in the band gap. The interface state occurs at the boundary between two opposite chiral sonic crystals. Owing to the resonant structure, we realize subwavelength topological sound transport with a subwavelength-transverse confinement. For the state carrying monopolar-mode symmetry, it is expected to preserve the mode symmetry under randomly introduced defects. As anticipated, the numerical results show that the topological sound has very strong robustness against various defects, such as the variation of positions and length of air tube. Finally, we utilize the field symmetry of topological sound in chiral sonic crystal to realize robust edge transport along soft or rigid boundary. Through the mirror symmetry operation of soft or rigid boundary, we construct an interface between the real lattice and its virtual image. The approach greatly reduces the dimension of sonic crystal device. Our work may conduce to the advances in topological acoustics, since the subwavelength-scale topological state promotes the applications of miniaturized acoustic devices. -
Keywords:
- sonic crystals /
- acoustic topological insulators /
- subwavelength scale
[1] Bernevig B A, Hughes T L, Zhang S C 2006 Science 314 1757
Google Scholar
[2] Hasan M Z, Kane C L 2010 Rev. Mod. Phys. 82 3045
Google Scholar
[3] Lu L, Joannopoulos J D, Soljačić M 2014 Nat. Photonics 8 821
Google Scholar
[4] Wu L H, Hu X 2015 Phys. Rev. Lett. 114 223901
Google Scholar
[5] Fleury R, Khanikaev A B, Alu A 2016 Nat. Commun. 7 11744
Google Scholar
[6] He C, Ni X, Ge H, Sun X C, Chen Y B, Lu M H, Liu X P, Chen Y F 2016 Nat. Phys. 12 1124
Google Scholar
[7] Peng Y G, Qin C Z, Zhao D G, Shen Y X, Xu X Y, Bao M, Jia H, Zhu X F 2016 Nat. Commun. 7 13368
Google Scholar
[8] Lu J, Qiu C, Ye L, Fan X, Ke M, Zhang F, Liu Z 2017 Nat. Phys. 13 369
Google Scholar
[9] Kang Y, Ni X, Cheng X, Khanikaev A B, Genack A Z 2018 Nat. Commun. 9 3029
Google Scholar
[10] Yan M, Lu J, Li F, Deng W, Huang X, Ma J, Liu Z 2018 Nat. Mater. 17 993
Google Scholar
[11] Ding Y, Peng Y, Zhu Y, Fan X, Yang J, Liang B, Zhu X, Wan X, Cheng J 2019 Phys. Rev. Lett. 122 014302
Google Scholar
[12] Kaina N, Lemoult F, Fink M, Lerosey G 2015 Nature 525 77
Google Scholar
[13] Zhu Y F, Zou X Y, Li R Q, Jiang X, Tu J, Liang B, Cheng J C 2015 Sci. Rep. 5 10966
Google Scholar
[14] Jiang X, Li Y, Liang B, Cheng J C, Zhang L 2016 Phys. Rev. Lett. 117 034301
Google Scholar
[15] Fan X D, Zhu Y F, Liang B, Cheng J C, Zhang L 2018 Phys. Rev. Appl. 9 034035
Google Scholar
[16] Yves S, Fleury R, Berthelot T, Fink M, Lemoult F, Lerosey G 2017 Nat. Commun. 8 16023
Google Scholar
[17] Yves S, Fleury R, Lemoult F, Fink M, Lerosey G 2017 New J. Phys. 19 075003
Google Scholar
[18] Chaunsali R, Chen C W, Yang J 2018 Phys. Rev. B 97 054307
Google Scholar
[19] Zhang Z, Cheng Y, Liu X 2018 Sci. Rep. 8 16784
Google Scholar
[20] He X T, Liang E T, Yuan J J, Qiu H Y, Chen X D, Zhao F L, Dong J W 2019 Nat. Commun. 10 872
Google Scholar
[21] Shalaev M I, Walasik W, Tsukernik A, Xu Y, Litchinitser N M 2019 Nat. Nanotechnol. 14 31
Google Scholar
[22] Orazbayev B, Fleury R 2019 Nanophotonics 8 1433
Google Scholar
[23] Plum E, Zhou J, Dong J, Fedotov V A, Koschny T, Soukoulis C M, Zheludev N I 2009 Phys. Rev. B 79 035407
Google Scholar
[24] Zhu R, Liu X N, Hu G K, Sun C T, Huang G L 2014 Nat. Commun. 5 5510
Google Scholar
[25] Goryachev M, Tobar M E 2016 Phys. Rev. Appl. 6 064006
Google Scholar
[26] Orazbayev B, Kaina N, Fleury R 2018 Phys. Rev. Appl. 10 054069
Google Scholar
[27] Geng Z G, Peng Y G, Li P Q, Shen Y X, Zhao D G, Zhu X F 2019 J. Phys.: Condens. Matter 31 245403
Google Scholar
[28] Chen X D, Zhao F L, Chen M, Dong J W 2017 Phys. Rev. B 96 020202
Google Scholar
[29] Geng Z G, Peng Y G, Shen Y X, Zhao D G, Zhu X F 2018 Appl. Phys. Lett. 113 033503
Google Scholar
[30] Chen X D, Shi F L, Liu H, Lu J C, Deng W M, Dai J Y, Cheng Q, Dong J W 2018 Phys. Rev. Appl. 10 044002
Google Scholar
-
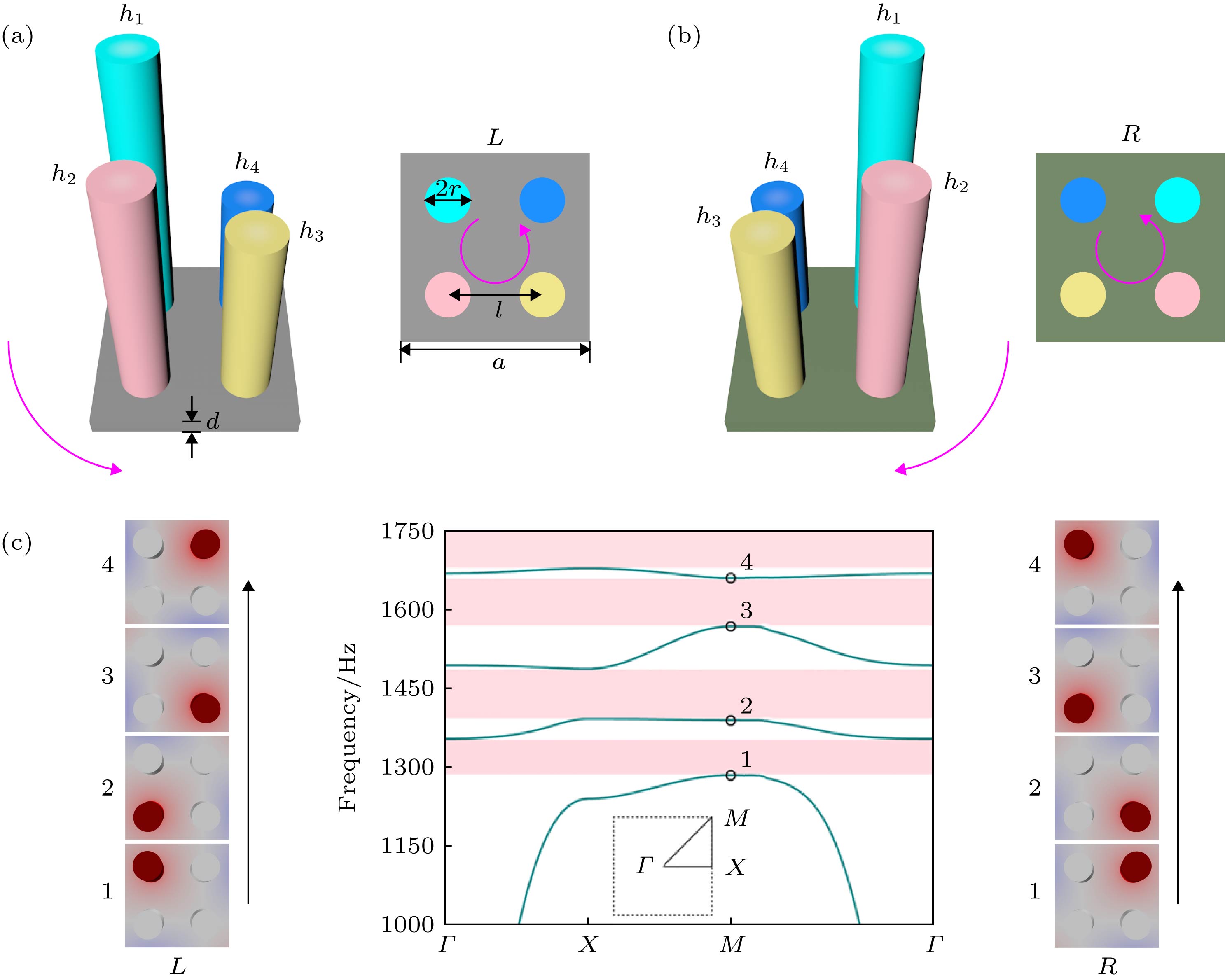
图 1 左手性和右手性原胞示意图 (a)四个共振的空气柱子组成左手性原胞, 其俯视图表现出逆时针旋向; (b)相同空气柱排成的右手性原胞, 其俯视图呈现顺时针旋向; (c)原胞的体能带图有四种声学模式, 左右两侧为不同手性原胞在高对称点的模式分布
Figure 1. Schematics of left- and right-handed unit cells: (a) Left-handed unit cell with four distinct air tubes, the top view of the unit cell shows a counterclockwise variation; (b) right-handed unit cell with the clockwise length variation of air tubes; (c) the bulk band structure with four bulk modes, where the eigenmodes for left-handed and right-handed unit cells are shown at the sides.
图 2 超胞色散和局域界面态 (a)相反手性原胞构成镜像对称界面; (b)带状超胞能带色散, 其中五条蓝色曲线代表界面态, 灰色曲线代表体带, 超胞由六个左手性的原胞和六个右手性的原胞组成, 其单极子模式局域在界面处; (c)单极子界面模式对于位置微扰和高度微扰有较强鲁棒性
Figure 2. Band structures of the interface states: (a) The mirror symmetric interface constructed by oppositely chiral unit cells; (b) the projected band structure of a supercell, the right part shows the supercell slab comprises 6 left-handed and 6 right-handed unit-cells with an even mode (fa1) localized at the interface; (c) the robustness of the even mode against randomly introduced position and height disorders of air tubes.
图 3 亚波长声波鲁棒传输 (a)镜像界面沿着x方向周期排布成直线波导通道; (b)单极子界面模式沿着波导通道局域传输; (c)在引入位置缺陷时, 声波沿直线局域的传输没有明显的背散射; (d)在引入高度微扰时, 声波沿直线局域的传输没有明显的背向散射; (e)几种不同情况下的传输效率曲线, 黑红蓝色曲线表示界面传输透射, 绿色曲线表示统一手性晶体中体态传输透射, 灰色区域表示禁带范围; (f)沿着横向路径的声压幅值分布
Figure 3. Robust transport of sound in subwavelength scale: (a) The schematic of the mirror interface between two oppositely chiral sonic crystals; (b) the propagation of even interface modes along the waveguide channel without defects; (c) the propagation of even interface modes along the waveguide channel with position variations of air tubes; (d) the propagation of even interface modes along the waveguide channel with length variations of air tubes; (e) acoustic transmission for interface states and bulk states, the gray ribbon marks the band gap from 1407 Hz to 1487 Hz; (f) pressure amplitude distributions in the transverse direction.
图 4 声波边缘传输 (a)借助偶极源, 奇模式声压场沿界面局域传输; (b)当软边界条件施加在截断的晶体表面时, 声学奇模式沿着边缘传输, 绿线标记软边界条件; (c)借助单极源, 偶模式声压场沿界面局域传输; (d)当硬边界条件施加在截断的晶体表面时, 声学偶模式沿着边缘传输, 红线标记硬边界条件
Figure 4. The robust edge transport of sound: (a) The interface transport of odd modes along the interface with a dipole source; (b) the edge transport of odd modes along the soft boundary denoted by the green line; (c) the interface transport of even modes along the interface with a monopole source; (b) the edge transport of even modes along the right boundary denoted by the red line.
-
[1] Bernevig B A, Hughes T L, Zhang S C 2006 Science 314 1757
Google Scholar
[2] Hasan M Z, Kane C L 2010 Rev. Mod. Phys. 82 3045
Google Scholar
[3] Lu L, Joannopoulos J D, Soljačić M 2014 Nat. Photonics 8 821
Google Scholar
[4] Wu L H, Hu X 2015 Phys. Rev. Lett. 114 223901
Google Scholar
[5] Fleury R, Khanikaev A B, Alu A 2016 Nat. Commun. 7 11744
Google Scholar
[6] He C, Ni X, Ge H, Sun X C, Chen Y B, Lu M H, Liu X P, Chen Y F 2016 Nat. Phys. 12 1124
Google Scholar
[7] Peng Y G, Qin C Z, Zhao D G, Shen Y X, Xu X Y, Bao M, Jia H, Zhu X F 2016 Nat. Commun. 7 13368
Google Scholar
[8] Lu J, Qiu C, Ye L, Fan X, Ke M, Zhang F, Liu Z 2017 Nat. Phys. 13 369
Google Scholar
[9] Kang Y, Ni X, Cheng X, Khanikaev A B, Genack A Z 2018 Nat. Commun. 9 3029
Google Scholar
[10] Yan M, Lu J, Li F, Deng W, Huang X, Ma J, Liu Z 2018 Nat. Mater. 17 993
Google Scholar
[11] Ding Y, Peng Y, Zhu Y, Fan X, Yang J, Liang B, Zhu X, Wan X, Cheng J 2019 Phys. Rev. Lett. 122 014302
Google Scholar
[12] Kaina N, Lemoult F, Fink M, Lerosey G 2015 Nature 525 77
Google Scholar
[13] Zhu Y F, Zou X Y, Li R Q, Jiang X, Tu J, Liang B, Cheng J C 2015 Sci. Rep. 5 10966
Google Scholar
[14] Jiang X, Li Y, Liang B, Cheng J C, Zhang L 2016 Phys. Rev. Lett. 117 034301
Google Scholar
[15] Fan X D, Zhu Y F, Liang B, Cheng J C, Zhang L 2018 Phys. Rev. Appl. 9 034035
Google Scholar
[16] Yves S, Fleury R, Berthelot T, Fink M, Lemoult F, Lerosey G 2017 Nat. Commun. 8 16023
Google Scholar
[17] Yves S, Fleury R, Lemoult F, Fink M, Lerosey G 2017 New J. Phys. 19 075003
Google Scholar
[18] Chaunsali R, Chen C W, Yang J 2018 Phys. Rev. B 97 054307
Google Scholar
[19] Zhang Z, Cheng Y, Liu X 2018 Sci. Rep. 8 16784
Google Scholar
[20] He X T, Liang E T, Yuan J J, Qiu H Y, Chen X D, Zhao F L, Dong J W 2019 Nat. Commun. 10 872
Google Scholar
[21] Shalaev M I, Walasik W, Tsukernik A, Xu Y, Litchinitser N M 2019 Nat. Nanotechnol. 14 31
Google Scholar
[22] Orazbayev B, Fleury R 2019 Nanophotonics 8 1433
Google Scholar
[23] Plum E, Zhou J, Dong J, Fedotov V A, Koschny T, Soukoulis C M, Zheludev N I 2009 Phys. Rev. B 79 035407
Google Scholar
[24] Zhu R, Liu X N, Hu G K, Sun C T, Huang G L 2014 Nat. Commun. 5 5510
Google Scholar
[25] Goryachev M, Tobar M E 2016 Phys. Rev. Appl. 6 064006
Google Scholar
[26] Orazbayev B, Kaina N, Fleury R 2018 Phys. Rev. Appl. 10 054069
Google Scholar
[27] Geng Z G, Peng Y G, Li P Q, Shen Y X, Zhao D G, Zhu X F 2019 J. Phys.: Condens. Matter 31 245403
Google Scholar
[28] Chen X D, Zhao F L, Chen M, Dong J W 2017 Phys. Rev. B 96 020202
Google Scholar
[29] Geng Z G, Peng Y G, Shen Y X, Zhao D G, Zhu X F 2018 Appl. Phys. Lett. 113 033503
Google Scholar
[30] Chen X D, Shi F L, Liu H, Lu J C, Deng W M, Dai J Y, Cheng Q, Dong J W 2018 Phys. Rev. Appl. 10 044002
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 14230
- PDF Downloads: 513
- Cited By: 0
