-
Single-pixel imaging is a computational imaging scheme that offers novel solutions for multi-spectral imaging, feature-based imaging, polarimetric imaging, three-dimensional imaging, holographic imaging, and optical encryption. The single-pixel imaging scheme can be used for imaging in wave band such as infrared and micro wave imaging, or will be useful in the case where the array detector technique is difficult to meet the requirement such as the sensitivity or the volume. The main limitation for its application comes from a trade-off between spatial resolution and acquisition time, in other words, from relatively high measurement and reconstruction time. Although compressive sensing technique can be used to improve the acquisition time by reducing the number of samplings, the computational time to reconstruct an image is not fast enough to satisfy the real-time video. In this paper, we propose to reduce the required signal acquisition time by using a novel sampling scheme based on optimized ordering of the Hadamard basis, and improve the image reconstruction efficiency by using fast Walsh-Hadamard transform. In our method, the Hadamard basis is rearranged in the ascendant order of the values of its " sparsity” coefficients which are obtained through " Daubechies wavelets 1 (Haar wavelets)”, " Daubechies wavelets 2” wavelet transform and discrete cosine transform, and then compute each total sum of the transformed coefficients’ absolute value, respectively. The measurement order of the Hadamard basis is then rearranged directly according to Walsh order and random permutation order. The peak signal-to-noise ratio (PSNR) and structural similarity index (SSIM) of the retrieved images are computed and compared to test all the five reordering schemes above both in our numerical simulation and outdoor experiments. We find that the reordering method based on Haar wavelet transform is the best PSNR and SSIM and it can reconstruct image under a sampling ratio of 25% which corresponds to the recovering time in which 300 frame per second @64 × 64 pixels single-pixel imaging can be achieved. The optimized measurement order of Hadamard basis greatly simplifies post processing, resulting in significantly faster image reconstruction, which steps further toward high frame rate single-pixel imaging’s applications. Moreover, we propose a novel method to optimize measurement basis in single-pixel imaging, which may be useful in other basis optimizing, such as optimized random speckles, etc.
-
Keywords:
- single-pixel imaging /
- Walsh-Hadamard transform /
- wavelet transform
[1] Candès E J, Romberg J, Tao T 2006 IEEE Trans. Inform. Theory 52 489
Google Scholar
[2] Romberg J 2008 IEEE Signal Proc. Mag. 25 14
Google Scholar
[3] Duarte M, Davenport M, Takhar D, Laska J, Sun T, Kelly K, Baraniuk R 2008 IEEE Signal Proc. Mag. 25 83
Google Scholar
[4] Gong W L, Han S S 2009 arXiv: 0911.4750
[5] Czajkowski K M, Pastuszczak A, Kotyński R 2017 arXiv: 1709.07739v2
[6] Olivas S J, Rachlin Y, Gu L, Gardiner B, Dawson R, Laine J P, Ford J E 2013 Appl. Opt. 52 4515
Google Scholar
[7] 李明飞, 莫小范, 张安宁 2016 导航与控制 5 1
Google Scholar
Li M F, Mo X F, Zhang A N 2016 Navigtion and Control 5 1
Google Scholar
[8] 李明飞, 莫小范, 赵连洁, 霍娟, 杨然, 李凯, 张安宁 2015 65 064201
Google Scholar
Li M F, Mo X F, Zhao L J, Huo J, Yang R, Li K, Zhang A N 2015 Acta Phys. Sin. 65 064201
Google Scholar
[9] Zhang Z, Wang X, Zheng G, Zhong J 2017 Sci. Rep. 7 12029
Google Scholar
[10] Chen M L., Li E R, Han S S 2014 Appl. Opt. 53 2924
Google Scholar
[11] Sun S, Liu W T, Lin H Z, Zhang E F, Liu J Y, Li Q, Chen P X, 2016 Sci. Rep. 6 37013
Google Scholar
[12] Sun M J, Meng L T, Edgar M P, Padgett M J, Radwell N 2017 Sci. Rep. 7 3464
Google Scholar
[13] Li M F, Zhang Y R, Liu X F, Yao X R, Luo K H, Fan H, Wu L A 2013 Appl. Phys. Lett. 103 211119
Google Scholar
[14] Li M F, Zhang Y R, Luo K H, Wu L A, Fan H 2013 Phys. Rev. A 87 033813
Google Scholar
[15] Liu X L, Shi J H, Wu X Y, Zeng G H 2018 Sci. Rep. 8 5012
Google Scholar
[16] Beer T 1981 Am. J. Phys. 49 466
Google Scholar
[17] Li Q, Zhou M L, Shi B C, Wang N C 1998 Chinese Science Bulletin 43 627
Google Scholar
[18] Ferri F, Magatti D, Lugiato L A, Gatti A 2010 Phys. Rev. Lett. 104 253603
Google Scholar
-
图 1 单像素成像原理示意图, 其中待成像物体经主透镜成像到DMD上, 经编码矩阵调制的光束被反射, 反射光由中继透镜汇聚到光电探测器, 探测器将光信号转为电信号, 电信号经过A/D转换由模拟信号转为数字信号, 重复刷新DMD调制矩阵将得到一系列数字信号, 将数字信号采集到计算设备即可利用算法重构图像
Figure 1. Schematic diagram of the experimental setup. Lena is the object to be recovered; the main lens imaging Lena onto the DMD, which is modulated by matrices, then the light is reflected and collected by the relay lens into the photo-detector. As DMD modulation matrices refreshing continuously, the analog-to-digital converter (A/D) connected with the photo-detector receives a series of digital signals, which are finally sent to recover the image by the computer.
图 2 不同排序方法下图像SNR与测量次数m的关系, 其中排序方法包括Haar小波变换序、Db2小波变换序、Dct序、Walsh排序和随机排序
Figure 2. Image SNR versus mth measurements under different ordering methods: Haar wavelet order, Db2 wavelet order and Dct order, Walsh order and random order.
图 3 测试图像及不同排序方法在25%采样下的图像重建结果 (a) 原始测试图像64 × 64像素; (b)—(f) 排序方法为Db2小波序、Dct序、Walsh序、随机排序和Haar小波排序下重图像重建结果
Figure 3. Original and recovered images under 25% full sampling: (a) The Lena, with 64 × 64 pixels; (b)−(f) images recovered corresponding to the ordering method of Db2 wavelet order, Dct order, Walsh order, random permutation order and Haar wavelet order, respectively.
图 5 不同排序方法25%采样下的实验数据, 即Haar小波变换、Db2小波变换、Dct、Walsh排序和随机随机排序对应的实验数据
Figure 5. Experimental data of different ordering methods: Haar wavelet, Db2 wavelet and Dct, Walsh order and random order.
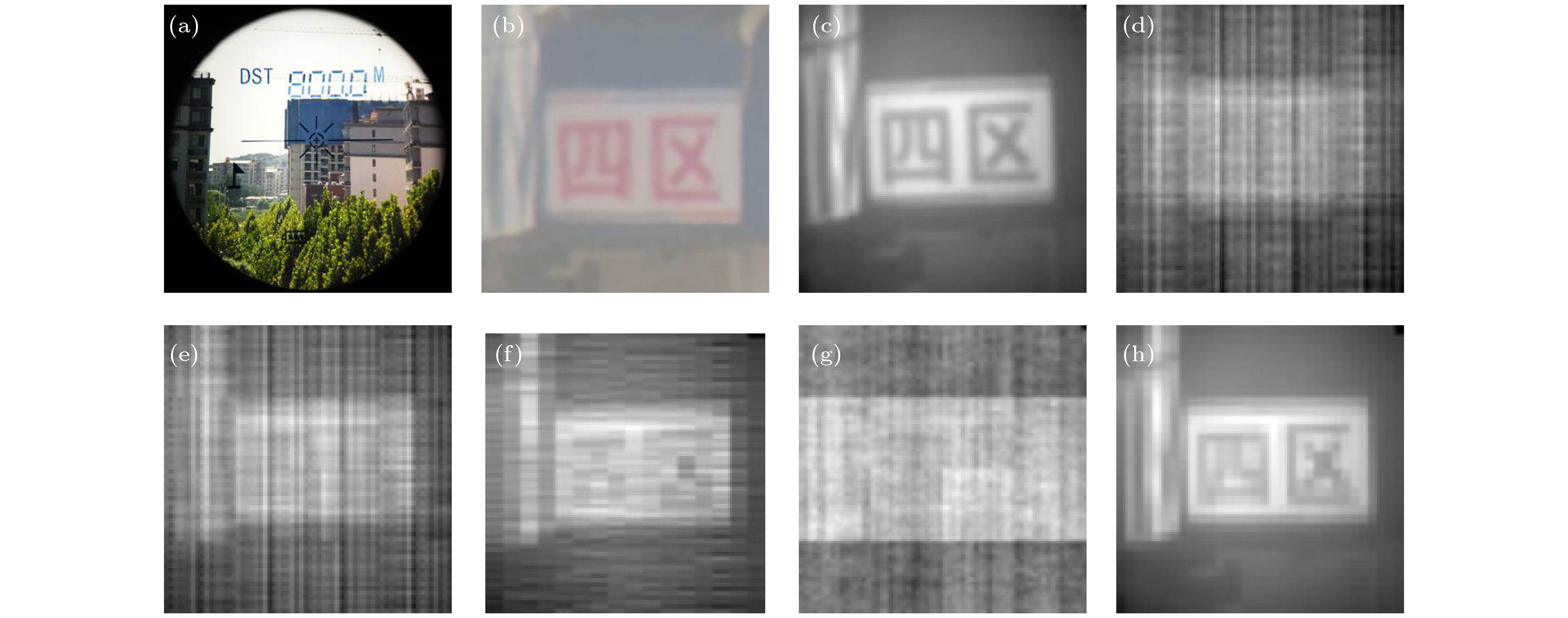
图 6 室外实验结果和不同排序方法在25%采样下的重建图像 (a)目标区域, 对应距离800 m; (b)相机拍摄的目标图像; (c) 无压缩单像素成像, 64 × 64像素, 100幅图像累加结果; (d)—(h) 分别对应排序方法为Db2小波序、Dct序、Walsh序、随机排序和Haar小波排序下重图像重建结果
Figure 6. Outdoor experiment and recovered images under 25% full sampling with different ordering methods: (a) The target region, with the distance 800 meters; (b) target image captured by camera; (c) image recovered by single-pixel camera, with 64 × 64 pixels, with 100 recovered images averaged; (d)−(h) images recovered corresponding to the ordering method of Db2 wavelet order, Dct order, Walsh order, random permutation order and Haar wavelet order, respectively.
-
[1] Candès E J, Romberg J, Tao T 2006 IEEE Trans. Inform. Theory 52 489
Google Scholar
[2] Romberg J 2008 IEEE Signal Proc. Mag. 25 14
Google Scholar
[3] Duarte M, Davenport M, Takhar D, Laska J, Sun T, Kelly K, Baraniuk R 2008 IEEE Signal Proc. Mag. 25 83
Google Scholar
[4] Gong W L, Han S S 2009 arXiv: 0911.4750
[5] Czajkowski K M, Pastuszczak A, Kotyński R 2017 arXiv: 1709.07739v2
[6] Olivas S J, Rachlin Y, Gu L, Gardiner B, Dawson R, Laine J P, Ford J E 2013 Appl. Opt. 52 4515
Google Scholar
[7] 李明飞, 莫小范, 张安宁 2016 导航与控制 5 1
Google Scholar
Li M F, Mo X F, Zhang A N 2016 Navigtion and Control 5 1
Google Scholar
[8] 李明飞, 莫小范, 赵连洁, 霍娟, 杨然, 李凯, 张安宁 2015 65 064201
Google Scholar
Li M F, Mo X F, Zhao L J, Huo J, Yang R, Li K, Zhang A N 2015 Acta Phys. Sin. 65 064201
Google Scholar
[9] Zhang Z, Wang X, Zheng G, Zhong J 2017 Sci. Rep. 7 12029
Google Scholar
[10] Chen M L., Li E R, Han S S 2014 Appl. Opt. 53 2924
Google Scholar
[11] Sun S, Liu W T, Lin H Z, Zhang E F, Liu J Y, Li Q, Chen P X, 2016 Sci. Rep. 6 37013
Google Scholar
[12] Sun M J, Meng L T, Edgar M P, Padgett M J, Radwell N 2017 Sci. Rep. 7 3464
Google Scholar
[13] Li M F, Zhang Y R, Liu X F, Yao X R, Luo K H, Fan H, Wu L A 2013 Appl. Phys. Lett. 103 211119
Google Scholar
[14] Li M F, Zhang Y R, Luo K H, Wu L A, Fan H 2013 Phys. Rev. A 87 033813
Google Scholar
[15] Liu X L, Shi J H, Wu X Y, Zeng G H 2018 Sci. Rep. 8 5012
Google Scholar
[16] Beer T 1981 Am. J. Phys. 49 466
Google Scholar
[17] Li Q, Zhou M L, Shi B C, Wang N C 1998 Chinese Science Bulletin 43 627
Google Scholar
[18] Ferri F, Magatti D, Lugiato L A, Gatti A 2010 Phys. Rev. Lett. 104 253603
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 13182
- PDF Downloads: 466
- Cited By: 0
