-
单羟基醇的主介电弛豫过程通常表现出典型的Debye特征, 近年来影响其速率的因素成为研究热点. 一般认为, 醇分子的亲水端(即羟基)在主介电弛豫过程中通过氢键网络发挥主要作用, 而疏水端则主要通过“稀释”体系中的羟基浓度, 间接影响该过程. 本研究通过经典分子动力学模拟系统地探讨了影响甲醇主介电弛豫速率的因素. 结果表明, 甲醇的疏水端不仅在稳固体系氢键网络方面对主介电弛豫过程产生间接影响, 甚至还能直接作用于弛豫过程. 甲醇的主介电弛豫过程是其亲水端和疏水端协同作用的结果. 此外还发现, 甲醇的主介电弛豫速率可能并不像著名的“等待-切换”模型所描述的那样, 主要受“氢键伙伴”浓度的影响, 而是多种因素共同作用的结果. 在某些情况下, “氢键伙伴”浓度的影响甚至被其他因素产生的影响所掩盖, 成为次要因素. 本研究有助于加深对醇类分子弛豫过程及其物理起源的理解.The primary dielectric relaxation process of monoalcohols typically exhibits characteristic Debye behavior, and the factors influencing its rate have become a research focus in recent years. It is generally believed that the hydrophilic end (i.e. the hydroxyl group) of alcohol molecule plays a major role in the primary dielectric relaxation process through a hydrogen bonding network, while the hydrophobic end mainly exerts an indirect effect by influencing the formation of intermolecular hydrogen bonds. In this work, the factors influencing the primary dielectric relaxation process of methanol are systematically investigated by using molecular dynamics simulations. Studying methanol, a simplest alcohol molecule, can provide insights into the common characteristics of monohydroxy alcohols and even alcohols in general. The well-known “wait-and-switch” model currently emphasizes the influence of hydrogen bond partner concentration on the primary dielectric relaxation rate of the system. In this work, we systematically investigate the factors influencing the primary dielectric relaxation rate of methanol by adjusting the O—H bond length (dOH), the C—O bond length (dCO), and the methyl diameter (σmethyl) of methanol molecules, respectively, and significantly extend the “wait-and-switch” model. 1) By adjusting dOH, we find that stronger total hydrogen bond energy (UHB) in the system can enhance the correlation of molecular motion, slow down the reorientation rate of molecules and, consequently, the primary dielectric relaxation process of the system. 2) By adjusting dCO, we discover that a longer hydrophobic end not only slows down the primary dielectric relaxation process by stabilizing the intermolecular hydrogen bond network but also directly reduces the rate of this process. 3) By adjusting σmethyl, we find that an excessively small σmethyl is detrimental to the stability of the hydrogen bond network, while an excessively large σmethyl hinders thehydrogen bonds from forming. Both of these situations will have a negative influence on the correlation of molecular motion. When σmethyl is at a moderate level, the main dielectric relaxation process of the system is the slowest. Ultimately, it is found that factors such as UHB and related motion volume (VCM), as well as the concentration of hydrogen bond partners in the system, collectively constitute the key factors affecting the primary dielectric relaxation rate of the system. Our results can reasonably explain experimental phenomena that the original “wait-and-switch” model cannot explain. This study contributes to a more in-depth understanding of the relaxation processes of alcohol molecules and their physical origins.
-
Keywords:
- methanol /
- dielectric relaxation /
- molecular dynamics simulations
[1] Rafikul Islam M, Rehan Alam M, Rayhan U, Khan F, Aldossari S A, Mohammad Wabaidur S, Rana S, Anamul Hoque M 2024 J. Mol. Liq. 393 123621
Google Scholar
[2] Chen B L, Liu K, Wang L, Zhang X X, Qu K G, Kong Y X, Liu M 2022 J. Chem. Eng. Data 67 3414
Google Scholar
[3] Jia W, Sutanto I R, Ndiaye M, Keppler J K, van der Goot A J 2022 Food Struct. 33 100274
Google Scholar
[4] Moraveji M K, Sajjadi B, Davarnejad R 2011 Chem. Eng. Technol. 34 465
Google Scholar
[5] 杨静, 韩晓静, 刘冬雪, 石标, 王鹏阳, 许盛之, 赵颖, 张晓丹 2024 73 158401
Google Scholar
Yang J, Han X J, Liu D X, Shi B, Wang P Y, Xu S Z, Zhao Y, Zhang X D 2024 Acta Phys. Sin. 73 158401
Google Scholar
[6] 赵振越, 张晓丹, 王奉友, 姜元建, 杜建, 高海波, 赵颖, 刘彩池 2014 63 136802
Google Scholar
Zhao Z Y, Zhang X D, Wang F Y, Jiang Y J, Du J, Gao H B, Zhao Y, Liu C C 2014 Acta Phys. Sin. 63 136802
Google Scholar
[7] 赵兴宇, 王丽娜, 韩宏博, 尚洁莹 2024 73 147701
Google Scholar
Zhao X Y, Wang L N, Han H B, Shang J Y 2024 Acta Phys. Sin. 73 147701
Google Scholar
[8] 王丽娜, 赵兴宇, 尚洁莹, 周恒为 2023 72 037701
Google Scholar
Wang L N, Zhao X Y, Shang J Y, Zhou H W 2023 Acta Phys. Sin. 72 037701
Google Scholar
[9] Sato T, Buchner R 2003 J. Chem. Phys. 118 4606
Google Scholar
[10] Sato T, Buchner R 2004 J. Phys. Chem. A 108 5007
Google Scholar
[11] Sato T, Buchner R 2005 J. Mol. Liq. 117 23
Google Scholar
[12] Kaatze U, Behrends R, Pottel R 2002 J. Non-Cryst. Solids. 305 19
Google Scholar
[13] Petong P, Pottel R, Kaatze U 1999 J. Phys. Chem. A 103 6114
Google Scholar
[14] Cardona J, Sweatman M B, Lue L 2018 J. Phys. Chem. B 122 1505
Google Scholar
[15] Li X, Chen Z M, Gao Y Q, Tu W K, Wang L M 2015 Front. Mater. 2 41
Google Scholar
[16] Xu D, Feng S, Wang J Q, Wang L M, Richert R 2020 J. Phys. Chem. Lett. 11 5792
Google Scholar
[17] Patil S, Sun R, Cheng S, Cheng S 2023 Phys. Rev. Lett. 130 098201
Google Scholar
[18] Pabst F, Helbling A, Gabriel J, Weigl P, Blochowicz T 2020 Phys. Rev. E 102 010606(R
Google Scholar
[19] Gainaru C, Meier R, Schildmann S, Lederle C, Hiller W, Rössler E A, Böhmer R 2010 Phys. Rev. Lett. 105 258303
Google Scholar
[20] Gabriel J, Pabst F, Helbling A, Böhmer T, Blochowicz T 2018 Phys. Rev. Lett. 121 035501
Google Scholar
[21] Koperwas K, Paluch M 2022 Phys. Rev. Lett. 129 025501
Google Scholar
[22] Fragiadakis D, Roland C M, Casalini R 2010 J. Chem. Phys. 132 144505
Google Scholar
[23] Soszka N, Hachuła B, Tarnacka M, Kaminska E, Pawlus S, Kaminski K, Paluch M 2021 J. Phys. Chem. B 125 2960
Google Scholar
[24] Hecksher T 2016 J. Chem. Phys. 144 161103
Google Scholar
[25] Jurkiewicz K, Kołodziej S, Hachuła B, Grzybowska K, Musiał M, Grelska J, Bielas R, Talik A, Pawlus S, Kamiński K, Paluch M 2020 J. Mol. Liq. 319 114084
Google Scholar
[26] Kalinovskaya O E, Vij J K, Johari G P 2001 J. Phys. Chem. A 105 5061
Google Scholar
[27] Zhang N, Li W Z, Chen C, Zuo J G, Weng L D 2013 Mol. Phys. 111 939
Google Scholar
[28] Zhang N, Shen Z L, Chen C, He G H, Hao C 2015 J. Mol. Liq. 203 90
Google Scholar
[29] Hiejima Y, Kajihara Y, Kohno H, Yao M 2001 J. Phys. : Condens. Matter 13 10307
Google Scholar
[30] Stubbs J M, Potoff J J, Siepmann J I 2004 J. Phys. Chem. B 108 17596
Google Scholar
[31] Chen B, Potoff J J, Siepmann J I 2001 J. Phys. Chem. B 105 3093
Google Scholar
[32] Wu Y, Tepper H L, Voth G A 2006 J. Chem. Phys. 124 024503
Google Scholar
[33] Abraham M J, Murtola T, Schulz R, Páll S, Smith J C, Hess B, Lindahl E 2015 SoftwareX 1-2 19
Google Scholar
[34] Hoover W G 1985 Phys. Rev. A 31 1695
Google Scholar
[35] Nosé S, Klein M L 1983 Mol. Phys. 50 1055
Google Scholar
[36] Darden T, York D, Pedersen L 1993 J. Chem. Phys. 98 10089
Google Scholar
[37] Carlson S, Brünig F N, Loche P, Bonthuis D J, Netz R R 2020 J. Phys. Chem. A 124 5599
Google Scholar
[38] Ono T, Horikawa K, Maeda Y, Ota M, Sato Y, Inomata H 2016 Fluid Phase Equilibr. 420 30
Google Scholar
[39] Kalinovskaya O E, Vij J K 2000 J. Chem. Phys. 112 3262
Google Scholar
-
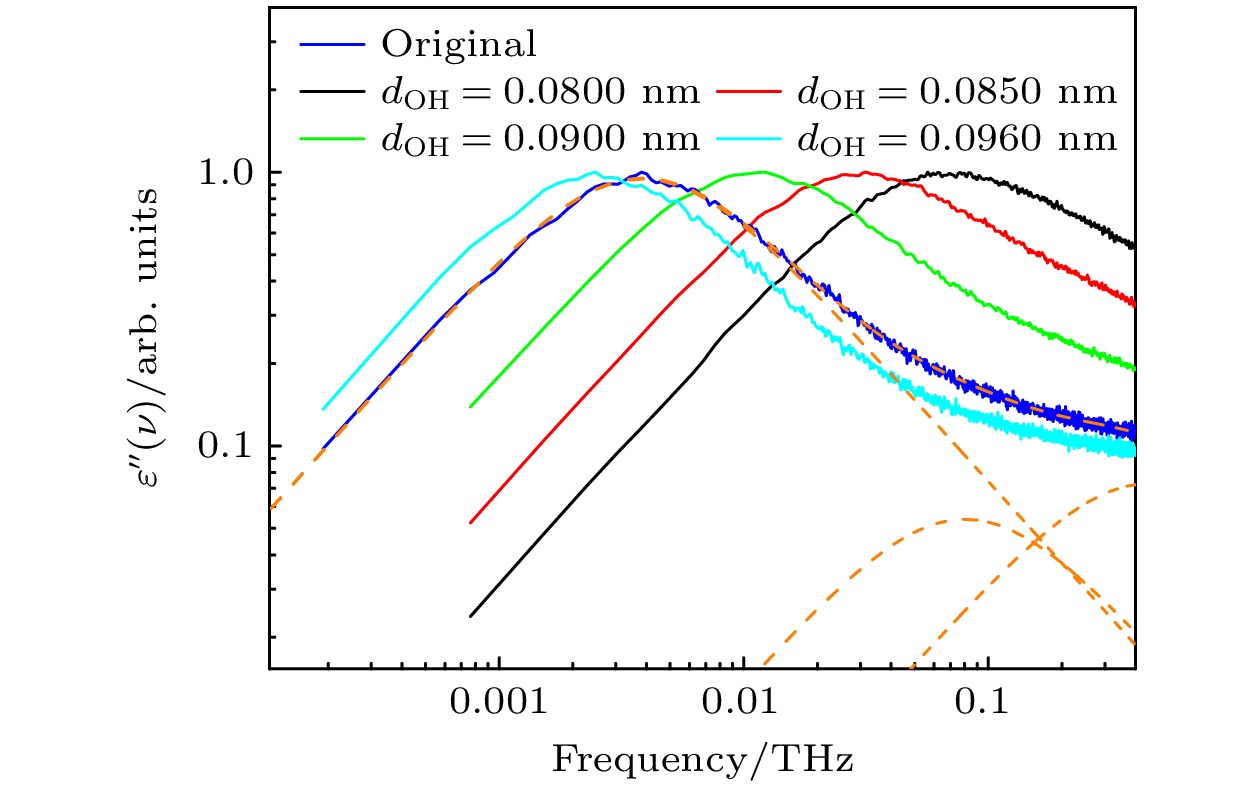
图 1 不同dOH下体系的介电损耗谱, 蓝色曲线为采用原始TraPPE-UA力场仿真得到的介电损耗谱, 橙色虚线为使用(1)式对其进行拟合的结果, 其中$ {\tau _1} $= 41.5 ps, $ \alpha $= 0.9946, $ {\tau _{2}} $= 1.98 ps, $ {\tau _{3}} $= 0.352 ps
Fig. 1. Dielectric loss spectra of the system with different dOH, the blue curve represents the dielectric loss spectrum obtained from simulations using the original TRAPPE-UA force field, while the orange dashed line shows the fitting results using Eq. (1), where $ {\tau _1} $= 41.5 ps, $ \alpha $= 0.9946, $ {\tau _{2}} $= 1.98 ps, and $ {\tau _{3}} $= 0.352 ps.
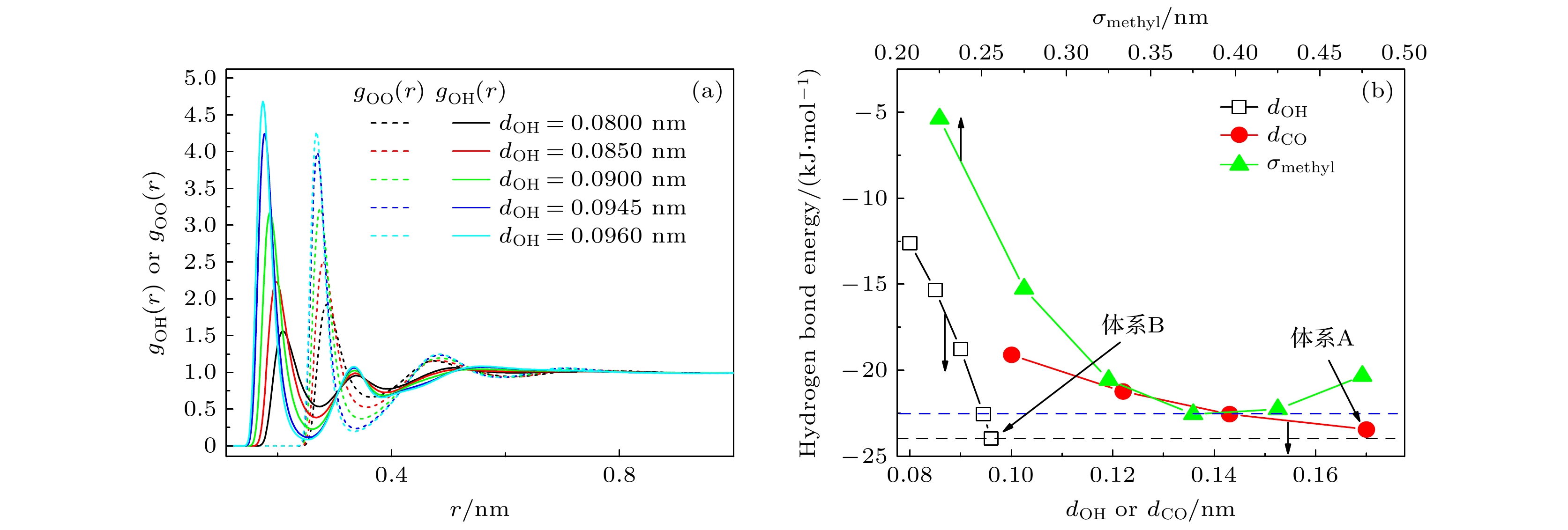
图 2 (a) 不同dOH系统中, 甲醇分子中氧原子和羟基氢原子围绕甲醇氧原子的径向分布函数; (b) 不同dOH, dCO和σmethyl系统中的平均氢键能 (蓝色和黑色虚线分别表示采用原始TraPPE-UA力场参数得到的平均氢键能和体系B的平均氢键能)
Fig. 2. (a) Radial distribution functions of oxygen and hydroxy hydrogen atoms of methanol around the methanol oxygen atoms in systems with different dOH; (b) the average hydrogen bond energy for systems with varying dOH, dCO, and σmethyl values (the blue and black dashed lines representing the average hydrogen bond energy derived from the original TraPPE-UA force field parameters and the average hydrogen bond energy of system B, respectively).
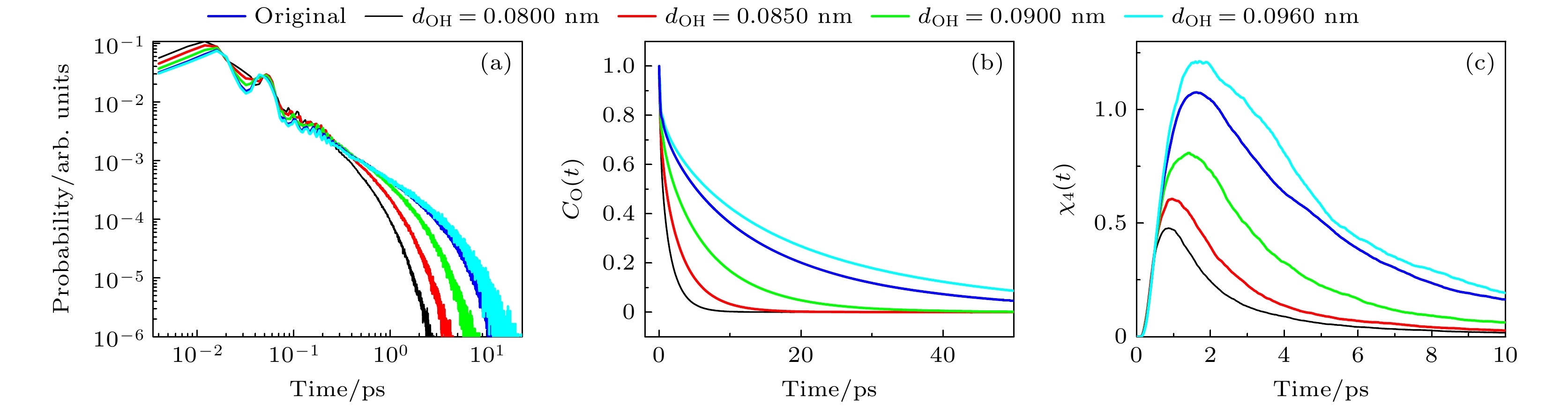
图 3 (a)不同dOH体系中, 氢键寿命的分布; (b) 不同dOH体系中, 甲醇分子的偶极取向自相关函数; (c) 不同dOH体系中, 四点关联函数
Fig. 3. (a) Distribution of hydrogen bond lifetimes of the systems with different dOH; (b) dipole orientation autocorrelation function of methanol molecules of the systems with different dOH; (c) time-dependent four-point susceptibilities of the systems with different dOH
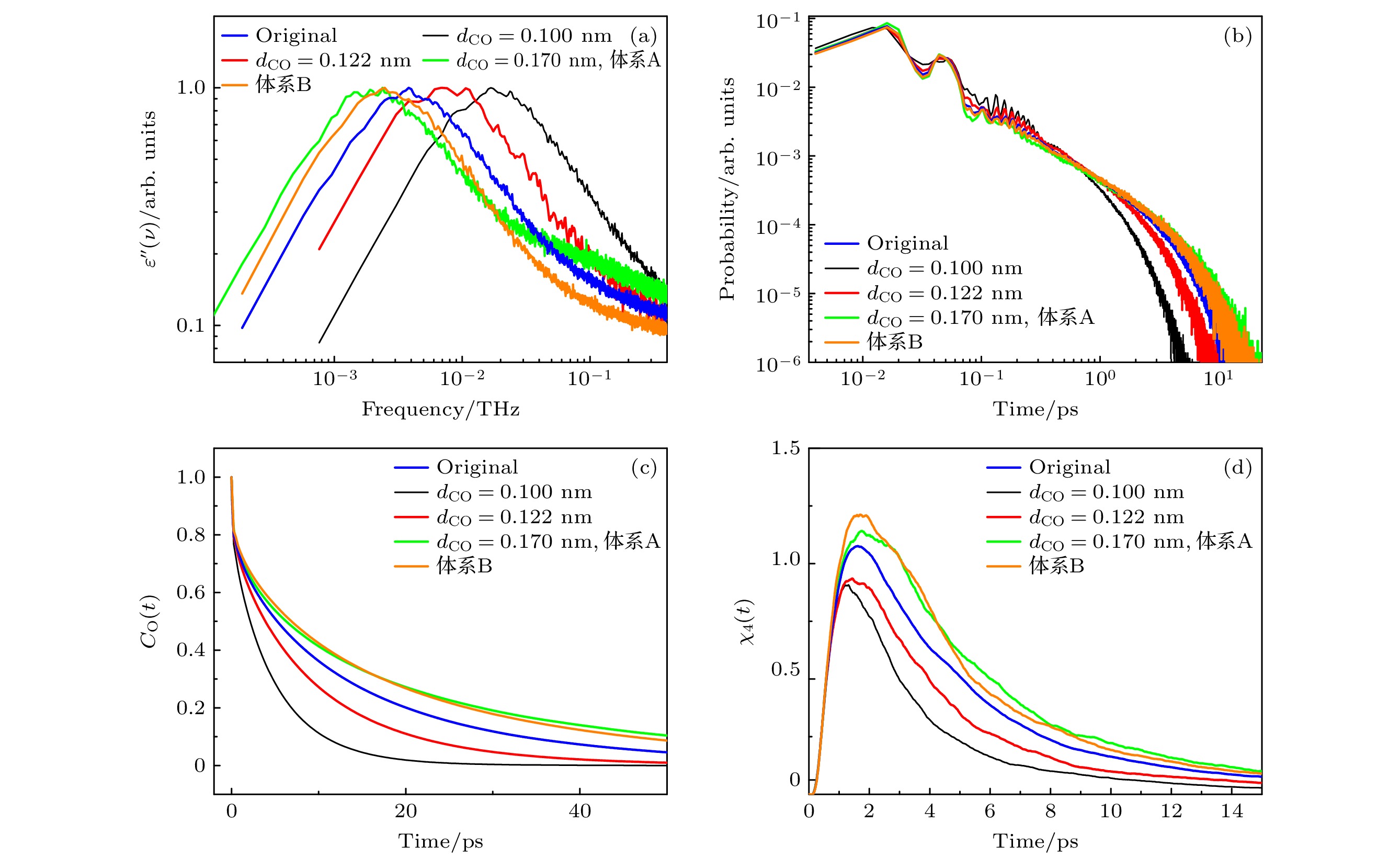
图 4 (a)不同dCO体系中, 介电损耗谱; (b)不同dCO体系中, 氢键寿命的分布; (c)不同dCO体系中, 甲醇分子的偶极取向自相关函数; (d)不同dCO体系中, 四点关联函数
Fig. 4. (a) Dielectric loss spectra of the system at different dCO values; (b) distribution of hydrogen bond lifetimes of the system at different dCO values; (c) dipole orientation self-correlation function of methanol molecules of the system at different dCO values; (d) time-dependent four-point susceptibilities of the system at different dCO values.
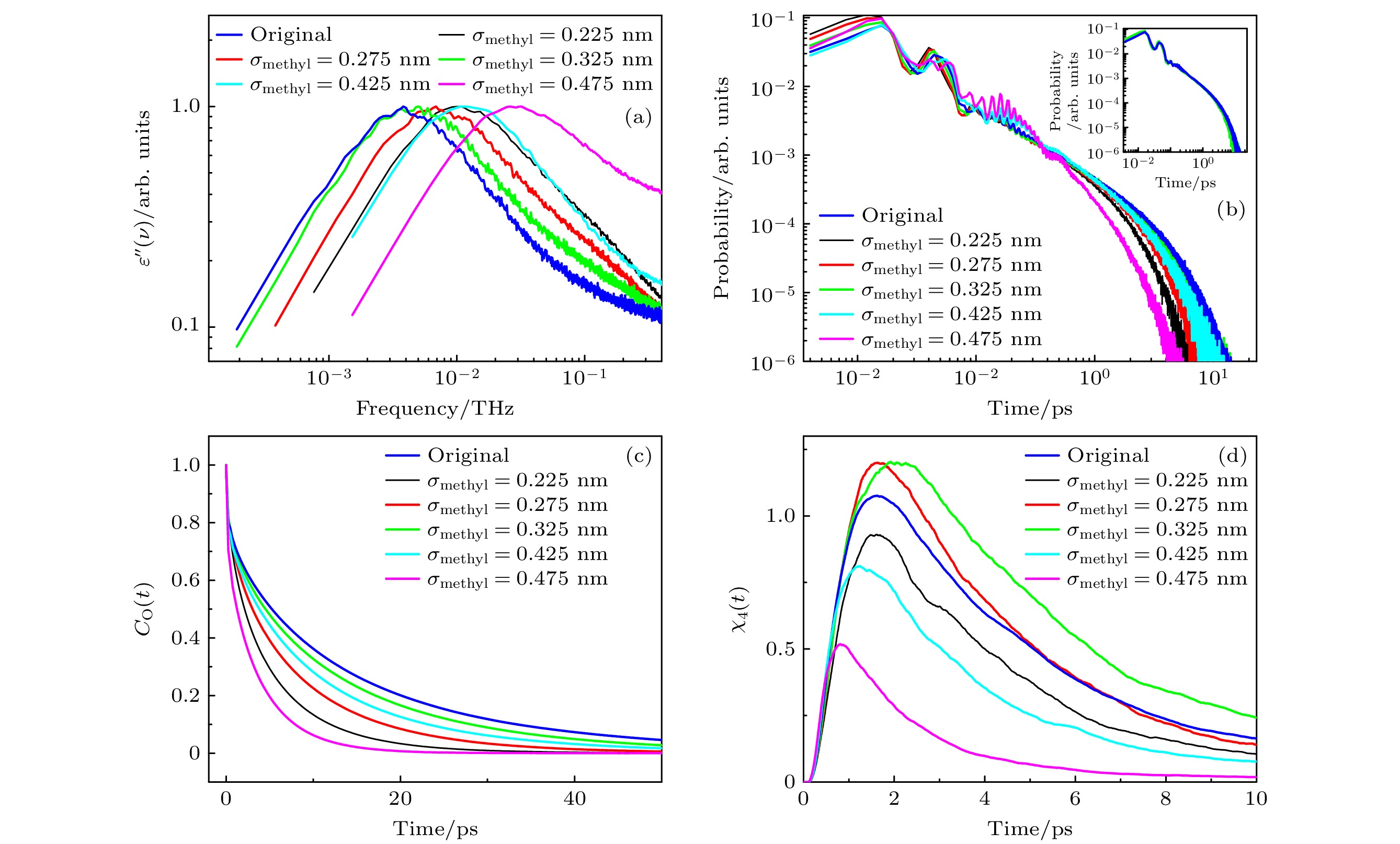
图 5 (a)不同σmethyl体系的介电损耗谱; (b)不同σmethyl体系氢键寿命的分布, 插图为σmethyl为0.325 nm和0.375 nm时氢键寿命的分布; (c)不同σmethyl体系, 甲醇分子的偶极取向自相关函数; (d)不同σmethyl体系, 四点关联函数
Fig. 5. (a) Dielectric loss spectra of the system at different σmethyl values; (b) distribution of hydrogen bond lifetimes of the system at different σmethyl values, the inset shows the distribution of hydrogen bond lifetimes at σmethyl values of 0.325 nm and 0.375 nm; (c) dipole orientation self-correlation function of methanol molecules of the system at different σmethyl values; (d) time-dependent four-point susceptibilities of the system at different σmethyl values.
表 1 不同dOH值体系中NHB, Npair和Nchain的值
Table 1. Values of NHB, Npair, and Nchain for the systems with varying dOH values.
dOH/nm NHB Npair Nchain 0.0800 1.31 1.76 6.13 0.0850 1.58 1.94 11.96 0.0900 1.80 2.05 28.21 0.0945 1.91 2.08 70.91 0.0960 1.94 2.08 98.67 
下载: 导出CSV
表 2 不同dCO体系中NHB, Npair和Nchain的值
Table 2. Values of NHB, Npair, and Nchain for the systems with varying dCO values.
dCO/nm NHB Npair Nchain 0.100 1.74 2.12 16.67 0.122 1.86 2.10 37.28 0.143 1.91 2.08 70.91 0.170 1.94 2.06 128.64
下载: 导出CSV
表 3 不同σmethyl体系中NHB, Npair和Nchain的值
Table 3. Values of NHB, Npair, and Nchain for the systems with varying σmethyl values.
σmethyl/nm NHB Npair Nchain 0.225 1.87 3.81 63.34 0.275 1.90 2.82 81.65 0.325 1.92 2.29 96.77 0.375 1.91 2.08 70.91 0.425 1.82 1.95 23.69 0.475 1.41 1.62 4.94
下载: 导出CSV
-
[1] Rafikul Islam M, Rehan Alam M, Rayhan U, Khan F, Aldossari S A, Mohammad Wabaidur S, Rana S, Anamul Hoque M 2024 J. Mol. Liq. 393 123621
Google Scholar
[2] Chen B L, Liu K, Wang L, Zhang X X, Qu K G, Kong Y X, Liu M 2022 J. Chem. Eng. Data 67 3414
Google Scholar
[3] Jia W, Sutanto I R, Ndiaye M, Keppler J K, van der Goot A J 2022 Food Struct. 33 100274
Google Scholar
[4] Moraveji M K, Sajjadi B, Davarnejad R 2011 Chem. Eng. Technol. 34 465
Google Scholar
[5] 杨静, 韩晓静, 刘冬雪, 石标, 王鹏阳, 许盛之, 赵颖, 张晓丹 2024 73 158401
Google Scholar
Yang J, Han X J, Liu D X, Shi B, Wang P Y, Xu S Z, Zhao Y, Zhang X D 2024 Acta Phys. Sin. 73 158401
Google Scholar
[6] 赵振越, 张晓丹, 王奉友, 姜元建, 杜建, 高海波, 赵颖, 刘彩池 2014 63 136802
Google Scholar
Zhao Z Y, Zhang X D, Wang F Y, Jiang Y J, Du J, Gao H B, Zhao Y, Liu C C 2014 Acta Phys. Sin. 63 136802
Google Scholar
[7] 赵兴宇, 王丽娜, 韩宏博, 尚洁莹 2024 73 147701
Google Scholar
Zhao X Y, Wang L N, Han H B, Shang J Y 2024 Acta Phys. Sin. 73 147701
Google Scholar
[8] 王丽娜, 赵兴宇, 尚洁莹, 周恒为 2023 72 037701
Google Scholar
Wang L N, Zhao X Y, Shang J Y, Zhou H W 2023 Acta Phys. Sin. 72 037701
Google Scholar
[9] Sato T, Buchner R 2003 J. Chem. Phys. 118 4606
Google Scholar
[10] Sato T, Buchner R 2004 J. Phys. Chem. A 108 5007
Google Scholar
[11] Sato T, Buchner R 2005 J. Mol. Liq. 117 23
Google Scholar
[12] Kaatze U, Behrends R, Pottel R 2002 J. Non-Cryst. Solids. 305 19
Google Scholar
[13] Petong P, Pottel R, Kaatze U 1999 J. Phys. Chem. A 103 6114
Google Scholar
[14] Cardona J, Sweatman M B, Lue L 2018 J. Phys. Chem. B 122 1505
Google Scholar
[15] Li X, Chen Z M, Gao Y Q, Tu W K, Wang L M 2015 Front. Mater. 2 41
Google Scholar
[16] Xu D, Feng S, Wang J Q, Wang L M, Richert R 2020 J. Phys. Chem. Lett. 11 5792
Google Scholar
[17] Patil S, Sun R, Cheng S, Cheng S 2023 Phys. Rev. Lett. 130 098201
Google Scholar
[18] Pabst F, Helbling A, Gabriel J, Weigl P, Blochowicz T 2020 Phys. Rev. E 102 010606(R
Google Scholar
[19] Gainaru C, Meier R, Schildmann S, Lederle C, Hiller W, Rössler E A, Böhmer R 2010 Phys. Rev. Lett. 105 258303
Google Scholar
[20] Gabriel J, Pabst F, Helbling A, Böhmer T, Blochowicz T 2018 Phys. Rev. Lett. 121 035501
Google Scholar
[21] Koperwas K, Paluch M 2022 Phys. Rev. Lett. 129 025501
Google Scholar
[22] Fragiadakis D, Roland C M, Casalini R 2010 J. Chem. Phys. 132 144505
Google Scholar
[23] Soszka N, Hachuła B, Tarnacka M, Kaminska E, Pawlus S, Kaminski K, Paluch M 2021 J. Phys. Chem. B 125 2960
Google Scholar
[24] Hecksher T 2016 J. Chem. Phys. 144 161103
Google Scholar
[25] Jurkiewicz K, Kołodziej S, Hachuła B, Grzybowska K, Musiał M, Grelska J, Bielas R, Talik A, Pawlus S, Kamiński K, Paluch M 2020 J. Mol. Liq. 319 114084
Google Scholar
[26] Kalinovskaya O E, Vij J K, Johari G P 2001 J. Phys. Chem. A 105 5061
Google Scholar
[27] Zhang N, Li W Z, Chen C, Zuo J G, Weng L D 2013 Mol. Phys. 111 939
Google Scholar
[28] Zhang N, Shen Z L, Chen C, He G H, Hao C 2015 J. Mol. Liq. 203 90
Google Scholar
[29] Hiejima Y, Kajihara Y, Kohno H, Yao M 2001 J. Phys. : Condens. Matter 13 10307
Google Scholar
[30] Stubbs J M, Potoff J J, Siepmann J I 2004 J. Phys. Chem. B 108 17596
Google Scholar
[31] Chen B, Potoff J J, Siepmann J I 2001 J. Phys. Chem. B 105 3093
Google Scholar
[32] Wu Y, Tepper H L, Voth G A 2006 J. Chem. Phys. 124 024503
Google Scholar
[33] Abraham M J, Murtola T, Schulz R, Páll S, Smith J C, Hess B, Lindahl E 2015 SoftwareX 1-2 19
Google Scholar
[34] Hoover W G 1985 Phys. Rev. A 31 1695
Google Scholar
[35] Nosé S, Klein M L 1983 Mol. Phys. 50 1055
Google Scholar
[36] Darden T, York D, Pedersen L 1993 J. Chem. Phys. 98 10089
Google Scholar
[37] Carlson S, Brünig F N, Loche P, Bonthuis D J, Netz R R 2020 J. Phys. Chem. A 124 5599
Google Scholar
[38] Ono T, Horikawa K, Maeda Y, Ota M, Sato Y, Inomata H 2016 Fluid Phase Equilibr. 420 30
Google Scholar
[39] Kalinovskaya O E, Vij J K 2000 J. Chem. Phys. 112 3262
Google Scholar
下载:
计量
- 文章访问数: 382
- PDF下载量: 7
- 被引次数: 0
