-
近年来, 基于非厄米拓扑理论, 研究者们通过调制声学晶体中的非互易耦合, 揭示了体态向界面塌陷的趋肤效应. 本工作实验设计了具有不同绕组数域之间的拓扑趋肤界面, 以操纵能量聚焦到非厄米一维声腔链的中间或两端. 首先, 通过电声耦合的方法实现了两个声学腔之间的非互易耦合, 并研究其特性. 其次, 将非互易耦合腔扩展成链状, 通过调制非互易电-声耦合来构建趋肤界面的位置. 实验结果表明, 对于不同的非互易耦合分布, 声音可以集中在中间界面或两端界面, 并且通过改变非互易耦合方向, 可以将趋肤界面从中间切换到两端. 本研究结果为设计控制声音传播的先进拓扑声学装置提供了一个新平台.Topological insulators possess strong topological protection properties and can manipulate the wave propagation to combat disorder and defects. And now they have grown into a large research field in photonic and phononic crystals. However, the conventional topological band theory is used to describe a closed photonic/phononic crystal that is assumed to be a Hermitian system. In fact, actual physical systems often couple with external environment, and generate non-Hermitian Hamiltonians with complex eigenvalues. Recently, many novel topological properties have been induced by the interaction between non-Hermitian phase and topological phase. A prominent example is non-Hermitian skin effect that all eigenstates are localized to the boundary in open system, which is different from the conventional topological edge state. This unique physical phenomenon has inspired various applications, such as wave funneling, enhanced sensing, and topological lasing. In this work, we describe the non-Hermitian skin effect by using winding number domains. The sign of the winding number domain determines the rotation direction of the loops in the complex frequency plane, whose sign can be controlled by the nonreciprocal coupling direction. In this work, we design different topological skin interfaces between different domains with opposite winding numbers to manipulate the energy focusing on middle or two-end of non-Hermitian one-dimensional acoustic cavity chain. In experiment, we use an electroacoustic coupling method, in which a unidirectional coupler composed of microphones, speakers, phase shifters, and amplifiers is used to introduce positive and negative non-reciprocal couplings between the two acoustic cavities, and study the characteristics of these non-reciprocal couplings. Then, the non-reciprocal coupling cavities are extended into a chain structure, and the magnitudes and signs of the non-reciprocal couplings are flexibly controlled by using phase shifters and amplifiers. Through this method, we successfully construct the interfaces between different winding number domains, achieving a one-dimensional non-Hermitian skin effect at various interfaces. The experimental results indicate that the sound can be focused on the middle interface or two-end interfaces for different nonreciprocal coupling distributions, and the skin interface can also be switched from middle to two-end by exchanging the nonreciprocal coupling direction of the domains. Our research results provide greater flexibility for designing acoustic devices and also a new platform for exploring advanced topological acoustic systems for controlling sound propagation.
-
Keywords:
- non-Hermitian skin effect /
- interface state /
- phononic crystal /
- resonant cavity
[1] Lu J Y, Qiu C Y, Ye L P, Fan X Y, Ke M Z, Zhang F, Liu Z Y 2017 Nat. Phys. 13 369
Google Scholar
[2] Peng Y G, Qin C Z, Zhao D G, Shen Y X, Xu X Y, Bao M, Jia H, Zhu X F 2016 Nat. Commun. 7 13368
Google Scholar
[3] 贾鼎, 葛勇, 袁寿其, 孙宏祥 2019 68 224301
Google Scholar
Jia D, Ge Y, Yuan S Q, Sun H X 2019 Acta Phys. Sin. 68 224301
Google Scholar
[4] Cheng Q Q, Pan Y M, Wang H Q, Zhang C S, Yu D, Gover A, Zhang H J, Li T, Zhou L, Zhu S N 2019 Phys. Rev. Lett. 122 173901
Google Scholar
[5] 耿治国, 彭玉桂, 沈亚西, 赵德刚, 祝雪丰 2019 68 227802
Google Scholar
Geng Z G, Peng Y G, Shen Y X, Zhao D G, Zhu X F 2019 Acta Phys. Sin. 68 227802
Google Scholar
[6] Wu L H, Hu X 2015 Phys. Rev. Lett. 114 223901
Google Scholar
[7] 王青海, 李锋, 黄学勤, 陆久阳, 刘正猷 2017 66 204501
Google Scholar
Wang Q H, Li F, Huang X Q, Lu J Y, Liu Z Y 2017 Acta Phys. Sin. 66 204501
Google Scholar
[8] Yang Z J, Gao F, Shi X H, Lin X, Gao Z, Chong Y D, Zhang B L 2015 Phys. Rev. Lett. 114 114301
Google Scholar
[9] He C, Ni X, Ge H, Sun X C, Chen Y B, Lu M H, Liu X P, Chen Y F 2016 Nat. Phys. 12 1124
Google Scholar
[10] Liu H, Xie B Y, Wang H N, Liu W W, Li Z C, Cheng H, Tian J G, Liu Z Y 2023 Phys. Rev. B 108 L161410
Google Scholar
[11] Shandarova K, Rüter C E, Kip D, Makris K G, Christodoulides D N, Peleg O, Segev M 2009 Phys. Rev. Lett. 102 123905
Google Scholar
[12] Iwanow R, May-Arrioja D A, Christodoulides D N, Stegeman G I, Min Y, Sohler W 2005 Phys. Rev. Lett. 95 053902
Google Scholar
[13] Shen Y X, Peng Y G, Zhao D G, Chen X C, Zhu J, Zhu X F 2019 Phys. Rev. Lett. 122 094501
Google Scholar
[14] Shen Y X, Zeng L S, Geng Z G, Zhao D G, Peng Y G, Zhu X F 2020 Phys. Rev. Appl. 14 014043
Google Scholar
[15] Shen Y X, Zeng L S, Geng Z G, Zhao D G, Peng Y G, Zhu J, Zhu X F 2021 Sci. China Phys. Mech. 64 244302
Google Scholar
[16] Tang S, Wu J L, Lü C, Yao J B, Pei Y B, Jiang Y Y 2023 Appl. Phys. Lett. 122 212201
Google Scholar
[17] Tang S, Wu J L, Lü C, Wang X S, Song J, Jiang Y Y 2022 Phys. Rev. B 105 104107
Google Scholar
[18] Tang S, Wu J L, Lü C, Yao J B, Wang X S, Song J, Jiang Y Y 2023 New J. Phys. 25 033032
Google Scholar
[19] Crespi A, Pepe F V, Facchi P, Sciarrino F, Mataloni P, Nakazato H, Pascazio S, Osellame R 2019 Phys. Rev. Lett. 122 130401
Google Scholar
[20] Pinkse P W H, Fischer T, Maunz P, Rempe G 2000 Nature 404 365
Google Scholar
[21] Schäfer F, Herrera I, Cherukattil S, Lovecchio C, Cataliotti F S, Caruso F, Smerzi A 2014 Nat. Commun. 5 3194
Google Scholar
[22] Raimond J M, Sayrin C, Gleyzes S, Dotsenko I, Brune M, Haroche S, Facchi P, Pascazio S 2010 Phys. Rev. Lett. 105 213601
Google Scholar
[23] Barontini G, Hohmann L, Haas F, Estève J, Reichel J 2015 Science 349 1317
Google Scholar
[24] Weidemann S, Kremer1 M, Helbig T, Hofmann T, Stegmaier A, Greiter M, Thomale R, Szameit A 2020 Science 368 311
Google Scholar
[25] Budich J C, Bergholtz E J 2020 Phys. Rev. Lett. 125 180403
Google Scholar
[26] McDonald A, Clerk A A 2020 Nat. Commun. 11 5382
Google Scholar
[27] Longhi S 2018 Ann. Phys. 530 1800023
Google Scholar
[28] Zhu B f, Wang Q, Leykam D, Xue H, Wang Q J, Chong Y D 2022 Phys. Rev. Lett. 129 013903
Google Scholar
[29] Hatano N, Nelson D R 1996 Phys. Rev. Lett. 77 570
Google Scholar
[30] Hatano N, Nelson D R 1998 Phys. Rev. B 58 8384
Google Scholar
[31] Xiao L, Deng T S, Wang K K, Zhu G Y, Wang Z, Yi W, Xue P 2020 Nat. Phys. 16 761
Google Scholar
[32] Helbig T, Hofmann T, Imhof S, Abdelghany M, Kiessling T, Molenkamp L W, Lee C H, Szameit A, Greiter M, Thomale R 2020 Nat. Phys. 16 747
Google Scholar
[33] Zhang Q C, Li Y T, Sun H F, Liu X, Zhao L K, Feng X L, Fan X Y, Qiu C Y 2023 Phys. Rev. Lett. 130 017201
Google Scholar
[34] Zhang L, Yang Y H, Ge Y, Guan Y J, Chen Q L, Yan Q H, Chen F J, Xi R, Li Y Z, Jia D, Yuan S Q, Sun H X, Chen H S, Zhang B L 2021 Nat. Commun. 12 6297
Google Scholar
[35] Gu Z M, Gao H, Xue H R, Li J S, Su Z Q, Zhu J 2022 Nat. Commun. 13 7668
Google Scholar
[36] Zhang K, Yang Z S, Fang C 2022 Nat. Commun. 13 2496
Google Scholar
[37] Zhang K, Fang C, Yang Z S 2023 Phys. Rev. Lett. 131 036402
Google Scholar
[38] Lee C H, Li L H, Gong J B 2019 Phys. Rev. Lett. 123 016805
Google Scholar
[39] Li L H, Lee C H, Gong J B 2020 Phys. Rev. Lett. 124 250402
Google Scholar
[40] Zhu W W, Gong J B 2022 Phys. Rev. B 106 035425
Google Scholar
-
图 1 (a) 通过交叉管连接的相同尺寸的谐振腔模型; (b) 单个谐振腔的本征模式和两个由交叉管连接的谐振腔的本征模式
Fig. 1. (a) Resonator models of the same size connected by cross tubes; (b) the eigenmodes of a single resonator and the eigenmode of two resonators connected by cross tubes.
图 2 (a) 两个相同尺寸的谐振腔1和2, 其中腔体通过交叉管连接, 通过单向耦合器实现非互易耦合; (b) 紧束缚模型示意图; (c) 当放大器关闭时, 对透射谱进行实验测量和数值拟合; (d), (e)当放大器打开时, 实验测量了负耦合(d)和正耦合(e), 并对透射谱进行了数值拟合, 其中红色圆圈与蓝色正方形为实验结果, 红色实线与蓝色实线为数值拟合结果
Fig. 2. (a) Two identical size resonators 1 and 2. The cavity is connected by cross pipe, and the non-reciprocal coupling is realized by unidirectional coupler. (b) Schematic diagram of a tight-binding model. (c) When the amplifier is turned off, the transmission spectrum is experimentally measured and numerically fitted. (d), (e) When the amplifier is turned on, the negative coupling (d) and positive coupling (e) are experimentally measured and numerically fitted to the transmission spectra. The red circle and blue square are experimental results, and the red solid line and blue solid line are numerical fitting results.
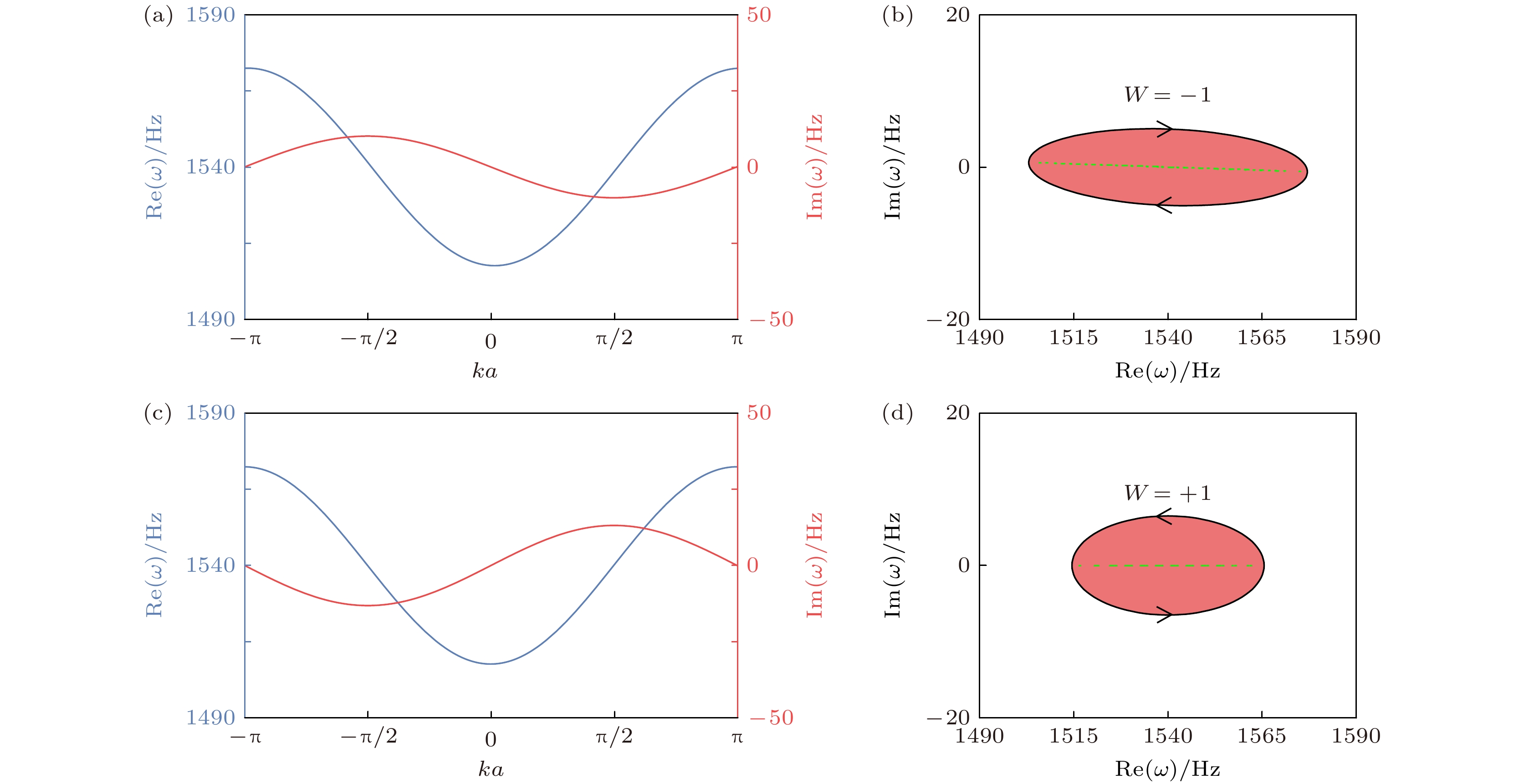
图 3 (a), (c) 当非互易耦合为正(a)或负(c)时, 周期边界条件下本征谱的实部和虚部; (b), (d) 红色区域表示在周期边界条件下, 复本征频谱在复频率平面上形成闭环; 绿色虚线对应于开放边界条件下的本征频谱; 单向耦合为正, 圈数$ W=-1 $ (b), 闭环顺时针旋转; 当单向耦合为负时, 圈数$ W=+1 $ (d), 闭环逆时针旋转
Fig. 3. (a), (c) When the non-reciprocal coupling is positive (a) or negative (c), the real and imaginary parts of the characteristic spectrum under periodic boundary conditions. (b), (d) The red region indicates that the complex eigenfrequency spectrum forms a closed loop in the complex frequency plane under periodic boundary conditions. The green dashed lines correspond to the eigenfrequency spectrum under open boundary conditions. The unidirectional coupling is positive, the winding number $ W=-1 $ (b), and the closed loop rotates clockwise. When the unidirectional coupling is negative, the winding number $ W=+1 $ (d), and the closed loop rotates counterclockwise.
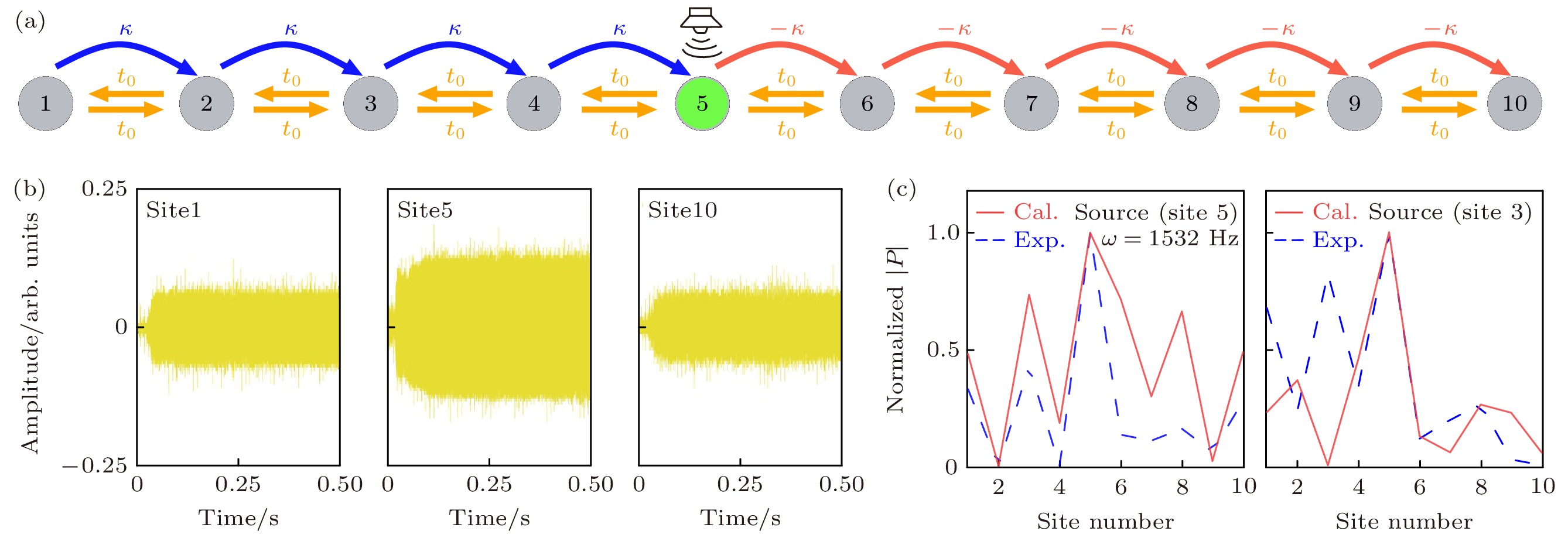
图 4 (a) 实验设备图片, 10个谐振腔通过交叉管和单向耦合器连接; (b) 声音集中在两端界面时的紧束缚模型示意图. 第1—5个空腔之间非互易耦合为负, 第5—10个空腔之间非互易耦合为正; (c) 时域信号传输的测量, 其中结果分别在1, 5, 10腔中测量; (d) 声音集中在两端界面时的声场分布(结果被归一化), 声源频率为1532 Hz
Fig. 4. (a) Experimental equipment picture, 10 resonators connected by cross tubes and unidirectional couplers. (b) Schematic diagram of a tight-binding model when sound is concentrated at the two-end interfaces. The non-reciprocal coupling between 1 to 5 cavities is negative, and the non-reciprocal coupling between 5 to 10 cavities is positive. (c) Measurement of signal transmission in time domain. The results are measured in cavity 1, 5 and 10 respectively. (d) Field distribution of sound locality at the two-end interfaces and the frequency of the sound source is 1532 Hz. The results are normalized.
图 5 (a) 声音集中在中间界面时的紧束缚模型示意图, 第1—5个空腔之间非互易耦合为正, 第5—10个空腔之间非互易耦合为负; (b) 时域信号传输测量, 其中结果分别在1, 5, 10腔中测量; (c) 声音集中在中间界面时的声场分布(结果被归一化), 声源的频率为1532 Hz
Fig. 5. (a) Schematic diagram of a tight-binding model when sound is concentrated at the intermediate interface. The non-reciprocal coupling between 1 to 5 cavities is positive, and the non-reciprocal coupling between 5 to 10 cavities is negative. (b) Measurement of signal transmission in time domain. The results are measured in cavity 1, 5 and 10 respectively. (c) Field distribution of sound locality at the middle interface. The excitation frequency of the sound source is 1532 Hz. The results are normalized.
-
[1] Lu J Y, Qiu C Y, Ye L P, Fan X Y, Ke M Z, Zhang F, Liu Z Y 2017 Nat. Phys. 13 369
Google Scholar
[2] Peng Y G, Qin C Z, Zhao D G, Shen Y X, Xu X Y, Bao M, Jia H, Zhu X F 2016 Nat. Commun. 7 13368
Google Scholar
[3] 贾鼎, 葛勇, 袁寿其, 孙宏祥 2019 68 224301
Google Scholar
Jia D, Ge Y, Yuan S Q, Sun H X 2019 Acta Phys. Sin. 68 224301
Google Scholar
[4] Cheng Q Q, Pan Y M, Wang H Q, Zhang C S, Yu D, Gover A, Zhang H J, Li T, Zhou L, Zhu S N 2019 Phys. Rev. Lett. 122 173901
Google Scholar
[5] 耿治国, 彭玉桂, 沈亚西, 赵德刚, 祝雪丰 2019 68 227802
Google Scholar
Geng Z G, Peng Y G, Shen Y X, Zhao D G, Zhu X F 2019 Acta Phys. Sin. 68 227802
Google Scholar
[6] Wu L H, Hu X 2015 Phys. Rev. Lett. 114 223901
Google Scholar
[7] 王青海, 李锋, 黄学勤, 陆久阳, 刘正猷 2017 66 204501
Google Scholar
Wang Q H, Li F, Huang X Q, Lu J Y, Liu Z Y 2017 Acta Phys. Sin. 66 204501
Google Scholar
[8] Yang Z J, Gao F, Shi X H, Lin X, Gao Z, Chong Y D, Zhang B L 2015 Phys. Rev. Lett. 114 114301
Google Scholar
[9] He C, Ni X, Ge H, Sun X C, Chen Y B, Lu M H, Liu X P, Chen Y F 2016 Nat. Phys. 12 1124
Google Scholar
[10] Liu H, Xie B Y, Wang H N, Liu W W, Li Z C, Cheng H, Tian J G, Liu Z Y 2023 Phys. Rev. B 108 L161410
Google Scholar
[11] Shandarova K, Rüter C E, Kip D, Makris K G, Christodoulides D N, Peleg O, Segev M 2009 Phys. Rev. Lett. 102 123905
Google Scholar
[12] Iwanow R, May-Arrioja D A, Christodoulides D N, Stegeman G I, Min Y, Sohler W 2005 Phys. Rev. Lett. 95 053902
Google Scholar
[13] Shen Y X, Peng Y G, Zhao D G, Chen X C, Zhu J, Zhu X F 2019 Phys. Rev. Lett. 122 094501
Google Scholar
[14] Shen Y X, Zeng L S, Geng Z G, Zhao D G, Peng Y G, Zhu X F 2020 Phys. Rev. Appl. 14 014043
Google Scholar
[15] Shen Y X, Zeng L S, Geng Z G, Zhao D G, Peng Y G, Zhu J, Zhu X F 2021 Sci. China Phys. Mech. 64 244302
Google Scholar
[16] Tang S, Wu J L, Lü C, Yao J B, Pei Y B, Jiang Y Y 2023 Appl. Phys. Lett. 122 212201
Google Scholar
[17] Tang S, Wu J L, Lü C, Wang X S, Song J, Jiang Y Y 2022 Phys. Rev. B 105 104107
Google Scholar
[18] Tang S, Wu J L, Lü C, Yao J B, Wang X S, Song J, Jiang Y Y 2023 New J. Phys. 25 033032
Google Scholar
[19] Crespi A, Pepe F V, Facchi P, Sciarrino F, Mataloni P, Nakazato H, Pascazio S, Osellame R 2019 Phys. Rev. Lett. 122 130401
Google Scholar
[20] Pinkse P W H, Fischer T, Maunz P, Rempe G 2000 Nature 404 365
Google Scholar
[21] Schäfer F, Herrera I, Cherukattil S, Lovecchio C, Cataliotti F S, Caruso F, Smerzi A 2014 Nat. Commun. 5 3194
Google Scholar
[22] Raimond J M, Sayrin C, Gleyzes S, Dotsenko I, Brune M, Haroche S, Facchi P, Pascazio S 2010 Phys. Rev. Lett. 105 213601
Google Scholar
[23] Barontini G, Hohmann L, Haas F, Estève J, Reichel J 2015 Science 349 1317
Google Scholar
[24] Weidemann S, Kremer1 M, Helbig T, Hofmann T, Stegmaier A, Greiter M, Thomale R, Szameit A 2020 Science 368 311
Google Scholar
[25] Budich J C, Bergholtz E J 2020 Phys. Rev. Lett. 125 180403
Google Scholar
[26] McDonald A, Clerk A A 2020 Nat. Commun. 11 5382
Google Scholar
[27] Longhi S 2018 Ann. Phys. 530 1800023
Google Scholar
[28] Zhu B f, Wang Q, Leykam D, Xue H, Wang Q J, Chong Y D 2022 Phys. Rev. Lett. 129 013903
Google Scholar
[29] Hatano N, Nelson D R 1996 Phys. Rev. Lett. 77 570
Google Scholar
[30] Hatano N, Nelson D R 1998 Phys. Rev. B 58 8384
Google Scholar
[31] Xiao L, Deng T S, Wang K K, Zhu G Y, Wang Z, Yi W, Xue P 2020 Nat. Phys. 16 761
Google Scholar
[32] Helbig T, Hofmann T, Imhof S, Abdelghany M, Kiessling T, Molenkamp L W, Lee C H, Szameit A, Greiter M, Thomale R 2020 Nat. Phys. 16 747
Google Scholar
[33] Zhang Q C, Li Y T, Sun H F, Liu X, Zhao L K, Feng X L, Fan X Y, Qiu C Y 2023 Phys. Rev. Lett. 130 017201
Google Scholar
[34] Zhang L, Yang Y H, Ge Y, Guan Y J, Chen Q L, Yan Q H, Chen F J, Xi R, Li Y Z, Jia D, Yuan S Q, Sun H X, Chen H S, Zhang B L 2021 Nat. Commun. 12 6297
Google Scholar
[35] Gu Z M, Gao H, Xue H R, Li J S, Su Z Q, Zhu J 2022 Nat. Commun. 13 7668
Google Scholar
[36] Zhang K, Yang Z S, Fang C 2022 Nat. Commun. 13 2496
Google Scholar
[37] Zhang K, Fang C, Yang Z S 2023 Phys. Rev. Lett. 131 036402
Google Scholar
[38] Lee C H, Li L H, Gong J B 2019 Phys. Rev. Lett. 123 016805
Google Scholar
[39] Li L H, Lee C H, Gong J B 2020 Phys. Rev. Lett. 124 250402
Google Scholar
[40] Zhu W W, Gong J B 2022 Phys. Rev. B 106 035425
Google Scholar
下载:
计量
- 文章访问数: 708
- PDF下载量: 79
- 被引次数: 0
