无阀纳米泵中水流的反常堵塞

李伟健; 周晓艳; 陆杭军

doi:10.7498/aps.73.20240115

摘要

对于颗粒物质, 在锥形通道的窄口处容易发生堵塞现象, 实验中通常利用机械振动进行疏通. 而对于无孔不入的水却不同, 即使在纳米尺度的碳纳米管中仍然可以快速通透. 本文利用分子动力学模拟研究了由锥形碳纳米管与石墨烯平面构成的无阀纳米泵, 发现水输运在一定条件下也会出现反常堵塞. 与颗粒物质截然不同的是振动方法无法恢复水流, 相反, 它促使水在纳米水泵的通道窄口处发生堵塞. 通过分析堵塞区水的密度分布、氢键寿命、水分子的结构特征, 揭示了反常堵塞是由腔体中振动膜的高频振动引发水的结构相变造成的.

关键词:

Abstract

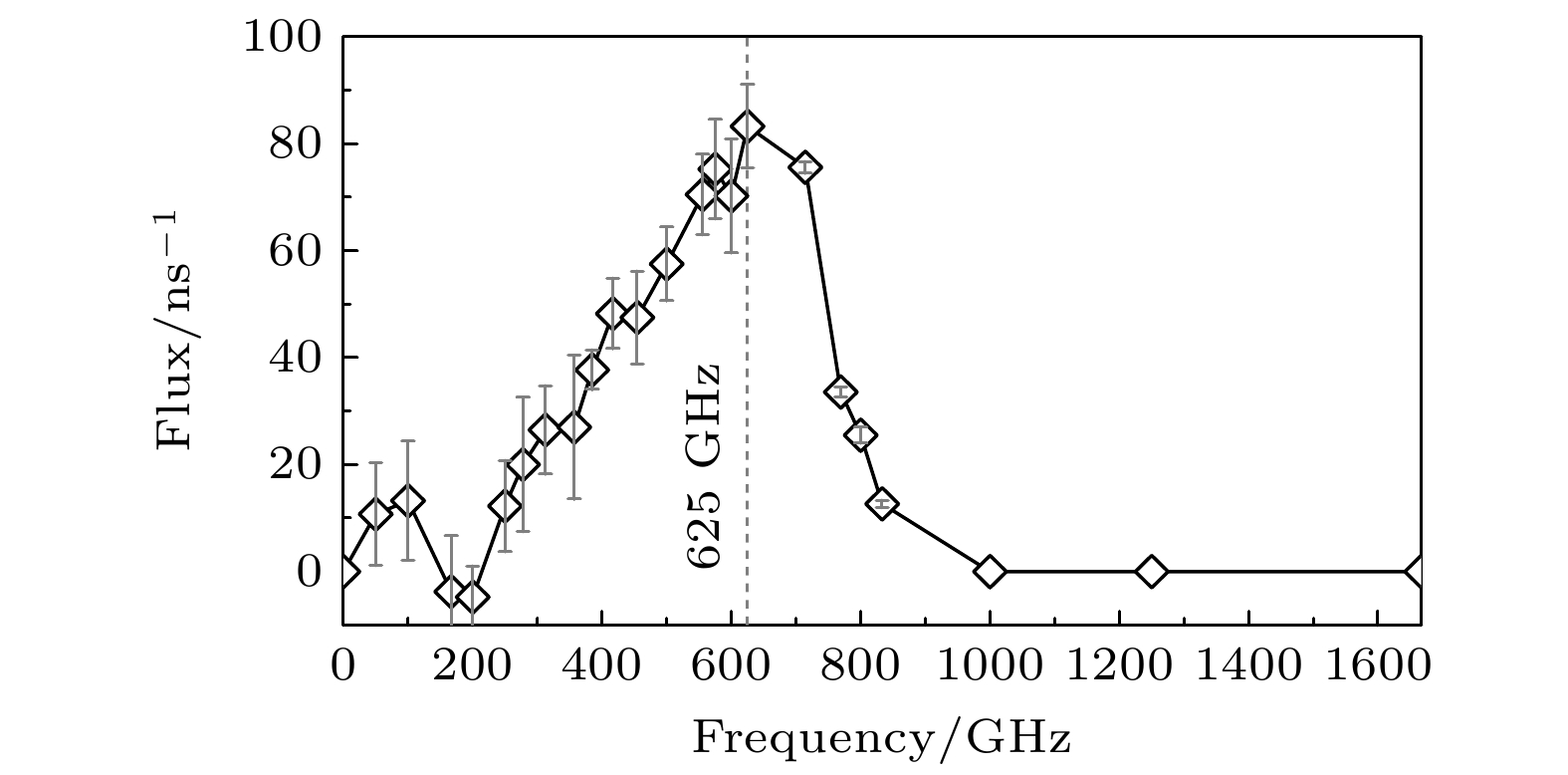
In the narrow orifice of a cone-shaped channel, blockage can occur for granular matter. However, water molecules can enter into and even permeate through carbon nanotubes of diameters down to 0.8 nm at ultrafast rates. Here we demonstrate by molecular dynamics simulations that clogging can also emerge unexpectedly in the water flowing through a nanoscale valve-less pump. The designed pump features two truncated carbon nanocones, with the narrowest region having a diameter of 1.2 nm (larger than that of (6, 6) carbon nanotube), linked to a fluid cavity volume, and is powered by the vibration of a graphene sheet. In the low frequency range, water molecules can be driven through the nanocones effectively by the vibration of the graphene sheet. The maximum flux reaches 83 ns^–1, which is approximately 20 times the measured value of (3.9 ± 0.6) ns^–1 for aquaporin-1. However, at higher frequencies, water molecules suffer blockage at the narrow exits. Much unlike granular matter, high-frequency vibration cannot restore water flow. The key to this phenomenon is that in the narrow exits of two nanocones acting as diffuser/nozzle, the number density of water molecules rapidly increases with frequency increasing, the tight hydrogen-bonding network is formed, and the mean lifetime of hydrogen bonds increases dramatically under high-frequency vibrations. High frequency fluctuations in the middle chamber make H-bond network between water molecules in the narrow exits more stable. The probability density distribution of water exhibits a non-equilibrium transition from a disordered state to ordered state. This work reveals a new mechanism of water flowing/blocking in a nanoscale valve-less pump based on two asymmetrical nanocones, offers valuable insights into understanding nonequilibrium jamming transition in nanoscale fluid.

Keywords:

作者及机构信息

浙江师范大学, 凝聚态物理研究所, 金华　321004

通信作者: 周晓艳, zxylu@zjnu.cn ; 陆杭军, zjlhjun@zjnu.cn

基金项目: 基金:国家自然科学基金(批准号: 11875237)资助的课题.

Authors and contacts

Institute of Condensed Matter Physics, Zhejiang Normal University, Jinhua 321004, China

Corresponding author: Zhou Xiao-Yan, zxylu@zjnu.cn ; Lu Hang-Jun, zjlhjun@zjnu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 11875237).

文章全文 : translate this paragraph

参考文献

[1]	Squires T M, Quake S R 2005 Rev. Mod. Phys. 77 977 Google Scholar
[2]	Laser D J, Santiago J G 2004 J. Micromech. Microeng. 14 R35 Google Scholar
[3]	Whitesides G M 2006 Nature 442 368 Google Scholar
[4]	Teh S Y, Lin R, Hung L H, Lee A P 2008 Lab Chip 8 198 Google Scholar
[5]	蒋丹, 李松晶, 杨平 2013 62 224703 Google Scholar Jiang D, Li S J, Yang P 2013 Acta Phys. Sin. 62 224703 Google Scholar
[6]	Hummer G, Rasaiah J C, Noworyta J P 2001 Nature 414 188 Google Scholar
[7]	Ghosh S, Sood A K, Kumar N 2003 Science 299 1042 Google Scholar
[8]	Chen X, Cao G, Han A, Punyamurtula V K, Qiao Y 2008 Nano Lett. 8 2988 Google Scholar
[9]	Holt J K, Park H G, Wang Y M, Stadermann M, Artyukhin A B, Grigoropoulos C P, Noy A, Bakajin O 2006 Science 312 1034 Google Scholar
[10]	Fang H P, Wan R Z, Gong X J, Lu H J, Li S Y 2008 J. Phys. D: Appl. Phys. 41 103002 Google Scholar
[11]	Lu H J, Li J Y, Gong X J, Wan R Z, Zeng L, Fang H P 2008 Phys. Rev. B 77 174115 Google Scholar
[12]	方海平 2016 65 186101 Google Scholar Fang H P 2016 Acta Phys. Sin. 65 186101 Google Scholar
[13]	Falk K, Sedlmeier F, Joly L, Netz R R, Bocquet L 2010 Nano Lett. 10 4067 Google Scholar
[14]	Liu C, Li Z G 2010 Phys. Rev. Lett. 105 174501 Google Scholar
[15]	Qiu H, Shen R, Guo W L 2011 Nano Res. 4 284 Google Scholar
[16]	Su J, Guo H 2011 ACS Nano 5 351 Google Scholar
[17]	Wang Y, Zhao Y J, Huang J P 2011 J. Phys. Chem. B 115 13275 Google Scholar
[18]	Li X P, Kong G P, Zhang X, He G W 2013 Appl. Phys. Lett. 103 143117 Google Scholar
[19]	Feng J W, Ding H M, Ren C L, Ma Y Q 2014 Nanoscale 6 13606 Google Scholar
[20]	Cao W, Wang J, Ma M 2018 Microfluid. Nanofluid. 22 125 Google Scholar
[21]	Leng J T, Ying T Q, Guo Z R, Zhang Y Y, Chang T C, Guo W L, Gao H J 2022 Carbon 191 175 Google Scholar
[22]	Zhang Q L, Jiang W Z, Liu J, Miao R D, Sheng N 2013 Phys. Rev. Lett. 110 254501 Google Scholar
[23]	Kou J, Lu H, Wu F, Fan J, Yao J 2014 Nano Lett. 14 4931 Google Scholar
[24]	Zhou X Y, Wu F M, Liu Y, Kou J L, Lu H, Lu H J 2015 Phys. Rev. E 92 053017 Google Scholar
[25]	Zhou X Y, Zhu F Q 2018 Phys. Rev. E 98 032410 Google Scholar
[26]	Zhu Z, Chang C, Shu Y, Song B 2020 J. Phys. Chem. Lett. 11 256 Google Scholar
[27]	Fan W, Chen J 2020 Phys. Rev. E 101 010101 Google Scholar
[28]	张忠强, 范晋伟, 张福建, 程广贵, 丁建宁 2020 69 110201 Google Scholar Zhang Z Q, Fan J W, Zhang F J, Cheng G G, Ding J N 2020 Acta Phys. Sin. 69 110201 Google Scholar
[29]	Olsson A, Stemme G, Stemme E 2000 Sens. Actuators A Phys. 84 165 Google Scholar
[30]	Chinappi M, De Angelis E, Melchionna S, Casciola C, Succi S, Piva R 2006 Phys. Rev. Lett. 97 144509 Google Scholar
[31]	Ai B Q, Liu L G 2008 J. Chem. Phys. 128 024706 Google Scholar
[32]	Tajkhorshid E, Nollert P, Jensen M O, Miercke L J W, O'connell J, Stroud R M, Schulten K 2002 Science 296 525 Google Scholar
[33]	Kosztin I, Schulten K 2004 Phys. Rev. Lett. 93 238102 Google Scholar
[34]	Zhou M, Morais-Cabral J H, Mann S, Mackinnon R 2001 Nature 411 657 Google Scholar
[35]	Tang J, Behringer R P 2011 Chaos 21 041107 Google Scholar
[36]	麻礼东, 杨光辉, 张晟, 林平, 田园, 杨磊 2018 67 044501 Google Scholar Ma L D, Yang G H, Zhang S, Lin P, Tian Y, Yang L 2018 Acta Phys. Sin. 67 044501 Google Scholar
[37]	Janda A, Maza D, Garcimartín A, Kolb E, Clément E 2009 Europhys. Lett. 87 24002 Google Scholar
[38]	Majumder M, Chopra N, Andrews R, Hinds B J 2005 Nature 438 44 Google Scholar
[39]	Lindahl E, Hess B, Van Der Spoel D 2001 J. Mol. Model. 7 306 Google Scholar
[40]	Bussi G, Donadio D, Parrinello M 2007 J. Chem. Phys. 126 014101 Google Scholar
[41]	Zeidel M, Ambudkar S, Smith B, Agre P 1992 Biochemistry 31 7436 Google Scholar

施引文献

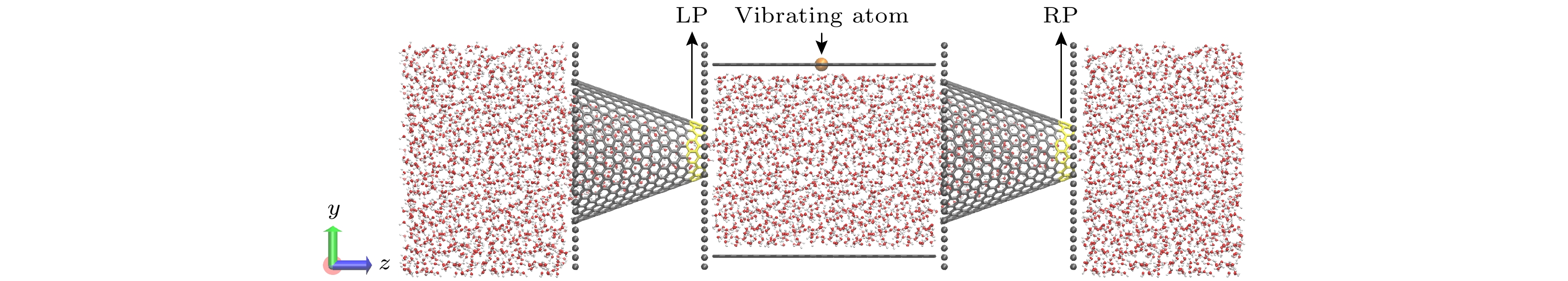
图 1 模拟系统图, 由左右两个锥形碳纳米管及中间泵体组成的无阀纳米泵, 通过两个碳平面(灰色小球)连接左右水浴, 红色小球和白色小球分别表示水分子中的氧原子和氢原子; 橘色小球代表振动原子; 左右锥形碳纳米管的窄口端用黄色标记, 分别记为LP (left pore)和RP (right pore)

Fig. 1. Simulation system. A valve-less nanopump consists of carbon nanocones on both sides, a fluid cavity in the center, connected by carbon planes (gray spheres) to two reservoirs. Red and white spheres represent oxygen and hydrogen atoms in water molecules, respectively. The orange sphere represents the vibrating atom. The narrow ends of the carbon nanocones are marked in yellow and labeled as LP (left pore) and RP (right pore), respectively.

下载: 全尺寸图片幻灯片

图 2 水流量随振动频率的变化

Fig. 2. Average water flux as a function of the vibration frequency.

下载: 全尺寸图片幻灯片

图 3 在锥形纳米管窄口端0.4 nm厚的区域内水分子的概率密度分布图　(a) f = 384 GHz; (b) f = 625 GHz; (c) f = 833 GHz; (d) f = 1250 GHz

Fig. 3. Probability density distributions of water molecules in LP for different vibrational frequency: (a) f = 384 GHz; (b) f = 625 GHz; (c) f = 833 GHz; (d) f = 1250 GHz.

下载: 全尺寸图片幻灯片

图 4 LP和RP区域的平均水分子数, 两个区域厚度为0.4 nm, 分别包含2层水分子, L₁和R₁分别表示两个区域中右侧的(管径最小)水分子层; L₂和R₂则表示左侧的水分子层

Fig. 4. Average number of water molecules in each water layer in LP and RP. L₁ and R₁ represent the right-side (minimum diameter) water molecule layers in the two regions, while L₂ and R₂ represent the left-side water molecule layers.

下载: 全尺寸图片幻灯片

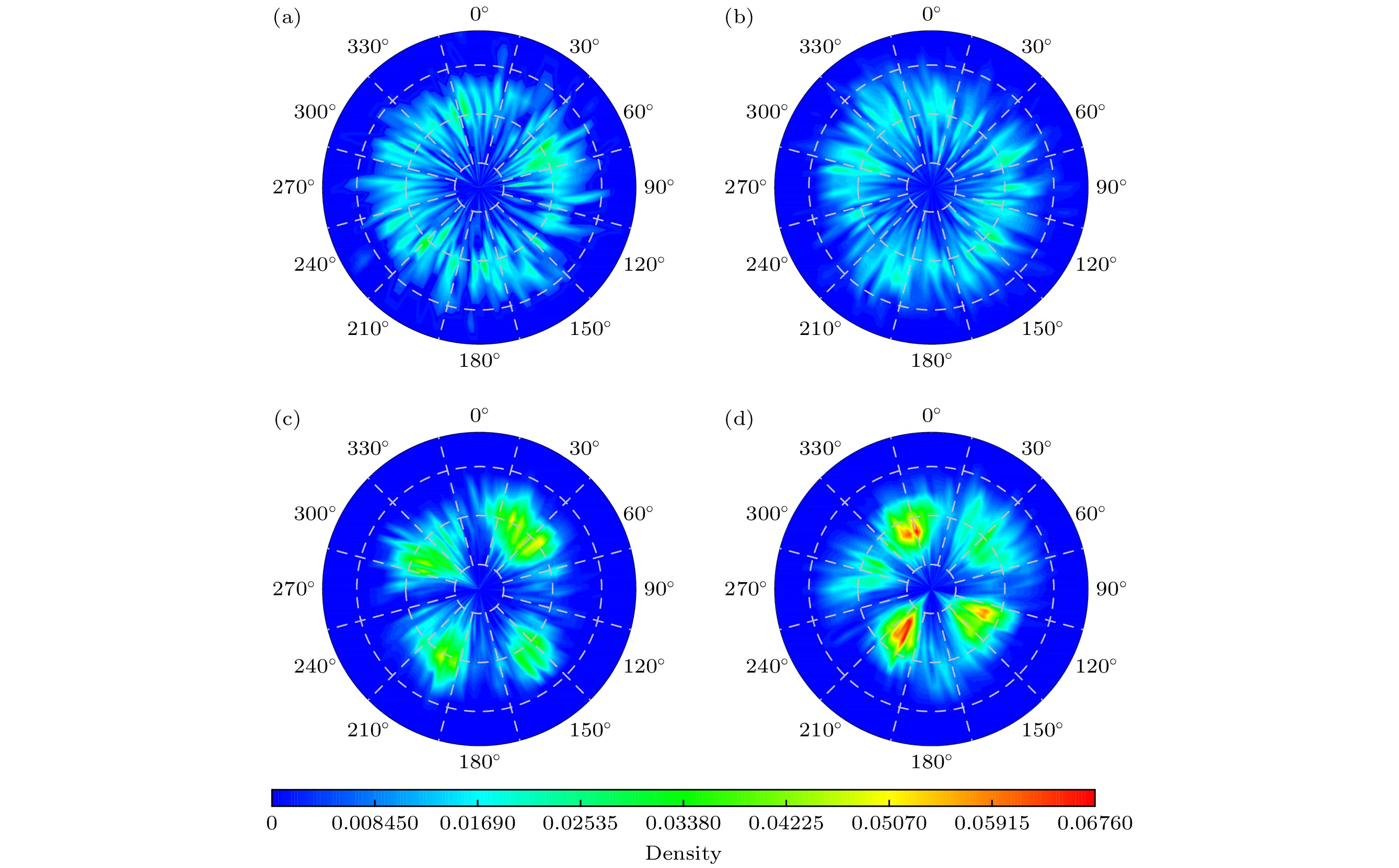
图 5 (a)氢键的平均寿命随频率的变化, 插图分别为f = 250 GHz和f = 1250 GHz对应的 LP里第1层水的模拟快照(青色小球表示碳原子, 红色和白色小球分别表示水分子中的氧原子和氢原子); (b) LP区域第1层水分子在横截面上的方位角概率分布图

Fig. 5. (a) Lifetime of hydrogen bonds as a function of the vibration frequency. Two typical snapshots are shown in insets. The cyan spheres represent carbon atoms, with red and white spheres representing oxygen and hydrogen atoms in water molecules. One is for the low frequency (f = 250 GHz) and the other is for the high frequency (f = 1250 GHz). (b) Probability distribution of the azimuthal angle of water molecules in the first layer in the LP region on the cross-section for f = 250 GHz and f = 1250 GHz.

下载: 全尺寸图片幻灯片

map

[1]	Squires T M, Quake S R 2005 Rev. Mod. Phys. 77 977 Google Scholar
[2]	Laser D J, Santiago J G 2004 J. Micromech. Microeng. 14 R35 Google Scholar
[3]	Whitesides G M 2006 Nature 442 368 Google Scholar
[4]	Teh S Y, Lin R, Hung L H, Lee A P 2008 Lab Chip 8 198 Google Scholar
[5]	蒋丹, 李松晶, 杨平 2013 62 224703 Google Scholar Jiang D, Li S J, Yang P 2013 Acta Phys. Sin. 62 224703 Google Scholar
[6]	Hummer G, Rasaiah J C, Noworyta J P 2001 Nature 414 188 Google Scholar
[7]	Ghosh S, Sood A K, Kumar N 2003 Science 299 1042 Google Scholar
[8]	Chen X, Cao G, Han A, Punyamurtula V K, Qiao Y 2008 Nano Lett. 8 2988 Google Scholar
[9]	Holt J K, Park H G, Wang Y M, Stadermann M, Artyukhin A B, Grigoropoulos C P, Noy A, Bakajin O 2006 Science 312 1034 Google Scholar
[10]	Fang H P, Wan R Z, Gong X J, Lu H J, Li S Y 2008 J. Phys. D: Appl. Phys. 41 103002 Google Scholar
[11]	Lu H J, Li J Y, Gong X J, Wan R Z, Zeng L, Fang H P 2008 Phys. Rev. B 77 174115 Google Scholar
[12]	方海平 2016 65 186101 Google Scholar Fang H P 2016 Acta Phys. Sin. 65 186101 Google Scholar
[13]	Falk K, Sedlmeier F, Joly L, Netz R R, Bocquet L 2010 Nano Lett. 10 4067 Google Scholar
[14]	Liu C, Li Z G 2010 Phys. Rev. Lett. 105 174501 Google Scholar
[15]	Qiu H, Shen R, Guo W L 2011 Nano Res. 4 284 Google Scholar
[16]	Su J, Guo H 2011 ACS Nano 5 351 Google Scholar
[17]	Wang Y, Zhao Y J, Huang J P 2011 J. Phys. Chem. B 115 13275 Google Scholar
[18]	Li X P, Kong G P, Zhang X, He G W 2013 Appl. Phys. Lett. 103 143117 Google Scholar
[19]	Feng J W, Ding H M, Ren C L, Ma Y Q 2014 Nanoscale 6 13606 Google Scholar
[20]	Cao W, Wang J, Ma M 2018 Microfluid. Nanofluid. 22 125 Google Scholar
[21]	Leng J T, Ying T Q, Guo Z R, Zhang Y Y, Chang T C, Guo W L, Gao H J 2022 Carbon 191 175 Google Scholar
[22]	Zhang Q L, Jiang W Z, Liu J, Miao R D, Sheng N 2013 Phys. Rev. Lett. 110 254501 Google Scholar
[23]	Kou J, Lu H, Wu F, Fan J, Yao J 2014 Nano Lett. 14 4931 Google Scholar
[24]	Zhou X Y, Wu F M, Liu Y, Kou J L, Lu H, Lu H J 2015 Phys. Rev. E 92 053017 Google Scholar
[25]	Zhou X Y, Zhu F Q 2018 Phys. Rev. E 98 032410 Google Scholar
[26]	Zhu Z, Chang C, Shu Y, Song B 2020 J. Phys. Chem. Lett. 11 256 Google Scholar
[27]	Fan W, Chen J 2020 Phys. Rev. E 101 010101 Google Scholar
[28]	张忠强, 范晋伟, 张福建, 程广贵, 丁建宁 2020 69 110201 Google Scholar Zhang Z Q, Fan J W, Zhang F J, Cheng G G, Ding J N 2020 Acta Phys. Sin. 69 110201 Google Scholar
[29]	Olsson A, Stemme G, Stemme E 2000 Sens. Actuators A Phys. 84 165 Google Scholar
[30]	Chinappi M, De Angelis E, Melchionna S, Casciola C, Succi S, Piva R 2006 Phys. Rev. Lett. 97 144509 Google Scholar
[31]	Ai B Q, Liu L G 2008 J. Chem. Phys. 128 024706 Google Scholar
[32]	Tajkhorshid E, Nollert P, Jensen M O, Miercke L J W, O'connell J, Stroud R M, Schulten K 2002 Science 296 525 Google Scholar
[33]	Kosztin I, Schulten K 2004 Phys. Rev. Lett. 93 238102 Google Scholar
[34]	Zhou M, Morais-Cabral J H, Mann S, Mackinnon R 2001 Nature 411 657 Google Scholar
[35]	Tang J, Behringer R P 2011 Chaos 21 041107 Google Scholar
[36]	麻礼东, 杨光辉, 张晟, 林平, 田园, 杨磊 2018 67 044501 Google Scholar Ma L D, Yang G H, Zhang S, Lin P, Tian Y, Yang L 2018 Acta Phys. Sin. 67 044501 Google Scholar
[37]	Janda A, Maza D, Garcimartín A, Kolb E, Clément E 2009 Europhys. Lett. 87 24002 Google Scholar
[38]	Majumder M, Chopra N, Andrews R, Hinds B J 2005 Nature 438 44 Google Scholar
[39]	Lindahl E, Hess B, Van Der Spoel D 2001 J. Mol. Model. 7 306 Google Scholar
[40]	Bussi G, Donadio D, Parrinello M 2007 J. Chem. Phys. 126 014101 Google Scholar
[41]	Zeidel M, Ambudkar S, Smith B, Agre P 1992 Biochemistry 31 7436 Google Scholar

[1]	黄远志, 杨传浩, 何颂平, 马瑞松, 郇庆. 基于干式制冷的低温扫描探针显微镜研究进展. , 2024, 73(22): 228701. doi: 10.7498/aps.73.20241367
[2]	田晓俊, 孔繁芳, 经士浩, 郁云杰, 张尧, 张杨, 董振超. 单分子瞬时带电态中电子-振动耦合特性的亚纳米荧光成像研究. , 2022, 71(6): 063301. doi: 10.7498/aps.71.20212003
[3]	段铜川, 闫韶健, 赵妍, 孙庭钰, 李阳梅, 朱智. 水的氢键网络动力学与其太赫兹频谱的关系. , 2021, 70(24): 248702. doi: 10.7498/aps.70.20211731
[4]	秦晓玲, 朱栩量, 曹靖雯, 王浩诚, 张鹏. 冰的氢键振动研究. , 2021, 70(14): 146301. doi: 10.7498/aps.70.20210013
[5]	史慧敏, 胡静, 王成会, 凤飞龙, 莫润阳. 有限长管内包膜微泡在磁-声复合场作用下的振动行为. , 2021, 70(21): 214303. doi: 10.7498/aps.70.20210559
[6]	张忠强, 范晋伟, 张福建, 程广贵, 丁建宁. 水流在旋转黑磷纳米管内轴向驱动特性. , 2020, 69(11): 110201. doi: 10.7498/aps.69.20200116
[7]	吴晔盛, 刘启, 曹杰, 李凯, 程广贵, 张忠强, 丁建宁, 蒋诗宇. 收集振动能的摩擦纳米发电机设计与输出性能. , 2019, 68(19): 190201. doi: 10.7498/aps.68.20190806
[8]	赵章风, 张文俊, 牛丽丽, 孟龙, 郑海荣. 基于微泡共振的快速微流体声学混合方法研究. , 2018, 67(19): 194302. doi: 10.7498/aps.67.20180705
[9]	刘国栋, 许新科, 刘炳国, 陈凤东, 胡涛, 路程, 甘雨. 基于振动抑制高精度宽带激光扫频干涉测量方法. , 2016, 65(20): 209501. doi: 10.7498/aps.65.209501
[10]	孙润智, 汪治中, 汪茂胜, 张季谦. 二维格子神经元网络的振动共振和非线性振动共振. , 2015, 64(11): 110501. doi: 10.7498/aps.64.110501
[11]	胡格丽, 倪志鹏, 王秋良. 结合振动控制的柱面纵向梯度线圈目标场设计方法. , 2014, 63(1): 018301. doi: 10.7498/aps.63.018301
[12]	南一冰, 唐义, 张丽君, 常月娥, 陈廷爱. 一种卫星平台振动光谱成像数据分块校正方法. , 2014, 63(1): 010701. doi: 10.7498/aps.63.010701
[13]	张富翁, 王立, 刘传平, 吴平. 竖直振动管中颗粒的上升运动. , 2014, 63(1): 014501. doi: 10.7498/aps.63.014501
[14]	蒋丹, 李松晶, 杨平. 无阀微泵腔内气泡对周期驱动压力的影响. , 2013, 62(22): 224703. doi: 10.7498/aps.62.224703
[15]	唐秋艳, 唐义, 曹玮亮, 王静, 南一冰, 倪国强. 卫星平台复杂振动引起的光谱成像退化仿真研究. , 2012, 61(7): 070202. doi: 10.7498/aps.61.070202
[16]	冯海冉, 李鹏, 郑雨军, 丁世良. 用李代数方法解析研究线性三原子分子振动的动力学纠缠. , 2010, 59(8): 5246-5250. doi: 10.7498/aps.59.5246
[17]	赵永志, 江茂强, 郑津洋. 巴西果效应分离过程的计算颗粒力学模拟研究. , 2009, 58(3): 1812-1818. doi: 10.7498/aps.58.1812
[18]	俞阿龙. 基于小波神经网络的振动速度传感器幅频特性补偿研究. , 2007, 56(6): 3166-3171. doi: 10.7498/aps.56.3166
[19]	姜泽辉, 陆坤权, 厚美瑛, 陈唯, 陈相君. 振动颗粒混合物中的三明治式分离. , 2003, 52(9): 2244-2248. doi: 10.7498/aps.52.2244
[20]	李宏年, 徐亚伯, 李海洋, 何丕模, 鲍世宁. 单层纳米碳管振动模的拉曼光谱研究. , 1999, 48(2): 273-278. doi: 10.7498/aps.48.273

计量

文章访问数: 1397
PDF下载量: 26
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

无阀纳米泵中水流的反常堵塞