微通道中一类生物流体在高Zeta势下的电渗流及传热特性

慕江勇; 崔继峰; 陈小刚; 赵毅康; 田祎琳; 于欣如; 袁满玉

doi:10.7498/aps.73.20231685

摘要

在高壁面Zeta势下, 研究滑移边界条件下满足牛顿流体模型的一类生物流体的电渗流动及传热特性, 流体在外加电场、磁场和焦耳加热共同作用下流动. 首先, 在不使用Debye-Hückel线性近似条件时, 利用切比雪夫谱方法给出非线性Poisson-Boltzmann方程和流函数满足的四阶微分方程及热能方程的数值解, 将所得结果与利用Debye-Hückel线性近似所得结果进行比较, 证明本文数值方法的有效性. 其次, 讨论电磁环境下壁面Zeta势、哈特曼数$H$、电渗参数$m$、滑移参数$\beta $对流动特性、泵送特性和捕获现象的影响, 并探究焦耳加热参数$\gamma $和布林克曼数$Br$等参数对传热特性的影响. 结果表明, 壁面Zeta势、电渗参数$m$、滑移参数$\beta $的增大对流体速度有促进作用, 而哈特曼数$H$的增大会抵抗流体流动. 研究进一步表明, 焦耳加热参数$\gamma $和布林克曼数$Br$的增大会导致温度升高.

关键词:

Abstract

Peristalsis is an important dynamic phenomenon in the field of biomedical research, and has great application prospects in microscale fluids. In recent years, this biomimetic (peristaltic) phenomenon has gained widespread attention due to its large-scale applications in various medical and industrial fields, such as radiation therapy, peristaltic blood pumps, and drug delivery systems. In this study, the electroosmotic flow and heat transfer characteristics are investigated under high wall Zeta potential and slip boundary conditions for a certain type of biological fluid that satisfies the Newtonian fluid model. Fluid flows under the joint action of external electric field, magnetic field, and Joule heating. Firstly, without using the Debye-Hückel linear approximation, the numerical solutions are given by using the Chebyshev spectral method for the nonlinear Poisson-Boltzmann equation, the fourth-order differential equation satisfied by the stream function, and the thermal energy equation. The results are compared with those obtained by using the Debye-Hückel linear approximation to demonstrate the effectiveness of the numerical method used in this study. Secondly, the effects of wall Zeta potential, Hartmann number $H$, electroosmotic parameter $m$, slip parameter $\beta $ are discussed on the flow characteristics, peristaltic pumping, and trapping phenomena under electromagnetic environments, and the influence of Joule heating parameter $\gamma $ and Brinkman number $Br$ is explored on heat transfer characteristics. The results show that 1) wall Zeta potential plays an important role in controlling the velocity of fluid peristaltic flow; 2) the increase of electroosmotic parameter $m$ and slip parameter $\beta $ increases the flow velocity in the central region of the channel, while the increase of Hartmann number $H$ hinders the flow of fluid; 3) these flow behaviors exhibit opposite trends near the channel walls; 4) the number of streamlines captured by peristaltic transport decreases with Hartmann number $H$ and electroosmotic parameter $m$ increasing; 5) the increase of Joule heating parameter $\gamma $ and Brinkman number $Br$ leads temperature to rise.

Keywords:

作者及机构信息

内蒙古工业大学理学院, 呼和浩特　010051

通信作者: 陈小刚, xiaogang_chen@imut.edu.cn

基金项目: 国家自然科学基金(批准号: 12062018, 12172333)、内蒙古自治区高等学校青年科技英才支持计划(批准号: NJYT22075)和内蒙古自治区直属高校基本科研业务费(批准号: JY20220331, ZTY2023014)资助的课题.

Authors and contacts

College of Science, Inner Mongolia University of Technology, Hohhot 010051, China

Corresponding author: Chen Xiao-Gang, xiaogang_chen@imut.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 12062018, 12172333), the Young Talents of Science and Technology in Universities of Inner Mongolia Autonomous Region, China (Grant No. NJYT22075), and the Directly Managed University Basic Research Business Fund Projects of Inner Mongolia Autonomous Region, China (Grant Nos. JY20220331, ZTY2023014).

文章全文 : translate this paragraph

参考文献

[1]	Maiti S, Pandey S K, Misra J C 2022 J. Eng. Math. 135 8 Google Scholar
[2]	Jaiswal S, Yadav P K 2022 Microfluid Nanofluid 26 100 Google Scholar
[3]	Patel H R, Patel S D, Darji R 2022 Int. J. Thermofluids 16 100232 Google Scholar
[4]	Sherief H H, Faltas M S, El-Sapa S 2019 Eur. J. Mech. B Fluids 77 211 Google Scholar
[5]	Chandra S, Kumar Pandey S 2018 J. Phys. Conf. Ser. 1141 012092 Google Scholar
[6]	Yasmin H, Nisar Z 2023 Mathematics 11 2673 Google Scholar
[7]	Mishra N K, Sharma B K, Sharma P, Muhammad T, Pérez L M 2023 Sci. Rep. 13 14483 Google Scholar
[8]	Guedri K, Lashin M M A, Abbasi A, Khan S U, Farooq W, Khan M I, Galal A M 2023 Chin. J. Phys. 83 650 Google Scholar
[9]	Maraj E N, Akbar N S, Zehra I, Butt A W, Ahmed Alghamdi H 2023 J. Magn. Magn. Mater. 576 170774 Google Scholar
[10]	Rafiq M, Shaheen S, Khan M I, Fadhl B M, Hassine S B H, ElDin S M 2023 Case Stud. Therm. Eng. 45 102990 Google Scholar
[11]	Alfwzan W, Riaz A, Alammari M, Hejazi H, Tag El-Din E M 2023 Front. Phys. 11 1121849 Google Scholar
[12]	Wang S, Li N, Zhao M, Azese M N 2018 Z. Naturforsch. A 73 825 Google Scholar
[13]	Sheikholeslami M, Chamkha A J 2016 Numer. Heat Tr. A-Appl. 69 781 Google Scholar
[14]	Tripathi D, Bhushan S, Bég O A 2016 Colloids Surf. A 506 32 Google Scholar
[15]	Shapiro A H, Jaffrin M Y, Weinberg S L 1969 J. Fluid Mech. Dig. Arch. 37 799 Google Scholar
[16]	Shit G C, Mondal A, Sinha A, Kundu P K 2016 Physica A 462 1040 Google Scholar
[17]	Chakraborty S 2007 Anal. Chim. Acta 605 175 Google Scholar
[18]	Bhardwaj A, Kumar A, Bhandari D S, Tripathi D 2024 Sensor. Actuat. A-Phys. 366 114956
[19]	Verma L, Meher R 2023 Pramana 97 155 Google Scholar
[20]	Chen L, Abbas M A, Khudair W S, Sun B 2022 Symmetry 14 953 Google Scholar
[21]	Mahabub M, Ferdows M, Murtaza M G, Lorenzini G, Tzirtzilakis E E 2022 Mathem. Mod. Eng. Probl. 9 215 Google Scholar
[22]	Madkhali H A 2023 Case Stud. Therm. Eng. 41 102655 Google Scholar
[23]	Upreti H, Bartwal P, Pandey A K, Makinde O D 2023 Numer. Heat Transf. B: Fundam. 84 415 Google Scholar
[24]	Yashkun U, Zaimi K, Sufahani S F, Eid M R, Ferdows M 2023 Appl. Math J. Chin. Univ. 38 373 Google Scholar
[25]	Mishra N K, Sharma B K, Sharma P, Muhammad T, Pérez L M 2023 Sci. Rep. 13 1 Google Scholar
[26]	Cordwell A, Chapple A A, Chung S, Wells F S, Willmott G R 2023 Soft Matter 19 4676 Google Scholar
[27]	Ma N L, Sun Y, Jian Y 2023 Micromachines 14 1617 Google Scholar
[28]	Sarkar S, Ganguly S 2017 J. Non-Newtonian Fluid 250 18 Google Scholar
[29]	Kikuchi Y 1995 Microvasc. Res. 50 288 Google Scholar
[30]	Ranjit N K, Shit G C, Tripathi D 2018 Microvasc. Res. 117 74 Google Scholar
[31]	Sadeghi A, Kazemi Y, Saidi M H 2013 Nanosc. Microsc. Therm. 17 173 Google Scholar
[32]	Tripathi D 2013 Math. Comput. Model 57 1270 Google Scholar

施引文献

图 1 流体流动示意图

Fig. 1. Fluid flow diagram.

下载: 全尺寸图片幻灯片

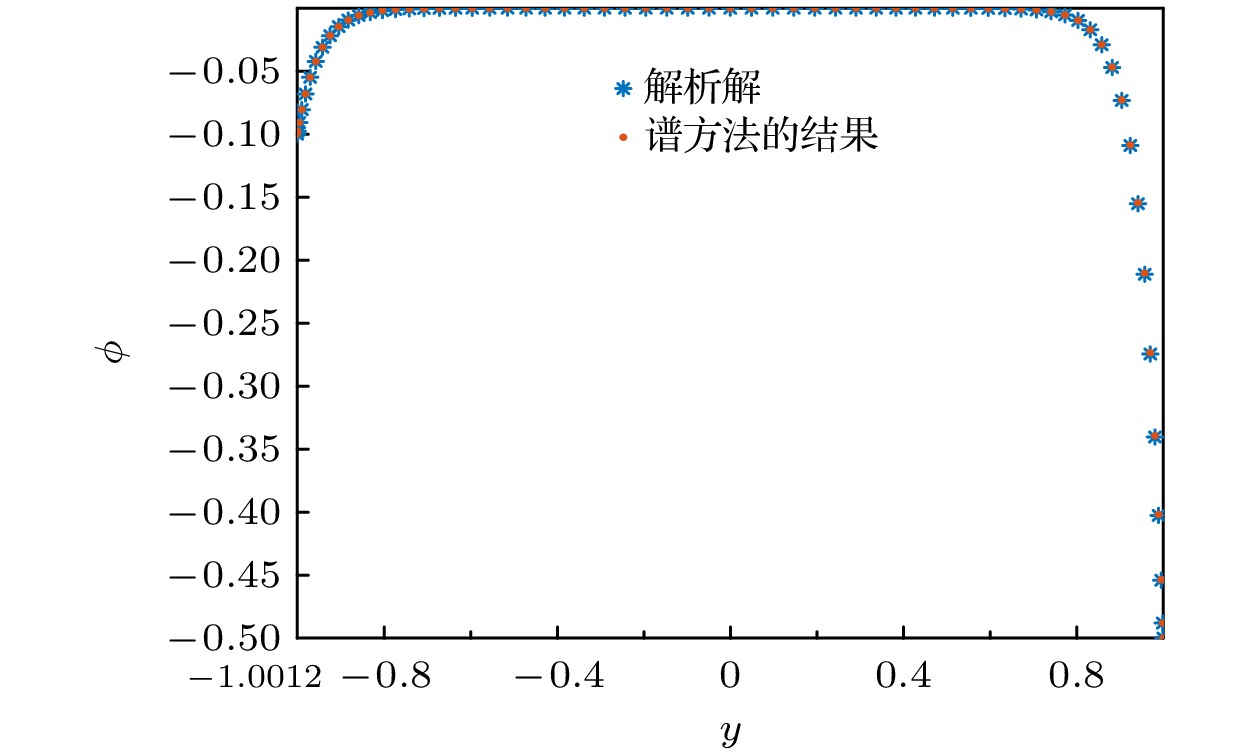
图 2 低Zeta势下P-B方程D-H近似解析解(蓝色)与切比雪夫谱方法(黄色)对比图, 其中$a = b = x = 0.5, \;\phi = $$ 0.05, \;d = 1.0, \;t = 0.0, \;F = 1.0$

Fig. 2. Comparison between approximate analytical solution of D-H for P-B equation (blue) and Chebyshev spectrum method (yellow) at low Zeta potential, $a = b = x = $$ 0.5, \;\phi = 0.05, \;d = 1.0, \;t = 0.0, \;F = 1.0$.

下载: 全尺寸图片幻灯片

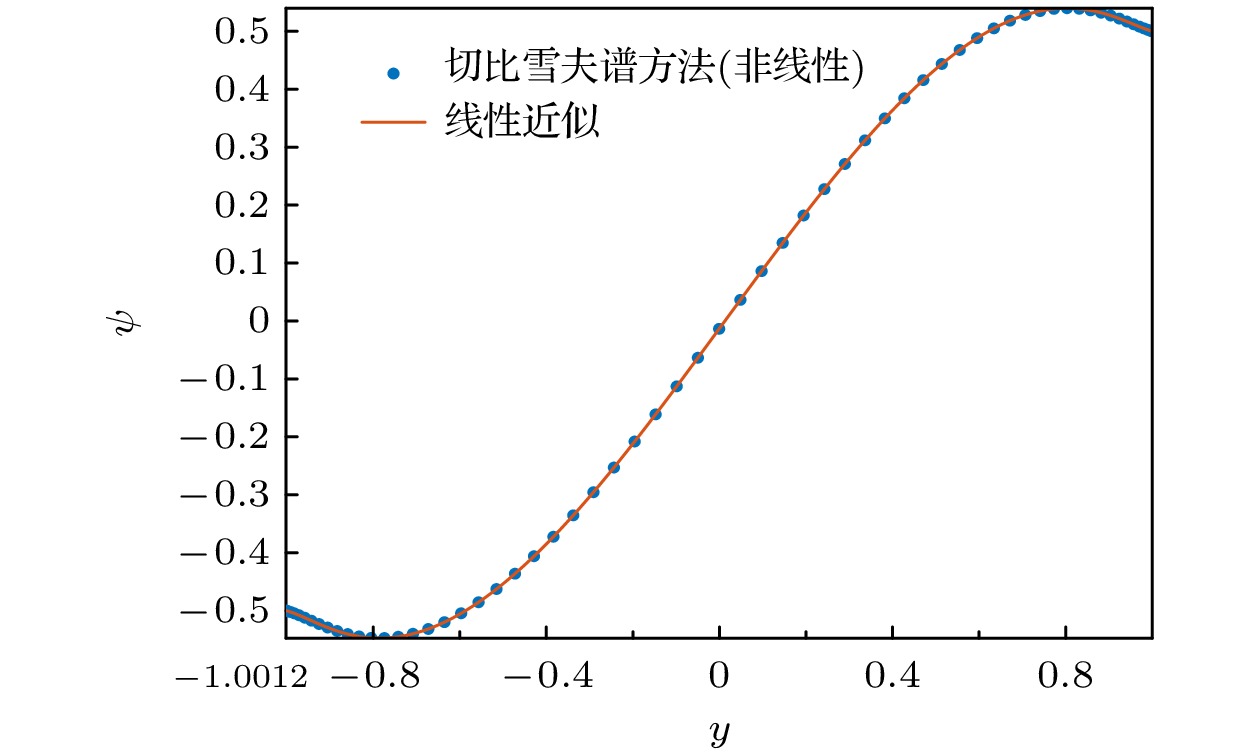
图 3 低Zeta势下流动方程D-H线性近似结果(实线)与切比雪夫谱方法(圆点)对比图, 其中$a = b = x = 0.5,\; \phi = $$ 0.05, \;d = 1.0, \;t = 0.0, \;F = 1.0$

Fig. 3. Comparison between the D-H approximate analytical solution (solid line) of nonlinear flow equation and Chebyshev-spectral method (dot) under the low Zeta potential, $a = b = x = 0.5, \, \;\phi = 0.05, \;d = 1.0, \;t = 0.0, \;F = 1.0$.

下载: 全尺寸图片幻灯片

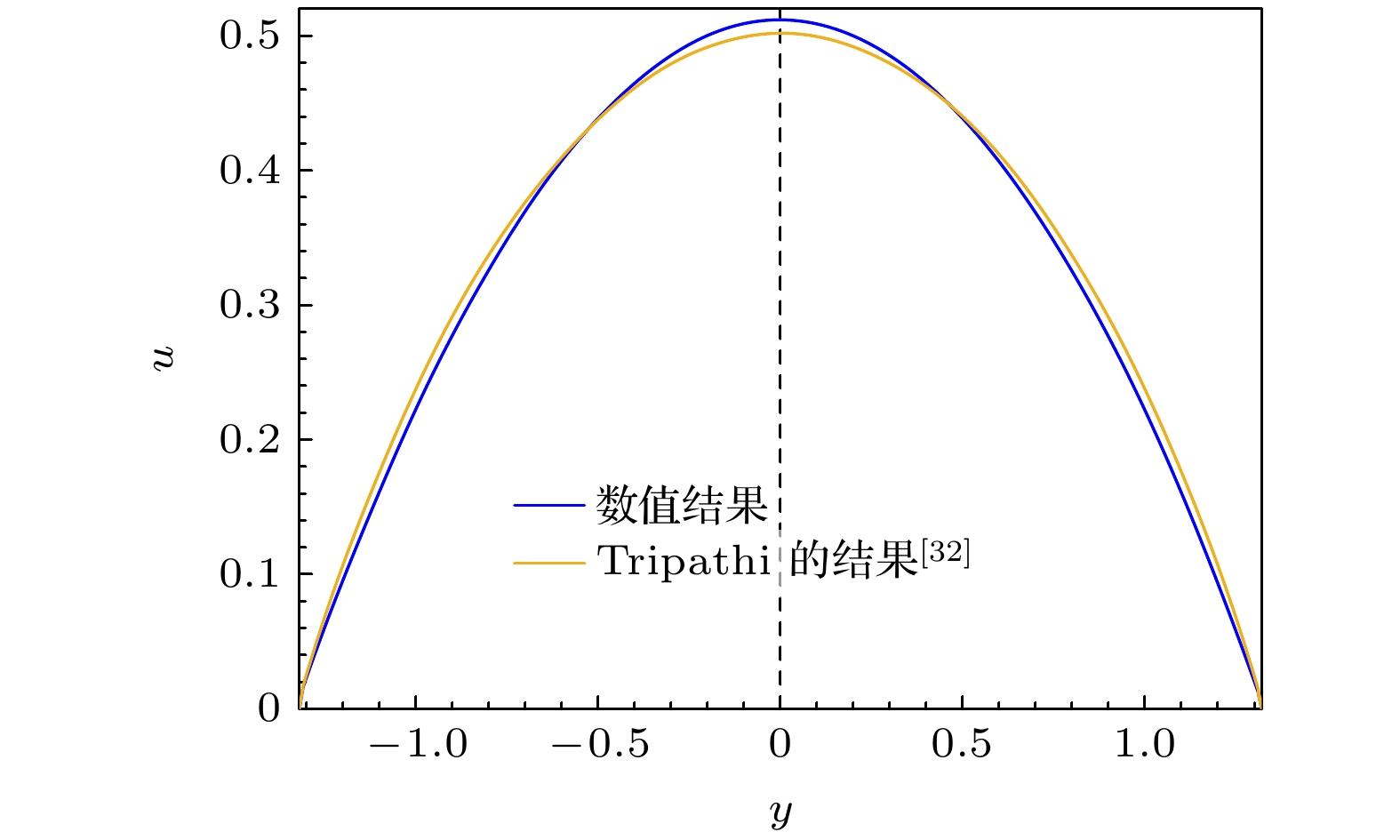
图 4 本文轴向速度分布与Tripathi^[32]研究结果的比较, 其中${\zeta _1} \to 0, \;{\zeta _2} \to 0, \;H \to 0, \;{\beta _1} = {\beta _2} = \beta = 0$

Fig. 4. Comparison between axial velocity distributions obtained by this study and Tripathi^[32], where ${\zeta _1} \to 0,\; {\zeta _2} \to 0, $$ H \to 0,\; {\beta _1} = {\beta _2} = \beta = 0$.

下载: 全尺寸图片幻灯片

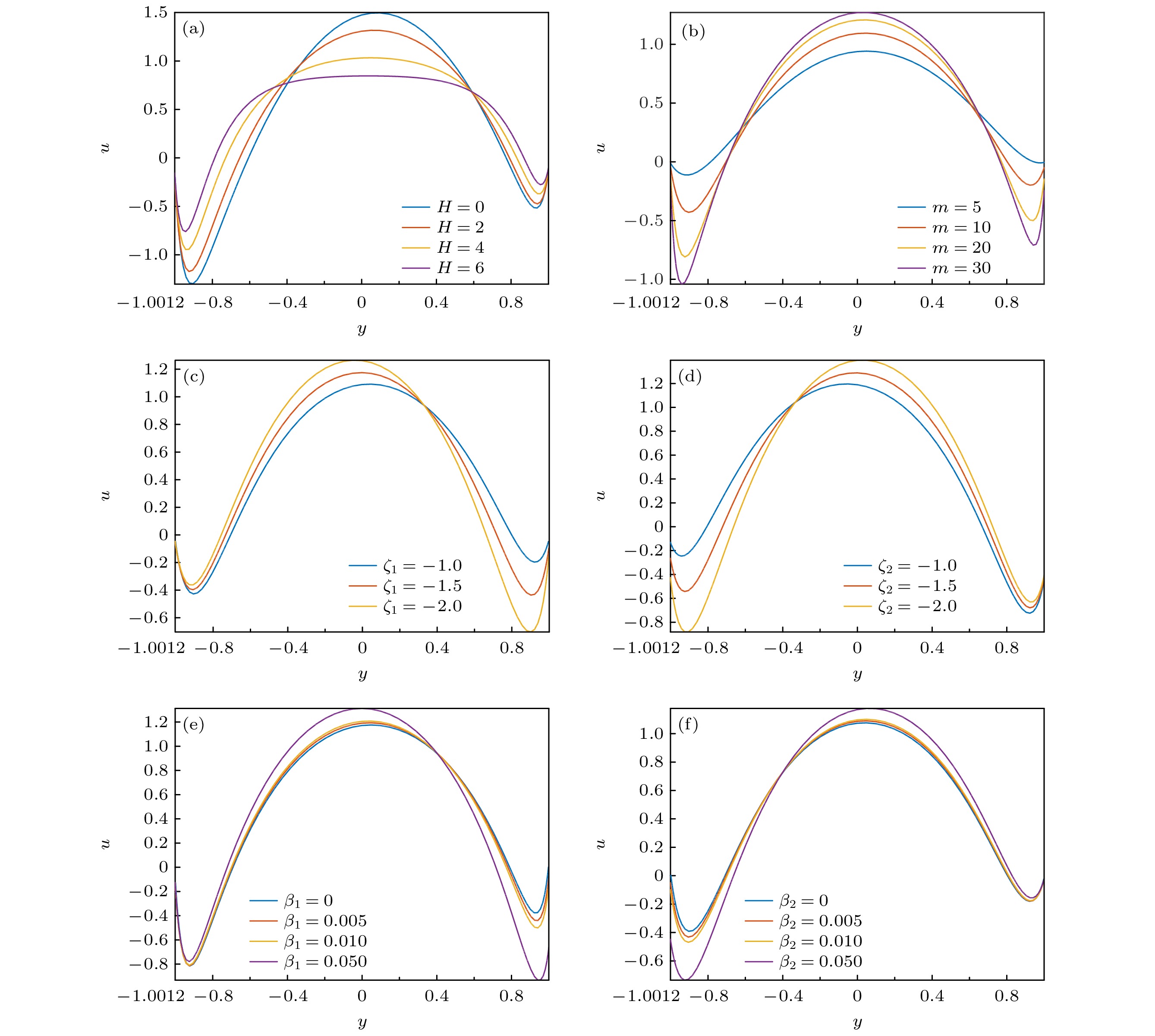
图 5 不同参数值对轴向速度的影响($a = b = x = 0.5, \;\phi = 0.05, \; d = 1.0, \;F = 1.0 $)　(a) H; (b) m; (c) ${\zeta _1}$; (d) ${\zeta _2}$; (e) ${\beta _1}$; (f) ${\beta _2}$

Fig. 5. Effects of different parameter values on axial velocity: (a) H; (b) m; (c) ${\zeta _1}$; (d) ${\zeta _2}$; (e) ${\beta _1}$; (f) ${\beta _2}$. $a = b = x = 0.5, $$ \;\phi = 0.05, \;\; d = 1.0, \;F = 1.0$.

下载: 全尺寸图片幻灯片

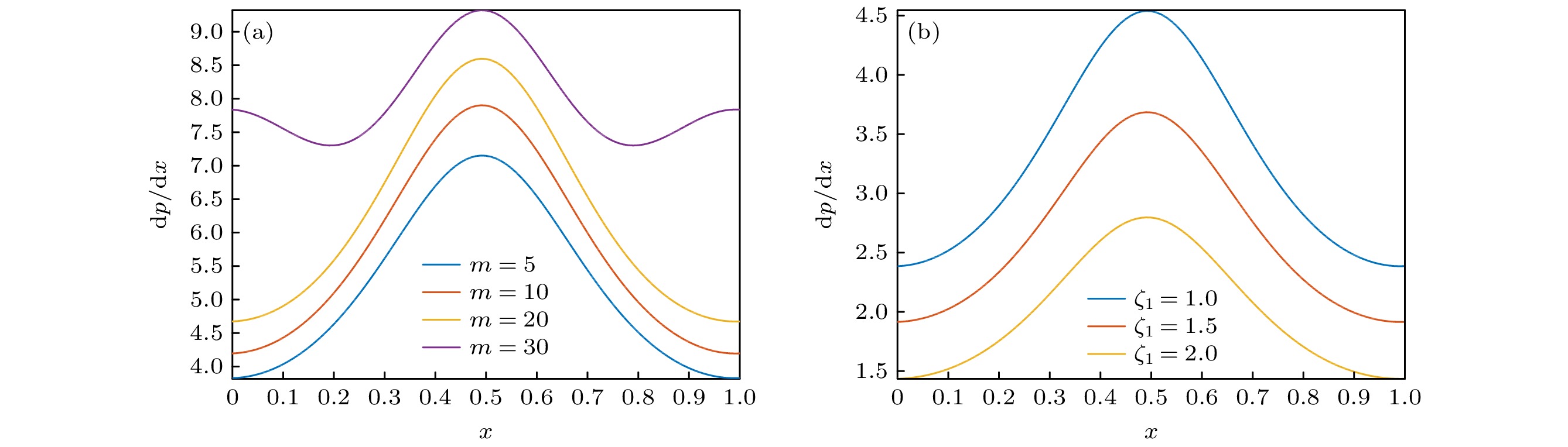
图 6 电渗参数$m$和Zeta电位对压力梯度的影响($ a = b = 0.5, {\text{ }}d = 1.0, {\text{ }}{\zeta _2} = - 1.5, {\text{ }}H = 2.0, \;{\beta _1} = 0.01, \;{\beta _2} = 0.005, {\text{ }}\phi {\text{ = 0}}{.05} $)　(a) m; (b) ${\zeta _1}$

Fig. 6. Effects of electroosmotic parameters $m$ and potential Zeta on pressure gradients: (a) m; (b) ${\zeta _1}$. $ a = b = 0.5, {\text{ }}d = 1.0, $$ {\text{ }}{\zeta _2} = - 1.5, {\text{ }}H = 2.0, \;{\beta _1} = 0.01, \;{\beta _2} = 0.005, {\text{ }}\phi {\text{ = 0}}{.05} $.

下载: 全尺寸图片幻灯片

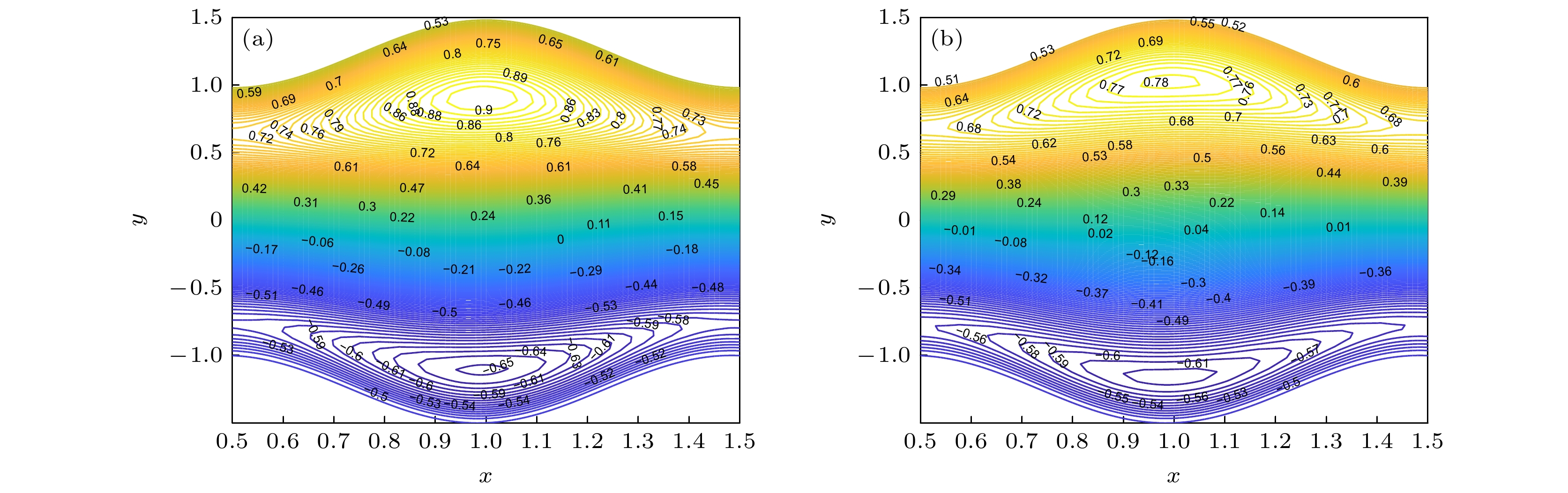
图 7 哈特曼数对流线分布$\psi $的影响($ \zeta_1 = - 1.5, ~\zeta_2 = -1, ~ m = 20, \beta_1 = 0.01, ~ \beta_2 = 0.005, ~\phi = 0.05 $)　(a) $H \to 0\;$; (b) H = 2

Fig. 7. Effect of Hammett number on streamline distribution $\psi $: (a) $H \to 0\;$; (b) H = 2. $ {\text{ }}{\zeta _1} = - 1.5{\text{ }}, \;{\zeta _2} = - 1{\text{ }}, {\text{ }}m = 20, {\beta _1} = $$ 0.01{\text{ }}, \;{\beta _2} = 0.005{\text{ }}, \;\phi = 0.05 $.

下载: 全尺寸图片幻灯片

图 8 电渗参数对流线分布$\psi $的影响(${\zeta _1} = - 1.5, \;{\zeta _2} = $$ - 1, \; H = 2.0, \; {\beta _1} = 0.01, \;{\beta _2} = 0.005,\; \phi = 0.05$)　(a) m = 5; (b) m = 20

Fig. 8. Effects of electroosmotic parameters on the streamline distribution $\psi $: (a) m = 5; (b) m = 20.${\zeta _1} = - 1.5, \;{\zeta _2} = $$ - 1, \;H = 2.0, \;{\beta _1} = 0.01, \;{\beta _2} = 0.005, \;\phi = 0.05$

下载: 全尺寸图片幻灯片

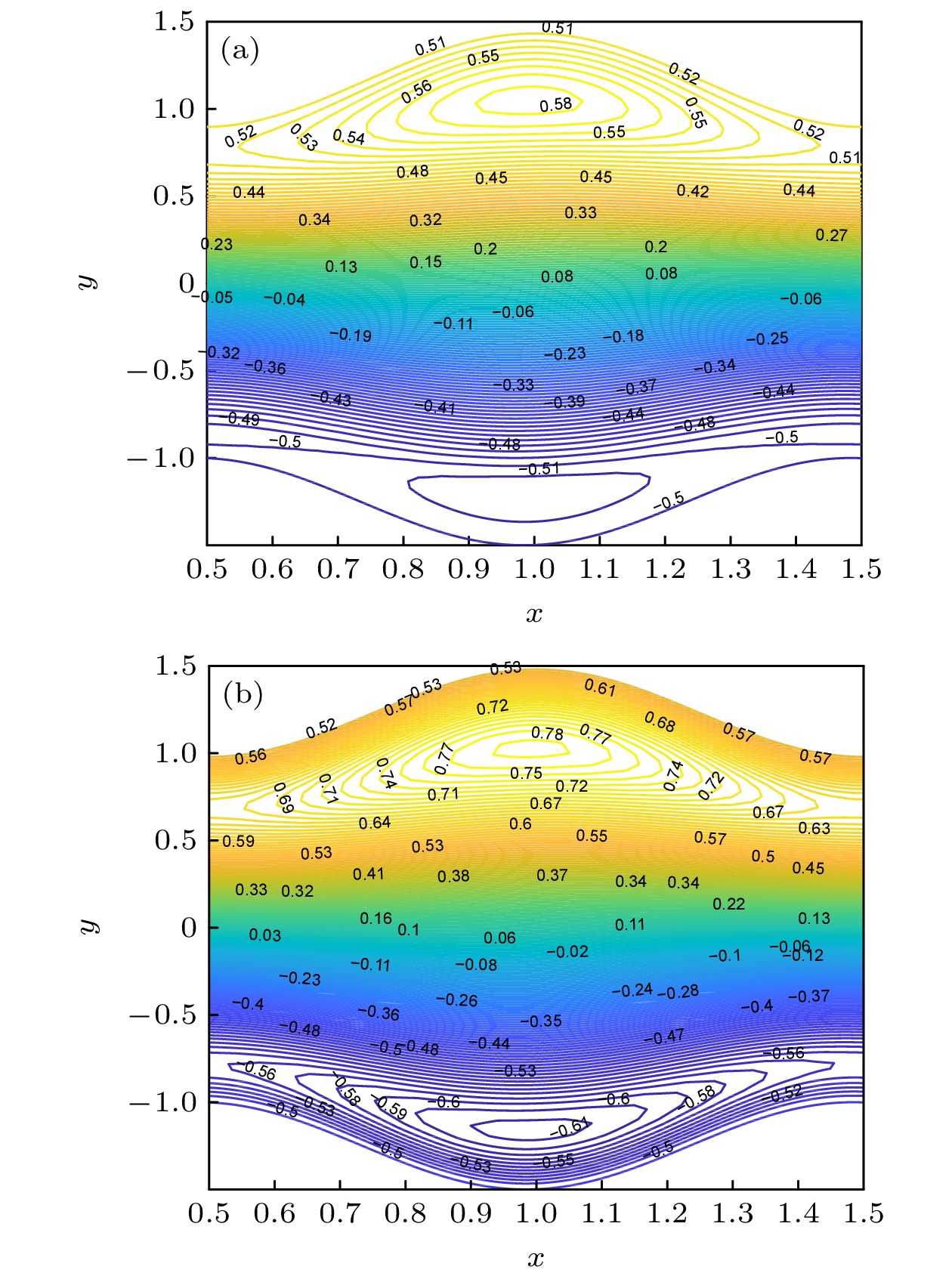
图 9 Zeta电位对流线分布$\psi $的影响($m = 20, \;H = 2.0, \; $$ {\beta _1} = 0.01, \;{\beta _2} = 0.005, \;\phi = 0.05$)　(a) ${\zeta _1} = - 1$; (b) ${\zeta _1} = $$ - 1.5$; (c) ${\zeta _1} = - 2$

Fig. 9. Effect of Zeta potential on streamline distribution $\psi $: (a) ${\zeta _1} = - 1$; (b) ${\zeta _1} = - 1.5$; (c) ${\zeta _1} = - 2$. $m = 20, \;H = $$ 2.0, \;{\beta _1} = 0.01, \;{\beta _2} = 0.005, \;\phi = 0.05$.

下载: 全尺寸图片幻灯片

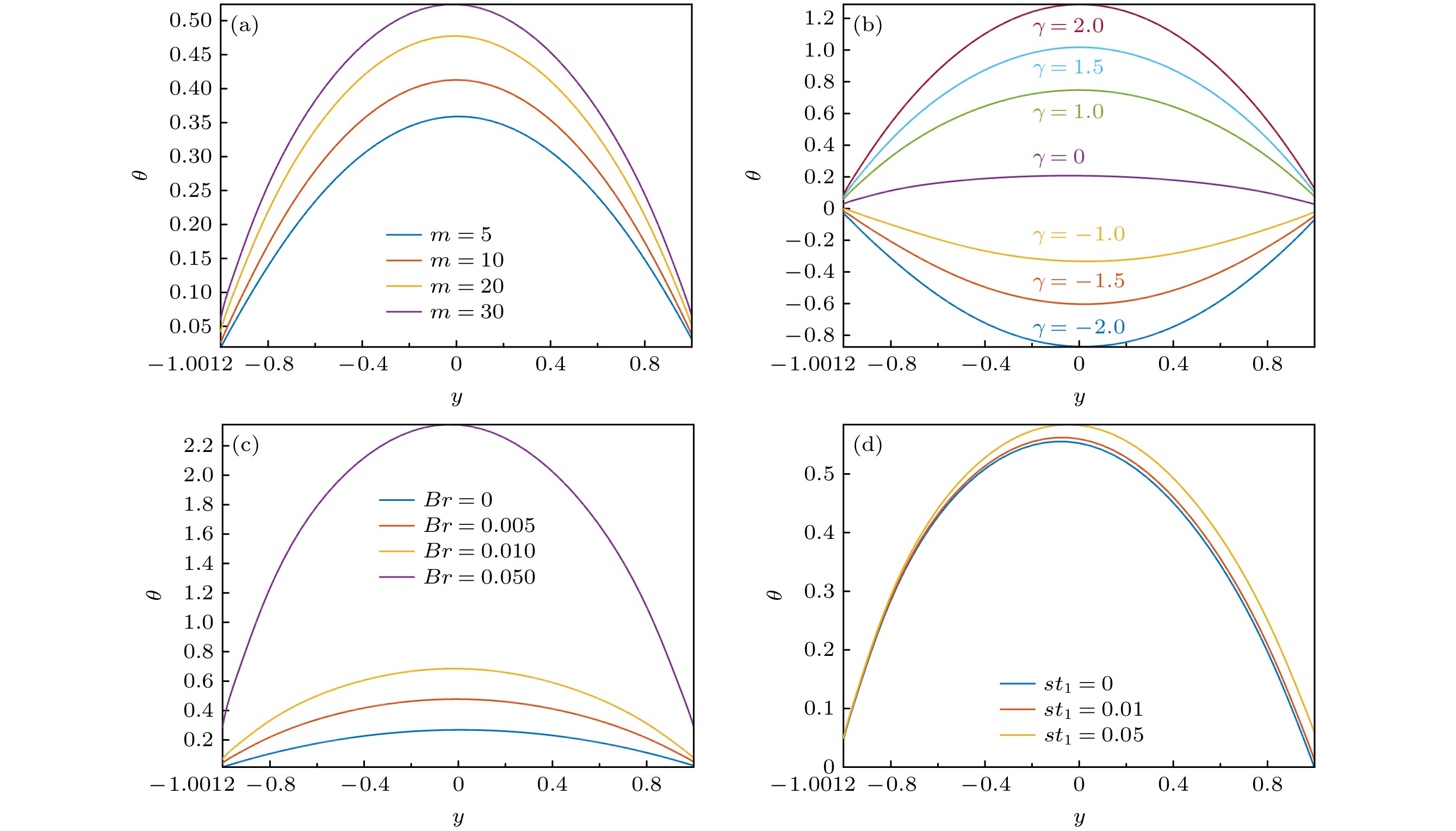
图 10 不同参数对温度分布$\theta $的影响($H = 2.0, \;{\beta _1} = 0.01, \;{\beta _2} = 0.005, \;\phi = s{t_2} = 0.05, \;{\zeta _1} = - 1.5, \;{\zeta _2} = - 1, x = 0.5, \;m = 20$)　(a) m; (b) γ; (c) Br ; (d) st₁

Fig. 10. Influences of different parameters on temperature distribution $\theta $: (a) m; (b) γ; (c) Br ; (d) st₁. $H = 2.0, \;{\beta _1} = 0.01, $$ \;{\beta _2} = 0.005, \;\phi = s{t_2} = 0.05, \;{\zeta _1} = - 1.5, \;{\zeta _2} = - 1, x = 0.5, \;m = 20$.

下载: 全尺寸图片幻灯片

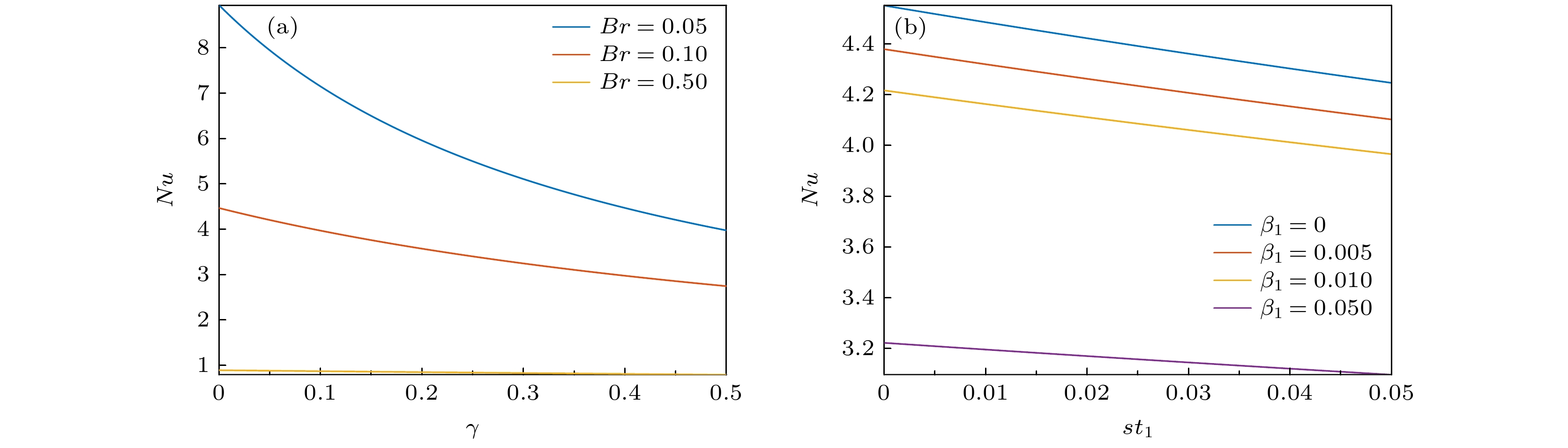
图 11 布林克曼数$Br$和速度滑移参数${\beta _1}$对努塞尔数$Nu$的影响($H = 2.0, \;{\beta _1} = 0.01, \;{\beta _2} = 0.005, \;\phi = s{t_2} = 0.05, \;{\zeta _1} = $$ - 1.5, \;{\zeta _2} = - 1, \;x = 0.5, \;m = 20$)　(a) Br ; (b) β₁

Fig. 11. Influences of Brinkman number Br and slip parameter β₁ on Nussle number: (a) Br ; (b) β₁. $H = 2.0, \;{\beta _1} = 0.01, $$ \;{\beta _2} = 0.005, \;\phi = s{t_2} = 0.05, \;{\zeta _1} = - 1.5, \;{\zeta _2} = - 1, \;x = 0.5, \;m = 20$.

下载: 全尺寸图片幻灯片

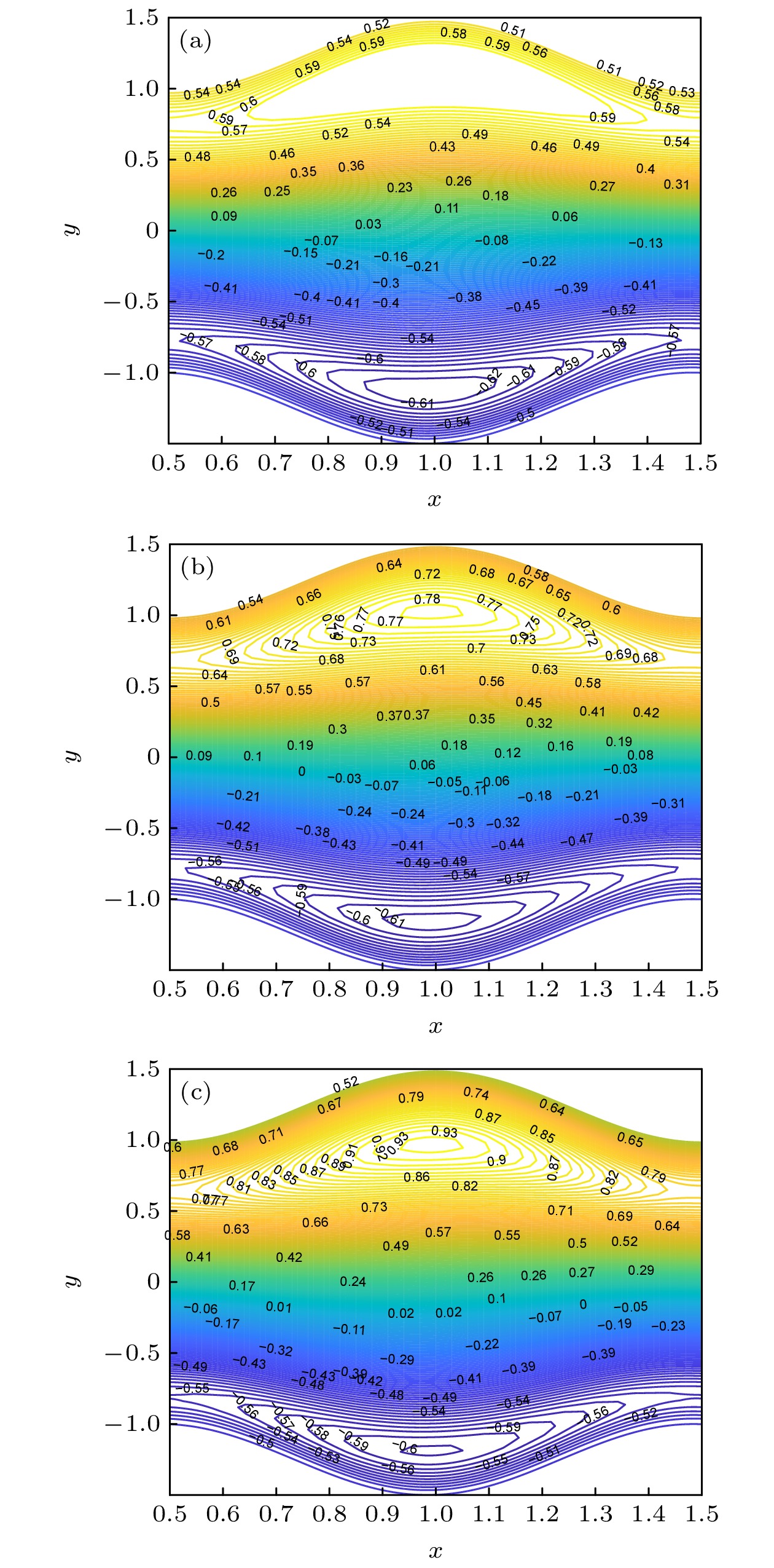
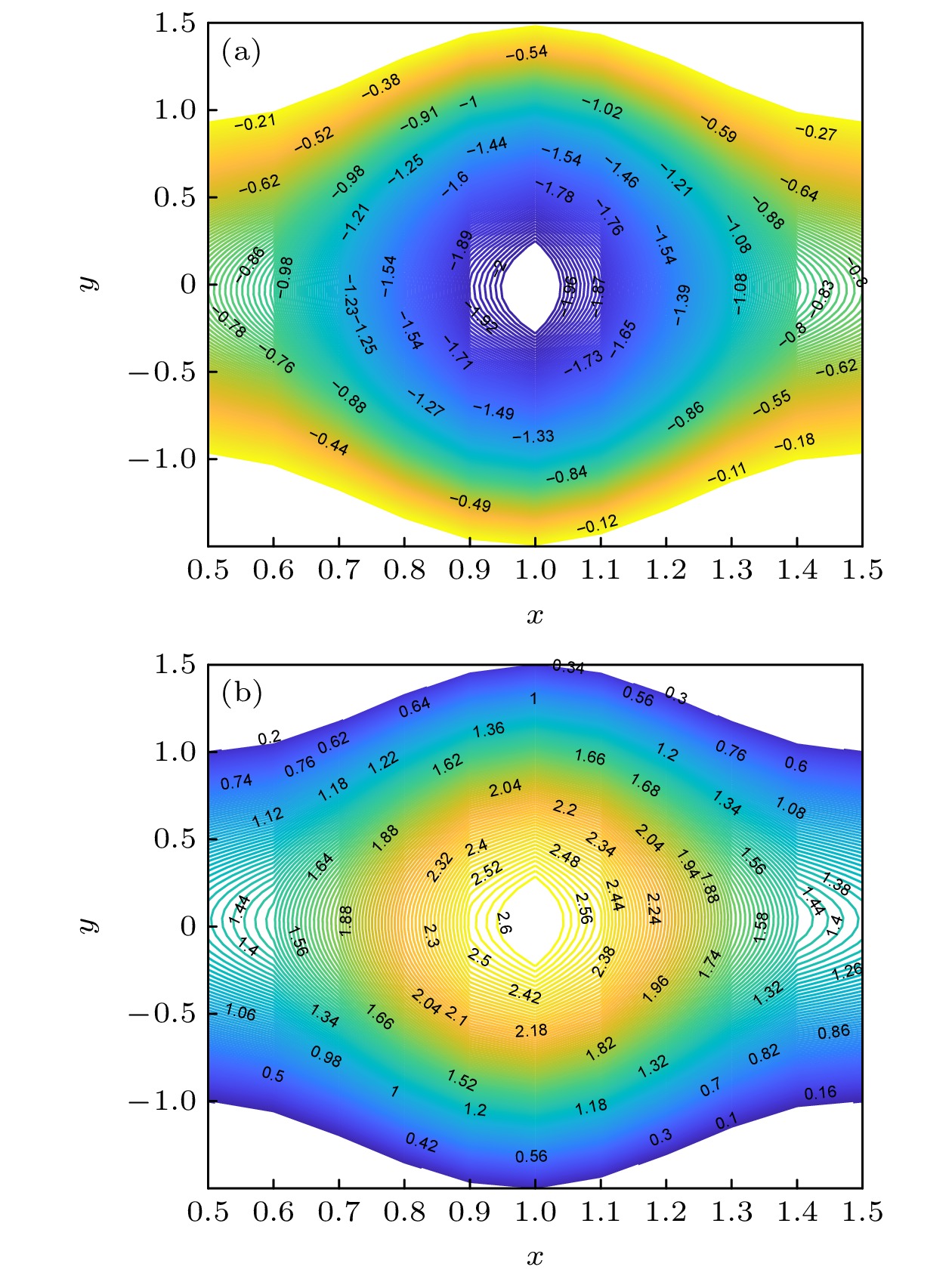
图 12 不同焦耳加热参数$\gamma $的等温图($H = 2.0, {\beta _1} = 0.01,$ ${\beta _2} = 0.005, $ $\phi = s{t_2} = 0.05, $ ${\zeta _1} = - 1.5, $ $ {\zeta _2} = - 1, $ $\;x = 0.5, $$ m = 20$)　(a) $\gamma = - 2$; (b) $\gamma = 2$

Fig. 12. Isothermal diagram of different joule heating parameters $\gamma $: (a) $\gamma = - 2$; (b) $\gamma = 2$. $H = 2.0, \;{\beta _1} = 0.01, $ $ {\beta _2} = 0.005,$ $ \phi = s{t_2} = 0.05, $ $ {\zeta _1} = - 1.5, $ ${\zeta _2} = - 1, $ $x = $$ 0.5, $ $ m = 20 $.

下载: 全尺寸图片幻灯片

map

[1]	Maiti S, Pandey S K, Misra J C 2022 J. Eng. Math. 135 8 Google Scholar
[2]	Jaiswal S, Yadav P K 2022 Microfluid Nanofluid 26 100 Google Scholar
[3]	Patel H R, Patel S D, Darji R 2022 Int. J. Thermofluids 16 100232 Google Scholar
[4]	Sherief H H, Faltas M S, El-Sapa S 2019 Eur. J. Mech. B Fluids 77 211 Google Scholar
[5]	Chandra S, Kumar Pandey S 2018 J. Phys. Conf. Ser. 1141 012092 Google Scholar
[6]	Yasmin H, Nisar Z 2023 Mathematics 11 2673 Google Scholar
[7]	Mishra N K, Sharma B K, Sharma P, Muhammad T, Pérez L M 2023 Sci. Rep. 13 14483 Google Scholar
[8]	Guedri K, Lashin M M A, Abbasi A, Khan S U, Farooq W, Khan M I, Galal A M 2023 Chin. J. Phys. 83 650 Google Scholar
[9]	Maraj E N, Akbar N S, Zehra I, Butt A W, Ahmed Alghamdi H 2023 J. Magn. Magn. Mater. 576 170774 Google Scholar
[10]	Rafiq M, Shaheen S, Khan M I, Fadhl B M, Hassine S B H, ElDin S M 2023 Case Stud. Therm. Eng. 45 102990 Google Scholar
[11]	Alfwzan W, Riaz A, Alammari M, Hejazi H, Tag El-Din E M 2023 Front. Phys. 11 1121849 Google Scholar
[12]	Wang S, Li N, Zhao M, Azese M N 2018 Z. Naturforsch. A 73 825 Google Scholar
[13]	Sheikholeslami M, Chamkha A J 2016 Numer. Heat Tr. A-Appl. 69 781 Google Scholar
[14]	Tripathi D, Bhushan S, Bég O A 2016 Colloids Surf. A 506 32 Google Scholar
[15]	Shapiro A H, Jaffrin M Y, Weinberg S L 1969 J. Fluid Mech. Dig. Arch. 37 799 Google Scholar
[16]	Shit G C, Mondal A, Sinha A, Kundu P K 2016 Physica A 462 1040 Google Scholar
[17]	Chakraborty S 2007 Anal. Chim. Acta 605 175 Google Scholar
[18]	Bhardwaj A, Kumar A, Bhandari D S, Tripathi D 2024 Sensor. Actuat. A-Phys. 366 114956
[19]	Verma L, Meher R 2023 Pramana 97 155 Google Scholar
[20]	Chen L, Abbas M A, Khudair W S, Sun B 2022 Symmetry 14 953 Google Scholar
[21]	Mahabub M, Ferdows M, Murtaza M G, Lorenzini G, Tzirtzilakis E E 2022 Mathem. Mod. Eng. Probl. 9 215 Google Scholar
[22]	Madkhali H A 2023 Case Stud. Therm. Eng. 41 102655 Google Scholar
[23]	Upreti H, Bartwal P, Pandey A K, Makinde O D 2023 Numer. Heat Transf. B: Fundam. 84 415 Google Scholar
[24]	Yashkun U, Zaimi K, Sufahani S F, Eid M R, Ferdows M 2023 Appl. Math J. Chin. Univ. 38 373 Google Scholar
[25]	Mishra N K, Sharma B K, Sharma P, Muhammad T, Pérez L M 2023 Sci. Rep. 13 1 Google Scholar
[26]	Cordwell A, Chapple A A, Chung S, Wells F S, Willmott G R 2023 Soft Matter 19 4676 Google Scholar
[27]	Ma N L, Sun Y, Jian Y 2023 Micromachines 14 1617 Google Scholar
[28]	Sarkar S, Ganguly S 2017 J. Non-Newtonian Fluid 250 18 Google Scholar
[29]	Kikuchi Y 1995 Microvasc. Res. 50 288 Google Scholar
[30]	Ranjit N K, Shit G C, Tripathi D 2018 Microvasc. Res. 117 74 Google Scholar
[31]	Sadeghi A, Kazemi Y, Saidi M H 2013 Nanosc. Microsc. Therm. 17 173 Google Scholar
[32]	Tripathi D 2013 Math. Comput. Model 57 1270 Google Scholar

[1]	程亮元, 徐进良. 流动方向对超临界二氧化碳流动传热特性的影响. , 2024, 73(2): 024401. doi: 10.7498/aps.73.20231142
[2]	于博文, 何孝天, 徐进良. 超临界CO₂池式传热流固耦合传热特性数值模拟. , 2024, 73(10): 104401. doi: 10.7498/aps.73.20231953
[3]	于欣如, 崔继峰, 陈小刚, 慕江勇, 乔煜然. 平行板微通道中一类不可压缩微极性流体在高Zeta势下的时间周期电渗流. , 2024, 73(16): 164701. doi: 10.7498/aps.73.20240591
[4]	胡剑, 张森, 娄钦. 电场和加热器特性对饱和池沸腾传热影响的介观数值方法研究. , 2023, 72(17): 176401. doi: 10.7498/aps.72.20230341
[5]	张天鸽, 任美蓉, 崔继峰, 陈小刚, 王怡丹. 变截面微管道中高zeta势下幂律流体的旋转电渗滑移流动. , 2022, 71(13): 134701. doi: 10.7498/aps.71.20212327
[6]	刘联胜, 刘轩臣, 贾文琪, 田亮, 杨华, 段润泽. 小液滴撞击壁面传热特性数值分析. , 2021, 70(7): 074402. doi: 10.7498/aps.70.20201354
[7]	方芳, 鲍麟, 童秉纲. 基于斜驻点模型的剪切层撞击壁面流动及传热特性. , 2020, 69(21): 214401. doi: 10.7498/aps.69.20201000
[8]	方明卫, 何建超, 包芸. 湍流热对流温度剖面双参数拟合及其变化特性. , 2020, 69(17): 174701. doi: 10.7498/aps.69.20200073
[9]	包芸, 何建超, 高振源. 二维湍流热对流羽流运动路径对传热特性的影响. , 2019, 68(16): 164701. doi: 10.7498/aps.68.20190323
[10]	段娟, 陈耀钦, 朱庆勇. 微扩张管道内幂律流体非定常电渗流动. , 2016, 65(3): 034702. doi: 10.7498/aps.65.034702
[11]	王小虎, 易仕和, 付佳, 陆小革, 何霖. 二维高超声速后台阶表面传热特性实验研究. , 2015, 64(5): 054706. doi: 10.7498/aps.64.054706
[12]	王刚, 于前锋, 王文, 宋钢, 吴宜灿. 氘氚聚变中子发生器旋转氚靶传热特性研究. , 2015, 64(10): 102901. doi: 10.7498/aps.64.102901
[13]	郭亚丽, 魏兰, 沈胜强, 陈桂影. 双液滴撞击平面液膜的流动与传热特性. , 2014, 63(9): 094702. doi: 10.7498/aps.63.094702
[14]	刘全生, 杨联贵, 苏洁. 微平行管道内Jeffrey流体的非定常电渗流动. , 2013, 62(14): 144702. doi: 10.7498/aps.62.144702
[15]	长龙, 菅永军. 平行板微管道间Maxwell流体的高Zeta势周期电渗流动. , 2012, 61(12): 124702. doi: 10.7498/aps.61.124702
[16]	姜洪源, 李姗姗, 侯珍秀, 任玉坤, 孙永军. 非对称电极表面微观形貌对交流电渗流速的影响. , 2011, 60(2): 020702. doi: 10.7498/aps.60.020702
[17]	姜洪源, 任玉坤, 陶冶. 微系统中转矩及电渗流作用下的微粒子电动旋转操控. , 2011, 60(1): 010701. doi: 10.7498/aps.60.010701
[18]	刘会师, 忻向军, 尹霄丽, 余重秀, 张琦. 切比雪夫光混沌发生器的优化. , 2009, 58(4): 2231-2234. doi: 10.7498/aps.58.2231
[19]	王立, 张小安, 杨治虎, 陈熙萌, 张红强, 崔莹, 邵剑雄, 徐徐. 高电荷态离子入射Al表面库仑势对靶原子特征谱线强度的影响. , 2008, 57(1): 137-142. doi: 10.7498/aps.57.137
[20]	李鹏程, 周效信, 董晨钟, 赵松峰. 强激光场中长程势与短程势原子产生高次谐波与电离特性研究. , 2004, 53(3): 750-755. doi: 10.7498/aps.53.750

计量

文章访问数: 1627
PDF下载量: 40
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

微通道中一类生物流体在高Zeta势下的电渗流及传热特性