搜索

x
中国物理学会期刊
Chinese Physics Letters Chinese Physics B 物理 中国物理学会期刊网
188app金宝搏

留言板

尊敬的读者、作者、审稿人, 关于本刊的投稿、审稿、编辑和出版的任何问题, 您可以本页添加留言。我们将尽快给您答复。谢谢您的支持!

姓名
邮箱
手机号码
标题
留言内容
验证码

变截面微管道中高zeta势下幂律流体的旋转电渗滑移流动

张天鸽 ,  任美蓉 ,  崔继峰 ,  陈小刚 ,  王怡丹

downloadPDF
引用本文:
shu
Citation:
shu
下一篇 上一篇

变截面微管道中高zeta势下幂律流体的旋转电渗滑移流动

张天鸽, 任美蓉, 崔继峰, 陈小刚, 王怡丹
, 2022, 71(13): 134701.
doi: 10.7498/aps.71.20212327

Rotational electroosmotic slip flow of power-law fluid at high zeta potential in variable-section microchannel

Zhang Tian-Ge, Ren Mei-Rong, Cui Ji-Feng, Chen Xiao-Gang, Wang Yi-Dan
Acta Phys. Sin., 2022, 71(13): 134701.
doi: 10.7498/aps.71.20212327
PDF
HTML
导出引用

  • 摘要

    本文研究高zeta势下具有Navier滑移边界条件的幂律流体, 在变截面微管道中的垂向磁场作用下的旋转电渗流动. 在不使用Debye–Hückel线性近似条件时, 利用有限差分法数值计算外加磁场的旋转电渗流的电势分布和速度分布. 当行为指数$n = 1$时得到的流体为牛顿流体, 将本文的分析结果与Debye–Hückel 线性近似所得解析近似解作比较, 证明本文数值方法的可行性. 除此之外, 还详细讨论行为指数n、哈特曼数Ha、旋转角速度$\varOmega$、电动宽度K及滑移参数$\beta $对速度分布的影响, 得到当哈特曼数Ha >1时, 速度随着哈特曼数 Ha 的增加而减小; 但当哈特曼数Ha <1时, x方向速度 u 的大小随着 Ha 的增加而增加.

    Abstract

    In this paper we study the rotating electroosmotic flow of a power-law fluid with Navier slip boundary conditions under high zeta potential subjected to the action of a vertical magnetic field in a variable cross-section microchannel. Without using the Debye–Hückel linear approximation, the finite difference method is used to numerically calculate the potential distribution and velocity distribution of the rotating electroosmotic flow subjected to an external magnetic field. When the behavior index $n = 1$, the fluid obtained is a Newtonian fluid. The analysis results in this paper are compared with the analytical approximate solutions obtained in the Debye–Hückel linear approximation to prove the feasibility of the numerical method in this paper. In addition, the influence of behavior index n, Hartmann number Ha, rotation angular velocity $\Omega $, electric width K and slip parameters $\beta $ on the velocity distribution are discussed in detail. It is obtained that when the Hartmann number Ha > 1, the velocity decreases with the increase of the Hartmann number Ha; but when the Hartmann number Ha < 1, the magnitude of the x-direction velocity u increases with the augment of Ha.

    作者及机构信息

    • 内蒙古工业大学理学院, 呼和浩特　010051

      • 通信作者: 陈小刚, xiaogang_chen@imut.edu.cn
    • 基金项目: 国家自然科学基金(批准号: 12062018, 12172333)、内蒙古自治区高等学校青年科技英才支持计划资助项目(批准号: NJYT22075)和内蒙古自然科学基金(批准号: 2020MS01015)资助的课题.

    Authors and contacts

    • College of Science, Inner Mongolia University of Technology, Hohhot 010051, China

      • Corresponding author: Chen Xiao-Gang, xiaogang_chen@imut.edu.cn
    • Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 12062018, 12172333), the Young Talents of Science and Technology in Universities of Inner Mongolia Autonomous Region, China (Grant No. NJYT22075), and the Natural Science Foundation of Inner Mongolia, China (Grant No. 2020MS01015).

    参考文献

    [1]

    Stone H A, Stroock A D, Ajdari A 2004 Annu. Rev. Fluid Mech. 36 381Google Scholar

    [2]

    Patel M, Kruthiventi S S H, Kaushik P 2020 Colloids Surf. B 193 111058Google Scholar

    [3]

    Srinivas, Bhadri 2016 Colloids Surf. A 492 144Google Scholar

    [4]

    Nekoubin N 2018 J. Non-Newtonian Fluid Mech. 260 54Google Scholar

    [5]

    Baños R D, Arcos J C, Bautista O, Méndez F, Merchán-Cruz E A 2021 J. Braz. Soc. Mech. Sci. 43 1Google Scholar

    [6]

    Baños R, Arcos J, Bautista O, Méndez F 2020 Defect Diffus. Forum 399 92Google Scholar

    [7]

    姜玉婷, 齐海涛 2015 64 174702Google Scholar

    Jiang Y T, Qi H T 2015 Acta Phys. Sin. 64 174702Google Scholar

    [8]

    Ajdari A 2002 Phys. Rev. E 65 16301Google Scholar

    [9]

    Chang C C, Wang C Y 2011 Phys. Rev. E 84 056320Google Scholar

    [10]

    Song J, Wang S W, Zhao M L, Li N 2020 Z. Naturforsch. A: Phys. Sci. 75 649Google Scholar

    [11]

    Shit G C, Mondal A, Sinha A, Kundu P K 2016 Colloids Surf. A 489 249Google Scholar

    [12]

    刘全生, 杨联贵, 苏洁 2013 62 144702Google Scholar

    Liu Q S, Yang L G, Su J 2013 Acta Phys. Sin. 62 144702Google Scholar

    [13]

    段娟, 陈耀钦, 朱庆勇 2016 65 034702Google Scholar

    Duan J, Chen Y Q, Zhu Q Y 2016 Acta Phys. Sin. 65 034702Google Scholar

    [14]

    Weston M C, Gerner M D, Fritsch I 2010 Anal. Chem. 82 3411Google Scholar

    [15]

    Jian Y J, Chang L 2015 AIP Adv. 5 057121Google Scholar

    [16]

    Xie Z Y, Jian Y J 2017 Colloids Surf. A 529 334Google Scholar

    [17]

    Habib U, Hayat T, Ahmad S, Alhodaly M S 2021 Int. Commun. Heat Mass Transfer 122 105111Google Scholar

    [18]

    Sarkar S, Ganguly S 2017 J. Non-Newtonian Fluid Mech. 250 18Google Scholar

    [19]

    Yang C H, Jian Y J, Xie Z Y, Li F Q 2020 Micromachines 11 418Google Scholar

    [20]

    Xie Z Y, Jian Y J 2017 Energy 139 1080Google Scholar

    [21]

    Wang S W, Li N, Zhao M L, Azese M N 2018 Z. Naturforsch. A: Phys. Sci. 73 825Google Scholar

    [22]

    Xie Z Y, Jian Y J 2014 Colloids Surf. A 461 231Google Scholar

    [23]

    Bird R B, Armstrong R C, Hassager O, Curtiss C F, Middleman S 1978 Phys. Today 31 54Google Scholar

  • 图 1  变截面微通道中流体流动示意图

    Fig. 1.  Schematic view of the flow in a variable cross-section microchannel.

    下载: 全尺寸图片 幻灯片

    图 2  目前数值解与Chang和Wang[9]解析解的比较, 其中 $ \beta = 0, $$ K = 30, $$\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, {\text{ }}{\bar \psi _\omega } = 1{\text{ }}{\rm{V}}, {\text{ }}a = 0, {\text{ }}Ha = 0, {\text{ }}S = 0$

    Fig. 2.  Comparison of the current numerical solution with the analytical solution of Chang and Wang [9], $ \beta = 0, $$ K = 30, $$\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, {\text{ }}{\bar \psi _\omega } = 1{\text{ }}{\rm{V}}, {\text{ }}a = 0, {\text{ }}Ha = 0, {\text{ }}S = 0$

    下载: 全尺寸图片 幻灯片

    图 3  当无滑移边界条件时, 幂律流体行为指数n对外加磁场的旋转电渗流速度的影响, 其中$\beta = 0, {\text{ }}K = 10, {\text{ }}\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, $ ${\text{ }}{\bar \psi _\omega } = 5 \;{\rm{V}}, {\text{ }}a = 0.05, {\text{ }}Ha = 1, {\text{ }}S = $1

    Fig. 3.  When there is a no-slip boundary condition, the influence of power-law fluid behavior index n on rotating electroosmotic flow velocity with the external magnetic field, $\beta = 0, {\text{ }}K = 10, {\text{ }}\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, {\text{ }}{\bar \psi _\omega } = 5 \;{\rm{V}}, {\text{ }}a = 0.05, {\text{ }}Ha = 1, {\text{ }}S = 1$

    下载: 全尺寸图片 幻灯片

    图 4  当存在滑移边界条件时, 幂律流体行为指数n对外加磁场的旋转电渗流速度的影响, 其中$ \beta = 0.1, {\text{ }}K = 10, {\text{ }}\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, $ ${\text{ }}{\bar \psi _\omega } = 5{\text{ }}{\rm{V}}, {\text{ }}a = 0.05, {\text{ }}Ha = 1, {\text{ }}S =$1

    Fig. 4.  When there is a slip boundary condition, the influence of the power-law fluid behavior index n on the rotating electroosmotic flow velocity with an external magnetic field, $\beta = 0.1, {\text{ }}K = 10, {\text{ }}\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, {\text{ }}{\bar \psi _\omega } = 5{\text{ }}{\rm{V}}, {\text{ }}a = 0.05, {\text{ }}Ha = 1, {\text{ }}S = 1$

    下载: 全尺寸图片 幻灯片

    图 5  哈特曼数Ha对外加磁场的旋转电渗流速度的影响, 其中$ n = 0.8, {\text{ }}K = 10, $ $ {\text{ }}\beta = 0.1, {\text{ }}\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, $ ${\text{ }}{\bar \psi _\omega } = 5{\text{ }}{\rm{V}}, {\text{ }}a = $$ 0.05, {\text{ }}S =$1

    Fig. 5.  The influence of Hartmann number Ha on the velocity of rotating electroosmotic flow with external magnetic field, $ n = 0.8, {\text{ }}K = 10, $$ {\text{ }}\beta = 0.1, {\text{ }}\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, {\text{ }}{\bar \psi _\omega } = 5{\text{ }}{\rm{V}}, {\text{ }}a = 0.05, {\text{ }}S = 1 $

    下载: 全尺寸图片 幻灯片

    图 6  哈特曼数Ha对外加磁场的旋转电渗流速度的影响, 其中$ n = 1.2, {\text{ }}K = 10, $ ${\text{ }}\beta = 0.1, {\text{ }}\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, $ ${\bar \psi _\omega } = 5{\text{ }}{\rm{V}}, {\text{ }}a = $$ 0.05, {\text{ }}S =$1

    Fig. 6.  The influence of Hartmann number Ha on the velocity of rotating electroosmotic flow with external magnetic field, $ n = 1.2, {\text{ }}K = 10, $$ {\text{ }}\beta = 0.1, {\text{ }}\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, {\text{ }}{\bar \psi _\omega } = 5{\text{ }}{\rm{V}}, {\text{ }}a = 0.05, {\text{ }}S = 1 $

    下载: 全尺寸图片 幻灯片

    图 7  旋转角速度$\varOmega $对外加磁场的旋转电渗流速度的影响, 其中$ n = 0.8, $$ {\bar \psi _\omega } = {\text{5}}\;{\rm{V}}, $$ a = 0.05, {\text{ }}Ha = 1, {\text{ }}S = 1 $ (a)$K = 10, {\text{ }}\beta = $$ 0.1;$ (b) $ K = 10, {\text{ }}\beta = 0.1; $ (c) $ K = 10, {\text{ }}\beta = 0; $ (d)$ K = 20, {\text{ }}\beta = 0.1. $

    Fig. 7.  The influence of the rotational angular velocity $\varOmega $ on the rotational electroosmotic flow velocity of the external magnetic field, $ n = 0.8, $$ {\bar \psi _\omega } = {\text{5 }}V, $$ a = 0.05, {\text{ }}Ha = 1, {\text{ }}S = 1 $(a) $ K = 10, {\text{ }}\beta = 0.1; $ (b) $ K = 10, {\text{ }}\beta = 0.1; $ (c) $ K = 10, {\text{ }}\beta = 0; $ (d)$K = 20, $$ {\text{ }}\beta = 0.1$

    下载: 全尺寸图片 幻灯片

    图 8  旋转角速度$\varOmega $对外加磁场的旋转电渗流速度的影响, 其中$ n = 1.2, $$ {\bar \psi _\omega } = 5{\text{ }}{\rm{V}}, {\text{ }} $$ a = 0.05, {\text{ }}Ha = 1, {\text{ }}S = 1 $ (a) $\beta = 0.1, {\text{ }}K = $$ 10.$ (b) $ \beta = 0.1, {\text{ }}K = 10. $ (c) $ \beta = 0, {\text{ }}K = 10. $ (d) $\beta = 0.1, {\text{ }}K = 30$

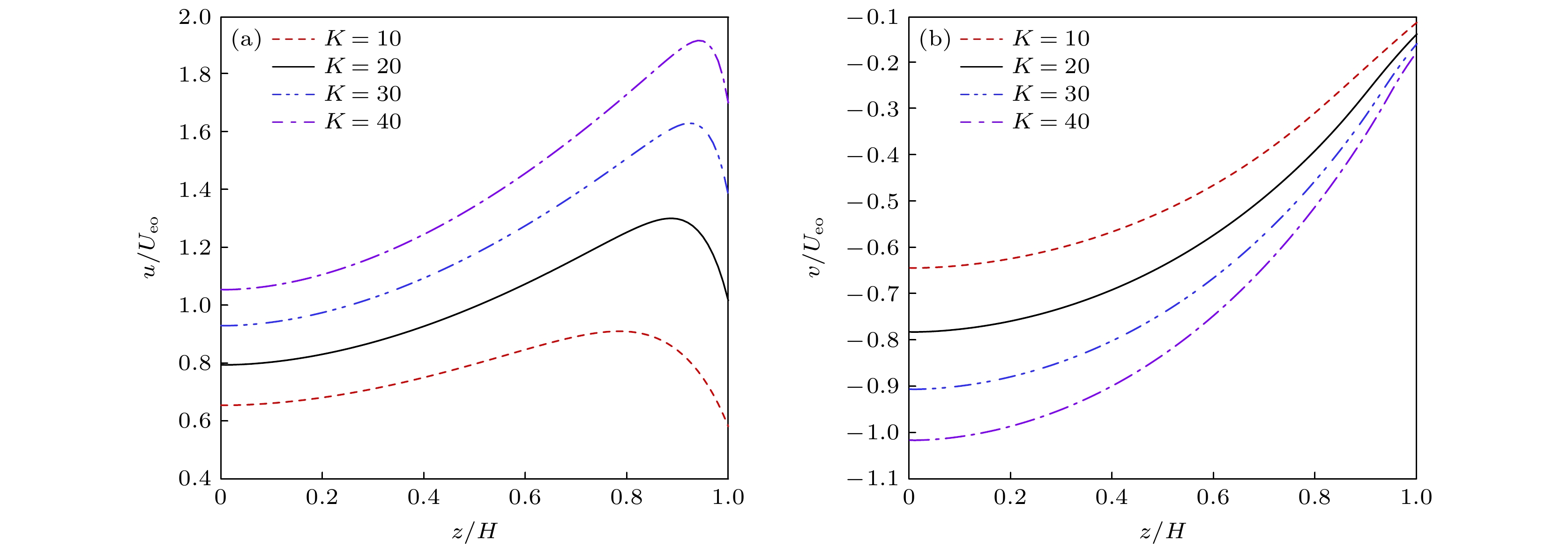
    Fig. 8.  The influence of the rotational angular velocity $\varOmega $ on the rotational electroosmotic flow velocity of the external magnetic field, $ n = 1.2, $$ {\bar \psi _\omega } = 5{\text{ }}V, {\text{ }} $$ a = 0.05, {\text{ }}Ha = 1, {\text{ }}S = 1 $ (a) $ \beta = 0.1, {\text{ }}K = 10. $ (b) $ \beta = 0.1, {\text{ }}K = 10. $ (c) $ \beta = 0, {\text{ }}K = 10. $ (d) $\beta = 0.1, $$ {\text{ }}K = 30$

    下载: 全尺寸图片 幻灯片

    图 9  电动宽度 K 对外加磁场的旋转电渗流速度分布的影响, 其中$ n = 0.8, $ $ \beta {\text{ = 0}}{\text{.1, }}\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, {\text{ }}{\bar \psi _\omega } = 5{\text{ }}{\rm{V}}, $ ${\text{ }}a = 0.05, {\text{ }}Ha = $$ 1, {\text{ }}S = $1

    Fig. 9.  The influence of the electric width K on the velocity distribution of rotating electroosmotic flow with external magnetic field, $n = 0.8, {\text{ }}\beta {\text{ = 0}}{\text{.1, }}\varOmega = 100{\text{ rad/s}}, {\text{ }}{\bar \psi _\omega } = 5{\text{ }}V, {\text{ }}$$ a = 0.05, {\text{ }}Ha = 1, {\text{ }}S = 1 $

    下载: 全尺寸图片 幻灯片

    图 10  电动宽度 K 对外加磁场的旋转电渗流速度分布的影响, 其中$ n = 1.2, $$ \beta {\text{ = 0}}{\text{.1, }}\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, {\text{ }}{\bar \psi _\omega } = 5{\text{ }}{\rm{V}}, {\text{ }}a = 0.05, {\text{ }}Ha = $$ 1, {\text{ }}S = $1

    Fig. 10.  The influence of the electric width K on the velocity distribution of rotating electroosmotic flow with external magnetic field, $ n = 1.2, {\text{ }}\beta {\text{ = 0}}{\text{.1, }}\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, {\text{ }}{\bar \psi _\omega } = 5{\text{ }}{\rm{V}}, {\text{ }} $$ a = 0.05, {\text{ }}Ha = 1, {\text{ }}S = 1 $

    下载: 全尺寸图片 幻灯片

    图 11  电动宽度 K 对外加磁场的旋转电渗流速度分布的影响, 其中$ n = 1.2, $$\beta {\text{ = 0, }}\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, {\text{ }}{\bar \psi _\omega } = 5{\text{ }}{\rm{V}}, {\text{ }}a = 0.05, {\text{ }}Ha = $$ 1, {\text{ }}S =$1

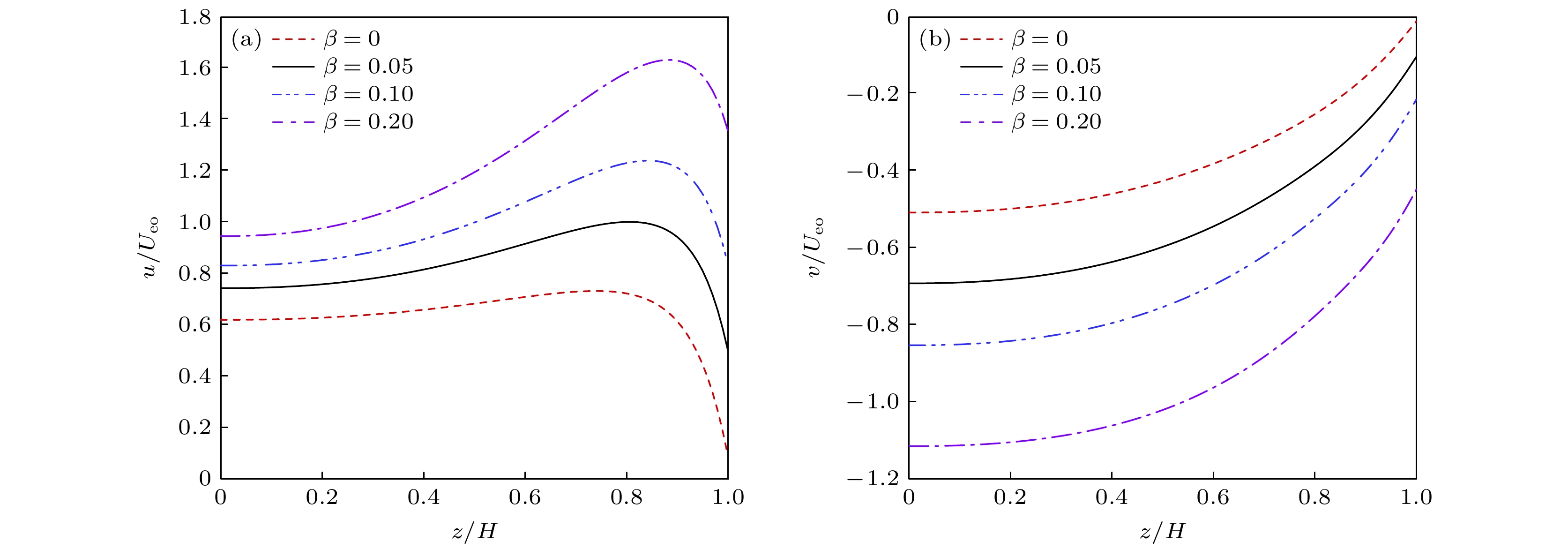
    Fig. 11.  The influence of the electric width K on the velocity distribution of rotating electroosmotic flow with external magnetic field, $ n = 1.2, {\text{ }}\beta {\text{ = 0, }}\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, {\text{ }}{\bar \psi _\omega } = 5{\text{ }}{\rm{V}}, {\text{ }} $$ a = 0.05, {\text{ }}Ha = 1, {\text{ }}S = 1 $

    下载: 全尺寸图片 幻灯片

    图 12  滑移参数$\beta $对外加磁场的旋转电渗流速度的影响, 其中$ n = 0.8, $$ K = 10, $$\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, {\text{ }}{\bar \psi _\omega } = 5{\text{ }}{\rm{V}}, {\text{ }}a = 0.05, {\text{ }}Ha = $$ 1, {\text{ }}S =$1

    Fig. 12.  The influence of the slip parameter$\beta $on the rotating electroosmotic flow velocity with an external magnetic field, $ n = 0.8, $$ K = 10, $$ \varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, {\text{ }}{\bar \psi _\omega } = 5{\text{ }}{\rm{V}}, {\text{ }}a = 0.05, {\text{ }}Ha = 1, {\text{ }}S = 1$

    下载: 全尺寸图片 幻灯片

    图 13  滑移参数$\beta $对外加磁场的旋转电渗流速度的影响, 其中$ n = 1.2, $$ K = 10, $$ \varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, {\text{ }}{\bar \psi _\omega } = 5{\text{ }}{\rm{V}}, {\text{ }}a = 0.05, {\text{ }}Ha = $$ 1, {\text{ }}S = 1 $

    Fig. 13.  The influence of the slip parameter$\beta $on the rotating electroosmotic flow velocity with an external magnetic field, $ n = 1.2, $$ K = 10, $$\varOmega = 100{\text{ }}{\rm{rad}}/{\rm{s}}, {\text{ }}{\bar \psi _\omega } = 5{\text{ }}{\rm{V}}, {\text{ }}a = 0.05, {\text{ }}Ha = 1, {\text{ }}S = 1$

    下载: 全尺寸图片 幻灯片
    Baidu
  • [1]

    Stone H A, Stroock A D, Ajdari A 2004 Annu. Rev. Fluid Mech. 36 381Google Scholar

    [2]

    Patel M, Kruthiventi S S H, Kaushik P 2020 Colloids Surf. B 193 111058Google Scholar

    [3]

    Srinivas, Bhadri 2016 Colloids Surf. A 492 144Google Scholar

    [4]

    Nekoubin N 2018 J. Non-Newtonian Fluid Mech. 260 54Google Scholar

    [5]

    Baños R D, Arcos J C, Bautista O, Méndez F, Merchán-Cruz E A 2021 J. Braz. Soc. Mech. Sci. 43 1Google Scholar

    [6]

    Baños R, Arcos J, Bautista O, Méndez F 2020 Defect Diffus. Forum 399 92Google Scholar

    [7]

    姜玉婷, 齐海涛 2015 64 174702Google Scholar

    Jiang Y T, Qi H T 2015 Acta Phys. Sin. 64 174702Google Scholar

    [8]

    Ajdari A 2002 Phys. Rev. E 65 16301Google Scholar

    [9]

    Chang C C, Wang C Y 2011 Phys. Rev. E 84 056320Google Scholar

    [10]

    Song J, Wang S W, Zhao M L, Li N 2020 Z. Naturforsch. A: Phys. Sci. 75 649Google Scholar

    [11]

    Shit G C, Mondal A, Sinha A, Kundu P K 2016 Colloids Surf. A 489 249Google Scholar

    [12]

    刘全生, 杨联贵, 苏洁 2013 62 144702Google Scholar

    Liu Q S, Yang L G, Su J 2013 Acta Phys. Sin. 62 144702Google Scholar

    [13]

    段娟, 陈耀钦, 朱庆勇 2016 65 034702Google Scholar

    Duan J, Chen Y Q, Zhu Q Y 2016 Acta Phys. Sin. 65 034702Google Scholar

    [14]

    Weston M C, Gerner M D, Fritsch I 2010 Anal. Chem. 82 3411Google Scholar

    [15]

    Jian Y J, Chang L 2015 AIP Adv. 5 057121Google Scholar

    [16]

    Xie Z Y, Jian Y J 2017 Colloids Surf. A 529 334Google Scholar

    [17]

    Habib U, Hayat T, Ahmad S, Alhodaly M S 2021 Int. Commun. Heat Mass Transfer 122 105111Google Scholar

    [18]

    Sarkar S, Ganguly S 2017 J. Non-Newtonian Fluid Mech. 250 18Google Scholar

    [19]

    Yang C H, Jian Y J, Xie Z Y, Li F Q 2020 Micromachines 11 418Google Scholar

    [20]

    Xie Z Y, Jian Y J 2017 Energy 139 1080Google Scholar

    [21]

    Wang S W, Li N, Zhao M L, Azese M N 2018 Z. Naturforsch. A: Phys. Sci. 73 825Google Scholar

    [22]

    Xie Z Y, Jian Y J 2014 Colloids Surf. A 461 231Google Scholar

    [23]

    Bird R B, Armstrong R C, Hassager O, Curtiss C F, Middleman S 1978 Phys. Today 31 54Google Scholar

  • [1] 慕江勇, 崔继峰, 陈小刚, 赵毅康, 田祎琳, 于欣如, 袁满玉. 微通道中一类生物流体在高Zeta势下的电渗流及传热特性.  , 2024, 73(6): 064701. doi: 10.7498/aps.73.20231685
    [2] 于欣如, 崔继峰, 陈小刚, 慕江勇, 乔煜然. 平行板微通道中一类不可压缩微极性流体在高Zeta势下的时间周期电渗流.  , 2024, 73(16): 164701. doi: 10.7498/aps.73.20240591
    [3] 徐明, 徐立清, 赵海林, 李颖颖, 钟国强, 郝保龙, 马瑞瑞, 陈伟, 刘海庆, 徐国盛, 胡建生, 万宝年, EAST团队. EAST反磁剪切qmin$\approx $2条件下磁流体力学不稳定性及内部输运垒物理实验结果简述.  , 2023, 72(21): 215204. doi: 10.7498/aps.72.20230721
    [4] 高效伟, 丁金兴, 刘华雩. 有限线法及其在流固域间耦合传热中的应用.  , 2022, 71(19): 190201. doi: 10.7498/aps.71.20220833
    [5] 何郁波, 唐先华, 林晓艳. 基于格子玻尔兹曼方法的一类FitzHugh-Nagumo系统仿真研究.  , 2016, 65(15): 154701. doi: 10.7498/aps.65.154701
    [6] 刘全, 于明, 林忠, 王瑞利. 流体力学拉氏守恒滑移线算法设计.  , 2015, 64(19): 194701. doi: 10.7498/aps.64.194701
    [7] 朱小敏, 任新成, 郭立新. 指数型粗糙地面与上方矩形截面柱宽带电磁散射的时域有限差分法研究.  , 2014, 63(5): 054101. doi: 10.7498/aps.63.054101
    [8] 刘建晓, 张郡亮, 苏明敏. 基于时域有限差分法的各向异性铁氧体圆柱电磁散射分析.  , 2014, 63(13): 137501. doi: 10.7498/aps.63.137501
    [9] 王光辉, 王林雪, 王灯山, 刘丛波, 石玉仁. K（m,n,p）方程多-Compacton相互作用的数值研究.  , 2014, 63(18): 180206. doi: 10.7498/aps.63.180206
    [10] 杨利霞, 马辉, 施卫东, 施丽娟, 于萍萍. 基于表面阻抗边界条件的等离子体薄涂层电磁散射的时域有限差分分析.  , 2013, 62(3): 034102. doi: 10.7498/aps.62.034102
    [11] 彭武, 何怡刚, 方葛丰, 樊晓腾. 二维泊松方程的遗传PSOR改进算法.  , 2013, 62(2): 020301. doi: 10.7498/aps.62.020301
    [12] 颛孙旭, 马西奎. 一种适用于任意阶空间差分时域有限差分方法的色散介质通用吸收边界条件算法.  , 2012, 61(11): 110206. doi: 10.7498/aps.61.110206
    [13] 长龙, 菅永军. 平行板微管道间Maxwell流体的高Zeta势周期电渗流动.  , 2012, 61(12): 124702. doi: 10.7498/aps.61.124702
    [14] 任新成, 郭立新, 焦永昌. 雪层覆盖的粗糙地面与上方矩形截面柱复合电磁散射的时域有限差分法研究.  , 2012, 61(14): 144101. doi: 10.7498/aps.61.144101
    [15] 尹经禅, 肖晓晟, 杨昌喜. 光纤中受激Brillouin散射动态弛豫振荡特性及其抑制方法.  , 2009, 58(12): 8316-8325. doi: 10.7498/aps.58.8316
    [16] 杨利霞, 葛德彪, 王 刚, 阎 述. 磁化铁氧体材料电磁散射递推卷积-时域有限差分方法分析.  , 2007, 56(12): 6937-6944. doi: 10.7498/aps.56.6937
    [17] 苍 宇, 鲁 欣, 武慧春, 张 杰. 有质动力和静电分离场对激光等离子体流体力学状态的影响.  , 2005, 54(2): 812-817. doi: 10.7498/aps.54.812
    [18] 谭新玉, 张端明, 李智华, 关 丽, 李 莉. 纳秒脉冲激光沉积薄膜过程中的烧蚀特性研究.  , 2005, 54(8): 3915-3921. doi: 10.7498/aps.54.3915
    [19] 赵红东, 宋殿友, 张智峰, 孙 静, 孙 梅, 武 一, 温幸饶. n型DBR中电势对垂直腔面发射激光器阈值的影响.  , 2004, 53(11): 3744-3747. doi: 10.7498/aps.53.3744
    [20] 梁昌洪, 褚庆昕. 运动边界的电磁场边界条件.  , 2002, 51(10): 2202-2204. doi: 10.7498/aps.51.2202
目录
计量
  • 文章访问数:  3405
  • PDF下载量:  47
  • 被引次数: 0
出版历程
  • 收稿日期:  2021-12-16
  • 修回日期:  2022-03-03
  • 上网日期:  2022-06-29
  • 刊出日期:  2022-07-05

/

返回文章
返回

作者中心

审稿中心

关于期刊

关于我们

版权所有 ©  

地址：北京市603信箱，《 》编辑部邮编：100190

电话：010-82649829，82649241,82649863Email：apsoffice@iphy.ac.cn   百度统计

Baidu
map