-
在实际量子密钥分发系统中, 实际器件不理想可能导致系统存在安全性隐患. 比如, 光源端的编码设备不理想, 可能导致量子态存在误差; 探测端的探测器存在缺陷, 可能产生后脉冲或死时间效应, 从而影响系统的实际安全性. 因此, 本文提出了一种同时考虑光源端和探测器缺陷的实用化态制备误差容忍参考系无关量子密钥分发协议. 本文采用三强度诱骗态方案开展建模分析与数值仿真计算. 本协议通过利用虚拟态方法估算相位误码率, 降低了态制备误差对密钥率的影响; 同时对探测器端的缺陷进行相应参数刻画, 具有较强的鲁棒性, 为参考系无关量子密钥分发协议的实际应用提供了重要参考价值.Quantum key distribution (QKD) enables the establishment of shared keys between two distant users, Alice and Bob, based on the fundamental principles of quantum mechanics, and it has proven to possess information-theoretic security. In most of QKD systems, Alice and Bob require a shared reference frame, and real-time calibration of the reference frame increases system costs and reduces its performance. Fortunately, the reference-frame-independent QKD protocol has been proposed, overcoming reference-frame drift issues and receiving widespread attention. However, in practical QKD systems, the non-ideal characteristics of realistic devices introduce certain inconsistency between the theory and the practice. In real-world quantum key distribution systems, device imperfections can lead to security vulnerabilities, thereby reducing system security. For example, imperfections in the encoding apparatus at the source end may result in errors in the quantum states. The inherent defects in the detection part may cause after-pulse effects and dead-time effects, thus reducing the key rate. Therefore, in this work, we propose a practical state-preparation error tolerant reference-frame-independent quantum key distribution protocol by taking imperfections in both the source and the detectors into account. Moreover, a three-intensity decoy-state scheme for modeling analysis and numerical simulations is employed. In this protocol, we reduce the influence of state-preparation errors on the key rate by utilizing virtual state methods to precisely estimate the phase-error rate. Furthermore, by characterizing the effects of after-pulses and dead-time on the key rate, our protocol exhibits higher robustness and can effectively address issues related to detector imperfections. This approach can also be extended to other quantum key distribution protocols with higher security levels, such as measurement-device-independent quantum key distribution protocol and twin-field quantum key distribution, further mitigating the influence of device imperfections on practical implementation of QKD system. Therefore, our present work provide important reference value for putting the quantum key distributions into practical application.
-
Keywords:
- quantum key distribution /
- state preparation error /
- reference-frame-independent protocol /
- after-pulse effect /
- dead-time effect
[1] Bennett C H, Brassard G 1984 Proceedings of IEEE International Conference on Computers, System and Signal Processing (Vol. 1 of 3) (Bangalore: IEEE) pp175–179
[2] Brassard G, Lütkenhaus N, Mor T, Sanders B C 2000 Phys. Rev. Lett. 85 1330
Google Scholar
[3] Yuan Z, Plews A, Takahashi R, Doi K, Tam W, Sharpe A W, Dixon A R, Lavelle E, Dynes J F, Murakami A, Kujiraoka M, Lucamarini M, Tanizawa Y, Sato H, Shields A J 2018 J. Light. Technol. 36 16
[4] Boaron A, Korzh B, Houlmann R, Boso G, Rusca D, Gray S, Li M, Nolan D, Martin A, Zbinden H 2018 Appl. Phys. Lett. 112 17
Google Scholar
[5] Minder M, Pittaluga M, Roberts G, Lucamarini M, Dynes J F, Yuan Z L, Shields A J 2019 Nat. Photonics 13 5
Google Scholar
[6] Liu Y, Yu Z W, Zhang W, Guan J Y, Chen J P, Zhang C, Hu X L, Li H, Jiang C, Lin J, Chen T Y, You L, Wang Z, Wang X B, Zhang Q, Pan J W 2019 Phys. Rev. Lett. 123 100505
Google Scholar
[7] Bennett C H, Bessette F, Brassard G, Salvail L, Smolin J 1992 J Cryptol 5 3
Google Scholar
[8] Kurtsiefer C, Zarda P, Halder M, Weinfurter H, Gorman P M, Tapster P R, Rarity J G 2002 Nature 419 450
Google Scholar
[9] Laing A, Scarani V, Rarity J G, O’Brien J L 2018 Phys. Rev. A 82 012304
Google Scholar
[10] Gottesman D, Lo H K, Lütkenhaus N, Preskill J 2004 Quantum Inf. Comput. 4 325
Google Scholar
[11] Tamaki K, Curty M, Kato G, Lo H K, Azuma K 2014 Phys. Rev. A 90 052314
Google Scholar
[12] Wang C, Sun S H, Ma X C, Tang G Z, Liang L M 2015 Phys. Rev. A 92 042319
Google Scholar
[13] Xu F H, Wei K J, Sajeed S, Kaiser S, Sun S H, Tang Z Y, Qian L, Makarov V, Lo H K 2015 Phys. Rev. A 92 032305
Google Scholar
[14] Tang Z Y, Wei K J, Bedroya O, Qian L, Lo H K 2016 Phys. Rev. A 93 042308
Google Scholar
[15] Zhou X Y, Ding H J, Zhang C H, Li J, Zhang C M, Wang Q 2020 Opt. Lett. 45 4176
Google Scholar
[16] Fan Y G J, Wang C, Wang S, Yin Z Q, Liu H, Chen W, He D Y, Han Z F, Guo G C 2018 Phys. Rev. Appl. 10 064032
Google Scholar
[17] Campbell L 1992 Rev. Sci. Instrum. 63 5794
Google Scholar
[18] Rusca D, Boaron A, Grünenfelder F, Martin A, Zbinden H 2018 Appl. Phys. Lett. 112 171104
Google Scholar
[19] 莫小范 2006 博士毕业论文 (合肥: 中国科学技术大学)
Mo X F 2006 Ph. D. Dissertation (Hefei: University of Science and Technology of China
[20] Wang W J, Zhou X Y, Zhang C H, Ding H J, Wang Q 2022 Quantum Inf. Process 21 1
Google Scholar
[21] 范元冠杰 2020 博士毕业论文 (合肥: 中国科学技术大学)
Fan Y G J 2020 Ph. D. Dissertation (Hefei: University of Science and Technology of China
[22] Wang X B 2005 Phys. Rev. Lett. 94 30503
Google Scholar
[23] 马啸, 孙铭铄, 刘靖阳, 丁华建, 王琴 2022 71 030301
Google Scholar
Ma X, Sun M S, Liu J Y, Ding H J, Wang Q 2022 Acta Phys. Sin. 71 030301
Google Scholar
[24] Fung C F, Tamaki K, Qi B, Lo H K, Ma X F 2009 Quantum Inf. Comput. 9 1533
Google Scholar
[25] Sun S H, Xu F H 2021 New J. Phys. 23 023011
Google Scholar
[26] Zhou Y H, Yu Z W, Wang X B 2016 Phys. Rev. A 93 042324
Google Scholar
[27] Zhang C H, Zhang C M, Guo G C, Wang Q 2018 Opt. Express 26 4219
Google Scholar
[28] Zhou X Y, Zhang C H, Zhang C M, Wang Q 2017 Phys. Rev. A 96 052337
Google Scholar
[29] Jiang C, Yu Z W, Hu X L, Wang X B 2021 Phys. Rev. A 103 012402
Google Scholar
[30] Huang L Y, Zhang Y C, Yu S 2021 Chin. Phys. Lett. 38 040301
Google Scholar
[31] Lucamarini M, Yuan Z L, Dynes J F, Shields A J 2018 Nature 557 400
Google Scholar
[32] Wang X B, Yu Z W, Hu X L 2018 Phys. Rev. A 98 062323
Google Scholar
-
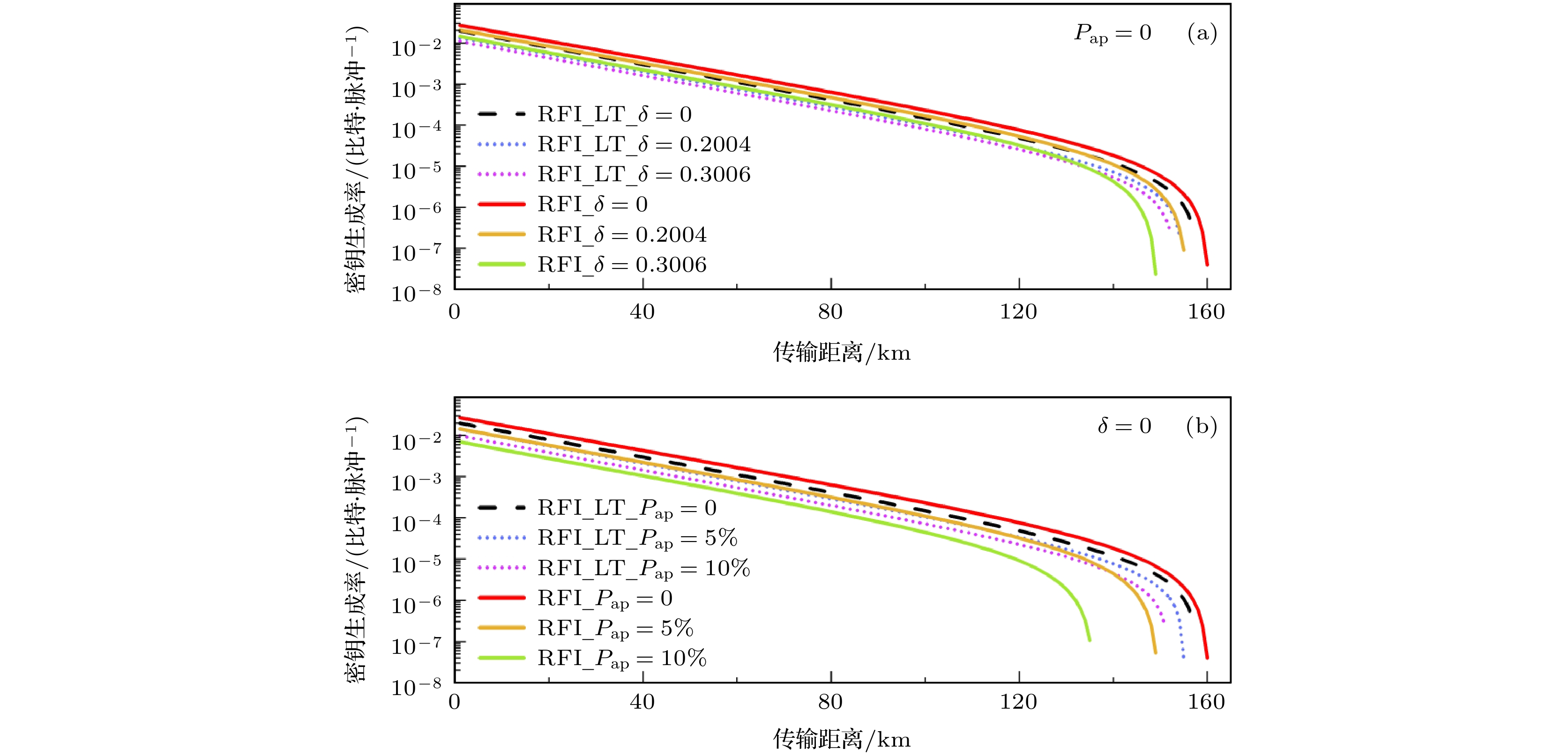
图 1 (a) 基于态制备缺陷$ \delta $的RFI协议以及LT-RFI协议的密钥生成率图; (b)基于后脉冲效应$ {P_{{\text{ap}}}} $的RFI协议以及LT-RFI协议的密钥生成率图
Fig. 1. (a) Key generation rates of the RFI protocol and LT-RFI protocol based on state preparation flaws $ \delta $; (b) the key generation rates of the RFI protocol and LT-RFI protocol based afterpulse effect $ {P_{{\text{ap}}}} $.
图 2 基于态制备缺陷和后脉冲效应的RFI 协议以及LT-RFI协议的密钥生成率图
Fig. 2. Key generation rates of the RFI protocol and LT-RFI protocol based on state preparation flaws and after-pulse effect.
图 3 基于态制备缺陷和后脉冲效应的RFI 协议与LT-RFI 协议的Eve获取的信息量
Fig. 3. Information leakage to Eve of the RFI protocols and LT-RFI protocols based on state preparation flaws and after-pulse effect.
图 4 基于不同设备缺陷的RFI协议以及LT-RFI协议密钥生成率图
Fig. 4. Key generation rates of the RFI protocol and LT-RFI protocol based on different defects in equipments.
表 1 基于后脉冲效应和死时间效应的LT-RFI协议仿真参数列表
Table 1. Parameter list used in simulation of LT-RFI protocol based on after-pulse effect and dead time effect.
Bob探测器
暗计数率$ {P_{{\text{dc}}}} $Bob探测器
效率$ {\eta _{{\text{Bob}}}} $系统纠错
系数fAlice发送的
总脉冲数N系统
重复频率F信道损耗系数
$ \alpha $/(dB·km–1)系统
本底误码$ {e_{\text{d}}} $3×10–6 0.145 1.16 1012 109 0.2 0.0015 
下载: 导出CSV
-
[1] Bennett C H, Brassard G 1984 Proceedings of IEEE International Conference on Computers, System and Signal Processing (Vol. 1 of 3) (Bangalore: IEEE) pp175–179
[2] Brassard G, Lütkenhaus N, Mor T, Sanders B C 2000 Phys. Rev. Lett. 85 1330
Google Scholar
[3] Yuan Z, Plews A, Takahashi R, Doi K, Tam W, Sharpe A W, Dixon A R, Lavelle E, Dynes J F, Murakami A, Kujiraoka M, Lucamarini M, Tanizawa Y, Sato H, Shields A J 2018 J. Light. Technol. 36 16
[4] Boaron A, Korzh B, Houlmann R, Boso G, Rusca D, Gray S, Li M, Nolan D, Martin A, Zbinden H 2018 Appl. Phys. Lett. 112 17
Google Scholar
[5] Minder M, Pittaluga M, Roberts G, Lucamarini M, Dynes J F, Yuan Z L, Shields A J 2019 Nat. Photonics 13 5
Google Scholar
[6] Liu Y, Yu Z W, Zhang W, Guan J Y, Chen J P, Zhang C, Hu X L, Li H, Jiang C, Lin J, Chen T Y, You L, Wang Z, Wang X B, Zhang Q, Pan J W 2019 Phys. Rev. Lett. 123 100505
Google Scholar
[7] Bennett C H, Bessette F, Brassard G, Salvail L, Smolin J 1992 J Cryptol 5 3
Google Scholar
[8] Kurtsiefer C, Zarda P, Halder M, Weinfurter H, Gorman P M, Tapster P R, Rarity J G 2002 Nature 419 450
Google Scholar
[9] Laing A, Scarani V, Rarity J G, O’Brien J L 2018 Phys. Rev. A 82 012304
Google Scholar
[10] Gottesman D, Lo H K, Lütkenhaus N, Preskill J 2004 Quantum Inf. Comput. 4 325
Google Scholar
[11] Tamaki K, Curty M, Kato G, Lo H K, Azuma K 2014 Phys. Rev. A 90 052314
Google Scholar
[12] Wang C, Sun S H, Ma X C, Tang G Z, Liang L M 2015 Phys. Rev. A 92 042319
Google Scholar
[13] Xu F H, Wei K J, Sajeed S, Kaiser S, Sun S H, Tang Z Y, Qian L, Makarov V, Lo H K 2015 Phys. Rev. A 92 032305
Google Scholar
[14] Tang Z Y, Wei K J, Bedroya O, Qian L, Lo H K 2016 Phys. Rev. A 93 042308
Google Scholar
[15] Zhou X Y, Ding H J, Zhang C H, Li J, Zhang C M, Wang Q 2020 Opt. Lett. 45 4176
Google Scholar
[16] Fan Y G J, Wang C, Wang S, Yin Z Q, Liu H, Chen W, He D Y, Han Z F, Guo G C 2018 Phys. Rev. Appl. 10 064032
Google Scholar
[17] Campbell L 1992 Rev. Sci. Instrum. 63 5794
Google Scholar
[18] Rusca D, Boaron A, Grünenfelder F, Martin A, Zbinden H 2018 Appl. Phys. Lett. 112 171104
Google Scholar
[19] 莫小范 2006 博士毕业论文 (合肥: 中国科学技术大学)
Mo X F 2006 Ph. D. Dissertation (Hefei: University of Science and Technology of China
[20] Wang W J, Zhou X Y, Zhang C H, Ding H J, Wang Q 2022 Quantum Inf. Process 21 1
Google Scholar
[21] 范元冠杰 2020 博士毕业论文 (合肥: 中国科学技术大学)
Fan Y G J 2020 Ph. D. Dissertation (Hefei: University of Science and Technology of China
[22] Wang X B 2005 Phys. Rev. Lett. 94 30503
Google Scholar
[23] 马啸, 孙铭铄, 刘靖阳, 丁华建, 王琴 2022 71 030301
Google Scholar
Ma X, Sun M S, Liu J Y, Ding H J, Wang Q 2022 Acta Phys. Sin. 71 030301
Google Scholar
[24] Fung C F, Tamaki K, Qi B, Lo H K, Ma X F 2009 Quantum Inf. Comput. 9 1533
Google Scholar
[25] Sun S H, Xu F H 2021 New J. Phys. 23 023011
Google Scholar
[26] Zhou Y H, Yu Z W, Wang X B 2016 Phys. Rev. A 93 042324
Google Scholar
[27] Zhang C H, Zhang C M, Guo G C, Wang Q 2018 Opt. Express 26 4219
Google Scholar
[28] Zhou X Y, Zhang C H, Zhang C M, Wang Q 2017 Phys. Rev. A 96 052337
Google Scholar
[29] Jiang C, Yu Z W, Hu X L, Wang X B 2021 Phys. Rev. A 103 012402
Google Scholar
[30] Huang L Y, Zhang Y C, Yu S 2021 Chin. Phys. Lett. 38 040301
Google Scholar
[31] Lucamarini M, Yuan Z L, Dynes J F, Shields A J 2018 Nature 557 400
Google Scholar
[32] Wang X B, Yu Z W, Hu X L 2018 Phys. Rev. A 98 062323
Google Scholar
下载:
计量
- 文章访问数: 2288
- PDF下载量: 129
- 被引次数: 0
