-
借助于电感型耦合器产生人造规范势(有效磁通), 本文研究了正方形传输子量子比特模型中的量子态输运性质. 理论计算发现, 单粒子本征态与单空穴本征态具有相同的本征能谱, 并且相同能量下, 两者的平均粒子流与平均空穴流受有效磁通正弦调制后互为相反数. 当初态为占据一个格点的单粒子与单空穴时, 如果系统时间反演对称(有效磁通为
$4{\rm \pi}$ 的整数倍), 单粒子与单空穴的含时波函数各分量相等, 否则不等. 分析证明, 以上计算结果是由于对体系哈密顿量的粒子-空穴操作等价于对其做时间反演. 此外还发现, 有效磁通为π时, 单粒子或单空穴只在初始比特与两个相邻比特之间输运, 有效磁通为0时, 单粒子或单空穴通过两个相邻比特输运到对角比特, 然后再反向输运; 无论有效磁通如何取值, 两者具有相同的平均(粒子或空穴)流和格点占据概率.In this paper, we study the transport properties of quantum states in the square-lattice quantum bit model by using inductive couplers to generate the artificial gauge potential (effective magnetic flux). It is found by theoretical calculation that the eigenstates of single particle and single hole have the same eigen energy spectrum, and the average particle and hole currents, sinusoidally modulated by the effective magnetic flux, are opposite to each other with respect to the same eigen energy. For an initial single-particle or single-hole state where only one lattice site is occuplied, if the time-inversion symmetry is preserved (the effective magnetic flux is an integral multiple of 4π), the components of the time-dependent wave functions of the single particle and the single hole are equal, otherwise they are not equal. The analysis demonstrates that the above calculation results are due to the fact that the particle-hole operation for the system Hamiltonian is equivalent to the time inversion. In addition, it is found that when the effective magnetic flux is π, a single particle or a single hole is only transported between the initial bit and two adjacent bits, and when the effective magnetic flux is 0, a single particle or a single hole is transported to the diagonal bit through two adjacent bits, and then transported in reverse. Regardless of the value of effective magnetic flux, both the single-particle and single-hole states share the same average (particle or hole) current and lattice site occupation probability.-
Keywords:
- gauge potential /
- quantum state transport /
- quantum state preparation
[1] Feynman R P 1982 Int. J. Theor. Phys. 21 467
Google Scholar
[2] Georgescu I M, Ashhab S, Nori F 2014 Rev. Mod. Phys. 86 153
Google Scholar
[3] Berry M V 1984 Proc. R. Soc. A 392 45
Google Scholar
[4] Hatsugai Y 1993 Phys. Rev. B 48 11851
[5] Tsui D C, Stormer H L, Gossard A C 1982 Phys. Rev. Lett. 48 1559
Google Scholar
[6] Laughlin R B 1983 Phys. Rev. Lett. 50 1395
Google Scholar
[7] Kane C L, Mele E J 2005 Phys. Rev. Lett. 95 146802
Google Scholar
[8] Bernevig B A, Hughes T L, Zhang S C 2006 Science 314 1757
Google Scholar
[9] Bernevig B A, Zhang S C 2006 Phys. Rev. Lett. 96 106802
Google Scholar
[10] Konig M, Wiedmann S, Brune C, Roth A, Buhmann H, Molenkamp L W, Qi X L, Zhang S C 2007 Science 318 766
Google Scholar
[11] Hsieh D, Qian D, Wray L, Xia Y, Hor Y S, Cava R J, Hasan M Z 2008 Nature 452 970
Google Scholar
[12] Chiu C K, Teo J C Y, Schnyder A P, Ryu S 2016 Rev. Mod. Phys. 88 035005
Google Scholar
[13] Goldman N, Juzeliunas G, Oehberg P, Spielman I B 2014 Rep. Prog. Phys. 77 126401
Google Scholar
[14] Lin Y J, Compton R L, Perry A R, Phillips W D, Porto J V, Spielman I B 2009 Phys. Rev. Lett. 102 130401
Google Scholar
[15] Lin Y J, Compton R L, Jimenez-Garcia K, Porto J V, Spielman I B 2009 Nature 462 628
Google Scholar
[16] Lin Y J, Compton R L, Jimenez-Garcia K, Phillips W D, Porto J V, Spielman I B 2011 Nat. Phys. 7 531
Google Scholar
[17] Aidelsburger M, Atala M, Nascimbene S, Trotzky S, Chen Y A, Bloch I 2011 Phys. Rev. Lett. 107 255301
Google Scholar
[18] Aidelsburger M, Atala M, Lohse M, Barreiro J T, Paredes B, Bloch I 2013 Phys. Rev. Lett. 111 185301
Google Scholar
[19] Krantz P, Kjaergaard M, Yan F, Orlando T P, Gustavsson S, Oliver W D 2019 Appl. Phys. Rev. 6 021318
Google Scholar
[20] Gu X, Kockum A F, Miranowicz A, Liu Y X, Nori F 2017 Phys. Rep. 718 1
Google Scholar
[21] Blais A, Grimsmo A L, Girvin S M, Wallraff A 2021 Rev. Mod. Phys. 93 025005
Google Scholar
[22] Nakamura Y, Pashkin Y A, Tsai J S 1999 Nature 398 786
Google Scholar
[23] Leek P J, Fink J M, Blais A, Bianchetti R, Göppl M, Gambetta J M, Schuster D I, Frunzio L, Schoelkopf R J, Wallraff A 2007 Science 318 1889
Google Scholar
[24] Möttönen M, Vartiainen J J, Pekola J P 2008 Phys. Rev. Lett. 100 177201
Google Scholar
[25] Neeley M, Ansmann M, Bialczak R C, Hofheinz M, Lucero E, O'Connell A D, Sank D, Wang H, Wenner J, Cleland A N, Geller M R, Martinis J M 2009 Science 325 722
Google Scholar
[26] Schroer M D, Kolodrubetz M H, Kindel W F, Sandberg M, Gao J, Vissers M R, Pappas D P, Polkovnikov A, Lehnert K W 2014 Phys. Rev. Lett. 113 050402
Google Scholar
[27] Roushan P, Neill C, Chen Y, et al. 2014 Nature 515 241
Google Scholar
[28] Barends R, Kelly J, Megrant A, et al. 2014 Nature 508 500
Google Scholar
[29] Tangpanitanon J, Bastidas V M, Al-Assam S, Roushan P, Jaksch D, Angelakis D G 2016 Phys. Rev. Lett. 117 213603
Google Scholar
[30] Alaeian H, Chang C W S, Moghaddam M V, Wilson C M, Solano E, Rico E 2019 Phys. Rev. A 99 053834
Google Scholar
[31] Zhao Y J, Xu X W, Wang H, Liu Y X, Liu W M 2020 Phys. Rev. A 102 053722
Google Scholar
[32] Koch J, Houck A A, Hur K L, Girvin S M 2010 Phys. Rev. A 82 043811
Google Scholar
[33] Yang Z H, Wang Y P, Xue Z Y, Yang W L, Hu Y, Gao J H, Wu Y 2016 Phys. Rev. A 93 062319
Google Scholar
[34] Roushan P, Neill C, Megrant A, et al. 2017 Nat. Phys. 13 146
Google Scholar
[35] Chen Y, Neill C, Roushan P, Leung N, Fang M, Barends R, Kelly J, Campbell B, Chen Z, Chiaro B 2014 Phys. Rev. Lett. 113 220502
Google Scholar
[36] Geller M R, Donate E, Chen Y, Neill C, Roushan P, Martinis J M 2015 Phys. Rev. A 92 012320
Google Scholar
[37] Berkovits R 2013 Phys. Rev. B 87 075141
Google Scholar
[38] Luttinger J M 1951 Phys. Rev. 84 814
Google Scholar
[39] Ahmed S, Muoz C S, Nori F, Kockum A F 2021 Phys. Rev. Lett. 127 140502
Google Scholar
-
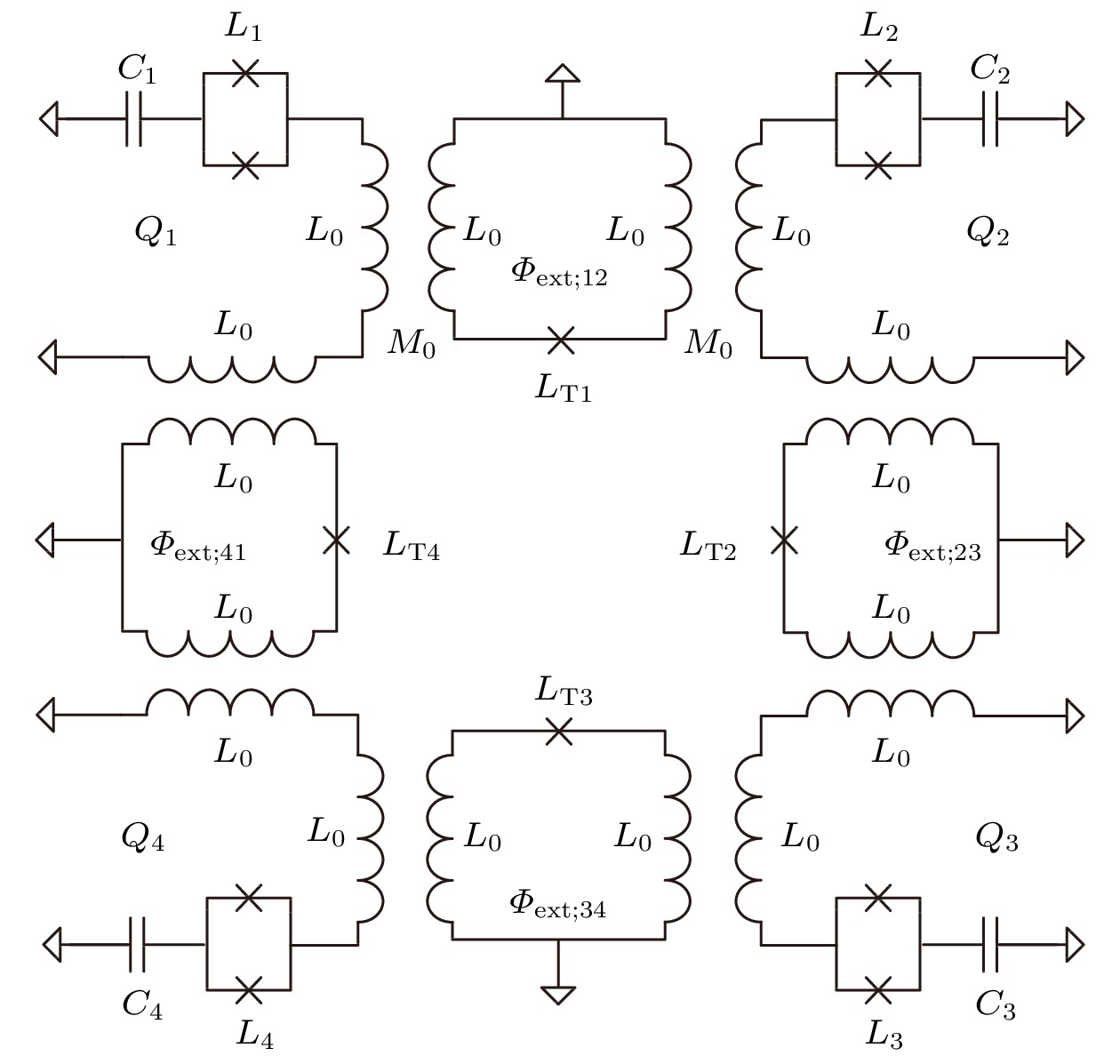
图 1 四量子比特电路结构示意图. 在该示意图中量子比特是由电容、电感和约瑟夫森结组成, 而耦合器由电感与约瑟夫森结组成的环路构成. 相邻两个量子比特之间通过耦合器实现耦合, 每一个耦合器可以调节外加磁通来实现可调耦合. 其中,
$ Q_{j} $ 表示量子比特,$ L_{j} $ 与$ L_{{\rm{T}}j} $ (j = 1, 2, 3, 4)都代表约瑟夫森结电感,$ C_{j} $ 代表电容,$ L_{0} $ 代表电感,$ M_{0} $ 表示相邻电感之间的互感Fig. 1. Schematic diagram of the four qubit circuit structure. In this diagram, a qubit is composed of a capacitor, an inductance and a Josephson junction, while a coupler is composed of a loop consisting of an inductance and a Josephson junction. The coupling between two adjacent qubits is realized by a coupler, where the external magnetic flux can be tuned to achieve adjustable coupling. Here,
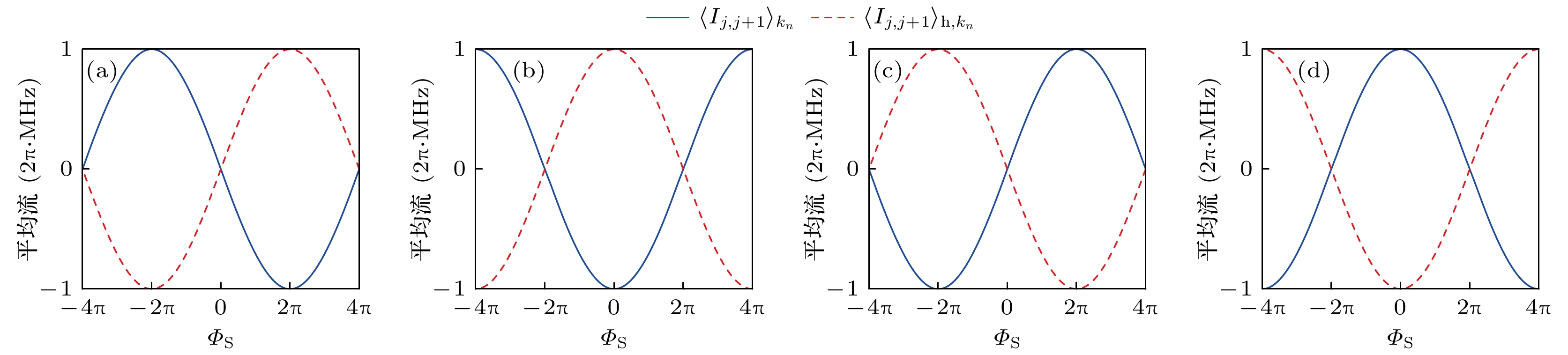
$ Q_{j} $ stands for a qubit,$ L_{j} $ and$ L_{{\rm{T}}j} $ (j = 1, 2, 3, 4) stand for Josephson junction inductance,$ C_ {j} $ stands for the capacitance,$ L_{0} $ stands for the inductance,$ M_{0} $ denotes the mutual inductance between adjacent inductances.
图 2 当n分别取(a) 1, (b) 2, (c) 3, (d) 4时, 单粒子(空穴)本征态
$ |k_{n} \rangle $ ($ |k_{n}\rangle_{\rm{h}} $ )下的平均粒子(空穴)流$ \langle I_{j, j+1}\rangle _{k_{n}} $ ($ \langle I_{j, j+1}\rangle _{{\rm{h}}, k_{n}} $ )随有效磁通$\varPhi_{{\rm{S}}}$ 的变化曲线. 取裸耦合强度$g_0/(2\pi)=4\operatorname{MHz}$ . 此外, 蓝色实线(红色虚线)代表平均粒子(空穴)流Fig. 2. Average particle (hole) current
$ \langle I_{j, j+1}\rangle _{k_{n}} $ ($ \langle I_{j, j+1}\rangle _{{\rm{h}}, k_{n}} $ ) in the single-particle (hole) eigenstate$ |k_n\rangle $ ($ |k_n\rangle_{\rm{h}} $ ) against the effective magnetic flux$\varPhi_{{\rm{S}}}$ for n taking (a) 1, (b) 2, (c) 3, and (d) 4, respectively. We have taken the coupling strength$g_0/(2\pi)=4\operatorname{MHz}$ . Additionally, the solid blue (dashed red) curves represent the particle (hole) current.
图 3 当有效磁通取
$\varPhi_{\rm{S}}=\pi$ ($\varPhi_{\rm{S}}=0$ )时, 单粒子态$ |1\rangle $ 演化时间t后(a)—(d) [(i)—(l)]比特$ Q_j- Q_{j+1} $ 之间的平均粒子流$ \langle I_{j, j+1}\rangle_1 $ 与(e)—(h) [(m)—(p)]量子态$ |j\rangle $ 上的占据概率$ P_{j} $ 的变化曲线. 其中, 蓝色虚线(红色实线)指排除(包含)环境影响, 此外, 取裸耦合强度$g_{0}/({2\pi})=4$ MHz,$ Q_j $ 弛豫速率$\gamma _{j}/({2\pi})\equiv0.05$ MHz,$ Q_j $ 退相速率$\varGamma_{j}/({2\pi})\equiv 0.1$ MHzFig. 3. Variation curves of both the (a)–(d) [(i)–(l)] average
$ Q_j $ –$ Q_{j+1} $ particle current$ \langle I_{j, j+1}\rangle_1 $ and (e)–(h) [(m)–(p)] occupation probability$ P_{j} $ on the quantum state$ |j\rangle $ for the effective magnetic flux taking$\varPhi_{\rm{S}}=\pi$ ($\varPhi_{\rm{S}}=0$ ) after the time t for which the single-particle state$ |1\rangle $ evolves. Here, the dashed blue (solid red) curves represent the environment influence is exluded (included). Additionally, we specify the bare coupling strength$g_{0}/({2\pi})=4$ MHz,$ Q_j $ relaxation rate$ \gamma _{j}/({2\pi})\equiv 0.05 $ MHz, and$ Q_j $ dephasing rate$ \varGamma_{j}/({2\pi})\equiv 0.1 $ MHz.
图 4 当有效磁通取
$\varPhi_{\rm{S}}=\pi$ ($\varPhi_{\rm{S}}=0$ )时, 单空穴态$ |1\rangle_{\rm{h}} $ 演化时间t后(a)—(d) [(i)—(l)]比特$Q_j $ —Qj+1之间的平均空穴流$ \langle I_{j, j+1}\rangle_{{\rm{h}}, 1} $ 与(e)—(h) [(m)—(p)]量子态$ |j\rangle_{\rm{h}} $ 上的占据概率$ P_{j}^{\rm{h}} $ 的变化曲线. 这里, 蓝色虚线(红色实线)指排除(包含)环境影响, 此外, 取裸耦合强度$g_{0}/({2\pi})=4$ MHz,$ Q_j $ 弛豫速率$\gamma _{j}/({2\pi})\equiv0.05$ MHz,$ Q_j $ 退相速率$\varGamma_{j}/({2\pi})\equiv 0.1$ MHzFig. 4. Variation curves of both the (a)–(d) [(i)–(l)] average
$ Q_j $ –$ Q_{j+1} $ hole current$ \langle I_{j, j+1}\rangle_1 $ and (e)–(h) [(m)–(p)] occupation probability$ P_{j}^{\rm{h}} $ on the quantum state$ |j\rangle_{\rm{h}} $ for the effective magnetic flux taking$\varPhi_{\rm{S}}=\pi$ ($ \varPhi_{\rm{S}}=0 $ ) after the time t for which the single-hole state$ |1\rangle_{\rm{h}} $ evolves. Here, the dashed blue (solid red) curves represent the environment influence is exluded (included). Additionally, we specify the bare coupling strength$g_{0}/({2\pi})=4$ MHz,$ Q_j $ relaxation rate$ \gamma _{j}/({2\pi})\equiv 0.05 $ MHz, and$ Q_j $ dephasing rate$ \varGamma_{j}/({2\pi})\equiv 0.1 $ MHz.
图 5 当有效磁通取
$\varPhi_{\rm{S}}=0$ (红色实线),$\varPhi_{\rm{S}}=\pi/4$ (蓝点段线)或$\varPhi_{\rm{S}}=\pi/2$ (绿色虚线)时, 演化时间t后单空穴态含时波函数$ |\psi^{\rm{h}}(t)\rangle $ 与单粒子态含时波函数$ |\psi(t)\rangle $ 的相近度$ |\langle \psi^{\rm{h}}(t)|\psi(t)\rangle| $ 的变化. 这里取裸耦合强度$g_{0}/({2\pi})=4$ MHzFig. 5. Variation curves of the similarity degree
$ |\langle \psi^{\rm{h}}(t)|\psi(t)\rangle| $ between the time-dependent wave function$ |\psi^{\rm{h}}(t)\rangle $ in the single hole state and the time-dependent wave function$ |\psi(t)\rangle $ in the single particle state for the effective magnetic flux taking$\varPhi_{\rm{S}}=0$ (solid red),$\varPhi_{\rm{S}}=\pi/4$ (dash-dotted blue) or$\varPhi_{\rm{S}}=\pi/2$ (dashed green) after an evolution time t. Here, we specify the bare coupling strength$g_{0}/({2\pi})=4$ MHz. -
[1] Feynman R P 1982 Int. J. Theor. Phys. 21 467
Google Scholar
[2] Georgescu I M, Ashhab S, Nori F 2014 Rev. Mod. Phys. 86 153
Google Scholar
[3] Berry M V 1984 Proc. R. Soc. A 392 45
Google Scholar
[4] Hatsugai Y 1993 Phys. Rev. B 48 11851
[5] Tsui D C, Stormer H L, Gossard A C 1982 Phys. Rev. Lett. 48 1559
Google Scholar
[6] Laughlin R B 1983 Phys. Rev. Lett. 50 1395
Google Scholar
[7] Kane C L, Mele E J 2005 Phys. Rev. Lett. 95 146802
Google Scholar
[8] Bernevig B A, Hughes T L, Zhang S C 2006 Science 314 1757
Google Scholar
[9] Bernevig B A, Zhang S C 2006 Phys. Rev. Lett. 96 106802
Google Scholar
[10] Konig M, Wiedmann S, Brune C, Roth A, Buhmann H, Molenkamp L W, Qi X L, Zhang S C 2007 Science 318 766
Google Scholar
[11] Hsieh D, Qian D, Wray L, Xia Y, Hor Y S, Cava R J, Hasan M Z 2008 Nature 452 970
Google Scholar
[12] Chiu C K, Teo J C Y, Schnyder A P, Ryu S 2016 Rev. Mod. Phys. 88 035005
Google Scholar
[13] Goldman N, Juzeliunas G, Oehberg P, Spielman I B 2014 Rep. Prog. Phys. 77 126401
Google Scholar
[14] Lin Y J, Compton R L, Perry A R, Phillips W D, Porto J V, Spielman I B 2009 Phys. Rev. Lett. 102 130401
Google Scholar
[15] Lin Y J, Compton R L, Jimenez-Garcia K, Porto J V, Spielman I B 2009 Nature 462 628
Google Scholar
[16] Lin Y J, Compton R L, Jimenez-Garcia K, Phillips W D, Porto J V, Spielman I B 2011 Nat. Phys. 7 531
Google Scholar
[17] Aidelsburger M, Atala M, Nascimbene S, Trotzky S, Chen Y A, Bloch I 2011 Phys. Rev. Lett. 107 255301
Google Scholar
[18] Aidelsburger M, Atala M, Lohse M, Barreiro J T, Paredes B, Bloch I 2013 Phys. Rev. Lett. 111 185301
Google Scholar
[19] Krantz P, Kjaergaard M, Yan F, Orlando T P, Gustavsson S, Oliver W D 2019 Appl. Phys. Rev. 6 021318
Google Scholar
[20] Gu X, Kockum A F, Miranowicz A, Liu Y X, Nori F 2017 Phys. Rep. 718 1
Google Scholar
[21] Blais A, Grimsmo A L, Girvin S M, Wallraff A 2021 Rev. Mod. Phys. 93 025005
Google Scholar
[22] Nakamura Y, Pashkin Y A, Tsai J S 1999 Nature 398 786
Google Scholar
[23] Leek P J, Fink J M, Blais A, Bianchetti R, Göppl M, Gambetta J M, Schuster D I, Frunzio L, Schoelkopf R J, Wallraff A 2007 Science 318 1889
Google Scholar
[24] Möttönen M, Vartiainen J J, Pekola J P 2008 Phys. Rev. Lett. 100 177201
Google Scholar
[25] Neeley M, Ansmann M, Bialczak R C, Hofheinz M, Lucero E, O'Connell A D, Sank D, Wang H, Wenner J, Cleland A N, Geller M R, Martinis J M 2009 Science 325 722
Google Scholar
[26] Schroer M D, Kolodrubetz M H, Kindel W F, Sandberg M, Gao J, Vissers M R, Pappas D P, Polkovnikov A, Lehnert K W 2014 Phys. Rev. Lett. 113 050402
Google Scholar
[27] Roushan P, Neill C, Chen Y, et al. 2014 Nature 515 241
Google Scholar
[28] Barends R, Kelly J, Megrant A, et al. 2014 Nature 508 500
Google Scholar
[29] Tangpanitanon J, Bastidas V M, Al-Assam S, Roushan P, Jaksch D, Angelakis D G 2016 Phys. Rev. Lett. 117 213603
Google Scholar
[30] Alaeian H, Chang C W S, Moghaddam M V, Wilson C M, Solano E, Rico E 2019 Phys. Rev. A 99 053834
Google Scholar
[31] Zhao Y J, Xu X W, Wang H, Liu Y X, Liu W M 2020 Phys. Rev. A 102 053722
Google Scholar
[32] Koch J, Houck A A, Hur K L, Girvin S M 2010 Phys. Rev. A 82 043811
Google Scholar
[33] Yang Z H, Wang Y P, Xue Z Y, Yang W L, Hu Y, Gao J H, Wu Y 2016 Phys. Rev. A 93 062319
Google Scholar
[34] Roushan P, Neill C, Megrant A, et al. 2017 Nat. Phys. 13 146
Google Scholar
[35] Chen Y, Neill C, Roushan P, Leung N, Fang M, Barends R, Kelly J, Campbell B, Chen Z, Chiaro B 2014 Phys. Rev. Lett. 113 220502
Google Scholar
[36] Geller M R, Donate E, Chen Y, Neill C, Roushan P, Martinis J M 2015 Phys. Rev. A 92 012320
Google Scholar
[37] Berkovits R 2013 Phys. Rev. B 87 075141
Google Scholar
[38] Luttinger J M 1951 Phys. Rev. 84 814
Google Scholar
[39] Ahmed S, Muoz C S, Nori F, Kockum A F 2021 Phys. Rev. Lett. 127 140502
Google Scholar
下载:
计量
- 文章访问数: 3146
- PDF下载量: 138
- 被引次数: 0
