自由场中大尺寸有源微穿孔板吸声器的低频吸声性能

王蕾; 马玺越; 陈克安; 刘韬

doi:10.7498/aps.72.20222151

摘要

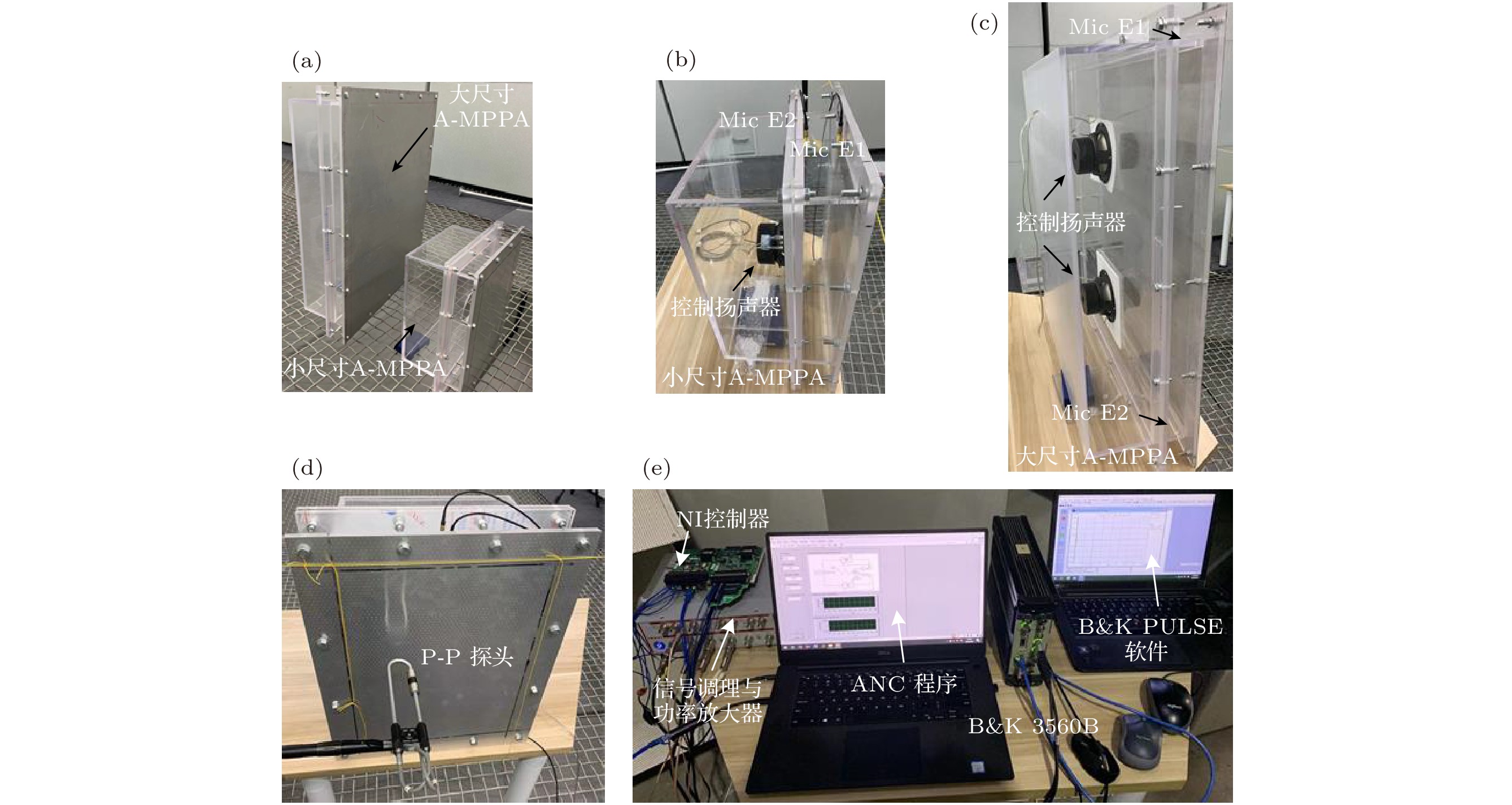
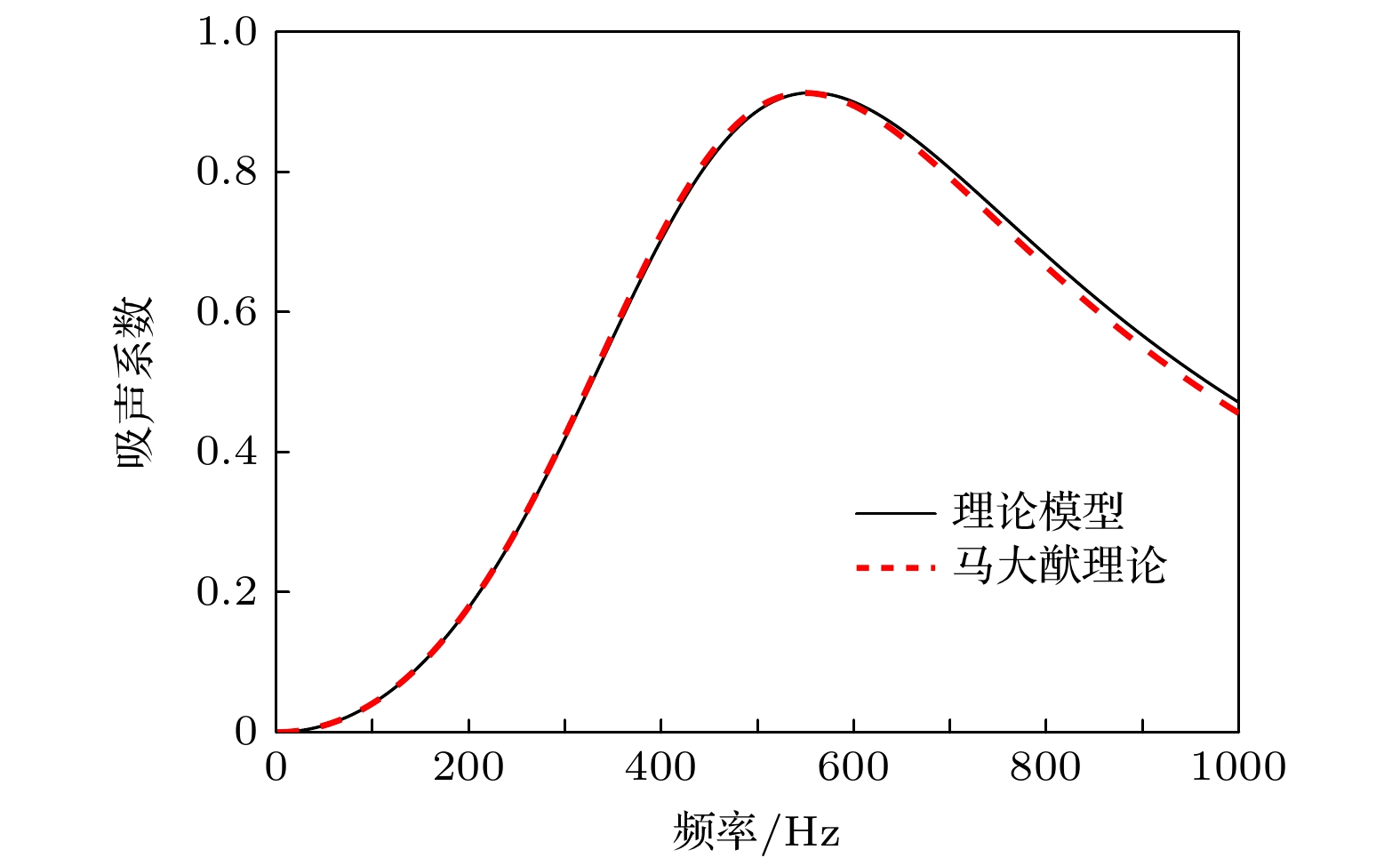
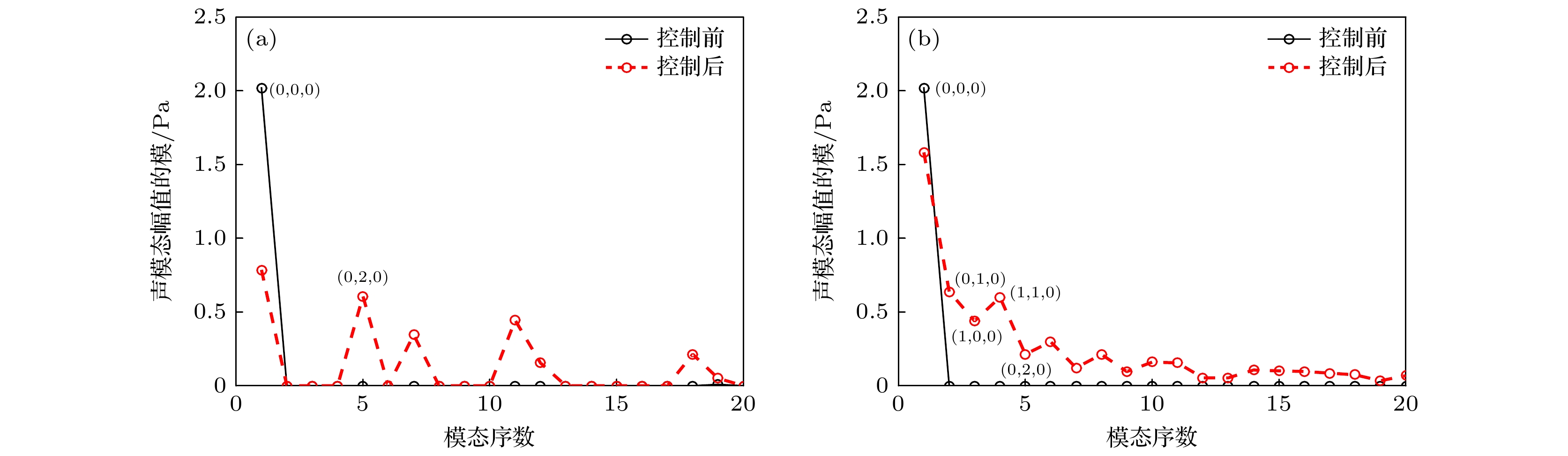
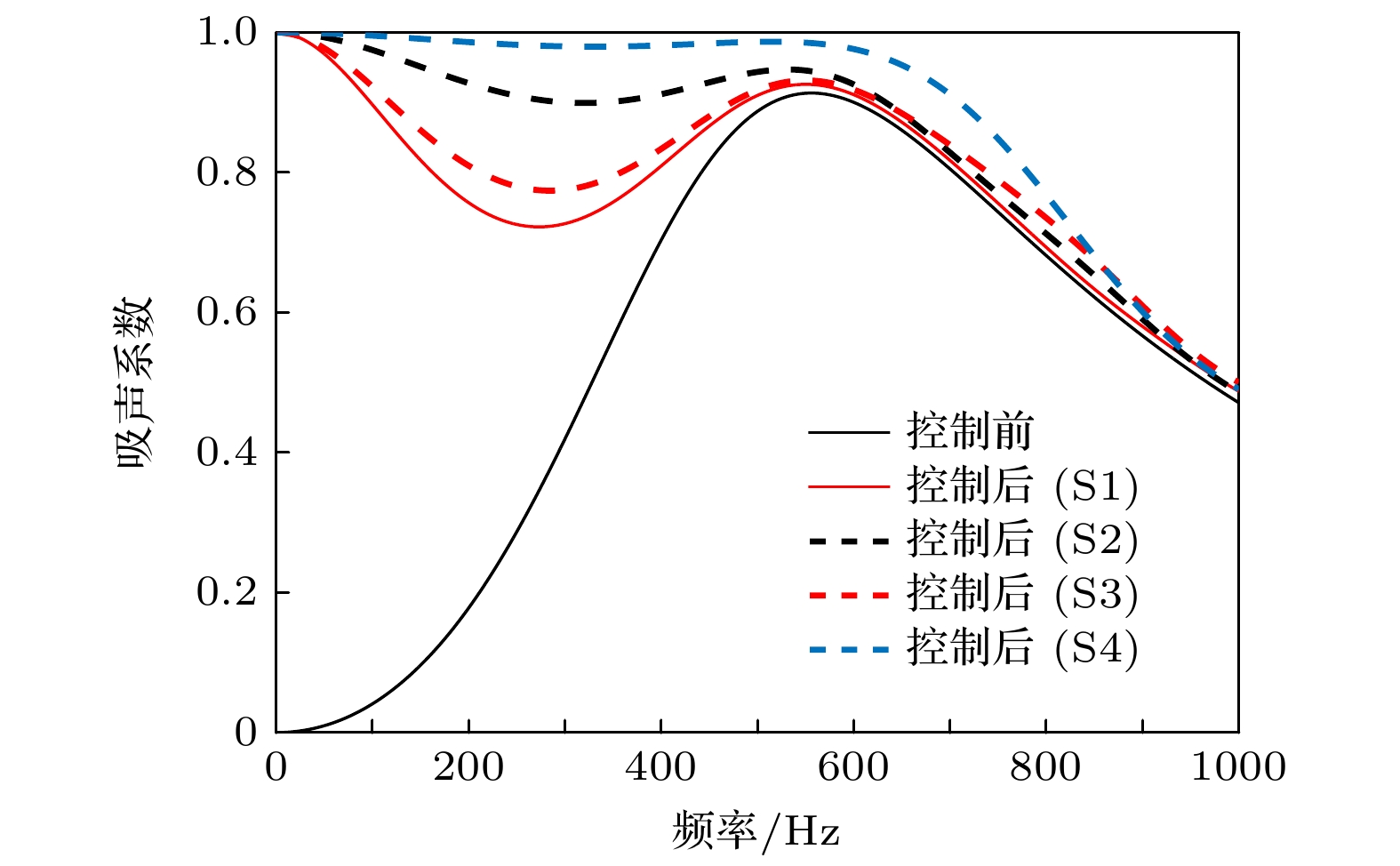
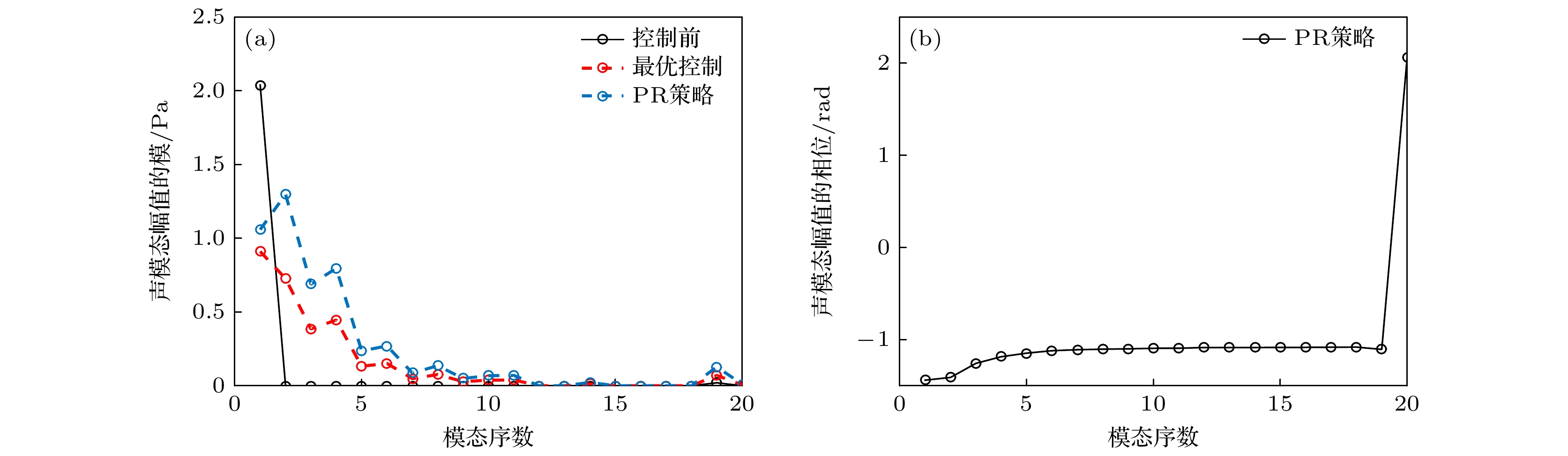
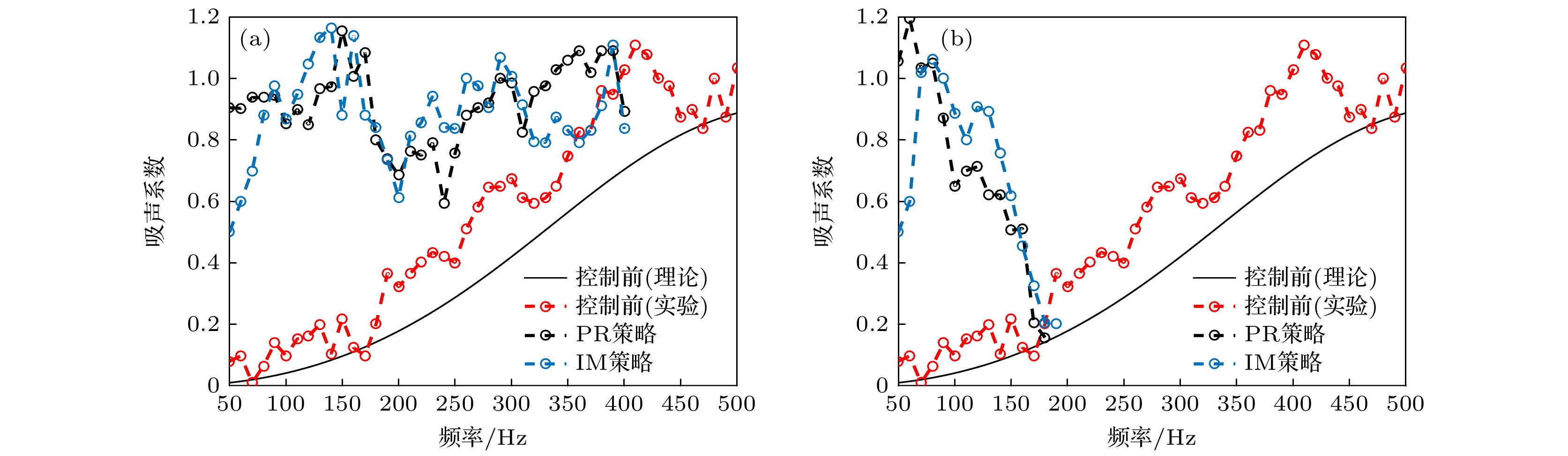
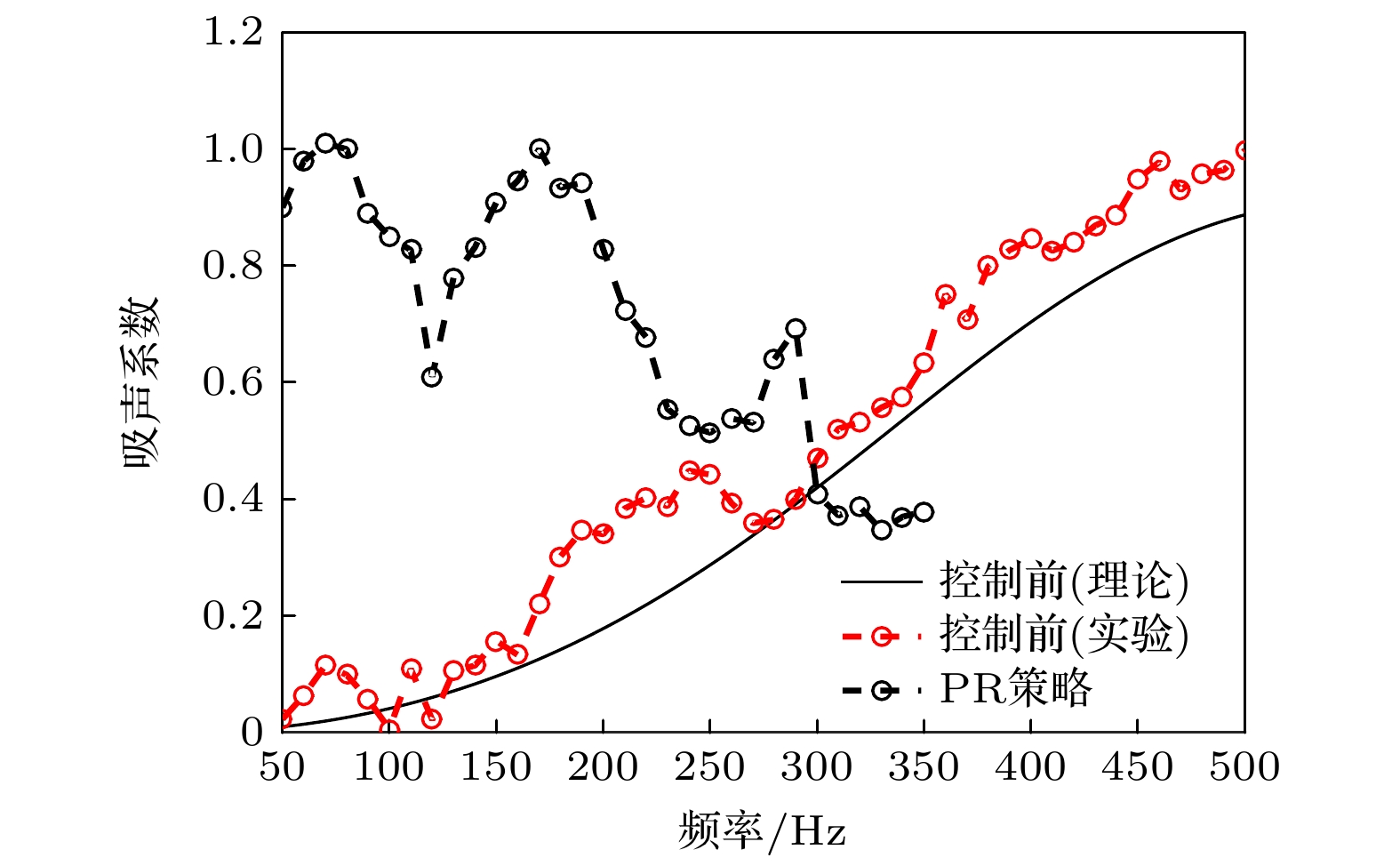
大尺寸有源微穿孔板吸声器的低频吸声性能依赖于入射声场环境, 故在现有管道声场的研究基础上, 探究了其在自由场环境中的有源吸声性能. 首先, 引入随位置变化的声压反射系数表征非局部反应表面在垂直入射平面波激励下的反射声场, 并结合模态分析法建立理论模型. 其次, 从空腔声模态对入射波的反射作用及对声吸收的贡献度揭示了自由场中有源吸声的物理机制, 构建了误差传感策略. 最后, 实验验证了理论模型与所获结论的正确性. 研究表明: 入射波激励起的(0, 0, 0)空腔声模态, 其幅值越大对入射波的反射作用越强; 控制源抑制该声模态并使其幅值降到最优值时, 它不再反射并会大幅吸收入射波, 低频吸声性能显著提升. 但控制源激发的高阶空腔声模态对入射波起完全反射作用, 将阻碍控制性能的提升. 故相比于管道声场, 自由场中的有源吸声性能有所减弱. 控制源在抑制(0, 0, 0)声模态的同时能确保不大幅激起高阶声模态时, 声压释放和阻抗匹配传感策略对自由场环境仍然适用.

关键词:

Abstract

The active micro-perforated panel absorber has excellent low frequency sound absorption performance, which is expected to realize low-frequency noise reduction in large space of the cabin. Since its active sound absorption performance depends on the incident sound field environment, on the basis of the existing research conclusions in the duct, the active sound absorption performance of the large-sized active micro-perforated panel absorber under the excitation of a normal incident plane wave in typical free field environment is in depth investigated. First, the theoretical model of the active micro-perforated panel absorber is established by using the modal analysis approach, in which a reflection coefficient varying with position is introduced to represent the reflected sound wave on the surface of the active micro-perforated panel absorber in free field. Then, the physical mechanism of active control is thoroughly analyzed and the error sensing strategy is established. Finally, an experiment is carried out to validate the theoretical model and findings. Results obtained demonstrate that the greater the amplitude of the (0, 0, 0) cavity mode excited by the incident plane wave, the stronger the reflection effect on the incident sound wave is, and vice versa. The control source suppresses the (0, 0, 0) mode so that this mode will not reflect and absorb the incident plane wave substantially when its amplitude is reduced to an optimal value. This is main mechanism of the sound absorption improvement in the low frequency range. However, the excited high order cavity modes (except for (0, 0, 0) mode) greatly reflect the incident sound energy in free field and exert a negative effect on sound absorption improvement. Thus, the control performance of the active micro-perforated panel absorber weakens in free field in comparison with that in the duct. The pressure-release and impedance-matching strategies are still applicable to free field as long as such a situation holds, i.e. the (0, 0, 0) cavity mode can be substantially suppressed by the control source and at the same time the high order cavity modes cannot be highly excited.

Keywords:

作者及机构信息

1.
西安建筑科技大学理学院, 西安　710055

2.
西北工业大学航海学院, 西安　710072

通信作者: 马玺越, xiyuema@nwpu.edu.cn

基金项目: 国家自然科学基金青年科学基金(批准号: 51705421)、陕西省自然科学基础研究计划(批准号: 2018JQ1025)和中国博士后科学基金(批准号: 2019M663821)资助的课题.

Authors and contacts

1.
School of Science, Xi’an University of Architecture and Technology, Xi’an 710055, China

2.
School of Marine Science and Technology, Northwestern Polytechnical University, Xi’an 710072, China

Corresponding author: Ma Xi-Yue, xiyuema@nwpu.edu.cn

Funds: Project supported by the Young Scientists Fund of the National Natural Science Foundation of China (Grant No. 51705421), the Natural Science Basic Research Plan in Shaanxi Province of China (Grant No. 2018JQ1025), and the China Postdoctoral Science Foundation (Grant No. 2019M663821).

文章全文 : translate this paragraph

参考文献

[1]	Maa D Y 1998 J. Acoust. Soc. Am. 104 2861 Google Scholar
[2]	Zhang Z M, Gu X T 1998 J. Sound Vib. 215 399 Google Scholar
[3]	Sakagami K, Nakamori T, Morimoto M, Yairi M 2009 Appl. Acoust. 70 703 Google Scholar
[4]	Sakagami K, Matsutani K, Morimoto M 2010 Appl. Acoust. 71 411 Google Scholar
[5]	Lee D H, Kwon Y P 2004 J. Sound Vib. 278 847 Google Scholar
[6]	Ruiz H, Cobo P, Jacobsen F 2011 Appl. Acoust. 72 772 Google Scholar
[7]	Tayong R B, Manyo J A, Siryabe E, Ntamack G E 2018 J. Acoust. Soc. Am. 143 2279 Google Scholar
[8]	Wang C, Huang L 2011 J. Acoust. Soc. Am. 130 208 Google Scholar
[9]	Wang C, Huang L, Zhang Y 2014 J. Sound Vib. 333 6828 Google Scholar
[10]	Wang C Q, Cheng L, Pan J, Yu G H 2010 J. Acoust. Soc. Am. 127 238 Google Scholar
[11]	Yang C, Cheng L, Pan J 2013 J. Acoust. Soc. Am. 133 201 Google Scholar
[12]	Bravo T, Maury C 2018 J. Sound Vib. 425 189 Google Scholar
[13]	Gai X, Li X, Zhang B, Xing T, Zhao J, Ma Z 2016 Appl. Acoust. 110 241 Google Scholar
[14]	Gai X, Xing T, Li X, Zhang B 2018 Appl. Acoust. 137 98 Google Scholar
[15]	Gai X, Xing T, Li X, Zhang B, Wang W 2016 Appl. Acoust. 114 260 Google Scholar
[16]	Toyoda M, Takahashi D 2008 J. Acoust. Soc. Am. 124 3594 Google Scholar
[17]	Liu J, Herrin D W 2010 Appl. Acoust. 71 120 Google Scholar
[18]	Xie S, Wang D, Feng Z, Yang S 2020 Appl. Acoust. 158 1 Google Scholar
[19]	Park S 2013 J. Sound Vib. 332 4895 Google Scholar
[20]	Zhao X, Fan X 2015 Appl. Acoust. 88 123 Google Scholar
[21]	Tao J, Jing R, Qiu X 2014 J. Acoust. Soc. Am. 135 231 Google Scholar
[22]	Cobo P, Pfretzschner J, Cuesta M, Anthony D K 2004 J. Acoust. Soc. Am. 116 2118 Google Scholar
[23]	Cobo P, Cuesta M 2007 J. Acoust. Soc. Am. 121 EL251 Google Scholar
[24]	Cobo P, Cuesta M 2009 J. Acoust. Soc. Am. 125 185 Google Scholar
[25]	Cobo P, Fernandez A, Doutres O 2003 J. Acoust. Soc. Am. 114 3211 Google Scholar
[26]	Ma X Y, Chen K A, Wang L, Liu Y, Ding S 2022 Appl. Acoust. 185 108424 Google Scholar
[27]	Ma X Y, Yurchenko D, Chen K A, Wang L, Liu Y, Yang K 2022 Mech. Syst. Signal Proc. 178 109295 Google Scholar
[28]	Ma X Y, Chen K A, Wang L, Liu Y 2021 Shock Vib. 2021 6691505 Google Scholar
[29]	邹海山, 邱小军 2019 68 054301 Google Scholar Zou H S, Qiu X J 2019 Acta Phys. Sin. 68 054301 Google Scholar
[30]	吴飞, 黄威, 陈文渊, 肖勇, 郁殿龙, 温激鸿 2020 69 134303 Google Scholar Wu F, Huang W, Chen W Y, Xiao Y, Yu D L, Wen J H 2020 Acta Phys. Sin. 69 134303 Google Scholar
[31]	张丰辉, 唐宇帆, 辛锋先, 卢天健 2018 67 234302 Google Scholar Zhang F H, Tang Y F, Xin F X, Lu T J 2018 Acta Phys. Sin. 67 234302 Google Scholar
[32]	Lee Y Y, Lee E W M, Ng C F 2005 J. Sound Vib. 287 227 Google Scholar
[33]	Bravo T, Maury C, Pinhede C 2012 J. Acoust. Soc. Am. 132 789 Google Scholar
[34]	马玺越, 陈克安, 丁少虎, 张冰瑞 2013 62 124301 Google Scholar Ma X Y, Chen K A, Ding S H, Zhang B R 2013 Acta Phys. Sin. 62 124301 Google Scholar
[35]	Bravo T, Maury C, Pinhede C 2012 J. Acoust. Soc. Am. 131 3853 Google Scholar

施引文献

图 1 A-MPPA侧视图　(a) 控制源为点源模型; (b) 控制源为活塞源模型

Fig. 1. Sketch of the A-MPPA: (a) The control source is point source; (b) the control source is piston source.

下载: 全尺寸图片幻灯片

图 2 理论模型的结果与马大猷理论所获结果的对比

Fig. 2. Sound absorption coefficients obtained by the theoretical model and Maa’s theory.

下载: 全尺寸图片幻灯片

图 3 点源位于不同位置时A-MPPA的控制结果(包括管道中的控制结果)　(a) S1; (b) S2; (c) S3; (d) S4

Fig. 3. Control results of the A-MPPA for different position of the point source (including the control results in the duct): (a) S1; (b) S2; (c) S3; (d) S4.

下载: 全尺寸图片幻灯片

图 4 点源与活塞源的布放位置　(a) 点源; (b) 活塞源

Fig. 4. Arrangement of the point source and the piston source: (a) Point source; (b) piston source.

下载: 全尺寸图片幻灯片

图 5 前四个高阶空腔声模态沿x-y平面的模态振型分布图($ X $与$ Y $代表A-MPPA的长度与宽度)

Fig. 5. Mode shape of the first four high order cavity modes along x-y plane ($ X $ and $ Y $are the length and width of the A-MPPA).

下载: 全尺寸图片幻灯片

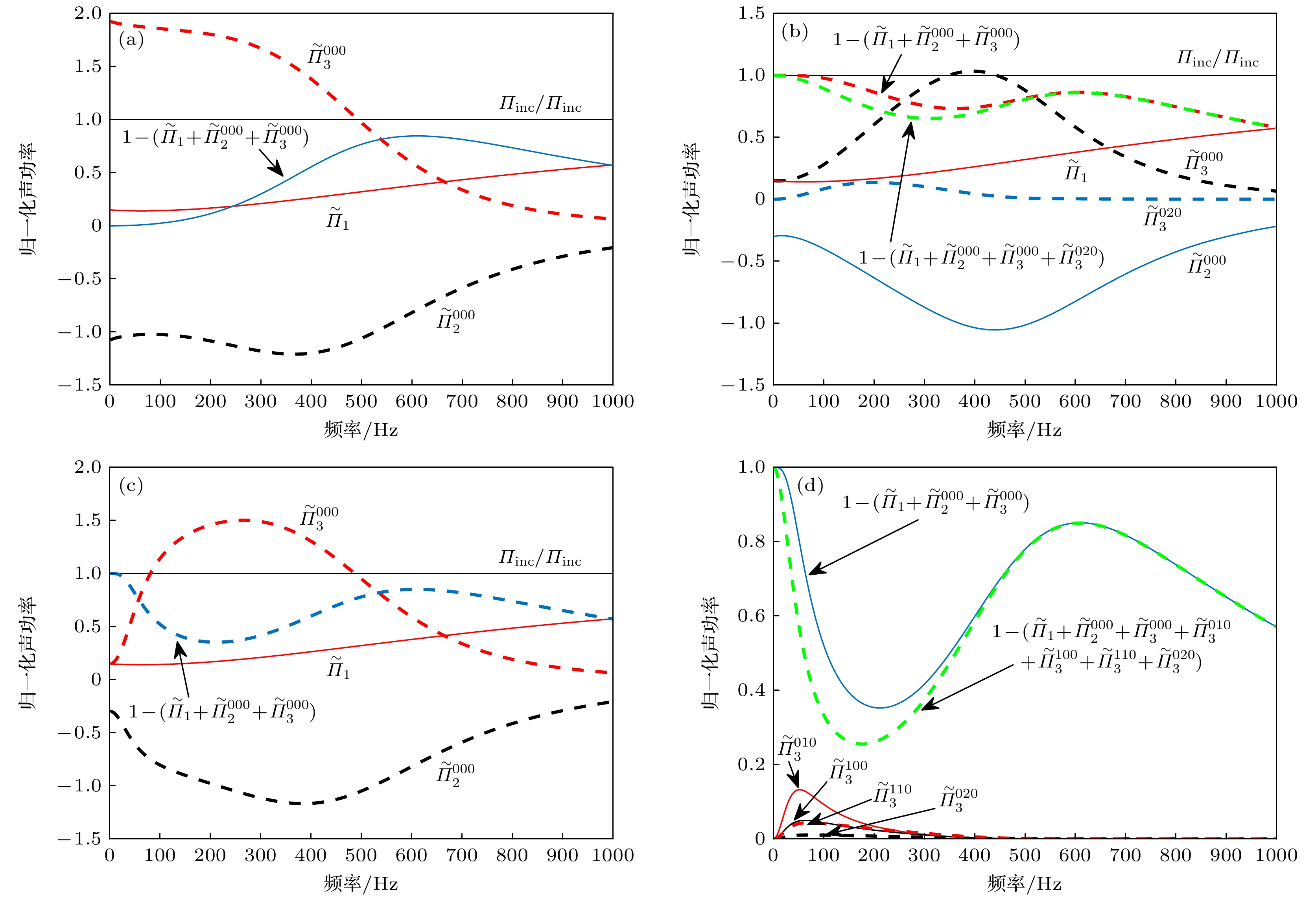
图 6 点源分别位于S1和S4时控制前后的归一化声功率$ {\tilde \varPi _1} $, $ {\tilde \varPi _{\text{2}}} $, $ {\tilde \varPi _3} $与$ 1 - ({\tilde \varPi _1} + {\tilde \varPi _2} + {\tilde \varPi _3}) $　(a) 控制前; (b) 点源位于S4时控制后; (c) 与(d)点源位于S1时控制后

Fig. 6. Normalized sound power $ {\tilde \varPi _1} $, $ {\tilde \varPi _1} $, $ {\tilde \varPi _3} $, and $ 1 - ({\tilde \varPi _1} + {\tilde \varPi _2} + {\tilde \varPi _3}) $ with and without control when the point source locates at S1 and S4: (a) Without control; (b) with control when the point source locates at S4; (c) and (d) with control when the point source locates at S1.

下载: 全尺寸图片幻灯片

图 7 点源分别位于S1与S4时控制前后空腔声模态幅值的模　(a) 点源位于S4; (b) 点源位于S1

Fig. 7. Module of the amplitude of the cavity mode with and without control when the point source locates at S1 and S4: (a) S4; (b) S1

下载: 全尺寸图片幻灯片

图 8 尺寸为0.3 m×0.4 m的A-MPPA中不同活塞源位置下的控制结果

Fig. 8. Control results of the A-MPPA (0.3 m×0.4 m) for different position of the piston source.

下载: 全尺寸图片幻灯片

图 9 活塞源分别位于S1与S4时PR与IM策略的控制结果　(a) S4; (b) S1

Fig. 9. Control results of the PR and IM strategies when the piston source locates at S1 and S4: (a) S4; (b) S1.

下载: 全尺寸图片幻灯片

图 10 (a) PR策略控制后空腔声模态幅值的模与最优控制状态下的结果对比; (b) PR策略控制后空腔声模态幅值的相位

Fig. 10. (a) Comparison between the modulus of the cavity mode amplitude under the control of PR strategy and the result of the optimal control state; (b) the phase of the cavity mode amplitude under the control of PR strategy.

下载: 全尺寸图片幻灯片

图 11 PR策略中点源与传声器的布局

Fig. 11. Layout of the point sources and the sensing microphones for PR strategy.

下载: 全尺寸图片幻灯片

图 12 PR策略的控制结果

Fig. 12. Control result of the PR strategy.

下载: 全尺寸图片幻灯片

图 13 实验原理示意图

Fig. 13. Schematic diagram of experimental principle.

下载: 全尺寸图片幻灯片

图 14 实验设备与系统　(a) A-MPPA样品; (b) 小尺寸A-MPPA; (c) 大尺寸A-MPPA; (d) P-P探头; (e) 测量与控制系统

Fig. 14. Experimental setup and system: (a) The sample of the A-MPPA; (b) the small sized A-MPPA; (c) the large sized A-MPPA; (d) the P-P probe; (e) the measurement and control system.

下载: 全尺寸图片幻灯片

图 15 小尺寸A-MPPA的实验结果　(a) 活塞源位于S4; (b) 活塞源位于S1

Fig. 15. Experimental results of the small sized A-MPPA: (a) The piston source locates at S4; (b) the piston source locates at S1.

下载: 全尺寸图片幻灯片

图 16 大尺寸A-MPPA的 PR策略实验结果

Fig. 16. Experimental results of the PR strategy for the large sized A-MPPA.

下载: 全尺寸图片幻灯片

表 1 模型的几何参数

Table 1. Geometric parameters of the model.

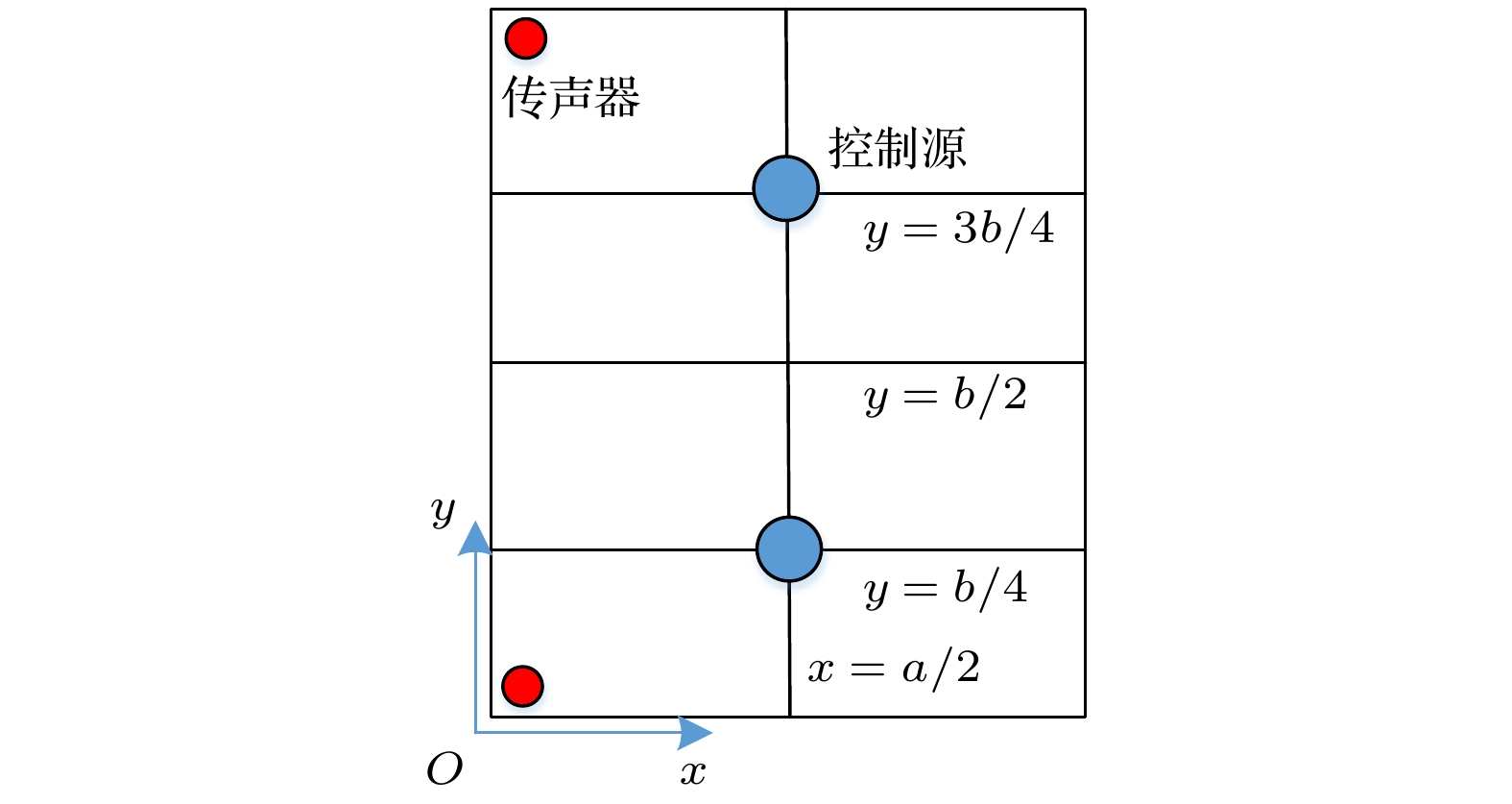
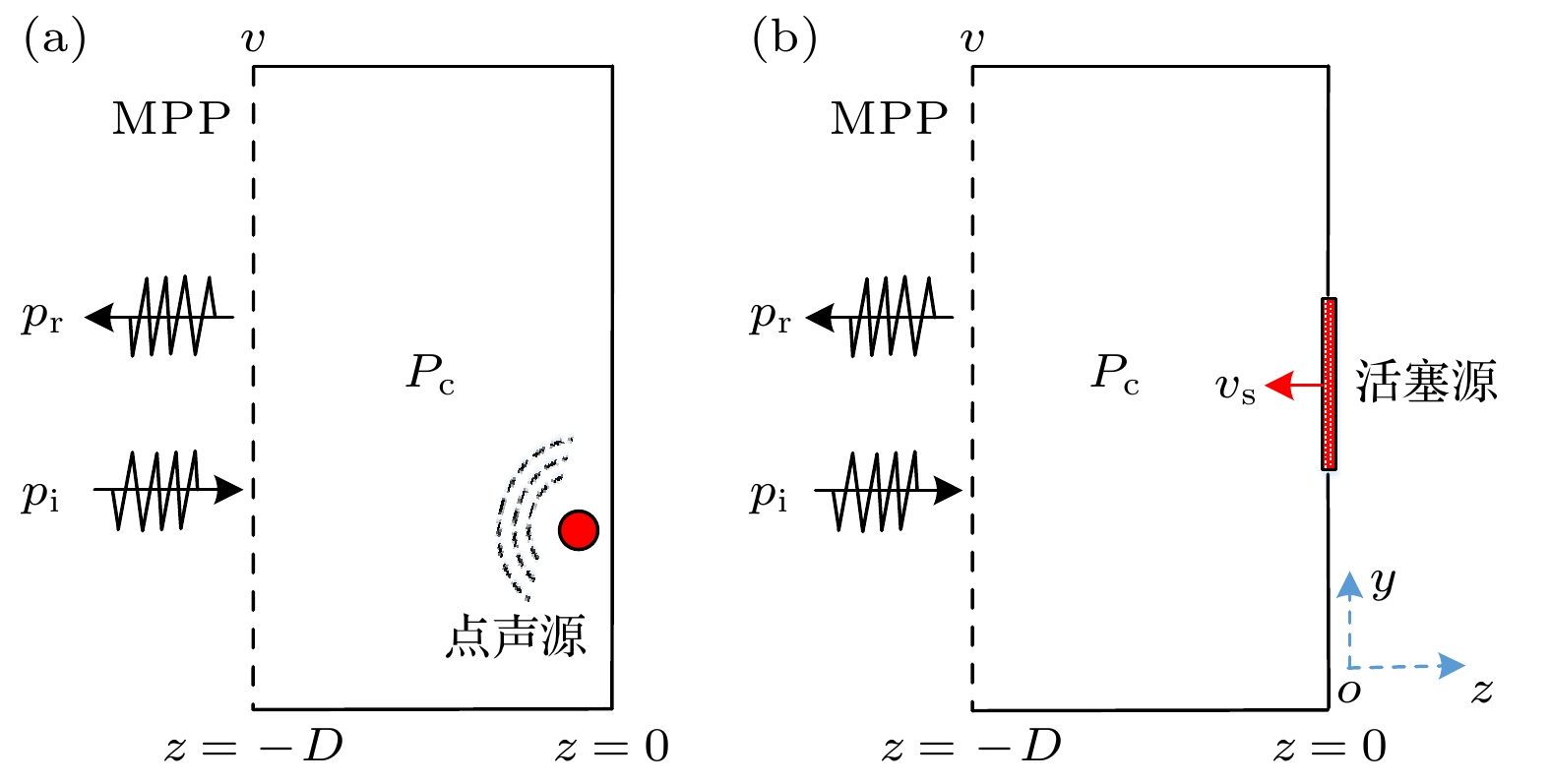
参数	数值
A-MPPA尺寸(长×宽)	0.3 m×0.4 m (活塞源)0.6 m×0.8 m (点声源)
MPP的孔隙直径/m	$ 0.4 \times {10^{ - 3}} $
MPP的厚度/m	$ 0.5 \times {10^{ - 3}} $
MPP的孔隙率/%	1
A-MPPA的空腔深度/m	0.08
空气的黏度系数/(Pa·s)	1.882×10^–5

下载: 导出CSV

表 2 点源与活塞源的位置

Table 2. Position of the point source and the piston source.

位置	坐标/m
位置	点源 (0.6 m×0.8 m)	活塞源的中心位置 (0.3 m×0.4 m)
S1	(0.01, 0.01, –0.001)	(0.05, 0.05, 0)
S2	(0.01, 0.4, –0.001)	(0.05, 0.2, 0)
S3	(0.3, 0.01, –0.001)	(0.15, 0.05, 0)
S4	(0.3, 0.4, –0.001)	(0.15, 0.2, 0)

下载: 导出CSV

表 3 两种尺寸下A-MPPA中空腔前五阶声模态的共振频率

Table 3. Resonant frequencies of the first five cavity modes for these two cases of A-MPPA size.

序号	空腔声模态	共振频率/Hz
序号	空腔声模态	0.6 m×0.8 m	0.3 m×0.4 m
1	(0, 0, 0)	0	0
2	(0, 1, 0)	215	430
3	(1, 0, 0)	287	574
4	(1, 1, 0)	358	716
5	(0, 2, 0)	430	860

下载: 导出CSV

map

[1]	Maa D Y 1998 J. Acoust. Soc. Am. 104 2861 Google Scholar
[2]	Zhang Z M, Gu X T 1998 J. Sound Vib. 215 399 Google Scholar
[3]	Sakagami K, Nakamori T, Morimoto M, Yairi M 2009 Appl. Acoust. 70 703 Google Scholar
[4]	Sakagami K, Matsutani K, Morimoto M 2010 Appl. Acoust. 71 411 Google Scholar
[5]	Lee D H, Kwon Y P 2004 J. Sound Vib. 278 847 Google Scholar
[6]	Ruiz H, Cobo P, Jacobsen F 2011 Appl. Acoust. 72 772 Google Scholar
[7]	Tayong R B, Manyo J A, Siryabe E, Ntamack G E 2018 J. Acoust. Soc. Am. 143 2279 Google Scholar
[8]	Wang C, Huang L 2011 J. Acoust. Soc. Am. 130 208 Google Scholar
[9]	Wang C, Huang L, Zhang Y 2014 J. Sound Vib. 333 6828 Google Scholar
[10]	Wang C Q, Cheng L, Pan J, Yu G H 2010 J. Acoust. Soc. Am. 127 238 Google Scholar
[11]	Yang C, Cheng L, Pan J 2013 J. Acoust. Soc. Am. 133 201 Google Scholar
[12]	Bravo T, Maury C 2018 J. Sound Vib. 425 189 Google Scholar
[13]	Gai X, Li X, Zhang B, Xing T, Zhao J, Ma Z 2016 Appl. Acoust. 110 241 Google Scholar
[14]	Gai X, Xing T, Li X, Zhang B 2018 Appl. Acoust. 137 98 Google Scholar
[15]	Gai X, Xing T, Li X, Zhang B, Wang W 2016 Appl. Acoust. 114 260 Google Scholar
[16]	Toyoda M, Takahashi D 2008 J. Acoust. Soc. Am. 124 3594 Google Scholar
[17]	Liu J, Herrin D W 2010 Appl. Acoust. 71 120 Google Scholar
[18]	Xie S, Wang D, Feng Z, Yang S 2020 Appl. Acoust. 158 1 Google Scholar
[19]	Park S 2013 J. Sound Vib. 332 4895 Google Scholar
[20]	Zhao X, Fan X 2015 Appl. Acoust. 88 123 Google Scholar
[21]	Tao J, Jing R, Qiu X 2014 J. Acoust. Soc. Am. 135 231 Google Scholar
[22]	Cobo P, Pfretzschner J, Cuesta M, Anthony D K 2004 J. Acoust. Soc. Am. 116 2118 Google Scholar
[23]	Cobo P, Cuesta M 2007 J. Acoust. Soc. Am. 121 EL251 Google Scholar
[24]	Cobo P, Cuesta M 2009 J. Acoust. Soc. Am. 125 185 Google Scholar
[25]	Cobo P, Fernandez A, Doutres O 2003 J. Acoust. Soc. Am. 114 3211 Google Scholar
[26]	Ma X Y, Chen K A, Wang L, Liu Y, Ding S 2022 Appl. Acoust. 185 108424 Google Scholar
[27]	Ma X Y, Yurchenko D, Chen K A, Wang L, Liu Y, Yang K 2022 Mech. Syst. Signal Proc. 178 109295 Google Scholar
[28]	Ma X Y, Chen K A, Wang L, Liu Y 2021 Shock Vib. 2021 6691505 Google Scholar
[29]	邹海山, 邱小军 2019 68 054301 Google Scholar Zou H S, Qiu X J 2019 Acta Phys. Sin. 68 054301 Google Scholar
[30]	吴飞, 黄威, 陈文渊, 肖勇, 郁殿龙, 温激鸿 2020 69 134303 Google Scholar Wu F, Huang W, Chen W Y, Xiao Y, Yu D L, Wen J H 2020 Acta Phys. Sin. 69 134303 Google Scholar
[31]	张丰辉, 唐宇帆, 辛锋先, 卢天健 2018 67 234302 Google Scholar Zhang F H, Tang Y F, Xin F X, Lu T J 2018 Acta Phys. Sin. 67 234302 Google Scholar
[32]	Lee Y Y, Lee E W M, Ng C F 2005 J. Sound Vib. 287 227 Google Scholar
[33]	Bravo T, Maury C, Pinhede C 2012 J. Acoust. Soc. Am. 132 789 Google Scholar
[34]	马玺越, 陈克安, 丁少虎, 张冰瑞 2013 62 124301 Google Scholar Ma X Y, Chen K A, Ding S H, Zhang B R 2013 Acta Phys. Sin. 62 124301 Google Scholar
[35]	Bravo T, Maury C, Pinhede C 2012 J. Acoust. Soc. Am. 131 3853 Google Scholar

[1]	王世场, 卢振洲, 梁燕, 王光义. N型局部有源忆阻器的神经形态行为. , 2022, 71(5): 050502. doi: 10.7498/aps.71.20212017
[2]	冯婕, 崔益豪, 李豫东, 文林, 郭旗. CMOS有源像素传感器辐射损伤对星敏感器星图识别影响机理与识别算法. , 2022, 71(18): 184208. doi: 10.7498/aps.71.20220894
[3]	杨生辉, 董明义, 渠超越, 田兴成, 董静, 吴冶, 马骁妍, 章红宇, 江晓山, 欧阳群, 李岚坤, 郑国恒. 基于单片有源像素传感器的探测模块测试研究. , 2021, 70(17): 170702. doi: 10.7498/aps.70.20210464
[4]	吴飞, 黄威, 陈文渊, 肖勇, 郁殿龙, 温激鸿. 基于微孔板与折曲通道的亚波长宽带吸声结构设计. , 2020, 69(13): 134303. doi: 10.7498/aps.69.20200368
[5]	黄清明, 陈珊珊, 张建青, 杨洋, 郑刚. 基于目标场点法和流函数的磁共振有源匀场线圈设计方法. , 2019, 68(19): 198301. doi: 10.7498/aps.68.20190612
[6]	张丰辉, 唐宇帆, 辛锋先, 卢天健. 微穿孔蜂窝-波纹复合声学超材料吸声行为. , 2018, 67(23): 234302. doi: 10.7498/aps.67.20181368
[7]	李红霞, 江阳, 白光富, 单媛媛, 梁建惠, 马闯, 贾振蓉, 訾月姣. 有源环形谐振腔辅助滤波的单模光电振荡器. , 2015, 64(4): 044202. doi: 10.7498/aps.64.044202
[8]	王燕, 邹男, 梁国龙. 强多途环境下水听器阵列位置近场有源校正方法. , 2015, 64(2): 024304. doi: 10.7498/aps.64.024304
[9]	汪波, 李豫东, 郭旗, 刘昌举, 文林, 任迪远, 曾骏哲, 玛丽娅. 质子辐射下互补金属氧化物半导体有源像素传感器暗信号退化机理研究. , 2015, 64(8): 084209. doi: 10.7498/aps.64.084209
[10]	胡海帆, 王颖, 陈杰, 赵士斌. 全三维电离粒子有源像素探测器优化仿真. , 2014, 63(10): 100702. doi: 10.7498/aps.63.100702
[11]	梁国龙, 庞福滨, 张光普. 吸声材料对水下小平台上矢量传感器声学特性的影响研究. , 2014, 63(3): 034303. doi: 10.7498/aps.63.034303
[12]	方伟, 宋鑫宏. 基于Voronoi图盲区的无线传感器网络覆盖控制部署策略. , 2014, 63(22): 220701. doi: 10.7498/aps.63.220701
[13]	汪波, 李豫东, 郭旗, 刘昌举, 文林, 玛丽娅, 孙静, 王海娇, 丛忠超, 马武英. 60Co-γ射线辐照CMOS有源像素传感器诱发暗信号退化的机理研究. , 2014, 63(5): 056102. doi: 10.7498/aps.63.056102
[14]	杨海滨, 李岳, 赵宏刚, 温激鸿, 温熙森. 一种含圆柱形谐振散射体的黏弹材料低频吸声机理研究. , 2013, 62(15): 154301. doi: 10.7498/aps.62.154301
[15]	马玺越, 陈克安, 丁少虎, 张冰瑞. 用于三层有源隔声结构误差传感的压电传感薄膜阵列及其优化设计. , 2013, 62(12): 124301. doi: 10.7498/aps.62.124301
[16]	殷敬伟, 杨森, 杜鹏宇, 余赟, 陈阳. 基于单矢量有源平均声强器的码分多址水声通信. , 2012, 61(6): 064302. doi: 10.7498/aps.61.064302
[17]	陈林辉, 饶长辉. 点源信标相关哈特曼-夏克波前传感器光斑偏移测量误差分析. , 2011, 60(9): 090701. doi: 10.7498/aps.60.090701
[18]	周梅, 赵德刚. 以弱p型为有源区的新型p-n结构GaN紫外探测器. , 2009, 58(10): 7255-7260. doi: 10.7498/aps.58.7255
[19]	徐刚毅, 李爱珍. 量子级联激光器有源核中界面声子的特性研究. , 2007, 56(1): 500-506. doi: 10.7498/aps.56.500
[20]	孙广荣, 魏荣爵. 甘蔗纤维板穿孔吸声的初步研究. , 1963, 19(3): 151-159. doi: 10.7498/aps.19.151

计量

文章访问数: 3496
PDF下载量: 77
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板