基于相对论自由电子的量子物理

李靖; 刘运全

doi:10.7498/aps.71.20221289

摘要

光和物质的相互作用是物理学中一个基本研究领域. 电子是最早被发现组成物质的基本粒子, 因此电子与光场(光子)的相互作用很早就引起人们的研究兴趣. 电子分为束缚电子与自由电子. 束缚电子系统的跃迁会受到能级固定、选择定则等约束, 自由电子则不然. 近十多年来, 随着超快电子显微镜技术的发展, 人们提出并发展了用于描述量子自由电子(电子波包)和光场相互作用的理论—基于光子诱导近场电子显微成像过程, 成功展示了许多新奇量子效应以及新应用. 目前, 人们把光子诱导近场电子显微拓展量子光学中并展示了许多新奇现象, 包括自由电子和腔光子的纠缠、自由电子和自由电子的纠缠、自由电子量子比特、新奇光量子态制备等，从而开启了基于自由电子的“量子光学”时代. 本文首先概述了电子与光子的相互作用研究, 随后综述了光子诱导近场电子显微成像的理论、实验进展, 介绍了其应用场景. 最后，我们对基于自由电子的量子物理研究目前遇到的困难进行了总结, 并对未来发展进行了展望.

关键词:

Abstract

The light-matter interaction is one of the fundamental research fields in physics. The electron is the first discovered elementary particle that makes up matter. Therefore, the interaction between electron and light field has long been the research interest of physicists. Electrons are divided into two kinds, i.e. bounded electrons and free electrons. The quantum transition of bounded electron system is constrained by the selection rules with the discrete energy levels, while the free electron systems are not. In the last decade, the experiments of photon-induced near-field electron microscopy (PINEM) have been demonstrated. The experimental setup of PINEM is based on ultrafast electron transmission microscopy (UTEM). The thoeritcal framworks have also been developed to describe the interaction between quantum free electrons and optical fields. Within macroscopic quantum electrodynamics, the concept of photon is extended to photonic quasi-particles. Solutions of maxwell's equations in medium that satisfy certain boundary conditions are called photonic quasiparticles, such as surface plasmon polaritons, phonon polaritons, or even magnetic field. The different dispersion relations of photonic quasi-particles produce abundant phenomena in the interaction between light and matter. The underlying information about the PINEM interaction can be inferred from the electron energy loss spectrum (EELS). It has been used for implementing the near-field imaging in its infancy. By now it is capable of not only realizing time-resolved dynamic imaging, reconstructing the dispersion relation of photonics crystal and its Bloch mode, but also measuring the mode lifetime directly. The PINEM has also been used to study free electron wavepacket reshaping, free electron comb, free electron attosecond pulse train, etc. Recently, this field has entered into the era of quantum optics, and people use PINEM to study novel phenomena in quantum optics, such as entanglement between free electrons and cavity photons, entanglement between free electrons and free electrons, free electron qubits, and preparation of novel light quantum states. In this paper, the theoretical and experimental development of free-electron quantum physics are reviewed. We have disscussed the application scenarios of quantum free electron system. The current difficulties and future development are envisaged.

Keywords:

photon-induced near field electron microscopy /
free electron /
quantum optics /
time-resolved imaging

作者及机构信息

李靖,
刘运全

北京大学物理学院, 人工微结构和介观物理国家重点实验室, 北京　100871

通信作者: 刘运全, yunquan.liu@pku.edu.cn

基金项目: 国家重点研发计划 (批准号: 2022YFA1604301)和国家自然科学基金(批准号: 92050201, 92250306) 资助的课题.

Authors and contacts

State Key Laboratory for Mesoscopic Physics and Collaborative Innovation Center of Quantum Matter, School of Physics, Peking University, Beijing 100871, China

Corresponding author: Liu Yun-Quan, yunquan.liu@pku.edu.cn

Funds: Project supported by the Key R&D Program of China (Grant No. 2022YFA1604301) and the National Natural Science Foundation of China (Grant Nos. 92050201, 92250306).

文章全文 : translate this paragraph

参考文献

[1]	Dirac P A M 1981 The Principles of Quantum Mechanics (London: Clarendon Press) pp204–207
[2]	Fox A M, Fox D P A M 2001 Optical Properties of Solids (New York: Oxford University Press) pp180–211
[3]	Vasa P 2020 Adv. Phys. X 5 1749884
[4]	Scully M O, Zubairy M S 1997 Quantum Optics (Cambridge: Cambridge University Press) pp145−217
[5]	Fox M, Fox D P A M 2006 Quantum Optics: An Introduction (New York: Oxford University Press) pp165–240
[6]	Walls D F, Milburn G J 2007 Quantum Optics (Heidelberg: Springer) pp197–211
[7]	Rivera N, Kaminer I 2020 Nat. Rev. Phys. 2 538 Google Scholar
[8]	Ashcroft N W, Mermin N D 1976 Solid State Physics (New York: Holt, Rinehart and Winston) pp131–150
[9]	Freimund D L, Aflatooni K, Batelaan H 2001 Nature 413 142 Google Scholar
[10]	Vanacore G M, Madan I, Berruto G, Wang K, Pomarico E, Lamb R J, McGrouther D, Kaminer I, Barwick B, Garcia de Abajo F J, Carbone F 2018 Nat. Commun. 9 2694 Google Scholar
[11]	Kaminer I, Katan Y T, Buljan H, Shen Y, Ilic O, Lopez J J, Wong L J, Joannopoulos J D, Soljacic M 2016 Nat. Commun. 7 11880 Google Scholar
[12]	Kaminer I, Mutzafi M, Levy A, Harari G, Herzig Sheinfux H, Skirlo S, Nemirovsky J, Joannopoulos J D, Segev M, Soljačić M 2016 Phys. Rev. X 6 011006
[13]	Gover A, Pan Y 2018 Phys. Lett. A 382 1550 Google Scholar
[14]	Lin X, Easo S, Shen Y, Chen H, Zhang B, Joannopoulos J D, Soljačić M, Kaminer I 2018 Nat. Phys. 14 816 Google Scholar
[15]	Pan Y, Gover A 2018 J. Phys. Commun. 2 115026 Google Scholar
[16]	Roques-Carmes C, Rivera N, Joannopoulos J D, Soljačić M, Kaminer I 2018 Phys. Rev. X 8 041013
[17]	Pan Y, Gover A 2019 Phys. Rev. A 99 052107 Google Scholar
[18]	Lin X, Hu H, Easo S, Yang Y, Shen Y, Yin K, Blago M P, Kaminer I, Zhang B, Chen H, Joannopoulos J, Soljacic M, Luo Y 2021 Nat. Commun. 12 5554 Google Scholar
[19]	Adiv Y, Hu H, Tsesses S, Dahan R, Wang K, Kurman Y, Gorlach A, Chen H, Lin X, Bartal G, Kaminer I 2022 arXiv: 2203.01698 [quant-ph]
[20]	Mizuno K, Ono S, Shimoe O 1975 Nature 253 184 Google Scholar
[21]	Tsesses S, Bartal G, Kaminer I 2017 Phys. Rev. A 95 013832 Google Scholar
[22]	Massuda A, Roques-Carmes C, Yang Y, Kooi S E, Yang Y, Murdia C, Berggren K K, Kaminer I, Soljačić M 2018 ACS Photonics 5 3513 Google Scholar
[23]	Rosolen G, Wong L J, Rivera N, Maes B, Soljacic M, Kaminer I 2018 Light Sci. Appl. 7 64 Google Scholar
[24]	Yang Y, Massuda A, Roques-Carmes C, Kooi S E, Christensen T, Johnson S G, Joannopoulos J D, Miller O D, Kaminer I, Soljačić M 2018 Nat. Phys. 14 894 Google Scholar
[25]	Remez R, Karnieli A, Trajtenberg-Mills S, Shapira N, Kaminer I, Lereah Y, Arie A 2019 Phys. Rev. Lett. 123 060401 Google Scholar
[26]	Roques-Carmes C, Kooi S E, Yang Y, Massuda A, Keathley P D, Zaidi A, Yang Y, Joannopoulos J D, Berggren K K, Kaminer I, Soljacic M 2019 Nat. Commun. 10 3176 Google Scholar
[27]	Jing L, Lin X, Wang Z, Kaminer I, Hu H, Li E, Liu Y, Chen M, Zhang B, Chen H 2021 Laser Photonics Rev. 15 2000426 Google Scholar
[28]	Karlovets D V, Pupasov-Maksimov A M 2021 Phys. Rev. A 103 012214 Google Scholar
[29]	Wong L J, Kaminer I 2021 Appl. Phys. Lett. 119 130502 Google Scholar
[30]	Yang Y, Roques-Carmes C, Kooi S E, Tang H, Beroz J, Mazur E, Kaminer I, Joannopoulos J D, Soljačić M 2021 arXiv: 2110.03550 [physics. optics]
[31]	Zhu J F, Du C H, Zhang Z W, Liu P K, Zhang L, Cross A W 2021 Opt. Lett. 46 4682 Google Scholar
[32]	Betzig E, Trautman J K 1992 Science 257 189 Google Scholar
[33]	Barwick B, Flannigan D J, Zewail A H 2009 Nature 462 902 Google Scholar
[34]	Di Giulio V, Kociak M, de Abajo F J G 2019 Optica 6 001524 Google Scholar
[35]	Kfir O 2019 Phys. Rev. Lett. 123 103602 Google Scholar
[36]	Reinhardt O, Mechel C, Lynch M, Kaminer I 2019 Conf Lasers Electro-Opt San Jose, California, USA, May 5, 2019 pFF1F.6
[37]	Di Giulio V, García de Abajo F J 2020 Optica 7 1820 Google Scholar
[38]	Ben Hayun A, Reinhardt O, Nemirovsky J, Karnieli A, Rivera N, Kaminer I 2021 Sci. Adv. 7 eabe4270 Google Scholar
[39]	Braiman G, Reinhardt O, Levi O, Mechel C, Kaminer I 2021 Conf Lasers Electro-Opt San Jose, California, USA, May 9, 2021 pFTh1N.6
[40]	Dahan R, Gorlach A, Haeusler U, Karnieli A, Eyal O, Yousefi P, Segev M, Arie A, Eisenstein G, Hommelhoff P, Kaminer I 2021 Science 373 eabj7128 Google Scholar
[41]	Gover A, Ran D, Zhang B, Pan Y M, Ianconescu R, Scheuer J, Friedman A, Yariv A 2021 Front Opt. Laser Sci. Washington DC, USA, November 1, 2021 pFTh6 D.1
[42]	Karnieli A, Rivera N, Arie A, Kaminer I 2021 Phys. Rev. Lett. 127 060403 Google Scholar
[43]	Reinhardt O, Mechel C, Lynch M, Kaminer I 2021 Ann. Phys. 533 2000254 Google Scholar
[44]	Rivera N, Sloan J, Kaminer I, Soljacic M 2021 arXiv: 2111.07010 [quant-ph]
[45]	Tsarev M V, Ryabov A, Baum P 2021 Phys. Rev. Res. 3 043033 Google Scholar
[46]	Zhang B, Ran D, Ianconescu R, Friedman A, Scheuer J, Yariv A, Gover A 2021 arXiv: 2111.13130
[47]	Baranes G, Ruimy R, Gorlach A, Kaminer I 2022 npj Quantum Inf. 8 32 Google Scholar
[48]	Bloch J, Cavalleri A, Galitski V, Hafezi M, Rubio A 2022 Nature 606 41 Google Scholar
[49]	Feist A, Huang G, Arend G, et al. 2022 arXiv: 2202.12821 [quant-ph]
[50]	Feist J, Fernández-Domínguez A I, García-Vidal F J 2021 Nanophotonics 10 477
[51]	Scheel S, Yoshi Buhmann S 2009 arXiv: 0902.3586 [quant-ph]
[52]	Henke J W, Raja A S, Feist A, et al. 2021 Nature 600 653 Google Scholar
[53]	Kfir O, Lourenco-Martins H, Storeck G, Sivis M, Harvey T R, Kippenberg T J, Feist A, Ropers C 2020 Nature 582 46 Google Scholar
[54]	Wang K, Dahan R, Shentcis M, Kauffmann Y, Ben Hayun A, Reinhardt O, Tsesses S, Kaminer I 2020 Nature 582 50 Google Scholar
[55]	Kurman Y, Dahan R, Sheinfux Hanan H, et al. 2021 Science 372 1181 Google Scholar
[56]	Barwick B, Park H S, Kwon O-H, Baskin J S, Zewail A H 2008 Science 322 1227 Google Scholar
[57]	Liu H, Baskin J S, Zewail A H 2016 Proc. Natl. Acad. Sci. U. S. A. 113 2041 Google Scholar
[58]	Sternbach A J, Chae S H, Latini S, et al. 2021 Science 371 617 Google Scholar
[59]	Yoxall E, Schnell M, Nikitin A Y, et al. 2015 Nat. Photonics 9 674 Google Scholar
[60]	Petek H, Ogawa S 1997 Prog. Surf. Sci. 56 239 Google Scholar
[61]	Davis T J, Janoschka D, Dreher P, Frank B, Heringdorf F J M z, Giessen H 2020 Science 368 eaba6415 Google Scholar
[62]	Balistreri M L M, Gersen H, Korterik J P, Kuipers L, Hulst N F v 2001 Science 294 1080 Google Scholar
[63]	Yamamoto N 2016 Microscopy 65 282 Google Scholar
[64]	Garcia de Abajo F J, Asenjo-Garcia A, Kociak M 2010 Nano Lett. 10 1859 Google Scholar
[65]	Park S T, Lin M, Zewail A H 2010 New J. Phys. 12 123028 Google Scholar
[66]	Park S T, Zewail A H 2012 J. Phys. Chem. A 116 11128 Google Scholar
[67]	Feist A, Echternkamp K E, Schauss J, Yalunin S V, Schafer S, Ropers C 2015 Nature 521 200 Google Scholar
[68]	Kociak M 2015 Nature 521 166 Google Scholar
[69]	Dahan R, Nehemia S, Shentcis M, Reinhardt O, Adiv Y, Shi X, Be’er O, Lynch M H, Kurman Y, Wang K, Kaminer I 2020 Nat. Phys. 16 1123 Google Scholar
[70]	Pan Y, Zhang B, Gover A 2019 Phys. Rev. Lett. 122 183204 Google Scholar
[71]	Kling P, Giese E, Endrich R, Preiss P, Sauerbrey R, Schleich W P 2015 New J. Phys. 17 123019 Google Scholar
[72]	Friedman A, Gover A, Kurizki G, Ruschin S, Yariv A 1988 Rev. Mod. Phys. 60 471 Google Scholar
[73]	Fu X, Barantani F, Gargiulo S, Madan I, Berruto G, LaGrange T, Jin L, Wu J, Vanacore G M, Carbone F, Zhu Y 2020 Nat. Commun. 11 5770 Google Scholar
[74]	Mkhitaryan V, Dias E J C, Carbone F, García de Abajo F J 2021 ACS Photonics 8 614 Google Scholar
[75]	Ruimy R, Gorlach A, Mechel C, Rivera N, Kaminer I 2021 Phys. Rev. Lett. 126 233403 Google Scholar
[76]	Echternkamp K E, Feist A, Schäfer S, Ropers C 2016 Nat. Phys. 12 1000 Google Scholar
[77]	Morimoto Y, Baum P 2017 Nat. Phys. 14 252
[78]	Priebe K E, Rathje C, Yalunin S V, Hohage T, Feist A, Schäfer S, Ropers C 2017 Nat. Photonics 11 793 Google Scholar
[79]	Cai W, Reinhardt O, Kaminer I, de Abajo F J G 2018 Phys. Rev. B 98 045424 Google Scholar
[80]	Kozak M, Schonenberger N, Hommelhoff P 2018 Phys. Rev. Lett. 120 103203 Google Scholar
[81]	Morimoto Y, Baum P 2018 Phys. Rev. A 97 033815 Google Scholar
[82]	Polman A, Kociak M, Garcia de Abajo F J 2019 Nat. Mater. 18 1158 Google Scholar
[83]	Vanacore G M, Berruto G, Madan I, et al. 2019 Nat. Mater. 18 573 Google Scholar
[84]	Harvey T R, Henke J W, Kfir O, Lourenco-Martins H, Feist A, Garcia de Abajo F J, Ropers C 2020 Nano Lett. 20 4377 Google Scholar
[85]	Reinhardt O, Kaminer I 2020 ACS Photonics 7 2859 Google Scholar
[86]	Garcia de Abajo F J, Konecna A 2021 Phys. Rev. Lett. 126 123901 Google Scholar
[87]	Kozák M 2021 ACS Photonics 8 431
[88]	Wong L J, Rivera N, Murdia C, Christensen T, Joannopoulos J D, Soljacic M, Kaminer I 2021 Nat. Commun. 12 1700 Google Scholar
[89]	Zhang B, Ran D, Ianconescu R, Friedman A, Scheuer J, Yariv A, Gover A 2021 arXiv: 2111.13130 [quant-ph]
[90]	Constantin Chirita Mihaila M, Weber P, Schneller M, Grandits L, Nimmrichter S, Juffmann T 2022 arXiv: 2203.07925 [physics. optics]
[91]	Madan I, Leccese V, Mazur A, Barantani F, La Grange T, Sapozhnik A, Gargiulo S, Rotunno E, Olaya J-C, Kaminer I, Grillo V, García de Abajo F J, Carbone F, Vanacore G M 2022 arXiv: 2206.02221 [quant-ph]
[92]	Pomarico E, Madan I, Berruto G, Vanacore G M, Wang K, Kaminer I, García de Abajo F J, Carbone F 2017 ACS Photonics 5 759
[93]	Dahan R, Baranes G, Gorlach A, Ruimy R, Rivera N, Kaminer I 2022 arXiv: 2206.08828 [quant-ph]
[94]	Ramsey N F 1950 Phys. Rev. 78 695 Google Scholar
[95]	Piazza L, Lummen T T, Quinonez E, Murooka Y, Reed B W, Barwick B, Carbone F 2015 Nat. Commun. 6 6407 Google Scholar
[96]	Yurtsever A, Baskin J S, Zewail A H 2012 Nano Lett. 12 5027 Google Scholar
[97]	Yurtsever A, Veen R M v d, Zewail A H 2012 Science 335 59 Google Scholar
[98]	Park S T, Yurtsever A, Baskin J S, Zewail A H 2013 Proc. Natl. Acad. Sci. U. S. A. 110 9277 Google Scholar
[99]	Schreiber A, Cassemiro K N, Potoček V, Gábris A, Jex I, Silberhorn C 2011 Phys. Rev. Lett. 106 180403 Google Scholar
[100]	Broome M A, Fedrizzi A, Lanyon B P, Kassal I, Aspuru-Guzik A, White A G 2010 Phys. Rev. Lett. 104 153602 Google Scholar
[101]	Harris N C, Steinbrecher G R, Prabhu M, et al. 2017 Nat. Photonics 11 447 Google Scholar
[102]	Dadras S, Gresch A, Groiseau C, Wimberger S, Summy G S 2018 Phys. Rev. Lett. 121 070402 Google Scholar
[103]	Karski M, Förster L, Choi J M, Steffen A, Alt W, Meschede D, Widera A 2009 Science 325 174 Google Scholar
[104]	Hong C K, Ou Z Y, Mandel L 1987 Phys. Rev. Lett. 59 2044 Google Scholar
[105]	Leonhardt U, Knight P L, Miller A 1997 Measuring the Quantum State of Light (Cambridge: Cambridge University Press)
[106]	Lvovsky A I, Raymer M G 2009 Rev. Mod. Phys. 81 299 Google Scholar
[107]	Asban S, García de Abajo F J 2021 npj Quantum Inf. 7 42 Google Scholar
[108]	Mutzafi M, Kaminer I, Harari G, Segev M 2017 Nat. Commun. 8 650 Google Scholar
[109]	Dikopoltsev A, Sharabi Y, Lyubarov M, Lumer Y, Tsesses S, Lustig E, Kaminer I, Segev M 2022 Proc. Natl. Acad. Sci. U.S.A. 119 6 e21197051
[110]	Feist J, Fernández-Domínguez A I, García-Vidal F J 2020 Nanophotonics 10 477 Google Scholar
[111]	Konecna A, de Abajo F J G 2020 Phys. Rev. Lett. 125 030801 Google Scholar
[112]	Schlawin F, Cavalleri A, Jaksch D 2019 Phys. Rev. Lett. 122 133602 Google Scholar
[113]	Turner A E, Johnson C W, Kruit P, McMorran B J 2021 Phys. Rev. Lett. 127 110401 Google Scholar
[114]	Shiloh R, Chlouba T, Hommelhoff P 2022 Phys. Rev. Lett. 128 235301 Google Scholar
[115]	Tsesses S, Dahan R, Wang K, Reinhardt O, Bartal G, Kaminer I 2022 arXiv: 2203.08518 [quant-ph]
[116]	Cox J D, Garcia de Abajo F J 2020 Nano Lett. 20 4792 Google Scholar
[117]	Konečná A, Di Giulio V, Mkhitaryan V, Ropers C, García de Abajo F J 2020 ACS Photonics 7 1290 Google Scholar
[118]	Talebi N 2020 Phys. Rev. Lett. 125 080401 Google Scholar
[119]	Luski A, Segev Y, David R, et al. 2021 Science 373 1105 Google Scholar
[120]	Akbari K, Di Giulio V, García de Abajo F J 2022 arXiv: 2203.07257 [physics. optics]

施引文献

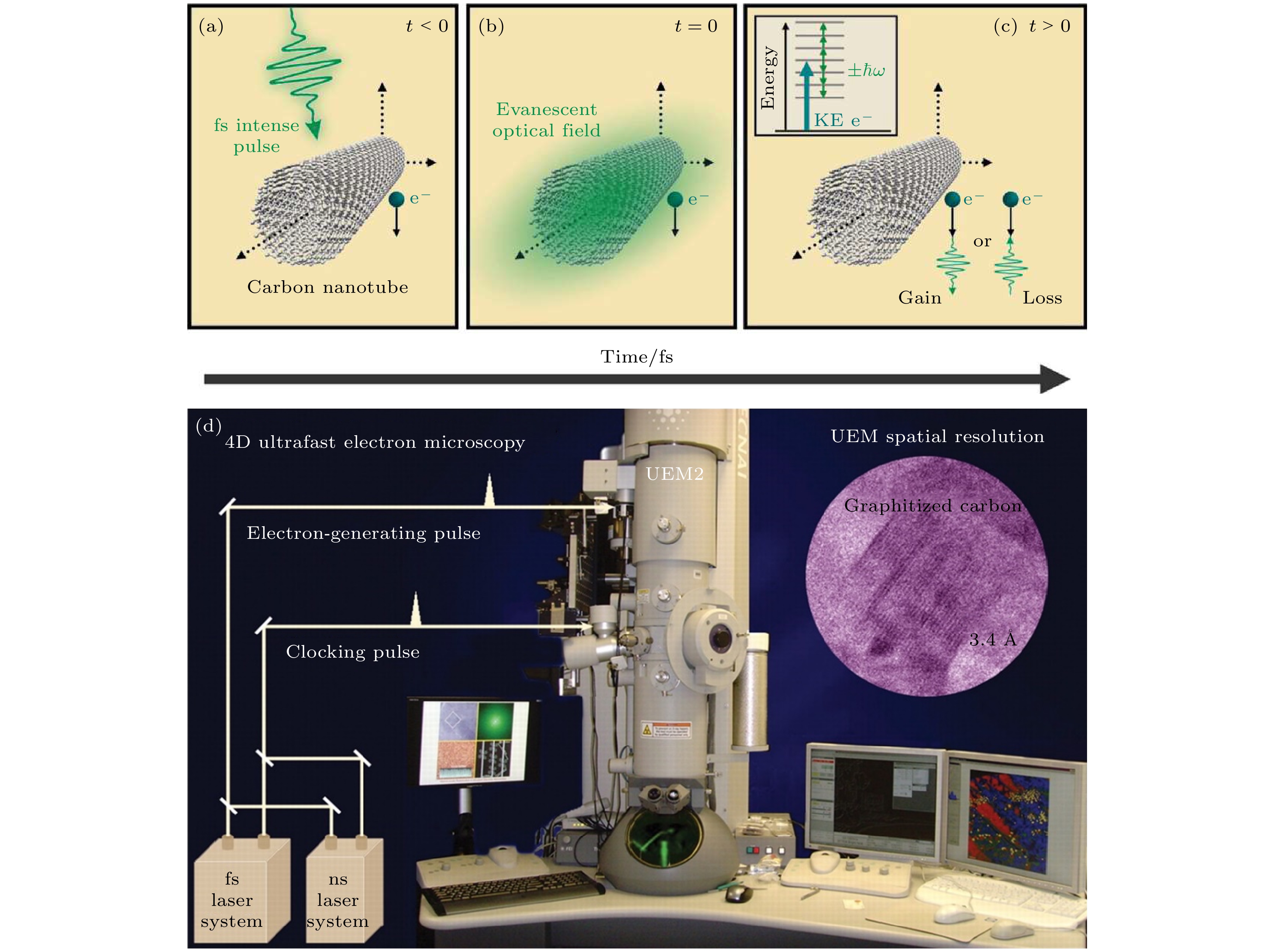
图 1 电子、光子和倏逝场相互作用的物理描述^[33]及实验装置^[56]　(a)飞秒激光脉冲到达前($t < 0$), 电子就已经过纳米管时的情况, 目前二者还没有发生时空重叠; (b) QEW、飞秒激光脉冲和倏逝场在碳纳米管上具有最大重叠时($t = 0$); (c) 在相互作用期间和随后瞬间($t > 0$) , 电子获得/失去的能量等于单光子能量的整数倍. 插图是在飞秒激光脉冲中成像电子和光子之间的自由-自由跃迁, 在连续体中可能的最终能量. KE表示动能; (d) UTEM实验装置的实物图

Fig. 1. Physical depiction of the interaction among the electron, photon, and the evanescent field^[33], and the picture of experimental set-up^[56]. (a) A frame when the electron packet arrives at the nanotube before the femtosecond laser pulse ($ t < 0 $), no spatial-temporal overlap has yet occurred. (b) The precise moment when the electron packet, femtosecond laser pulse, and evanescent field are at maximum overlap at the carbon nanotube. (c) Illustration of the process during and immediately after the interaction ($t > 0$) when the electron gains/loses energy equal to integer multiples of femtosecond laser photons. Inset, the possible final energies in the continuum due to the free-free transitions between the imaging electron and the photons in the femtosecond laser pulse. KE, kinetic energy. (d) Photograph of the UTEM.

下载: 全尺寸图片幻灯片

图 2 光学近场相干非弹性电子散射原理与示意图^[67]　(a) 实验示意图; (b) 非弹性电子散射谱中能量截断的光栅扫描图像; (c) 入射动能谱, 峰位于$ {E}_{0} =120\text{ keV} $, 其半高全宽(full-width at half-maximum, FWHM)为$0.7{\text{ eV}}$; (d) 梯状能级图, 箭头表示连续的多态布居转移(类型I)和量子干涉路径(类型II); (e) 近场相互作用后的能谱示例

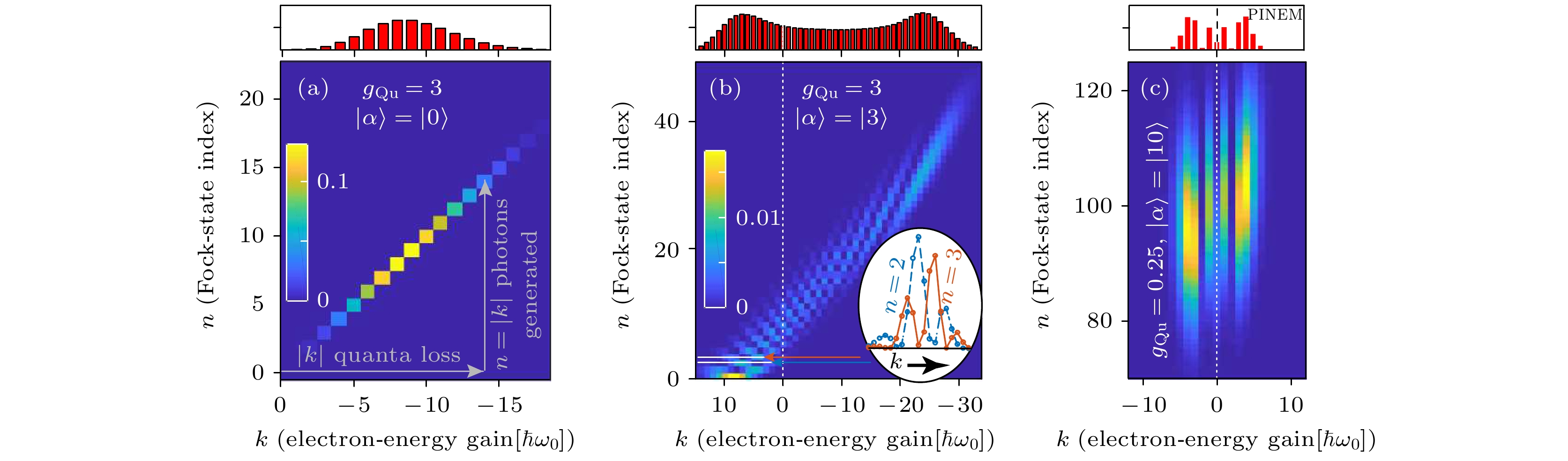
Fig. 2. Schematic and principles of coherent inelastic electron scattering by optical near-fields^[67]: (a) Experimental scheme; (b) raster-scanned image of the energy cutoff in the inelastic electron scattering spectra; (c) incident kinetic energy spectrum (full-width at half-maximum, $0.7{\text{ eV}}$) centered at ${E_0} = 120{\text{ keV}}$; (d) energy level diagram of ladder states, arrows indicate sequential multistate population transfer (type I) and interfering quantum paths (type II); (e) example of kinetic energy spectrum after the near-field interaction.

下载: 全尺寸图片幻灯片

图 3 电子和光子的纠缠模式^[35]　(a) 腔处于真空态时; (b) 腔处于相干态$ \left| {\left. \alpha \right\rangle = } \right|\left. 3 \right\rangle $时出现了丰富的纠缠现象; (c) 腔处于$| \alpha \rangle = $$ | {10} \rangle$且${g_{{\text{Qu}}}} = 0.25$时的弱耦合情况

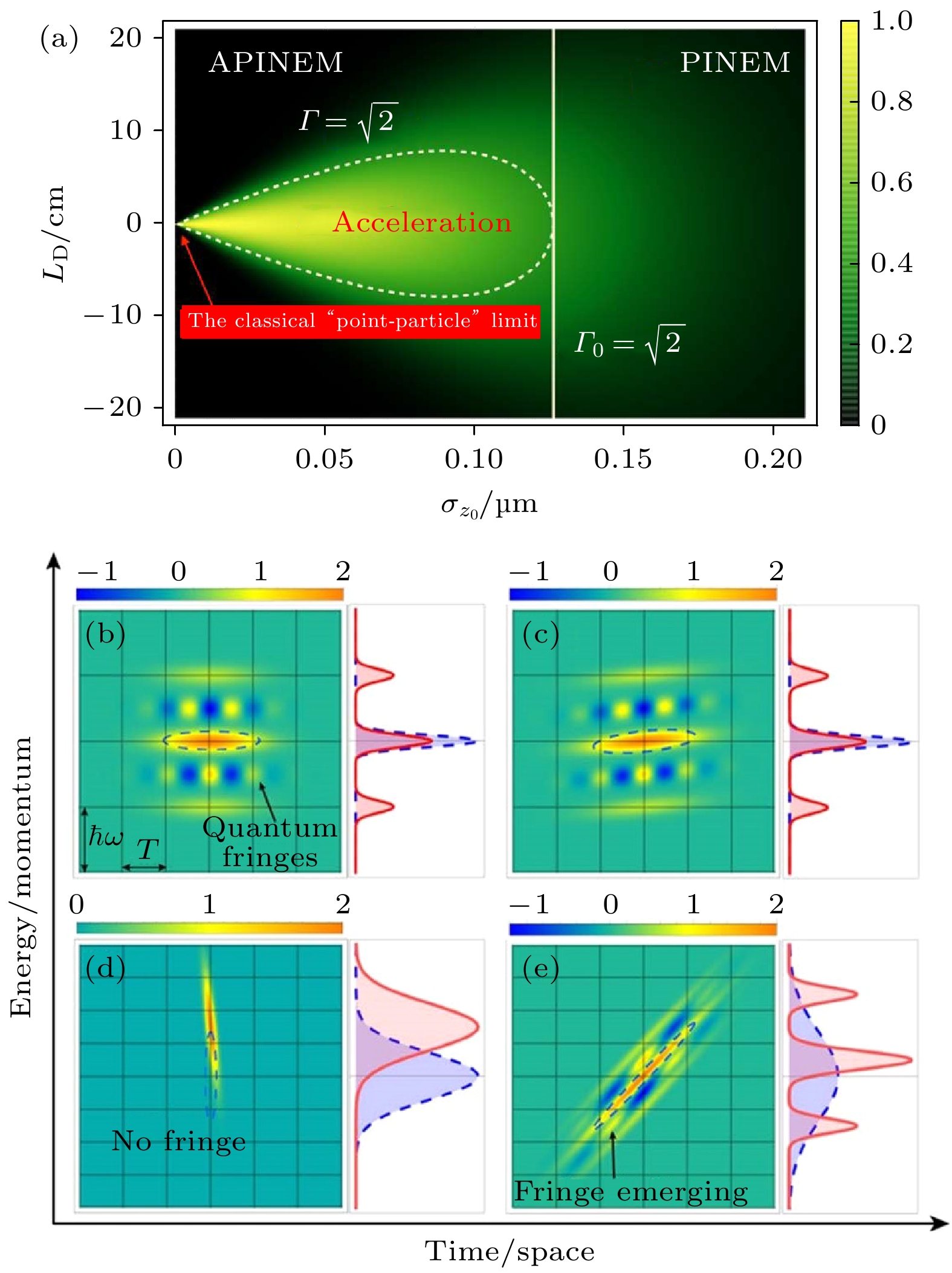
Fig. 3. Electron-photon entanglement patterns^[35]: (a) When the cavity is in a vacuum state; (b) rich entanglement features for an initial coherent state$|\alpha \rangle = |3\rangle$ in the cavity; (c) weak coupling and highly populated cavity (${g_{{\text{Qu}}}} = 0.25$, $\left| {\left. \alpha \right\rangle = } \right|\left. {10} \right\rangle $).

下载: 全尺寸图片幻灯片

图 4 (a) 根据量子电子波函数初始参数(${\sigma _{{z_0}}}, {L_{\text{D}}}$)对光与电子相互作用的分类. (b)—(e) PINEM、加速和APINEM在相互作用前(折线椭圆)后(红色和黄色表示正值, 蓝色表示负值) 的相空间表示及其能量(动量)分布. QEW的初始分布$ {W}^{\left(00\right)} $的面积是$h/2$, 单元格面积为普朗克常量$h$. PINEM情况下, 初始分布在时间方向扩展, 而其能量展宽较窄, 其中(b)为无啁啾, (c)为预啁啾; (d) 类粒子加速, 初始时间短且相位明确的QEW具有净动量转移; (e) APINEM情况. 初始量子电子束进行了强啁啾, 产生了量子干涉条纹^[70]

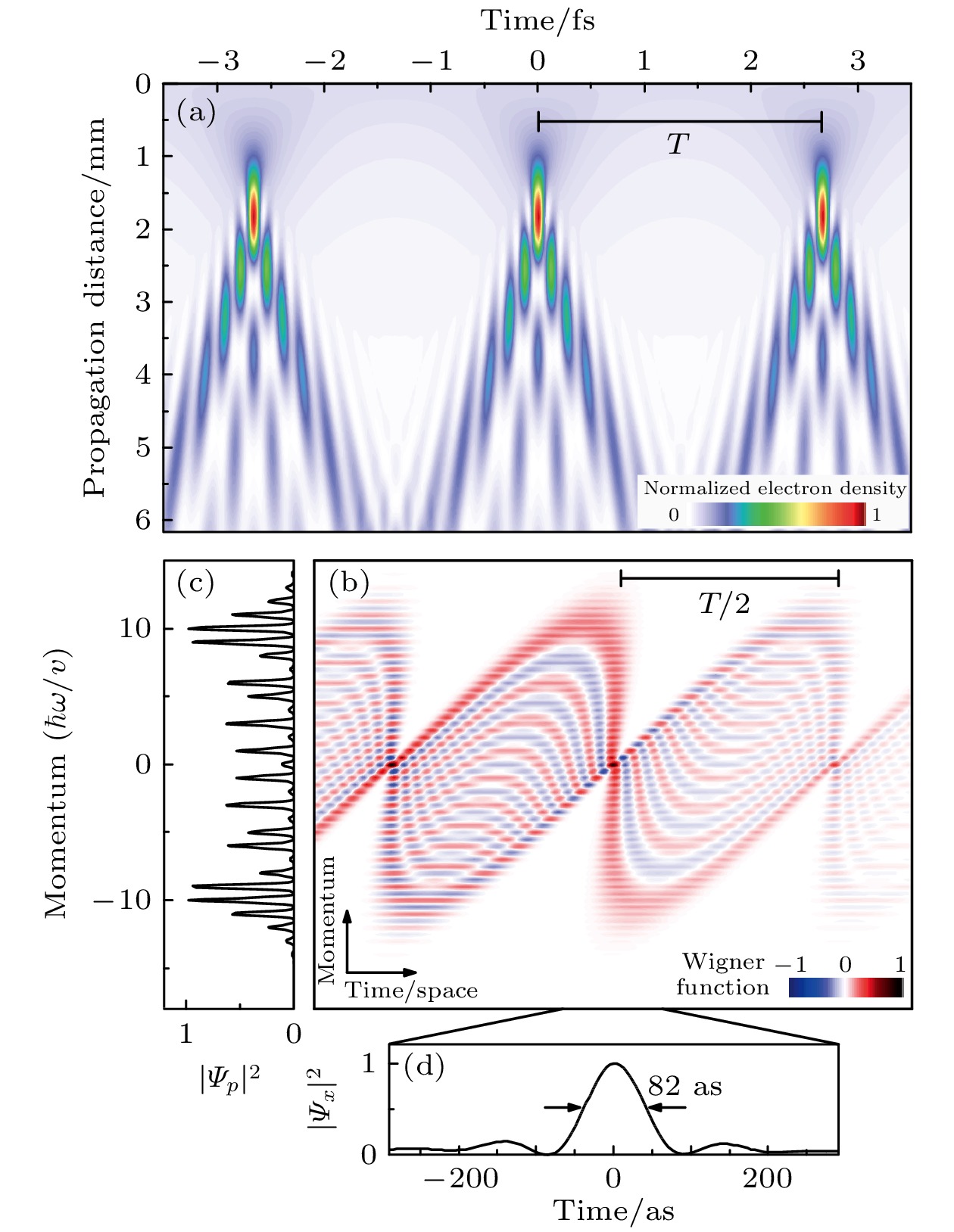
Fig. 4. (a) Universal classification of light-matter interaction regimes in terms of the initial parameters of the quantum electron wave function: its minimal axial waist size ${\sigma _{{z_0}}}$ and the pre-interaction drift length from this point, ${L_{\text{D}}}$. (b)–(e) Illustrations of PINEM, acceleration, and APINEM processes in phase-space representation before (broken-line ellipses) and after (positive, red and yellow; negative, blue) interaction and their energy (momentum) distributions. The initial distributions $ {W}^{\left(00\right)} $ of the QEW of area $ h/2 $ are overlaid over a grid of area $h$ (Planck constant) tiles: PINEM case, the initial distribution is temporally (or longitudinally) expanded, and its energy spread is narrow: (b) unchirped; (c) prechirped; (d) particle-like acceleration with net momentum shift for an initially temporally short QEW with well-defined phase; (e) APINEM case. Expanded and strongly prechirped initial QEW, with quantum interference fringes emerging^[70].

下载: 全尺寸图片幻灯片

图 5 (a) 周期调制的电子脉冲结构的演化成阿秒脉冲, 其中电子密度作为近场相互作用后传播距离的函数(数值模拟采用$ \left|g\right| = 5.7 $); (b) 电子量子态在时间焦点位置处 (图(a)中传播距离为$1.8{\text{ mm}}$处)光调制一个周期的相空间表示; (c) Wigner函数的动量投影; (d) Wigner函数空间投影的中心部分, 脉冲持续时间(FWHM)仅为$82{\text{ as}}$^[67]

Fig. 5. (a) A periodically modulated electron pulse structure evolved into attosecond pulse (electron density) as a function of the propagation distance after the near-field interaction (numerical simulation for $ \left|g\right| = 5.7 $); (b) phase space (Wigner) representation of one period of the light-modulated electron quantum state at the temporal focus position (propagation distance of $1.8{\text{ mm}}$ in panel (a)); (c) momentum projection of Wigner function exhibiting spectral modulations as observed in the experiments; (d) central part of spatial projection. A peak with a duration of only $82{\text{ as}}$ (FWHM) is produced^[67].

下载: 全尺寸图片幻灯片

图 6 (a) 拉姆齐型自由电子干涉仪的工作原理: 同一个电子脉冲(绿色)依次作用于空间分离的两个场${g_1}$和${g_2}$; (b) 具有两个相互作用区域的纳米结构的扫描电子显微图(俯视图和侧视图), 金片间距$5{\text{ μm}}$; (c)控制激发纳米结构的实验场景示意图; (d) 局域耦合强度$\left| {{g_{{\text{tot}}}}} \right|$的空间分布图像, 此时激励条件几乎满足角落区域对电子能谱进行完全再压缩^[76]

Fig. 6. (a) Working principle of the Ramsey-type free electron interferometer: an electron pulse (green) is acted on at two spatially separated nodes ${g_1}$ and ${g_2}$; (b) scanning electron micrographs of the nanostructure featuring two interaction zones (top and side view), distance between gold paddles is $5{\text{ μm}}$; (c) sketch of the experimental scenario displaying polarization-controlled excitation of the nanostructure; (d) raster-scanned image of the local coupling strength $\left| {{g_{{\text{tot}}}}} \right|$ for excitation conditions near complete recompression in the corner region^[76].

下载: 全尺寸图片幻灯片

图 7 时域阿秒脉冲整形的模拟　(a) 模拟所得能谱图, 每个光周期中有$80{\text{ as}}$的时间聚焦, 约占光周期$3{\text{%}} $; (b) 相应的Wigner函数; (c) Wigner函数的时间投影显示出对密度函数的调制, 脉冲持续时间(FWHM)为$531{\text{ as}}$(减去阴影部分顶部的基线后, 脉冲持续时间有效值为$296{\text{ as}}$); (d) 对应的电子能谱(动量投影) ^[78]

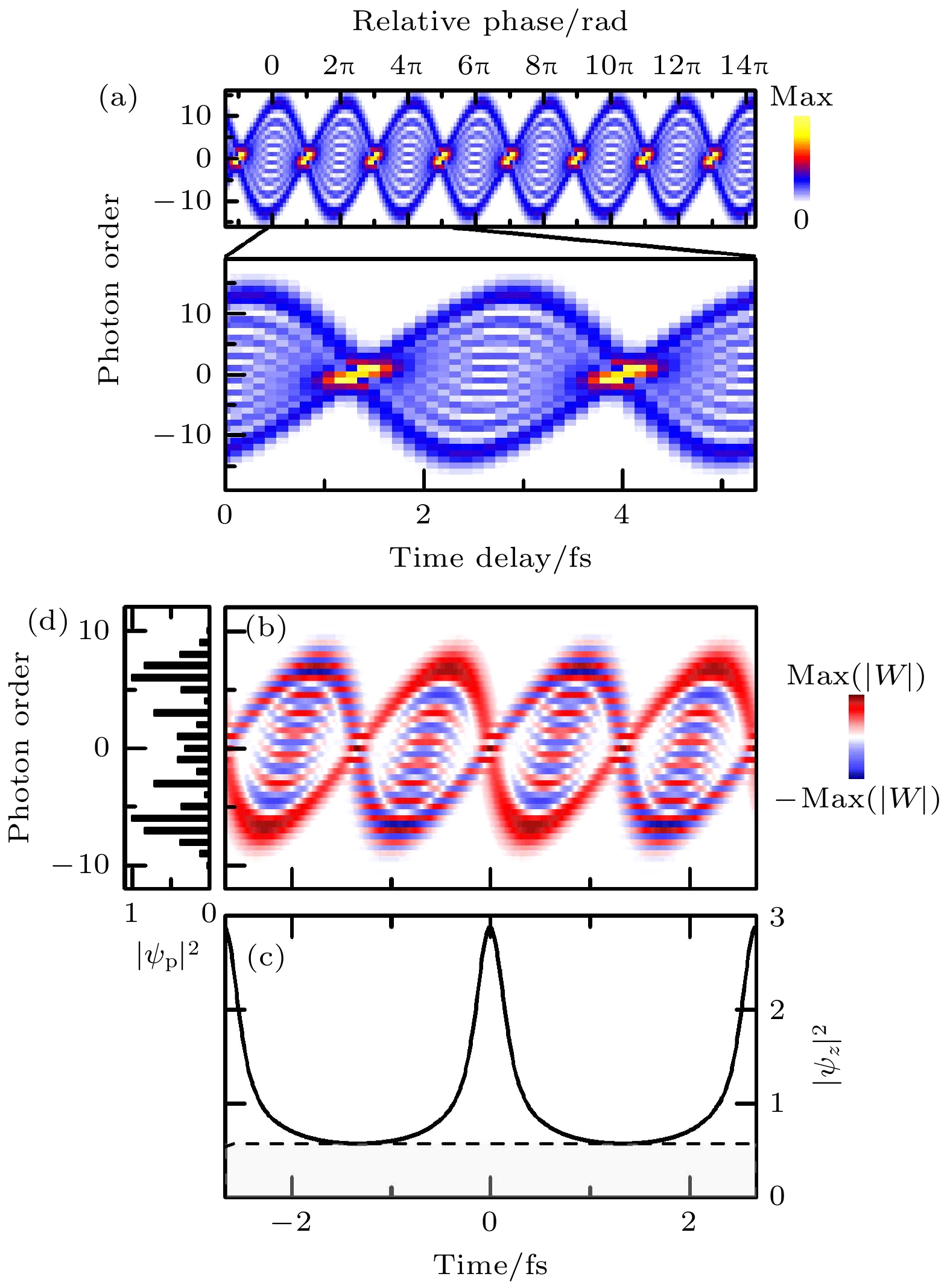
Fig. 7. Simulation of attosecond temporal reshaping a simulated spectrogram assuming: (a) Energy spectrum obtained by simulation, including a small timing jitter of 80 as (3% of the optical period); (b) corresponding Wigner function; (c) temporal projection of the Wigner function exhibits density modulations with a FWHM pulse duration of $531{\text{ as}}$ (after baseline subtraction, rms pulse duration: $296 \text{ as}$); (d) corresponding electron energy spectrum (momentum projection) ^[78].

下载: 全尺寸图片幻灯片

图 8 阿秒电子脉冲序列的实验演示　(a) 使用两个石墨薄片来制备阿秒电子脉冲序列的实验装置示意图, 插图为定制的TEM样品架; (b)多个光周期的实验光谱图(上)以及其中两个周期的特写(下); (c) 重构的Wigner函数; (d) Wigner函数的时间投影展示了对密度的调制, FWHM为$655{\text{ as}}$(减去阴影区域上部基线后, 脉冲有效持续时间$277{\text{ as}}$); (e) 对应的电子能谱(动量投影)^[78]

Fig. 8. Experimental demonstration of attosecond electron pulse trains: (a) Sketch of the experimental set-up employing two graphite flakes for the preparation (upper plane) and characterization (lower plane) of attosecond electron pulse trains, and the inset is photograph showing the custom-built TEM sample holder; (b) experimental spectrogram recorded over multiple optical cycles (top) and close-up of two cycles (bottom); (c) reconstructed Wigner function; (d) temporal projection of the Wigner function exhibits density modulations with a FWHM of $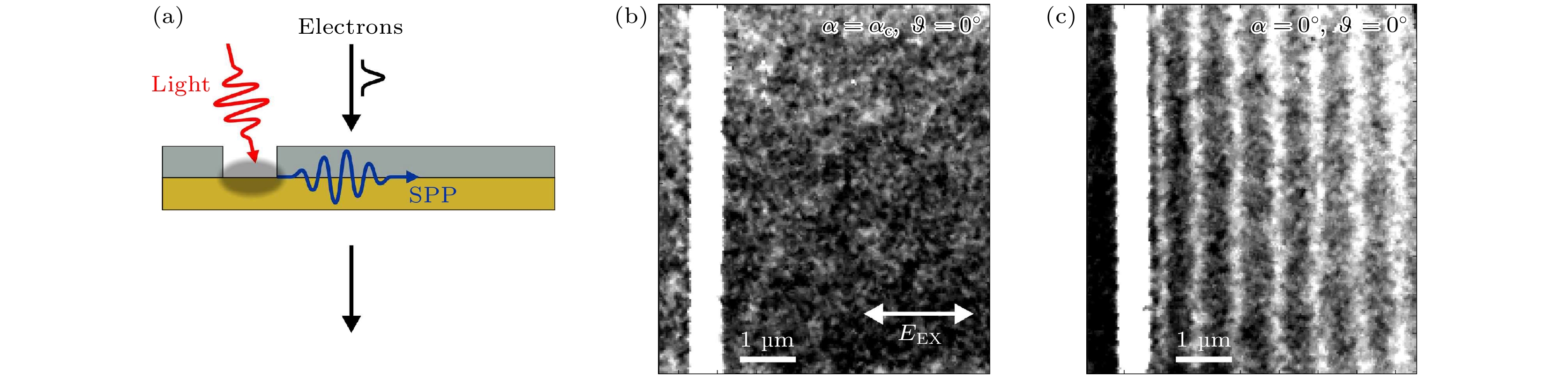655{\text{ as}}$ (after subtraction of a baseline indicated by the grey-shaded area; r.m.s. pulse duration of $277{\text{ as}}$); (e) corresponding electron energy spectrum (momentum projection) ^[78].

下载: 全尺寸图片幻灯片

图 9 自由电子与光激发的SPP相互作用的示意图　(a) 在银层中雕刻的一维纳米腔通过光照明产生SPP的示意图; (b) 旋转样品方向, 使得实验测量中电子-光相互作用为零时的能量滤波图像; (c) 实验测量中具有不可忽略的电子-光相互作用的能量过滤图像. 只有在后一种构型中, 传播光和SPP场才会在电子束的作用下产生位置相关的干涉, 从而产生一个空间振荡的场振幅, 可以在真实空间中成像^[10]

Fig. 9. Visualization of propagating surface-plasmon polaritons: (a) Schematic representation of the generation of surface plasmon polaritons by optical illumination at the edge of a nanocavity carved in the Ag layer; (b) experimentally measured energy-filtered image for a sample orientation such to have a vanishing electron-light interaction; (c) experimentally measured energy-filtered image for a sample orientation such to have a non-negligible electron-light interaction. Only in the latter configuration a position-dependent interference of the propagating light and SPP fields as mediated by the electron beam occurs giving rise to a spatially oscillating field amplitude that can be imaged in real-space^[10].

下载: 全尺寸图片幻灯片

图 10 (a) 实验过程示意图; (b) 非局域全息法的示意图; (c) 电子-SPP相互作用后非弹性散射电子的空间分布, 比例尺为$2{\text{ μm}}$; (d) 利用文中详细介绍的半解析理论计算的实空间电子强度分布, 比例尺为$2{\text{ μm}}$; (e) FDTD模拟得到的界面总电场z分量的相位图(比例尺为$1{\text{ μm}}$)^[83]

Fig. 10. (a) Schematic representation of the experimental geometry; (b) schematic representation of the non-local holographic method; (c) experimentally measured spatial distribution of the inelastically scattered electrons following the electron-plasmon interaction, scale bar, 2 μm; (d) calculated real-space electron intensity distribution using the semi-analytical theory detailed in the text (scale bar, 2 μm); (e) simulated phase map of the $z$ component of the total electric field at the interface obtained from FDTD simulations (scale bar, 1 μm)^[83].

下载: 全尺寸图片幻灯片

图 11 (a) UTEM中自由电子与光子腔的量子相互作用的五个自由度. (b)—(d) 光子晶体能带结构的重建与Bloch模的直接成像　(b)通过扫描入射光角度和波长而测得的能带结构; (c) 光子晶体和入射泵浦激光脉冲的示意图; (d) 在(b)中标记的角度和波长处测得的光子晶体的Bloch模式. 比例尺:$300{\text{ nm}}$^[54]

Fig. 11. (a) The UTEM setup offers five degrees of freedom to measure the interactions. (b)–(d) Reconstruction of band structure and direct imaging of the Bloch modes of the photonic crystal: (b) Band structure measured by scanning over incident laser angles and wavelengths; (c) layout of the photonic crystal and incident pump laser pulse; (d) Bloch modes of the photonic crystal measured at the angles and wavelengths marked in panel (b). 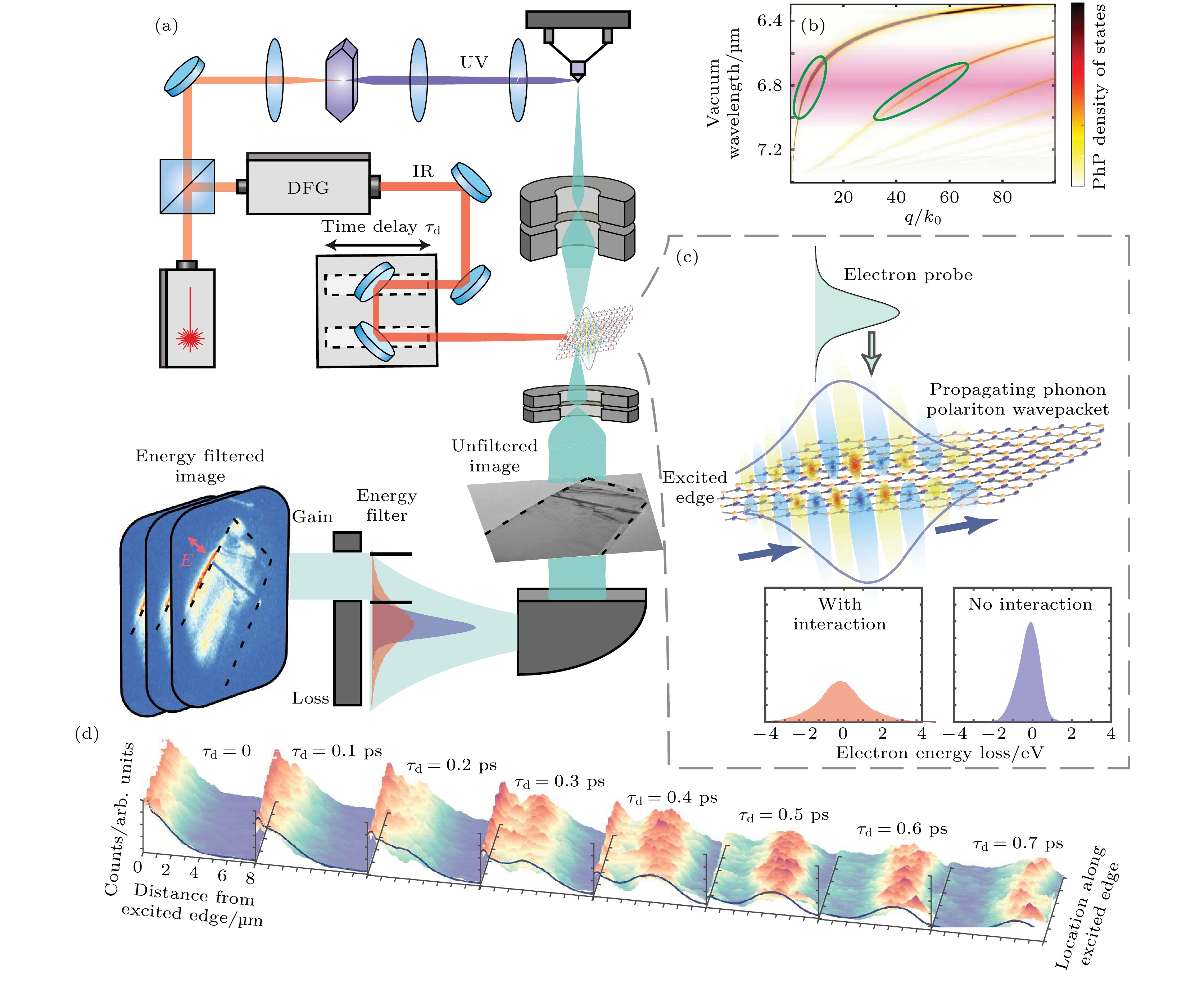Scale bar, $300{\text{ nm}}$^[54].

下载: 全尺寸图片幻灯片

图 12 由UTEM观测二维极化激元波包^[55]　(a) 实验装置以及实验过程示意图; (b)样品的色散关系; (c) 自由电子探测hBN内部(TM偏振)传播的PhP波包, 插图为激光开启(左)和关闭(右)时的EELS; (d) 测量不同时间延迟${\tau _{\text{d}}}$下的电子的能量滤波, 显示了PhP波包的传播动力学

Fig. 12. Direct observation of two dimensional (2D) polariton wave packets using UTEM^[55]: (a) Experimental setup and the process; (b) dispersion relation of the sample; (c) free electron probing the (TM polarized) propagating PhP wave packet inside the hBN, and the insets show EELS spectra with the laser on (left) and off (right); (d) measurement of the energy-filtered electrons for different time delays ${\tau _{\text{d}}}$ showing the propagation dynamics of the PhP wave packe.

下载: 全尺寸图片幻灯片

图 13 强耦合的纠缠特性, 由符合测量概率$ |c_{n, k}^{{\text{e - e}}}{|^2} $表示^[35]　(a) 耦合系数${g_{{\text{Qu}}}} = 1$; (b)耦合系数${g_{{\text{Qu}}}} = 3$

Fig. 13. Electron-electron interaction for two distant electrons in a beam, mediated by long-lived photons^[35]. The color map ${\left| {c_{n, k}^{{\text{e - e}}}} \right|^2}$ is the coincident probability: (a) ${g_{{\text{Qu}}}} = 1$; (b) ${g_{{\text{Qu}}}} = 3$.

下载: 全尺寸图片幻灯片

图 14 用自干涉法测量电子密度矩阵^[37]　(a) 通过分束器后沿两条不同长度($z$和$z'$)的电子路径探索电子自相关的实验示意图; (b)—(i) 对不同的PINEM光场而言, 电子密度矩阵的实部(左)和虚部(右)作为两个电子各自的位移时间$\tau , \tau '$的函数

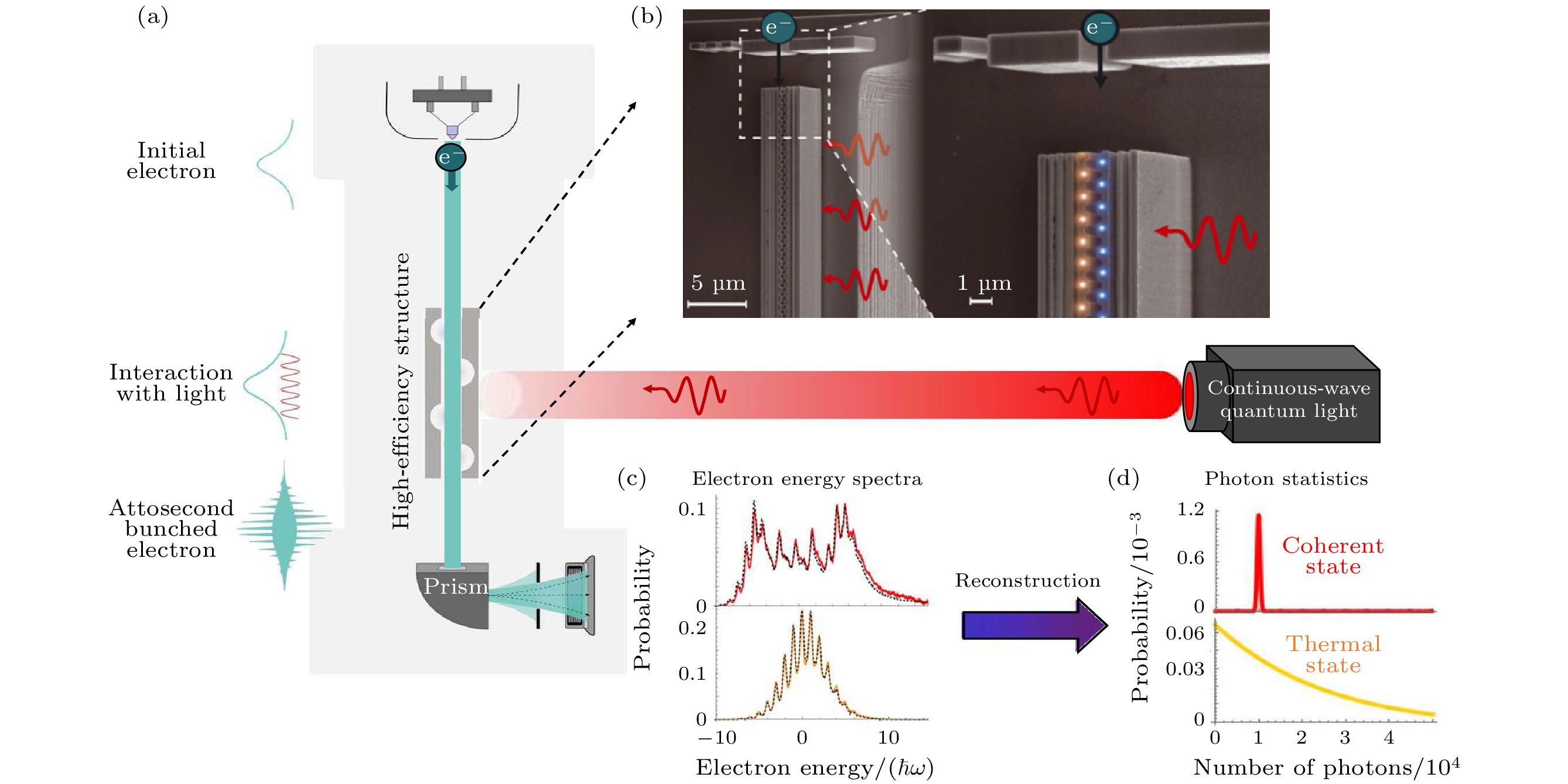
Fig. 14. Measuring the electron density matrix through self-interference^[37]: (a) Sketch of an experimental arrangement to explore electron auto-correlation by means of a beam splitter and different lengths $\left( z \right.$ and $\left. {z'} \right)$ along the two electron paths before recombination at the detection region; (b)–(i) real (left panels) and imaginary (right panels) parts of the electron density matrix as a function of shifted times $\tau $ and $\tau '$ for different statistics of the PINEM light.

下载: 全尺寸图片幻灯片

图 15 集成在电子显微镜中的硅光子器件提供了有效的电子与连续波光的相互作用, 使量子光子统计的检测成为可能^[40]　(a) 透射电镜中电子波函数的连续波调制; (b) 硅-光子纳米结构(扫描电子显微镜图像), 包括一个布拉格镜和一个周期通道; (c) 与相干光态和热光态相互作用后的电子能谱; (d) 由测量光谱重构的相应光子统计量

Fig. 15. A silicon-photonics device integrated in an electron microscope provides efficient electron interactions with CW light, enabling the detection of the quantum photon statistics^[40]: (a) CW modulation of electron wave functions in transmission electron microscopy; (b) silicon-photonic nanostructure (scanning electron microscope image), consisting of a Bragg mirror and a periodic channel; (c) electron energy spectrum after the interaction with two types of light states: coherent and thermal; (d) corresponding photon statistics reconstructed from the measured spectra.

下载: 全尺寸图片幻灯片

图 16 自由电子-光相互作用在电子能谱上刻印了光子的量子统计^[40]　(a) 电子行走者与光子进行连续的相互作用; (b) 电子行走理论与Q-PINEM理论完全匹配; (c) 相干态和(d) 热态的电子能谱随电场振幅的变化

Fig. 16. Free-electron-light interactions imprint the quantum photon statistics on the electron energy spectra, demonstrating the transition from quantum walk to classical random walk of a free electron^[40]: (a) Electron walker performs consecutive interactions with the photons; (b) electron walker theory exactly matches with the Q-PINEM theory; lectron energy spectra for (c) coherent and (d) thermal states evolving with the electric field amplitude.

下载: 全尺寸图片幻灯片

图 17 利用Q-PINEM塑造具有新奇量子统计的光子态^[38]　(a) Q-PINEM相互作用的物理实现示意图; (b) 单次Q-PINEM相互作用的方案

Fig. 17. Shaping photonic states of novel quantum statistics using Q-PINEM interactions^[38]: (a) Schematic for a physical realization of a Q-PINEM interaction; (b) interaction scheme of a single Q-PINEM interaction.

下载: 全尺寸图片幻灯片

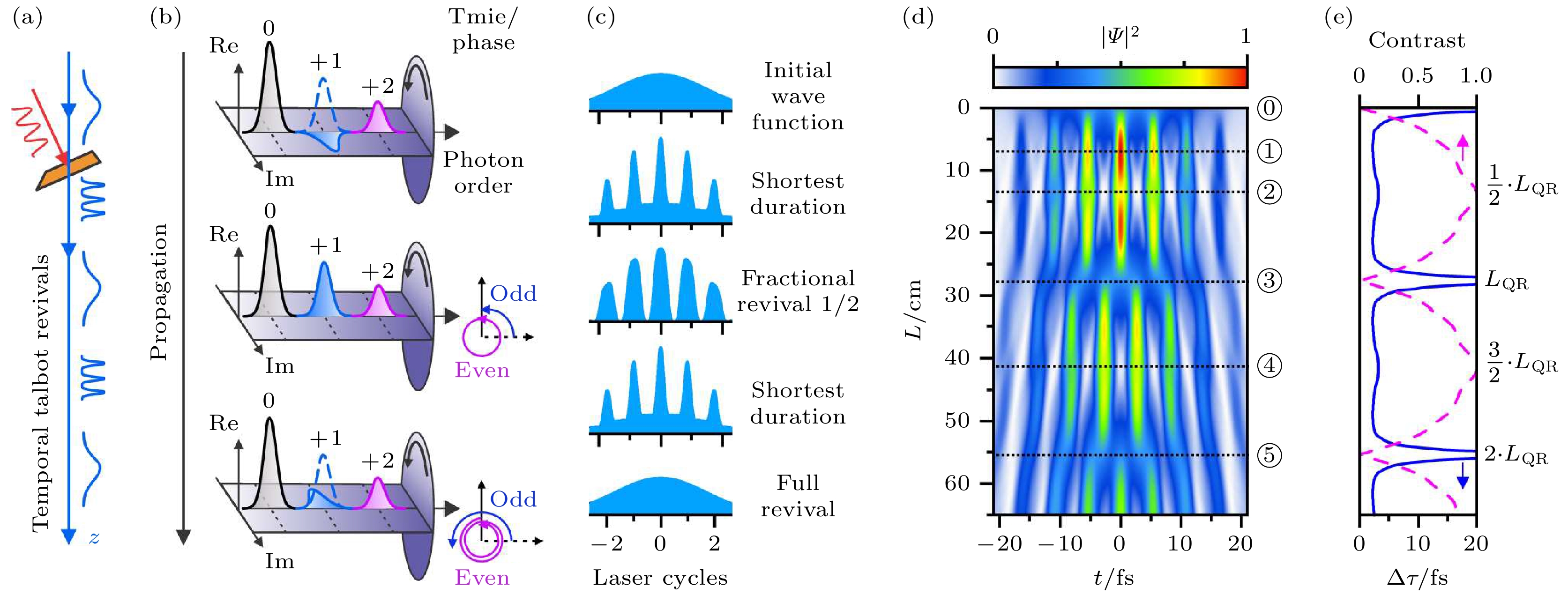
图 18 最大对比度下的量子复苏和亚光周期电子显微示意图^[45]　(a) 阿秒电子脉冲的示意以及自由电子量子比特的概念; (b) 能谱和边带相位的演化; (c) 电子的时域波包脉冲; (d) 模拟的电子波包$|\varPsi {|^2}$; (e) 电子脉冲持续时间$\tau $(实线)和时间对比度(虚线)作为$L$的函数

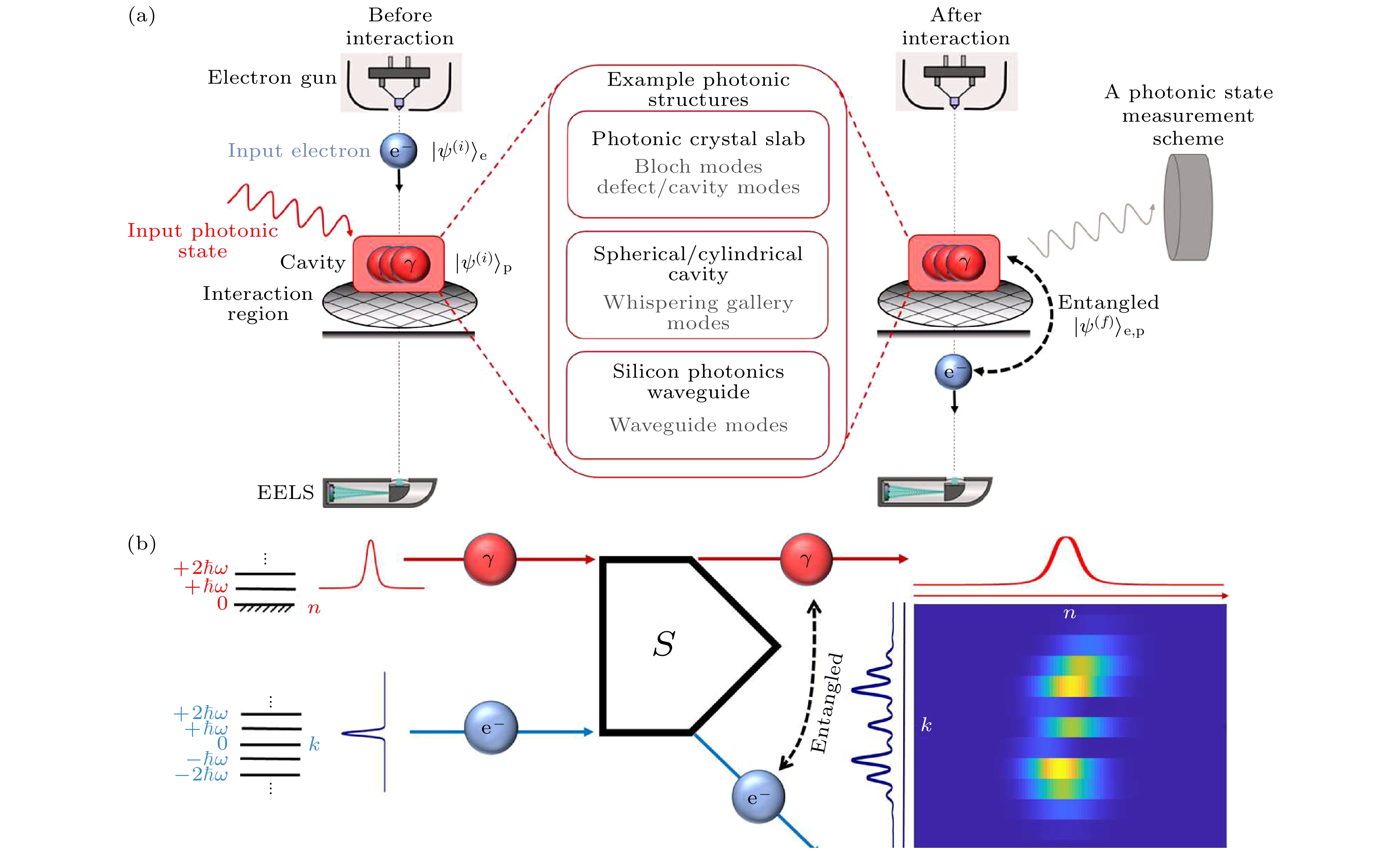
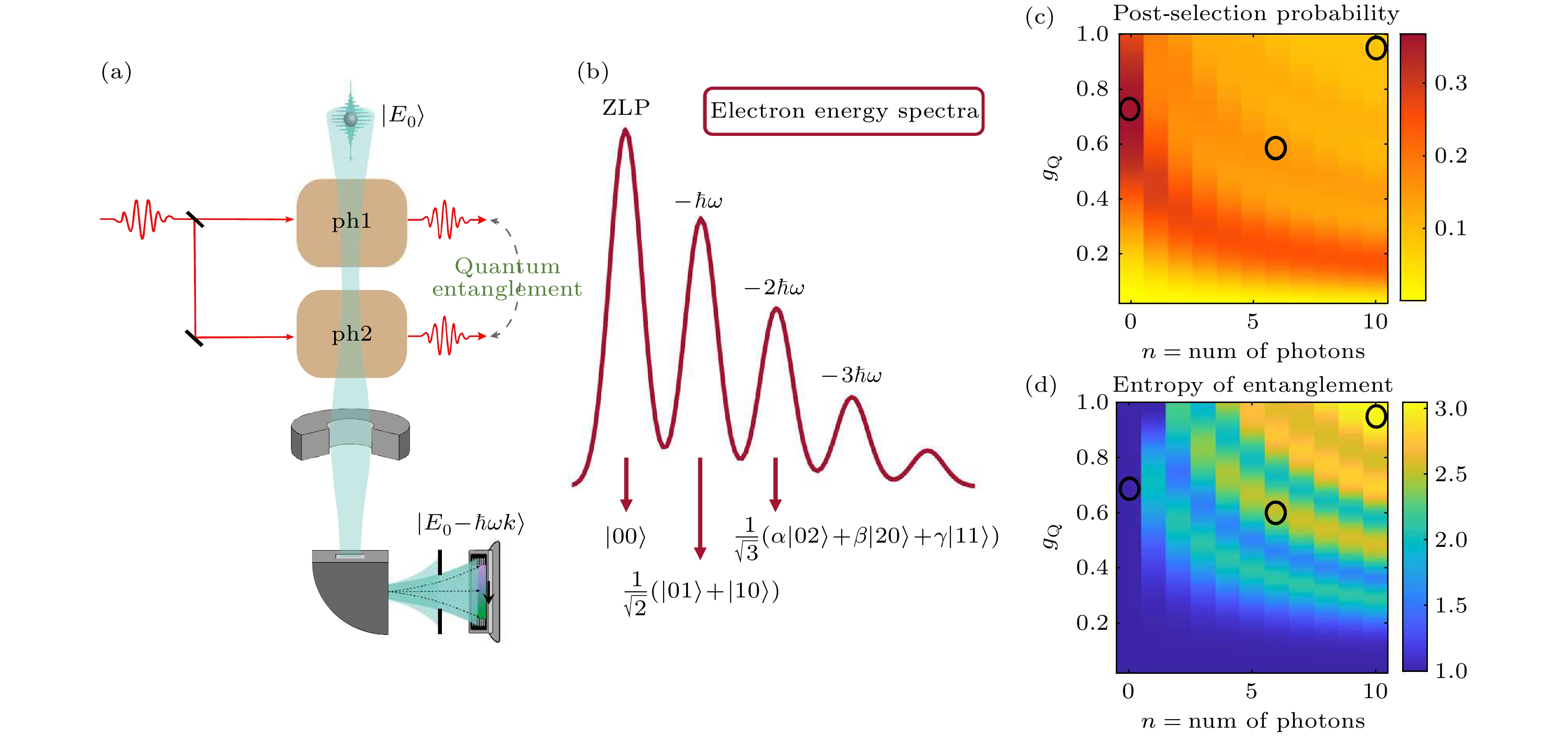
Fig. 18. Quantum revivals and sub-light-cycle electron microscopy at maximum contrast^[45]: (a) Concept for exploiting quantum revivals for generating attosecond electron pulses and qubits; (b) evolution of the energy spectrum and sideband phases; (c) wave packets and pulses in the time domain; (d) simulated quantum carpet $|\varPsi {|^2}$ of an electron wave packet; (e) electron pulse duration ${{\Delta }}\tau $ (solid) and temporal contrast (dashed) as a function of $L$.

下载: 全尺寸图片幻灯片

图 19 (a) 实验方案; (b) 相互作用后, 每个电子能态被纠缠到不同的光子态; (c) 对于不同的初始参数, 一次相互作用后对能量为${E_0} - \hbar \omega $ (即$k = 1$)的电子进行后选择的概率图; (d) 对能量为${E_0} - \hbar \omega $的电子(即$ k =1 $)进行后选择后两个光量子态之间纠缠熵的图^[47]

Fig. 19. (a) A scheme of the proposed experiment; (b) each electron energy is entangled to a different photonic state after the interaction; (c) a map of the probability to post-select the electron with energy ${E_0} - \hbar \omega $ (i.e., $k = 1$) after one interaction for different initial parameters$;$ (d) a map of the entropy of entanglement between the two states of light after post-selecting on electrons with energy ${E_0} - \hbar \omega $ (i.e., $k = 1$)^[47].

下载: 全尺寸图片幻灯片

map

[1]	Dirac P A M 1981 The Principles of Quantum Mechanics (London: Clarendon Press) pp204–207
[2]	Fox A M, Fox D P A M 2001 Optical Properties of Solids (New York: Oxford University Press) pp180–211
[3]	Vasa P 2020 Adv. Phys. X 5 1749884
[4]	Scully M O, Zubairy M S 1997 Quantum Optics (Cambridge: Cambridge University Press) pp145−217
[5]	Fox M, Fox D P A M 2006 Quantum Optics: An Introduction (New York: Oxford University Press) pp165–240
[6]	Walls D F, Milburn G J 2007 Quantum Optics (Heidelberg: Springer) pp197–211
[7]	Rivera N, Kaminer I 2020 Nat. Rev. Phys. 2 538 Google Scholar
[8]	Ashcroft N W, Mermin N D 1976 Solid State Physics (New York: Holt, Rinehart and Winston) pp131–150
[9]	Freimund D L, Aflatooni K, Batelaan H 2001 Nature 413 142 Google Scholar
[10]	Vanacore G M, Madan I, Berruto G, Wang K, Pomarico E, Lamb R J, McGrouther D, Kaminer I, Barwick B, Garcia de Abajo F J, Carbone F 2018 Nat. Commun. 9 2694 Google Scholar
[11]	Kaminer I, Katan Y T, Buljan H, Shen Y, Ilic O, Lopez J J, Wong L J, Joannopoulos J D, Soljacic M 2016 Nat. Commun. 7 11880 Google Scholar
[12]	Kaminer I, Mutzafi M, Levy A, Harari G, Herzig Sheinfux H, Skirlo S, Nemirovsky J, Joannopoulos J D, Segev M, Soljačić M 2016 Phys. Rev. X 6 011006
[13]	Gover A, Pan Y 2018 Phys. Lett. A 382 1550 Google Scholar
[14]	Lin X, Easo S, Shen Y, Chen H, Zhang B, Joannopoulos J D, Soljačić M, Kaminer I 2018 Nat. Phys. 14 816 Google Scholar
[15]	Pan Y, Gover A 2018 J. Phys. Commun. 2 115026 Google Scholar
[16]	Roques-Carmes C, Rivera N, Joannopoulos J D, Soljačić M, Kaminer I 2018 Phys. Rev. X 8 041013
[17]	Pan Y, Gover A 2019 Phys. Rev. A 99 052107 Google Scholar
[18]	Lin X, Hu H, Easo S, Yang Y, Shen Y, Yin K, Blago M P, Kaminer I, Zhang B, Chen H, Joannopoulos J, Soljacic M, Luo Y 2021 Nat. Commun. 12 5554 Google Scholar
[19]	Adiv Y, Hu H, Tsesses S, Dahan R, Wang K, Kurman Y, Gorlach A, Chen H, Lin X, Bartal G, Kaminer I 2022 arXiv: 2203.01698 [quant-ph]
[20]	Mizuno K, Ono S, Shimoe O 1975 Nature 253 184 Google Scholar
[21]	Tsesses S, Bartal G, Kaminer I 2017 Phys. Rev. A 95 013832 Google Scholar
[22]	Massuda A, Roques-Carmes C, Yang Y, Kooi S E, Yang Y, Murdia C, Berggren K K, Kaminer I, Soljačić M 2018 ACS Photonics 5 3513 Google Scholar
[23]	Rosolen G, Wong L J, Rivera N, Maes B, Soljacic M, Kaminer I 2018 Light Sci. Appl. 7 64 Google Scholar
[24]	Yang Y, Massuda A, Roques-Carmes C, Kooi S E, Christensen T, Johnson S G, Joannopoulos J D, Miller O D, Kaminer I, Soljačić M 2018 Nat. Phys. 14 894 Google Scholar
[25]	Remez R, Karnieli A, Trajtenberg-Mills S, Shapira N, Kaminer I, Lereah Y, Arie A 2019 Phys. Rev. Lett. 123 060401 Google Scholar
[26]	Roques-Carmes C, Kooi S E, Yang Y, Massuda A, Keathley P D, Zaidi A, Yang Y, Joannopoulos J D, Berggren K K, Kaminer I, Soljacic M 2019 Nat. Commun. 10 3176 Google Scholar
[27]	Jing L, Lin X, Wang Z, Kaminer I, Hu H, Li E, Liu Y, Chen M, Zhang B, Chen H 2021 Laser Photonics Rev. 15 2000426 Google Scholar
[28]	Karlovets D V, Pupasov-Maksimov A M 2021 Phys. Rev. A 103 012214 Google Scholar
[29]	Wong L J, Kaminer I 2021 Appl. Phys. Lett. 119 130502 Google Scholar
[30]	Yang Y, Roques-Carmes C, Kooi S E, Tang H, Beroz J, Mazur E, Kaminer I, Joannopoulos J D, Soljačić M 2021 arXiv: 2110.03550 [physics. optics]
[31]	Zhu J F, Du C H, Zhang Z W, Liu P K, Zhang L, Cross A W 2021 Opt. Lett. 46 4682 Google Scholar
[32]	Betzig E, Trautman J K 1992 Science 257 189 Google Scholar
[33]	Barwick B, Flannigan D J, Zewail A H 2009 Nature 462 902 Google Scholar
[34]	Di Giulio V, Kociak M, de Abajo F J G 2019 Optica 6 001524 Google Scholar
[35]	Kfir O 2019 Phys. Rev. Lett. 123 103602 Google Scholar
[36]	Reinhardt O, Mechel C, Lynch M, Kaminer I 2019 Conf Lasers Electro-Opt San Jose, California, USA, May 5, 2019 pFF1F.6
[37]	Di Giulio V, García de Abajo F J 2020 Optica 7 1820 Google Scholar
[38]	Ben Hayun A, Reinhardt O, Nemirovsky J, Karnieli A, Rivera N, Kaminer I 2021 Sci. Adv. 7 eabe4270 Google Scholar
[39]	Braiman G, Reinhardt O, Levi O, Mechel C, Kaminer I 2021 Conf Lasers Electro-Opt San Jose, California, USA, May 9, 2021 pFTh1N.6
[40]	Dahan R, Gorlach A, Haeusler U, Karnieli A, Eyal O, Yousefi P, Segev M, Arie A, Eisenstein G, Hommelhoff P, Kaminer I 2021 Science 373 eabj7128 Google Scholar
[41]	Gover A, Ran D, Zhang B, Pan Y M, Ianconescu R, Scheuer J, Friedman A, Yariv A 2021 Front Opt. Laser Sci. Washington DC, USA, November 1, 2021 pFTh6 D.1
[42]	Karnieli A, Rivera N, Arie A, Kaminer I 2021 Phys. Rev. Lett. 127 060403 Google Scholar
[43]	Reinhardt O, Mechel C, Lynch M, Kaminer I 2021 Ann. Phys. 533 2000254 Google Scholar
[44]	Rivera N, Sloan J, Kaminer I, Soljacic M 2021 arXiv: 2111.07010 [quant-ph]
[45]	Tsarev M V, Ryabov A, Baum P 2021 Phys. Rev. Res. 3 043033 Google Scholar
[46]	Zhang B, Ran D, Ianconescu R, Friedman A, Scheuer J, Yariv A, Gover A 2021 arXiv: 2111.13130
[47]	Baranes G, Ruimy R, Gorlach A, Kaminer I 2022 npj Quantum Inf. 8 32 Google Scholar
[48]	Bloch J, Cavalleri A, Galitski V, Hafezi M, Rubio A 2022 Nature 606 41 Google Scholar
[49]	Feist A, Huang G, Arend G, et al. 2022 arXiv: 2202.12821 [quant-ph]
[50]	Feist J, Fernández-Domínguez A I, García-Vidal F J 2021 Nanophotonics 10 477
[51]	Scheel S, Yoshi Buhmann S 2009 arXiv: 0902.3586 [quant-ph]
[52]	Henke J W, Raja A S, Feist A, et al. 2021 Nature 600 653 Google Scholar
[53]	Kfir O, Lourenco-Martins H, Storeck G, Sivis M, Harvey T R, Kippenberg T J, Feist A, Ropers C 2020 Nature 582 46 Google Scholar
[54]	Wang K, Dahan R, Shentcis M, Kauffmann Y, Ben Hayun A, Reinhardt O, Tsesses S, Kaminer I 2020 Nature 582 50 Google Scholar
[55]	Kurman Y, Dahan R, Sheinfux Hanan H, et al. 2021 Science 372 1181 Google Scholar
[56]	Barwick B, Park H S, Kwon O-H, Baskin J S, Zewail A H 2008 Science 322 1227 Google Scholar
[57]	Liu H, Baskin J S, Zewail A H 2016 Proc. Natl. Acad. Sci. U. S. A. 113 2041 Google Scholar
[58]	Sternbach A J, Chae S H, Latini S, et al. 2021 Science 371 617 Google Scholar
[59]	Yoxall E, Schnell M, Nikitin A Y, et al. 2015 Nat. Photonics 9 674 Google Scholar
[60]	Petek H, Ogawa S 1997 Prog. Surf. Sci. 56 239 Google Scholar
[61]	Davis T J, Janoschka D, Dreher P, Frank B, Heringdorf F J M z, Giessen H 2020 Science 368 eaba6415 Google Scholar
[62]	Balistreri M L M, Gersen H, Korterik J P, Kuipers L, Hulst N F v 2001 Science 294 1080 Google Scholar
[63]	Yamamoto N 2016 Microscopy 65 282 Google Scholar
[64]	Garcia de Abajo F J, Asenjo-Garcia A, Kociak M 2010 Nano Lett. 10 1859 Google Scholar
[65]	Park S T, Lin M, Zewail A H 2010 New J. Phys. 12 123028 Google Scholar
[66]	Park S T, Zewail A H 2012 J. Phys. Chem. A 116 11128 Google Scholar
[67]	Feist A, Echternkamp K E, Schauss J, Yalunin S V, Schafer S, Ropers C 2015 Nature 521 200 Google Scholar
[68]	Kociak M 2015 Nature 521 166 Google Scholar
[69]	Dahan R, Nehemia S, Shentcis M, Reinhardt O, Adiv Y, Shi X, Be’er O, Lynch M H, Kurman Y, Wang K, Kaminer I 2020 Nat. Phys. 16 1123 Google Scholar
[70]	Pan Y, Zhang B, Gover A 2019 Phys. Rev. Lett. 122 183204 Google Scholar
[71]	Kling P, Giese E, Endrich R, Preiss P, Sauerbrey R, Schleich W P 2015 New J. Phys. 17 123019 Google Scholar
[72]	Friedman A, Gover A, Kurizki G, Ruschin S, Yariv A 1988 Rev. Mod. Phys. 60 471 Google Scholar
[73]	Fu X, Barantani F, Gargiulo S, Madan I, Berruto G, LaGrange T, Jin L, Wu J, Vanacore G M, Carbone F, Zhu Y 2020 Nat. Commun. 11 5770 Google Scholar
[74]	Mkhitaryan V, Dias E J C, Carbone F, García de Abajo F J 2021 ACS Photonics 8 614 Google Scholar
[75]	Ruimy R, Gorlach A, Mechel C, Rivera N, Kaminer I 2021 Phys. Rev. Lett. 126 233403 Google Scholar
[76]	Echternkamp K E, Feist A, Schäfer S, Ropers C 2016 Nat. Phys. 12 1000 Google Scholar
[77]	Morimoto Y, Baum P 2017 Nat. Phys. 14 252
[78]	Priebe K E, Rathje C, Yalunin S V, Hohage T, Feist A, Schäfer S, Ropers C 2017 Nat. Photonics 11 793 Google Scholar
[79]	Cai W, Reinhardt O, Kaminer I, de Abajo F J G 2018 Phys. Rev. B 98 045424 Google Scholar
[80]	Kozak M, Schonenberger N, Hommelhoff P 2018 Phys. Rev. Lett. 120 103203 Google Scholar
[81]	Morimoto Y, Baum P 2018 Phys. Rev. A 97 033815 Google Scholar
[82]	Polman A, Kociak M, Garcia de Abajo F J 2019 Nat. Mater. 18 1158 Google Scholar
[83]	Vanacore G M, Berruto G, Madan I, et al. 2019 Nat. Mater. 18 573 Google Scholar
[84]	Harvey T R, Henke J W, Kfir O, Lourenco-Martins H, Feist A, Garcia de Abajo F J, Ropers C 2020 Nano Lett. 20 4377 Google Scholar
[85]	Reinhardt O, Kaminer I 2020 ACS Photonics 7 2859 Google Scholar
[86]	Garcia de Abajo F J, Konecna A 2021 Phys. Rev. Lett. 126 123901 Google Scholar
[87]	Kozák M 2021 ACS Photonics 8 431
[88]	Wong L J, Rivera N, Murdia C, Christensen T, Joannopoulos J D, Soljacic M, Kaminer I 2021 Nat. Commun. 12 1700 Google Scholar
[89]	Zhang B, Ran D, Ianconescu R, Friedman A, Scheuer J, Yariv A, Gover A 2021 arXiv: 2111.13130 [quant-ph]
[90]	Constantin Chirita Mihaila M, Weber P, Schneller M, Grandits L, Nimmrichter S, Juffmann T 2022 arXiv: 2203.07925 [physics. optics]
[91]	Madan I, Leccese V, Mazur A, Barantani F, La Grange T, Sapozhnik A, Gargiulo S, Rotunno E, Olaya J-C, Kaminer I, Grillo V, García de Abajo F J, Carbone F, Vanacore G M 2022 arXiv: 2206.02221 [quant-ph]
[92]	Pomarico E, Madan I, Berruto G, Vanacore G M, Wang K, Kaminer I, García de Abajo F J, Carbone F 2017 ACS Photonics 5 759
[93]	Dahan R, Baranes G, Gorlach A, Ruimy R, Rivera N, Kaminer I 2022 arXiv: 2206.08828 [quant-ph]
[94]	Ramsey N F 1950 Phys. Rev. 78 695 Google Scholar
[95]	Piazza L, Lummen T T, Quinonez E, Murooka Y, Reed B W, Barwick B, Carbone F 2015 Nat. Commun. 6 6407 Google Scholar
[96]	Yurtsever A, Baskin J S, Zewail A H 2012 Nano Lett. 12 5027 Google Scholar
[97]	Yurtsever A, Veen R M v d, Zewail A H 2012 Science 335 59 Google Scholar
[98]	Park S T, Yurtsever A, Baskin J S, Zewail A H 2013 Proc. Natl. Acad. Sci. U. S. A. 110 9277 Google Scholar
[99]	Schreiber A, Cassemiro K N, Potoček V, Gábris A, Jex I, Silberhorn C 2011 Phys. Rev. Lett. 106 180403 Google Scholar
[100]	Broome M A, Fedrizzi A, Lanyon B P, Kassal I, Aspuru-Guzik A, White A G 2010 Phys. Rev. Lett. 104 153602 Google Scholar
[101]	Harris N C, Steinbrecher G R, Prabhu M, et al. 2017 Nat. Photonics 11 447 Google Scholar
[102]	Dadras S, Gresch A, Groiseau C, Wimberger S, Summy G S 2018 Phys. Rev. Lett. 121 070402 Google Scholar
[103]	Karski M, Förster L, Choi J M, Steffen A, Alt W, Meschede D, Widera A 2009 Science 325 174 Google Scholar
[104]	Hong C K, Ou Z Y, Mandel L 1987 Phys. Rev. Lett. 59 2044 Google Scholar
[105]	Leonhardt U, Knight P L, Miller A 1997 Measuring the Quantum State of Light (Cambridge: Cambridge University Press)
[106]	Lvovsky A I, Raymer M G 2009 Rev. Mod. Phys. 81 299 Google Scholar
[107]	Asban S, García de Abajo F J 2021 npj Quantum Inf. 7 42 Google Scholar
[108]	Mutzafi M, Kaminer I, Harari G, Segev M 2017 Nat. Commun. 8 650 Google Scholar
[109]	Dikopoltsev A, Sharabi Y, Lyubarov M, Lumer Y, Tsesses S, Lustig E, Kaminer I, Segev M 2022 Proc. Natl. Acad. Sci. U.S.A. 119 6 e21197051
[110]	Feist J, Fernández-Domínguez A I, García-Vidal F J 2020 Nanophotonics 10 477 Google Scholar
[111]	Konecna A, de Abajo F J G 2020 Phys. Rev. Lett. 125 030801 Google Scholar
[112]	Schlawin F, Cavalleri A, Jaksch D 2019 Phys. Rev. Lett. 122 133602 Google Scholar
[113]	Turner A E, Johnson C W, Kruit P, McMorran B J 2021 Phys. Rev. Lett. 127 110401 Google Scholar
[114]	Shiloh R, Chlouba T, Hommelhoff P 2022 Phys. Rev. Lett. 128 235301 Google Scholar
[115]	Tsesses S, Dahan R, Wang K, Reinhardt O, Bartal G, Kaminer I 2022 arXiv: 2203.08518 [quant-ph]
[116]	Cox J D, Garcia de Abajo F J 2020 Nano Lett. 20 4792 Google Scholar
[117]	Konečná A, Di Giulio V, Mkhitaryan V, Ropers C, García de Abajo F J 2020 ACS Photonics 7 1290 Google Scholar
[118]	Talebi N 2020 Phys. Rev. Lett. 125 080401 Google Scholar
[119]	Luski A, Segev Y, David R, et al. 2021 Science 373 1105 Google Scholar
[120]	Akbari K, Di Giulio V, García de Abajo F J 2022 arXiv: 2203.07257 [physics. optics]

[1]	李锦芳, 何东山, 王一平. 一维耦合腔晶格中磁子-光子拓扑相变和拓扑量子态的调制. , 2024, 73(4): 044203. doi: 10.7498/aps.73.20231519
[2]	郑智勇, 陈立杰, 向吕, 王鹤, 王一平. 一维超导微波腔晶格中反旋波效应对拓扑相变和拓扑量子态的调制. , 2023, 72(24): 244204. doi: 10.7498/aps.72.20231321
[3]	常宸, 孙帅, 杜隆坤, 聂镇武, 何林贵, 张翼, 陈鹏, 鲍可, 刘伟涛. 室外环境中的关联成像研究进展. , 2023, 72(18): 183301. doi: 10.7498/aps.72.20231245
[4]	王伟, 王一平. 一维超导传输线腔晶格中的拓扑相变和拓扑量子态的调制. , 2022, 71(19): 194203. doi: 10.7498/aps.71.20220675
[5]	刘浪, 王一平. 基于可调频光力晶格中声子-光子拓扑性质的模拟和探测. , 2022, 71(22): 224202. doi: 10.7498/aps.71.20221286
[6]	林月钗, 刘仿, 黄翊东. 基于超构材料的Cherenkov辐射. , 2020, 69(15): 154103. doi: 10.7498/aps.69.20200260
[7]	赵士平, 刘玉玺, 郑东宁. 新型超导量子比特及量子物理问题的研究. , 2018, 67(22): 228501. doi: 10.7498/aps.67.20180845
[8]	马亚云, 冯晋霞, 万振菊, 高英豪, 张宽收. 连续变量1.34 m量子纠缠态光场的实验制备. , 2017, 66(24): 244205. doi: 10.7498/aps.66.244205
[9]	冉茂怡, 胡耀垓, 赵正予, 张援农. 高功率微波注入对流层对氟利昂的影响. , 2017, 66(4): 045101. doi: 10.7498/aps.66.045101
[10]	卢道明. 腔量子电动力学系统中耦合三原子的纠缠特性. , 2014, 63(6): 060301. doi: 10.7498/aps.63.060301
[11]	卢道明, 邱昌东. 弱相干场原子-腔-光纤系统中的量子失协. , 2014, 63(11): 110303. doi: 10.7498/aps.63.110303
[12]	李锐奇, 卢道明. 原子与耦合腔相互作用系统中的量子失协. , 2014, 63(3): 030301. doi: 10.7498/aps.63.030301
[13]	王菊霞. 二能级原子与多模光场简并多光子共振相互作用系统中量子保真度的演化特性. , 2014, 63(18): 184203. doi: 10.7498/aps.63.184203
[14]	周媛媛, 张合庆, 周学军, 田培根. 基于标记配对相干态光源的诱骗态量子密钥分配性能分析. , 2013, 62(20): 200302. doi: 10.7498/aps.62.200302
[15]	卢道明. 三参数双模压缩粒子数态的量子特性. , 2012, 61(21): 210302. doi: 10.7498/aps.61.210302
[16]	周媛媛, 周学军. 基于弱相干态光源的非正交编码被动诱骗态量子密钥分配. , 2011, 60(10): 100301. doi: 10.7498/aps.60.100301
[17]	陈达鑫, 陈志峰, 徐初东, 赖天树. 铁磁薄膜中圆偏振光感应的瞬态磁光Kerr峰的物理起源. , 2010, 59(10): 7362-7367. doi: 10.7498/aps.59.7362
[18]	张英杰, 夏云杰, 任廷琦, 杜秀梅, 刘玉玲. 反Jaynes-Cummings模型下纠缠相干光场量子特性的研究. , 2009, 58(2): 722-728. doi: 10.7498/aps.58.722
[19]	江金环, 王永龙, 李子平. 稳态光折变空间孤子传输的量子理论. , 2004, 53(12): 4070-4074. doi: 10.7498/aps.53.4070
[20]	王丹翎, 龚旗煌, 汪凯戈, 杨国健. 光学简并参量振荡中的量子非破坏性测量. , 2000, 49(8): 1484-1489. doi: 10.7498/aps.49.1484

计量

文章访问数: 7956
PDF下载量: 313
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

基于相对论自由电子的量子物理