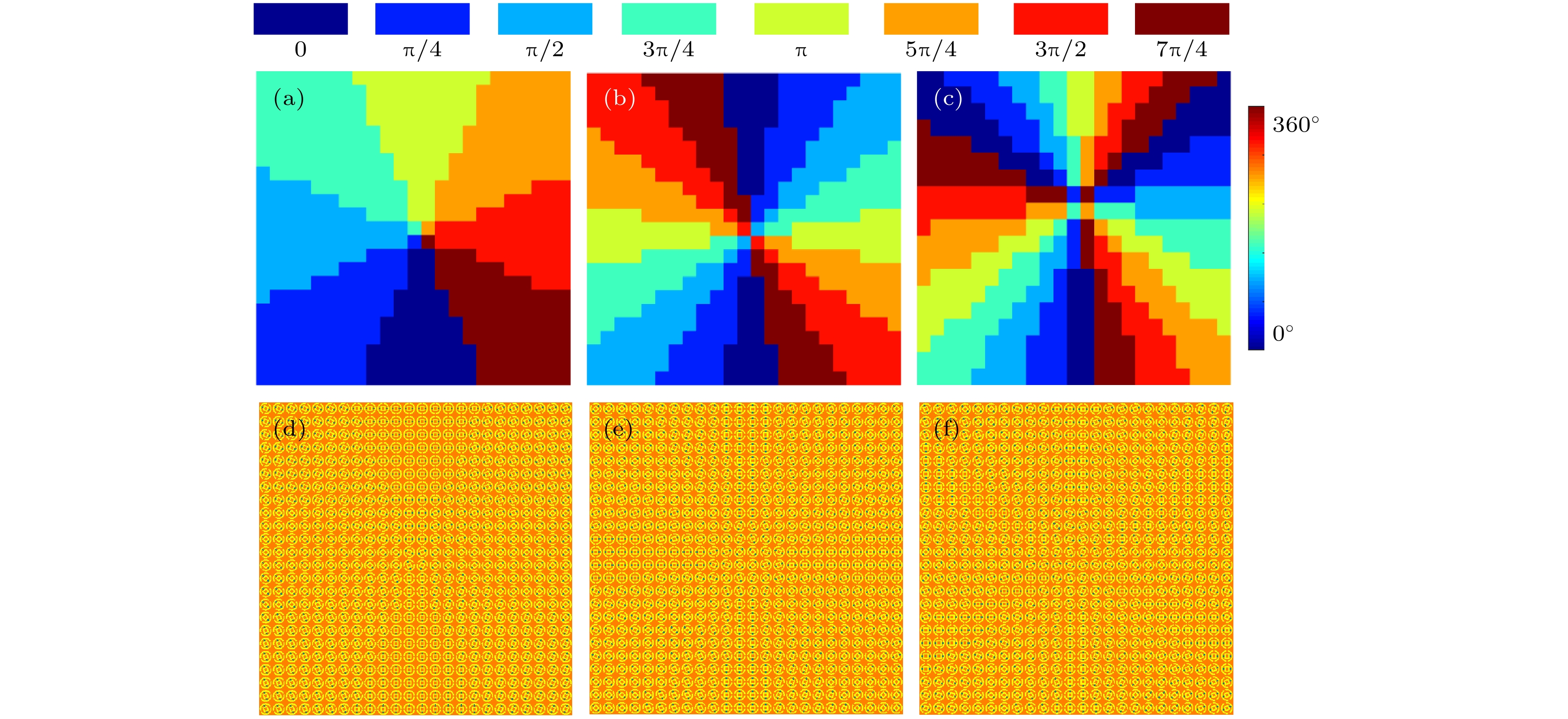
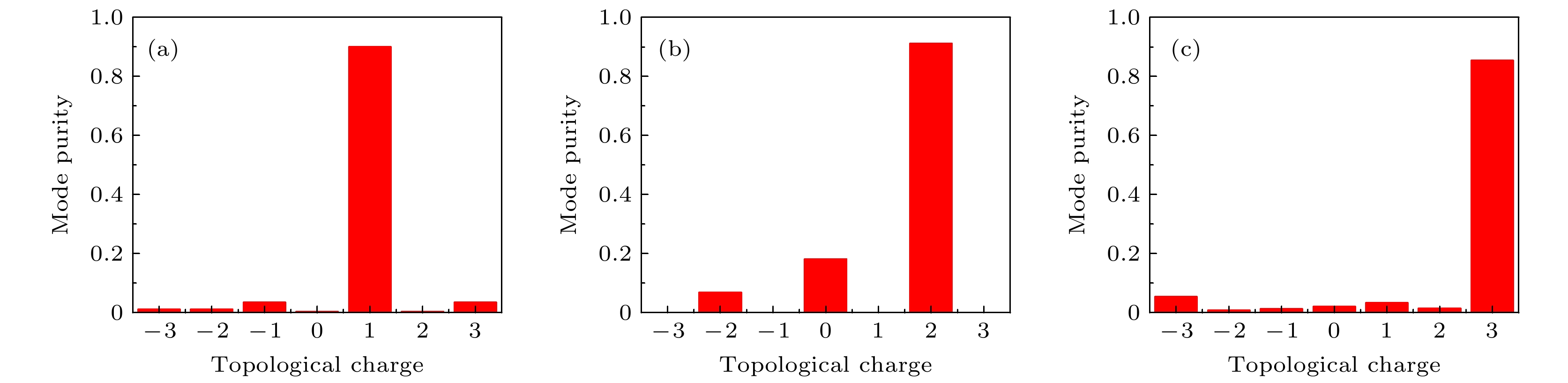
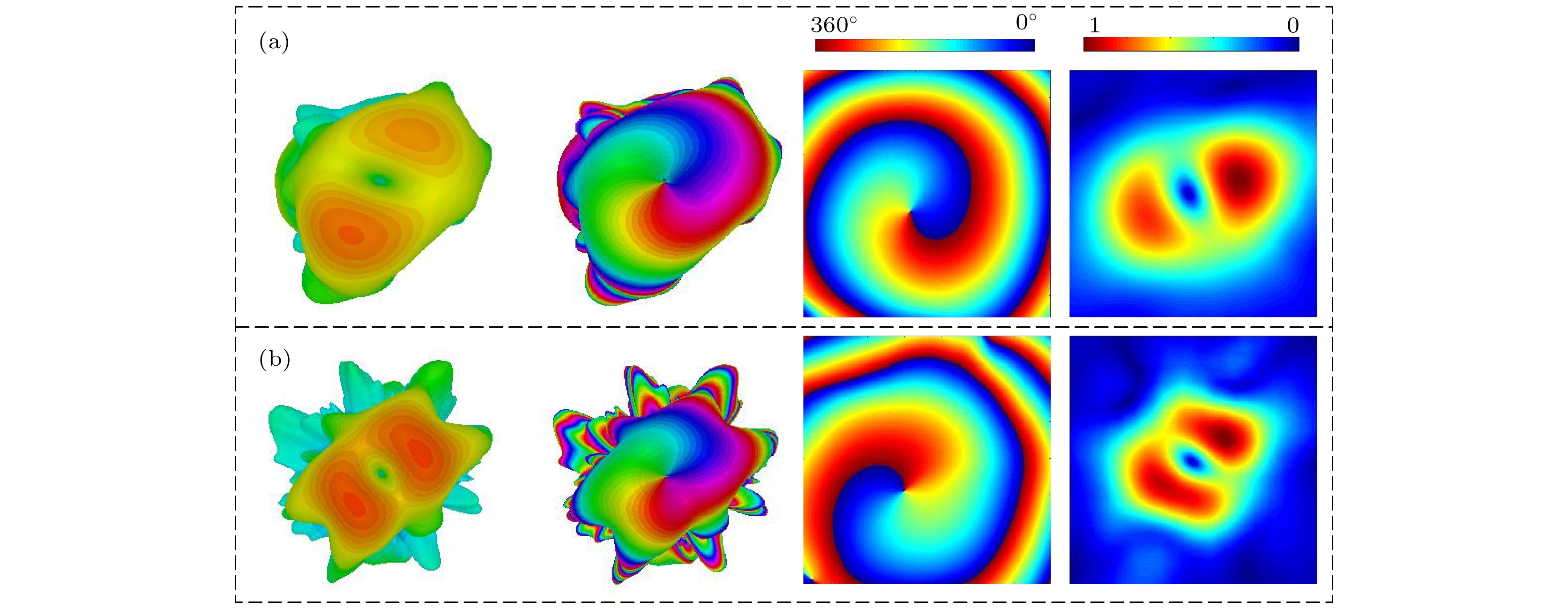
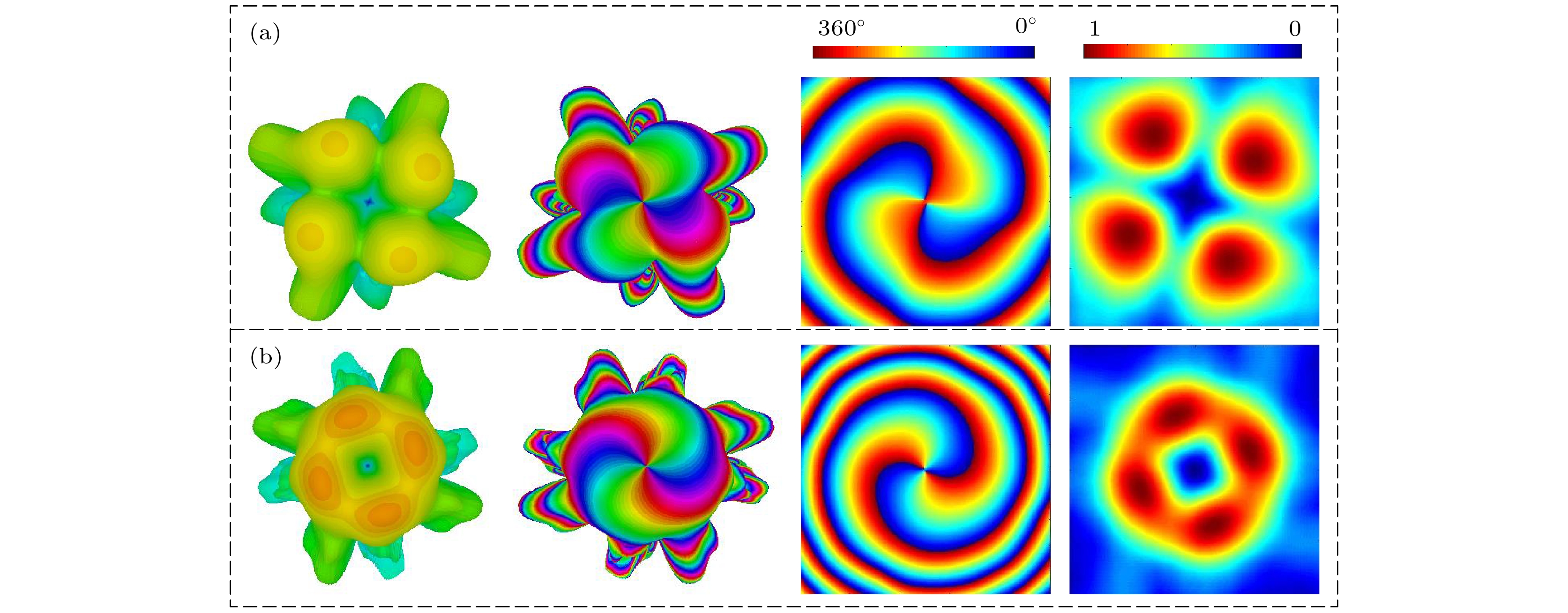
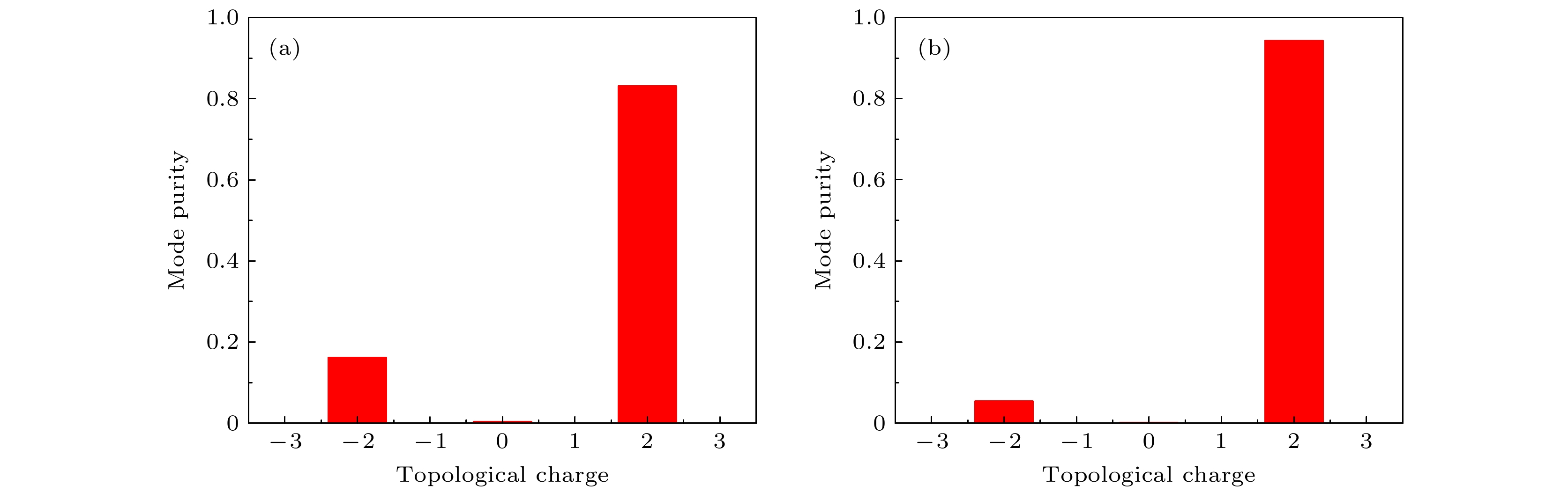
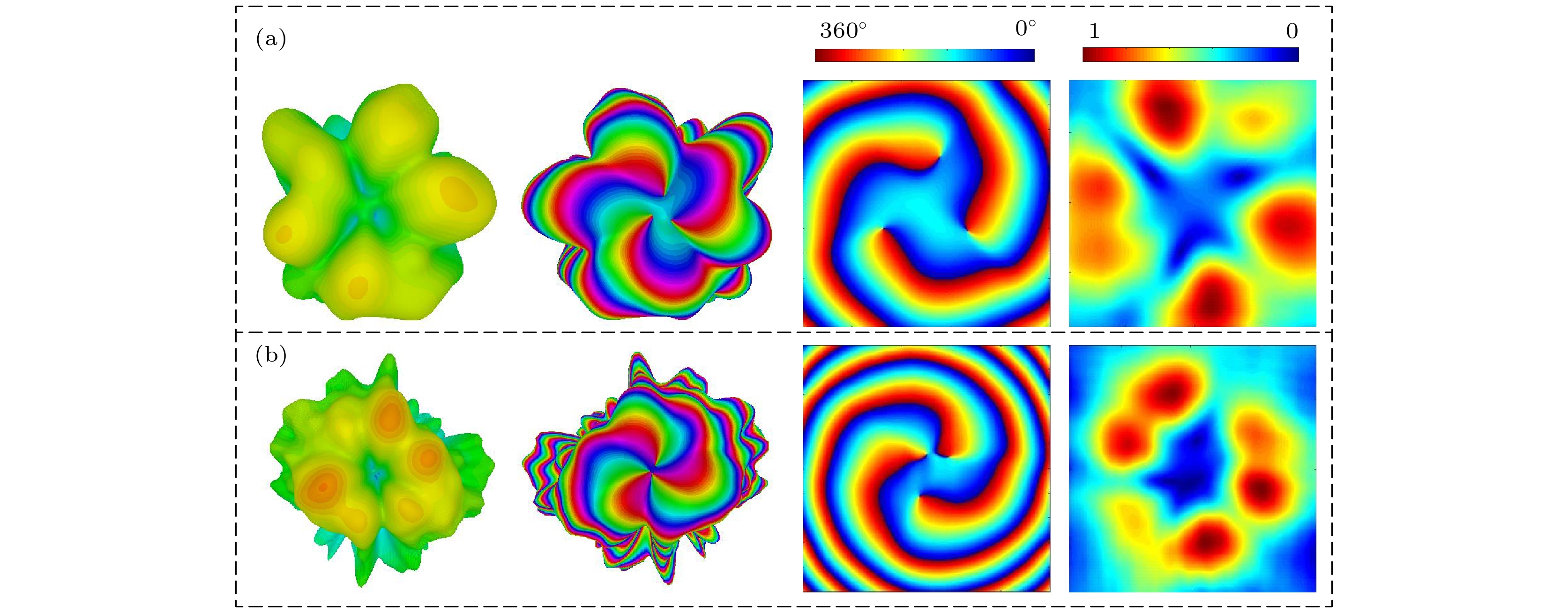
-
已报道的太赫兹涡旋波束产生器大多数是在固定频率产生涡旋波束, 限制了它的实际应用场景. 本文提出一种频率可切换太赫兹涡旋波束超表面, 通过改变外部温度, 二氧化钒相态也随之改变, 该超表面可以实现在单频模式和双频模式的自由切换. 室温下, 所设计的超表面在频率1.1 THz处可以产生具有不同拓扑电荷数的涡旋波束, 而且它们的模式纯度均在 85%以上. 外部温度变为68 ℃时, 该超表面工作频率切换到两个频率点0.7和1.23 THz, 产生不同拓扑荷数的涡旋波束, 模式纯度均大于 60%. 设计的频率可切换太赫兹涡旋产生器为无线太赫兹通信中工作频率调制提供了一个新的设计思路.Most of the reported vortex beam generators generate vortex beams at a fixed frequency, which limits the practical applications. Therefore, it is inevitable to explore a vortex beam generator, which can actively control the operating frequency. We propose a switchable frequency terahertz vortex beam metasurface, it is freely switchable under single-frequency mode and dual-frequency mode by changing the external temperature, the phase state of vanadium dioxide (VO2) is also changeable. External temperature changes will cause VO2 to transform from insulating state to metallic state. Generally, VO2 conductivity can increase by several orders of magnitude as operating temperature changes. By using the phase change property of VO2, we can obtain a metasurface with switchable operating frequencies. For operating at room temperature, the proposed metasurface behaves as a single-frequency terahertz vortex generator. When (left-handed circularly polarized, LCP) terahertz wave is vertically incident on the metasurface, it generates vortex beams with different topological charge numbers at a frequency of 1.1 THz, and the mode purity is above 85%. The simulation results show that the mode purity of the vortex beam with the topological charge l = 1 is 90%, and the mode purity is about 91.1% for the vortex beam with l = 2, and 85.4% for the vortex beam with l = 3. When the external temperature is of 68 ℃, the designed metasurface becomes a dual-frequency vortex beam generator. At this time, the operating frequencies of vortex beams with different topological charges (l = 1, 2, 3) are 0.7 and 1.23 THz, whose mode purities are both above 60%. That is to say, the corresponding mode purities at topological charge with l = 1 for two operating frequencies are 89.1% and 71.6%, respectively. The mode purities are 83.2% and 94.4% with topological charge l = 2, respectively. The mode purities are 62.4% and 68.2% with topological charge l = 3, respectively. Therefore, the proposed switchable frequency terahertz vortex generator provides a new design idea for working frequency modulation in wireless terahertz communication.
-
Keywords:
- terahertz vortex /
- switchable /
- frequency regulation
[1] Wang D, Li N N, Li Z S, Chen C, Lee B, Wang Q H 2022 Opt. Express 30 3157
Google Scholar
[2] Inoue K, Anand A, Cho M 2021 Opt. Lett. 46 1470
Google Scholar
[3] Yang Z B, Tang D Y, Hu J, Tang M J, Zhang M K, Cui H L, Wang L H, Chang C, Fan C H, Li J, Wang H B 2021 Small 17 2005814
Google Scholar
[4] Oda N 2010 C. R. Physique 11 496
Google Scholar
[5] Zhou J, Wang X M, Wang Y X, Huang G R, Yang X, Zhang Y, Xiong Y, Liu L, Zhao X, Fu W L 2021 Talanta 228 122213
Google Scholar
[6] Zhou Z, Cao Z J, Pi Y M 2018 Sensors 18 10
Google Scholar
[7] Han J Q, Li L, Yi H, Shi Y 2018 Opt. Mater. Express 8 3470
Google Scholar
[8] Bao L, Fu X J, Wu R Y, Ma Q, Cui T J 2021 Adv. Mater. Technol. 6 2001032
Google Scholar
[9] 李晓楠, 周璐, 赵国 2019 68 238101
Google Scholar
Li X N, Zhou L, Zhao G Z 2019 Acta Phys. Sin. 68 238101
Google Scholar
[10] Li W Y, Zhao G Z, Meng T H, Sun R, Guo J Y 2021 Chin. Phys. B 30 058103
Google Scholar
[11] Zhang X D, Kong D P, Yuan Y, Mei S, Wang L L, Wang G X 2020 Opt. Commun. 465 125561
Google Scholar
[12] Tang S W, Li X K, Pan W K, Zhou J, Jiang T, Ding F 2019 Opt. Express 27 4281
Google Scholar
[13] Akram M R, Mehmood M Q, Bai X D, Jin R H, Premaratne M, Zhu W R 2019 Adv. Opt. Mater. 7 1801628
Google Scholar
[14] Liu KY, Wang G M, Cai T, Dai B J, Xia Y, Li H P, Guo W L 2019 J. Phys. D: Appl. Phys. 52 255002
Google Scholar
[15] Xin M B, Xie R S, Zhai G H, Gao J J, Zhang D J, Wang X, An S S, Zheng B W, Zhang H L, Ding J 2020 Opt. Express 28 17374
Google Scholar
[16] Xie J F, Guo H M, Zhuang S L, Hu J B 2021 Opt. Express 29 3081
Google Scholar
[17] Cheng K X, Hu Z D, Kong X L, Shen X P, Wang J C 2022 Opt. Commun. 507 127631
Google Scholar
[18] Ding F, Zhong S M, Bozhevolnyi S I 2018 Adv. Opt. Mater. 6 1701204
Google Scholar
[19] Liu X B, Wang Q, Zhang X Q, Li H, Xu Q, Xu Y H, Chen X Y, Li S X, Liu M, Tian Z, Zhang C H, Zou C W, Han J G, Zhang W L 2019 Adv. Opt. Mater. 7 1900175
Google Scholar
[20] Fan J P, Cheng Y Z 2020 J. Phys. D: Appl. Phys. 53 025109
Google Scholar
[21] Bi F, Ba Z L, Wang X 2018 Opt. Express 26 25693
Google Scholar
[22] Jiang S, Chen C, Zhang H L, Chen W D 2018 Opt. Express 26 6466
Google Scholar
-
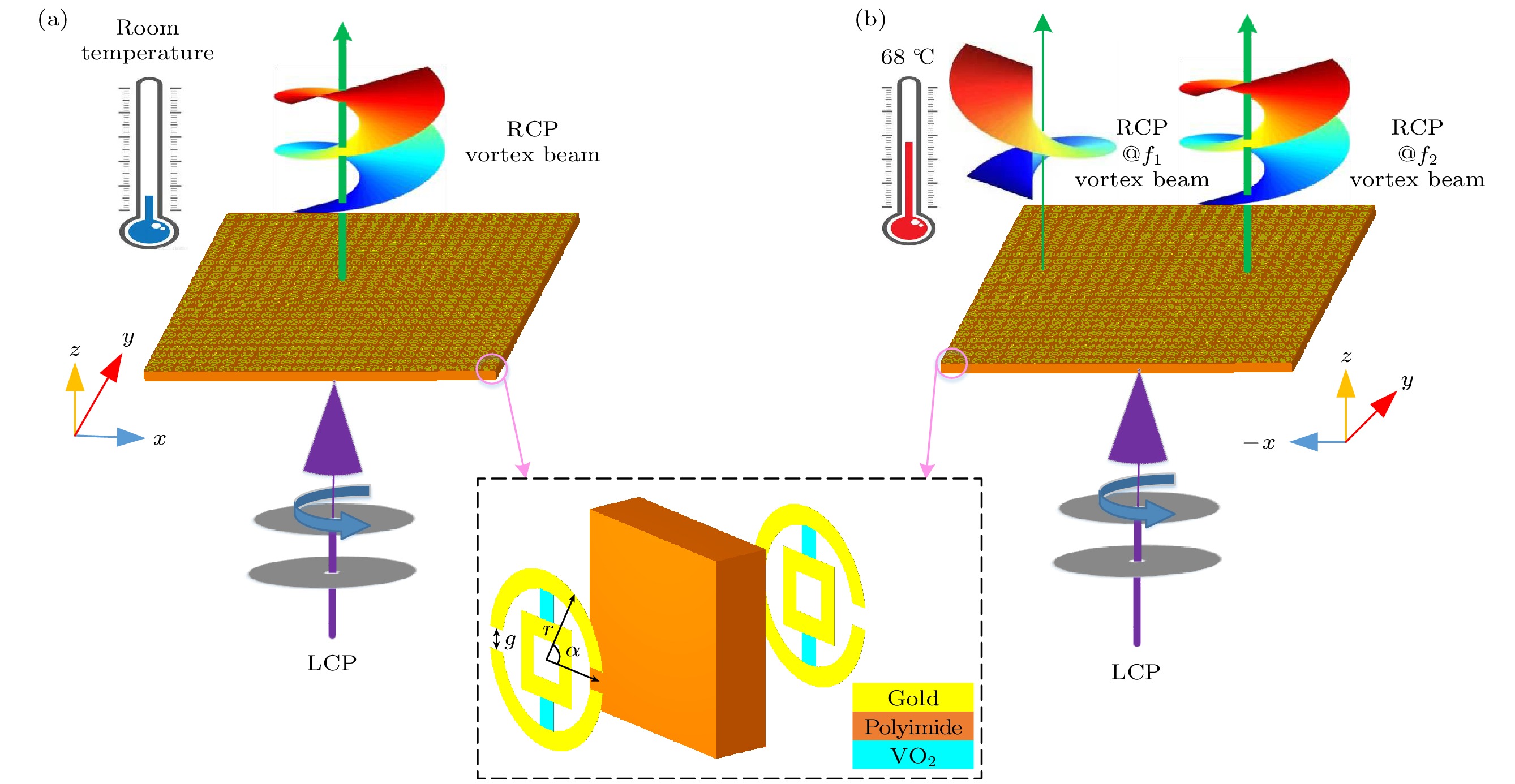
图 1 频率可切换太赫兹涡旋波束调控示意图 (a) 25 ℃时, LCP波入射、RCP涡旋波输出; (b) 68 ℃时, LCP波入射、两个频率的RCP涡旋波输出
Fig. 1. Schematic diagram of switchable frequency terahertz vortex beam regulation: (a) At room temperature, the LCP wave incidence and RCP vortex wave output; (b) at 68 ℃, the LCP wave is incidence and RCP vortex wave output under two frequencies.
图 2 不同温度下超表面单元结构的(a), (c)透射振幅和(b), (d)相位 (a), (b) 室温; (c), (d) 68 ℃
Fig. 2. (a), (c) Transmission amplitudes and (b), (d) phases of metasurface cell structure under different temperatures: (a), (b) Room temperature; (c), (d) 68 ℃.
图 3 l = 1, 2, 3时, 频率可切换太赫兹涡旋波束超表面的(a)—(c)相位分布与(d)—(f)单元阵列排布 (a), (d) l = 1; (b), (e) l = 2; (c), (f) l = 3
Fig. 3. Phase distribution (a)–(c) and cell array arrangement (d)–(f) of switchable frequency terahertz vortex beam metasurface (l = 1, 2, 3): (a), (d) l = 1; (b), (e) l = 2; (c), (f) l = 3.
图 4 室温下, f = 1.1 THz涡旋波束在不同拓扑荷数下的远场强度、远场相位、电场相位和振幅图 (a) l = 1; (b) l = 2; (c) l = 3
Fig. 4. At room temperature, far-field intensity, far-field phase, electric field phase and amplitude of vortex beam with different topological charges at a frequency of 1.1 THz: (a) l = 1; (b) l = 2; (c) l = 3.
图 5 室温下, f = 1.1 THz涡旋波束在不同拓扑荷数下的模式纯度 (a) l = 1; (b) l = 2; (c) l = 3
Fig. 5. At room temperature, mode purity of vortex beam with different topological charges at a frequency of 1.1 THz: (a) l = 1; (b) l = 2; (c) l = 3.
图 6 温度为68 ℃时, 拓扑荷数l = 1的涡旋波束的远场强度、远场相位、电场相位和振幅图 (a) f = 0.7 THz; (b) f = 1.23 THz
Fig. 6. Far-field intensity, far-field phase, electric field phase and amplitude of vortex beam with topological charge l = 1 at 68 ℃: (a) f = 0.7 THz; (b) f = 1.23 THz.
图 7 温度为68 ℃时, 拓扑荷数l = 1的涡旋波束的模式纯度 (a) f = 0.7 THz; (b) f = 1.23 THz
Fig. 7. Mode purity of vortex beam with topological charge l = 1 at 68 ℃: (a) f = 0.7 THz; (b) f = 1.23 THz.
图 8 温度为68 ℃时, 拓扑荷数l = 2的涡旋波束远场强度、远场相位、电场相位和振幅图 (a) f = 0.7 THz; (b) f = 1.23 THz
Fig. 8. Far-field intensity, far-field phase, electric field phase and amplitude of vortex beam with topological charge l = 2 at 68 ℃: (a) f = 0.7 THz; (b) f = 1.23 THz.
图 9 68 ℃时, 拓扑荷数l = 2的涡旋波束的模式纯度 (a) f = 0.7 THz; (b) f = 1.23 THz
Fig. 9. Mode purity of vortex beam with topological charge l = 2 at 68 ℃: (a) f = 0.7 THz; (b) f = 1.23 THz.
图 10 温度为68 ℃时, 拓扑荷数l = 3的涡旋波束远场强度、远场相位、电场相位和振幅图 (a) f = 0.7 THz; (b) f = 1.23 THz
Fig. 10. Far-field intensity, far-field phase, electric field phase and amplitude of vortex beam with topological charge l = 3 at 68 ℃: (a) f = 0.7 THz; (b) f = 1.23 THz.
图 11 温度为68 ℃时, 拓扑荷数l = 3的涡旋波束的模式纯度 (a) f = 0.7 THz; (b) f = 1.23 THz
Fig. 11. Mode purity of vortex beam with topological charge l = 3 at 68 ℃: (a) f = 0.7 THz; (b) f = 1.23 THz.
-
[1] Wang D, Li N N, Li Z S, Chen C, Lee B, Wang Q H 2022 Opt. Express 30 3157
Google Scholar
[2] Inoue K, Anand A, Cho M 2021 Opt. Lett. 46 1470
Google Scholar
[3] Yang Z B, Tang D Y, Hu J, Tang M J, Zhang M K, Cui H L, Wang L H, Chang C, Fan C H, Li J, Wang H B 2021 Small 17 2005814
Google Scholar
[4] Oda N 2010 C. R. Physique 11 496
Google Scholar
[5] Zhou J, Wang X M, Wang Y X, Huang G R, Yang X, Zhang Y, Xiong Y, Liu L, Zhao X, Fu W L 2021 Talanta 228 122213
Google Scholar
[6] Zhou Z, Cao Z J, Pi Y M 2018 Sensors 18 10
Google Scholar
[7] Han J Q, Li L, Yi H, Shi Y 2018 Opt. Mater. Express 8 3470
Google Scholar
[8] Bao L, Fu X J, Wu R Y, Ma Q, Cui T J 2021 Adv. Mater. Technol. 6 2001032
Google Scholar
[9] 李晓楠, 周璐, 赵国 2019 68 238101
Google Scholar
Li X N, Zhou L, Zhao G Z 2019 Acta Phys. Sin. 68 238101
Google Scholar
[10] Li W Y, Zhao G Z, Meng T H, Sun R, Guo J Y 2021 Chin. Phys. B 30 058103
Google Scholar
[11] Zhang X D, Kong D P, Yuan Y, Mei S, Wang L L, Wang G X 2020 Opt. Commun. 465 125561
Google Scholar
[12] Tang S W, Li X K, Pan W K, Zhou J, Jiang T, Ding F 2019 Opt. Express 27 4281
Google Scholar
[13] Akram M R, Mehmood M Q, Bai X D, Jin R H, Premaratne M, Zhu W R 2019 Adv. Opt. Mater. 7 1801628
Google Scholar
[14] Liu KY, Wang G M, Cai T, Dai B J, Xia Y, Li H P, Guo W L 2019 J. Phys. D: Appl. Phys. 52 255002
Google Scholar
[15] Xin M B, Xie R S, Zhai G H, Gao J J, Zhang D J, Wang X, An S S, Zheng B W, Zhang H L, Ding J 2020 Opt. Express 28 17374
Google Scholar
[16] Xie J F, Guo H M, Zhuang S L, Hu J B 2021 Opt. Express 29 3081
Google Scholar
[17] Cheng K X, Hu Z D, Kong X L, Shen X P, Wang J C 2022 Opt. Commun. 507 127631
Google Scholar
[18] Ding F, Zhong S M, Bozhevolnyi S I 2018 Adv. Opt. Mater. 6 1701204
Google Scholar
[19] Liu X B, Wang Q, Zhang X Q, Li H, Xu Q, Xu Y H, Chen X Y, Li S X, Liu M, Tian Z, Zhang C H, Zou C W, Han J G, Zhang W L 2019 Adv. Opt. Mater. 7 1900175
Google Scholar
[20] Fan J P, Cheng Y Z 2020 J. Phys. D: Appl. Phys. 53 025109
Google Scholar
[21] Bi F, Ba Z L, Wang X 2018 Opt. Express 26 25693
Google Scholar
[22] Jiang S, Chen C, Zhang H L, Chen W D 2018 Opt. Express 26 6466
Google Scholar
下载:
计量
- 文章访问数: 3198
- PDF下载量: 66
- 被引次数: 0
