-
With the increasing demand for potential applications of vortex beams in various fields of modern optics, the generation of optical vortex beams has attracted great interest. Based on a flat plate made of an electro-optical crystal, a method to generate optical vortex beams assisted by the Pockels effect is proposed. This method allows an orbital-angular-momentum-tunable range of
$ \pm 2\hbar$ with a finite phase-modulated region. We simulate the propagation of optical beams transmitted from the flat plate and investigate the orbital-angular-momentum-mode spectra of the transmitted optical beams. The mode spectra accord well with the simulation results. The proposed method will be applied to fields where tunable optical vortex beams are required, such as optical communication and optical manipulation.-
Keywords:
- phase modulation /
- orbital angular momentum /
- vortex beam
[1] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Phys. Rev. A 45 8185
 Google Scholar
Google Scholar
[2] Xie Z, Gao S, Lei T, Feng S, Zhang Y, Li F, Zhang J, Li Z, Yuan X 2018 Photon. Res. 6 743
 Google Scholar
Google Scholar
[3] Gecevičius M, Drevinskas R, Beresna M, Kazansky P G 2014 Appl. Phys. Lett. 104 231110
 Google Scholar
Google Scholar
[4] Li Y, Zhou L M, Zhao N 2021 Opt. Lett. 46 106
 Google Scholar
Google Scholar
[5] Kozawa Y, Matsunaga D, Sato S 2018 Optica 5 86
 Google Scholar
Google Scholar
[6] Stav T, Faerman A, Maguid E, Oren D, Kleiner V, Hasman E, Segev M 2018 Science 361 1101
 Google Scholar
Google Scholar
[7] Beijersbergen M W, Coerwinkel R P C, Kristensen M, Woerdman J P 1994 Opt. Commun. 112 321
 Google Scholar
Google Scholar
[8] Sueda K, Miyaji G, Miyanaga N, Nakatsuka M 2004 Opt. Express 12 3548
 Google Scholar
Google Scholar
[9] Khonina S N, Podlipnov V V, Karpeev S V, Ustinov A V, Volotovsky S G, Ganchevskaya S V 2020 Opt. Express 28 18407
 Google Scholar
Google Scholar
[10] Marrucci L, Manzo C, Paparo D 2006 Phys. Rev. Lett. 96 163905
 Google Scholar
Google Scholar
[11] Piccirillo B, D'Ambrosio V, Slussarenko S, Marrucci L, Santamato E 2010 Appl. Phys. Lett. 97 241104
 Google Scholar
Google Scholar
[12] Brasselet E 2018 Phys. Rev. Lett. 121 033901
 Google Scholar
Google Scholar
[13] Forbes A, Dudley A, McLaren M 2016 Adv. Opt. Photon. 8 200
 Google Scholar
Google Scholar
[14] Shalaev M I, Sun J, Tsukernik A, Pandey A, Nikolskiy K, Litchinitser N M 2015 Nano Lett. 15 6261
 Google Scholar
Google Scholar
[15] Yu N, Genevet P, Kats M A, Aieta F, Tetienne J P, Capasso F, Gaburro Z 2011 Science 334 333
 Google Scholar
Google Scholar
[16] Cai X, Wang J, Strain M J, Johnson-Morris B, Zhu J, Sorel M, O’Brien J L, Thompson M G, Yu S 2012 Science 338 363
 Google Scholar
Google Scholar
[17] Wang A, Zhu L, Liu J, Du C, Mo Q, Wang J 2015 Opt. Express 23 29457
 Google Scholar
Google Scholar
[18] Lyubopytov V S, Porfirev A P, Gurbatov S O, Paul S, Schumann M F, Cesar J, Malekizi M, Haidar M T, Wegener M, Chipouline A, Küppers F 2017 Opt. Express 25 9634
 Google Scholar
Google Scholar
[19] Zhang W, Wei K, Huang L, Mao D, Jiang B, Gao F, Zhang G, Mei T, Zhao J 2016 Opt. Express 24 19278
 Google Scholar
Google Scholar
[20] Yao S, Ren G, Shen Y, JiangY, Zhu B, Jian S 2018 IEEE Photon. Tech. Lett. 30 99
 Google Scholar
Google Scholar
[21] Shen Y, Meng Y, Fu X, Gong M 2018 Opt. Lett. 43 291
 Google Scholar
Google Scholar
[22] Wang S, Zhang S-l, Li P, Hao M h, Yang H m, Xie J, Feng G Y, Zhou S h 2018 Opt. Express 26 18164
 Google Scholar
Google Scholar
[23] Zhang Z, Qiao X, Midya B, Liu K, Sun J, Wu T, Liu W, Agarwal R, Jornet J M, Longhi S, Litchinitser N M, Feng L 2020 Science 368 760
 Google Scholar
Google Scholar
[24] Ji Z, Liu W, Krylyuk S, Fan X, Zhang Z, Pan A, Feng L, Davydov A, Agarwal R 2020 Science 368 763
 Google Scholar
Google Scholar
[25] Thomaschewski M, ZeninV A, Wolff C, Bozhevolnyi S I 2020 Nat. Commun. 11 748
 Google Scholar
Google Scholar
[26] Alexander K, George J P, Verbist J, Neyts K, Kuyken B, Thourhout D Van, Beeckman J 2018 Nat. Commun. 9 3444
 Google Scholar
Google Scholar
[27] Boyd RW 2008 Nonlinear Optics (Third Edition) (Beijing: Academic Press)
[28] Hourmand M, Sarhan A A D, Sayuti M 2017 Int. J. Adv. Manuf. Tech. 91 1023
 Google Scholar
Google Scholar
[29] Kulkarni G U, Kiruthika S, Gupta R, Rao K D M 2015 Curr. Opin. Chem. Eng. 8 60
 Google Scholar
Google Scholar
[30] Zhu W, She W 2012 Opt. Express 20 25876
 Google Scholar
Google Scholar
[31] Khonina S N, Podlipnov V V, Volotovskiĭ S G 2018 J. Opt. Tech. 85 388
 Google Scholar
Google Scholar
[32] Chu H, Li Y, Zhao S 2011 Appl. Opt. 50 360
 Google Scholar
Google Scholar
[33] Zhang M, BuscainB, Wang C, Shams-Ansari A, Reimer C, Zhu R, Kahn J M, Lončar M 2019 Nature 568 373
 Google Scholar
Google Scholar
[34] Lao G M, Zhang Z H, Zhao D M 2016 Opt. Express 24 18082
 Google Scholar
Google Scholar
[35] Molina-Terriza G, Torres J P, Torner L 2001 Phys. Rev. Lett. 88 013601
 Google Scholar
Google Scholar
-
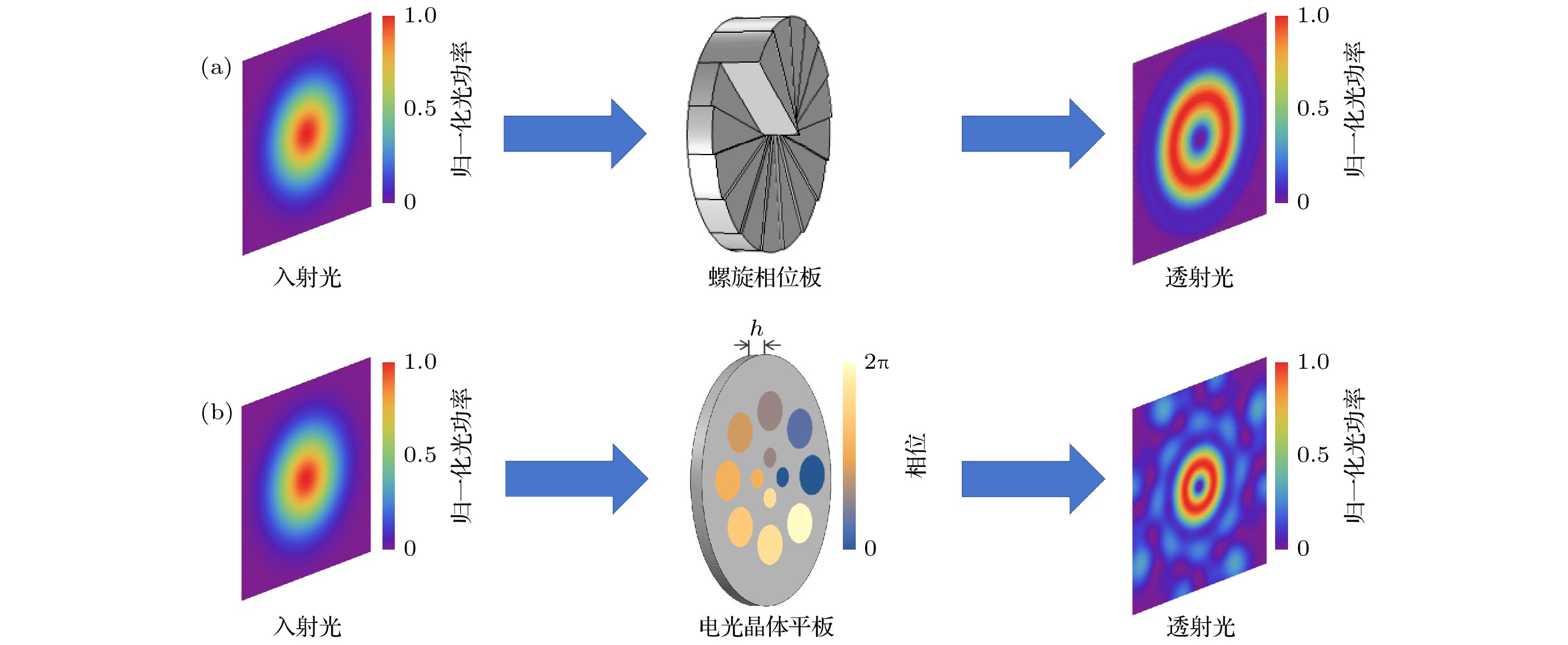
图 1 (a)通过传统SPP产生的涡旋光束, 入射光具有高斯强度分布; (b)通过由电光晶体制成的平板产生涡旋光束, 其中局部折射率由微电极板阵列控制, 从而部分调制入射光的相位
Fig. 1. (a) The generation of optical vortex beams through a traditional SPP, the incident light has a Gaussian intensity distribution; (b) the generation of optical vortex beams through a flat plate made of electro-optical crystals, where the local refractive index is controlled by a micro-electrode plate array so that the phase of the incident light is partially modulated.
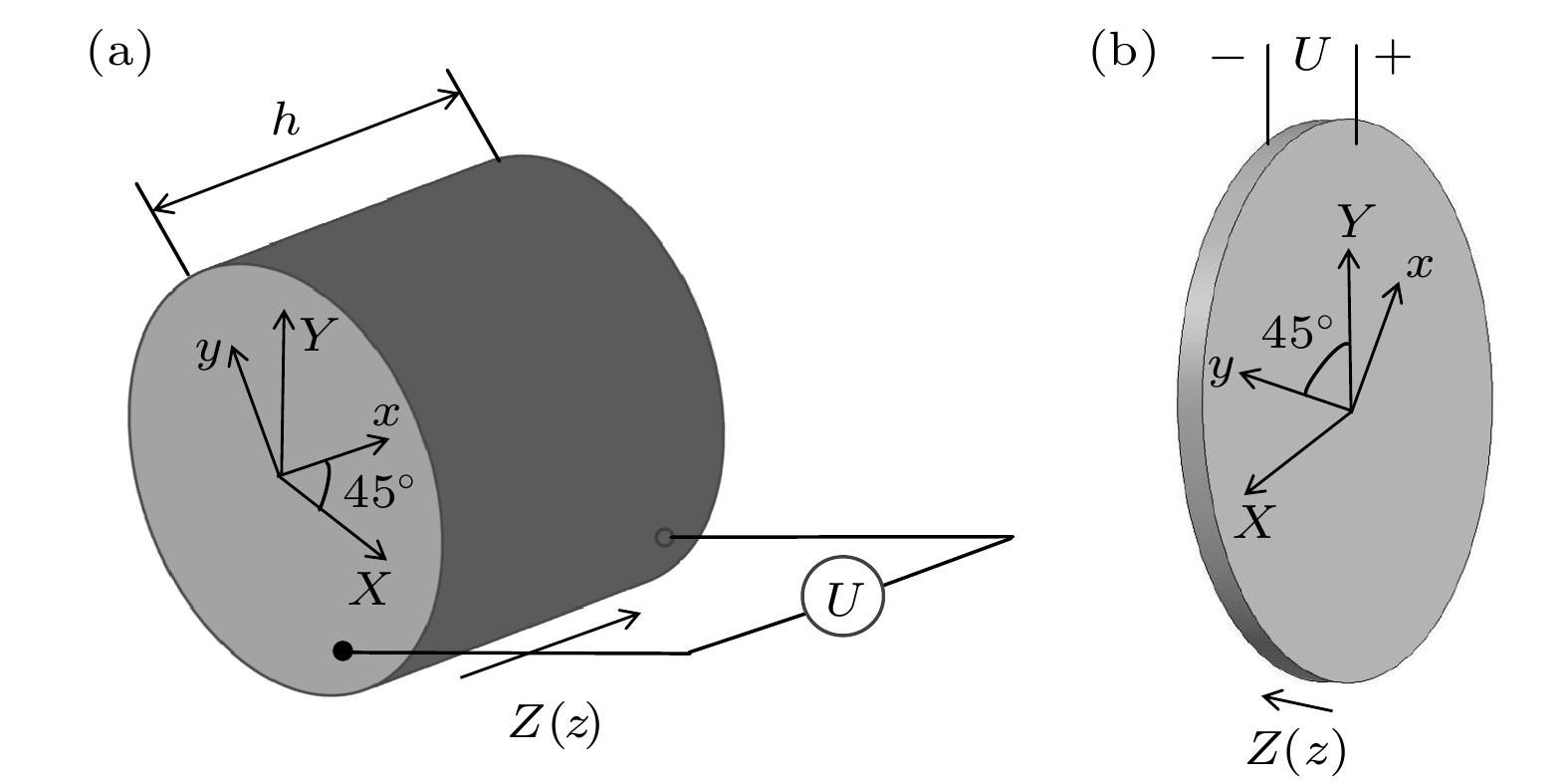
图 2 电场施加区域之一的示意图. XYZ坐标是没有施加电场的KDP晶体的所谓主轴坐标. 晶体的光轴沿Z方向. 当施加外部电场时, 由于 Pockels效应[29], 主轴围绕Z轴旋转45°
Fig. 2. Schematic of one of the electric-field-applied regions. The XYZ coordinate is the so-called principal-axis coordinate of the KDP crystal with no applied electric field. The crystal is prepared cut so that the optical axis is in the Z direction. When an external electric field applies, the principal axes rotate 45° about the Z-axis due to the Pockels effect [29].
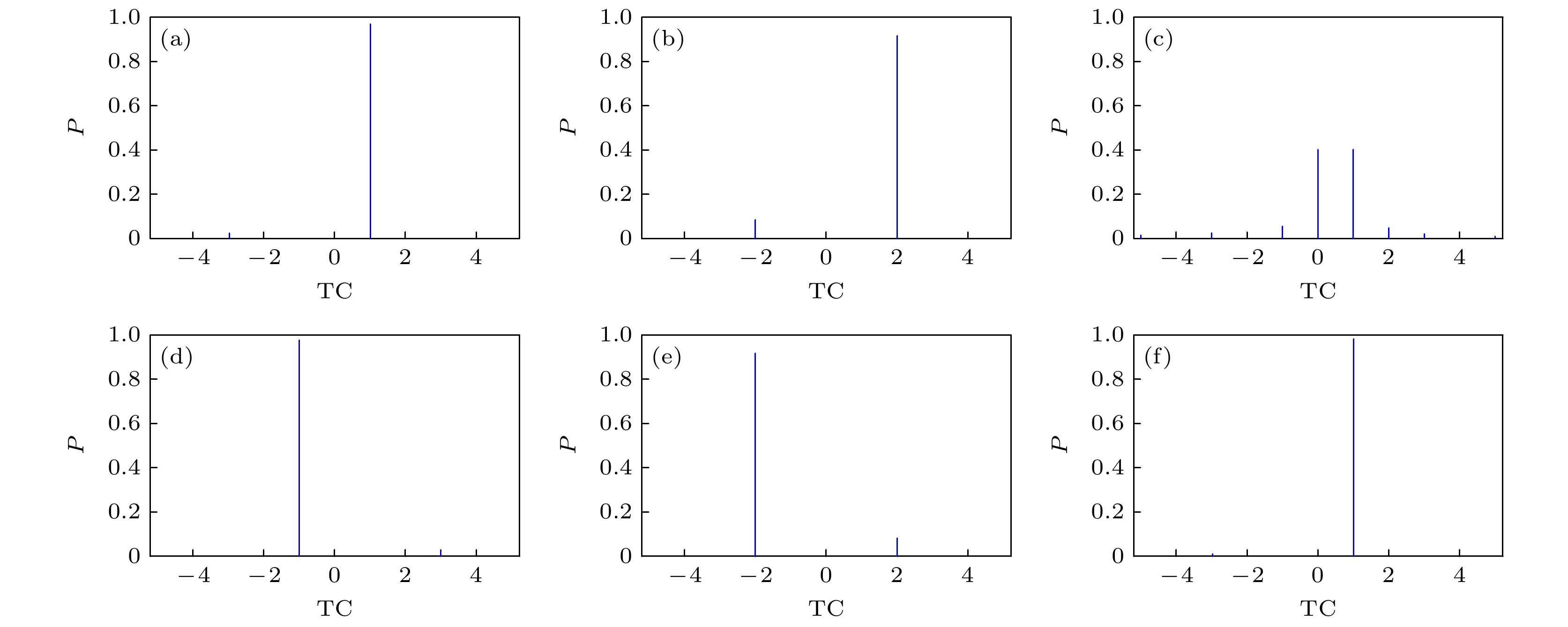
图 3 所有入射光穿过板时, 透射光在自由空间中传播时的光强分布. 第1列中的黑色圆圈表示具有高斯模式的入射光的调制区域, 白色值表示方位角调制的光学相位, 其相应的施加电压可以通过(4)式计算, 未调制区域相应的光学相位为
${{\text{π}}/4}$ (a)$ 2{\text{π }} $ 相移; (b)$-2{\text{π }} $ 相移; (c)$ 4{\text{π }} $ 相移; (d)$ -4{\text{π }} $ 相移; (e)$ {\text{π }} $ 相移Fig. 3. The intensity distribution of the transmitted light propagating in free space for the case that the whole incident light passing through the plate. The black circles in the first column denote the modulation regions of the incident light with Gaussian mode, and the white values represent the azimuthally modulated optical phase whose corresponding applied voltages can be calculated by Eq. (4). The corresponding optical phase of the unmodulated region is
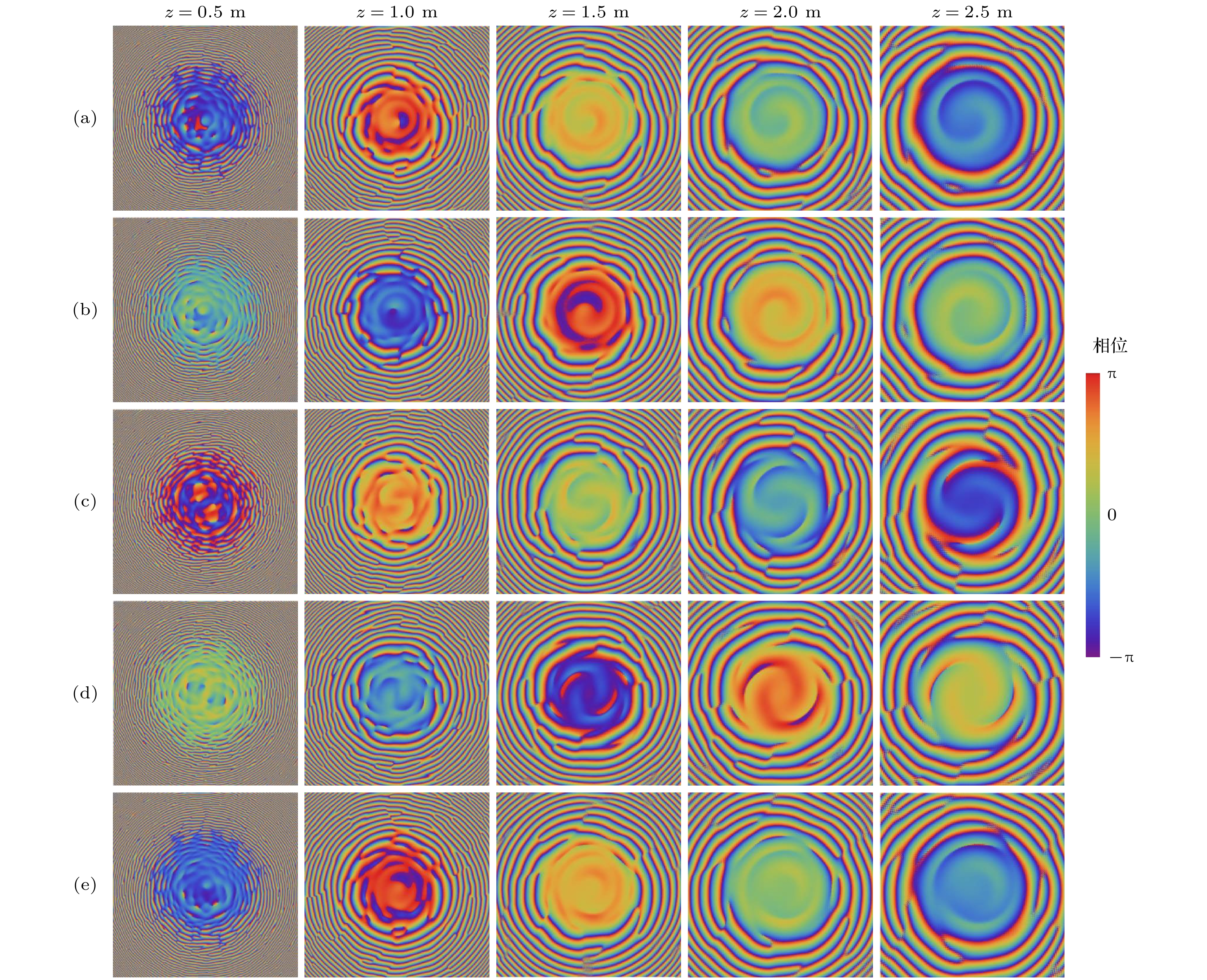
${{\text{π}}/4}$ : (a)$ 2{\text{π}} $ phase shift; (b)$ -2{\text{π}} $ phase shift; (c)$ 4{\text{π}} $ phase shift; (d)$-4{\text{π}} $ phase shift; (e)$ {\text{π}} $ phase shift.图 4 所有入射光穿过板时, 透射光在自由空间中传播时的相位分布. 每一行的相位调制方案与图3中的相位调制方案一致 (a)
$ 2{\text{π }} $ 相移; (b)$-2{\text{π }} $ 相移; (c)$ 4{\text{π }} $ 相移; (d)$ -4{\text{π }} $ 相移; (e)$ {\text{π }} $ 相移Fig. 4. The phase distribution of the transmitted light propagating in free space for the case that the whole incident light passing through the plate. The phase modulation scheme of each row is consistent with those of Fig. 3: (a)
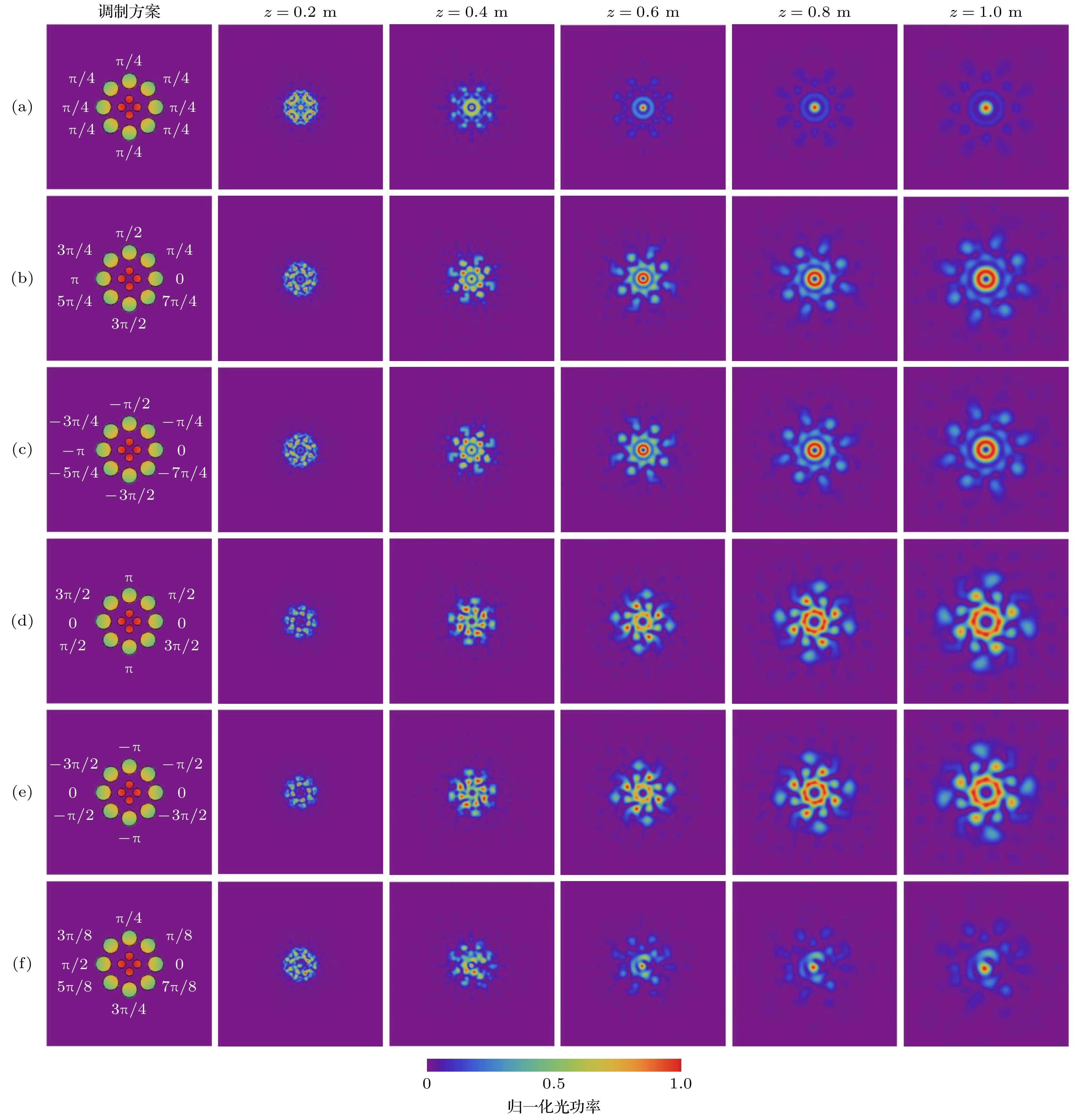
$ 2{\text{π }} $ phase shift; (b)$ -2{\text{π }} $ phase shift; (c)$ 4{\text{π }} $ phase shift; (d)$ -4{\text{π }} $ phase shift; (e)$ {\text{π }} $ phase shift.图 5 只有调制区域的入射光通过板时, 透射光在自由空间中传播时的光强分布. 第1列显示了调制方案 (a) 没有施加电场; (b)
$ 2{\text{π }} $ 相移; (c)$ -2{\text{π }} $ 相移; (d)$ 4{\text{π }} $ 相移; (e)$ -4{\text{π }} $ 相移; (f)$ {\text{π }} $ 相移Fig. 5. The intensity distribution of the transmitted light propagating in free space for the case that only the modulated regions of the incident light passing through the plate. The first column shows the modulation schemes for (a) absence of applied electric field; (b)
$ 2{\text{π }} $ phase shift; (c)$-2{\text{π }} $ phase shift; (d)$ 4{\text{π }} $ phase shift; (e)$ -4{\text{π }} $ phase shift; (f)$ {\text{π }} $ phase shift.图 6 只有调制区域的入射光通过板时, 透射光在自由空间中传播时的相位分布. 每一行的相位调制方案与图5中的相位调制方案一致 (a) 没有施加电场; (b)
$ 2{\text{π }} $ 相移; (c)$ -2{\text{π }} $ 相移; (d)$ 4{\text{π }} $ 相移; (e)$ -4{\text{π }} $ 相移; (f)$ {\text{π }} $ 相移.Fig. 6. The phase distribution of the transmitted light propagating in free space for the case that only the modulated regions of the incident light passing through the plate. The phase modulation scheme of each row is consistent with that in Fig. 5: (a) Absence of applied electric field; (b)
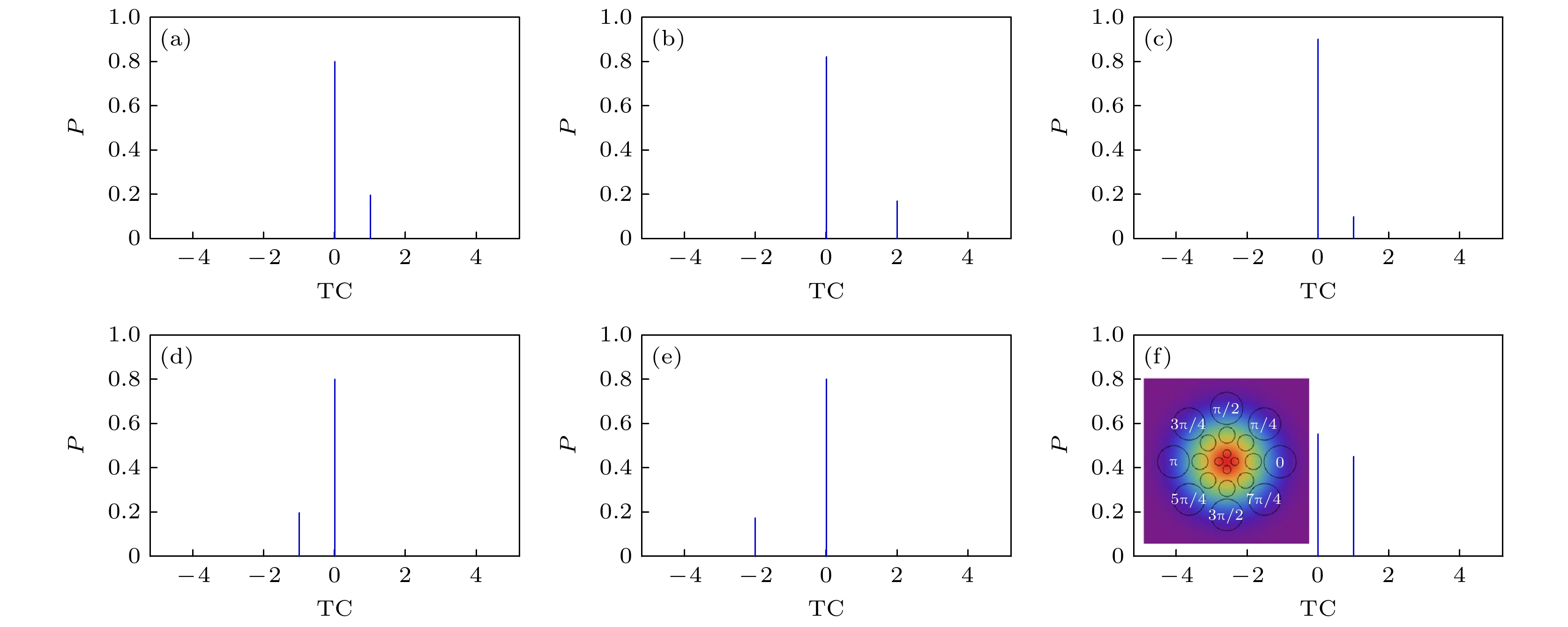
$ 2{\text{π }} $ phase shift; (c)$ -2{\text{π }} $ phase shift; (d)$ 4{\text{π }} $ phase shift; (e)$ -4{\text{π }} $ phase shift; (f)$ {\text{π }} $ phase shift.图 7 所有入射光通过板时, 不同调制方案的OAM模式光谱 (a)—(e) 的调制方案分别与图3(a)—(e) 中的相同; (f) 插入显示调制方案的模式频谱, 其中也实现了
$ 2{\text{π }} $ 相移, 但调制区域的面积大于 (a) 中的区域Fig. 7. OAM-mode spectra with different modulation schemes for the case that the whole incident light passing through the plate. The modulation schemes of (a)–(e) are the same as in Fig. 3 (a)-(e), respectively. (f) The mode spectrum with the insert showing the modulation scheme, in which a
$ 2{\text{π }} $ phase shift is also achieved but the area of modulated regions is more than the one in (a).图 8 仅调制区域的入射光通过板时, 具有不同调制方案的OAM模式谱. (a)—(f)的调制方案分别与图7(a)—(f)相同
Fig. 8. OAM-mode spectra with different modulation schemes for the case that only the modulated regions of the incident light passing through the plate. The modulation schemes of (a)–(f) are the same as in Fig. 7 (a)-(f), respectively.
-
[1] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Phys. Rev. A 45 8185
 Google Scholar
Google Scholar
[2] Xie Z, Gao S, Lei T, Feng S, Zhang Y, Li F, Zhang J, Li Z, Yuan X 2018 Photon. Res. 6 743
 Google Scholar
Google Scholar
[3] Gecevičius M, Drevinskas R, Beresna M, Kazansky P G 2014 Appl. Phys. Lett. 104 231110
 Google Scholar
Google Scholar
[4] Li Y, Zhou L M, Zhao N 2021 Opt. Lett. 46 106
 Google Scholar
Google Scholar
[5] Kozawa Y, Matsunaga D, Sato S 2018 Optica 5 86
 Google Scholar
Google Scholar
[6] Stav T, Faerman A, Maguid E, Oren D, Kleiner V, Hasman E, Segev M 2018 Science 361 1101
 Google Scholar
Google Scholar
[7] Beijersbergen M W, Coerwinkel R P C, Kristensen M, Woerdman J P 1994 Opt. Commun. 112 321
 Google Scholar
Google Scholar
[8] Sueda K, Miyaji G, Miyanaga N, Nakatsuka M 2004 Opt. Express 12 3548
 Google Scholar
Google Scholar
[9] Khonina S N, Podlipnov V V, Karpeev S V, Ustinov A V, Volotovsky S G, Ganchevskaya S V 2020 Opt. Express 28 18407
 Google Scholar
Google Scholar
[10] Marrucci L, Manzo C, Paparo D 2006 Phys. Rev. Lett. 96 163905
 Google Scholar
Google Scholar
[11] Piccirillo B, D'Ambrosio V, Slussarenko S, Marrucci L, Santamato E 2010 Appl. Phys. Lett. 97 241104
 Google Scholar
Google Scholar
[12] Brasselet E 2018 Phys. Rev. Lett. 121 033901
 Google Scholar
Google Scholar
[13] Forbes A, Dudley A, McLaren M 2016 Adv. Opt. Photon. 8 200
 Google Scholar
Google Scholar
[14] Shalaev M I, Sun J, Tsukernik A, Pandey A, Nikolskiy K, Litchinitser N M 2015 Nano Lett. 15 6261
 Google Scholar
Google Scholar
[15] Yu N, Genevet P, Kats M A, Aieta F, Tetienne J P, Capasso F, Gaburro Z 2011 Science 334 333
 Google Scholar
Google Scholar
[16] Cai X, Wang J, Strain M J, Johnson-Morris B, Zhu J, Sorel M, O’Brien J L, Thompson M G, Yu S 2012 Science 338 363
 Google Scholar
Google Scholar
[17] Wang A, Zhu L, Liu J, Du C, Mo Q, Wang J 2015 Opt. Express 23 29457
 Google Scholar
Google Scholar
[18] Lyubopytov V S, Porfirev A P, Gurbatov S O, Paul S, Schumann M F, Cesar J, Malekizi M, Haidar M T, Wegener M, Chipouline A, Küppers F 2017 Opt. Express 25 9634
 Google Scholar
Google Scholar
[19] Zhang W, Wei K, Huang L, Mao D, Jiang B, Gao F, Zhang G, Mei T, Zhao J 2016 Opt. Express 24 19278
 Google Scholar
Google Scholar
[20] Yao S, Ren G, Shen Y, JiangY, Zhu B, Jian S 2018 IEEE Photon. Tech. Lett. 30 99
 Google Scholar
Google Scholar
[21] Shen Y, Meng Y, Fu X, Gong M 2018 Opt. Lett. 43 291
 Google Scholar
Google Scholar
[22] Wang S, Zhang S-l, Li P, Hao M h, Yang H m, Xie J, Feng G Y, Zhou S h 2018 Opt. Express 26 18164
 Google Scholar
Google Scholar
[23] Zhang Z, Qiao X, Midya B, Liu K, Sun J, Wu T, Liu W, Agarwal R, Jornet J M, Longhi S, Litchinitser N M, Feng L 2020 Science 368 760
 Google Scholar
Google Scholar
[24] Ji Z, Liu W, Krylyuk S, Fan X, Zhang Z, Pan A, Feng L, Davydov A, Agarwal R 2020 Science 368 763
 Google Scholar
Google Scholar
[25] Thomaschewski M, ZeninV A, Wolff C, Bozhevolnyi S I 2020 Nat. Commun. 11 748
 Google Scholar
Google Scholar
[26] Alexander K, George J P, Verbist J, Neyts K, Kuyken B, Thourhout D Van, Beeckman J 2018 Nat. Commun. 9 3444
 Google Scholar
Google Scholar
[27] Boyd RW 2008 Nonlinear Optics (Third Edition) (Beijing: Academic Press)
[28] Hourmand M, Sarhan A A D, Sayuti M 2017 Int. J. Adv. Manuf. Tech. 91 1023
 Google Scholar
Google Scholar
[29] Kulkarni G U, Kiruthika S, Gupta R, Rao K D M 2015 Curr. Opin. Chem. Eng. 8 60
 Google Scholar
Google Scholar
[30] Zhu W, She W 2012 Opt. Express 20 25876
 Google Scholar
Google Scholar
[31] Khonina S N, Podlipnov V V, Volotovskiĭ S G 2018 J. Opt. Tech. 85 388
 Google Scholar
Google Scholar
[32] Chu H, Li Y, Zhao S 2011 Appl. Opt. 50 360
 Google Scholar
Google Scholar
[33] Zhang M, BuscainB, Wang C, Shams-Ansari A, Reimer C, Zhu R, Kahn J M, Lončar M 2019 Nature 568 373
 Google Scholar
Google Scholar
[34] Lao G M, Zhang Z H, Zhao D M 2016 Opt. Express 24 18082
 Google Scholar
Google Scholar
[35] Molina-Terriza G, Torres J P, Torner L 2001 Phys. Rev. Lett. 88 013601
 Google Scholar
Google Scholar
计量
- 文章访问数: 5630
- PDF下载量: 63
- 被引次数: 0















 下载:
下载: