-
通过构造两个线性耦合的拉盖尔-高斯旋转腔系统, 实现携带轨道角动量的涡旋光束的非互易传输现象. 系统中, 两个拉盖尔-高斯旋转腔模通过扭力与中间的旋转镜耦合, 同时两个涡旋腔场通过光纤直接耦合起来. 两个强光场分别驱动不同的腔模, 并利用一个弱探测场从系统一侧入射, 从而对该系统两个传播方向的光响应特性进行研究. 利用该系统哈密顿量和海森伯-郎之万方程, 结合输入-输出关系可得到系统的输出光谱. 结果表明此系统中的涡旋光束的非互易性来源于光旋转相互作用以及涡旋腔场相互作用之间的量子干涉效应. 因此, 可以通过调节非互易相位差来对系统的非互易传输进行调制. 此外, 两个涡旋光束所携带的拓扑荷比值会显著影响传输特性; 在适当的拓扑荷比值下, 该系统可以实现涡旋光束的单向传输. 本研究成果有望用于实现理想的涡旋光隔离器.By constructing an optorotational system composed of two linearly coupled Laguerre-Gaussian rotational cavities, we realize the nonreciprocal transmission of the vortex beam with the orbital angular momentum. Two vortex beam cavity modes driven by strong fields are coupled with a rotational mirror via the torsion, and two cavity modes interact with each other via the optical fiber. A weak probe field is incident from one side of the system for examining the optical response along one propagating direction. With the Hamiltonian of the system and the Heisenberg-Langevin equation, we can obtain the transmission of the output light field from the input-output theory. The result shows that the optical nonreciprocity of the vortex beam arises from the quantum interference between the optorotational interaction and the linear coupling interaction between two vortex beam modes, and the phase difference can be used to adjust the optical nonreciprocity. The phase difference can determine not only the occurrence of the nonreciprocity but also the direction of nonreciprocity. Moreover, the ratio of the topological charges carried by the two vortex beam fields has an influence on the transmission. Under an appropriate topological charge ratio, the unidirectional transmission of the vortex beam can be realized in such a system. It is found that whether the topological charge ratio is positive or negative, i.e. whether the vortex beam is left-hand beam or right-hand beam, does not affect the transmission; the influence of the topological charge on the transmission amplitude actually comes from the topological charge number carried by the vortex beam, due to the fact that the coupling strength between the rotating mirror mode and the cavity mode depends on the topological charge number. In addition, we also obtain the condition that the system damping rates should meet for realizing the perfect nonreciprocal propagation of the vortex beam. Finally, we can achieve the nonreciprocal group velocity of the slow light. The direction of the nonreciprocal slow light can be controlled via phase modulation. Our work provides a possible application in manipulating the vortex beam propagation. Furthermore, we extend the nonreciprocity of ordinary beams in the optomechanical system to the nonreciprocity of the vortex beam in the optorotational system. The results are expected to be applied to fabricating the ideal optical isolators for the vortex beam carrying the orbital angular momentum in optical communication.
-
Keywords:
- vortex beam /
- Laguerre-Gaussian rotational cavity /
- nonreciprocal propagation /
- topological charge
[1] Allen L, Beijersbergen M W, Spreeuw R J, Woerdman J P 1992 Phys. Rev. A 45 8185
 Google Scholar
Google Scholar
[2] Yao A M, Padgett M J 2011 Adv. Opt. Photonics 3 161
 Google Scholar
Google Scholar
[3] He H, Friese M E J, Heckenberg N R, Rubinsztein-Dunlop H 1995 Phys. Rev. Lett. 75 826
 Google Scholar
Google Scholar
[4] Andersen M F, Ryu C, Clade P, Natarajan V, Vaziri A, Helmerson K, Phillips W D 2006 Phys. Rev. Lett. 97 170406
 Google Scholar
Google Scholar
[5] Volpe G, Petrov D 2006 Phys. Rev. Lett. 97 210603
 Google Scholar
Google Scholar
[6] Bhattacharya M, Meystre P 2007 Phys. Rev. Lett. 99 153603
 Google Scholar
Google Scholar
[7] Liu Y M, Bai C H, Wang D Y, Wang T, Zheng M H, Wang H F, Zhu A D, Zhang S 2018 Opt. Express 26 6143
 Google Scholar
Google Scholar
[8] Peng J X, Chen Z, Yuan Q Z, Feng X L 2019 Phys. Rev. A 99 043817
 Google Scholar
Google Scholar
[9] Zhang Z, Wang Y P, Wang X 2021 Front. Phys. 16 32503
 Google Scholar
Google Scholar
[10] Kazemi S H, Mahmoudi M 2020 Phys. Scr. 95 045107
 Google Scholar
Google Scholar
[11] Bhattacharya M, Giscard P L, Meystre P 2008 Phys. Rev. A 77 013827
 Google Scholar
Google Scholar
[12] Cheng H J, Zhou S J, Peng J X, Kundu A, Li H X, Jin L, Feng X L 2021 J. Opt. Soc. Am. B 38 285
 Google Scholar
Google Scholar
[13] Xiong H, Huang Y M, Wu Y 2021 Phys. Rev. A 103 043506
 Google Scholar
Google Scholar
[14] Law C K 1995 Phys. Rev. A 51 2537
 Google Scholar
Google Scholar
[15] Xiong H, Si L G, Zheng A S, Yang X, Wu Y 2012 Phys. Rev. A 86 013815
 Google Scholar
Google Scholar
[16] Tian L 2013 Phys. Rev. Lett. 110 233602
 Google Scholar
Google Scholar
[17] Deng Z J, Habraken S J M, Marquardt F 2016 New. J. Phys. 18 063022
 Google Scholar
Google Scholar
[18] Deng Z J, Yan X B, Wang Y D, Wu C W 2016 Phys. Rev. A 93 033842
 Google Scholar
Google Scholar
[19] Yan X B 2017 Phys. Rev. A 96 053831
 Google Scholar
Google Scholar
[20] 张秀龙, 鲍倩倩, 杨明珠, 田雪松 2018 67 104203
 Google Scholar
Google Scholar
Zhang X L, Bao Q Q, Yang M Z, Tian X S 2018 Acta Phys. Sin. 67 104203
 Google Scholar
Google Scholar
[21] Agarwal G S, Huang S M 2010 Phys. Rev. A 81 041803
 Google Scholar
Google Scholar
[22] Shu J 2011 Chin. Phys. Lett. 28 104203
 Google Scholar
Google Scholar
[23] 陈华俊, 米贤武 2011 60 124206
 Google Scholar
Google Scholar
Chen H J, Mi X W 2011 Acta Phys. Sin. 60 124206
 Google Scholar
Google Scholar
[24] He B, Yang L, Lin Q, Xiao M 2017 Phys. Rev. Lett. 118 233604
 Google Scholar
Google Scholar
[25] Li Y, Wu L A, Wang Z D 2011 Phys. Rev. A 83 043804
 Google Scholar
Google Scholar
[26] Xiong H, Fan Y W, Yang X, Wu Y 2016 Appl. Phys. Lett. 109 061108
 Google Scholar
Google Scholar
[27] Chen Y T, Du L, Zhang Y, Wu J H 2021 Phys. Rev. A 103 053712
 Google Scholar
Google Scholar
[28] Ge Y Q, Chen Y T, Yin K, Zhang Y 2020 Phys. Lett. A 384 126836
 Google Scholar
Google Scholar
[29] Chen Y T, Du L, Liu Y M, Zhang Y 2020 Opt. Express 28 7095
 Google Scholar
Google Scholar
[30] Bi L, Hu J, Jiang P, Kim D H, Dionne G F, Kimerling L C, Ross C A 2011 Nat. Photonics 5 758
 Google Scholar
Google Scholar
[31] Aplet L J, Carson J W 1964 Appl. Opt. 3 544
 Google Scholar
Google Scholar
[32] Korneeva Y P, Vodolazov D Y, Semenov A V, Florya I N, Simonov N, Baeva E, Korneev A A, Goltsman G N, Klapwijk T M 2018 Phys. Rev. Appl. 9 064037
 Google Scholar
Google Scholar
[33] Shen Z, Zhang Y L, Chen Y, Zou C L, Xiao Y F, Zou X B, Sun F W, Guo G C, Dong C H 2016 Nat. Photonics 10 657
 Google Scholar
Google Scholar
[34] Maayani S, Dahan R, Kligerman Y, Moses E, Hassan A U, Jing H, Nori F, Christodoulides D N, Carmon T 2018 Nature 558 569
 Google Scholar
Google Scholar
[35] Li B, Huang R, Xu X W, Miranowicz A, Jing H 2019 Photonics Res. 7 630
 Google Scholar
Google Scholar
[36] Li Y, Huang Y Y, Zhang X Z, Tian L 2017 Opt. Express 25 018907
 Google Scholar
Google Scholar
[37] Mirza I M, Ge W C, Jing H 2019 Opt. Express 27 25515
 Google Scholar
Google Scholar
[38] Jiang C, Song L N, Li Y 2018 Phys. Rev. A 97 053812
 Google Scholar
Google Scholar
[39] Jiang C, Baowei J I, Cui Y S, Zuo F, Shi J, Chen G 2018 Opt. Express 26 15255
 Google Scholar
Google Scholar
[40] Jiang C, Song L N, Li Y 2019 Phys. Rev. A 99 023823
 Google Scholar
Google Scholar
[41] Xu X W, Li Y 2015 Phys. Rev. A 91 053854
 Google Scholar
Google Scholar
[42] Xu X W, Li Y, Chen A X, Liu Y X 2016 Phys. Rev. A 93 023827
 Google Scholar
Google Scholar
[43] Xu X W, Song L N, Zheng Q, Wang Z H, Li Y 2018 Phys. Rev. A 98 063845
 Google Scholar
Google Scholar
[44] Yan X B, Lu H L, Gao F, Yang L 2019 Front. Rhys. 14 52601
 Google Scholar
Google Scholar
[45] Xia C C, Yan X B, Tian X D, Gao F 2019 Opt. Commun. 451 197
 Google Scholar
Google Scholar
[46] 张利巍, 李贤丽, 杨柳 2019 68 170701
 Google Scholar
Google Scholar
Zhang L W, Li X L, Yang L 2019 Acta Phys. Sin. 68 170701
 Google Scholar
Google Scholar
[47] Agarwal G S, Huang S 2014 New J. Phys. 16 033023
 Google Scholar
Google Scholar
[48] Yan X B, Cui C L, Gu K H, Tian X D, Fu C B, Wu J H 2014 Opt. Express 22 4886
 Google Scholar
Google Scholar
[49] Safavi-Naeini A H, Mayer Alegre T P, Chan J, Eichenfield M, Winger M, Lin Q, Hill J T, Chang D E, Painter O 2011 Nature 472 69
 Google Scholar
Google Scholar
[50] Weis S, Riviere R, Deleglise S, Gavartin E, Arcizet O, Schliesser A, Kippenberg T J 2010 Science 330 1520
 Google Scholar
Google Scholar
[51] Oemrawsingh S S R, Eliel E R, Woerdman J P, Verstegen E J K, Kloosterboer J G, 't Hooft G W 2004 J. Opt. A: Pure Appl. Opt. 6 S228
[52] Li L, Allen Y Y, Huang C, Grewell D A, Benatar A, Chen Y 2006 Opt. Eng. 45 113401
 Google Scholar
Google Scholar
[53] Shi H, Bhattacharya M 2016 J. Phys. B 49 153001
 Google Scholar
Google Scholar
[54] Shen Y, Campbell G T, Hage B, Zou H, Buchler B C, Lam P K 2013 J. Opt. 15 044005
 Google Scholar
Google Scholar
-
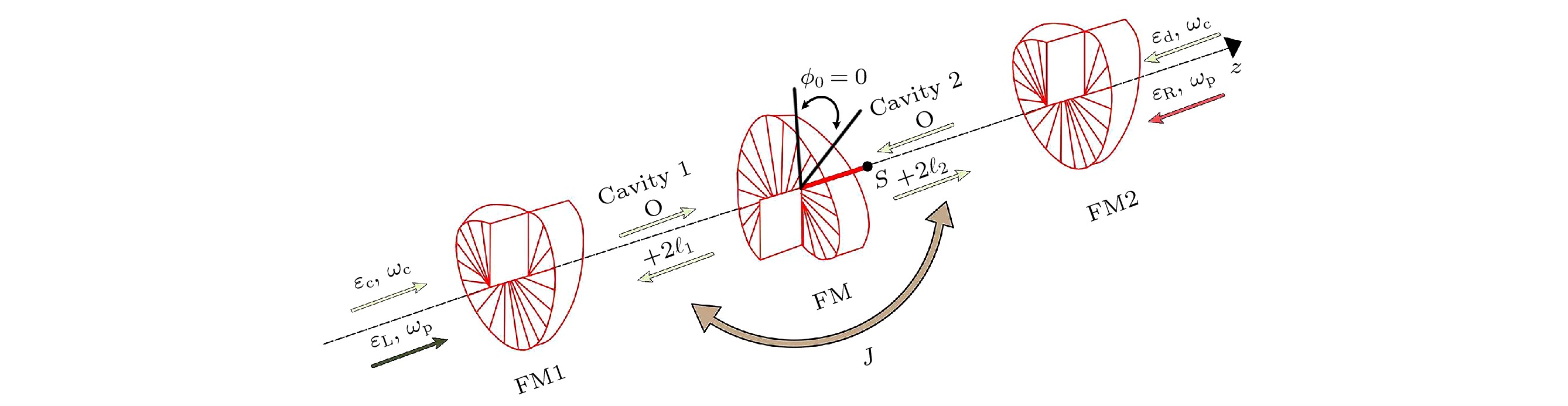
图 1 双拉盖尔-高斯旋转腔示意图, 两L-G腔场通过光旋转相互作用与中间的旋转腔镜耦合, 振幅为
$ {\varepsilon _{\text{c}}} $ 和$ {\varepsilon _{\text{d}}} $ ($ {\varepsilon _{\text{L}}} $ 和$ {\varepsilon _{\text{R}}} $ )的强驱动场(弱探测场)分别从两侧入射系统来驱动L-G腔模$ {c_1} $ 和$ {c_{\text{2}}} $ , 同时用光纤将两L-G腔模线性耦合. 旋转腔镜的平衡位置为$ {\phi _{\text{0}}} $ , 在扭力作用下的角位移用$ \phi $ 角表示Fig. 1. Schematic diagram of double Laguerre-Gaussian (L-G) rotational-cavity. The two L-G cavity modes are coupled with a rotating cavity mirror in the middle via the optical rotation interaction. Two strong pump fields (weak probe fields) with amplitudes
$ {\varepsilon _{\text{c}}} $ and$ {\varepsilon _{\text{d}}} $ ($ {\varepsilon _{\text{L}}} $ and$ {\varepsilon _{\text{R}}} $ ) are incident on the system from both sides to drive the L-G cavity modes$ {c_1} $ and$ {c_{\text{2}}} $ , and the two L-G cavity modes are linearly coupled with an optical fiber. The equilibrium position of the rotational mirror is$ {\phi _{\text{0}}} $ , and the angular displacement is indicated by angle$ \phi $ under the action of the torsion.图 2 传输振幅
$ {T_{12}} $ (红色实线)和$ {T_{21}} $ (蓝色虚线)在不同非互易相位差$ \theta $ 下随标准化失谐$ x/{\kappa _1} $ 的演化 (a)$ \theta = 0 $ ; (b)$ \theta = - {\text{π }}/4 $ ; (c)$ \theta = {\text{π }}/4 $ ; (d)$ \theta = - {\text{π }}/2 $ ; (e)$ \theta = {\text{π }}/2 $ . (f)在标准化失谐为零(x = 0)时, 传输振幅$ {T_{12}} $ (红色实线)和$ {T_{21}} $ (蓝色虚线)随非互易相位差$ \theta $ 的演化. 其他参数为$ {\kappa _1} = 1 $ ,$ {\kappa _2} = 1 $ ,$ \gamma = 1 $ ,$ {G_i} = \sqrt {{\kappa _i}\gamma } /2 $ ,$ J = \sqrt {{\kappa _1}{\kappa _2}} /2 $ .Fig. 2. Transmission amplitudes
$ {T_{12}} $ (red solid line) and$ {T_{21}} $ (blue dotted line) versus normalized detuning$ x/{\kappa _1} $ under different nonreciprocal phase difference: (a)$ \theta = 0 $ ; (b)$ \theta = - {\text{π }}/4 $ ; (c)$ \theta = {\text{π }}/4 $ ; (d)$ \theta = - {\text{π }}/2 $ ; (e)$ \theta = {\text{π }}/2 $ . (f) Transmission amplitudes$ {T_{12}} $ (red solid line) and$ {T_{21}} $ (blue dotted line) versus nonreciprocal phase difference with$ x = 0 $ . Other parameters are$ {\kappa _1} = 1 $ ,$ {\kappa _2} = 1 $ ,$ \gamma = 1 $ ,$ {G_i} = \sqrt {{\kappa _i}\gamma } /2 $ ,$ J = \sqrt {{\kappa _1}{\kappa _2}} /2 $ .图 3 (a)传输振幅
$ {T_{12}} $ 和(b)传输振幅$ {T_{{\text{21}}}} $ 作为标准化失谐$ x/{\kappa _1} $ 和旋转镜与腔$ {c_1} $ 耗散比$ \gamma /{\kappa _1} $ 的函数; (c)传输振幅$ {T_{12}} $ 和(d)传输振幅$ {T_{{\text{21}}}} $ 作为标准化失谐$ x/{\kappa _1} $ 和腔$ {c_2} $ 与腔$ {c_1} $ 耗散比$ {\kappa _2}/{\kappa _1} $ 的函数. 其他参数为$ \theta = {\text{π }}/2 $ ,$ {\kappa _1} = 1 $ ,$ {G_1} = {G_2} = J = 1/2 $ Fig. 3. (a) Transmission amplitude
$ {T_{12}} $ and (b) transmission amplitude$ {T_{21}} $ as a function of the normalized detuning$ x/{\kappa _1} $ and the dissipation ratio$ \gamma /{\kappa _1} $ of rotating mirror to cavity$ {c_1} $ ; (c) transmission amplitudes$ {T_{12}} $ and (d) transmission amplitudes$ {T_{21}} $ as a function of the normalized detuning$ x/{\kappa _1} $ and the dissipation ratio$ {\kappa _2}/{\kappa _1} $ of cavity$ {c_2} $ to cavity$ {c_1} $ . Other parameters are$ \theta = {\text{π }}/2 $ ,$ {\kappa _1} = 1 $ ,$ {G_1} = {G_2} = J = 1/2 $ .图 4 传输振幅
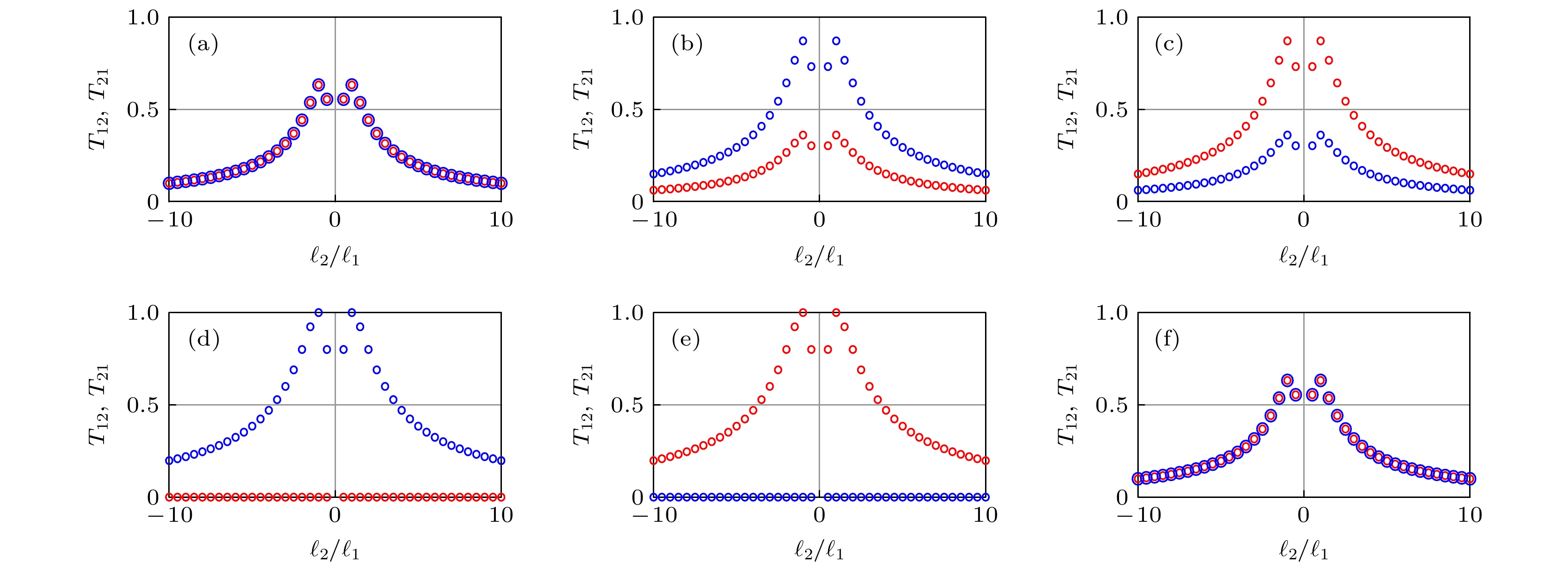
$ {T_{12}} $ (红色圆圈)和$ {T_{21}} $ (蓝色圆圈)在不同非互易相位差$ \theta $ 下随两个涡旋光场所携带拓扑荷之比$ {\ell _2}/{\ell _1} $ 的演化 (a)$\theta = 0$ ; (b)$ \theta = - {\text{π }}/4 $ ; (c)$ \theta = {\text{π }}/4 $ ; (d)$ \theta = - {\text{π }}/2 $ ; (e)$ \theta = {\text{π }}/2 $ ; (f)$ \theta = {\text{π }} $ . 其他参数为$ {\kappa _1} = 1 $ ,$ {\kappa _2} = 1 $ ,$ \gamma = 1 $ ,$ J = 2{G_1}{G_2}/\gamma $ Fig. 4. Transmission amplitudes
$ {T_{12}} $ (red circle) and$ {T_{21}} $ (blue circle) with ratio of topological charges carried by two vortex optical fields$ {\ell _2}/{\ell _1} $ under different nonreciprocal phase difference: (a)$ \theta = 0 $ ; (b)$ \theta = - {\text{π }}/4 $ ; (c)$ \theta = {\text{π }}/4 $ ; (d)$ \theta = - {\text{π }}/2 $ ; (e)$ \theta = {\text{π }}/2 $ ; (f)$ \theta = {\text{π }} $ . Other parameters are$ {\kappa _1} = 1 $ ,$ {\kappa _2} = 1 $ ,$ \gamma = 1 $ ,$ J = 2{G_1}{G_2}/\gamma $ .图 5 群延迟
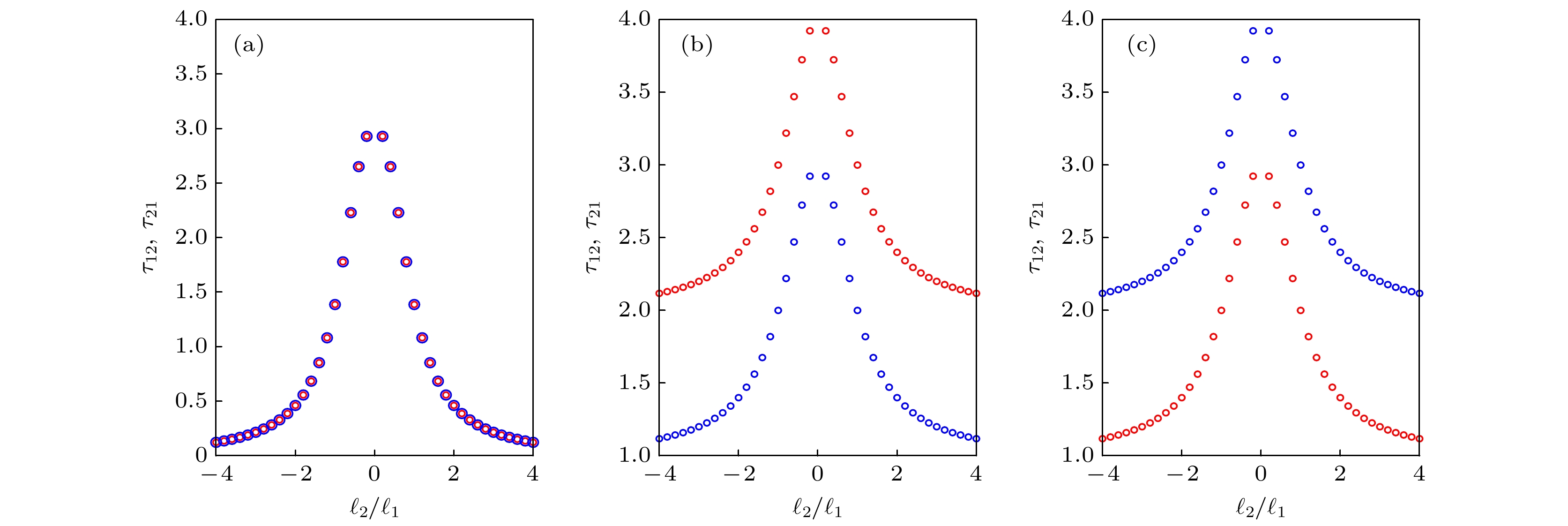
$ {\tau _{12}} $ (红色圆圈)和$ {\tau _{21}} $ (蓝色圆圈)在不同非互易相位差$ \theta $ 下随两个涡旋光场所携带拓扑荷比$ {\ell _2}/{\ell _1} $ 的演化 (a)$\theta = 0$ ; (b)$ \theta = - {\text{π }}/2 $ ; (c)$ \theta = {\text{π }}/2 $ . 其他参数为$ {\kappa _1} = 1 $ ,$ {\kappa _2} = 1 $ ,$ \gamma = 1 $ ,$ J = 2{G_1}{G_2}/\gamma $ Fig. 5. Group delay
$ {\tau _{12}} $ (red circle) and$ {\tau _{21}} $ (blue circle) with ratio of topological charges carried by two vortex optical fields$ {\ell _2}/{\ell _1} $ under different nonreciprocal phase difference: (a)$ \theta = 0 $ ; (b)$ \theta = - {\text{π }}/2 $ ; (c)$ \theta = {\text{π }}/2 $ . Other parameters are$ {\kappa _1} = 1 $ ,$ {\kappa _2} = 1 $ ,$ \gamma = 1 $ ,$ J = 2{G_1}{G_2}/\gamma $ . -
[1] Allen L, Beijersbergen M W, Spreeuw R J, Woerdman J P 1992 Phys. Rev. A 45 8185
 Google Scholar
Google Scholar
[2] Yao A M, Padgett M J 2011 Adv. Opt. Photonics 3 161
 Google Scholar
Google Scholar
[3] He H, Friese M E J, Heckenberg N R, Rubinsztein-Dunlop H 1995 Phys. Rev. Lett. 75 826
 Google Scholar
Google Scholar
[4] Andersen M F, Ryu C, Clade P, Natarajan V, Vaziri A, Helmerson K, Phillips W D 2006 Phys. Rev. Lett. 97 170406
 Google Scholar
Google Scholar
[5] Volpe G, Petrov D 2006 Phys. Rev. Lett. 97 210603
 Google Scholar
Google Scholar
[6] Bhattacharya M, Meystre P 2007 Phys. Rev. Lett. 99 153603
 Google Scholar
Google Scholar
[7] Liu Y M, Bai C H, Wang D Y, Wang T, Zheng M H, Wang H F, Zhu A D, Zhang S 2018 Opt. Express 26 6143
 Google Scholar
Google Scholar
[8] Peng J X, Chen Z, Yuan Q Z, Feng X L 2019 Phys. Rev. A 99 043817
 Google Scholar
Google Scholar
[9] Zhang Z, Wang Y P, Wang X 2021 Front. Phys. 16 32503
 Google Scholar
Google Scholar
[10] Kazemi S H, Mahmoudi M 2020 Phys. Scr. 95 045107
 Google Scholar
Google Scholar
[11] Bhattacharya M, Giscard P L, Meystre P 2008 Phys. Rev. A 77 013827
 Google Scholar
Google Scholar
[12] Cheng H J, Zhou S J, Peng J X, Kundu A, Li H X, Jin L, Feng X L 2021 J. Opt. Soc. Am. B 38 285
 Google Scholar
Google Scholar
[13] Xiong H, Huang Y M, Wu Y 2021 Phys. Rev. A 103 043506
 Google Scholar
Google Scholar
[14] Law C K 1995 Phys. Rev. A 51 2537
 Google Scholar
Google Scholar
[15] Xiong H, Si L G, Zheng A S, Yang X, Wu Y 2012 Phys. Rev. A 86 013815
 Google Scholar
Google Scholar
[16] Tian L 2013 Phys. Rev. Lett. 110 233602
 Google Scholar
Google Scholar
[17] Deng Z J, Habraken S J M, Marquardt F 2016 New. J. Phys. 18 063022
 Google Scholar
Google Scholar
[18] Deng Z J, Yan X B, Wang Y D, Wu C W 2016 Phys. Rev. A 93 033842
 Google Scholar
Google Scholar
[19] Yan X B 2017 Phys. Rev. A 96 053831
 Google Scholar
Google Scholar
[20] 张秀龙, 鲍倩倩, 杨明珠, 田雪松 2018 67 104203
 Google Scholar
Google Scholar
Zhang X L, Bao Q Q, Yang M Z, Tian X S 2018 Acta Phys. Sin. 67 104203
 Google Scholar
Google Scholar
[21] Agarwal G S, Huang S M 2010 Phys. Rev. A 81 041803
 Google Scholar
Google Scholar
[22] Shu J 2011 Chin. Phys. Lett. 28 104203
 Google Scholar
Google Scholar
[23] 陈华俊, 米贤武 2011 60 124206
 Google Scholar
Google Scholar
Chen H J, Mi X W 2011 Acta Phys. Sin. 60 124206
 Google Scholar
Google Scholar
[24] He B, Yang L, Lin Q, Xiao M 2017 Phys. Rev. Lett. 118 233604
 Google Scholar
Google Scholar
[25] Li Y, Wu L A, Wang Z D 2011 Phys. Rev. A 83 043804
 Google Scholar
Google Scholar
[26] Xiong H, Fan Y W, Yang X, Wu Y 2016 Appl. Phys. Lett. 109 061108
 Google Scholar
Google Scholar
[27] Chen Y T, Du L, Zhang Y, Wu J H 2021 Phys. Rev. A 103 053712
 Google Scholar
Google Scholar
[28] Ge Y Q, Chen Y T, Yin K, Zhang Y 2020 Phys. Lett. A 384 126836
 Google Scholar
Google Scholar
[29] Chen Y T, Du L, Liu Y M, Zhang Y 2020 Opt. Express 28 7095
 Google Scholar
Google Scholar
[30] Bi L, Hu J, Jiang P, Kim D H, Dionne G F, Kimerling L C, Ross C A 2011 Nat. Photonics 5 758
 Google Scholar
Google Scholar
[31] Aplet L J, Carson J W 1964 Appl. Opt. 3 544
 Google Scholar
Google Scholar
[32] Korneeva Y P, Vodolazov D Y, Semenov A V, Florya I N, Simonov N, Baeva E, Korneev A A, Goltsman G N, Klapwijk T M 2018 Phys. Rev. Appl. 9 064037
 Google Scholar
Google Scholar
[33] Shen Z, Zhang Y L, Chen Y, Zou C L, Xiao Y F, Zou X B, Sun F W, Guo G C, Dong C H 2016 Nat. Photonics 10 657
 Google Scholar
Google Scholar
[34] Maayani S, Dahan R, Kligerman Y, Moses E, Hassan A U, Jing H, Nori F, Christodoulides D N, Carmon T 2018 Nature 558 569
 Google Scholar
Google Scholar
[35] Li B, Huang R, Xu X W, Miranowicz A, Jing H 2019 Photonics Res. 7 630
 Google Scholar
Google Scholar
[36] Li Y, Huang Y Y, Zhang X Z, Tian L 2017 Opt. Express 25 018907
 Google Scholar
Google Scholar
[37] Mirza I M, Ge W C, Jing H 2019 Opt. Express 27 25515
 Google Scholar
Google Scholar
[38] Jiang C, Song L N, Li Y 2018 Phys. Rev. A 97 053812
 Google Scholar
Google Scholar
[39] Jiang C, Baowei J I, Cui Y S, Zuo F, Shi J, Chen G 2018 Opt. Express 26 15255
 Google Scholar
Google Scholar
[40] Jiang C, Song L N, Li Y 2019 Phys. Rev. A 99 023823
 Google Scholar
Google Scholar
[41] Xu X W, Li Y 2015 Phys. Rev. A 91 053854
 Google Scholar
Google Scholar
[42] Xu X W, Li Y, Chen A X, Liu Y X 2016 Phys. Rev. A 93 023827
 Google Scholar
Google Scholar
[43] Xu X W, Song L N, Zheng Q, Wang Z H, Li Y 2018 Phys. Rev. A 98 063845
 Google Scholar
Google Scholar
[44] Yan X B, Lu H L, Gao F, Yang L 2019 Front. Rhys. 14 52601
 Google Scholar
Google Scholar
[45] Xia C C, Yan X B, Tian X D, Gao F 2019 Opt. Commun. 451 197
 Google Scholar
Google Scholar
[46] 张利巍, 李贤丽, 杨柳 2019 68 170701
 Google Scholar
Google Scholar
Zhang L W, Li X L, Yang L 2019 Acta Phys. Sin. 68 170701
 Google Scholar
Google Scholar
[47] Agarwal G S, Huang S 2014 New J. Phys. 16 033023
 Google Scholar
Google Scholar
[48] Yan X B, Cui C L, Gu K H, Tian X D, Fu C B, Wu J H 2014 Opt. Express 22 4886
 Google Scholar
Google Scholar
[49] Safavi-Naeini A H, Mayer Alegre T P, Chan J, Eichenfield M, Winger M, Lin Q, Hill J T, Chang D E, Painter O 2011 Nature 472 69
 Google Scholar
Google Scholar
[50] Weis S, Riviere R, Deleglise S, Gavartin E, Arcizet O, Schliesser A, Kippenberg T J 2010 Science 330 1520
 Google Scholar
Google Scholar
[51] Oemrawsingh S S R, Eliel E R, Woerdman J P, Verstegen E J K, Kloosterboer J G, 't Hooft G W 2004 J. Opt. A: Pure Appl. Opt. 6 S228
[52] Li L, Allen Y Y, Huang C, Grewell D A, Benatar A, Chen Y 2006 Opt. Eng. 45 113401
 Google Scholar
Google Scholar
[53] Shi H, Bhattacharya M 2016 J. Phys. B 49 153001
 Google Scholar
Google Scholar
[54] Shen Y, Campbell G T, Hage B, Zou H, Buchler B C, Lam P K 2013 J. Opt. 15 044005
 Google Scholar
Google Scholar
计量
- 文章访问数: 5849
- PDF下载量: 189
- 被引次数: 0





























 下载:
下载: