-
针对实际多导体传输线结构中不同线缆导体之间处于非平行分布状态的几何特征, 讨论了基于传输线模型和分布参数模型构建的线缆电磁耦合模型, 通过对每个线缆网格分别进行分布参数提取和代入到传输线模型中计算, 实现将线缆电磁耦合模型应用到非平行线缆结构电磁耦合计算. 以实际线缆单元结构为对象, 将所提模型的计算结果分别与全波算法计算结果和实验结果对比, 完成了非平行线缆电磁耦合模型的准确性验证. 在完成准确性验证的基础上, 对比分析了平行线缆和非平行线缆两种结构的电磁耦合响应结果, 展示了线缆非平行结构特征对实际线缆电磁耦合响应的显著影响, 证实了在实际线缆电磁耦合仿真中必须考虑非平行线缆结构特征的影响 .
Owing to the random bundling of the cable bundle, the different conductors within the cable present non-parallel distribution structural features. Such a structural feature leads to different cross-sectional structures at different positions along the line. The variations of spacing between different conductors and the height from the ground can lead the distribution parameters to change. This requires that when solving the electromagnetic coupling of the cable bundle, the cable bundle should be divided into multiple grid segments along the line, and the distribution parameters should be calculated according to the cross-sectional geometry information at different grid segment positions. On the basis of the integration of the transmission line model and the distributed parameter model, the distribution parameters of different mesh segments are calculated according to the cross-sectional structure of different mesh segments of the cable in this paper. When the parameters are brought into the transmission line model, an electromagnetic coupling model suitable for non-parallel cable structures is established. In order to verify the accuracy of the model, a unit cable structure from real cable with non-parallel distribution characteristics is designed. The calculation results from the proposed model are compared with the calculation results from the full-wave algorithm and the experimental results, showing they are in good agreement with each other. In order to understand the influence of the cable non-parallel structure characteristics on the response of electromagnetic coupling to cable, the comparison of electromagnetic coupling response results between the parallel cable and non-parallel cable structures are made and analyzed, showing that the non-parallel structure characteristics of the cable have a significant effect on the electromagnetic coupling. It is confirmed that the influence of the non-parallel cable structure characteristics must be considered in the electromagnetic coupling simulation of the real cable. -
Keywords:
- cable bundle /
- transmission line model /
- distribution parameter /
- method of moment
[1] Taylor C D, Satterwhite R, Harrision C W 1965 IEEE Trans. Antennas Propagat. 13 987
Google Scholar
[2] Agrawal A K, Price H J, Gurbaxani S H 1980 IEEE Trans. Electromagn. Compat. 22 119
Google Scholar
[3] Rachidi F 1993 IEEE Trans. Electromagn. Compat. 35 404
Google Scholar
[4] 叶志红, 张杰, 周健健, 苟胆 2020 69 060701
Google Scholar
Ye Z H, Zhang J, Zhou J J, Gou D 2020 Acta Phys. Sin. 69 060701
Google Scholar
[5] 肖培, 李佳维, 贺佳港, 李锦新, 刘柱, 李高升 2021 70 100702
Google Scholar
Xiao P, Li J W, He J G, Li J X, Liu Z, Li G S 2021 Acta Phys. Sin. 70 100702
Google Scholar
[6] 吴振军, 王丽芳, 廖承林 2009 59 6146
Google Scholar
Wu Z J, Wang L F, Liao C L 2009 Acta Phys. Sin. 59 6146
Google Scholar
[7] 孙亚秀, 卓庆坤, 姜庆辉, 李千 2015 64 044102
Google Scholar
Sun Y X, Zhuo Q K, Jiang Q H, Li Q 2015 Acta Phys. Sin. 64 044102
Google Scholar
[8] Spadacini G, Grassi F, Pignari S A 2013 China Commun. 10 119
Google Scholar
[9] Spadacini G, Grassi F, Pignari S A 2015 IEEE Trans. Electromagn. Compat. 57 1246
Google Scholar
[10] Wu M L, Beetner D G, Hubing T H, Ke H X, Sun S H 2009 IEEE Trans. Electromagn. Compat. 51 842
Google Scholar
[11] Li X, Wu M L, Beetner D, Hubing T 2010 IEEE International Symposium on Electromagnetic Compatibility Florida, USA, July 25–30, 2010 p614
[12] Clements J C, Paul C R, Adams A T 1975 IEEE Trans. Electromagn. Compat. 10 238
Google Scholar
[13] Giri D V, Tesche F M, Chang S K 1979 IEEE Trans. Electromagn. Compat. 21 220
Google Scholar
[14] Savage J S, Smith W T 1995 IEEE Trans. Electromagn. Compat. 37 131
Google Scholar
[15] Li G H, Hess G, Hoeckele R, Davidson S, Jalbert P, Victor V 2015 IEEE Trans. Electromagn. Compat. 57 827
Google Scholar
[16] Sun S S, Liu G P, Drewniak J L, Pommerenke D J 2007 IEEE Trans. Electromagn. Compat. 49 708
Google Scholar
[17] Pignari S A, Spadacini G, Grassi F 2017 IEEE Electromagn. Compat. Magazine 6 85
Google Scholar
[18] 魏兵, 李小勇, 王飞, 葛德彪 2009 58 6174
Google Scholar
Wei B, Li X Y, Wang F, Ge D B 2009 Acta Phys. Sin. 58 6174
Google Scholar
[19] 魏兵, 葛德彪, 王飞 2008 57 6290
Google Scholar
Wei B, Ge D B, Wang F 2008 Acta Phys. Sin. 57 6290
Google Scholar
[20] 葛德彪, 闫玉波 2005 电磁波时域有限差分方法 (第二版) (西安: 西安电子科技大学出版社) 第11—13页
Ge D B, Yan Y B 2005 Finite Difference Time Domain Method for Electromagnetic Waves (2nd Ed) (Xi’an: Xidian University Press) pp11–13 (in Chinese)
[21] 邓莉亭, 钟龙权, 刘强, 孙子涵, 闫丽萍, 赵翔 2021 强激光与粒子束 33 083002
Google Scholar
Deng L T, Zhong L Q, Liu Q, Sun Z H, Yan L P, Zhao X 2021 High Power Laser and Particle Beams 33 083002
Google Scholar
-
图 1 线缆等效传输线电路模型示意图 (a) 平行线缆; (b) 非平行线缆
Fig. 1. Equivalent transmission line model of the cable: (a) Parall cable; (b) non-parallel cable.
图 2 理想导电板上非平行3导线串扰 (a) 计算模型; (b) 实验测试系统图
Fig. 2. Crosstalk of non-parallel three-conductor above perfect conducting ground: (a) Calculation model; (b) measurement setup.
图 3 理想导电板上3导线计算模型串扰结果对比 (a) 1线近端串扰; (b) 1线远端串扰; (c) 3线近端串扰; (d) 3线远端串扰
Fig. 3. Comparison of three-conductor crosstalk results: (a) NEXT of conductor 1; (b) FEXT of conductor 1; (c) NEXT of conductor 3; (d) FEXT of conductor 3.
图 4 理想导电板上非平行6导线串扰 (a) 计算模型; (b) 实验测试系统
Fig. 4. Crosstalk of non-parallel six-conductor above perfect conducting ground: (a) Calculation model; (b) measurement setup.
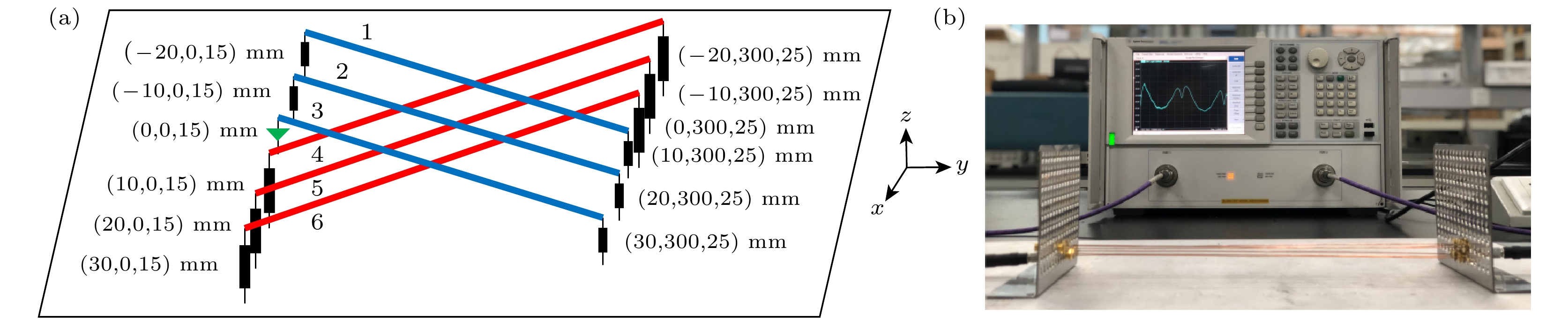
图 5 理想导电板上6导线计算模型串扰结果对比 (a) 1线近端串扰; (b) 1线远端串扰; (c) 2线近端串扰; (d) 2线远端串扰; (e) 4线近端串扰; (f) 4线远端串扰; (g) 5线近端串扰; (h) 5线远端串扰; (i) 6线近端串扰; (j) 6线远端串扰
Fig. 5. Comparison of six-conductor crosstalk results: (a) NEXT of conductor 1; (b) FEXT of conductor 1; (c) NEXT of conductor 2; (d) FEXT of conductor 2; (e) NEXT of conductor 4; (f) FEXT of conductor 4; (g) NEXT of conductor 5; (h) FEXT of conductor 5; (i) NEXT of conductor 6; (j) FEXT of conductor 6.
图 6 理想导电板上3导线传输线串扰模型 (a) 平行分布; (b) 非平行分布
Fig. 6. Crosstalk model of three-conductor transmission line above perfect conducting ground: (a) Parallel; (b) non-parallel.
图 7 理想导电板上3导线计算模型串扰结果对比 (a) 1线负载端口; (b) 2线近端串扰; (c) 2线远端串扰; (d) 3线近端串扰; (e) 3线远端串扰
Fig. 7. Comparison of three-conductor crosstalk results: (a) Response of conductor 1; (b) NEXT of conductor 2; (c) FEXT of conductor 2; (d) NEXT of conductor 3; (e) FEXT of conductor 3.
表 1 不同线缆电磁耦合计算方法的计算时间对比
Table 1. Comparison of the cable coupling computation time with different methods.
算例 线缆电磁耦合模型/s FDTD算法/s 算例1: 非平行3导线 12 30 算例2: 非平行6导线 13 35 
下载: 导出CSV
-
[1] Taylor C D, Satterwhite R, Harrision C W 1965 IEEE Trans. Antennas Propagat. 13 987
Google Scholar
[2] Agrawal A K, Price H J, Gurbaxani S H 1980 IEEE Trans. Electromagn. Compat. 22 119
Google Scholar
[3] Rachidi F 1993 IEEE Trans. Electromagn. Compat. 35 404
Google Scholar
[4] 叶志红, 张杰, 周健健, 苟胆 2020 69 060701
Google Scholar
Ye Z H, Zhang J, Zhou J J, Gou D 2020 Acta Phys. Sin. 69 060701
Google Scholar
[5] 肖培, 李佳维, 贺佳港, 李锦新, 刘柱, 李高升 2021 70 100702
Google Scholar
Xiao P, Li J W, He J G, Li J X, Liu Z, Li G S 2021 Acta Phys. Sin. 70 100702
Google Scholar
[6] 吴振军, 王丽芳, 廖承林 2009 59 6146
Google Scholar
Wu Z J, Wang L F, Liao C L 2009 Acta Phys. Sin. 59 6146
Google Scholar
[7] 孙亚秀, 卓庆坤, 姜庆辉, 李千 2015 64 044102
Google Scholar
Sun Y X, Zhuo Q K, Jiang Q H, Li Q 2015 Acta Phys. Sin. 64 044102
Google Scholar
[8] Spadacini G, Grassi F, Pignari S A 2013 China Commun. 10 119
Google Scholar
[9] Spadacini G, Grassi F, Pignari S A 2015 IEEE Trans. Electromagn. Compat. 57 1246
Google Scholar
[10] Wu M L, Beetner D G, Hubing T H, Ke H X, Sun S H 2009 IEEE Trans. Electromagn. Compat. 51 842
Google Scholar
[11] Li X, Wu M L, Beetner D, Hubing T 2010 IEEE International Symposium on Electromagnetic Compatibility Florida, USA, July 25–30, 2010 p614
[12] Clements J C, Paul C R, Adams A T 1975 IEEE Trans. Electromagn. Compat. 10 238
Google Scholar
[13] Giri D V, Tesche F M, Chang S K 1979 IEEE Trans. Electromagn. Compat. 21 220
Google Scholar
[14] Savage J S, Smith W T 1995 IEEE Trans. Electromagn. Compat. 37 131
Google Scholar
[15] Li G H, Hess G, Hoeckele R, Davidson S, Jalbert P, Victor V 2015 IEEE Trans. Electromagn. Compat. 57 827
Google Scholar
[16] Sun S S, Liu G P, Drewniak J L, Pommerenke D J 2007 IEEE Trans. Electromagn. Compat. 49 708
Google Scholar
[17] Pignari S A, Spadacini G, Grassi F 2017 IEEE Electromagn. Compat. Magazine 6 85
Google Scholar
[18] 魏兵, 李小勇, 王飞, 葛德彪 2009 58 6174
Google Scholar
Wei B, Li X Y, Wang F, Ge D B 2009 Acta Phys. Sin. 58 6174
Google Scholar
[19] 魏兵, 葛德彪, 王飞 2008 57 6290
Google Scholar
Wei B, Ge D B, Wang F 2008 Acta Phys. Sin. 57 6290
Google Scholar
[20] 葛德彪, 闫玉波 2005 电磁波时域有限差分方法 (第二版) (西安: 西安电子科技大学出版社) 第11—13页
Ge D B, Yan Y B 2005 Finite Difference Time Domain Method for Electromagnetic Waves (2nd Ed) (Xi’an: Xidian University Press) pp11–13 (in Chinese)
[21] 邓莉亭, 钟龙权, 刘强, 孙子涵, 闫丽萍, 赵翔 2021 强激光与粒子束 33 083002
Google Scholar
Deng L T, Zhong L Q, Liu Q, Sun Z H, Yan L P, Zhao X 2021 High Power Laser and Particle Beams 33 083002
Google Scholar
下载:
计量
- 文章访问数: 3482
- PDF下载量: 62
- 被引次数: 0
