-
纳米线电极在充/放电过程中引起电极的屈曲失稳行为可能会对结构造成力学损伤. 本文针对纳米线电极结构, 建立了包含锂扩散、应力、浓度影响弹性模量的多场耦合理论模型. 基于构建的模型, 研究了表面效应对纳米线电极屈曲失稳的影响. 结果表明表面效应能够提高纳米线电极的抗屈曲性, 延迟纳米线电极的临界屈曲时间. 同时, 表面效应的影响表现出半径尺寸和长细比的依赖性, 即随着电极半径尺寸的增大而减小, 而随着电极长细比的增大而增大. 此外, 模型还显示, 在有表面效应的条件下, 相对于弹性硬化属性的纳米线电极, 具有弹性软化属性的电极因为具有更好的抗失稳性而更适宜作为电极材料. 研究结果为纳米线电极的力学可靠性设计提供了一定的帮助.Nanowire-based electrodes have attracted much attention due to their high surface energy, short distance for lithium insertion, and the ability to accommodate the enormous strain. However, the buckling behavior may occur during lithiation for such wire-like electrodes, which would lead the battery performance to deteriorate. Therefore, it is vital to quantitatively understand the mechanism about the bucking behavior of the nanowire-based electrodes. Although the buckling behavior of wire-like electrode has been extensively studied in the past few decades, the influence of surface effect on it has not yet been thoroughly explored. For this purpose, a theoretical model of surface effects on buckling of nanowire electrode is presented by taking into account the lithium diffusion, stress, and concentration-dependent elastic properties. Based on the established model, the effects of the residual surface tension and elastic hardening/softening coefficients on buckling are investigated. The results show that surface effects can improve the mechanical reliability, thus delaying the critical buckling time of nanowire electrode. In addition, it is indicated that the surface effects depend on the radius size and slenderness ratio of the nanowire electrode, specifically, the smaller the radius size and the larger the slenderness ratio, the greater the influence of the surface effect is. Furthermore, compared with elastic hardening, with the participation of surface effects, the larger the elastic softening coefficient, the longer it takes for the nanowire electrode to reach the buckled state, and the better the stability of the electrode is. The novelty of this work is that the proposed models highlight the importance of surface effects on buckling of nanowire electrode. These findings provide a prospective insight into the designing of higher structural reliability of electrode.
-
Keywords:
- surface effects /
- lithium-ion batteries /
- nanowire /
- buckling
[1] Larcher D, Tarascon J 2015 Nat. Chem. 7 19
Google Scholar
[2] Scrosati B, Garche J 2010 J. Power Sources 195 2419
Google Scholar
[3] Zhang S, Zhao K, Zhu T, Li J 2017 Prog. Mater. Sci. 89 479
Google Scholar
[4] Gür T 2018 Energy Environ. Sci. 11 2696
Google Scholar
[5] Edge J, O’Kane S, Prosser R, Kirkaldy N, Patel A, Hales A, Ghosh A, Ai W, Chen J, Yang J, Li S, Pang M, Bravo D, Tomaszewska A, Marzook M, Radhakrishnan K, Wang H, Patel Y, Wu B, Offer G 2021 Phys. Chem. Chem. Phys. 23 8200
Google Scholar
[6] Zhang W 2011 J. Power Sources 196 13
Google Scholar
[7] Chan C, Peng H, Liu G, McIlwrath K, Zhang X, Huggins R, Cui Y 2008 Nat. Nanotechnol. 3 31
Google Scholar
[8] Yu C, Li X, Ma T, Rong J, Zhang R, Shaffer J, An Y, Liu Q, Wei B, Jiang H 2012 Adv. Energy Mater. 2 68
Google Scholar
[9] Yao Y, McDowell M, Ryu I, Wu H, Liu N, Hu L, Nix W, Cui Y 2011 Nano Lett. 11 2949
Google Scholar
[10] Kim H, Han B, Choo J, Cho J 2008 Angew. Chem. 120 10305
Google Scholar
[11] Dasgupta N P, Sun J, Liu C, Brittman S, Andrews S C, Lim J, Gao H, Yan R, Yang P 2014 Adv. Mater. 26 2137
Google Scholar
[12] Chen L, Lu N, Xu C, Yu H, Wang T 2009 Electrochim. Acta 54 4198
Google Scholar
[13] Chan C, Zhang X, Cui Y 2008 Nano Lett. 8 307
Google Scholar
[14] Liu, X, Fan F, Yang H, Zhang S, Huang J, Zhu T 2013 ACS Nano 7 1495
Google Scholar
[15] Huang J, Li Z, Chong M, John P, Wu X, Li Q, Scott X, Nicholas S, Xiao H, Arunkumar S 2010 Science 330 1515
Google Scholar
[16] Wang X, Fan F, Wang J, Wang H, Tao S, Yang A, Liu Y, Beng C, Mao S, Zhu T, Xia S 2015 Nat. Commun. 6 8417
Google Scholar
[17] Chakraborty J, Please C, Goriely A, Chapman S 2015 Int. J. Solids Struct. 54 66
Google Scholar
[18] Zhang K, Li Y, Wu J, Zheng B, Yang F 2018 Int. J. Solids Struct. 144 289
Google Scholar
[19] Shen X, Wan Y 2021 Meccanica 1 1
Google Scholar
[20] Li Y, Zhang K, Zheng B, Yang F 2016 J. Phys. D: Appl. Phys. 49 285602
Google Scholar
[21] Zhang K, Chen J, Li Y, Liu D, Zheng B, Kai Y 2020 Results Phys. 16 103018
Google Scholar
[22] Li Y, Mao W, Zhang K, Jia Y, Yang F 2019 J. Phys. D: Appl. Phys. 52 435502
Google Scholar
[23] Zhang Y, Zhan S, Zhang K, Zheng B, Lyu L 2021 Eur. J. Mech. A/Solids 85 104111
Google Scholar
[24] Xing H, Liu Y, Wang B 2019 Acta Mech. 230 4145
Google Scholar
[25] Yeerella R, Boddeda H, Sengupta A, Chakraborty J 2020 J. Appl. Phys. 128 234901
Google Scholar
[26] Herring C 1951 Phys. Rev. 82 87
Google Scholar
[27] Gurtin M, Murdoch A 1975 Arch. Ration. Mech. Anal. 57 291
Google Scholar
[28] Miller R, Shenoy V 2000 Nanotechnology 11 139
Google Scholar
[29] Cammarata R 1994 Prog. Surf. Sci. 46 1
Google Scholar
[30] Cheng Y, Verbrugge M 2008 J. Appl. Phys. 104 083521
Google Scholar
[31] Deshpande R, Cheng Y, Verbrugge M 2010 J. Power Sources 195 5081
Google Scholar
[32] Hao F, Gao X, Fang D 2012 J. Appl. Phys. 112 103507
Google Scholar
[33] Sengupta A, Chakraborty J 2019 Acta Mech. 231 999
Google Scholar
[34] Gao Y, Zhou M 2011 J. Appl. Phys. 109 014310
Google Scholar
[35] Gao X, Fang D, Qu J 2015 Proc. R. Soc. London, Ser. A 471 20150366
Google Scholar
[36] Jia N, Peng Z, Wang S, Li J, Yao Y, Chen S 2020 Sci. China Ser. E: Technol. Sci. 63 2413
Google Scholar
[37] Zhang X, Chen H, Fang D 2020 Int. J. Mech. Sci. 169 105323
Google Scholar
[38] Stein P, Zhao Y, Xu B 2016 J. Power Sources 332 154
Google Scholar
[39] Bucci G, Swamy T, Bishop S, Sheldon B, Chiang Y, Carter W 2017 J. Electrochem. Soc. 164 A645
Google Scholar
[40] Lu Y, Zhang P, Wang F, Zhang K, Zhao X 2018 Electrochim. Acta 274 359
Google Scholar
[41] Young S, Ji L, Chang S, Fang T, Hsueh T, Meen T, Chen I 2007 Nanotechnology 18 225603
Google Scholar
[42] Riaz M, Nur O, Willander M, Klason P 2008 Appl. Phys. Lett. 92 103118
Google Scholar
[43] Wang G, Feng X 2009 Appl. Phys. Lett. 94 141913
Google Scholar
[44] Shenoy V, Johari P, Qi Y 2010 J. Power Sources 195 6825
Google Scholar
[45] Qi Y, Guo H, Hector J, L G, Timmons A 2010 J. Electrochem. Soc. 157 A558
Google Scholar
[46] Qi Y, Hector L, James C, Kim K 2014 J. Electrochem. Soc. 161 F3010
Google Scholar
[47] Swaminathan N, Balakrishnan S, George K 2015 J. Electrochem. Soc. 163 A488
Google Scholar
[48] Sharma P, Ganti S, Bhate N 2003 Appl. Phys. Lett. 82 535
Google Scholar
[49] Zhang X, Shyy W, Marie S 2007 J. Electrochem. Soc. 154 A910
Google Scholar
[50] Tian L, Rajapakse R 2007 Int. J. Solids Struct. 44 7988
Google Scholar
[51] He L, Li Z 2006 Int. J. Solids Struct. 43 6208
Google Scholar
[52] Wang S, Li X, Yi X, Duan H 2021 Extreme Mech. Lett. 44 101211
Google Scholar
[53] Wang G, Feng X 2007 Appl. Phys. Lett. 90 231904
Google Scholar
[54] He J, Lilley C 2008 Nano Lett. 8 1798
Google Scholar
[55] Song X, Lu Y, Wang F, Zhao X, Chen H 2020 J. Power Sources 452 227803
Google Scholar
[56] Zhang K, Li Y, Zheng B, Wu G, Wu J, Yang F 2017 Int. J. Solids Struct. 108 230
Google Scholar
[57] Lee S, McDowell M, Berla L, Nix W, Cui Y 2012 Proc. Natl. Acad. Sci. U. S. A. 109 4080
Google Scholar
-
图 1 纳米线电极在锂化过程中屈曲的示意图 (a)有表面效应; (b)无表面效应
Fig. 1. Schematic illustration of nanowire electrode buckling during lithiation: (a) With surface effect; (b) without surface effect.
图 2 临界屈曲压力的结果 (a)临界屈曲压力随时间的变化情况, 其中实线表示考虑了表面效应, 点虚线表示没有考虑表面效应, 且红色线表示弹性硬化(
$ k=0.5 $ ), 靛蓝色线表示无弹性硬化/软化($ k=0 $ ), 黑色线表示弹性软化($ k=-0.5 $ ); (b)有表面效应与无表面效应的初始临界屈曲压力之比随电极半径的变化情况; (c)有表面效应与无表面效应的初始临界屈曲压力之比随电极长细比的变化情况Fig. 2. Results of critical buckling load: (a) Evolution of critical buckling load with time (The solid lines represent considering the surface effect, dotted lines represent without considering the surface effect, and red lines denote
$ k=0.5 $ , indigo lines denote$ k=0 $ , black lines denote$ k=-0.5 $ ); (b) ratio of initial critical buckling load between with and without surface effect as a function of radius; (c) ratio of initial critical buckling load between with and without surface effect as a function of slenderness ratio.
图 3 纳米线电极轴力的结果 (a)轴力随时间的变化情况, 其中实线表示考虑了表面效应, 点虚线表示没有考虑表面效应, 且红色线表示弹性硬化(
$ k=0.5 $ ), 靛蓝色线表示无弹性硬化/软化($ k=0 $ ), 黑色线表示弹性软化($ k=-0.5 $ ); (b) 初始轴力随纳米线电极半径的变化情况Fig. 3. Results of axial force
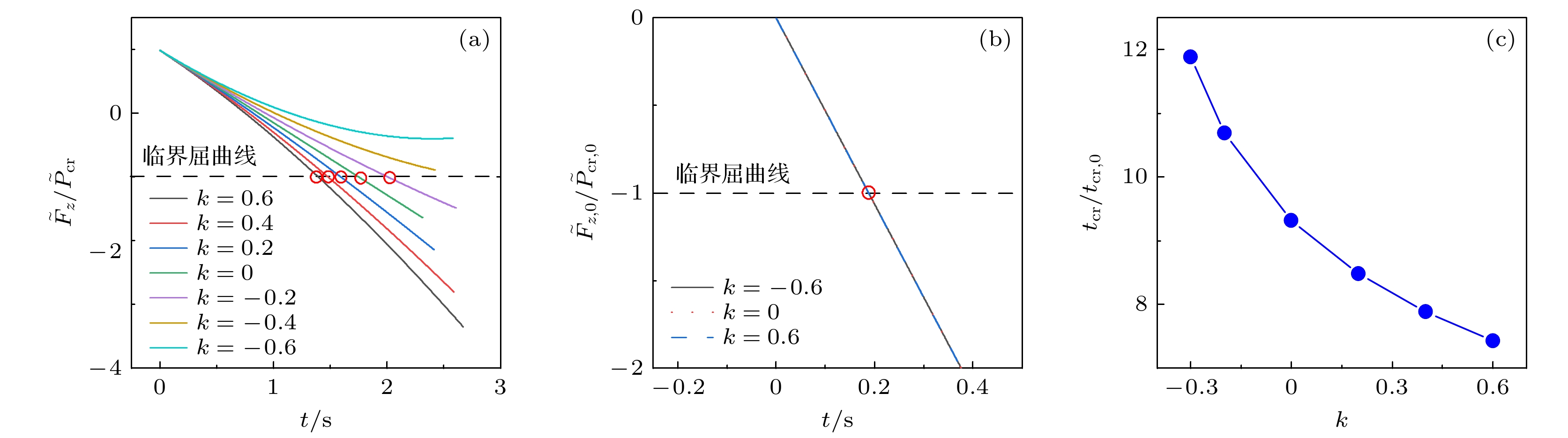
$ {\stackrel{~}{F}}_{z} $ : (a) Variation of axial force$ {\stackrel{~}{F}}_{z} $ with time$ \stackrel{~}{t} $ (The solid lines represent considering the surface effect, dotted lines represent without considering the surface effect, and red lines denote$ k=0.5 $ , indigo lines denote$ k=0 $ , black lines denote$ k=-0.5 $ ); (b) initial axial force with surface effect as a function of radius
图 4 纳米线电极屈曲的结果情况 (a)有表面效应的条件下, 轴力与临界屈曲压力之比在不同的电极长细比下随时间的变化情况; (b)无表面效应的条件下, 轴力与临界屈曲压力之比在不同的电极长细比下随时间的变化情况; (c)有表面效应与无表面效应的纳米线电极达到临界屈曲所需的时间之比随电极长细比的变化情况; (d)有表面效应的条件下, 轴力与临界屈曲压力之比在不同的电极尺寸下随时间的变化情况; (e)无表面效应的条件下, 轴力与临界屈曲压力之比在不同的电极尺寸下随时间的变化情况; (f)有表面效应与无表面效应的纳米线电极达到临界屈曲所需的时间之比随电极尺寸的变化情况
Fig. 4. Results of nanowire electrode buckling: (a) Under the condition of with surface effect, the ratio of axial force to critical buckling load as a function of time for different slenderness ratio; (b) under the condition of without surface effect, the ratio of axial force to critical buckling load as a function of time for different slenderness ratio; (c) ratio of the time required for nanowire electrodes with surface effect and without surface effect to reach critical buckling varies with slenderness ratio; (d) under the condition of with surface effect, the ratio of axial force to critical buckling load as a function of time for different radius; (e) under the condition of without surface effect, the ratio of axial force to critical buckling load as a function of time for different radius; (f) ratio of the time required for nanowire electrodes with surface effect and without surface effect to reach critical buckling varies with radius.
图 5 弹性硬化/软化系数对屈曲的影响 (a)在有表面效应的条件下, 轴力与临界屈曲压力之比在不同的电极弹性硬化/软化系数下随时间的变化情况; (b)无表面效应的条件下, 轴力与临界屈曲压力之比在不同的电极弹性硬化/软化系数下随时间的变化情况; (c)有表面效应与无表面效应的纳米线电极达到临界屈曲所需的时间之比随电极弹性硬化/软化系数的变化情况
Fig. 5. Effect of elastic hardening/softening coefficient on buckling: (a) Under the condition of with surface effect, the ratio of axial force to critical buckling load as a function of time for different hardening/softening coefficient; (b) under the condition of without surface effect, the ratio of axial force to critical buckling load as a function of time for different hardening/softening coefficient; (c) ratio of the time required for nanowire electrodes with surface effect and without surface effect to reach critical buckling varies with hardening/softening coefficient.
表 1 材料参数
Table 1. Material parameters[55].
物理参数 符号/单位 值 未锂化的弹性模量 $ {E}_{0} $/GPa 70 泊松比 v 0.2 偏摩尔体积 $\varOmega /({\mathrm{m} }^{3}{\cdot}{\mathrm{m}\mathrm{o}\mathrm{l} }^{-1})$ 1.92 × 10–6 锂的最大浓度 ${c}_{\mathrm{m} }/(\mathrm{m}\mathrm{o}\mathrm{l}{\cdot} {\mathrm{m} }^{-3})$ 2.33 × 104 扩散系数 ${D}_{0}/({\mathrm{m} }^{2}{\cdot} {\mathrm{s} }^{-1})$ 1.76 × 10–15 表面残余张力 $\tau /(\mathrm{J}{\cdot}{\mathrm{m} }^{-2})$ 2 长细比 $ {l}_{0}/{r}_{0} $ 50 电极半径尺寸 $ {r}_{0} $/nm 10 
下载: 导出CSV
-
[1] Larcher D, Tarascon J 2015 Nat. Chem. 7 19
Google Scholar
[2] Scrosati B, Garche J 2010 J. Power Sources 195 2419
Google Scholar
[3] Zhang S, Zhao K, Zhu T, Li J 2017 Prog. Mater. Sci. 89 479
Google Scholar
[4] Gür T 2018 Energy Environ. Sci. 11 2696
Google Scholar
[5] Edge J, O’Kane S, Prosser R, Kirkaldy N, Patel A, Hales A, Ghosh A, Ai W, Chen J, Yang J, Li S, Pang M, Bravo D, Tomaszewska A, Marzook M, Radhakrishnan K, Wang H, Patel Y, Wu B, Offer G 2021 Phys. Chem. Chem. Phys. 23 8200
Google Scholar
[6] Zhang W 2011 J. Power Sources 196 13
Google Scholar
[7] Chan C, Peng H, Liu G, McIlwrath K, Zhang X, Huggins R, Cui Y 2008 Nat. Nanotechnol. 3 31
Google Scholar
[8] Yu C, Li X, Ma T, Rong J, Zhang R, Shaffer J, An Y, Liu Q, Wei B, Jiang H 2012 Adv. Energy Mater. 2 68
Google Scholar
[9] Yao Y, McDowell M, Ryu I, Wu H, Liu N, Hu L, Nix W, Cui Y 2011 Nano Lett. 11 2949
Google Scholar
[10] Kim H, Han B, Choo J, Cho J 2008 Angew. Chem. 120 10305
Google Scholar
[11] Dasgupta N P, Sun J, Liu C, Brittman S, Andrews S C, Lim J, Gao H, Yan R, Yang P 2014 Adv. Mater. 26 2137
Google Scholar
[12] Chen L, Lu N, Xu C, Yu H, Wang T 2009 Electrochim. Acta 54 4198
Google Scholar
[13] Chan C, Zhang X, Cui Y 2008 Nano Lett. 8 307
Google Scholar
[14] Liu, X, Fan F, Yang H, Zhang S, Huang J, Zhu T 2013 ACS Nano 7 1495
Google Scholar
[15] Huang J, Li Z, Chong M, John P, Wu X, Li Q, Scott X, Nicholas S, Xiao H, Arunkumar S 2010 Science 330 1515
Google Scholar
[16] Wang X, Fan F, Wang J, Wang H, Tao S, Yang A, Liu Y, Beng C, Mao S, Zhu T, Xia S 2015 Nat. Commun. 6 8417
Google Scholar
[17] Chakraborty J, Please C, Goriely A, Chapman S 2015 Int. J. Solids Struct. 54 66
Google Scholar
[18] Zhang K, Li Y, Wu J, Zheng B, Yang F 2018 Int. J. Solids Struct. 144 289
Google Scholar
[19] Shen X, Wan Y 2021 Meccanica 1 1
Google Scholar
[20] Li Y, Zhang K, Zheng B, Yang F 2016 J. Phys. D: Appl. Phys. 49 285602
Google Scholar
[21] Zhang K, Chen J, Li Y, Liu D, Zheng B, Kai Y 2020 Results Phys. 16 103018
Google Scholar
[22] Li Y, Mao W, Zhang K, Jia Y, Yang F 2019 J. Phys. D: Appl. Phys. 52 435502
Google Scholar
[23] Zhang Y, Zhan S, Zhang K, Zheng B, Lyu L 2021 Eur. J. Mech. A/Solids 85 104111
Google Scholar
[24] Xing H, Liu Y, Wang B 2019 Acta Mech. 230 4145
Google Scholar
[25] Yeerella R, Boddeda H, Sengupta A, Chakraborty J 2020 J. Appl. Phys. 128 234901
Google Scholar
[26] Herring C 1951 Phys. Rev. 82 87
Google Scholar
[27] Gurtin M, Murdoch A 1975 Arch. Ration. Mech. Anal. 57 291
Google Scholar
[28] Miller R, Shenoy V 2000 Nanotechnology 11 139
Google Scholar
[29] Cammarata R 1994 Prog. Surf. Sci. 46 1
Google Scholar
[30] Cheng Y, Verbrugge M 2008 J. Appl. Phys. 104 083521
Google Scholar
[31] Deshpande R, Cheng Y, Verbrugge M 2010 J. Power Sources 195 5081
Google Scholar
[32] Hao F, Gao X, Fang D 2012 J. Appl. Phys. 112 103507
Google Scholar
[33] Sengupta A, Chakraborty J 2019 Acta Mech. 231 999
Google Scholar
[34] Gao Y, Zhou M 2011 J. Appl. Phys. 109 014310
Google Scholar
[35] Gao X, Fang D, Qu J 2015 Proc. R. Soc. London, Ser. A 471 20150366
Google Scholar
[36] Jia N, Peng Z, Wang S, Li J, Yao Y, Chen S 2020 Sci. China Ser. E: Technol. Sci. 63 2413
Google Scholar
[37] Zhang X, Chen H, Fang D 2020 Int. J. Mech. Sci. 169 105323
Google Scholar
[38] Stein P, Zhao Y, Xu B 2016 J. Power Sources 332 154
Google Scholar
[39] Bucci G, Swamy T, Bishop S, Sheldon B, Chiang Y, Carter W 2017 J. Electrochem. Soc. 164 A645
Google Scholar
[40] Lu Y, Zhang P, Wang F, Zhang K, Zhao X 2018 Electrochim. Acta 274 359
Google Scholar
[41] Young S, Ji L, Chang S, Fang T, Hsueh T, Meen T, Chen I 2007 Nanotechnology 18 225603
Google Scholar
[42] Riaz M, Nur O, Willander M, Klason P 2008 Appl. Phys. Lett. 92 103118
Google Scholar
[43] Wang G, Feng X 2009 Appl. Phys. Lett. 94 141913
Google Scholar
[44] Shenoy V, Johari P, Qi Y 2010 J. Power Sources 195 6825
Google Scholar
[45] Qi Y, Guo H, Hector J, L G, Timmons A 2010 J. Electrochem. Soc. 157 A558
Google Scholar
[46] Qi Y, Hector L, James C, Kim K 2014 J. Electrochem. Soc. 161 F3010
Google Scholar
[47] Swaminathan N, Balakrishnan S, George K 2015 J. Electrochem. Soc. 163 A488
Google Scholar
[48] Sharma P, Ganti S, Bhate N 2003 Appl. Phys. Lett. 82 535
Google Scholar
[49] Zhang X, Shyy W, Marie S 2007 J. Electrochem. Soc. 154 A910
Google Scholar
[50] Tian L, Rajapakse R 2007 Int. J. Solids Struct. 44 7988
Google Scholar
[51] He L, Li Z 2006 Int. J. Solids Struct. 43 6208
Google Scholar
[52] Wang S, Li X, Yi X, Duan H 2021 Extreme Mech. Lett. 44 101211
Google Scholar
[53] Wang G, Feng X 2007 Appl. Phys. Lett. 90 231904
Google Scholar
[54] He J, Lilley C 2008 Nano Lett. 8 1798
Google Scholar
[55] Song X, Lu Y, Wang F, Zhao X, Chen H 2020 J. Power Sources 452 227803
Google Scholar
[56] Zhang K, Li Y, Zheng B, Wu G, Wu J, Yang F 2017 Int. J. Solids Struct. 108 230
Google Scholar
[57] Lee S, McDowell M, Berla L, Nix W, Cui Y 2012 Proc. Natl. Acad. Sci. U. S. A. 109 4080
Google Scholar
下载:
计量
- 文章访问数: 4540
- PDF下载量: 60
- 被引次数: 0
