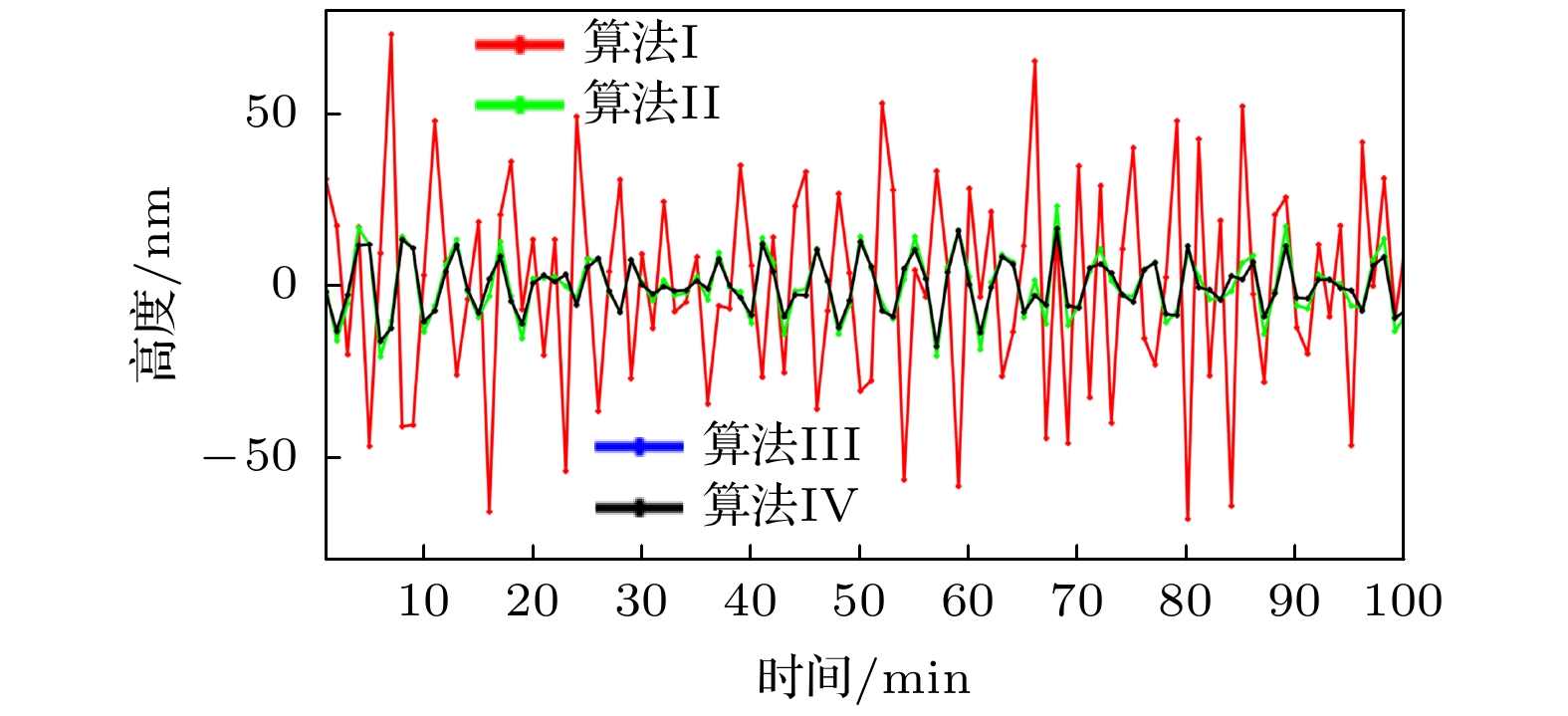
-
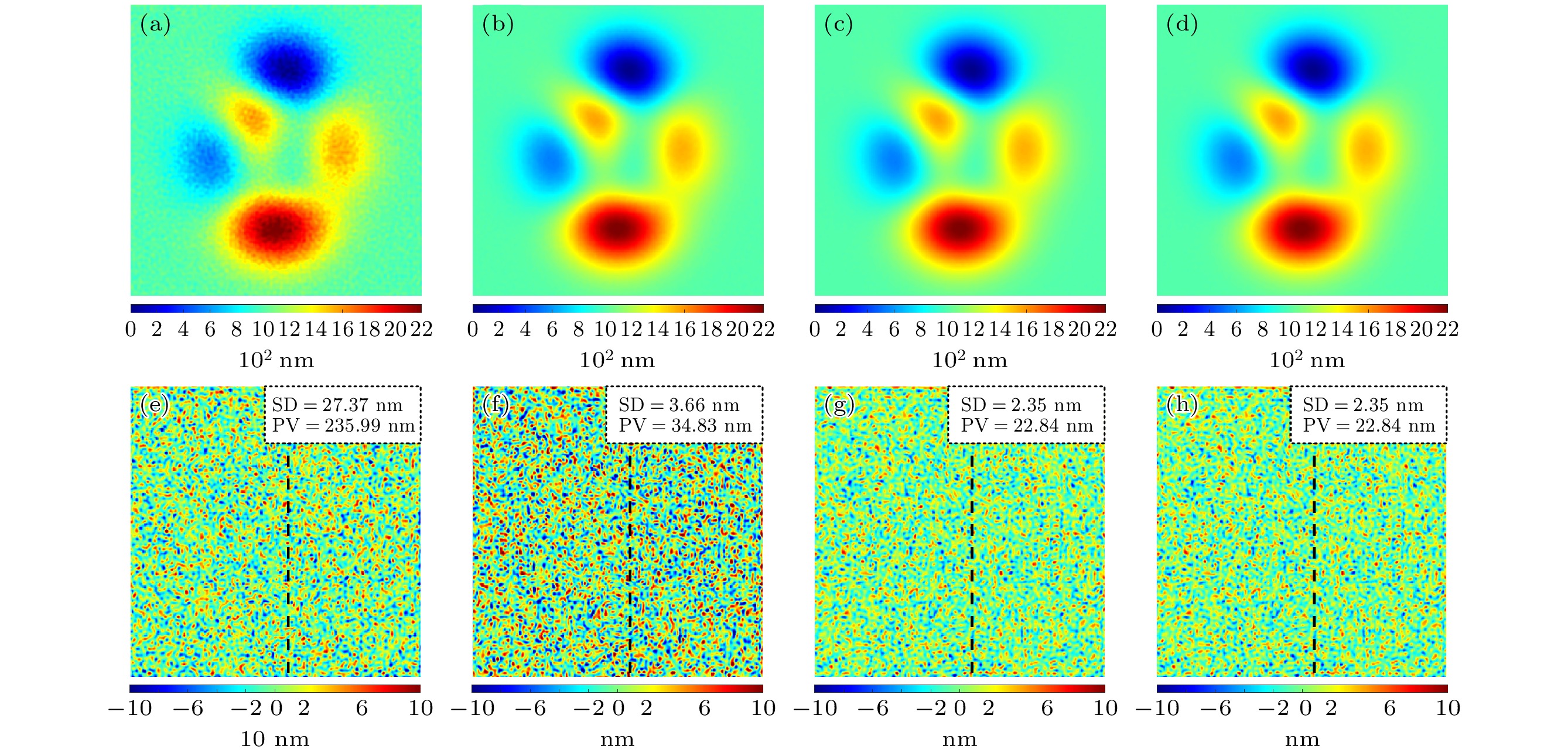
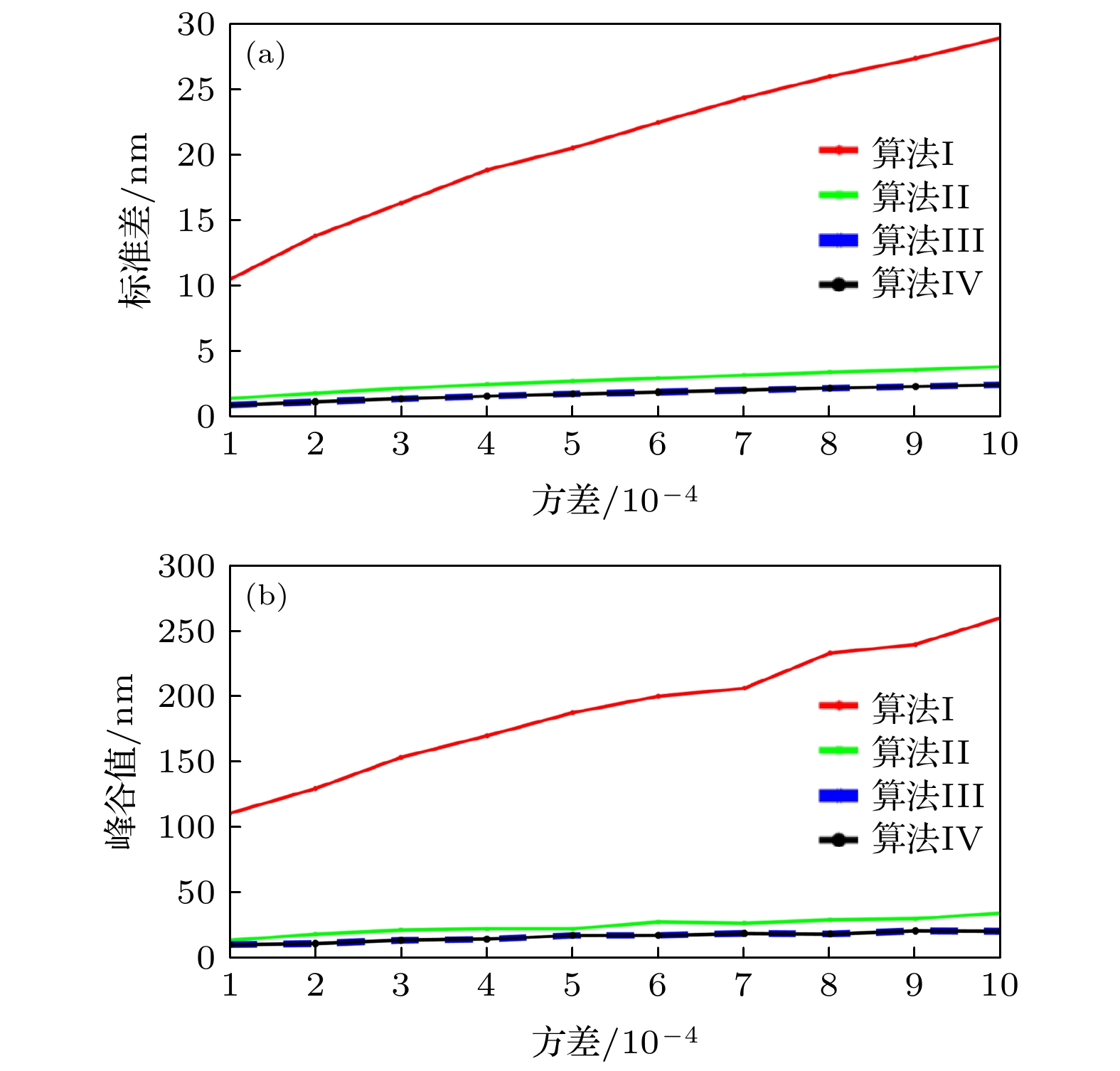
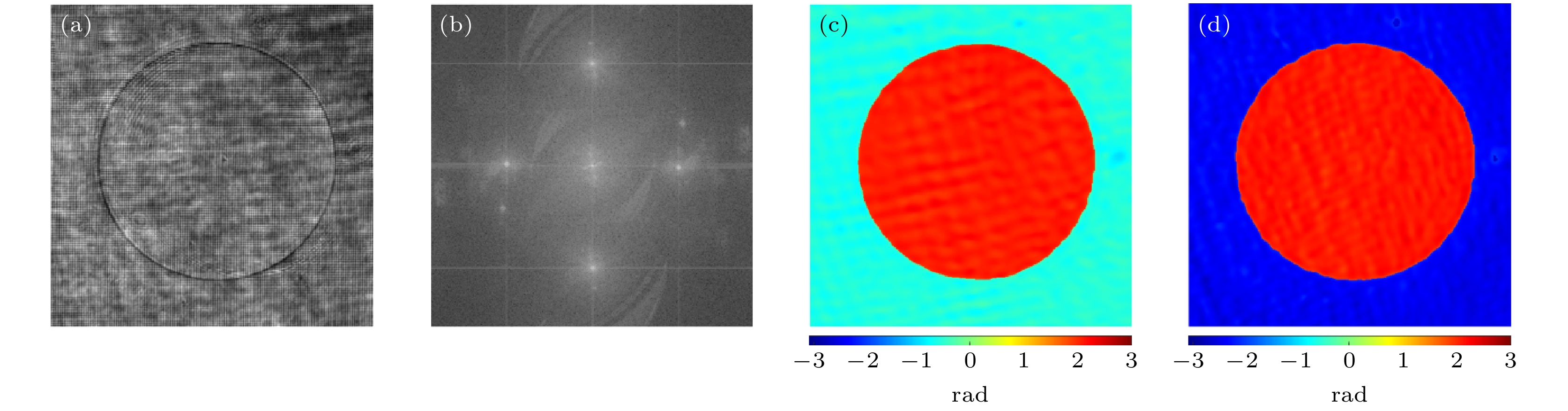
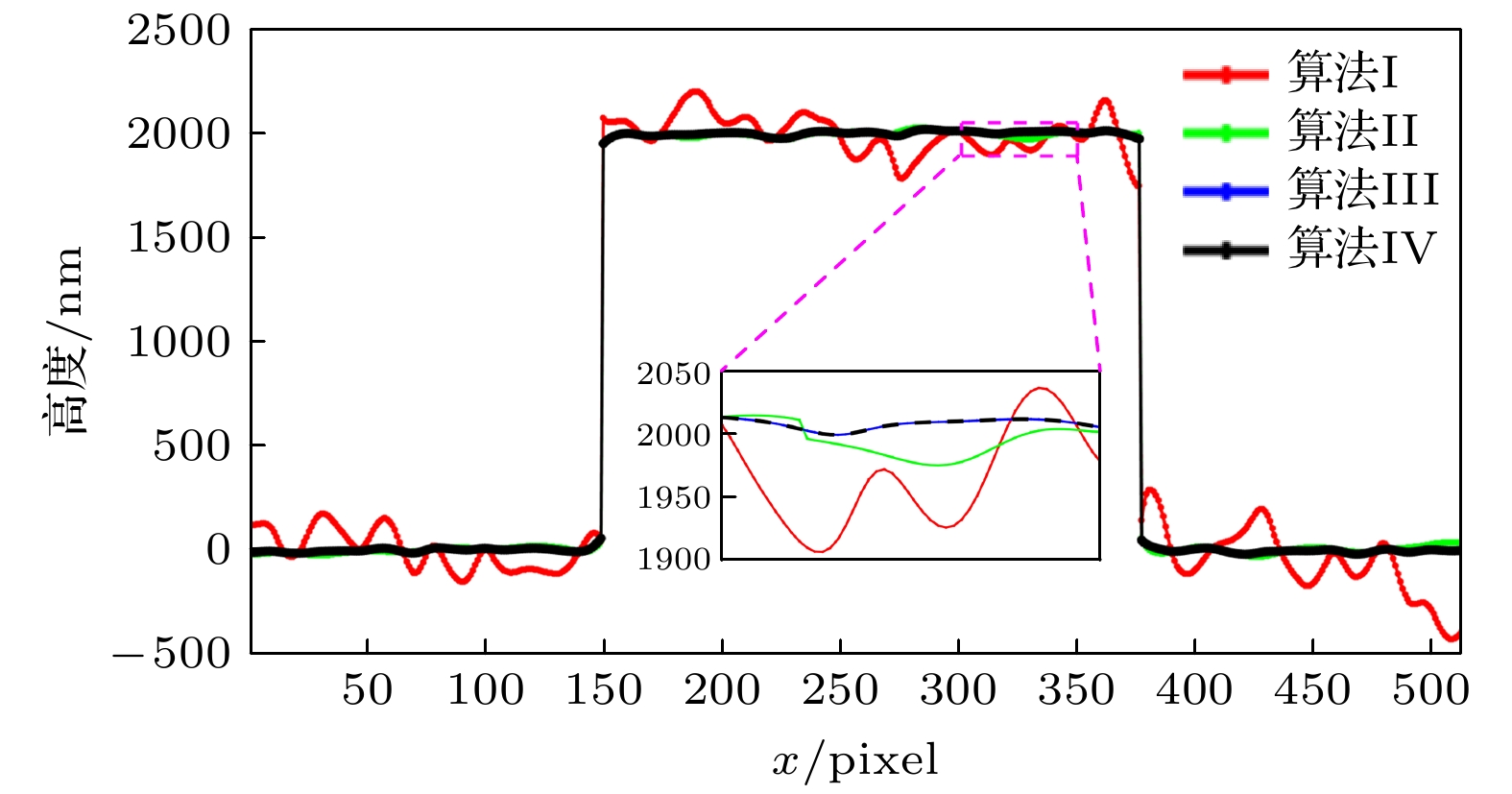
Dual-wavelength digital holography can expand the unambiguous measurement depth in phase unwrapping by using a differential synthetic wavelength which is longer than the single illumination wavelength. However, the phase noise is significantly amplified due to the magnification of the differential synthetic wavelength, resulting in a lower measurement accuracy. On the other hand, a lower noise level can be achieved by using additive synthetic-wavelength which is shorter than the single illumination wavelength. However, the corresponding unambiguous measurement depth is greatly reduced due to the phase ambiguity. In this case, combining the merits of the differential synthetic-wavelength and the additive synthetic-wavelength, different low noise phase unwrapping algorithms have been developed in recent years. However, these algorithms are complex and time consuming because they need to calculate multiple intermediate variables or search for the constrained boundary conditions in two-dimensional space. Therefore, in this paper, we develop a hierarchical phase unwrapping algorithm by using the two synthetic wavelengths for dual-wavelength digital holography to realize low noise and fast unambiguous measurement with large depth. In this algorithm, the unwrapped phase difference obtained by the differential synthetic wavelength is used to guide the wrapped phase of one single wavelength to realize phase unwrapping, and then the optical path difference obtained by the single-wavelength unwrapped phase is employed to guide the wrapped phase sum, and thus realizing phase unwrapping. As a result, the phase noise is attenuated and the depth sensitivity is preserved for dual-wavelength phase unwrapping. After theoretical analysis, a series of simulation experiments is carried out on the reconstructed quality, anti-noise characteristics and speed through comparing with state-of-the-art dual-wavelength phase unwrapping algorithms, including the conventional algorithm, the linear programming algorithm and the direct linear programming algorithm. In this case, a flipping dual-wavelength common-path digital holography with orthogonal carrier is built to acquire multiplexed off-axis hologram in one shot and illustrate the operation of the algorithm with circular step target, and stability test of the setup. Both the simulation and experimental results show that the proposed method can be simplified and deterministic, resulting in a lower noise phase unwrapping in a time of 20.5 ms for a phase map of one megapixel. We expect that the proposed method can have practical applications in measurement that requires high accuracy, fast speed, and large depth.
[1] Gabor D 1948 Nature 161 777
 Google Scholar
Google Scholar
[2] Lu X X, Chen J P, Liu S D, Ma Z J, Zhang Z, Zhong L Y 2012 Opt. Las. Eng. 50 1431
 Google Scholar
Google Scholar
[3] Popescu P, Ikeda T, Dasari R R, Feld M S 2006 Opt. lett. 31 775
 Google Scholar
Google Scholar
[4] Girshovitz P Shaked N T 2013 Opt. Express 21 5701
 Google Scholar
Google Scholar
[5] Guo R L, Yao B L, Gao P, Min J W, Han J, Yu X, Lei M, Yan S H, Yang Y L, Dan D, Ye T 2013 Appl. Opt. 52 3484
 Google Scholar
Google Scholar
[6] Hao B G, Diao M, Shan M G, Zhang Y B, Zhong Z 2013 Opt. Express 21 2126
 Google Scholar
Google Scholar
[7] Frenklach I, Girshovitz P, Shaked N T 2014 Opt. Lett. 39 1525
 Google Scholar
Google Scholar
[8] Rawat S, Komatsu S, Markman A, Anand A, Javidi B 2017 Appl. Opt. 56 D127
 Google Scholar
Google Scholar
[9] Hu C F, Zhu S S, Gao L, Popescu G 2018 Opt. Lett. 43 3373
 Google Scholar
Google Scholar
[10] Picazo-Bueno J A, Trusiak M, MicóV 2019 Opt. Express 27 5655
 Google Scholar
Google Scholar
[11] Shaked N T, Micó V, Trusiak M, Kuś A, Mirsky S K 2020 Adv. Opt. Photon. 12 556
 Google Scholar
Google Scholar
[12] Polhemus C 1973 Appl. Opt. 12 2071
 Google Scholar
Google Scholar
[13] Gass J, Dakoff A, Kim M K 2003 Opt. Lett. 28 1141
 Google Scholar
Google Scholar
[14] Kühn J, Colomb T, Montfort F, Charrière F, Emery Y, Cuche E, Marquet P, Depeursinge C 2007 Opt. Express 15 7231
 Google Scholar
Google Scholar
[15] Min J W, Yao B L, Zhou M L, Guo R L, Lei M, Yang Y L, Dan D, Yan S H, Peng T 2014 J. Opt. 16 125409
 Google Scholar
Google Scholar
[16] 袁飞, 袁操今, 聂守平, 朱竹青, 马青玉, 李莹, 朱文艳, 冯少彤 2014 63 166
 Google Scholar
Google Scholar
Yuan F, Yuan C J, Nie S P, Zhu Z Q, Ma Q Y, Li Y, Zhu W Y, Feng S T 2014 Acta Phys. Sin. 63 166
 Google Scholar
Google Scholar
[17] Khmaladze A, Matz R L, Zhang C, Wang T, Banaszak Holl M M, Chen Z 2011 Opt. Lett. 36 912
 Google Scholar
Google Scholar
[18] Wang Z M, Jiao J N, Qu W J, Yang F, Li H R, Tian A L, Asundi A 2017 Appl. Opt. 56 424
 Google Scholar
Google Scholar
[19] Di J L, Zhang J W, Xi T L, Ma C J, Zhao J L 2015 J. Micro/Nanolithography, MEMS, and MOEMS 14 041313
 Google Scholar
Google Scholar
[20] Xiong J X, Zhong L Y, Liu S D, Qiu X, Zhou Y F, Tian J D, Lu X X 2017 Opt. Express 25 7181
 Google Scholar
Google Scholar
[21] Shan M G, Liu L, Zhong Z, Liu B, Zhang Y B 2019 Opt. Las. Eng. 117 1
 Google Scholar
Google Scholar
[22] Liu Q, Li L L, Huang X J, Zhang H, Yue X B 2020 J. Opt. 22 045701
 Google Scholar
Google Scholar
[23] Shan M G, Liu L, Zhong Z, Liu B, Luan G Y, Zhang Y B 2017 Opt. Express 25 26253
 Google Scholar
Google Scholar
[24] Liu L, Shan M G, Zhong Z, Liu B, Luan G Y, Diao M, Zhang Y B 2017 Opt. Lett. 42 4331
 Google Scholar
Google Scholar
-
-
[1] Gabor D 1948 Nature 161 777
 Google Scholar
Google Scholar
[2] Lu X X, Chen J P, Liu S D, Ma Z J, Zhang Z, Zhong L Y 2012 Opt. Las. Eng. 50 1431
 Google Scholar
Google Scholar
[3] Popescu P, Ikeda T, Dasari R R, Feld M S 2006 Opt. lett. 31 775
 Google Scholar
Google Scholar
[4] Girshovitz P Shaked N T 2013 Opt. Express 21 5701
 Google Scholar
Google Scholar
[5] Guo R L, Yao B L, Gao P, Min J W, Han J, Yu X, Lei M, Yan S H, Yang Y L, Dan D, Ye T 2013 Appl. Opt. 52 3484
 Google Scholar
Google Scholar
[6] Hao B G, Diao M, Shan M G, Zhang Y B, Zhong Z 2013 Opt. Express 21 2126
 Google Scholar
Google Scholar
[7] Frenklach I, Girshovitz P, Shaked N T 2014 Opt. Lett. 39 1525
 Google Scholar
Google Scholar
[8] Rawat S, Komatsu S, Markman A, Anand A, Javidi B 2017 Appl. Opt. 56 D127
 Google Scholar
Google Scholar
[9] Hu C F, Zhu S S, Gao L, Popescu G 2018 Opt. Lett. 43 3373
 Google Scholar
Google Scholar
[10] Picazo-Bueno J A, Trusiak M, MicóV 2019 Opt. Express 27 5655
 Google Scholar
Google Scholar
[11] Shaked N T, Micó V, Trusiak M, Kuś A, Mirsky S K 2020 Adv. Opt. Photon. 12 556
 Google Scholar
Google Scholar
[12] Polhemus C 1973 Appl. Opt. 12 2071
 Google Scholar
Google Scholar
[13] Gass J, Dakoff A, Kim M K 2003 Opt. Lett. 28 1141
 Google Scholar
Google Scholar
[14] Kühn J, Colomb T, Montfort F, Charrière F, Emery Y, Cuche E, Marquet P, Depeursinge C 2007 Opt. Express 15 7231
 Google Scholar
Google Scholar
[15] Min J W, Yao B L, Zhou M L, Guo R L, Lei M, Yang Y L, Dan D, Yan S H, Peng T 2014 J. Opt. 16 125409
 Google Scholar
Google Scholar
[16] 袁飞, 袁操今, 聂守平, 朱竹青, 马青玉, 李莹, 朱文艳, 冯少彤 2014 63 166
 Google Scholar
Google Scholar
Yuan F, Yuan C J, Nie S P, Zhu Z Q, Ma Q Y, Li Y, Zhu W Y, Feng S T 2014 Acta Phys. Sin. 63 166
 Google Scholar
Google Scholar
[17] Khmaladze A, Matz R L, Zhang C, Wang T, Banaszak Holl M M, Chen Z 2011 Opt. Lett. 36 912
 Google Scholar
Google Scholar
[18] Wang Z M, Jiao J N, Qu W J, Yang F, Li H R, Tian A L, Asundi A 2017 Appl. Opt. 56 424
 Google Scholar
Google Scholar
[19] Di J L, Zhang J W, Xi T L, Ma C J, Zhao J L 2015 J. Micro/Nanolithography, MEMS, and MOEMS 14 041313
 Google Scholar
Google Scholar
[20] Xiong J X, Zhong L Y, Liu S D, Qiu X, Zhou Y F, Tian J D, Lu X X 2017 Opt. Express 25 7181
 Google Scholar
Google Scholar
[21] Shan M G, Liu L, Zhong Z, Liu B, Zhang Y B 2019 Opt. Las. Eng. 117 1
 Google Scholar
Google Scholar
[22] Liu Q, Li L L, Huang X J, Zhang H, Yue X B 2020 J. Opt. 22 045701
 Google Scholar
Google Scholar
[23] Shan M G, Liu L, Zhong Z, Liu B, Luan G Y, Zhang Y B 2017 Opt. Express 25 26253
 Google Scholar
Google Scholar
[24] Liu L, Shan M G, Zhong Z, Liu B, Luan G Y, Diao M, Zhang Y B 2017 Opt. Lett. 42 4331
 Google Scholar
Google Scholar
计量
- 文章访问数: 7284
- PDF下载量: 127
- 被引次数: 0













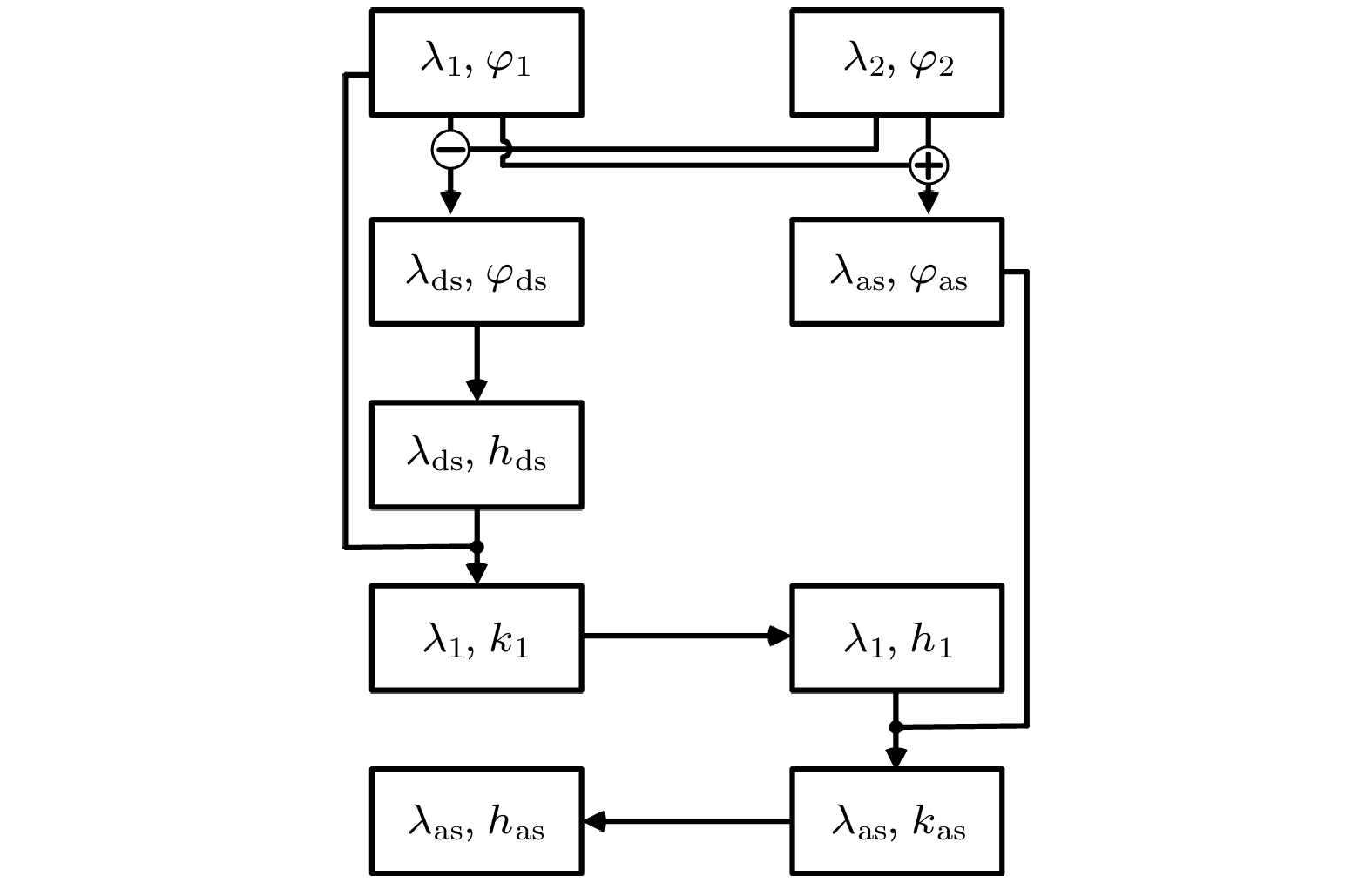
 下载:
下载: