低噪声超导量子干涉器件磁强计设计与制备

韩昊轩; 张国峰; 张雪; 梁恬恬; 应利良; 王永良; 彭炜; 王镇

doi:10.7498/aps.68.20190483

摘要

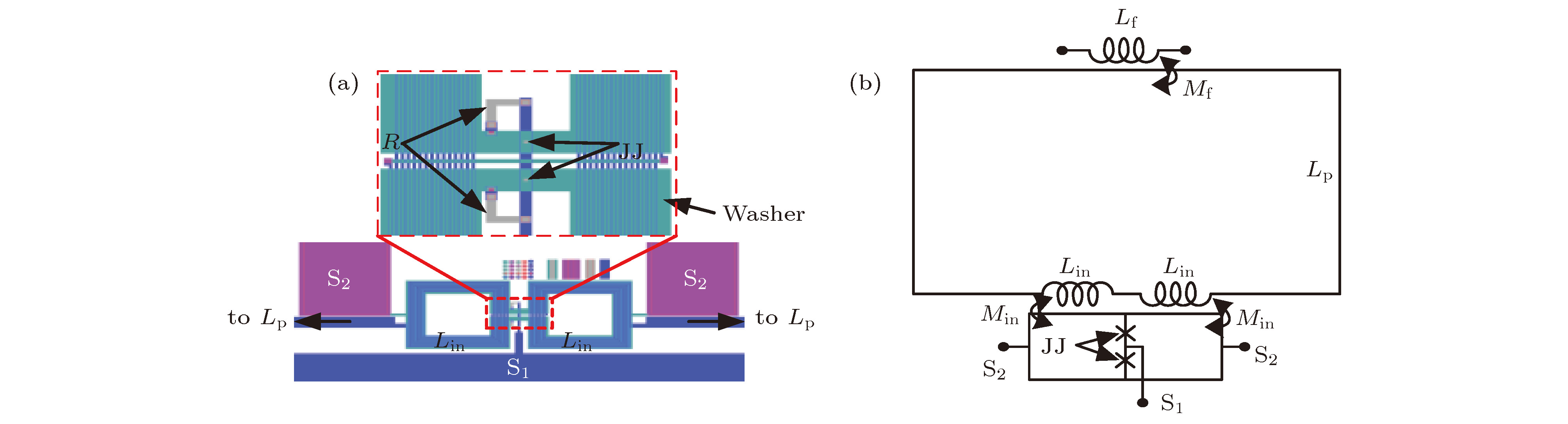
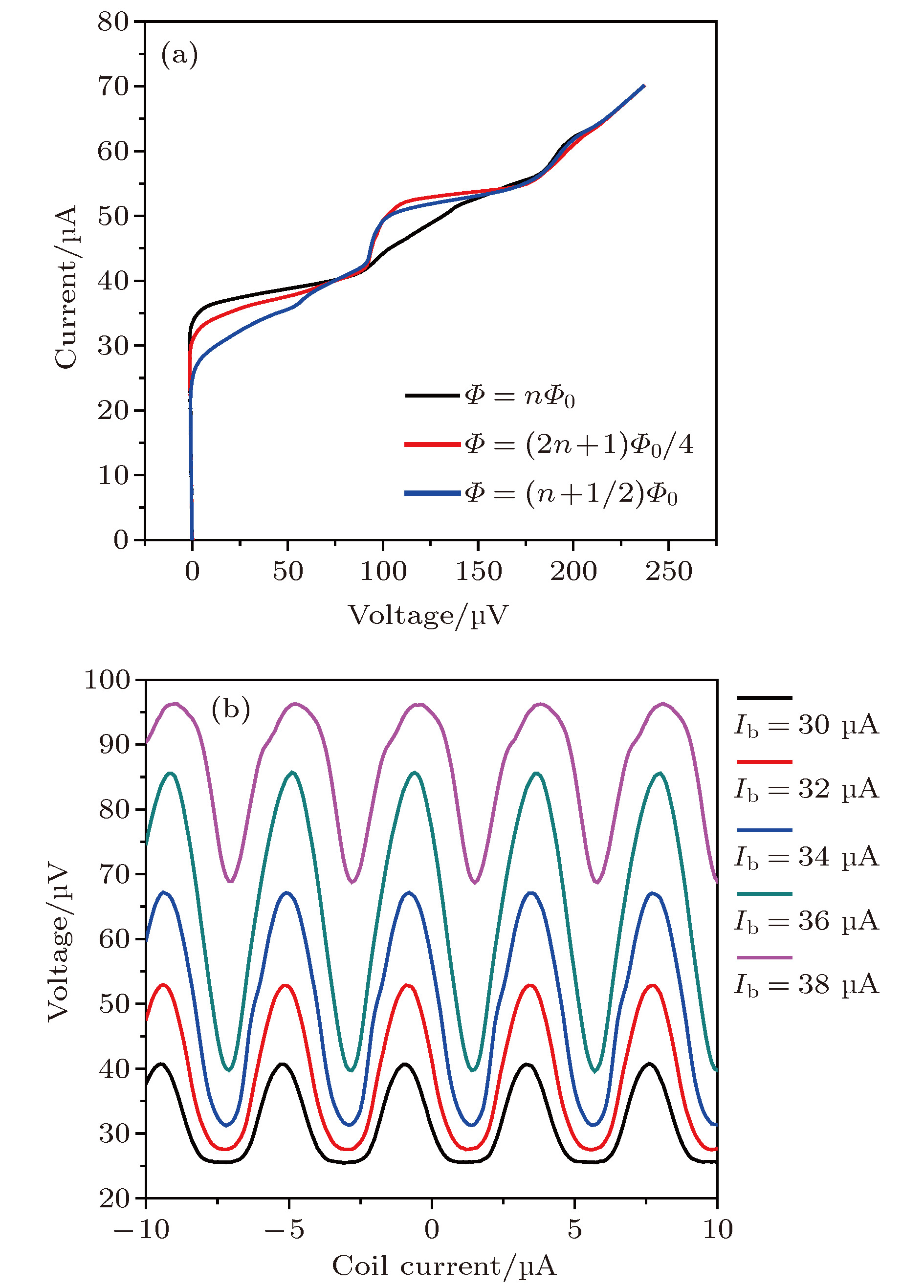
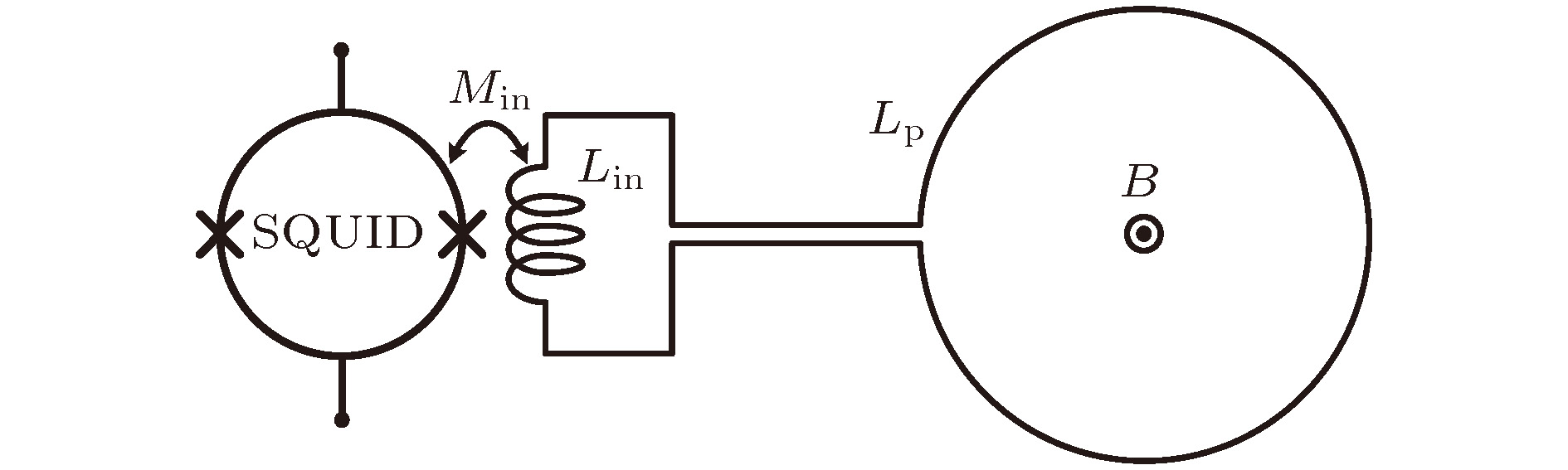
超导量子干涉器件(superconducting quantum interference device, SQUID)作为一种极灵敏的磁通传感器, 在生物磁探测、低场核磁共振、地球物理等领域得到广泛应用. 本文介绍了一种基于SQUID的高灵敏度磁强计, 由SQUID和一组磁通变压器组成. SQUID采用一阶梯度构型, 增强其抗干扰性. 磁通变压器由多匝螺旋的输入线圈和大尺寸单匝探测线圈组成, 其中输入线圈与SQUID通过互感进行磁通耦合. 利用自主工艺平台, 在4英寸硅衬底上完成了基于Nb/Al-AlO_x/Nb约瑟夫森隧道结的SQUID磁强计制备. 低温测试结果显示, 该磁强计磁场灵敏度为0.36 nT/Φ₀, 白噪声段磁通噪声为8 μΦ₀/√Hz, 等效磁场噪声为2.88 fT/√Hz.

关键词:

Abstract

Superconducting quantum interference device (SQUID) is the most sensitive magnetic flux sensor known, which is widely used in biomagnetism, low-field nuclear magnetic resonance, geophysics, etc. In this paper, we introduce a high-sensitivity SQUID magnetometer, which consists of an SQUID and a flux transformer. The SQUID is first-order gradiometer configuration, which is insensitive to interference noise. The flux transformer includes a multi-turn spiral input coil and a large-sized pickup coil. And the input coil is inductively coupled to the SQUID through mutual inductance. We present an SQUID magnetometer fabricated with Nb/Al-AlO_x/Nb Josephson junction technology on a 4-inch silicon wafer at our superconducting electronics facilities. We develop a fabrication process based on selective niobium etching process consisting of five mask levels. In the first two mask levels, the trilayer is patterned by a dry etch to define base electrode, contact pads, and interconnects. The shunt resistor and a dielectric insulating layer are then deposited and patterned by using lift-off and dry etchant, respectively. Finally, the niobium wiring layer is deposited and patterned by using reactive ion etching to define input, pickup and feedback coils. The measurement of the SQUID magnetometer is performed inside a magnetically shielded room. The operating temperature is realized by immersing the SQUID into the liquid helium (4.2 K). Moreover, a superconducting niobium tube is employed to protect the SQUID from being disturbed by external environments. A homemade readout electronics instrument with low input voltage noise is used to characterize the SQUID magnetometer. The results of low-temperature measurements indicate that the magnetometer has a magnetic field sensitivity of 0.36 nT/Φ₀ and a white flux noise of 8 μΦ₀/√Hz,corresponding to a white field noise of 2.88 fT/√Hz. This kind of SQUID magnetometer is suitable for multi-channel systems, e.g., magnetocardiography, magnetoencephalography, etc. Although the SQUID process development benefits from the rapid advance of semiconductor process technology, the uniformity of the SQUID on one wafer is fluctuated due to the film deposition. Now, we have realized a best SQUID yield of 50% on a 4-inch wafer. In the future, the SQUID chip yield should be improved by well controlling the optimizing process. The device yield is expected to reach as high as 80%.

Keywords:

superconducting quantum interference device /
magnetometer /
flux noise

作者及机构信息

1.
中国科学院上海微系统与信息技术研究所，信息功能材料国家重点实验室，上海　200050

2.
中国科学院超导电子学卓越创新中心，上海　200050

3.
中国科学院大学，北京　100049

通信作者: 张国峰, gfzhang@mail.sim.ac.cn

Authors and contacts

1.
State Key Laboratory of Functional Materials for Informatics, Shanghai Institute of Microsystem and Information Technology, Chinese Academy of Sciences, Shanghai 200050, China

2.
Chinese Academy of Sciences, Center for Excellence in Superconducting Electronics, Shanghai 200050, China

3.
University of Chinese Academy of Sciences, Beijing 100049, China

Corresponding author: Zhang Guo-Feng, gfzhang@mail.sim.ac.cn

文章全文 : translate this paragraph

参考文献

[1]	Zhang S, Zhang G, Wang Y, Liu M, Li H, Qiu Y, Zeng, J, Kong X, Xie X 2013 Chin. Phys. B 22 128501 Google Scholar
[2]	Dong H, Wang Y, Zhang S, Sun Y, Xie X 2008 Supercond. Sci. Technol. 21 115009 Google Scholar
[3]	荣亮亮, 蒋坤, 裴易峰, 伍俊, 王远 2016 仪器仪表学报 37 12 Rong L, Jiang K, Pei Y, Wu J, Wang Y 2016 Chin. J. Sci. Instrum. 37 12
[4]	Josephson B D 1962 Phys. Lett. 1 251 Google Scholar
[5]	Tesche C D, Clarke J 1977 J. Low Temp. Phys. 27 301
[6]	Drung D, Matz H, Koch H 1995 Rev. Sci. Instrum. 66 3008 Google Scholar
[7]	Schmelz M, Stolz R, Zakosarenko V, Schoenau T, Anders A, Fritzsch L, Mueck M, Meyer H G 2011 Supercond. Sci. Technol. 24 065009 Google Scholar
[8]	Xie X, Zhang Y, Wang H, Wang Y, Mueck M, Dong H, Krause H J, Braginski A I, Offenhaeusser A, Jiang M 2010 Supercond. Sci. Technol. 23 065016 Google Scholar
[9]	Zeng J, Zhang Y, Mueck M, Krause H J, Braginski A I, Kong X, Xie X, Offenhaeusser A, Jiang M 2013 Appl. Phys. Lett. 103 042601 Google Scholar
[10]	Gurvitch M, Washington M A, Hugins H A 1983 Appl. Phys. Lett. 42 472 Google Scholar
[11]	Zhang G, Zhang Y, Zhang S, Krause H J, Wang Y, Liu C, Zeng J, Qiu Y, Kong X, Dong H, Xie X, Offenhaeusser A, Jiang M 2012 Physica C 480 10 Google Scholar
[12]	Xiong W, Xu W, Wu Y, Li G, Ying L, Peng W, Ren J, Wang Z 2018 IEEE Trans. Appl. Supercond. 28 1300605
[13]	Yuda M, Kuroda K, Nakano J 1987 Jpn. J. Appl. Phys. 2 6
[14]	Kuroda K, Yuda M 1988 Jpn. J. Appl. Phys. 63 2352 Google Scholar
[15]	Imamura T, Hasuo S 1989 IEEE Trans. Magn. 3 3029
[16]	Booi P A A, Livingston C A, Benz S P 1993 IEEE Trans. Appl. Supercond. MAG-25 1119
[17]	Sukuda K, Kawai J, Uehara G, Kado H 1993 IEEE Trans. Magn. 3 2944
[18]	Amos R S, Breyer P E, Huang H H, Lichtenberger A W 1995 IEEE Trans. Magn. 5 2326
[19]	Kang X, Ying L, Wang H, Zhang G, Peng W, Kong X, Xie X, Wang Z 2014 Physica C 503 29 Google Scholar
[20]	Zhao J, Zhang Y, Lee Y H, Krause H J 2014 Rev. Sci. Instrum. 85 054707 Google Scholar
[21]	Clarke J, Goubau W M, Ketchen M B 1976 J. Low. Temp. Phys. 25 99
[22]	Zhang X, Zhang G, Ying L, Xiong W, Han H, Wang Y, Rong L, Xie X, Wang Z 2018 Physica C 548 1

施引文献

图 1 SQUID磁强计示意图

Fig. 1. Schematic diagram of SQUID magnetometer.

下载: 全尺寸图片幻灯片

图 2 (a) SQUID磁强计设计图; (b)等效电路图

Fig. 2. (a) Design of SQUID magnetometer and (b) the schematic diagram.

下载: 全尺寸图片幻灯片

图 3 器件工艺流程图

Fig. 3. Process flow chart of SQUID magnetometer.

下载: 全尺寸图片幻灯片

图 4 (a)电流-电压特性曲线; (b)不同偏置电流下的电压-线圈电流(磁通)调制曲线, 其中调制周期为4.3 μA/Φ₀

Fig. 4. (a) Current-voltage curves; (b) voltage-coil current (flux) curves under different bias currents with a period of 4.3 μA/Φ₀.

下载: 全尺寸图片幻灯片

图 5 SQUID磁强计噪声曲线曲线中出现的杂峰主要是实验室震动干扰导致, 插图显示的是最佳工作点(W)时调制曲线

Fig. 5. Noise figure of SQUID magnetometer, in which the lines between 10–200 Hz were mainly caused by vibrations in the laboratory. The inset shows the modulation curve with the best working point.

下载: 全尺寸图片幻灯片

表 1 SQUID磁强计设计参数

Table 1. Design parameters of SQUID~magnetometer

参数	数值	单位
约瑟夫森结Josephson junction
尺寸A_J	4 × 4	μm²
临界电流I_c	8	μA
结电容C^[12]	0.56	pF
并联电阻R	10	Ω
SQUID
垫圈内边长a	100	μm
线宽w_s	300	μm
总电感L_s	350	pH
输入线圈
线圈匝数N	15	—
线宽w_in	3	μm
线距s_in	3	μm
探测线圈
线圈尺寸A_p	15 × 15	mm²
线宽w_p	100	μm

下载: 导出CSV

表 2 Nb/Al-AlOx/Nb三层膜生长参数

Table 2. Deposition parameters of Nb/Al-AlO_x/Nb trilayer.

薄膜	背景真空	Ar流量	Ar气压	恒电流	生长速率	厚度
底层Nb	2.3 × 10^–5 Pa	10 sccm	0.573 Pa	1.5 A	1.2 nm/s	100 nm
Al	3 × 10^–5 Pa	10 sccm	0.573 Pa	0.3 A	0.25 nm/s	10 nm
AlOx	氧气气压：2.6 kPa; 氧化时间：12 h					~2 nm
顶层Nb	2.3 × 10^–5 Pa	10 sccm	0.573 Pa	1.5 A	1.2 nm/s	100 nm

下载: 导出CSV

表 3 SQUID磁强计测试结果

Table 3. Measured results of SQUID magnetometer

参数	数值	单位
临界电流I₀	32	μA
正常态电阻R_n	5	Ω
反馈线圈耦合系数1/M_f	4.3	μA/Φ₀
最大调制峰峰值V_pp	47	μV
磁通噪声√S_Φ(白噪声)	8	μΦ₀/√Hz
磁场灵敏度1/A_eff	0.36	nT/Φ₀
磁场噪声√S_B(白噪声)	2.88	fT/√Hz

下载: 导出CSV

map

[1]	Zhang S, Zhang G, Wang Y, Liu M, Li H, Qiu Y, Zeng, J, Kong X, Xie X 2013 Chin. Phys. B 22 128501 Google Scholar
[2]	Dong H, Wang Y, Zhang S, Sun Y, Xie X 2008 Supercond. Sci. Technol. 21 115009 Google Scholar
[3]	荣亮亮, 蒋坤, 裴易峰, 伍俊, 王远 2016 仪器仪表学报 37 12 Rong L, Jiang K, Pei Y, Wu J, Wang Y 2016 Chin. J. Sci. Instrum. 37 12
[4]	Josephson B D 1962 Phys. Lett. 1 251 Google Scholar
[5]	Tesche C D, Clarke J 1977 J. Low Temp. Phys. 27 301
[6]	Drung D, Matz H, Koch H 1995 Rev. Sci. Instrum. 66 3008 Google Scholar
[7]	Schmelz M, Stolz R, Zakosarenko V, Schoenau T, Anders A, Fritzsch L, Mueck M, Meyer H G 2011 Supercond. Sci. Technol. 24 065009 Google Scholar
[8]	Xie X, Zhang Y, Wang H, Wang Y, Mueck M, Dong H, Krause H J, Braginski A I, Offenhaeusser A, Jiang M 2010 Supercond. Sci. Technol. 23 065016 Google Scholar
[9]	Zeng J, Zhang Y, Mueck M, Krause H J, Braginski A I, Kong X, Xie X, Offenhaeusser A, Jiang M 2013 Appl. Phys. Lett. 103 042601 Google Scholar
[10]	Gurvitch M, Washington M A, Hugins H A 1983 Appl. Phys. Lett. 42 472 Google Scholar
[11]	Zhang G, Zhang Y, Zhang S, Krause H J, Wang Y, Liu C, Zeng J, Qiu Y, Kong X, Dong H, Xie X, Offenhaeusser A, Jiang M 2012 Physica C 480 10 Google Scholar
[12]	Xiong W, Xu W, Wu Y, Li G, Ying L, Peng W, Ren J, Wang Z 2018 IEEE Trans. Appl. Supercond. 28 1300605
[13]	Yuda M, Kuroda K, Nakano J 1987 Jpn. J. Appl. Phys. 2 6
[14]	Kuroda K, Yuda M 1988 Jpn. J. Appl. Phys. 63 2352 Google Scholar
[15]	Imamura T, Hasuo S 1989 IEEE Trans. Magn. 3 3029
[16]	Booi P A A, Livingston C A, Benz S P 1993 IEEE Trans. Appl. Supercond. MAG-25 1119
[17]	Sukuda K, Kawai J, Uehara G, Kado H 1993 IEEE Trans. Magn. 3 2944
[18]	Amos R S, Breyer P E, Huang H H, Lichtenberger A W 1995 IEEE Trans. Magn. 5 2326
[19]	Kang X, Ying L, Wang H, Zhang G, Peng W, Kong X, Xie X, Wang Z 2014 Physica C 503 29 Google Scholar
[20]	Zhao J, Zhang Y, Lee Y H, Krause H J 2014 Rev. Sci. Instrum. 85 054707 Google Scholar
[21]	Clarke J, Goubau W M, Ketchen M B 1976 J. Low. Temp. Phys. 25 99
[22]	Zhang X, Zhang G, Ying L, Xiong W, Han H, Wang Y, Rong L, Xie X, Wang Z 2018 Physica C 548 1

[1]	刘怀远, 肖建飞, 吕昭征, 吕力, 屈凡明. Bi₂O₂Se纳米线的生长及其超导量子干涉器件. , 2024, 73(4): 047803. doi: 10.7498/aps.73.20231600
[2]	何安, 薛存. 缺陷调控临界温度梯度超导膜的磁通整流反转效应. , 2022, 71(2): 027401. doi: 10.7498/aps.71.20211157
[3]	闻海虎. 高温超导体磁通钉扎和磁通动力学研究简介. , 2021, 70(1): 017405. doi: 10.7498/aps.70.20201881
[4]	郑东宁. 超导量子干涉器件. , 2021, 70(1): 018502. doi: 10.7498/aps.70.20202131
[5]	徐达, 钟青, 曹文会, 王雪深, 王仕建, 李劲劲, 刘建设, 陈炜. 二阶梯度交叉耦合超导量子干涉仪电流传感器研制. , 2021, 70(12): 128501. doi: 10.7498/aps.70.20201816
[6]	梁恬恬, 张国峰, 伍文涛, 倪志, 王永良, 应利良, 伍俊, 荣亮亮, 彭炜, 高波. 串联超导量子干涉器件阵列制备与测试分析. , 2021, 70(17): 178501. doi: 10.7498/aps.70.20210467
[7]	梁超, 张洁, 赵可, 羊新胜, 赵勇. 拓扑超导体FeSe_xTe_1–_x单晶超导性能与磁通钉扎. , 2020, 69(23): 237401. doi: 10.7498/aps.69.20201125
[8]	李耀义, 贾金锋. 在人工拓扑超导体磁通涡旋中寻找Majorana零能模. , 2019, 68(13): 137401. doi: 10.7498/aps.68.20181698
[9]	张永升, 邱阳, 张朝祥, 李华, 张树林, 王永良, 徐小峰, 丁红胜, 孔祥燕. 多通道心磁系统标定方法研究. , 2014, 63(22): 228501. doi: 10.7498/aps.63.228501
[10]	刘明, 徐小峰, 王永良, 曾佳, 李华, 邱阳, 张树林, 张国峰, 孔祥燕, 谢晓明. 超导量子干涉器件读出电路中匹配变压器的传输特性研究. , 2013, 62(18): 188501. doi: 10.7498/aps.62.188501
[11]	王杨婧, 谢拥军, 雷振亚. 用于射频超导量子干涉器的新型单CSRR磁通聚焦器和谐振器. , 2012, 61(9): 094210. doi: 10.7498/aps.61.094210
[12]	张树林, 刘扬波, 曾佳, 王永良, 孔祥燕, 谢晓明. 基于低温超导量子干涉器件的脑听觉激励磁场探测. , 2012, 61(2): 020701. doi: 10.7498/aps.61.020701
[13]	周世平, 瞿海, 廖红印. 高温超导混合配对态与磁通涡旋格子. , 2002, 51(10): 2355-2361. doi: 10.7498/aps.51.2355
[14]	刘旭东, 王进, 刘楣, 邢定钰. 磁通密度对第Ⅱ类超导体磁通动力学的影响. , 2002, 51(5): 1122-1127. doi: 10.7498/aps.51.1122
[15]	董正超. 铁磁-绝缘层-铁磁-d波超导结中的量子干涉效应对微分电导与散粒噪声的影响. , 2002, 51(4): 894-897. doi: 10.7498/aps.51.894
[16]	马平, 姚坤, 谢飞翔, 张升原, 邓鹏, 何东风, 张凡, 刘乐园, 聂瑞娟, 王福仁, 王守证, 戴远东. 利用单通道高温超导磁梯度计获取心磁地图. , 2002, 51(2): 224-227. doi: 10.7498/aps.51.224
[17]	冯　勇, 周　廉, 杨万民, 张翠萍, 汪京荣, 于泽铭, 吴晓祖. PMP法YGdBaCuO超导体的磁通钉扎研究. , 2000, 49(1): 146-152. doi: 10.7498/aps.49.146
[18]	瞿海, 周世平. 高温超导体混合态磁通涡旋结构. , 1999, 48(2): 352-362. doi: 10.7498/aps.48.352
[19]	杜胜望, 戴远东, 王世光. 用射频超导量子干涉器件测量高温超导体序参量的位相. , 1999, 48(12): 2364-2368. doi: 10.7498/aps.48.2364
[20]	陈莺飞, S.ZAREMBINSKI. 阻尼型高温直流超导量子干涉器磁强计的设计. , 1998, 47(8): 1369-1377. doi: 10.7498/aps.47.1369

计量

文章访问数: 11464
PDF下载量: 272
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板