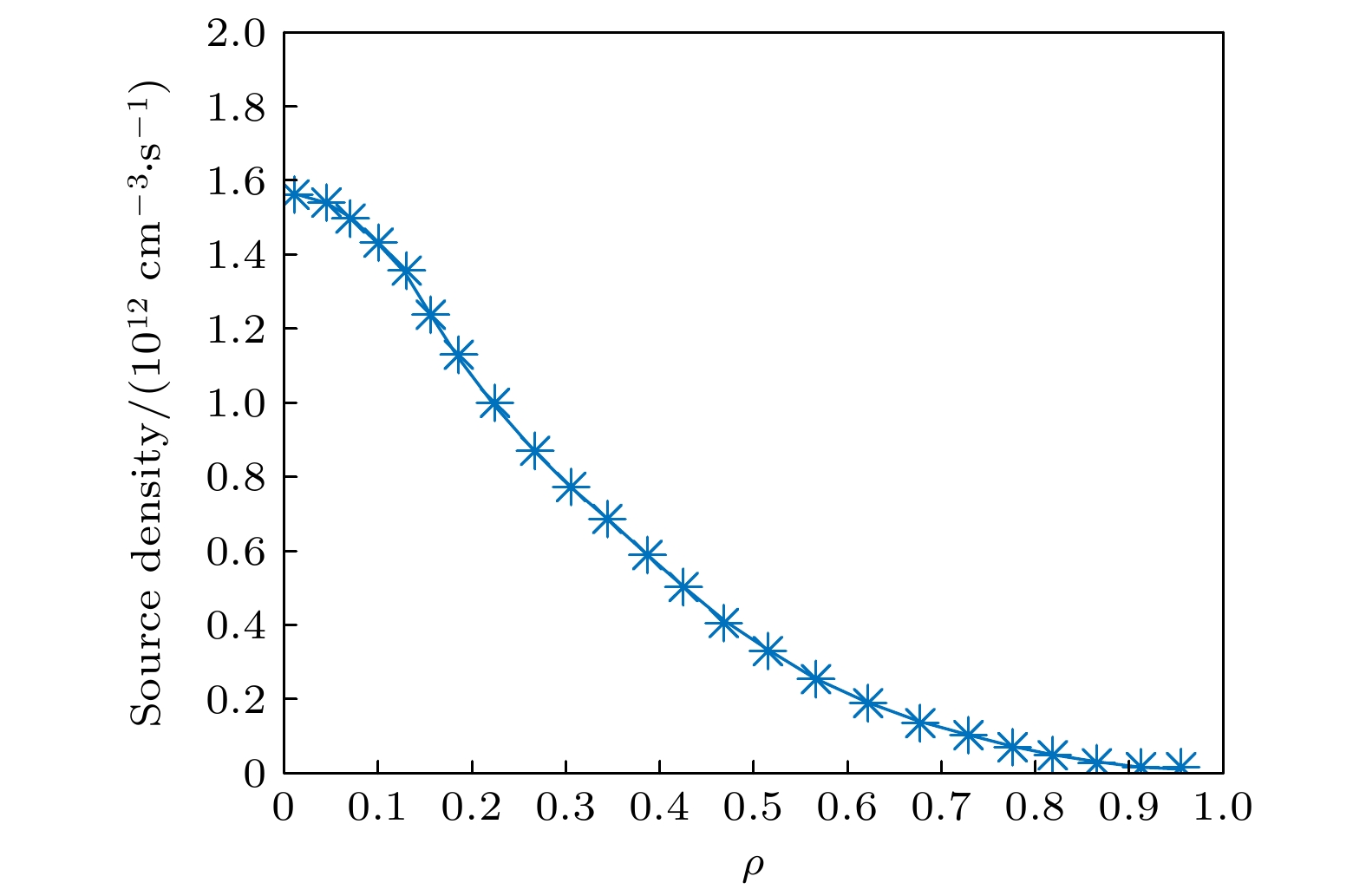
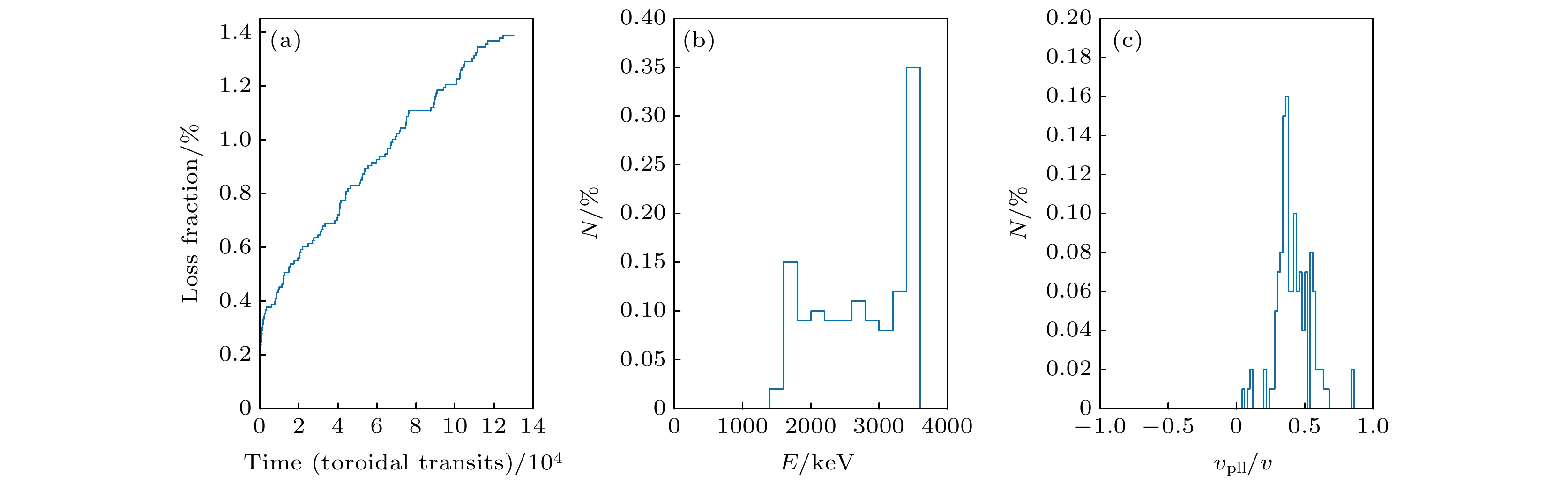
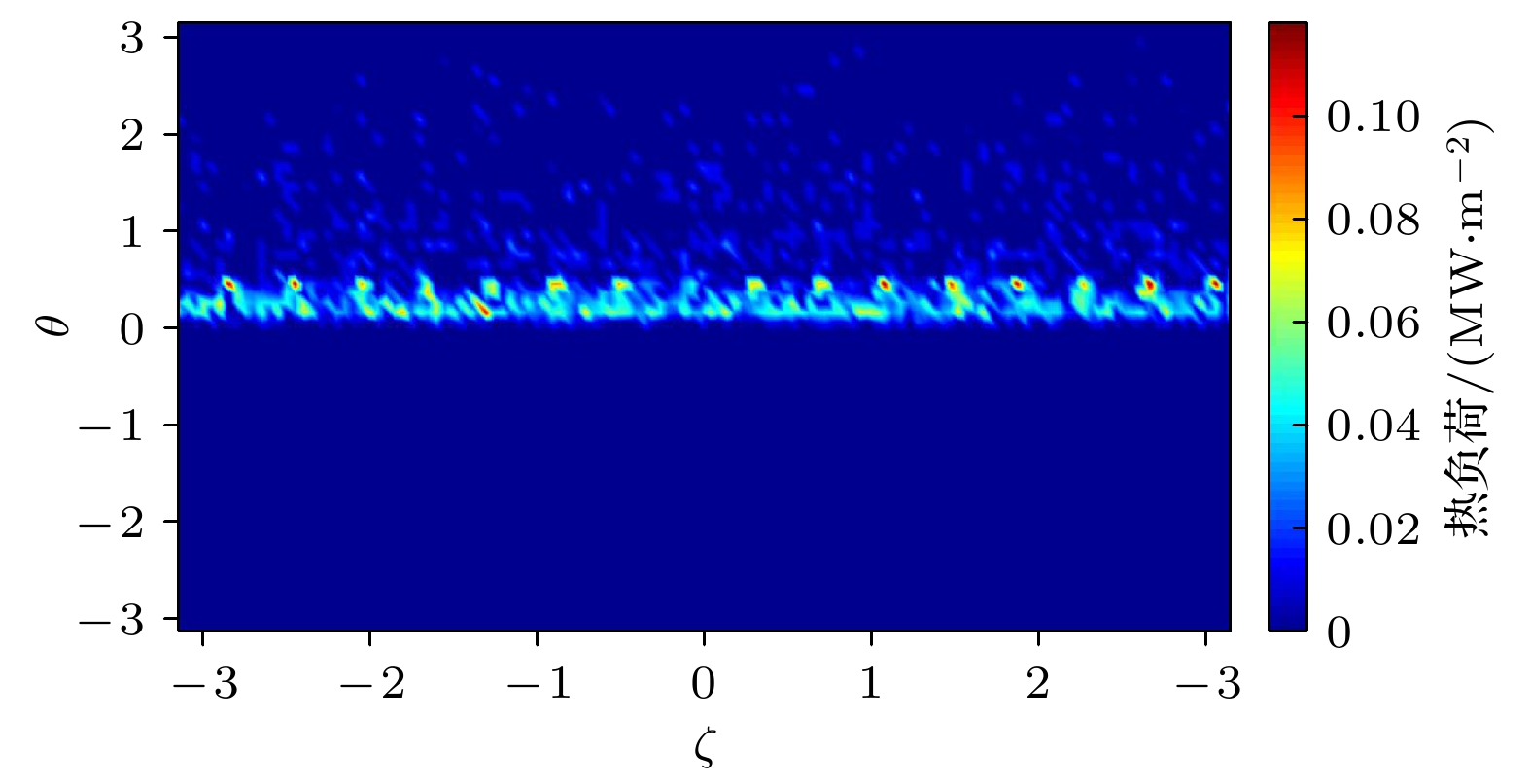
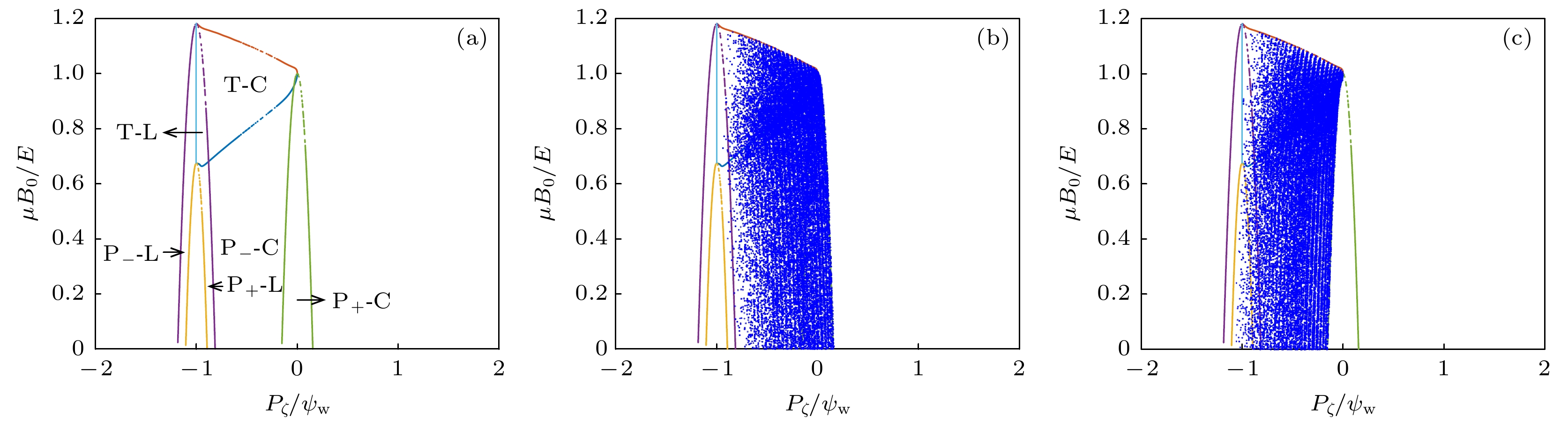
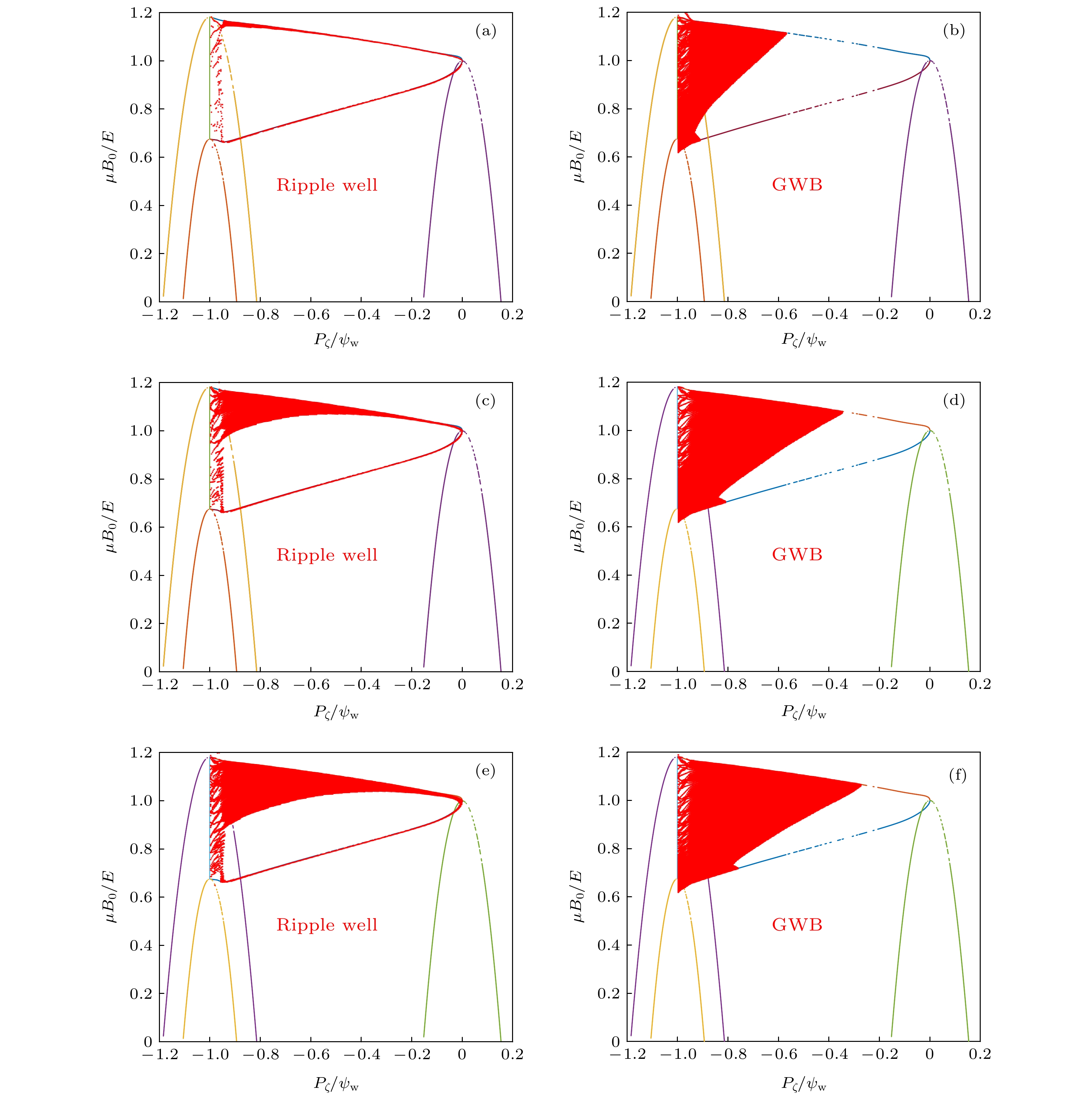
-
托卡马克聚变堆中高能量alpha粒子的良好约束是获得稳态燃烧等离子体的前提, 除了磁场波纹损失, 不稳定性也会引起额外的损失. 本文基于中国聚变工程试验堆(CFETR)参数, alpha粒子初始分布和新经典撕裂模(NTM)扰动分布, 利用粒子导心跟踪方法分别对磁场波纹和NTM两种扰动及叠加下的粒子损失进行了详细的数值模拟. 结果显示粒子损失份额不随NTM扰动幅度增大而增大, 两种扰动的叠加效应不明显. 通过扫描装置波纹度大小以及分析相空间粒子密度和波纹损失区分布, 确认原因是CFETR波纹损失区较小, 没有覆盖高能量粒子(EP)密度和NTM扰动主要分布区. 此外, NTM没有引起直接粒子损失和俘获粒子剖面坍塌. 显著的俘获粒子密度剖面展平, 并扩展到波纹损失区是两种扰动叠加效应显著的前提. 无碰撞波纹随机扩散是CFETR初始分布alpha粒子的主要损失通道, 通行粒子约束不受磁场波纹影响. 本文研究结果对CFETR概念设计中alpha粒子物理和低频不稳定性下的EP行为具有重要意义.Confinement of fusion born alpha particles in tokamak is the key issue to burning plasma. Apart from toroidal field ripple, instabilities can induce energetic particles to lose and be redistributed. Based on the parameters of China Fusion Engineering Testing Reactor (CFETT) hybrid scenario, alpha particle distribution and neoclassical tearing mode structure, the alpha particle loss induced under perturbation of ripple and neoclassical tearing mode (NTM) is calculated with the guiding center code ORBIT. The inputs have the initial distribution of alpha particles which is obtained with the TRANSP/NUBEAM code, the static NTM perturbation with different amplitudes which is obtained from TM1 code, and the ripple field from engineering design. The results show that the heat load on last closed flux surface is about 0.1 MW/m2, with ripple and collision included. The collisionless stochastic ripple diffusion is the main loss channel of initial alpha particle distribution in the CFETR, and the ripple perturbation has no influence on passing particles. The loss fraction does not increase with the NTM perturbation amplitude increasing, the synergistic effect is negligible. The scanning of ripple amplitude shows that the synergistic effect is slight. The monoenergetic initial distribution of alpha particles can give different types of orbits in the plane of (
$ {P_\zeta },\mu $ ), such as the domains of trapped particle and passing particle, lost particle and confined particle. The trapped fraction of initial alpha particles is about 27%, ripple loss region in phase space is narrow and away from the main trapped particle distribution. The increasing of ripple perturbation in simulation does enlarge the ripple loss domain in the phase space ($ {P_\zeta },\mu $ ), which is corresponding to a lager ripple loss fraction and has more trapped-passing boundaries. The NTM perturbation does enlarge the orbit excursions of trapped particles, and thus increasing the trapped passing transition near the boundary. The slight synergistic effect in calculation with larger ripple amplitude is explained by ripple loss region having more trapped-passing boundaries, not by the profile flattening of trapped particles. The NTM perturbation and finite collision can transit the passing particle to trapped particle near the boundary. With the help of kinetic Poincare plot, neither direct particle loss nor profile flattening of trapped particles is observed. The loss fraction enhancement can happen only when the profile flattening of trapped particles takes place within the ripple loss region, which is not the case in CFETR. The conclusion of this work contributes a lot to the design of CFETR and the study of alpha particle physics.-
Keywords:
- tokamak /
- alpha particle /
- magnetic ripple /
- neoclassical tearing mode
[1] Zhuang G, Li G Q, Li J, et al. 2019 Nucl. Fusion 59 112010
Google Scholar
[2] Wan Y X, Li J, Liu Y, et al. 2017 Nucl. Fusion 57 102009
Google Scholar
[3] Chen J, Jian X, Chan V, et al. 2017 Plasma Phys. Controlled Fusion 59 075005
Google Scholar
[4] Gorelenkov N N, Pinches S D, Toi K, et al. 2014 Nucl. Fusion 54 125001
Google Scholar
[5] Fasoli A, Gormenzano C, Berk H L, et al. 2007 Nucl. Fusion 47 S267
[6] Pinches S D, Chapman I T, Lauber Ph W, et al. 2015 Phys. Plasmas. 22 021807
Google Scholar
[7] White R B, Rutherford P H, Colestock P, et al. 1988 Phys. Rev. Lett. 60 2038
Google Scholar
[8] Chapman I T 2011 Plasma Phys. Controlled Fusion 53 013001
Google Scholar
[9] Igochine V 2015 Active Control of Magneto-hydrodynamic Instabilities in Hot Plasmas (Berlin: Springer) p259
[10] Poli E, Garcia-Munoz M, Fahrbach H, et al. 2008 Phys. Plasmas 15 032501
Google Scholar
[11] Gobbin M, Marrelli L, Fahrbach H, et al. 2009 Nucl. Fusion 49 095021
Google Scholar
[12] Strumberger E, Gunter S, Schwarz E, et al. 2008 New J. Phys. 10 023017
Google Scholar
[13] Garcia-Munoz M, Fahrbach H, Pinches S D, et al. 2009 Nucl. Fusion 49 085014
Google Scholar
[14] Garcia-Munoz M, Martin P, Fahrbach H, et al. 2007 Nucl. Fusion 47 L10
Google Scholar
[15] Mynick H E 1993 Phys. Fluids B 5 1471
Google Scholar
[16] 郝保龙, 陈伟, 蔡辉山, 等 2020 中国科学: 物理学 力学 天文学 50 065201
Google Scholar
Hao B L, Chen W, Cai H S, et al. 2020 Sci. Sin. Phys. Mech. Astron. 50 065201
Google Scholar
[17] White R B 2014 The theory of toroidally confined plasmas (3th Ed.) (Singapore: World scientific publishing company) p73
[18] Pankin A, McCune D, Andre R, et al. 2004 Computer Physics Communications 159 157
Google Scholar
[19] 高翔, 万宝年, 宋云涛, 等 2019 中国科学: 物理学 力学 天文学 49 045202
Google Scholar
Gao X, Wan B N, Song Y T, et al. 2019 Sci. Sin.-Phys. Mech. Astron. 49 045202
Google Scholar
[20] Yu Q, Gunter S, Scott B D 2003 Phys. Plasmas. 10 798
[21] Wang X J, Yu Q, Zhang X D, et al. 2018 Nucl. Fusion 58 016045
Google Scholar
[22] Hao B L, White R B, Gao X, et al. 2019 Nucl. Fusion 59 076040
Google Scholar
[23] Wu B, Hao B L, White R B, et al. 2017 Plasma Phys. Controlled Fusion 59 025004
Google Scholar
[24] Boozer A H, Kuo-Petravic G 1981 Phys. Fluids 24 851
Google Scholar
[25] Zhao R, Wang Z X, Wang F, et al. 2020 Plasma Phys. Controlled Fusion 62 115001
Google Scholar
[26] Carolipio E M, Heidbrink W W, Forest C B, et al. 2002 Nucl. Fusion 42 853
Google Scholar
-
图 1 CFETR混杂运行模式(v201806)中背景电子密度、温度和离子温度分布
Fig. 1. Distributions of bulk electron density, electron temperature, and bulk ion temperature in CFETR hybrid scenario (v201806).
图 2 CFETR初始alpha粒子密度分布
Fig. 2. Density profile of alpha particle initial distribution in CFETR.
图 3 CFETR纵场波纹度分布 (a) 解析函数实现值; (b) 工程设计值
Fig. 3. Distributions of toroidal field ripple perturbation amplitude in CFETR: (a) Ripple data by analytical equation; (b) engineering data in design.
图 4 CFETR混杂运行模式 (a) 2/1和3/2 NTM的平衡分量和安全因子分布; (b), (c)一阶分量和高斯拟合曲线
Fig. 4. (a) Safety factor profile and equilibrium helical flux of 2/1 and 3/2 NTM in CFETR hybrid scenario; (b), (c) the first order harmonics and Gaussian fitting results.
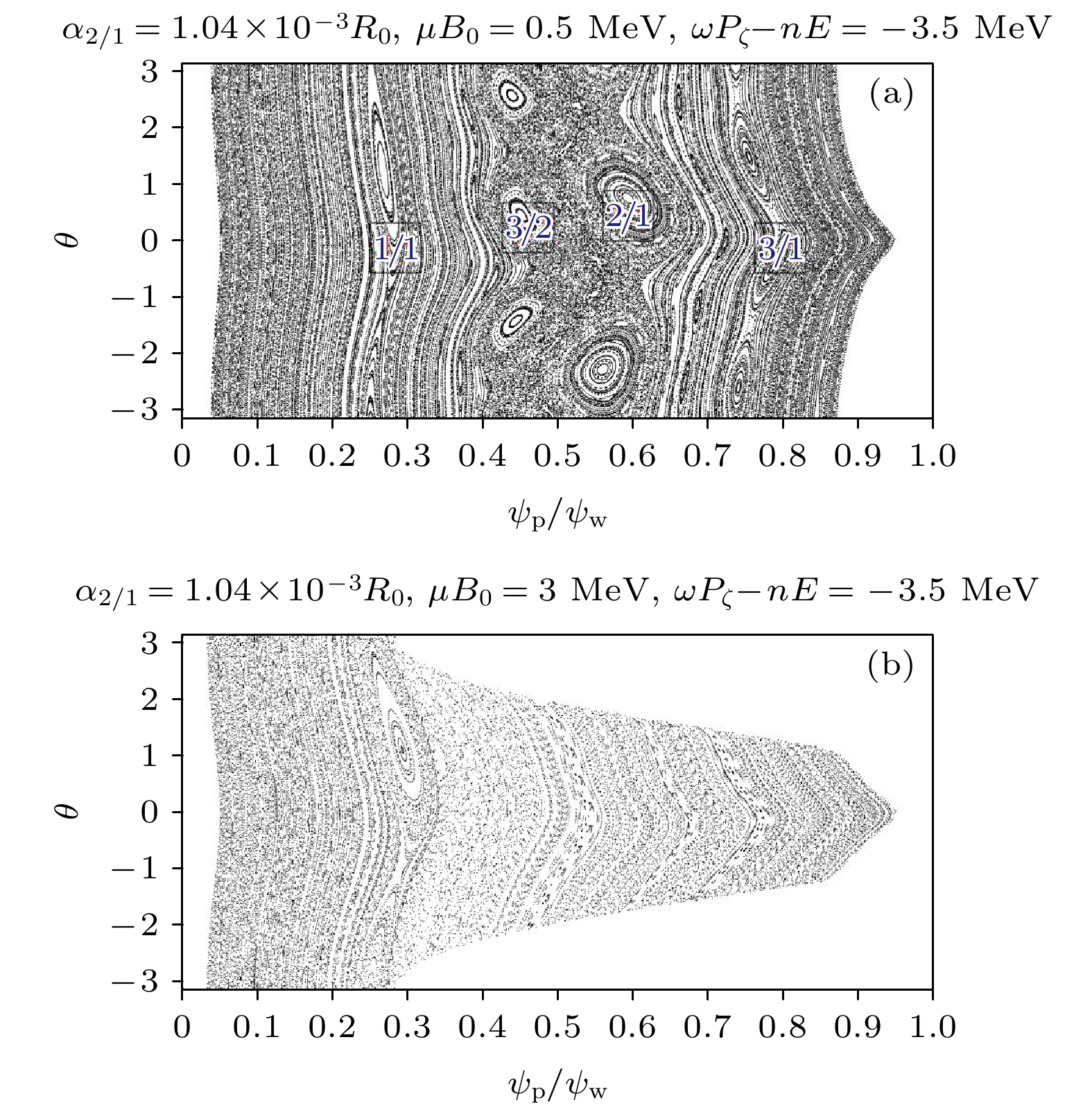
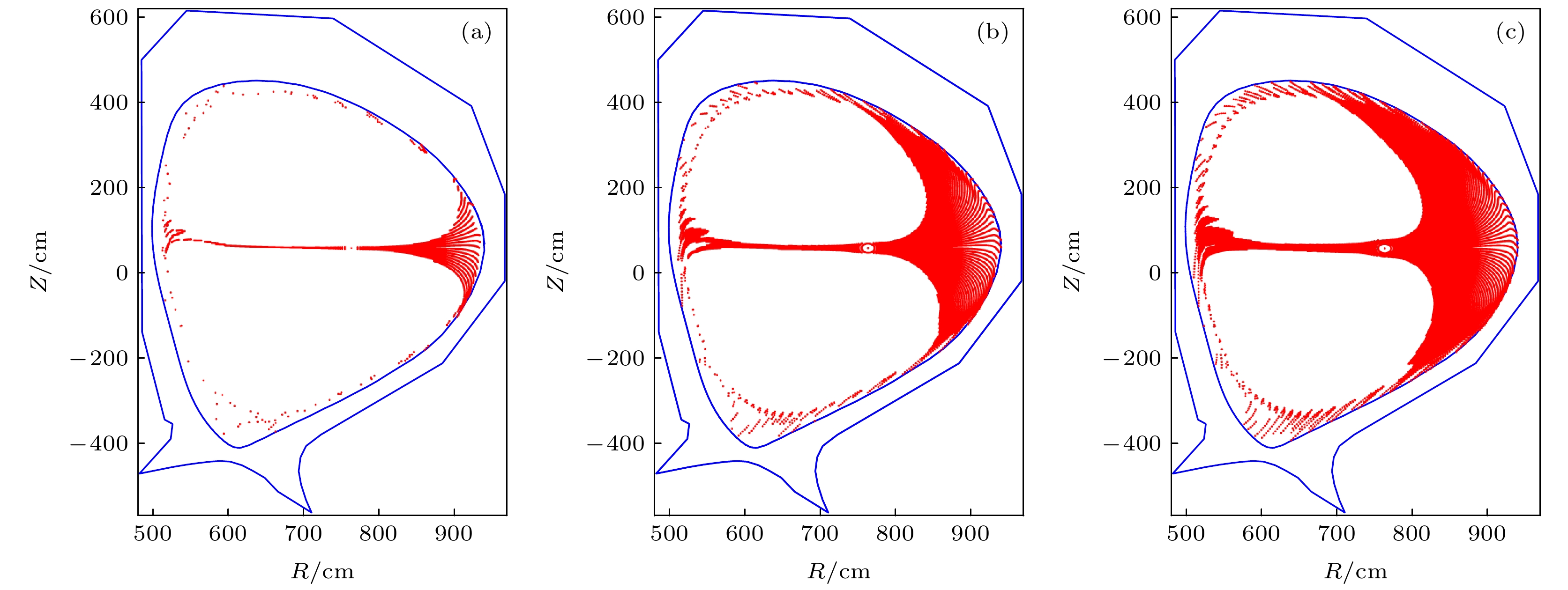
图 5 固定2/1和3/2模扰动幅度比例下扫描NTM扰动幅度下的庞加莱图 (a)
${\alpha _{2/1}} = 1.64 \times {10^{ - 4}}{R_0}$ ; (b)${\alpha _{2/1}} = l $ $ 6.00 \times {10^{ - 4}}{R_0}$ Fig. 5. Poincare plot under different NTM perturbation amplitude with the ratio fixed
${{{\alpha _{2/1}}} / {{\alpha _{3/2}}}} = 4.38$ : (a)${\alpha _{2/1}} = $ $ 1.64 \times {10^{ - 4}}{R_0}$ ; (b)${\alpha _{2/1}} = 6.00 \times {10^{ - 4}}{R_0}$ .
图 6 在半个EP慢化时间内不同物理效应下计算得到的粒子损失份额
Fig. 6. The particle loss fraction after following half a slowing down time under different perturbation and collision.
图 7 纵场波纹扰动下损失alpha粒子信息 (a) 损失份额随时演化; (b) 损失粒子能量分布; (c) 损失粒子螺距角分布
Fig. 7. Information of lost alpha particles under collision and toroidal field ripple: (a) Evolution of loss fraction; (b) energy distribution of lost particles; (c) pitch angle distribution of lost particles.
图 8 一个慢化时间后初始分布alpha粒子波纹损失局域沉积在LCFS处得到的热负荷
Fig. 8. The heat load at the last closed flux surface due to ripple loss of initial alpha particle distribution after a slowing down time.
图 14 初始分布alpha粒子香蕉轨道漂移频率分布 (a) 极向回弹频率; (b) 环向进动频率
Fig. 14. The drift frequency distributions of banana orbit of initial alpha particles: (a) Poloidal bounce frequency; (b) toroidal precession frequency.
图 9 同向alpha粒子动理学庞加莱图 (a) 通行粒子; (b) 俘获粒子
Fig. 9. The kinetic Poincare plot of co-current alpha particles: (a) Passing particle; (b) trapped particle.
图 10 半个慢化时间内不同磁场波纹度和NTM扰动幅度下的初始分布alpha粒子损失份额
Fig. 10. The loss fraction of initial alpha particles under different toroidal ripple and NTM perturbation amplitude.
图 11 CFETR平衡位形中不同波纹度形成的波纹磁阱 (a) 工程设计值; (b) 5倍波纹度分布; (c) 9倍波纹度分布
Fig. 11. The ripple well domain in CFETR equilibrium with different ripple amplitude: (a) Distribution with engineering design; (b) 5 times of ripple; (c) 9 times of ripple.
图 12 (a) (
${P_{\zeta} }, {{\mu {B_0}} / E}$ )平面初始alpha粒子轨道类型分布; (b) 正值螺距角粒子分布; (c) 负值螺距角粒子分布Fig. 12. (a) Orbit classification in the plane of
$({P_{\zeta} }, {{\mu {B_0}} / E})$ with initial alpha particles; (b) particles with positive pitch angle; (c) particles with negative pitch angle.
图 13 不同倍数波纹度分布时的波纹磁阱和随机波纹扩散区域 (a), (b) 工程设计值; (c), (d) 5倍波纹度分布; (e), (f) 9倍波纹度分布
Fig. 13. The ripple well trapping and stochastic ripple diffusion domain with different times of ripple distribution: (a), (b) Ripple in engineering design; (c), (d) 5 times of ripple; (e), (f) 9 times of ripple.
表 1 CFETR与其他托卡马克装置主机参数对比
Table 1. Main parameters comparison of CFETR and other tokomak facilities.
参数 CFETR ITER EAST 磁轴场强${B_{{\rm{T}}0}}$/T 6.5 5.3 2 等体大半径${R_0}$/m 7.2 6.2 1.9 等体小半径a/m 2.2 2.0 0.5 等体电流${I_{\rm{p}}}$/MA 14 15 1 纵场磁体柄数N 16 18 16 
下载: 导出CSV
-
[1] Zhuang G, Li G Q, Li J, et al. 2019 Nucl. Fusion 59 112010
Google Scholar
[2] Wan Y X, Li J, Liu Y, et al. 2017 Nucl. Fusion 57 102009
Google Scholar
[3] Chen J, Jian X, Chan V, et al. 2017 Plasma Phys. Controlled Fusion 59 075005
Google Scholar
[4] Gorelenkov N N, Pinches S D, Toi K, et al. 2014 Nucl. Fusion 54 125001
Google Scholar
[5] Fasoli A, Gormenzano C, Berk H L, et al. 2007 Nucl. Fusion 47 S267
[6] Pinches S D, Chapman I T, Lauber Ph W, et al. 2015 Phys. Plasmas. 22 021807
Google Scholar
[7] White R B, Rutherford P H, Colestock P, et al. 1988 Phys. Rev. Lett. 60 2038
Google Scholar
[8] Chapman I T 2011 Plasma Phys. Controlled Fusion 53 013001
Google Scholar
[9] Igochine V 2015 Active Control of Magneto-hydrodynamic Instabilities in Hot Plasmas (Berlin: Springer) p259
[10] Poli E, Garcia-Munoz M, Fahrbach H, et al. 2008 Phys. Plasmas 15 032501
Google Scholar
[11] Gobbin M, Marrelli L, Fahrbach H, et al. 2009 Nucl. Fusion 49 095021
Google Scholar
[12] Strumberger E, Gunter S, Schwarz E, et al. 2008 New J. Phys. 10 023017
Google Scholar
[13] Garcia-Munoz M, Fahrbach H, Pinches S D, et al. 2009 Nucl. Fusion 49 085014
Google Scholar
[14] Garcia-Munoz M, Martin P, Fahrbach H, et al. 2007 Nucl. Fusion 47 L10
Google Scholar
[15] Mynick H E 1993 Phys. Fluids B 5 1471
Google Scholar
[16] 郝保龙, 陈伟, 蔡辉山, 等 2020 中国科学: 物理学 力学 天文学 50 065201
Google Scholar
Hao B L, Chen W, Cai H S, et al. 2020 Sci. Sin. Phys. Mech. Astron. 50 065201
Google Scholar
[17] White R B 2014 The theory of toroidally confined plasmas (3th Ed.) (Singapore: World scientific publishing company) p73
[18] Pankin A, McCune D, Andre R, et al. 2004 Computer Physics Communications 159 157
Google Scholar
[19] 高翔, 万宝年, 宋云涛, 等 2019 中国科学: 物理学 力学 天文学 49 045202
Google Scholar
Gao X, Wan B N, Song Y T, et al. 2019 Sci. Sin.-Phys. Mech. Astron. 49 045202
Google Scholar
[20] Yu Q, Gunter S, Scott B D 2003 Phys. Plasmas. 10 798
[21] Wang X J, Yu Q, Zhang X D, et al. 2018 Nucl. Fusion 58 016045
Google Scholar
[22] Hao B L, White R B, Gao X, et al. 2019 Nucl. Fusion 59 076040
Google Scholar
[23] Wu B, Hao B L, White R B, et al. 2017 Plasma Phys. Controlled Fusion 59 025004
Google Scholar
[24] Boozer A H, Kuo-Petravic G 1981 Phys. Fluids 24 851
Google Scholar
[25] Zhao R, Wang Z X, Wang F, et al. 2020 Plasma Phys. Controlled Fusion 62 115001
Google Scholar
[26] Carolipio E M, Heidbrink W W, Forest C B, et al. 2002 Nucl. Fusion 42 853
Google Scholar
-
[1] 张启凡, 乐文成, 张羽昊, 葛忠昕, 邝志强, 萧声扬, 王璐. 钨杂质辐射对托卡马克等离子体大破裂快速热猝灭阶段热能损失过程的影响. , 2024, 73(18): 185201. doi: 10.7498/aps.73.20240730 [2] 王福琼, 徐颖峰, 查学军, 钟方川. 托卡马克边界等离子体中钨杂质输运的多流体及动力学模拟. , 2023, 72(21): 215213. doi: 10.7498/aps.72.20230991 [3] 刘冠男, 李新霞, 刘洪波, 孙爱萍. HL-2M托卡马克装置中螺旋波与低杂波的协同电流驱动. , 2023, 72(24): 245202. doi: 10.7498/aps.72.20231077 [4] 吴相凤, 王丰, 林展宏, 陈罗玉, 于召客, 吴凯邦, 王正汹. CFETR参数下 $\boldsymbol \alpha$ 粒子慢化过程的数值模拟. , 2023, 72(21): 215209. doi: 10.7498/aps.72.20230700[5] 沈勇, 董家齐, 何宏达, 潘卫, 郝广周. 托卡马克理想导体壁与磁流体不稳定性. , 2023, 72(3): 035203. doi: 10.7498/aps.72.20222043 [6] 郝保龙, 李颖颖, 陈伟, 郝广周, 顾翔, 孙恬恬, 王嵎民, 董家齐, 袁保山, 彭元凯, 石跃江, 谢华生, 刘敏胜, ENN TEAM. EXL-50U球形环中快离子磁场波纹损失的优化模拟研究. , 2023, 72(21): 215215. doi: 10.7498/aps.72.20230749 [7] 朱霄龙, 陈伟, 王丰, 王正汹. 托卡马克中低频磁流体不稳定性协同作用引起快粒子输运的混合模拟研究. , 2023, 72(21): 215210. doi: 10.7498/aps.72.20230620 [8] 刘朝阳, 章扬忠, 谢涛, 刘阿娣, 周楚. 托卡马克无碰撞捕获电子模在时空表象中的群速度. , 2021, 70(11): 115203. doi: 10.7498/aps.70.20202003 [9] 张重阳, 刘阿娣, 李弘, 陈志鹏, 李斌, 杨州军, 周楚, 谢锦林, 兰涛, 刘万东, 庄革, 俞昌旋. 双极化频率调制微波反射计在J-TEXT托卡马克上的应用. , 2014, 63(12): 125204. doi: 10.7498/aps.63.125204 [10] 杜海龙, 桑超峰, 王亮, 孙继忠, 刘少承, 汪惠乾, 张凌, 郭后扬, 王德真. 东方超环托卡马克高约束模式边界等离子体输运数值模拟研究. , 2013, 62(24): 245206. doi: 10.7498/aps.62.245206 [11] 卢洪伟, 查学军, 胡立群, 林士耀, 周瑞杰, 罗家融, 钟方川. HT-7托卡马克slide-away放电充气对等离子体行为的影响. , 2012, 61(7): 075202. doi: 10.7498/aps.61.075202 [12] 洪斌斌, 陈少永, 唐昌建, 张新军, 胡有俊. 托卡马克中电子回旋波与低杂波协同驱动的物理研究. , 2012, 61(11): 115207. doi: 10.7498/aps.61.115207 [13] 卢洪伟, 胡立群, 林士耀, 钟国强, 周瑞杰, 张继宗. HT-7托卡马克等离子体slide-away放电研究. , 2010, 59(8): 5596-5601. doi: 10.7498/aps.59.5596 [14] 徐强, 高翔, 单家方, 胡立群, 赵君煜. HT-7托卡马克大功率低混杂波电流驱动的实验研究. , 2009, 58(12): 8448-8453. doi: 10.7498/aps.58.8448 [15] 龚学余, 彭晓炜, 谢安平, 刘文艳. 托卡马克等离子体不同运行模式下的电子回旋波电流驱动. , 2006, 55(3): 1307-1314. doi: 10.7498/aps.55.1307 [16] 徐 伟, 万宝年, 谢纪康. HT-6M托卡马克装置杂质输运. , 2003, 52(8): 1970-1978. doi: 10.7498/aps.52.1970 [17] 王文浩, 俞昌旋, 许宇鸿, 闻一之, 凌必利, 宋梅, 万宝年. HT-7超导托卡马克边界等离子体参量及其涨落的实验研究. , 2001, 50(8): 1521-1527. doi: 10.7498/aps.50.1521 [18] 张先梅, 万宝年, 阮怀林, 吴振伟. HT-7托卡马克等离子体欧姆放电时电子热扩散系数的研究. , 2001, 50(4): 715-720. doi: 10.7498/aps.50.715 [19] 王文浩, 许宇鸿, 俞昌旋, 闻一之, 凌必利, 宋梅, 万宝年. HT-7超导托卡马克边缘涨落谱特征及湍流输运研究. , 2001, 50(10): 1956-1963. doi: 10.7498/aps.50.1956 [20] 石秉仁. 托卡马克低混杂波电流驱动实验中低混杂波传播的解析分析. , 2000, 49(12): 2394-2398. doi: 10.7498/aps.49.2394
下载:
计量
- 文章访问数: 5091
- PDF下载量: 72
- 被引次数: 0
