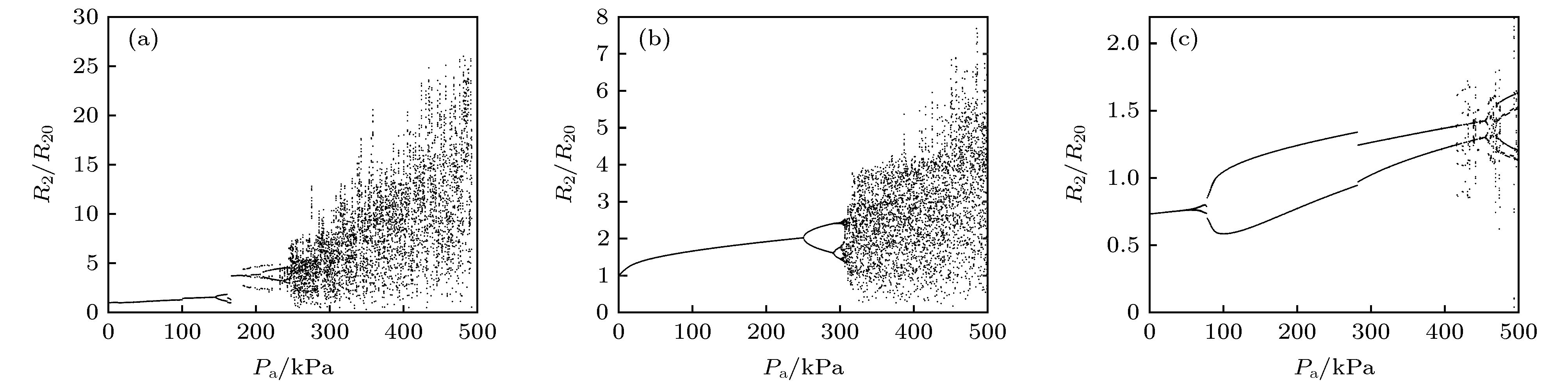
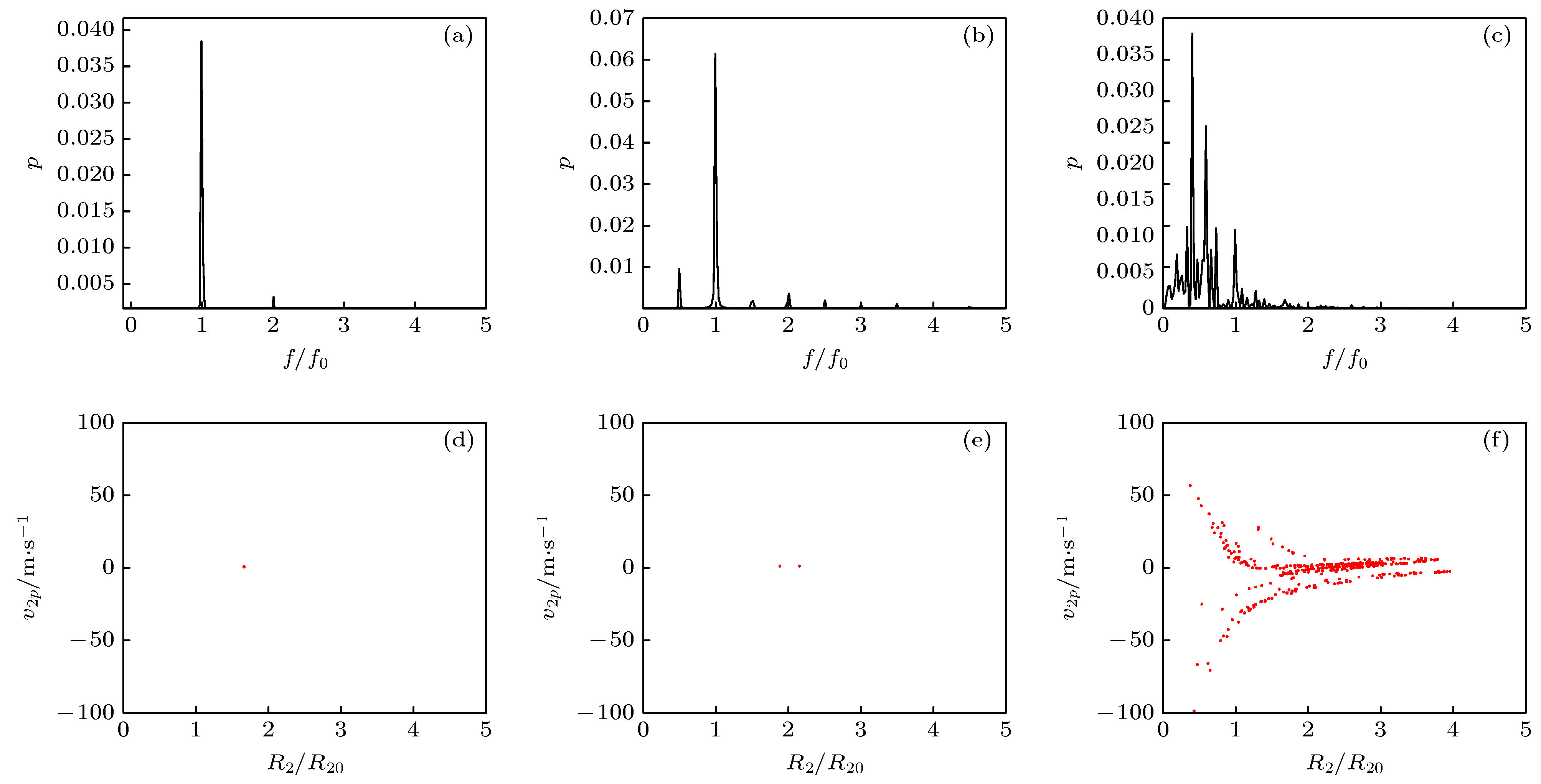
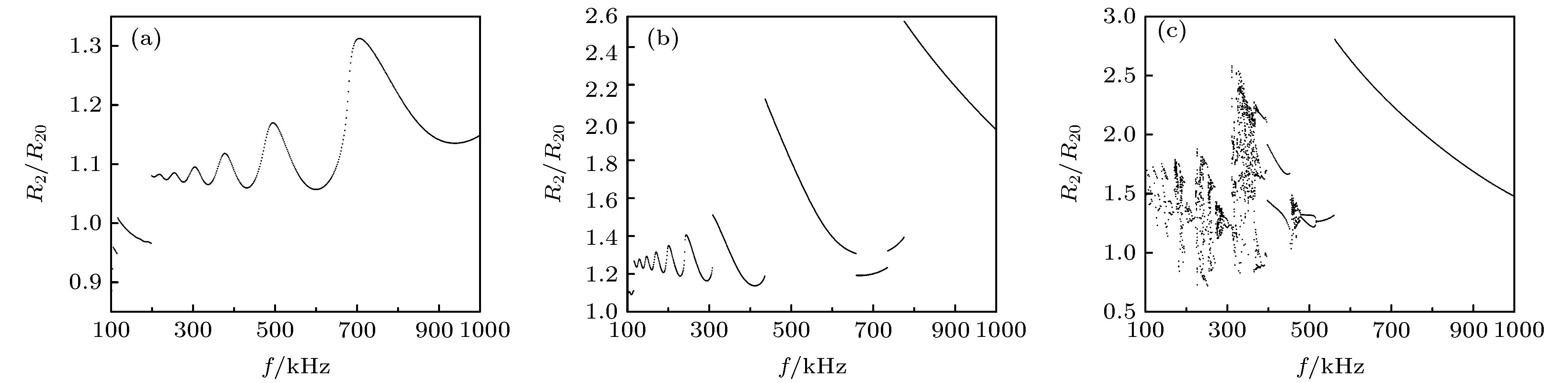
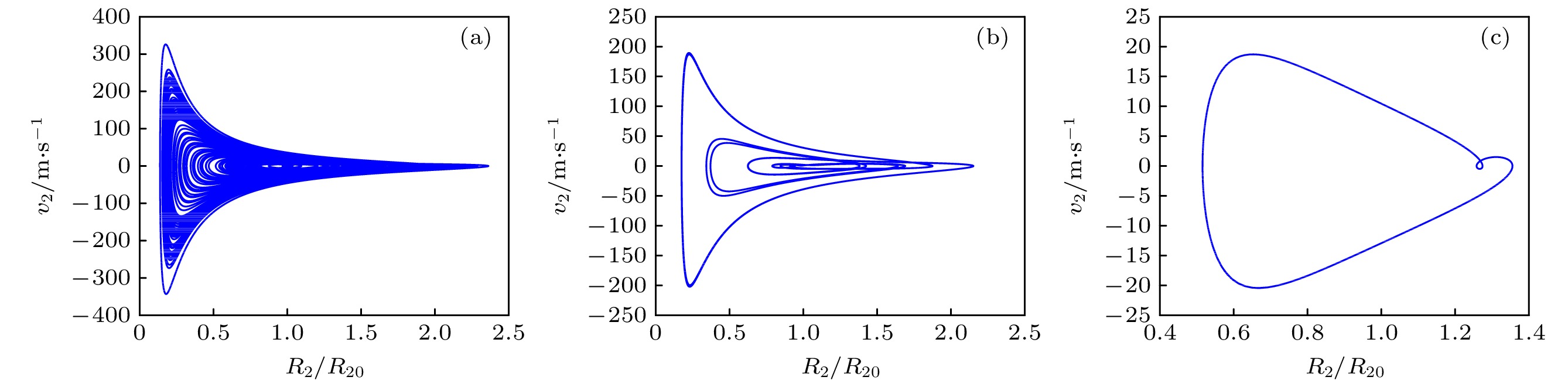
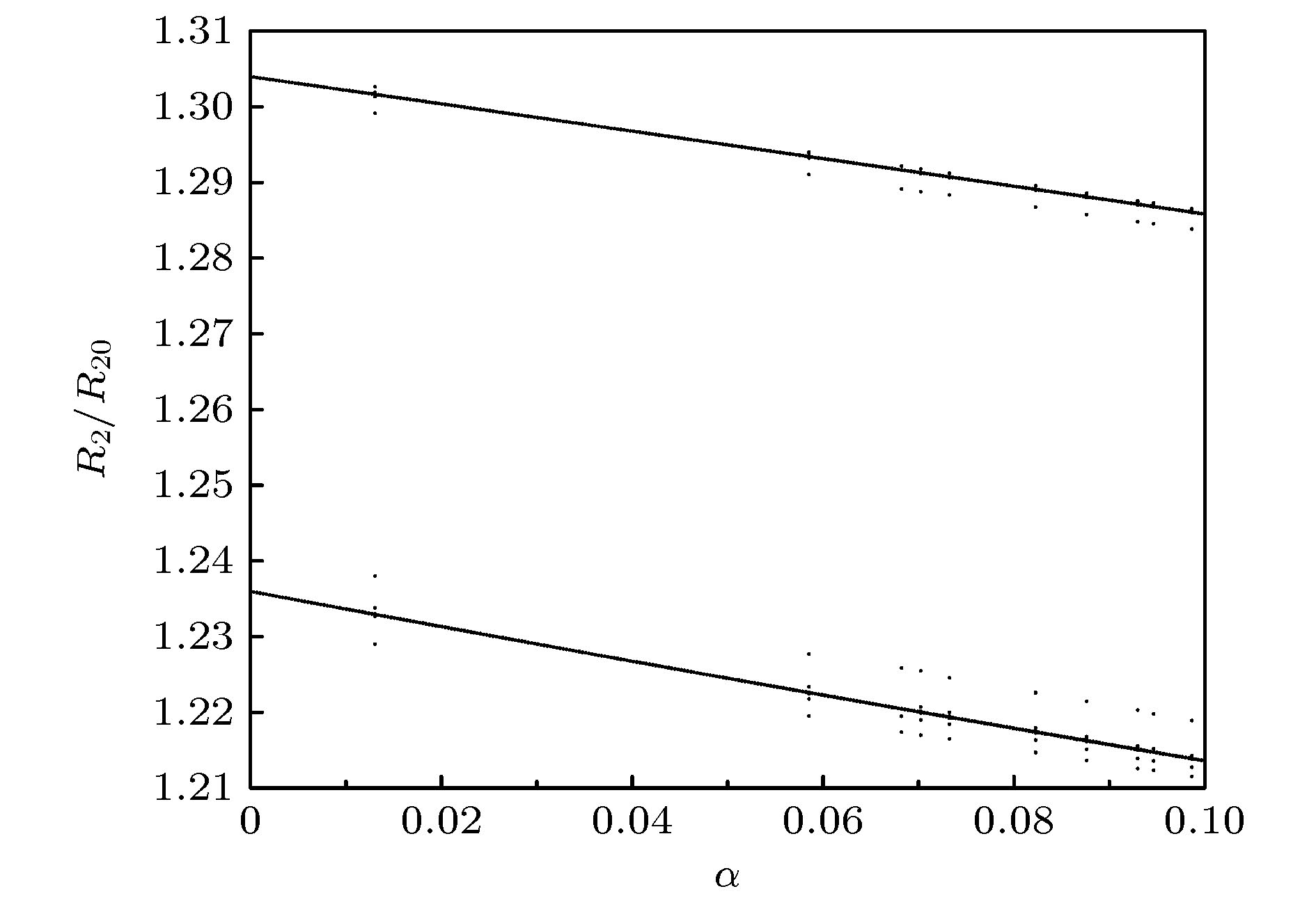
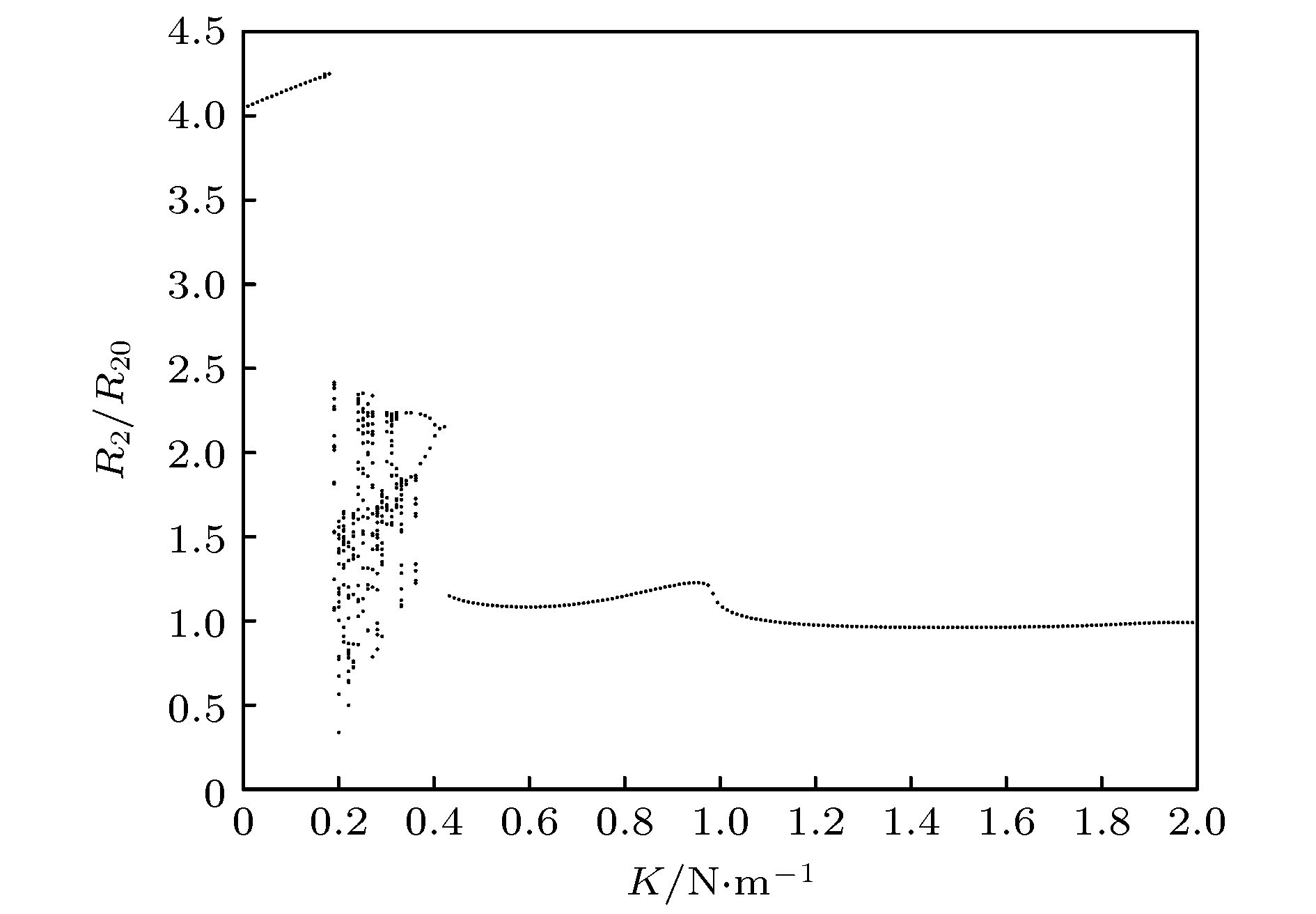
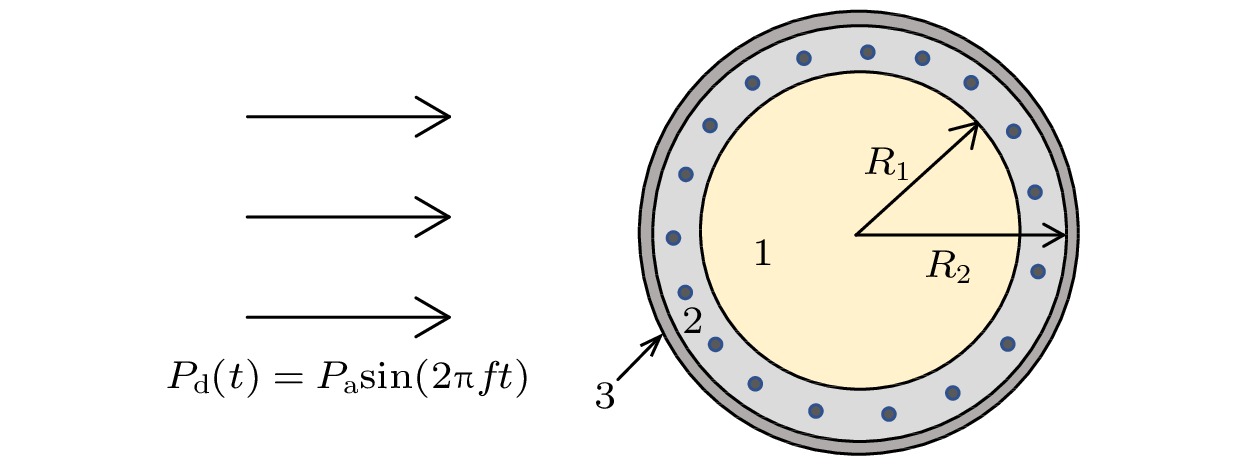
-
超顺磁性氧化铁纳米粒子与造影剂微泡结合形成磁性微泡, 用于产生多模态造影剂, 以增强医学超声和磁共振成像. 将装载有纳米磁性颗粒的微泡包膜层看作由磁流体膜与磷脂膜组合而成的双层膜结构, 同时考虑磁性纳米颗粒体积分数α对膜密度及黏度的影响, 从气泡动力学基本理论出发, 构建多层膜结构磁性微泡非线性动力学方程. 数值分析了驱动声压和频率等声场参数、颗粒体积分数、膜层厚度以及表面张力等膜壳参数对微泡声动力学行为的影响. 结果表明, 当磁性颗粒体积分数较小且α ≤ 0.1时, 磁性微泡声响应特性与普通包膜微泡相似, 微泡的声频响应与其初始尺寸和驱动压有关; 当驱动声场频率f为磁性微泡共振频率f0的2倍(f = 2f0)时, 微泡振动失稳临界声压最低; 磁性颗粒的存在抑制了泡的膨胀和收缩但抑制效果非常有限; 磁性微泡外膜层材料的表面张力参数K及膜层厚度d也会影响微泡的振动, 当表面张力参数及膜厚取值分别为0.2—0.4 N/m及50—150 nm时, 可观察到气泡存在不稳定振动响应区.The combination of superparamagnetic iron oxide nanoparticles (SPIOs) with ultrasonic contrast agent (UCA) microbubble is called magnetic microbubble (MMB) and has been used to produce multimodal contrast agents to enhance medical ultrasound and magnetic resonance imaging. The nanoparticles are either covalently linked to the shell or physically entrapped into the shell. Considering the effect of the volume fraction of SPIOs on the shell density and viscosity, a nonlinear dynamic equation of magnetic microbubbles (MMBs) with multilayer membrane structure is constructed based on the basic theory of bubble dynamics. The influences of the driving sound pressure and frequency, particle volume fraction, shell thickness and surface tension on the acoustic-dynamics behavior of microbubbles are numerically analyzed. The results show that when the volume fraction of magnetic particles is small and α ≤ 0.1, the acoustic properties of magnetic microbubbles are similar to those of ordinary UCA microbubbles. The acoustic response of the microbubble depends on its initial size and driving pressure. The critical sound pressure of microbubble vibration instability is lowest when the driving sound field frequency is twice the magnetic microbubble resonance frequency f0 (f = 2f0). The presence of magnetic particles inhibits the bubbles from expanding and contracting, but the inhibition effect is very limited. The surface tension parameter K of the outer film material and thickness of the shell also affect the vibration of the microbubble. When K and film thickness are 0.2–0.4 N/m and 50–150 nm respectively, it is observed that the bubble has an unstable vibration response region.
-
Keywords:
- magnetic microbubbles /
- nonlinear vibration /
- power spectrum /
- bifurcation diagram
[1] He W, Yang F, Wu Y H, Wen S, Chen P, Zhang Y, Gu N 2012 Mater. Lett. 68 64
Google Scholar
[2] Porter T R, Feinstein S B, Ten Cate F J, Van den Bosch A E 2020 Ultrasound Med. Biol. 46 1071
Google Scholar
[3] Stride E, Porter C, Prieto A G, Pankhurs Q 2009 Ultrasound Med. Biol. 35 861
Google Scholar
[4] Dimcevski G, Kotopoulis S, Bjnes T, Hoem D 2016 J. Controlled Release 243 172
Google Scholar
[5] Duan L, Yang F, Song L N, Fang K,Tian J L, Liang Y J, Li M X, Xu N, Chen Z D, Zhang Y, Gu N 2015 Soft Matter 11 5492
Google Scholar
[6] Cho E, Chung S K, Rhee K 2015 Ultrasonics 62 66
Google Scholar
[7] Hyun S M, Sejin S, Dong G Y, Tae W L, Jangwook L, Sangmin L, Ji Y Y, Jaeyoung L, Moon H H, Jae H P, Sun H K, Kuiwon C, Kinam P, Kwangmeyung K, Ick C K 2016 Biomaterials 108 57
Google Scholar
[8] Gao Y, Chan C U, Gu Q S, Lin X D, Zhang W C, David Yeo C L, Astrid M A, Manish A, Mark Chong S K, Shi P, Claus D O and Xu C J 2016 NPG. Asia Mater. 8 e260
Google Scholar
[9] Zhou T, Cai W B, Yang H L, Zhang H Z, Hao M H, Yuan L J, Liu J, Zhang L, Yang Y L, Liu X, Deng J L, Zhao P, Yang G D, Duan Y Y 2018 J. Controlled Release 276 113
Google Scholar
[10] Sciallero C, Grishenkov D, Kothapalli S V, Oddo L, Trucco A 2013 J. Acoust. Soc. Am. 134 3918
Google Scholar
[11] Gu Y Y, Chen C Y, Tu J, Guo X S, Wu H Y, Zhang D 2016 Ultrason. Sonochem. 29 309
Google Scholar
[12] Marlies O, Valeria G, Jeroen S, Benjamin D, Nico D J, Detlef L, Michel V 2010 Ultrasound Med. Biol. 36 2080
Google Scholar
[13] Mulvana H, Eckersley R J, Tang M X, Pankhurst Q, Stride E 2012 Ultrasound Med. Biol. 38 864
Google Scholar
[14] Behnia S, Mobadersani F, Yahyavi M, Rezavand A 2013 Nonlinear Dyn. 74 559
Google Scholar
[15] Hongray T, Ashok B, Balakrishnan J 2015 Pramana 84 517
Google Scholar
[16] Shi J, Yang D S, Shi S G, Hu B, Zhang H Y, Hu S Y 2016 Chin. Phys. B 25 024304
Google Scholar
[17] Church C C 1995 J. Acoust. Soc. Am. 97 1510
Google Scholar
[18] Beguin E, Bau L, Shrivastava S, Stride E 2019 ACS Appl. Mater. Interfaces 11 1829
Google Scholar
[19] Malvar S, Gontijo R G, Cunha F R 2018 J. Eng. Math. 108 143
Google Scholar
[20] 莫润阳, 吴临燕, 詹思楠, 张引红 2015 64 124301
Google Scholar
Mo R Y, Wu L Y, Zhan S N, Zhang Y H 2015 Acta Phys. Sin. 64 124301
Google Scholar
[21] Zhang D, Guo G P, Lu L, Yin L L, Tu J, Guo X S, Xu D, Wu J R 2014 Phys. Med. Biol. 59 6729
Google Scholar
[22] Hosseini S M, Ghasemi E, Fazlali A, Henneke E 2012 J. Nanopart. Res. 14 858
Google Scholar
[23] 陈伟中 2014 声空化物理 (北京 科学出版社) 第415−417页
Chen W Z 2014 Acoustic Cavitation Physics (Beijing: Science Press) pp415−417 (in Chinese)
[24] Doinikov A, Dayton P A 2007 J. Acoust. Soc. Am. 121 3331
Google Scholar
[25] Sijl J, Dollet B, Overvelde M, Garbinet V, Rozendal T, De Jong N, Lohse De, Versluis M 2010 J. Acoust. Soc. Am. 128 3239
Google Scholar
[26] 杨芳, 李熠鑫, 陈忠平, 顾宁 2009 科学通报 54 1181
Yang F, Li Y X, Chen Z P, Gu N 2009 Chin. Sci. Bull. 54 1181
[27] 沈壮志, 林书玉 2011 60 104302
Google Scholar
Shen Z Z, Lin S Y 2011 Acta Phys. Sin. 60 104302
Google Scholar
-
图 1 磁性微泡几何模型. 1-空气, 2-磁流体层, 3-磷脂薄层
Fig. 1. The geometric model of MMBs. 1-air, 2-magnetic fluid, 3-thin layer of phospholipid regardless of thickness.
图 2 声压响应分岔图 (R20 = 5 μm) (a) f = f0/2; (b) f = f0; (c) f = 2f0
Fig. 2. Bifurcation diagram of an MMB with pressure amplitude for R20 =5 μm: (a) f = f0/2; (b) f = f0; (c) f = 2f0.
图 3 基频激励时MMB频谱图 ((a)—(c))和庞加莱截面图((d)—(f)) (R20 = 5 μm) (a), (d) Pa = 100 kPa; (b), (e) Pa = 255 kPa; (c), (f) Pa = 350 kPa
Fig. 3. Spectra diagram and Poincaré cross-section of an MMB at f = f0 (R20 = 5 μm): (a), (d) Pa = 100 kPa; (b), (e) Pa =255 kPa; (c), (f) Pa = 350 kPa
图 4 不同尺寸MMB频响曲线(Pa = 150 kPa) (a) R20 = 3 μm; (b) R20 = 4 μm; (c) R20 = 5 μm
Fig. 4. Bifurcation diagrams of an MMB with driving frequency as the control parameter at Pa = 150 kPa: (a) R20 = 3 μm; (b) R20 = 4 μm; (c) R20 = 5 μm.
图 5 混沌态微泡振动相图 (Pa = 150 kPa, R20 = 5 μm) (a) f = 350 kHz; (b) f = 381 kHz; (c) f = 550 kHz
Fig. 5. MMB phase diagram in chaotic motion (Pa = 150 kPa, R20 = 5 μm): (a) f = 350 kHz; (b) f = 381 kHz; (c) f = 550 kHz.
-
[1] He W, Yang F, Wu Y H, Wen S, Chen P, Zhang Y, Gu N 2012 Mater. Lett. 68 64
Google Scholar
[2] Porter T R, Feinstein S B, Ten Cate F J, Van den Bosch A E 2020 Ultrasound Med. Biol. 46 1071
Google Scholar
[3] Stride E, Porter C, Prieto A G, Pankhurs Q 2009 Ultrasound Med. Biol. 35 861
Google Scholar
[4] Dimcevski G, Kotopoulis S, Bjnes T, Hoem D 2016 J. Controlled Release 243 172
Google Scholar
[5] Duan L, Yang F, Song L N, Fang K,Tian J L, Liang Y J, Li M X, Xu N, Chen Z D, Zhang Y, Gu N 2015 Soft Matter 11 5492
Google Scholar
[6] Cho E, Chung S K, Rhee K 2015 Ultrasonics 62 66
Google Scholar
[7] Hyun S M, Sejin S, Dong G Y, Tae W L, Jangwook L, Sangmin L, Ji Y Y, Jaeyoung L, Moon H H, Jae H P, Sun H K, Kuiwon C, Kinam P, Kwangmeyung K, Ick C K 2016 Biomaterials 108 57
Google Scholar
[8] Gao Y, Chan C U, Gu Q S, Lin X D, Zhang W C, David Yeo C L, Astrid M A, Manish A, Mark Chong S K, Shi P, Claus D O and Xu C J 2016 NPG. Asia Mater. 8 e260
Google Scholar
[9] Zhou T, Cai W B, Yang H L, Zhang H Z, Hao M H, Yuan L J, Liu J, Zhang L, Yang Y L, Liu X, Deng J L, Zhao P, Yang G D, Duan Y Y 2018 J. Controlled Release 276 113
Google Scholar
[10] Sciallero C, Grishenkov D, Kothapalli S V, Oddo L, Trucco A 2013 J. Acoust. Soc. Am. 134 3918
Google Scholar
[11] Gu Y Y, Chen C Y, Tu J, Guo X S, Wu H Y, Zhang D 2016 Ultrason. Sonochem. 29 309
Google Scholar
[12] Marlies O, Valeria G, Jeroen S, Benjamin D, Nico D J, Detlef L, Michel V 2010 Ultrasound Med. Biol. 36 2080
Google Scholar
[13] Mulvana H, Eckersley R J, Tang M X, Pankhurst Q, Stride E 2012 Ultrasound Med. Biol. 38 864
Google Scholar
[14] Behnia S, Mobadersani F, Yahyavi M, Rezavand A 2013 Nonlinear Dyn. 74 559
Google Scholar
[15] Hongray T, Ashok B, Balakrishnan J 2015 Pramana 84 517
Google Scholar
[16] Shi J, Yang D S, Shi S G, Hu B, Zhang H Y, Hu S Y 2016 Chin. Phys. B 25 024304
Google Scholar
[17] Church C C 1995 J. Acoust. Soc. Am. 97 1510
Google Scholar
[18] Beguin E, Bau L, Shrivastava S, Stride E 2019 ACS Appl. Mater. Interfaces 11 1829
Google Scholar
[19] Malvar S, Gontijo R G, Cunha F R 2018 J. Eng. Math. 108 143
Google Scholar
[20] 莫润阳, 吴临燕, 詹思楠, 张引红 2015 64 124301
Google Scholar
Mo R Y, Wu L Y, Zhan S N, Zhang Y H 2015 Acta Phys. Sin. 64 124301
Google Scholar
[21] Zhang D, Guo G P, Lu L, Yin L L, Tu J, Guo X S, Xu D, Wu J R 2014 Phys. Med. Biol. 59 6729
Google Scholar
[22] Hosseini S M, Ghasemi E, Fazlali A, Henneke E 2012 J. Nanopart. Res. 14 858
Google Scholar
[23] 陈伟中 2014 声空化物理 (北京 科学出版社) 第415−417页
Chen W Z 2014 Acoustic Cavitation Physics (Beijing: Science Press) pp415−417 (in Chinese)
[24] Doinikov A, Dayton P A 2007 J. Acoust. Soc. Am. 121 3331
Google Scholar
[25] Sijl J, Dollet B, Overvelde M, Garbinet V, Rozendal T, De Jong N, Lohse De, Versluis M 2010 J. Acoust. Soc. Am. 128 3239
Google Scholar
[26] 杨芳, 李熠鑫, 陈忠平, 顾宁 2009 科学通报 54 1181
Yang F, Li Y X, Chen Z P, Gu N 2009 Chin. Sci. Bull. 54 1181
[27] 沈壮志, 林书玉 2011 60 104302
Google Scholar
Shen Z Z, Lin S Y 2011 Acta Phys. Sin. 60 104302
Google Scholar
下载:
计量
- 文章访问数: 5090
- PDF下载量: 69
- 被引次数: 0
