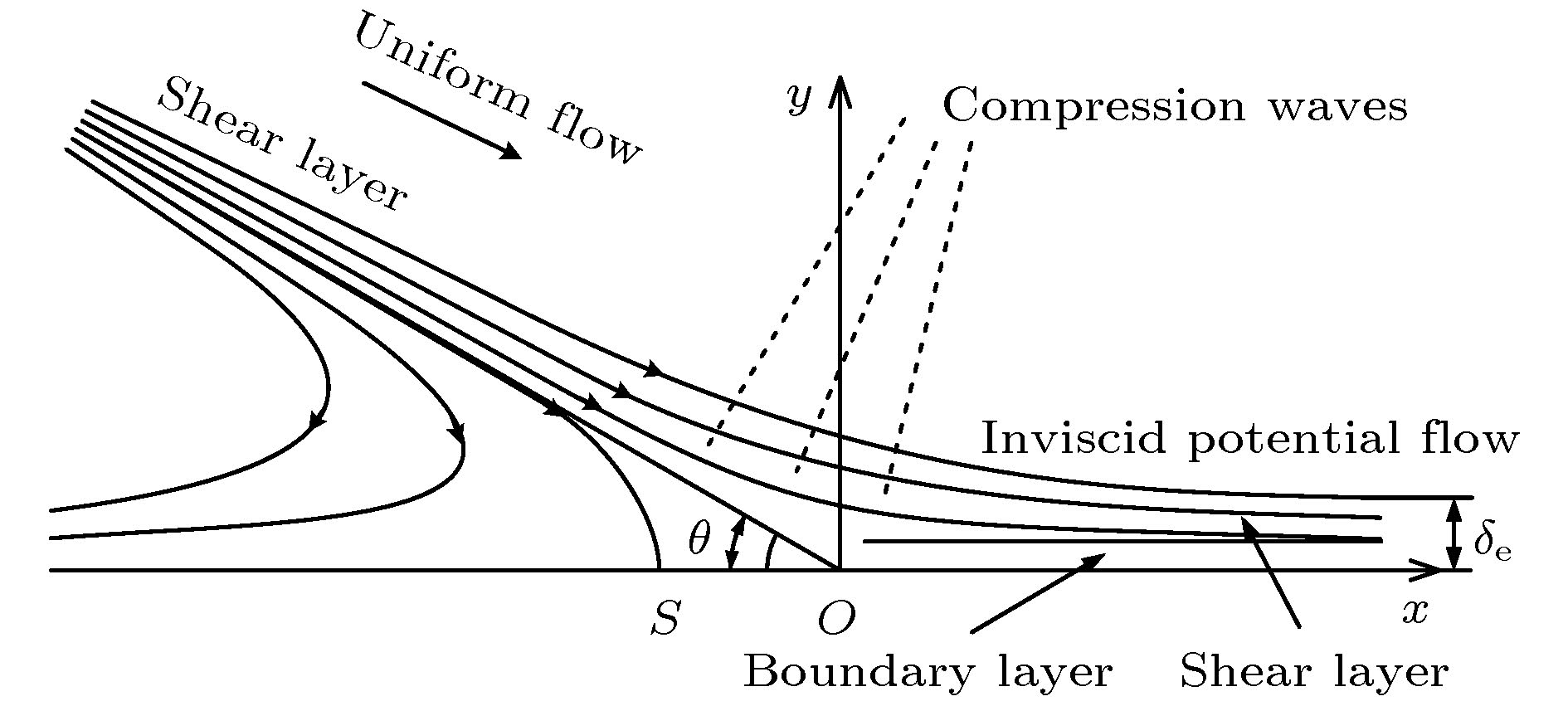
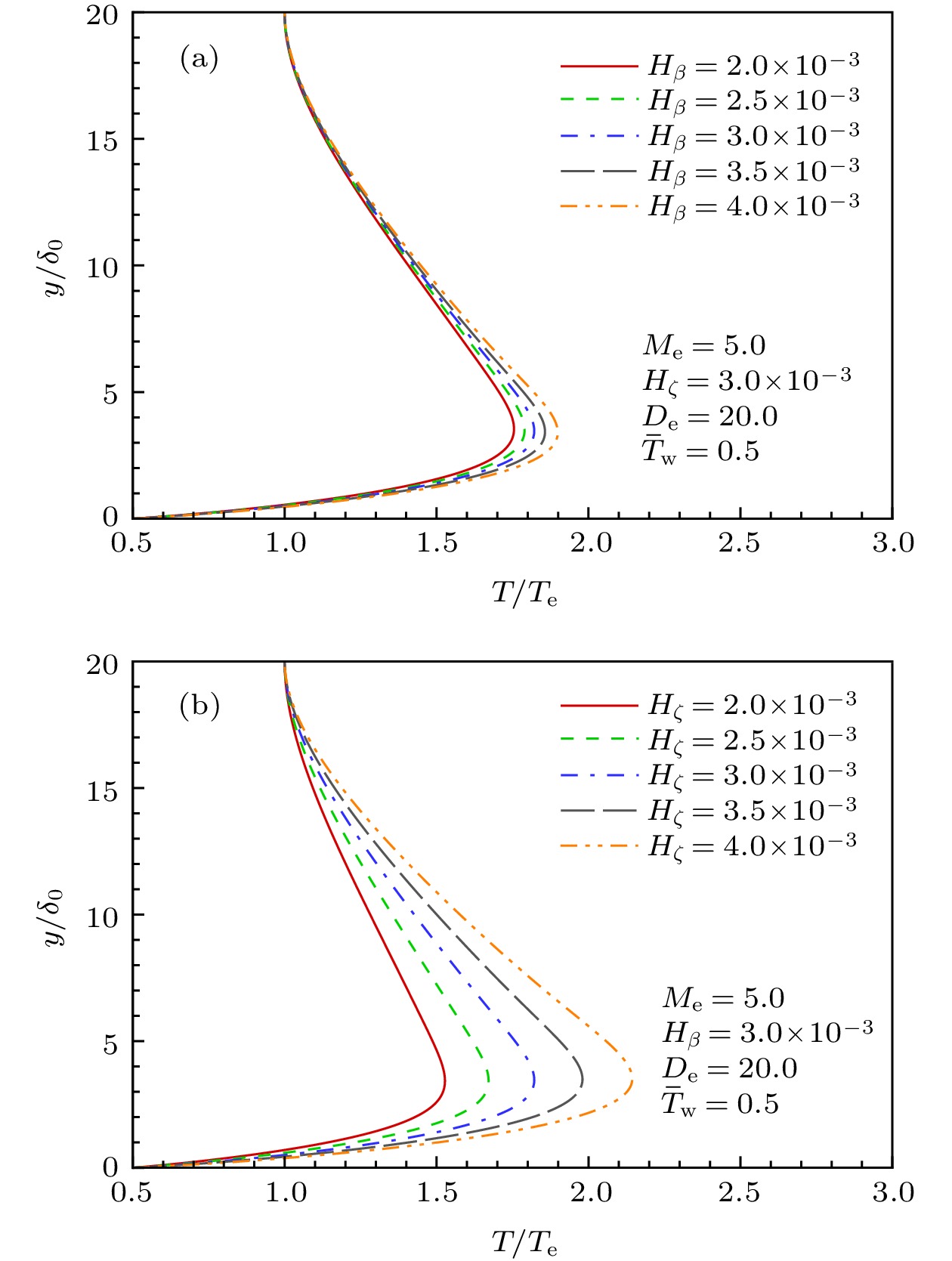
-
高速流体以一定角度冲击壁面可以简化为斜驻点流动, 包括正驻点流动分量和剪切流动分量. 以往研究集中在不可压缩的斜驻点流动, 本文针对高速可压缩的斜驻点流动模型, 给出了新的自相似求解方案. 通过与数值结果对比验证, 发现该模型能很好地模拟高超声速再附产生的均匀剪切层撞击壁面流动. 通过对比流动中能量输运项和做功项的贡献, 发现斜驻点流动的剪切分量带来较强的压缩效应和耗散效应, 造成流动具有显著的流向对流传热, 表现为再附后近壁流体温度迅速升高, 最终产生壁面上的高热流值. 参数分析表明壁面热流系数与无量纲壁面温度梯度和边界层厚度相关, 前者主要受斜驻点剪切分量参数控制, 后者与驻点分量参数呈负相关.Hypersonic reattachment flow usually causes extremely high wall heat flux, which is of great concern in engineering. The flow can be modeled as a high-speed shear layer impinging on a wall at a certain angle, which forms an oblique stagnation-point flow in the vicinity of the stagnation point, including stagnation-component and shear-component. Previous work focused on incompressible oblique stagnation-point flow, while in the present study a semi-analytical-semi-numeric solution is given via the self-similar method for compressible oblique stagnation-point flow. Through the comparison and validation with the numerical results, it is found that the flow model can well simulate the heat transfer near the wall in the flow of uniform shear layer impinging on the wall caused by hypersonic reattachment. With the analysis of the contribution of the energy transport and the heat production in the flow model, it is found that the compression effect and dissipation effect are mainly caused by the shear-component, leading to a significant convective heat transfer in the flow direction, which is different from the case of the classic boundary layer. Then the temperature of the flow near the wall rises rapidly after reattachment, resulting in a high heat flux to the wall. Parameter analysis indicates that the wall heat-transfer coefficient is related to the dimensionless wall temperature gradient and the thickness of the boundary layer. The former is mainly controlled by the shear-component parameter
$H_{\zeta}$ , and the latter is negatively correlated with the stagnation-component parameter$H_{\beta}$ . Further analysis shows that the wall heat-transfer coefficient has a linear relationship with$H_{\zeta}$ and is proportional to$\sqrt{H_{\beta}}$ when$H_{\beta}$ is small. This work provides a theoretical basis and solution for studying on the hypersonic reattachment flow, and it is also an extension of the asymmetric stagnation boundary layer theory.-
Keywords:
- oblique stagnation-point /
- reattachment /
- shear layer /
- heat transfer
[1] Dolling D S 2001 AIAA J. 39 1517
Google Scholar
[2] van Driest E R 1956 Aeronaut. Eng. Rev. 15 26
[3] Lees L 1956 J. Jet Propul. 26 259
Google Scholar
[4] Fay J A, Riddell F R 1958 J. Aerosp. Sci. 25 73
Google Scholar
[5] 李邦明 2013 博士学位论文 (北京: 中国科学院大学)
Li B M 2013 Ph. D. Dissertation (Beijing: University of Chinese Academy of Sciences) (in Chinese)
[6] Stuart J T 1959 J. Aerosp. Sci. 26 124
Google Scholar
[7] Tamada K 1979 J. Phys. Soc. Jpn. 46 310
Google Scholar
[8] Dorrepaal J M 1986 J. Fluid Mech. 163 141
Google Scholar
[9] Tooke R M, Blyth M G 2008 Phys. Fluids 20 33101
Google Scholar
[10] Lyell M J 1990 Phys. Fluids A 2 456
Google Scholar
[11] Chapman D R, Kuehn D M, Larson H K 1958 Investigation of Separated Flows in Supersonic and Subsonic Streams with Emphasis on the Effect of Transition (Washington, D.C.: National Advisory Committee for Aeronautics) Report No. NACA-TR-1356
[12] Zapryagaev V I, Kavun I N, Lipatov, I I 2013 Prog. Flight Phys. 5 349
Google Scholar
[13] Gadd G E, Holder D W, Regan J D 1954 Proc. R. Soc. London, Ser. A 226 227
Google Scholar
[14] Bernardini M, Asproulias I, Larsson J, Pirozzoli S, Grasso F 2016 Phys. Rev. Fluids 1 84403
Google Scholar
[15] Shang J S, Hankey Jr W L, Law C 1976 AIAA J. 14 1451
Google Scholar
[16] Davis R T 2003 J. Spacecr. Rockets 40 787
Google Scholar
[17] Dorodnitsyn A A 1942 Prikl. Mat. Mekh. 6 449
[18] Howarth L 1948 Proc. R. Soc. London, Ser. A 194 16
Google Scholar
[19] Hayes W D and Probstein R F 1959 Hypersonic Flow Theory (New York and London: Academic Press) pp343–344
[20] Simeonides G 1992 Ph. D. Dissertation (Bristol: University of Bristol)
[21] Eckert E R G 1955 J. Aeronaut. Sci. 22 585
Google Scholar
[22] Anderson Jr J D 2006 Hypersonic and High-Temperature Gas Dynamics (2nd Ed.) (Reston, Virginia: AIAA Inc.) pp341–343
[23] Moss J N, Bird G A 2005 AIAA J. 43 2565
Google Scholar
[24] Wang Z H, Yu Y L, Bao L 2018 AIAA J. 56 3593
Google Scholar
-
图 1 压缩拐角流动速度云图(横坐标和纵坐标分别为距前缘点的水平和垂直距离)
Fig. 1. Flow field of a ramp flow (The abscissa and the ordinate are the horizontal and vertical distances from the leading edge, respectively)
图 2 经典不可压缩斜驻点流动示意图 (a) 斜驻点流动; (b) 驻点流动与剪切流动的叠加
Fig. 2. Sketch of incompressible oblique stagnation-point flow: (a) Oblique stagnation-point flow; (b) stagnation-point flow and shear flow.
图 3 高速剪切层撞击壁面形成的斜驻点流动模型
Fig. 3. The model of compressible oblique-stagnation-point flow induced by impinging shear-layer.
图 4 理论结果与DSMC结果的温度剖面对比(横坐标为温度, 纵坐标为距壁面的法向距离)
Fig. 4. Comparision of temperature profile between theoretical results and DSMC simulations.
图 5 理论结果与DSMC结果的当地热流系数对比
Fig. 5. Comparision of the local heat-transfer coefficient (Stanton number) between theoretical results and DSMC simulations.
图 7
$ H_{\beta} $ 和$ H_{\zeta} $ 对温度剖面的影响 (a) 不同$ H_{\beta} $ 下的温度剖面; (b) 不同$ H_{\zeta} $ 下的温度剖面Fig. 7. Influences of
$ H_{\beta} $ and$ H_{\zeta} $ on heat transfer: (a) Temperature profiles under different$ H_{\beta} $ ; (b) temperature profiles under different$ H_{\zeta} $ .
图 8
$ H_{\beta} $ 和$ H_{\zeta} $ 对$ \varTheta_0^{\prime}(0) $ 的影响规律Fig. 8. Influences of
$ H_{\beta} $ and$ H_{\zeta} $ on$ \varTheta_0^{\prime}(0) $ .
图 9 参数
$ H_{\beta} $ 和$ H_{\zeta} $ 对壁面热流系数的影响 (a)$ H_{\beta} $ 的影响; (b)$ H_{\zeta} $ 的影响.Fig. 9. Influences of
$ H_{\beta} $ and$ H_{\zeta} $ on the wall heat-transfer coefficient: (a) Influence of$ H_{\beta} $ ; (b) influence of$ H_{\zeta} $ .表 A1 DSMC模拟压缩拐角分离-再附流动的流动参数
Table A1. Flow conditions of the compression ramp flow in DSMC simulations.
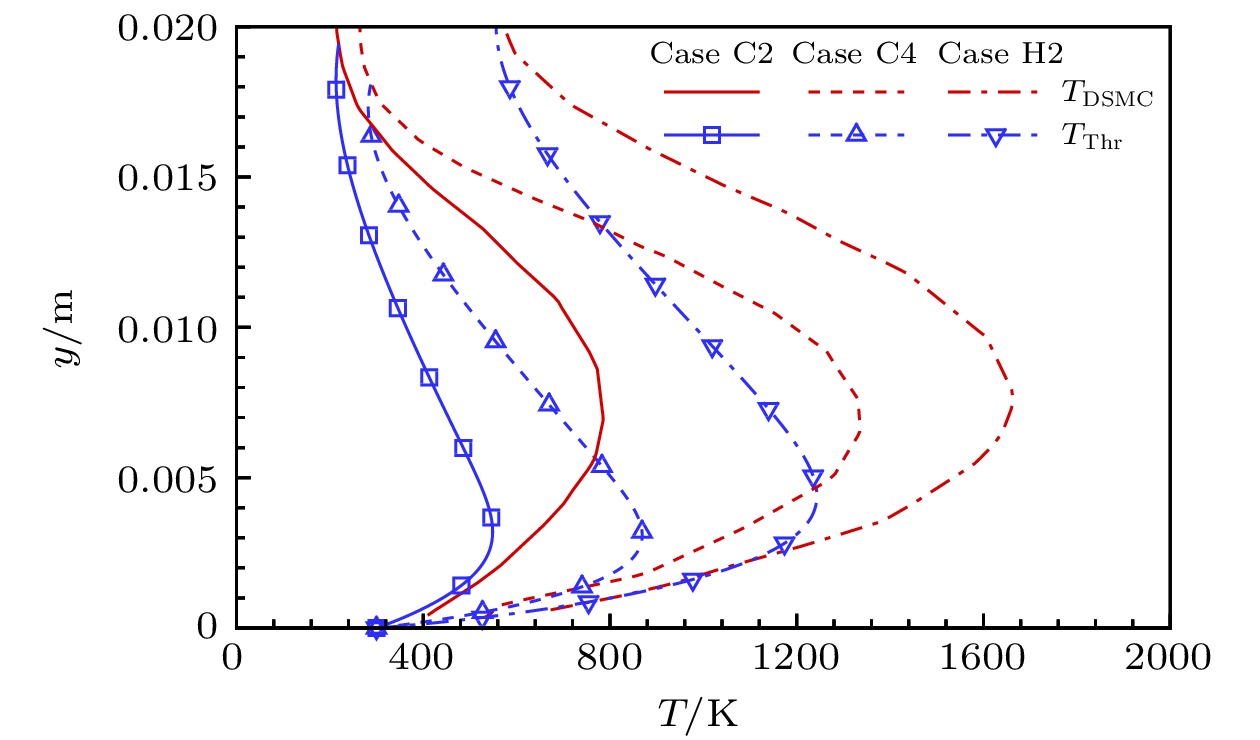
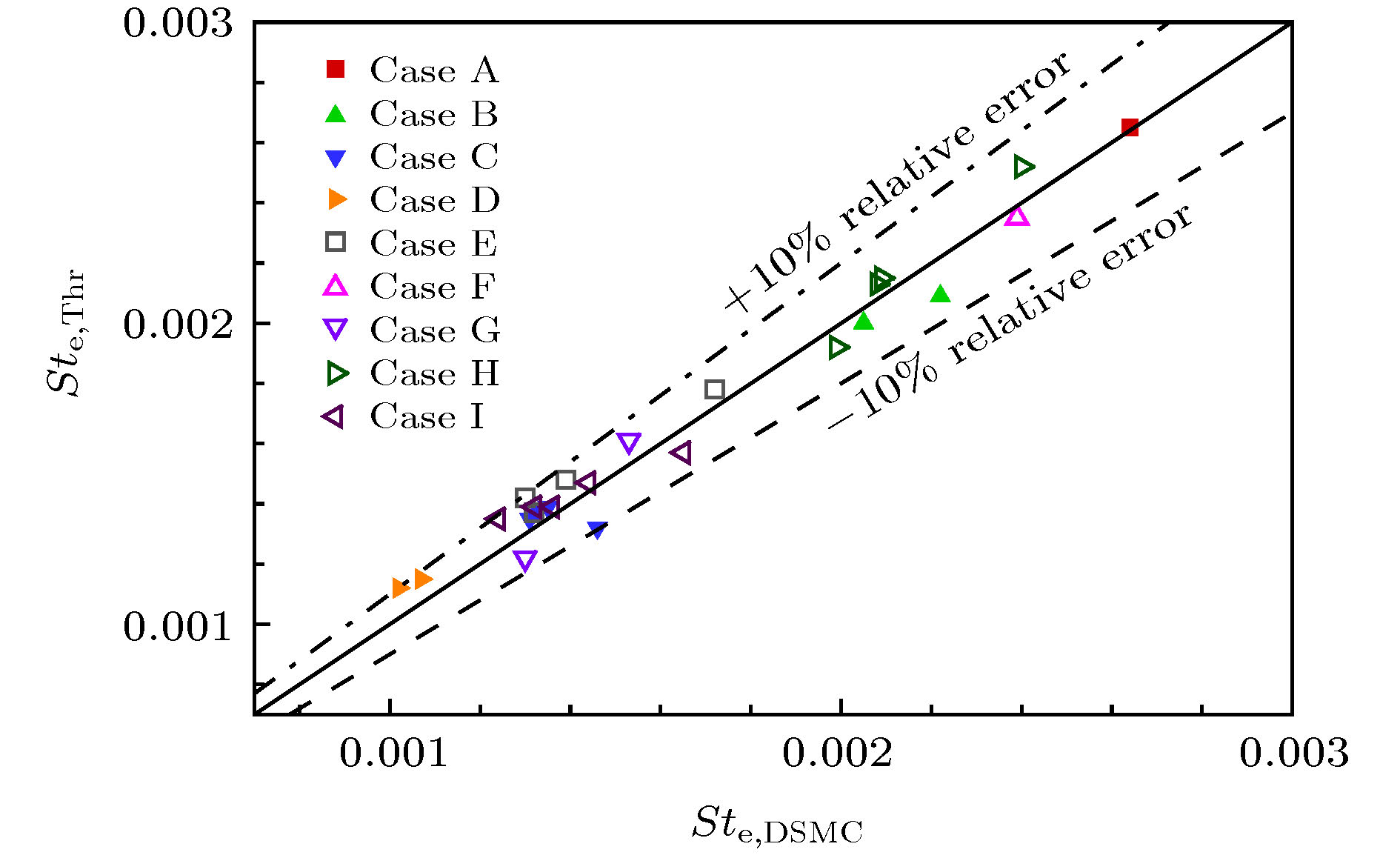
Case No. $ M_\infty $ $ {Re}_L / 10^4 $ $ \theta /(^{\circ}) $ $ T_\infty $/K $ T_{\rm{w}} $/K L/m A1 9 1 25 60 300 1 B1 9 2 25 60 300 1 B2 11 2 25 60 300 1 C1 9 5 25 60 300 1 C2 11 5 25 60 300 1 C3 13 5 25 60 300 1 C4 15 5 25 60 300 1 D1 13 10 25 60 300 1 D2 15 10 25 60 300 1 E1 11 5 21 60 297 1 E2 11 5 24 60 297 1 E3 11 5 27 60 297 1 E4 11 5 30 60 297 1 F1 15 2 30 60 300 1 G1 15 5 24 60 300 1 G2 15 5 27 60 300 1 H1 11 2 25 100 300 1 H2 11 2 25 150 300 1 H3 11 2 25 200 300 1 H4 11 2 25 250 300 1 I1 11 5 25 60 100 1 I2 11 5 25 60 200 1 I3 11 5 25 60 250 1 I4 11 5 25 60 350 1 I5 11 5 25 60 450 1 
下载: 导出CSV
表 A2 再附点后局部的无量纲流动参数
Table A2. Dimensionless parameters of the vicinity behind the reattachment point
Case No. $ M_{\rm{e}} $ $ H_{\beta} \times 10^4 $ $ H_{\zeta} \times 10^3 $ $ D_{\rm{e}} $ $ {\bar T}_{\rm{w}} $ $ \theta $ A1 4.82 1.79 12.25 15.2 1.64 13.6 B1 4.60 1.60 10.27 16.4 1.51 14.0 B2 5.57 1.16 6.45 19.9 1.43 15.0 C1 4.69 0.97 5.21 23.1 1.57 15.3 C2 5.44 0.78 3.89 25.6 1.37 15.8 C3 6.15 0.66 3.22 27.5 1.23 16.0 C4 6.55 0.68 3.10 27.7 1.04 16.5 D1 6.00 0.51 2.32 32.4 1.18 16.6 D2 6.28 0.51 2.17 32.9 0.96 17.0 E1 5.90 0.57 4.93 23.0 1.56 12.1 E2 5.60 0.71 4.07 25.1 1.42 14.8 E3 5.18 0.93 3.86 25.4 1.25 17.2 E4 4.71 1.28 3.99 24.5 1.07 19.7 F1 5.71 1.75 5.17 21.0 0.82 20.2 G1 7.05 0.50 2.61 30.4 1.19 15.5 G2 5.99 0.90 3.40 26.1 0.88 18.0 H1 5.48 1.17 6.40 18.9 0.83 15.1 H2 5.38 1.22 6.52 18.2 0.54 15.3 H3 5.46 1.12 5.90 18.9 0.41 15.4 H4 5.08 1.36 7.29 16.8 0.36 15.3 I1 5.35 0.90 3.88 23.4 0.45 16.9 I2 5.50 0.78 3.53 25.7 0.93 16.6 I3 5.50 0.75 3.57 26.1 1.17 16.2 I4 5.43 0.80 4.10 25.5 1.59 15.6 I5 5.45 0.73 4.06 27.1 2.07 15.0
下载: 导出CSV
-
[1] Dolling D S 2001 AIAA J. 39 1517
Google Scholar
[2] van Driest E R 1956 Aeronaut. Eng. Rev. 15 26
[3] Lees L 1956 J. Jet Propul. 26 259
Google Scholar
[4] Fay J A, Riddell F R 1958 J. Aerosp. Sci. 25 73
Google Scholar
[5] 李邦明 2013 博士学位论文 (北京: 中国科学院大学)
Li B M 2013 Ph. D. Dissertation (Beijing: University of Chinese Academy of Sciences) (in Chinese)
[6] Stuart J T 1959 J. Aerosp. Sci. 26 124
Google Scholar
[7] Tamada K 1979 J. Phys. Soc. Jpn. 46 310
Google Scholar
[8] Dorrepaal J M 1986 J. Fluid Mech. 163 141
Google Scholar
[9] Tooke R M, Blyth M G 2008 Phys. Fluids 20 33101
Google Scholar
[10] Lyell M J 1990 Phys. Fluids A 2 456
Google Scholar
[11] Chapman D R, Kuehn D M, Larson H K 1958 Investigation of Separated Flows in Supersonic and Subsonic Streams with Emphasis on the Effect of Transition (Washington, D.C.: National Advisory Committee for Aeronautics) Report No. NACA-TR-1356
[12] Zapryagaev V I, Kavun I N, Lipatov, I I 2013 Prog. Flight Phys. 5 349
Google Scholar
[13] Gadd G E, Holder D W, Regan J D 1954 Proc. R. Soc. London, Ser. A 226 227
Google Scholar
[14] Bernardini M, Asproulias I, Larsson J, Pirozzoli S, Grasso F 2016 Phys. Rev. Fluids 1 84403
Google Scholar
[15] Shang J S, Hankey Jr W L, Law C 1976 AIAA J. 14 1451
Google Scholar
[16] Davis R T 2003 J. Spacecr. Rockets 40 787
Google Scholar
[17] Dorodnitsyn A A 1942 Prikl. Mat. Mekh. 6 449
[18] Howarth L 1948 Proc. R. Soc. London, Ser. A 194 16
Google Scholar
[19] Hayes W D and Probstein R F 1959 Hypersonic Flow Theory (New York and London: Academic Press) pp343–344
[20] Simeonides G 1992 Ph. D. Dissertation (Bristol: University of Bristol)
[21] Eckert E R G 1955 J. Aeronaut. Sci. 22 585
Google Scholar
[22] Anderson Jr J D 2006 Hypersonic and High-Temperature Gas Dynamics (2nd Ed.) (Reston, Virginia: AIAA Inc.) pp341–343
[23] Moss J N, Bird G A 2005 AIAA J. 43 2565
Google Scholar
[24] Wang Z H, Yu Y L, Bao L 2018 AIAA J. 56 3593
Google Scholar
下载:
计量
- 文章访问数: 6389
- PDF下载量: 119
- 被引次数: 0
