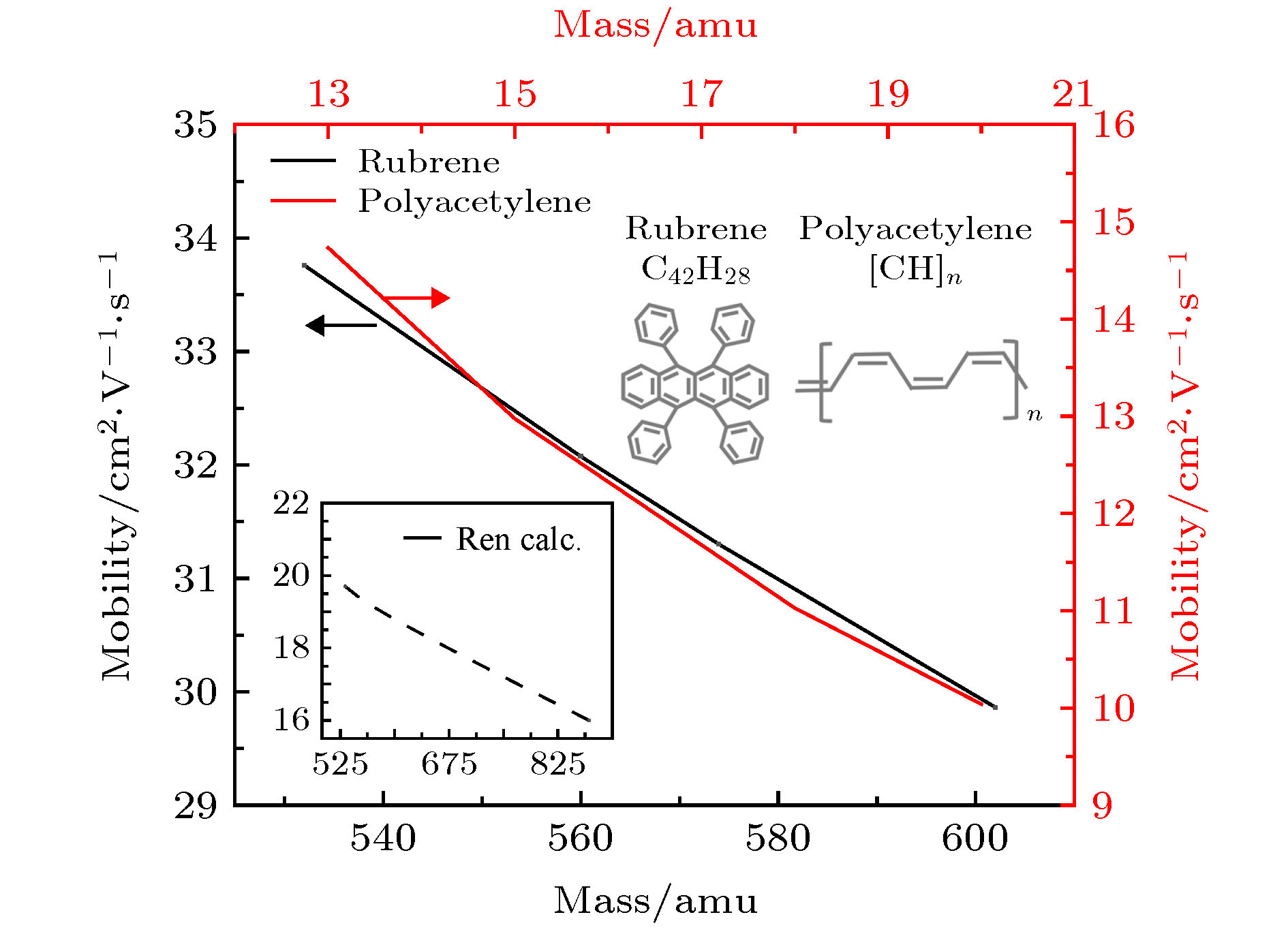
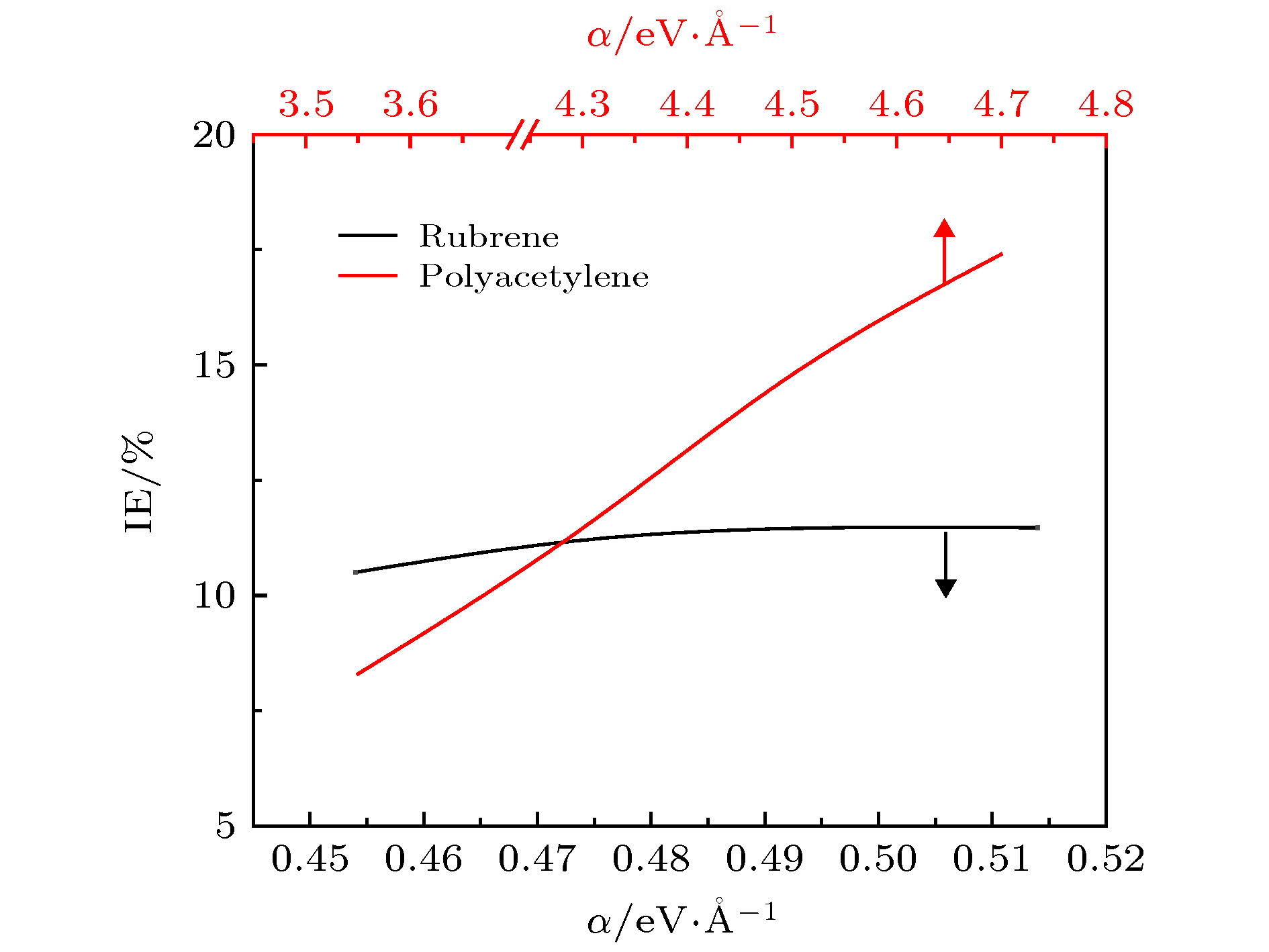
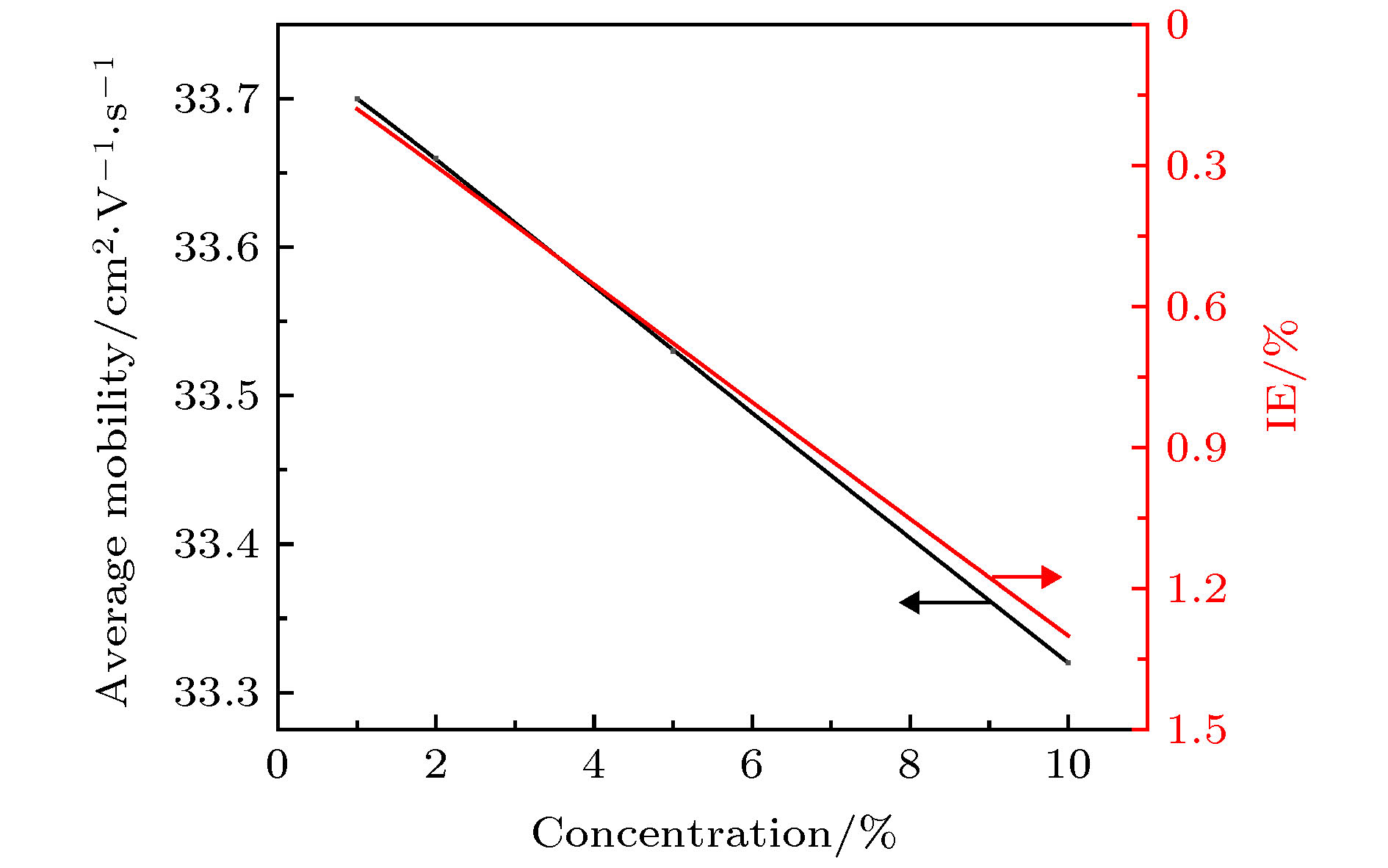
-
Isotopic substitution can effectively tune the device performances of organic semiconductors. According to the experimental results of isotope effects in electric, light and magnetic process in organic semiconductors, we adopt the tight-binding model with strong electron-phonon coupling to study the isotope effects on carrier transport. We try to give a quantificational explanation and show the physical origin of isotope effects on mobility in organic semiconductors in this work. Using polaron transport dynamics with diabatic approach, we simulate the carrier transport in an array of small molecule crystals under weak bias. Because of strong electron-phonon coupling in organic materials, an injected electron will induce lattice distortion, and the carriers are no longer free electrons or holes, but elementary excitations such as solitons, polarons or bipolarons. Our simulation results indicate that the existence of deuterium and 13C element will reduce the mobility of organic material, which means that the isotopic substitution can be utilized to manifest organic device performance. Besides, we also find that the isotope effect on mobility will increase with electron-phonon coupling increasing. This suggests that both the mass of lattice groups and electron-phonon coupling should be taken into account to understand the isotope effects in organic semiconductors. With the consideration of that, we derive the effective mass of polaron based on the continuum model, and verify that effective mass can successfully describe the isotope effect on mobility. The effective mass of carrier can be measured to represent the property of a material, which can tell us whether we need the isotopic substitution in organic layer to improve the device performance. Then we present the microcosmic movement of a polaron at the moment when it encounters isotopic substituted molecules. We come to the conclusion that the isotopic distribution will affect the instantaneous speed of the carrier, but has little effect on the mobility of the whole device when the substituted concentration remains constant. In conclusion, after simulating various possible isotope effects in materials, analyzing its physical mechanism and comparing calculation results in experiment, we provide a theoretical foundation for describing the isotope effects on mobility, which can be a basis of improving the performances of organic semiconductor devices.
-
Keywords:
- isotope effect /
- orginic small molecular crystal /
- tight-binding model
[1] Root S E, Savagatrup S, Printz A D, Rodriquez D, Lipomi D J 2017 Chem. Rev. 117 6467
Google Scholar
[2] Taniguchi T, Fukui K, Asahi R, Urabe Y, Ikemoto A, Nakamoto J, Inada Y, Yamao T, Hotta S 2017 Synth. Met. 227 162
Google Scholar
[3] de Jong M P 2016 Open Physics 14 337
Google Scholar
[4] Groves C 2017 Rep. Prog. Phys. 80 37
Google Scholar
[5] Danos A, MacQueen R W, Cheng Y Y, Dvorak M, Darwish T A, McCamey D R, Schmidt T W 2015 J. Phys. Chem. Lett. 6 3061
Google Scholar
[6] Stoltzfus D M, Joshi G, Popli H, Jamali S, Kavand M, Milster S, Grunbaum T, Bange S, Nahlawi A, Teferi M Y, Atwood SI, Leung A E, Darwish T A, Malissa H, Burn P L, Lupton J M, Boehme C 2020 J. Mater. Chem. C 8 2764
Google Scholar
[7] Wang P, Wang F F, Chen Y, Niu Q, Lu L, Wang H M, Gao X C, Wei B, Wu H W, Caic X, Zou D C 2013 J. Mater. Chem. C 1 4821
Google Scholar
[8] Nguyen T D, Hukic-Markosian G, Wang F, Wojcik L, Li X G, Ehrenfreund E, Vardeny Z V 2010 Nat. Mater. 9 345
Google Scholar
[9] Li L W, Li T Y, Arras M M L, Bonnesen P V, Peng X F, Li W, Hong K L 2020 Polymer 193 122375
Google Scholar
[10] Bartell L S, Roskos R R 1966 J. Chem. Phys. 44 457
Google Scholar
[11] White R P, Lipson J E G, Higgins J S 2010 Macromolecules 43 4287
Google Scholar
[12] Jiang J W, Lan J, Wang J S, Li B W 2010 J. Appl. Phys. 107 054314
Google Scholar
[13] Chang D, Li T, Li L, Jakowski J, Huang J, Keum J K, Lee B, Bonnesen P V, Zhou M, Garashchuk S, Sumpter B G, Hong K 2018 Macromolecules 51 9393
Google Scholar
[14] Shi C, Zhang X, Yu C H, Yao Y F, Zhang W 2018 Nat. Commun. 9 481
Google Scholar
[15] Jakowski J, Huang J, Garashchuk S, Luo Y, Hong K, Keum J, Sumpter B G 2017 J. Phys. Chem. Lett. 8 4333
Google Scholar
[16] Jiang Y, Peng Q, Geng H, Ma H, Shuai Z 2015 Phys. Chem. Chem. Phys. 17 3273
Google Scholar
[17] Tong C C, Hwang K C 2007 J. Phys. Chem. C 111 3490
Google Scholar
[18] Shao M, Keum J, Chen J, He Y, Chen W, Browning J F, Jakowski J, Sumpter B G, Ivanov I N, Ma Y Z, Rouleau C M, Smith S C, Geohegan D B, Hong K, Xiao K 2014 Nat. Commun. 5 3180
Google Scholar
[19] Fratini S, Nikolka M, Salleo A, Schweicher G, Sirringhaus H 2020 Nat. Mater. 19 491
Google Scholar
[20] Ren X, Bruzek M J, Hanifi D A, Schulzetenberg A, Wu Y, Kim C H, Zhang Z, Johns J E, Salleo A, Fratini S, Troisi A, Douglas C J, Frisbie C D 2017 Adv. Electron. Mater. 3 1700018
Google Scholar
[21] Jiang Y, Geng H, Shi W, Peng Q, Zheng X, Shuai Z 2014 J. Phys. Chem. Lett. 5 2267
Google Scholar
[22] Jiang Y, Geng H, Li W, Shuai Z 2019 J. Chem. Theory Comput. 15 1477
Google Scholar
[23] Low F E, Pines D 1953 Phys. Rev. 91 193
Google Scholar
[24] Li W, Ren J, Shuai Z 2020 J. Phys. Chem. Lett. 11 4930
Google Scholar
[25] Liu X, Gao K, Fu J, Li Y, Wei J, Xie S 2006 Phys. Rev. B 74 172301
Google Scholar
[26] Troisi A, Orlandi G 2006 Phys. Rev. Lett. 96 086601
Google Scholar
[27] Johansson A A, Stafström S 2004 Phys. Rev. B 69 235205
Google Scholar
[28] Brankin R W, Gladwell I, Shampine L F http://www.netlib.org [2019-11-3]
[29] Köhler A, Bässler H 2015 Electronic Processes in Organic Semiconductors: An Introduction (Weinheim: Wiley-VCH) pp193–292
[30] Takayama H, Linliu Y R, Maki K 1980 Phys. Rev. B 21 2388
Google Scholar
[31] Miyata A, Mitioglu A, Plochocka P, Portugall O, Wang J T W, Stranks S D, Snaith H J, Nicholas R J 2015 Nat. Phys. 11 582
Google Scholar
[32] Zhong M, Zeng W, Tang H, Wang L X, Liu F S, Tang B, Liu Q J 2019 Sol. Energy 190 617
Google Scholar
-
图 1 无机材料(inorganic materials, IM)与有机材料(organic materials, OM)中的同位素对迁移率影响示意图
Fig. 1. Schematic diagram of isotope effects on mobility in inorganic materials (IM) and organic materials (OM).
图 3 同位素效应随电声耦合的变化
Fig. 3. Variation of isotope effect (IE) with electron-phonon coupling.
图 4 瞬时迁移率的同位素效应 (a) 单分子同位素取代; (b) 多分子同位素连续取代, 取代起始位置均为第125格点; (c)多分子同位素不连续取代. 图(a)和图(c)中圆点表示同位素取代分子所在位置, 取代分子中H与C元素均被取代
Fig. 4. Isotope effects on instantaneous mobility: (a) Isotopic substitution of one molecule; (b) isotopic substitution of continuous molecules, the initial position of all the substitution is on the 125th site; (c) isotopic substitution of discontinuous molecules. The dots in panels (a) and (c) indicate the locations of molecules in which both hydrogen and carbon are substituted.
图 5 极化子平均迁移率及同位素效应与同位素浓度的关系
Fig. 5. Avergae mobility and isotope effects depend on substituted concentration.
-
[1] Root S E, Savagatrup S, Printz A D, Rodriquez D, Lipomi D J 2017 Chem. Rev. 117 6467
Google Scholar
[2] Taniguchi T, Fukui K, Asahi R, Urabe Y, Ikemoto A, Nakamoto J, Inada Y, Yamao T, Hotta S 2017 Synth. Met. 227 162
Google Scholar
[3] de Jong M P 2016 Open Physics 14 337
Google Scholar
[4] Groves C 2017 Rep. Prog. Phys. 80 37
Google Scholar
[5] Danos A, MacQueen R W, Cheng Y Y, Dvorak M, Darwish T A, McCamey D R, Schmidt T W 2015 J. Phys. Chem. Lett. 6 3061
Google Scholar
[6] Stoltzfus D M, Joshi G, Popli H, Jamali S, Kavand M, Milster S, Grunbaum T, Bange S, Nahlawi A, Teferi M Y, Atwood SI, Leung A E, Darwish T A, Malissa H, Burn P L, Lupton J M, Boehme C 2020 J. Mater. Chem. C 8 2764
Google Scholar
[7] Wang P, Wang F F, Chen Y, Niu Q, Lu L, Wang H M, Gao X C, Wei B, Wu H W, Caic X, Zou D C 2013 J. Mater. Chem. C 1 4821
Google Scholar
[8] Nguyen T D, Hukic-Markosian G, Wang F, Wojcik L, Li X G, Ehrenfreund E, Vardeny Z V 2010 Nat. Mater. 9 345
Google Scholar
[9] Li L W, Li T Y, Arras M M L, Bonnesen P V, Peng X F, Li W, Hong K L 2020 Polymer 193 122375
Google Scholar
[10] Bartell L S, Roskos R R 1966 J. Chem. Phys. 44 457
Google Scholar
[11] White R P, Lipson J E G, Higgins J S 2010 Macromolecules 43 4287
Google Scholar
[12] Jiang J W, Lan J, Wang J S, Li B W 2010 J. Appl. Phys. 107 054314
Google Scholar
[13] Chang D, Li T, Li L, Jakowski J, Huang J, Keum J K, Lee B, Bonnesen P V, Zhou M, Garashchuk S, Sumpter B G, Hong K 2018 Macromolecules 51 9393
Google Scholar
[14] Shi C, Zhang X, Yu C H, Yao Y F, Zhang W 2018 Nat. Commun. 9 481
Google Scholar
[15] Jakowski J, Huang J, Garashchuk S, Luo Y, Hong K, Keum J, Sumpter B G 2017 J. Phys. Chem. Lett. 8 4333
Google Scholar
[16] Jiang Y, Peng Q, Geng H, Ma H, Shuai Z 2015 Phys. Chem. Chem. Phys. 17 3273
Google Scholar
[17] Tong C C, Hwang K C 2007 J. Phys. Chem. C 111 3490
Google Scholar
[18] Shao M, Keum J, Chen J, He Y, Chen W, Browning J F, Jakowski J, Sumpter B G, Ivanov I N, Ma Y Z, Rouleau C M, Smith S C, Geohegan D B, Hong K, Xiao K 2014 Nat. Commun. 5 3180
Google Scholar
[19] Fratini S, Nikolka M, Salleo A, Schweicher G, Sirringhaus H 2020 Nat. Mater. 19 491
Google Scholar
[20] Ren X, Bruzek M J, Hanifi D A, Schulzetenberg A, Wu Y, Kim C H, Zhang Z, Johns J E, Salleo A, Fratini S, Troisi A, Douglas C J, Frisbie C D 2017 Adv. Electron. Mater. 3 1700018
Google Scholar
[21] Jiang Y, Geng H, Shi W, Peng Q, Zheng X, Shuai Z 2014 J. Phys. Chem. Lett. 5 2267
Google Scholar
[22] Jiang Y, Geng H, Li W, Shuai Z 2019 J. Chem. Theory Comput. 15 1477
Google Scholar
[23] Low F E, Pines D 1953 Phys. Rev. 91 193
Google Scholar
[24] Li W, Ren J, Shuai Z 2020 J. Phys. Chem. Lett. 11 4930
Google Scholar
[25] Liu X, Gao K, Fu J, Li Y, Wei J, Xie S 2006 Phys. Rev. B 74 172301
Google Scholar
[26] Troisi A, Orlandi G 2006 Phys. Rev. Lett. 96 086601
Google Scholar
[27] Johansson A A, Stafström S 2004 Phys. Rev. B 69 235205
Google Scholar
[28] Brankin R W, Gladwell I, Shampine L F http://www.netlib.org [2019-11-3]
[29] Köhler A, Bässler H 2015 Electronic Processes in Organic Semiconductors: An Introduction (Weinheim: Wiley-VCH) pp193–292
[30] Takayama H, Linliu Y R, Maki K 1980 Phys. Rev. B 21 2388
Google Scholar
[31] Miyata A, Mitioglu A, Plochocka P, Portugall O, Wang J T W, Stranks S D, Snaith H J, Nicholas R J 2015 Nat. Phys. 11 582
Google Scholar
[32] Zhong M, Zeng W, Tang H, Wang L X, Liu F S, Tang B, Liu Q J 2019 Sol. Energy 190 617
Google Scholar
下载:
计量
- 文章访问数: 6872
- PDF下载量: 112
- 被引次数: 0
