电磁场中施主中心量子点内磁极化子态寿命与qubit退相干

白旭芳; 陈磊; 额尔敦朝鲁

doi:10.7498/aps.69.20200242

摘要

采用Lee-Low-Pines变换和Pekar类型变分法推导出非对称高斯势施主中心量子点中磁极化子的基态和激发态能量和波函数, 进而构造了qubit所需的二能级结构. 基于费米黄金规则研究了磁极化子基态的衰变. 通过研究电磁场下材料的介电常数比、电声耦合常数和温度对非对称高斯势施主中心量子点中磁极化子基态寿命的影响, 揭示了材料属性与温度、电磁场等环境因素对量子点qubit退相干的影响, 进而揭示了体纵光学声子效应导致量子点qubit退相干的机理.

关键词:

Abstract

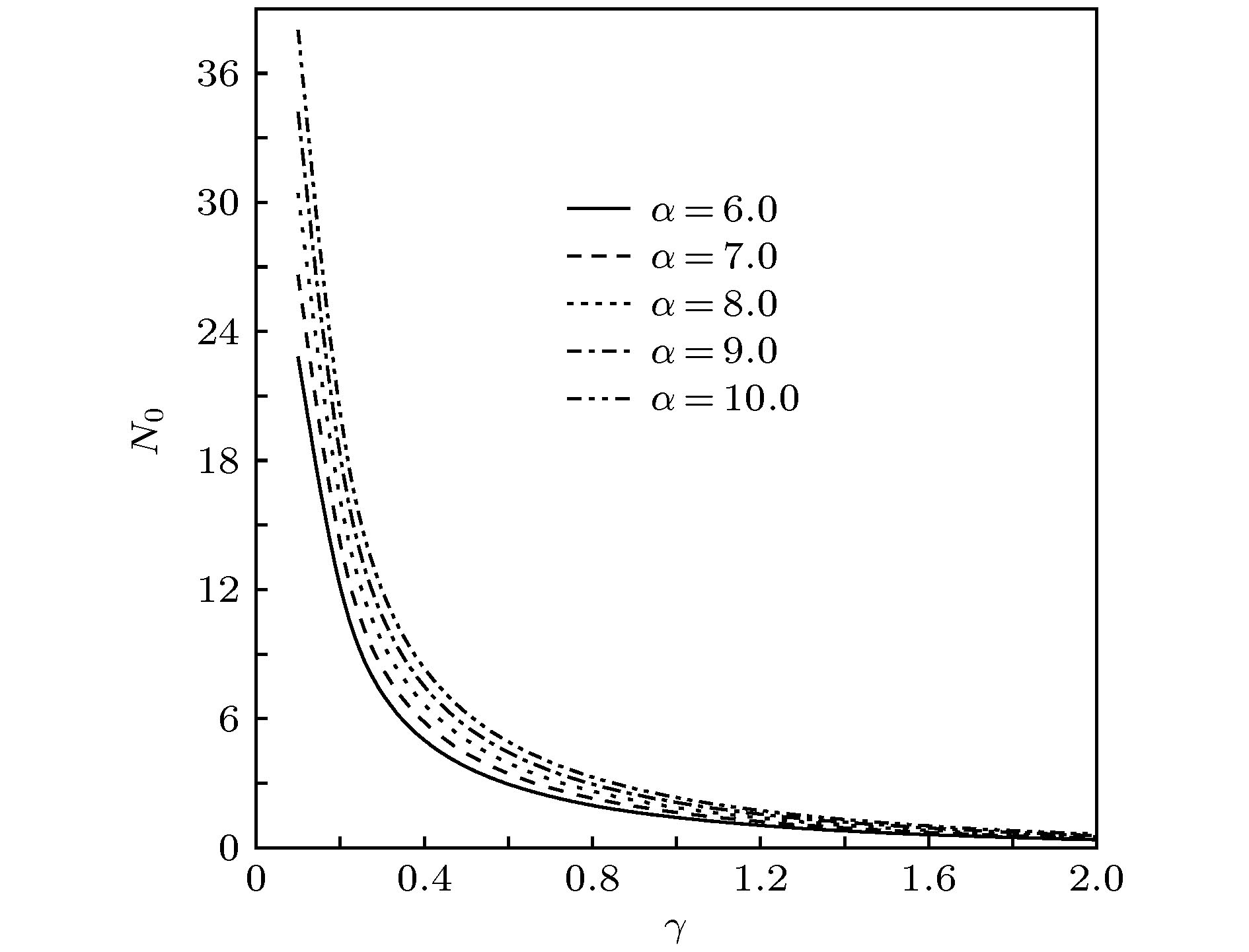
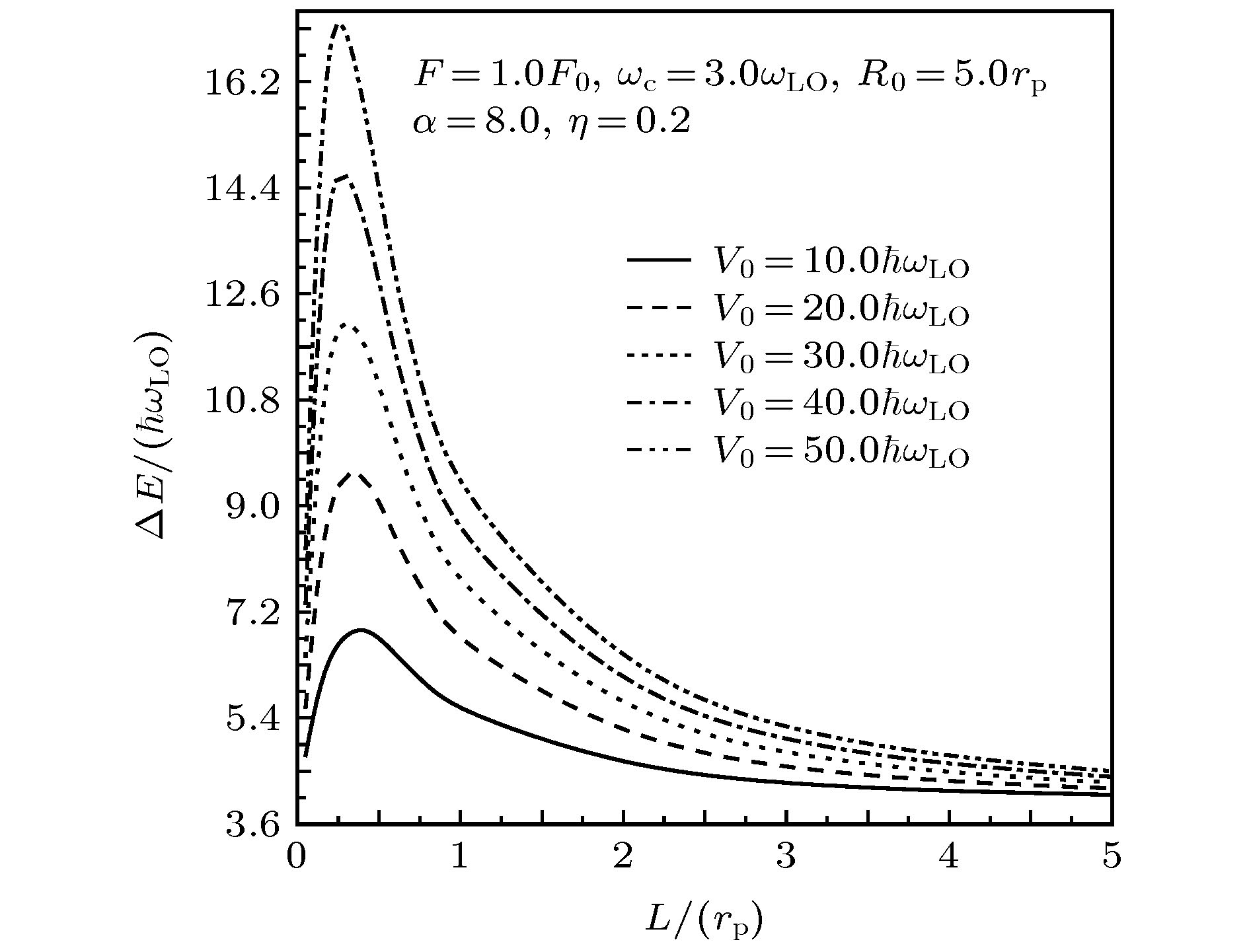
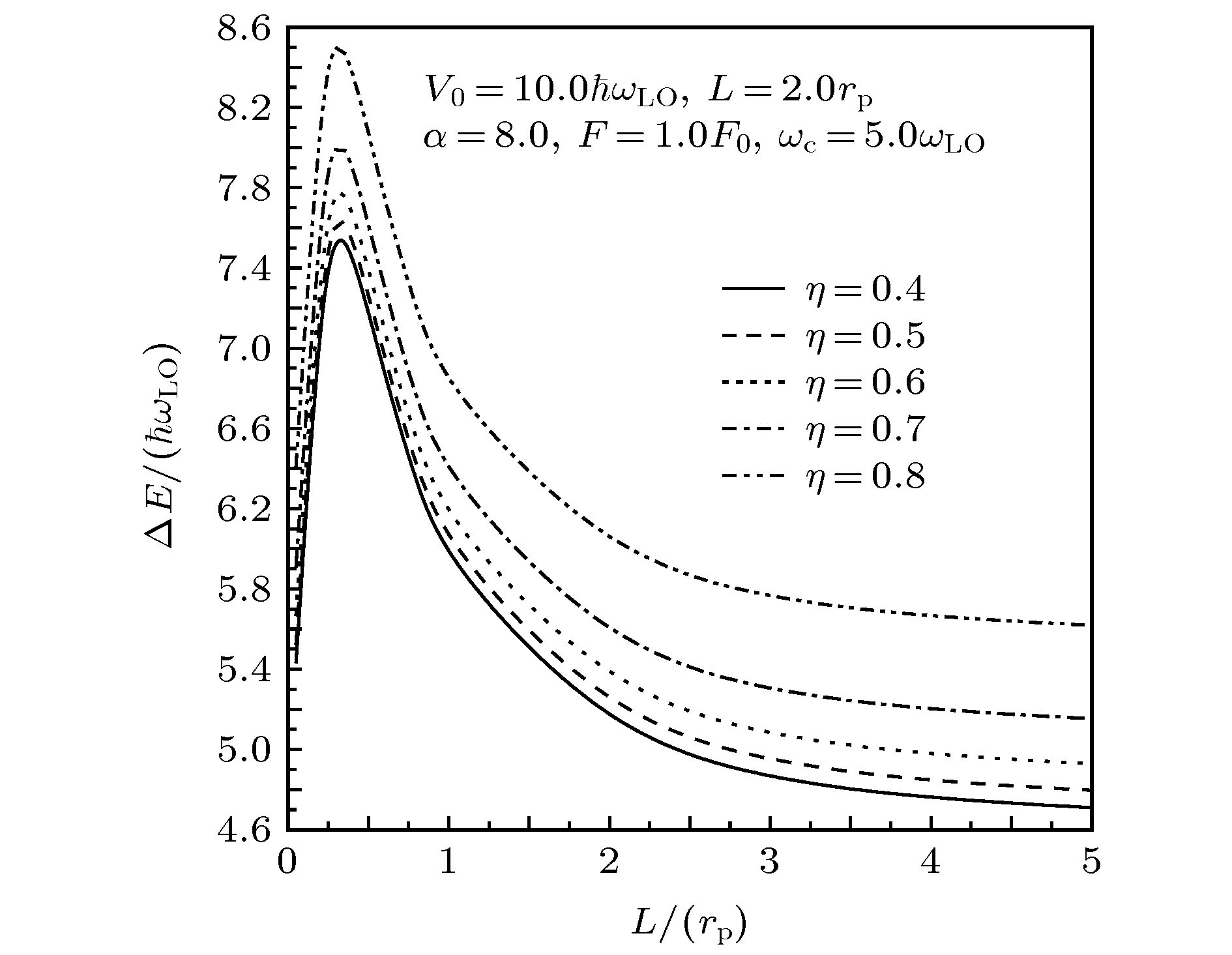
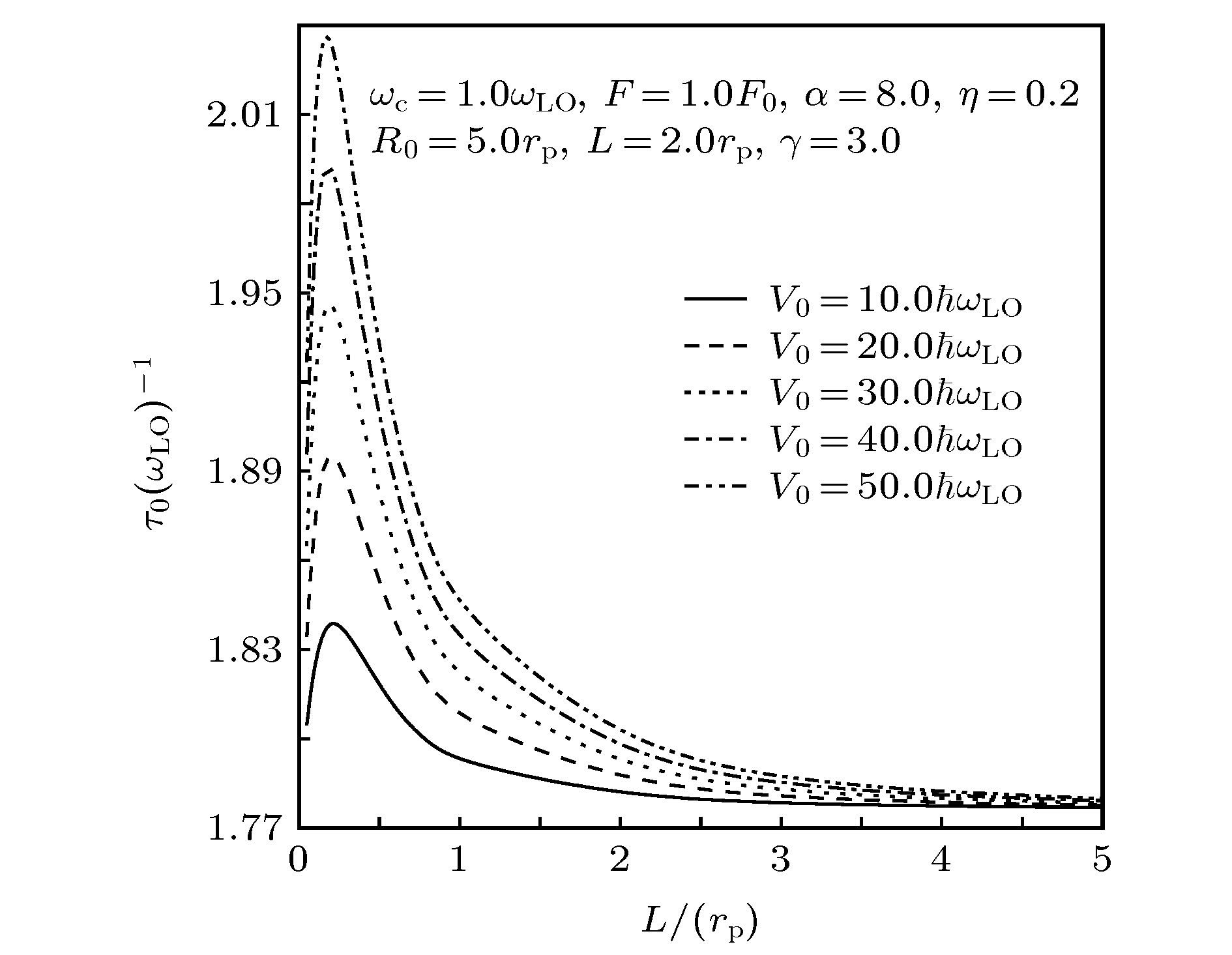
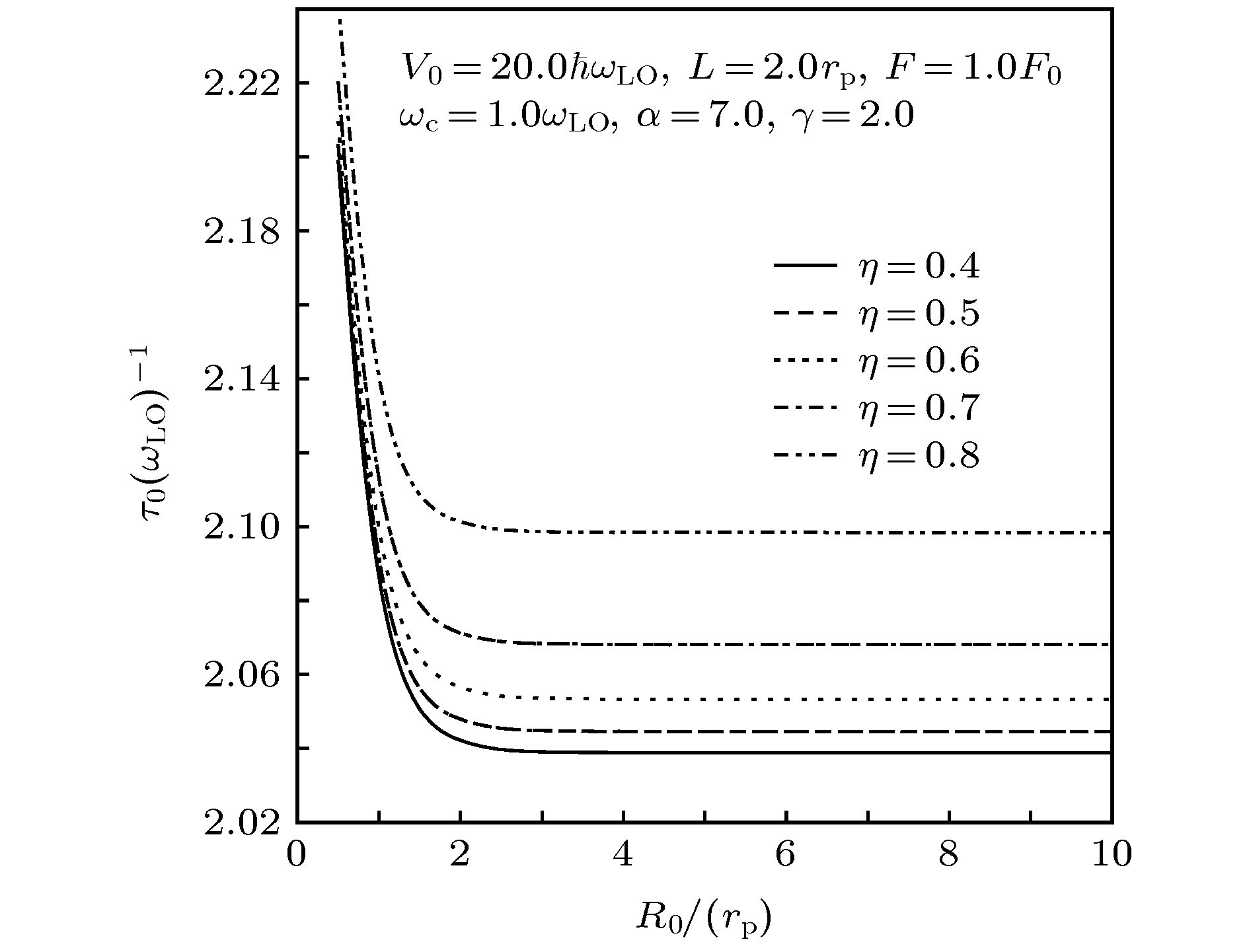
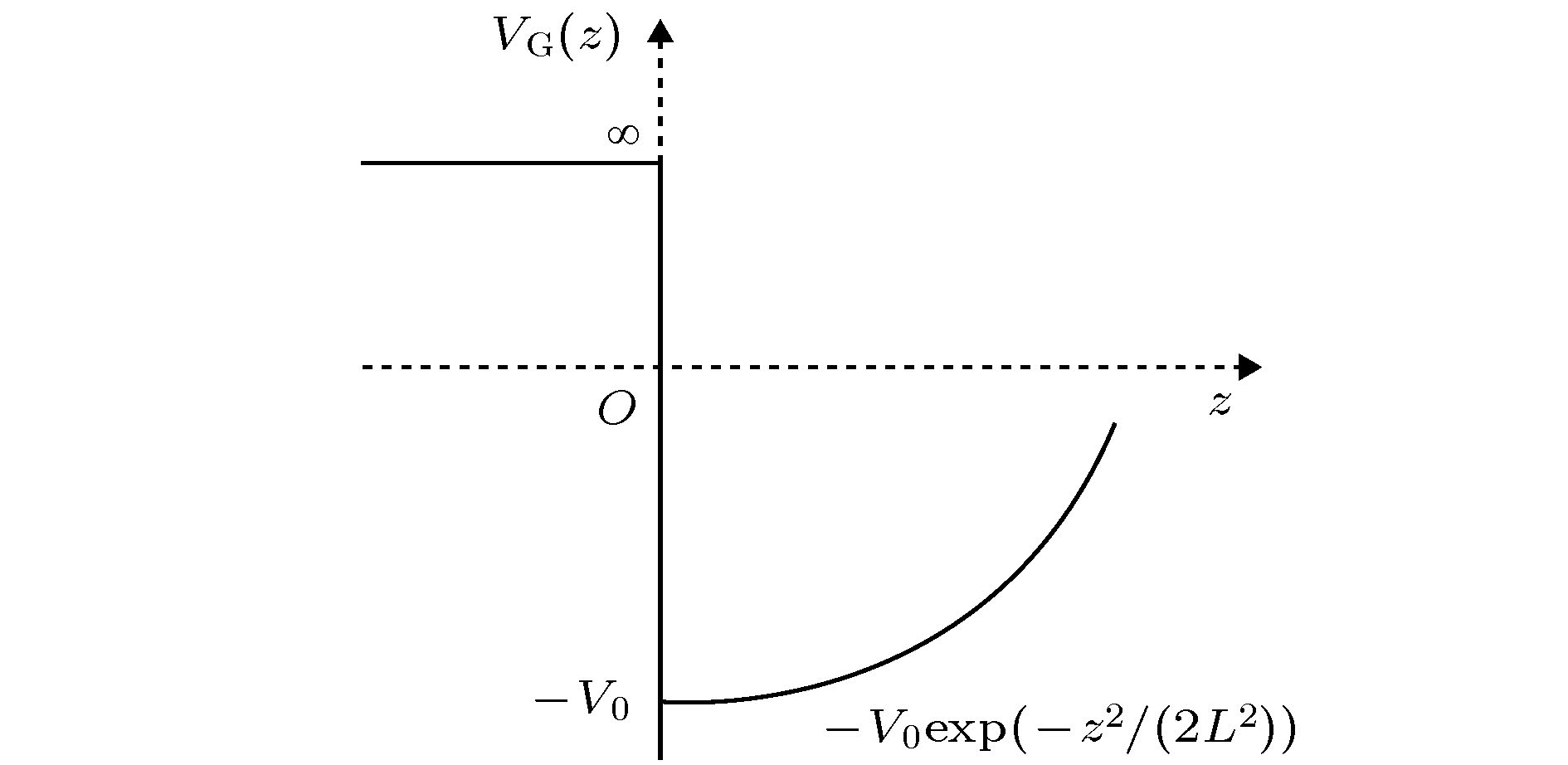
Recently, the measurement scheme of quantum dot qubit decocoherence quantized by the longitudinal optical (LO) phonon spontaneous emission rate has attracted the attention and discussion of many researchers. However, it is not difficult to see that the above-mentioned measurement scheme still has some insufficient and imperfect aspects that are to be studied urgently. Considering from the physical mechanism, the essence of the above scheme is to quantify the decoherence time of qubit by using the excited state decay time or excited state lifetime of the polaron. However, so far, there is little research on how the ground state decay time and ground state lifetime of two-state polaron affect the coherence of qubit. There is no doubt that this is an equally important research topic. This is because, firstly, for the coherence of the quantum state of polaron, both the decay of the excited state and the decay of the ground state will destroy or attenuate the qubit coherence, secondly, the transition rate of the two-state polaron from the ground state to the excited state after absorbing an LO phonon is also a function quantifying the qubit decoherence time of two-state system of which the inverse is called the ground state decay time or the ground state lifetime. It may be called a measure of qubit decoherence time quantized by the ground state decay time or ground state lifetime of polaron. In this article, the ground-state and excited-state energy and wave function of the magnetopolaron in a donor-center quantum dot with asymmetric Gaussian potential are derived by Lee-Low-Pines transformation and Pekar-type variational methodd, and then the two-level structure for a qubit is constructed. The measure of qubit decoherence time of quantum dots quantified by ground state decay time of two-state polaron is established, which is compared with the well-known measure of qubit decoherence time of quantum dots quantified by polaron excited state decay time, and their physical mechanisms are revealed. By studying the influence of dielectric constant ratio, electro-phonons coupling constant, temperature and electromagnetic field on the ground state lifetime of magnetopolaron in the donor-center quantum dots with asymmetric Gaussian potential, the influences of material properties, temperature, electromagnetic field and other environmental factors on qubit decoherence of quantum dots are revealed, thereby revealing the mechanism of qubit decoherence caused by LO phonon effect.

Keywords:

作者及机构信息

1.
内蒙古民族大学物理与电子信息学院, 通辽　028043

2.
河北科技师范学院凝聚态物理研究所, 秦皇岛　066004

通信作者: 陈磊, eedcl2603@hevttc.edu.cn

基金项目: 国家级-国家自然科学基金(51902085)

Authors and contacts

Bai Xu-Fang¹,
Chen Lei²,

1.
College of Physics and Electronic Information, Inner Mongolia University for Nationalities, Tongliao 028043, China

2.
Institute of Condensed Matter Physics, Hebei Normal University of Science and Technology, Qinhuangdao 066004, China

Corresponding author: Chen Lei, eedcl2603@hevttc.edu.cn

文章全文 : translate this paragraph

参考文献

[1]	Tiotsop M, Fotue A J, Talla P K, Kenfack S C, Fautso K G, Fotsin H, Fai L C 2018 Iran. J. Sci. Technol. A 42 933 Google Scholar
[2]	Lang Z H, Cai C U, Xiao J L 2019 Int. J. Theor. Phys. 58 2320 Google Scholar
[3]	Jordan K, Stephen J P 2005 Phys. Rev. B 71 125332 Google Scholar
[4]	Liang Z H, Xiao J L 2018 Indian J. Phys. 92 437 Google Scholar
[5]	Chi F, Li S S 2006 J. Appl. Phys. 99 043705 Google Scholar
[6]	Li S S, Xia J B, Yang F H, Niu Z C, Feng S L, Zheng H Z 2001 J. Appl. Phys. 90 6151 Google Scholar
[7]	Petta J R, Johnson A C, Taylor J M, Laird E A, Yacoby A, Lukin M D, Marcus C M, Hanson M P, Gossard A C 2005 Science 309 2180 Google Scholar
[8]	Varwig S, René A, Greilich A, Yakovlev D R, Reuter D, Wieck A D, Bayer B 2013 Phys. Rev. B 87 115307 Google Scholar
[9]	Sun Y, Xiao J L 2019 Opt. Quantum Electron. 51 110 Google Scholar
[10]	Xiao J L 2019 J. Low Temp. Phys. 195 442 Google Scholar
[11]	Ma X J, Xiao J L 2018 Opt. Quantum Electron. 50 144 Google Scholar
[12]	Xiao J L 2018 J. Low Temp. Phys. 192 41 Google Scholar
[13]	Baumgratz T, Cramer M, Plenio M B 2014 Phys. Rev. Lett. 113 140401 Google Scholar
[14]	Shao L H, Xi Z J, Fan H, Li Y M 2015 Phys. Rev. A 91 042120 Google Scholar
[15]	Rana S, Parashar P, Lewenstein M 2016 Phys. Rev. A 93 012110 Google Scholar
[16]	Streltsov A, Singh U, Dhar H S, Bera M N, Adesso G 2015 Phys. Rev. Lett. 115 020403 Google Scholar
[17]	Ma J J, Yadin B, Girolami D, Vedral V, Gu M 2016 Phys. Rev. Lett. 116 160407 Google Scholar
[18]	Davide G 2014 Phys. Rev. Lett. 113 170401 Google Scholar
[19]	Pires D P, Céleri L C, Soares-Pinto D O 2015 Phys. Rev. A 91 042330 Google Scholar
[20]	Fotue A J, Fobasso M F C, Kenfack S C, Tiotsop M, Djomou J R D, Ekosso C M, Nguimeya G P, Danga J E, Keumo Tsiaze R M, Fai L C 2016 Eur. Phys. J. Plus 131 205 Google Scholar
[21]	Xiao W, Xiao J L 2016 Int. J. Theor. Phys. 55 2936 Google Scholar
[22]	Sun Y, Ding Z H, Xiao J L 2014 J. Low Temp. Phys. 177 151 Google Scholar
[23]	Sun Y, Ding Z H, Xiao J L 2017 J. Electron. Mater. 46 439 Google Scholar
[24]	Bai X F, Xin W, Eerdunchaolu 2019 Int. J. Mod. Phys. B 33 1950322 Google Scholar
[25]	乌云其木格, 韩超, 额尔敦朝鲁 2019 68 247803 Google Scholar Wuyunqimuge, Han C, Eerdunchaolu 2019 Acta Phys. Sin. 68 247803 Google Scholar
[26]	Boucaud P, Sauvage S, Bras F, Fishman G, Ortéga J M, Gérard J M 2005 Physica E 26 59 Google Scholar
[27]	AZibik E, Wilson L R, Green R P, Wells J P R, Phillips P J, Carder D A, Cockburn J W, Skolnick M S, Steer M J, Liu H Y, Hopkinson M 2004 Physica E 21 405 Google Scholar
[28]	Verzelen O, Ferreira R, Bastard G 2002 Physica E 13 309 Google Scholar
[29]	Yu Y F, Xiao J L, Yin J W, Wang Z W 2008 Chin. Phys. B 17 2236 Google Scholar
[30]	Khordad R, Goudarzi S, Bahramiyan H 2016 Indian J. Phys. 90 659 Google Scholar
[31]	Li Z X 2019 Indian J. Phys. 93 707 Google Scholar
[32]	Lee T D 1953 Phys. Rev. 90 297 Google Scholar
[33]	Landau L D, Pekar S I 1948 Zh. Eksp. Teor. Fiz. 18 419
[34]	Brummell M A, Nicholas R J, Hopkins M A, Harris J J, Foxon C T 1987 Phys. Rev. Lett. 58 77 Google Scholar
[35]	Bai X F, Xin W, Yin H W, Eerdunchaolu 2017 J. Korean Phys. Soc. 70 956 Google Scholar

施引文献

图 1 非对称高斯势曲线

Fig. 1. Asymmetric Gaussian (AG) potential curve.

下载: 全尺寸图片幻灯片

图 2 LO声子平均数${N_0}$在不同电声耦合常数$\alpha $下随温度参数$\gamma $的变化

Fig. 2. Mean number ${N_0}$ of LO phonons as a function of the temperature parameter $\gamma $ at different electron-phonon coupling (EPC) constant $\alpha $.

下载: 全尺寸图片幻灯片

图 14 磁极化子基态寿命${\tau _0}$在不同电场强度$F$下随抛物势范围${R_0}$的变化

Fig. 14. The ground-state lifetime ${\tau _0}$ as a function of the range ${R_0}$ of the parabolic potential at different electric field $F$.

下载: 全尺寸图片幻灯片

图 3 磁极化子基态能量${E_0}$及其各组成部分随高斯势阱宽$L$的变化

Fig. 3. Ground state energy ${E_0}$ of the magnetopolaron and its components versus the well width $L$ of the AG potential.

下载: 全尺寸图片幻灯片

图 4 能隙$\Delta E$在非对称高斯势不同阱深${V_0}$下随其阱宽$L$的变化

Fig. 4. Energy gap $\Delta E$ as a function of the well width $L$ at different well depth ${V_0}$ of the AG potential.

下载: 全尺寸图片幻灯片

图 5 能隙$\Delta E$在不同介电常数比$\eta $下随高斯势阱宽$L$的变化

Fig. 5. Energy gap $\Delta E$ versus the well width $L$ of the AG potential under different dielectric constant (DC) ratio $\eta $.

下载: 全尺寸图片幻灯片

图 6 能隙$\Delta E$在不同电声耦合常数$\alpha $下随高斯势阱深${V_0}$的变化

Fig. 6. Energy gap $\Delta E$ as a function of the well depth ${V_0}$ of the AG potential at different EPC constant $\alpha $.

下载: 全尺寸图片幻灯片

图 7 能隙$\Delta E$在不同电场强度$F$下随高斯势阱深${V_0}$的变化

Fig. 7. Energy gap $\Delta E$ versus the well depth ${V_0}$ of the AG potential under different electric field $F$.

下载: 全尺寸图片幻灯片

图 8 能隙$\Delta E$在不同磁场的回旋频率${\omega _{\rm{c}}}$下随抛物势范围${R_0}$的变化

Fig. 8. Energy gap $\Delta E$ as a function of the range ${R_0}$ of the parabolic potential at different magnetic-field cyclotron (MFC) frequency ${\omega _{\rm{c}}}$.

下载: 全尺寸图片幻灯片

图 9 磁极化子基态寿命${\tau _0}$在高斯势不同阱深${V_0}$下随其阱宽$L$的变化

Fig. 9. The ground-state lifetime ${\tau _0}$ of the magntopolaron as a function of the well width $L$ at different well depth ${V_0}$ of the AG potential.

下载: 全尺寸图片幻灯片

图 10 基态寿命${\tau _0}$在不同电声耦合常数$\alpha $下随高斯势阱宽$L$的变化

Fig. 10. The ground-state lifetime ${\tau _0}$ as a function of the well width $L$ of the AG potential at different EPC constant $\alpha $.

下载: 全尺寸图片幻灯片

图 11 基态寿命${\tau _0}$在不同温度参数$\gamma $下随高斯势阱宽$L$的变化

Fig. 11. The ground-state lifetime ${\tau _0}$ as a function of the well width $L$ of the AG potential at different temperature parameter $\gamma $.

下载: 全尺寸图片幻灯片

图 12 基态寿命${\tau _0}$在不同介电常数比$\eta $下随抛物势范围${R_0}$的变化

Fig. 12. The ground-state lifetime ${\tau _0}$ as a function of the range ${R_0}$ of the parabolic potential at different DC ratio $\eta $

下载: 全尺寸图片幻灯片

图 13 基态寿命${\tau _0}$在磁场的不同回旋频率${\omega _{\rm{c}}}$下随高斯势阱深${V_0}$的变化

Fig. 13. The ground-state lifetime ${\tau _0}$ versus the well depth ${V_0}$ of the AG potential under different MFC frequencies ${\omega _{\rm{c}}}$

下载: 全尺寸图片幻灯片

map

[1]	Tiotsop M, Fotue A J, Talla P K, Kenfack S C, Fautso K G, Fotsin H, Fai L C 2018 Iran. J. Sci. Technol. A 42 933 Google Scholar
[2]	Lang Z H, Cai C U, Xiao J L 2019 Int. J. Theor. Phys. 58 2320 Google Scholar
[3]	Jordan K, Stephen J P 2005 Phys. Rev. B 71 125332 Google Scholar
[4]	Liang Z H, Xiao J L 2018 Indian J. Phys. 92 437 Google Scholar
[5]	Chi F, Li S S 2006 J. Appl. Phys. 99 043705 Google Scholar
[6]	Li S S, Xia J B, Yang F H, Niu Z C, Feng S L, Zheng H Z 2001 J. Appl. Phys. 90 6151 Google Scholar
[7]	Petta J R, Johnson A C, Taylor J M, Laird E A, Yacoby A, Lukin M D, Marcus C M, Hanson M P, Gossard A C 2005 Science 309 2180 Google Scholar
[8]	Varwig S, René A, Greilich A, Yakovlev D R, Reuter D, Wieck A D, Bayer B 2013 Phys. Rev. B 87 115307 Google Scholar
[9]	Sun Y, Xiao J L 2019 Opt. Quantum Electron. 51 110 Google Scholar
[10]	Xiao J L 2019 J. Low Temp. Phys. 195 442 Google Scholar
[11]	Ma X J, Xiao J L 2018 Opt. Quantum Electron. 50 144 Google Scholar
[12]	Xiao J L 2018 J. Low Temp. Phys. 192 41 Google Scholar
[13]	Baumgratz T, Cramer M, Plenio M B 2014 Phys. Rev. Lett. 113 140401 Google Scholar
[14]	Shao L H, Xi Z J, Fan H, Li Y M 2015 Phys. Rev. A 91 042120 Google Scholar
[15]	Rana S, Parashar P, Lewenstein M 2016 Phys. Rev. A 93 012110 Google Scholar
[16]	Streltsov A, Singh U, Dhar H S, Bera M N, Adesso G 2015 Phys. Rev. Lett. 115 020403 Google Scholar
[17]	Ma J J, Yadin B, Girolami D, Vedral V, Gu M 2016 Phys. Rev. Lett. 116 160407 Google Scholar
[18]	Davide G 2014 Phys. Rev. Lett. 113 170401 Google Scholar
[19]	Pires D P, Céleri L C, Soares-Pinto D O 2015 Phys. Rev. A 91 042330 Google Scholar
[20]	Fotue A J, Fobasso M F C, Kenfack S C, Tiotsop M, Djomou J R D, Ekosso C M, Nguimeya G P, Danga J E, Keumo Tsiaze R M, Fai L C 2016 Eur. Phys. J. Plus 131 205 Google Scholar
[21]	Xiao W, Xiao J L 2016 Int. J. Theor. Phys. 55 2936 Google Scholar
[22]	Sun Y, Ding Z H, Xiao J L 2014 J. Low Temp. Phys. 177 151 Google Scholar
[23]	Sun Y, Ding Z H, Xiao J L 2017 J. Electron. Mater. 46 439 Google Scholar
[24]	Bai X F, Xin W, Eerdunchaolu 2019 Int. J. Mod. Phys. B 33 1950322 Google Scholar
[25]	乌云其木格, 韩超, 额尔敦朝鲁 2019 68 247803 Google Scholar Wuyunqimuge, Han C, Eerdunchaolu 2019 Acta Phys. Sin. 68 247803 Google Scholar
[26]	Boucaud P, Sauvage S, Bras F, Fishman G, Ortéga J M, Gérard J M 2005 Physica E 26 59 Google Scholar
[27]	AZibik E, Wilson L R, Green R P, Wells J P R, Phillips P J, Carder D A, Cockburn J W, Skolnick M S, Steer M J, Liu H Y, Hopkinson M 2004 Physica E 21 405 Google Scholar
[28]	Verzelen O, Ferreira R, Bastard G 2002 Physica E 13 309 Google Scholar
[29]	Yu Y F, Xiao J L, Yin J W, Wang Z W 2008 Chin. Phys. B 17 2236 Google Scholar
[30]	Khordad R, Goudarzi S, Bahramiyan H 2016 Indian J. Phys. 90 659 Google Scholar
[31]	Li Z X 2019 Indian J. Phys. 93 707 Google Scholar
[32]	Lee T D 1953 Phys. Rev. 90 297 Google Scholar
[33]	Landau L D, Pekar S I 1948 Zh. Eksp. Teor. Fiz. 18 419
[34]	Brummell M A, Nicholas R J, Hopkins M A, Harris J J, Foxon C T 1987 Phys. Rev. Lett. 58 77 Google Scholar
[35]	Bai X F, Xin W, Yin H W, Eerdunchaolu 2017 J. Korean Phys. Soc. 70 956 Google Scholar

[1]	张治达, 易康源, 陈远珍, 燕飞. 多能级系统中的动力学解耦. , 2023, 72(10): 100305. doi: 10.7498/aps.72.20222398
[2]	巩龙延, 杨慧, 赵生妹. 中间测量对受驱单量子比特统计复杂度的影响. , 2020, 69(23): 230301. doi: 10.7498/aps.69.20200802
[3]	闫婕, 魏苗苗, 邢燕霞. HgTe/CdTe量子阱中自旋拓扑态的退相干效应. , 2019, 68(22): 227301. doi: 10.7498/aps.68.20191072
[4]	乌云其木格, 韩超, 额尔敦朝鲁. 色散和杂质对双参量非对称高斯势量子点量子比特的影响. , 2019, 68(24): 247803. doi: 10.7498/aps.68.20190960
[5]	马莉, 沈光地, 陈依新, 蒋文静, 郭伟玲, 徐晨, 高志远. 新型AlGaInP系发光二极管饱和特性与寿命的研究. , 2014, 63(3): 037201. doi: 10.7498/aps.63.037201
[6]	常锋, 王晓茜, 盖永杰, 严冬, 宋立军. 光与物质相互作用系统中的量子Fisher信息和自旋压缩. , 2014, 63(17): 170302. doi: 10.7498/aps.63.170302
[7]	白旭芳, 乌云其木格, 辛伟, 额尔敦朝鲁. Rashba自旋-轨道相互作用影响下量子盘中强耦合磁极化子性质的研究. , 2014, 63(17): 177803. doi: 10.7498/aps.63.177803
[8]	党文佳, 曾晓东, 冯喆珺. 目标粗糙对合成孔径激光雷达回波的退相干效应. , 2013, 62(2): 024204. doi: 10.7498/aps.62.024204
[9]	张浩亮, 贾芳, 徐学翔, 郭琴, 陶向阳, 胡利云. 光子增减叠加相干态在热环境中的退相干. , 2013, 62(1): 014208. doi: 10.7498/aps.62.014208
[10]	赵虎, 李铁夫, 刘建设, 陈炜. 基于超导量子比特的电磁感应透明研究进展. , 2012, 61(15): 154214. doi: 10.7498/aps.61.154214
[11]	赵文垒, 王建忠, 豆福全. 混沌微扰导致的量子退相干. , 2012, 61(24): 240302. doi: 10.7498/aps.61.240302
[12]	冯志刚, 张好, 张临杰, 李昌勇, 赵建明, 贾锁堂. 超冷铯Rydberg原子寿命的测量. , 2011, 60(7): 073202. doi: 10.7498/aps.60.073202
[13]	陈依新, 沈光地, 韩金茹, 李建军, 郭伟玲. 不同表面结构的半导体发光二极管的效率与寿命的研究. , 2010, 59(1): 545-549. doi: 10.7498/aps.59.545
[14]	王小霞, 廖显恒, 罗积润, 赵青兰, 张晓伟. 新型贮存式氧化物阴极寿命机理的初步探讨. , 2009, 58(2): 1280-1286. doi: 10.7498/aps.58.1280
[15]	额尔敦朝鲁, 于若蒙. 非对称量子点中磁极化子性质的磁场和温度依赖性. , 2008, 57(11): 7100-7107. doi: 10.7498/aps.57.7100
[16]	刘绍鼎, 程木田, 周慧君, 李耀义, 王取泉, 薛其坤. 双激子和浸润层泄漏以及俄歇俘获对量子点Rabi振荡衰减的影响. , 2006, 55(5): 2122-2127. doi: 10.7498/aps.55.2122
[17]	谷娟, 梁九卿. 施主中心量子点能谱分析. , 2005, 54(11): 5335-5338. doi: 10.7498/aps.54.5335
[18]	王丽, 胡响明. 耦合场线宽：抑制电磁诱导吸收. , 2004, 53(8): 2551-2555. doi: 10.7498/aps.53.2551
[19]	鲁　欣, 奚婷婷, 李英竣, 张　杰. 超短超强脉冲激光在空气中产生的电离通道的寿命研究. , 2004, 53(10): 3404-3408. doi: 10.7498/aps.53.3404
[20]	肖春涛, 韩立安, 薛德胜, 赵俊慧, H.Kunkel, G.Williams. La0.67Pb0.33MnO3的磁性及输运特性. , 2003, 52(5): 1245-1249. doi: 10.7498/aps.52.1245

计量

文章访问数: 6013
PDF下载量: 49
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

电磁场中施主中心量子点内磁极化子态寿命与qubit退相干