旋量玻色-爱因斯坦凝聚体拓扑性质的研究进展

王力; 刘静思; 李吉; 周晓林; 陈向荣; 刘超飞; 刘伍明

doi:10.7498/aps.69.20191648

摘要

实现玻色-爱因斯坦凝聚的原子大多具备内部自旋自由度, 在光势阱下原子内部自旋被解冻, 从而使原子可以凝聚到各个超精细量子态上, 形成旋量玻色-爱因斯坦凝聚体. 灵活的自旋自由度成为体系相关的动力学变量, 可以使体系出现新奇的拓扑量子态, 如自旋畴壁、涡旋、磁单极子、斯格明子等. 本文综述了旋量玻色-爱因斯坦凝聚的实验和理论研究, 旋量玻色-爱因斯坦凝聚体中拓扑缺陷的种类, 以及两分量、三分量玻色-爱因斯坦凝聚体中拓扑缺陷的研究进展.

关键词:

Abstract

Most of the atoms that realize Bose-Einstein condensation have internal spin degree of freedom. In the optical potential trap, the internal spin of the atom is thawed, and the atom can be condensed into each hyperfine quantum state to form the spinor Bose-Einstein condensate. Flexible spin degrees of freedom become dynamic variables related to the system, which can make the system appear novel topological quantum states, such as spin domain wall, vortex, magnetic monopole, skymion, and so on. In this paper, the experimental and theoretical study of spinor Bose-Einstein condensation, the types of topological defects in spinor Bose-Einstein condensate, and the research progress of topological defects in spinor two-component and three-component Bose-Einstein condensate are reviewed.

Keywords:

作者及机构信息

1.
四川大学物理学院, 成都　610065

2.
北京景山学校朝阳学校, 北京　100012

3.
太原师范学院物理系, 晋中　030619

4.
四川师范大学物理与电子工程学院, 成都　610101

5.
江西理工大学理学院, 赣州　341000

6.
中国科学院物理研究所, 北京凝聚态物理国家实验室, 北京　100190

7.
中国科学院大学物理学院, 北京　100190

通信作者: 刘超飞, liuchaofei0809@163.com ; 刘伍明, wmliu@iphy.ac.cn

基金项目: 国家重点研发计划“量子调控与量子信息”重点专项(批准号: 2016YFA0301500)和国家自然科学基金(批准号: 11434015, 61835013, 11875149, 61565007)资助的课题

Authors and contacts

1.
College of Physics, Sichuan University, Chengdu 610065, China

2.
Beijing Jingshan School Chaoyang Branch School, Beijing 100012, China

3.
College of Physics, Taiyuan Normal University, Jinzhong, 030619, China

4.
School of Physics and Electronic engineering, Sichuan Normal University, Chengdu 610101, China

5.
School of Science, Jiangxi University of Science and Technology, Ganzhou 341000, China

6.
Beijing National Laboratory for Condensed Matter Physics, Institute of Physics, Chinese Academy of Sciences, Beijing 100190, China

7.
School of Physical Sciences, University of Chinese Academy of Sciences, Beijing 100190, China

Corresponding author: Liu Chao-Fei, liuchaofei0809@163.com ; Liu Wu-Ming, wmliu@iphy.ac.cn

Funds: Project supported by the NKRDP, China (Grant No. 2016YFA0301500) and the National Natural Science Foundation of China (Grant Nos. 11434015, 61835013, 11875149, 61565007)

文章全文 : translate this paragraph

参考文献

[1]	Coen S, Haelterman M 2001 Phys. Rev. Lett. 87 140401 Google Scholar
[2]	Williams J E, Holland M J 1999 Nature 401 568 Google Scholar
[3]	Abo-Shaeer J R, Raman C, Vogels J M, Ketterle W 2001 Science 292 476 Google Scholar
[4]	Leanhardt A E, Shin Y, Kielpinski D, Pritchard D E, Ketterle W 2003 Phys. Rev. Lett. 90 140403 Google Scholar
[5]	Sadler L E, Higbie J M, Leslie S R, Vengalattore M, Stamper-Kurn D M 2006 Nature 443 312 Google Scholar
[6]	Alan L M, John V P, William D P 1985 Phys. Rev. Lett. 54 2596 Google Scholar
[7]	Reichel J, Hansel W, Hansch T W 1999 Phys. Rev. Lett. 83 3398 Google Scholar
[8]	Wolfgang P, Michael H A, Jason R E 1995 Phys. Rev. Lett. 74 3352 Google Scholar
[9]	Pethick C, Smith H 2008 Bose-Einstein Condensation in Dilute Gases (UK: Cambridge Univ. Press) p569-584
[10]	Pitaevskii L, Stringari S 2002 Bose-Einstein Condensation(Oxford: Clarendon Press)p382-395
[11]	Stenger J, Stamper-Kurn D M, Andrews M R, Chikkatur A P, Inouye S, Miesner H J, Ketterle W 1998 J. Low Temp. Phys. 113 167 Google Scholar
[12]	Bloch I, Dali bard J, Zwerger W 2008 Rev. Mod. Phys. 80 885 Google Scholar
[13]	Stenger J, Inouye S, Stamper-Kurn D M, Miesner H-J, Chikkatur A P, Ketterle W 1988 Nature 396 345
[14]	Kawaguchi Y, Ueda M 2012 Phys. Rep. 520 253 Google Scholar
[15]	Weiler C N, Neely T W, Scherer D R, Bradley A S, Davis M J, Anderson B P 2008 Nature 455 948
[16]	Stamper-Kurn D M, Andrews M R, Chikkatur A P, Inouye S, Miesner H-J, Stenger J, Ketterle W 1998 Phys. Rev. Lett. 80 2027 Google Scholar
[17]	Barrett M D, Sauer J A, Chapman M S 2001 Phys. Rev. Lett. 87 010404 Google Scholar
[18]	Gustavson T L, leanhardt A E, Chikkatur A P 2003 Phys. Rev. Lett. 90 090401 Google Scholar
[19]	Chang M S, Hamley C D, Barrett M D 2004 Phys. Rev. Lett. 92 140403 Google Scholar
[20]	Schmaljohann H, Erhard M, Kronjager J 2004 Phys. Rev. Lett. 92 040402 Google Scholar
[21]	Kuwamoto T, Araki K, Eno T 2004 Phys. Rev. A 69 063604 Google Scholar
[22]	Pasquiou B, Marechal E, Vernac L 2012 Phys. Rev. Lett. 108 045307 Google Scholar
[23]	Lin Y J, Jimenez G K, Spielman I B 2011 Nature 471 83
[24]	Galitshi V, Spielman I B 2013 Nature 494 49
[25]	Dalibard J, Gerbier F, Juzeliunas G, Ohberg P 2011 Rev. Mod. Phys. 83 1523 Google Scholar
[26]	Zhai H 2012 Int. J. Mod. Phys. B 26 1230001 Google Scholar
[27]	Goldman N, Juzeliunas G, Ohberg P, Spielman I B 2014 Rep. Prog. Phys. 77 126401 Google Scholar
[28]	Zhang J Y, Ji S C, Chen Z, Zhang L, Du Z D, Yan B, Pan G S, Zhao B 2012 Phys. Rev. Lett. 109 115301 Google Scholar
[29]	Wang P J, Yu Z Q, Fu Z K, Miao J, Huang L H 2012 Phys. Rev. Lett. 109 095301 Google Scholar
[30]	Cheuk L W, Sommer A T, Hadzibabic Z, Yefsah T, Bakr W S, Zwierlein M W 2012 Phys. Rev. Lett. 109 095302 Google Scholar
[31]	Liao R, Huang Z G, Lin X M, Fialko O 2014 Phys. Rev. A 89 063614 Google Scholar
[32]	Bhat I A, Mithun T, Malomed B A, Porsezian K 2015 Phys. Rev. A 92 063606 Google Scholar
[33]	Hu F Q, Wang J J, Yu Z F, Zhang A X, Xue J K 2016 Phys. Rev. E 93 022214 Google Scholar
[34]	Li Y, Pitaevskii L P, Stringari S 2012 Phys. Rev. Lett. 108 225301 Google Scholar
[35]	Qu C, Hamner C, Gong M, Zhang C, Engels P 2013 Phys. Rev. A 88 021604 Google Scholar
[36]	Leblanc L J, Beeler M C, Garcia K J, Perry A R, Sugawa S, Williams R A, Spielman I B 2013 New J. Phys. 15 073011 Google Scholar
[37]	Beeler M C, Williams R A, Garcia K J, LeBlanc L J, Perry A R, Spielman I B 2013 Nature 498 201
[38]	Kennedy C J, Siviloglou G A, Miyake H, Burton W C, Ketterle W 2013 Phys. Rev. Lett. 111 225301 Google Scholar
[39]	Liu X J, Law K T, Ng T K 2014 Phys. Rev. Lett. 112 086401 Google Scholar
[40]	Gong M, Tewari S, Zhang C 2011 Phys. Rev. Lett. 107 195303 Google Scholar
[41]	Ho T L 1988 Phys. Rev. Lett. 81 742
[42]	Ohmi T, Machida K 1998 J. Phys. Soc. Jpn. 67 1822 Google Scholar
[43]	Law C K, Pu H, Bigelow N P 1998 Phys. Rev. Lett. 81 5257 Google Scholar
[44]	Koashi M, Ueda M 2000 Phys. Rev. Lett. 84 1066 Google Scholar
[45]	Ueda M, Koashi M 2002 Phys. Rev. A 65 063602 Google Scholar
[46]	Ciobanu C V, Yip S K, Ho T L 2000 Phys. Rev. A 61 033607 Google Scholar
[47]	Zhou F, Semenoff G W 2006 Phys. Rev. Lett. 97 180411 Google Scholar
[48]	Santos L, Pfau T 2006 Phys. Rev. Lett. 96 190404 Google Scholar
[49]	Diener R B, Ho T L 2006 Phys. Rev. Lett. 96 190405 Google Scholar
[50]	Makela H, Suominen K A 2007 Phys. Rev. A 75 033610 Google Scholar
[51]	Yip S K 2007 Phys. Rev. A 75 023625 Google Scholar
[52]	李吉 2018 博士学位论文 (北京: 中国科学院大学) Li J 2018 Ph.D. Dissertation (Beijing: Chinese Academy of Sciences) (in Chinese)
[53]	靳晶晶 2014 博士学位论文 (太原: 山西大学) Jin J J 2014 Ph. D. Dissertation (Taiyuan: Shanxi University) (in Chinese)
[54]	Modugno G, Modugno M, Riboli F, Roati G, Inguscio M 2002 Phys. Rev. Lett. 89 19040
[55]	Papp S B, Pino J M, Wieman C E 2008 Phys. Rev. Lett. 101 040402 Google Scholar
[56]	Schweikhard V, Coddington I, Engels P, Tung S, Cornell E A 2004 Phys. Rev. Lett. 93 210403 Google Scholar
[57]	Leslie L S, Hansen A, Wright K C, Deutsch B M, Bigelow N P 2009 Phys. Rev. Lett. 103 250401 Google Scholar
[58]	Matthews M R, Anderson B P, Haljan P C, Hall D S, Wieman C E, Cornell E A 1999 Phys. Rev. Lett. 83 2498 Google Scholar
[59]	Zhou F 2001 Phys. Rev. Lett. 87 080401 Google Scholar
[60]	Yip S K 1999 Phys. Rev. Lett. 83 4677 Google Scholar
[61]	Leonhardt U, Volovik G E 2000 JETP Lett. 72 46 Google Scholar
[62]	Isoshima T, Machida K, Ohmi T 2001 J. Phys. Soc. Jpn. 70 1604 Google Scholar
[63]	Makela H, Zhang Y, Suominen K A 2003 J. Phys. A: Math. Gen. 36 8555
[64]	Semeno G W, Zhou F 2007 Phys. Rev. Lett. 98 100401 Google Scholar
[65]	Kobayashi M, Kawaguchi Y, Nitta M, Ueda M 2009 Phys. Rev. Lett. 103 115301 Google Scholar
[66]	Stoof H T C, Vliegen E, Khawaja U A 2001 Phys. Rev. Lett. 87 120407 Google Scholar
[67]	Blaha S 1976 Phys. Rev. Lett. 36 874 Google Scholar
[68]	Ruostekoshi J, Anglin J R 2003 Phys. Rev. Lett. 91 190402 Google Scholar
[69]	Shankar R 1977 J. Phys. 38 1405 Google Scholar
[70]	Volovik G E, Mineev V P 1976 Pis'ma Zh. Eksp. Teor. Fiz. 23 647
[71]	Khawaja U A, Stoof H 2001 Nature 411 918 Google Scholar
[72]	Kawaguchi Y, Nitta M, Ue da 2008 Phys. Rev. Lett. 100 180403 Google Scholar
[73]	Jin J J, Zhang S Y, Han W 2011 J. Phys. B: At. Mol. Opt. Phys. 44 165302 Google Scholar
[74]	刘静思 2017 博士学位论文 (北京: 中国科学院大学) Liu J S 2017 Ph.D. Dissertation (Beijing: Chinese Academy of Sciences) (in Chinese)
[75]	Eto M, Kasamatsu K, Nitta M, Taeuchi H, Tsubota M 2011 Phys. Rev. A 83 063603 Google Scholar
[76]	Volovik G E 2000 Proc. Natl. Acad. Sci. USA 97 2431
[77]	Liu C F, Liu W M 2017 Opt. Exp. 25 32800 Google Scholar
[78]	Huhtamaki J A M, Simula T P, Kobayashi M 2009 Phys. Rev. A 80 051601
[79]	Fert A, Cros V, Sampaio J 2013 Nature Nanotech. 8 152 Google Scholar
[80]	Ray M W, Ruokokoski E, Kandel S, Möttönen M, Hall D S 2014 Nature 505 657 Google Scholar
[81]	Ray M W, Ruokokoski E, Tiurev K, Möttönen M, Hall D S 2015 Science 348 544 Google Scholar
[82]	Ruostekoski J, Anglin J R 2001 Phys. Rev. Lett. 86 3934 Google Scholar
[83]	Kawakami T, Mizushima T, Nitta M, Machida K 2012 Phys. Rev. Lett. 109 015301 Google Scholar
[84]	Stamper-Kurn D M, Ueda M 2013 Rev. Mod. Phys. 85 1191
[85]	Choi J Y, kwon W J, Shin Y I 2012 Phys. Rev. Lett. 108 035301
[86]	Hall D S, Matthews M R, Ensher J R, Wieman C E, Cornell E A 1998 Phys. Rev. Lett. 81 1539 Google Scholar
[87]	Madison K W, Chevy F, Wohlleben W, Dalibard J 2000 Phys. Rev. Lett. 84 806
[88]	Anderson B P, Haljan P C, Regal C A, Feder D L, Collins L A, Clark C W, Cornell E A 2001 Phys. Rev. Lett. 86 2926 Google Scholar
[89]	Hall D S, Ray M W, Tiurev K, Ruokokoski E, Gheorghe A H, Möttönen M 2016 Nat. Phys. 12 478 Google Scholar
[90]	Leanhardt A E, Gorlitz A, Chikkatur A P 1999 Phys. Rev. Lett. 89 190403
[91]	Battye R A, Cooper N R, Sutcliffe P M 2002 Phys. Rev. Lett. 88 080401 Google Scholar
[92]	Martikainen J P, Collin A, Suominen K A 2002 Phys. Rev. Lett. 88 090404 Google Scholar
[93]	Kasamatsu K, Tsubota M 2004 Phys. Rev. Lett. 93 100402 Google Scholar
[94]	Wang C J, Cao C, Jian C M, Zhai H 2010 Phys. Rev. Lett. 105 160403 Google Scholar
[95]	Sinha S, Nath R, Santos L 2011 Phys. Rev. Lett. 107 270401 Google Scholar
[96]	Hu H, Ramachandhran B, Pu H, Liu X J 2012 Phys. Rev. Lett. 108 010402 Google Scholar
[97]	Xu X Q, Han J H 2011 Phys. Rev. Lett. 107 200401 Google Scholar
[98]	Zhou X F, Zhou J, Wu C J 2011 Phys. Rev. A 84 063624 Google Scholar
[99]	Liu C F, Fan H, Zhang Y C, Wang D S, Liu W M 2012 Phys. Rev. A 86 053616 Google Scholar
[100]	Wang X, Tan R B, Du Z J, Zhao W Y, Zhang X F, Zhang S G 2014 Chin. Phys. B 23 070308 Google Scholar
[101]	Fetter A L 2014 Phys. Rev. A 89 023629 Google Scholar
[102]	Sakaguchi H, Umeda K 2016 J. Phys. Soc. Jpn. 85 064402 Google Scholar
[103]	Sakaguchi H 2017 Phys. Rev. A 96 043620 Google Scholar
[104]	Wang H, Wen L H, Yang H, Shi C X, Li J H 2017 J. Phys. B: At. Mol. Opt. Phys. 50 155301 Google Scholar
[105]	Kato M, Zhang X F, Saito H 2017 Phys. Rev. A 95 043605 Google Scholar
[106]	Shi C X, Wen L H, Wang Q B, Yang H, Wang H 2018 J. Phys. Soc. Jpn. 87 094003 Google Scholar
[107]	李吉, 刘伍明 2018 67 110302 Google Scholar Li J, Liu W M 2018 Acta Phys. Sin. 67 110302 Google Scholar
[108]	Pu H, Raghavan S, Bigelow N P 2001 Phys. Rev. A 63 063603 Google Scholar
[109]	Ogawa S I, Möttöen M, Nakahara M, Ohmi T, Shimada H 2002 Phys. Rev. A 66 013617 Google Scholar
[110]	Itin A P, Morishita T, Satoh M, Tolstikhin O I, Watanabe S 2006 Phys. Rev. A 73 063615 Google Scholar
[111]	Isoshima T, Machida K 2002 Phys. Rev. A 66 053610 Google Scholar
[112]	Mizushima T, Machida K, Kita T 2002 Phys. Rev. Lett. 89 030401 Google Scholar
[113]	Saito H, Kawaguchi Y, Ueda M 2006 Phys. Rev. Lett. 96 065302 Google Scholar
[114]	Saito H, Kawaguchi Y, Ueda M 2007 Phys. Rev. A 75 013621
[115]	Turner A M 2009 Phys. Rev. Lett. 103 080603 Google Scholar
[116]	Pietila V, Möttönen M, Virtanen S M 2007 Phys. Rev. A 76 023610 Google Scholar
[117]	Ji A C, Liu W M, Song J L, Zhou F 2008 Phys. Rev. Lett. 101 010402 Google Scholar
[118]	Liu C F, Liu W M 2012 Phys. Rev. A 86 033602 Google Scholar
[119]	刘超飞万文娟张赣源 2013 62 200306 Google Scholar Liu C F, Wan W J, Zhang G Y 2013 Acta Phys. Sin. 62 200306 Google Scholar
[120]	Song S W, Zhang Y C, Zhao H, Wang Xuan, Liu W M 2014 Phys. Rev. A 89 063613 Google Scholar
[121]	Lovegrove J, Borgh M O, Ruostekoski 2014 Phys. Rev. Lett. 112 075301 Google Scholar
[122]	Zhao D, Song S W, Wen L, Li Z D, Luo H G, Liu W M 2015 Phys. Rev. A 91 013619 Google Scholar
[123]	Gautam S, Adhikari S K 2016 Phys. Rev. A 93 013630 Google Scholar
[124]	刘静思李吉刘伍明 2017 66 130305 Google Scholar Liu J S, Li J, Liu W M 2017 Acta Phys. Sin. 66 130305 Google Scholar
[125]	Li J, Yu Y M, Zhuang L, Liu W M 2017 Phys. Rev. A 95 043633 Google Scholar

施引文献

图 1 光势阱中F = 1 ²³Na凝聚体的超精细态^[16]. (a) 250 ms时光势阱中钠原子的吸收图像; (b) 340 ms时光势阱中钠原子的吸收图像

Fig. 1. Optical trapping of ²³Na condensates in all F = 1 hyperfine states: shown are absorption images after (a) 250 ms and (b) 340 ms of optical confinement.

下载: 全尺寸图片幻灯片

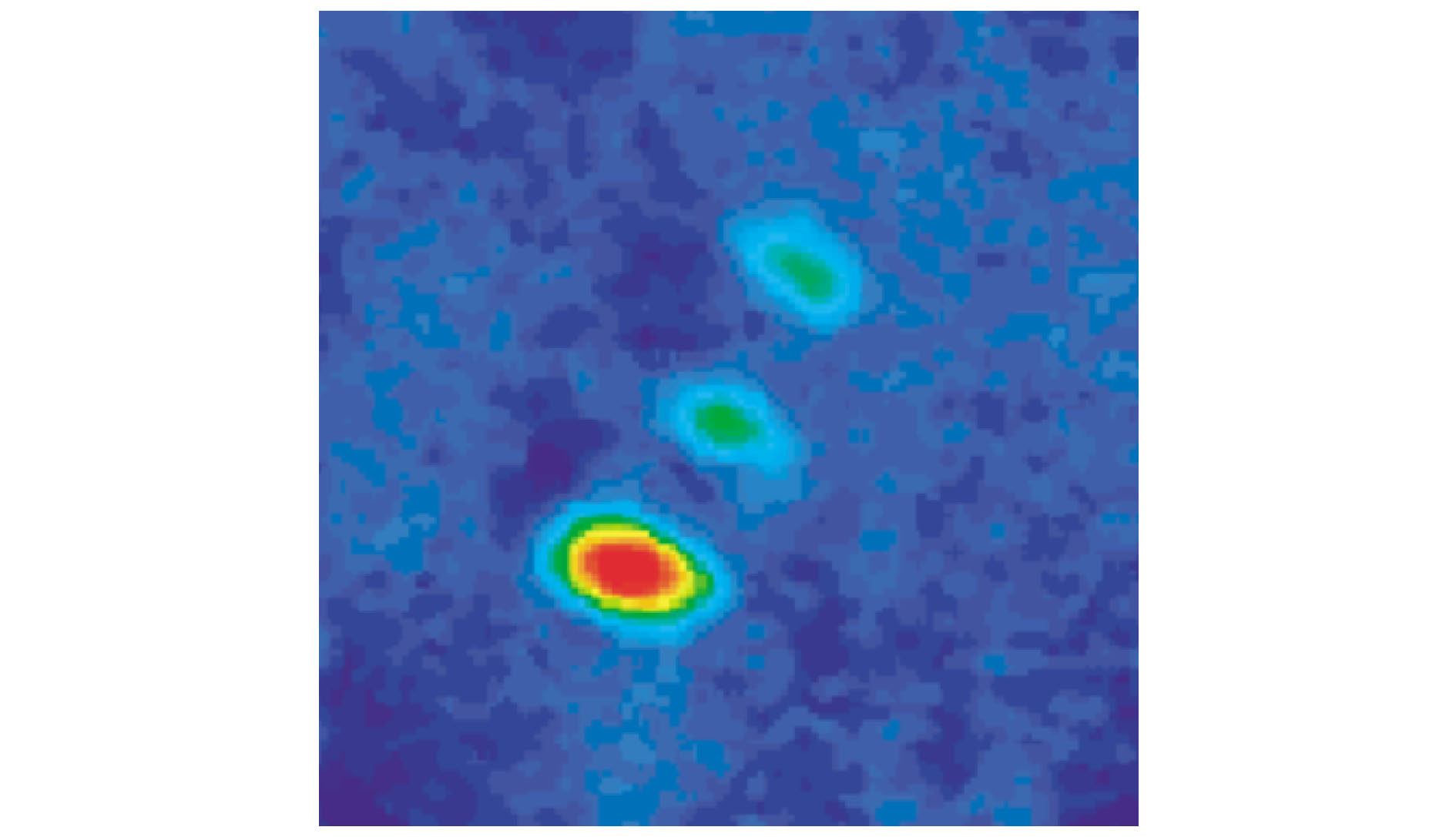
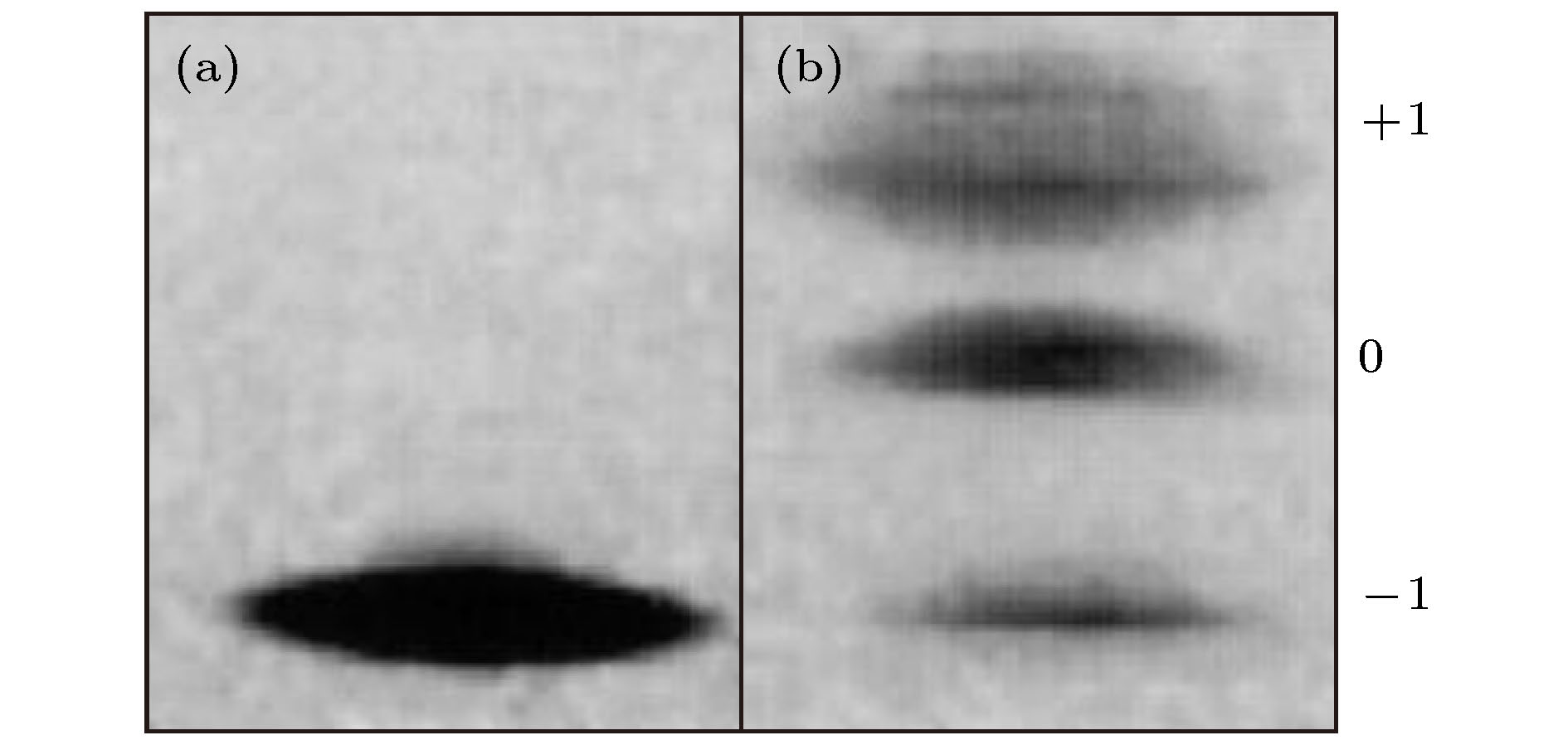
图 2 铷原子云在Stern-Gerlach梯度磁场中自由膨胀10 ms后的吸收图像^[17]. 从下到上分别是F = 1, m_F = (–1, 0, 1)凝聚体的三个分量

Fig. 2. Absorptive image of Rb atomic cloud after 10 ms free expansion in a Stern-Gerlach magnetic field gradient. Three distinct components are observed corresponding to F = 1, m_F = (–1, 0, 1) spin projections from bottom to top, respectively.

下载: 全尺寸图片幻灯片

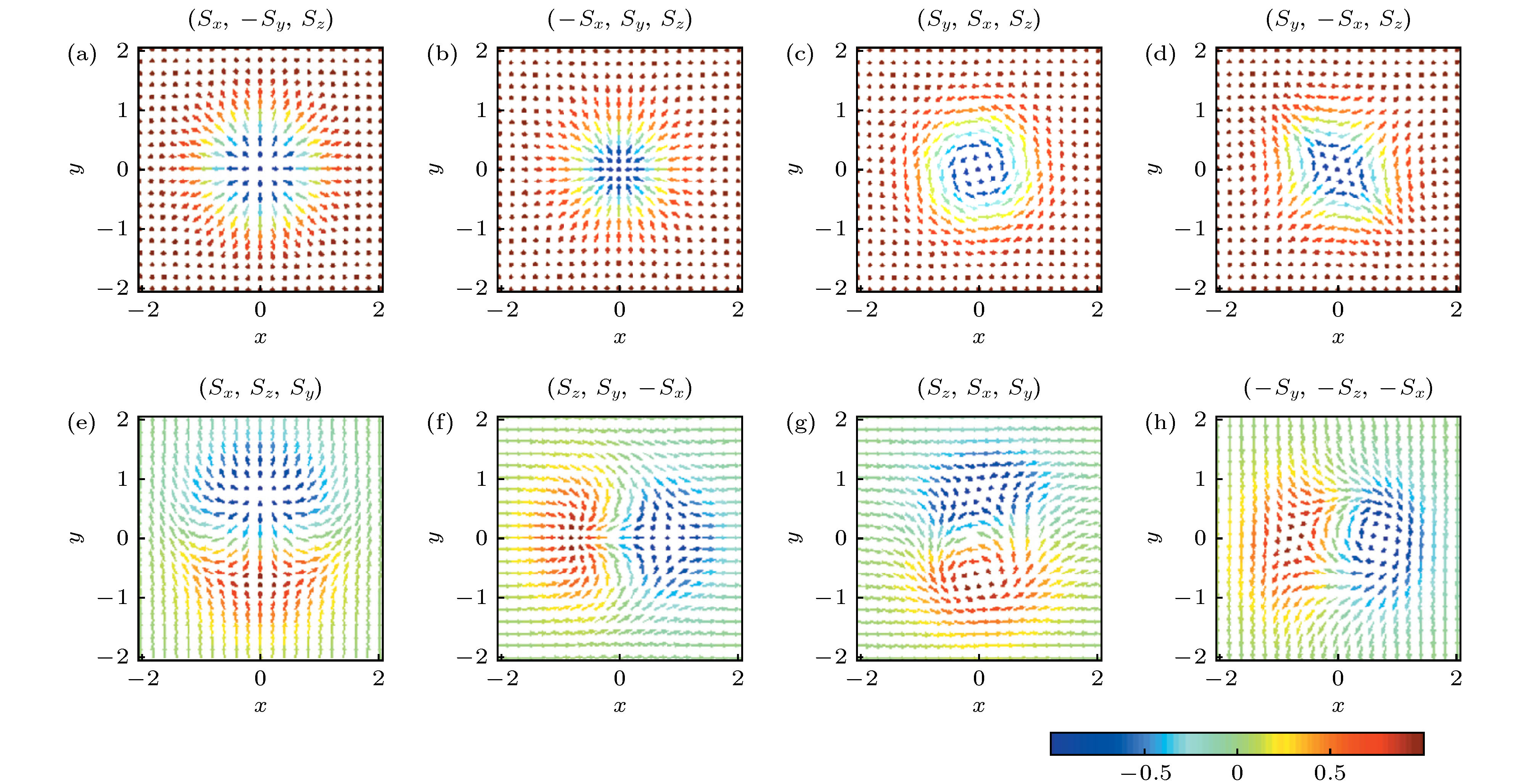
图 3 赝自旋密度S_z, S_x, S_y的空间分布^[73]　(a)−(c)表示旋转角频率为0; (d)自旋纹理投影到x-y平面内的矢量表示

Fig. 3. The pseudospin density distribution for (a) S_z, (b) S_x and (c) S_y for Ω = 0; (d) the vectorial representation of the spin texture projected onto the x-y plane.

下载: 全尺寸图片幻灯片

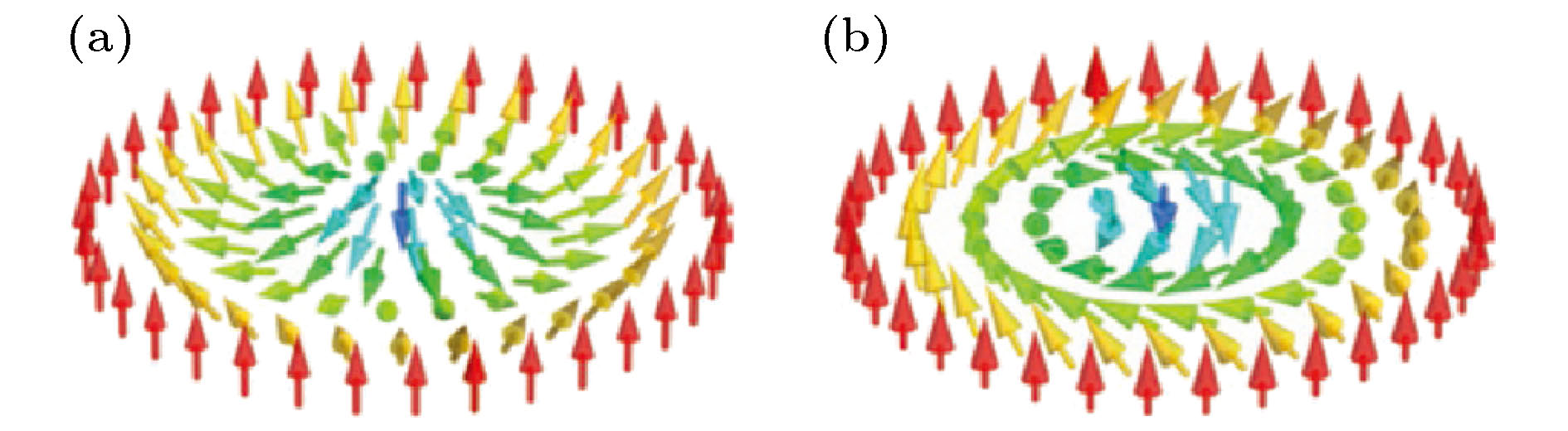
图 4 自旋1 BEC中半量子涡旋的近似解和相应的奇异自旋纹理^[77]　(a)和(b)对应$\left| {F = 1, {{{m}}_{\rm F}} = 0} \right\rangle $和$\left| {F = 1, {{{m}}_{\rm F}} = - 1} \right\rangle $分量的密度; (c)和(d)是对应的相; (e)为半量子涡旋的分布; (f)|S|自旋密度; (g) |S|自旋密度分布; (h)自旋纹理; (i)拓扑荷密度$q\left( {x, y} \right)$

Fig. 4. Approximate half-quantum vortex solution in the spin-1 BEC and the corresponding singular spin texture: (a) and (b) are the densities of the $\left| {F = 1, {m_{\rm F}} = 0} \right\rangle $和$\left| {F = 1, {m_F} = - 1} \right\rangle $ components, respectively; (c) and (d) are the corresponding phases; (e) shows the profile of the half-quantum vortex; (f) spin density|S|; (g) the profile of the spin density|S|; (h) spin texture; (i) topological charge density $q\left( {x, y} \right)$.

下载: 全尺寸图片幻灯片

图 5 两种常见的二维skyrmions的矢量场构型^[79]　(a) 豪猪型skyrmion; (b) 螺旋型skyrmion

Fig. 5. Two common vector field configurations of two-dimensional skyrmions: (a) The hedgehog type skyrmion; (b) the spiral type skyrmion.

下载: 全尺寸图片幻灯片

图 6 稳定的三维skyrmions在x-y和z-x平面的空间分布^[83]　(a)中的箭头和颜色分别表示贋自旋方向和OP的U(1)相分布. 彩图(b)和(c)分别表示$\left| {{\varPsi _ \uparrow }\left( {\rm{r}} \right)} \right|$和$\left| {{\Psi _ \downarrow }\left( {\rm{r}} \right)} \right|$的振幅

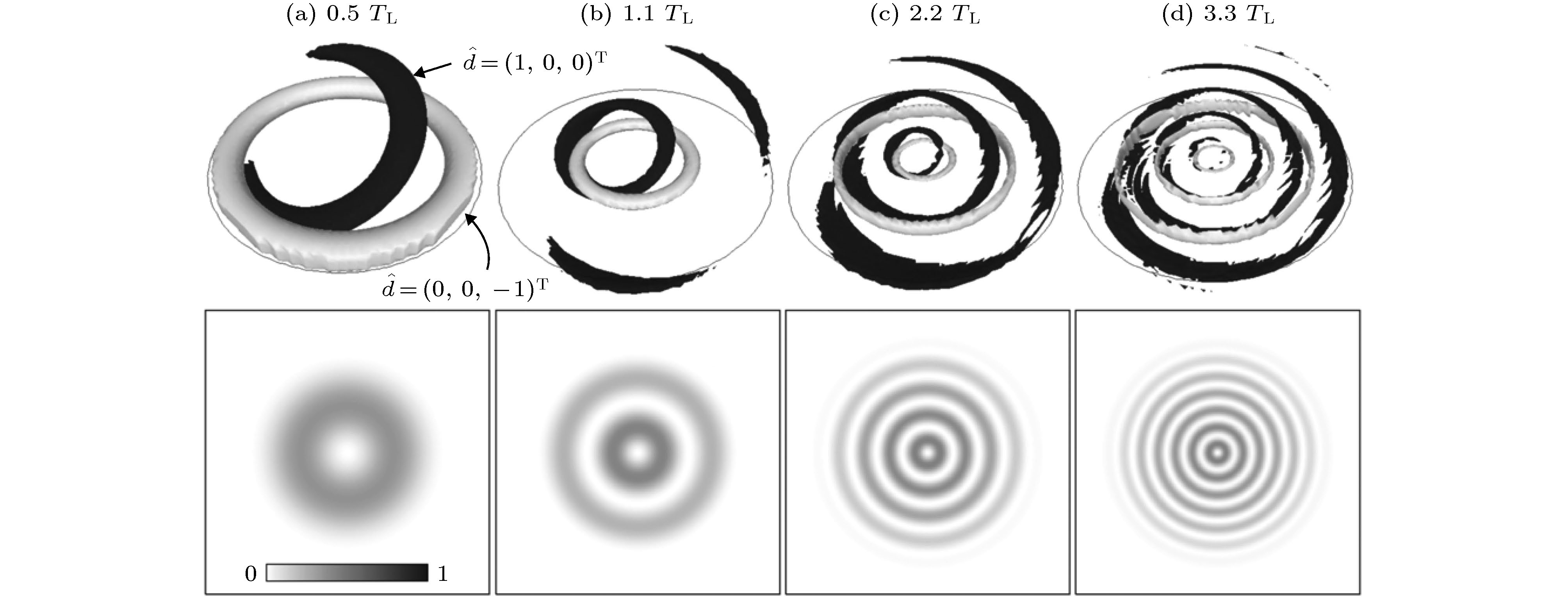
Fig. 6. The spatial profile of the stable 3D skyrmions in the x-y and z-x planes: The arrows and their colors in (a) indicate the pseudospin direction and the U(1) phase of the OP, respectively; the color maps of (b) and (c) give the amplitudes $\left| {{\varPsi _ \uparrow }\left( {\rm{r}} \right)} \right|$ and $\left| {{\varPsi _ \downarrow }\left( {\rm{r}} \right)} \right|$, respectively.

下载: 全尺寸图片幻灯片

图 7 四极场作用下球形光势阱中扭结产生的动力学过程^[85]. 上一行表示${{\hat d}} = {\left( {0, 0, - 1} \right)^{\rm{T}}}$和${{\hat d}}$ = (1, 0, 0)^T的图像快照, 下一行表示x-y平面上m = –1分量的密度截面

Fig. 7. Dynamics of the creation of knots in a spherical optical trap under a quadrupole magnetic field. Snapshots of the preimages of ${{\hat d}}$ = (0, 0, –1)^T and ${{\hat d}}$ = (1, 0, 0)^T(top), and the cross sections of the density for the m = –1 components on the x–y plane (bottom).

下载: 全尺寸图片幻灯片

图 8 扭结孤子的结构及其产生方法^[89]　(a)和(b)为扭结形成之前和形成过程中磁感应线的示意图, 绿色椭圆为对应的凝聚体; (c)和(d)显示扭结形成时, 最初的z方向的向列相矢量(黑色箭头)沿着局部磁场(青色线)的方向进动, 以实现最终的结构(彩色箭头). 灰色虚线表示d_z = 0, 白线表示孤子核(d_z = –1), 深灰色线表示体积V (d_z = 1)的边界; (e)表示实空间中扭结孤子的构型及其与S²中向列矢量${{\hat d}}$的关系

Fig. 8. Structure of the knot soliton and the method of its creation: Schematic magnetic field lines before (a) and during (b) the knot formation, with respect to the condensate (green ellipse); (c), (d) as the knot is tied, the initially z-pointing nematic vector (black arrows) precesses about the direction of the local magnetic field (cyan lines) to achieve the final configuration (coloured arrows); the dashed grey line shows where d_z = 0, the white line indicates the soliton core (d_z = –1), and the dark grey line defines the boundary of the volume V (d_z= 1); (e) the knot soliton configuration in real space and its relation to the nematic vector ${{\hat d}}$ in S² (inset).

下载: 全尺寸图片幻灯片

图 9 Skyrmions的类型(λ = 0.5)^[99]　(a)−(h)表示自旋矢量的模式: (a)径向-向外skyrmion, (b)径向-向内skyrmion, (c)环形skyrmion, (d)双曲skyrmion, (e)双曲-径向向外skyrmion, (f)双曲-径向向内skyrmion, (g)环形-双曲skyrmion-I, (h)环形-双曲skyrmion-II

Fig. 9. Configuration of the skyrmion where λ = 0.5: The (a)−(h) figures indicate the mode of the spin vectors: (a) radial-out skyrmion, (b) radial-in skyrmion, (c) circular skyrmion, (d) hyperbolic skyrmion, (e) hyperbolic-radial(out) skyrmion, (f) hyperbolic-radial (in) skyrmion, (g) circular-hyperbolic skyrmion-I, and (h) circular-hyperbolic skyrmion-II^[99].

下载: 全尺寸图片幻灯片

图 10 不同自旋-轨道耦合强度下梯度磁场中两分量⁸⁷RbBEC基态粒子数密度分布(第1、2列)和相位分布(第3、4列)^[107]　(a)−(d)的${\tilde {\rm{\kappa}} }$值分别为0, 0.2, 0.8, 2

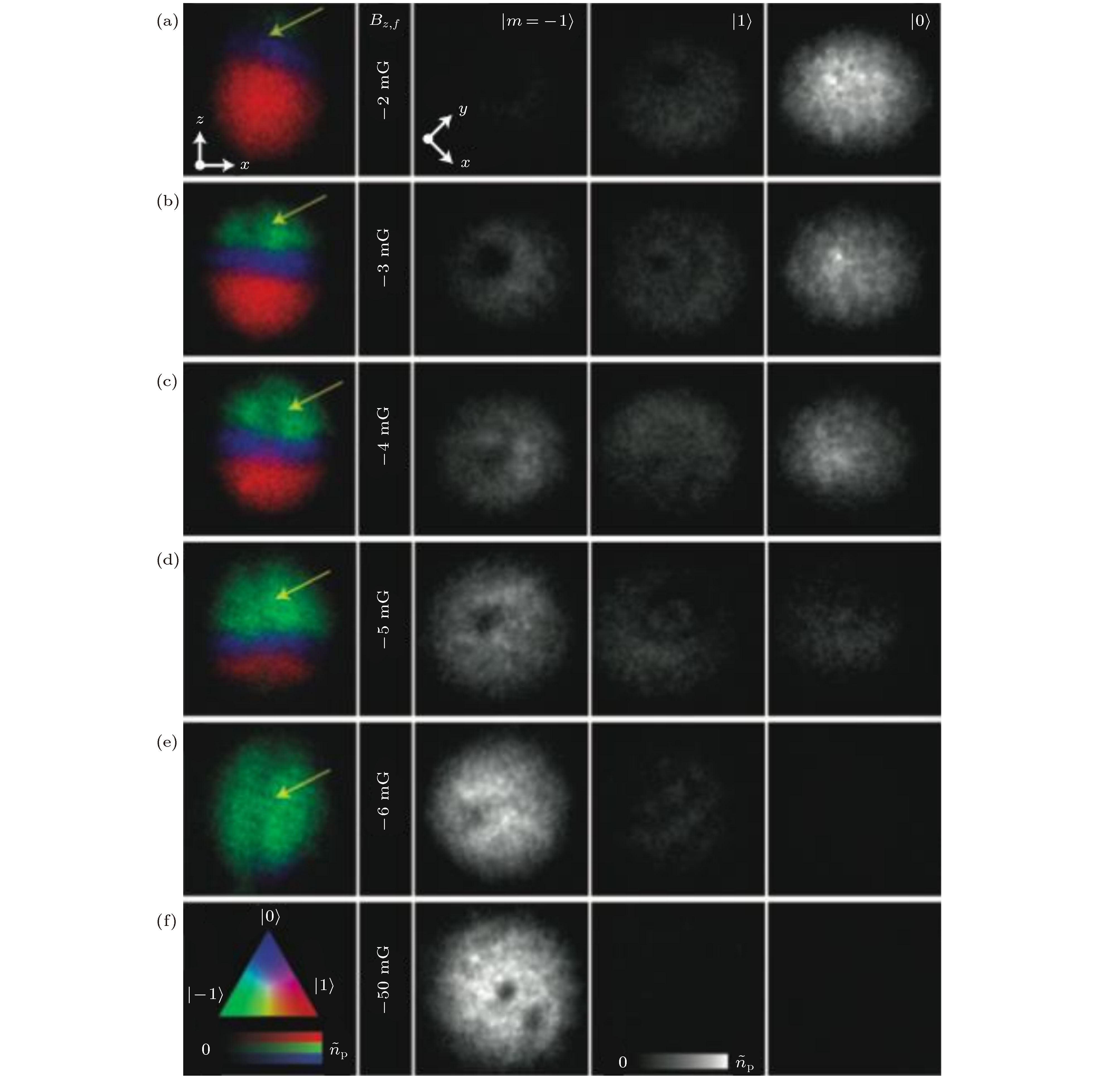
Fig. 10. Particle number densities (the first and second columns) and phase distributions (the third and fourth columns) of ground state of the two-component BEC of ⁸⁷Rb for the different spin-orbit coupling strengths: the parameters of ${\tilde {\rm{\kappa}} }$ in (a)−(d) are 0, 0.2, 0.8, 2, respectively^[107].

下载: 全尺寸图片幻灯片

图 11 涡旋的动力学形成^[110]. 涡旋形成于凝聚体的所有分量中, 在ψ_–1分量中占99%以上, 在ψ₀分量中动态涡旋和拓扑涡旋共存

Fig. 11. Dynamical formation of vortices: vortices are formed in all components, more than 99% of total population is in ψ_–₁ component. In the ψ₀ component, dynamical and topological vortices coexist^[110].

下载: 全尺寸图片幻灯片

图 12 狄拉克磁单极子的实验产生^[80]　(a)−(f)每一行都包含单个凝聚体的图像. 最左边的列显示了三种自旋状态$\left\{ {\left| 1 \right\rangle, \left| 0 \right\rangle, \left| { - 1} \right\rangle } \right\}$沿水平轴的柱状密度彩色图像; 最右边三列显示沿纵轴拍摄的图像

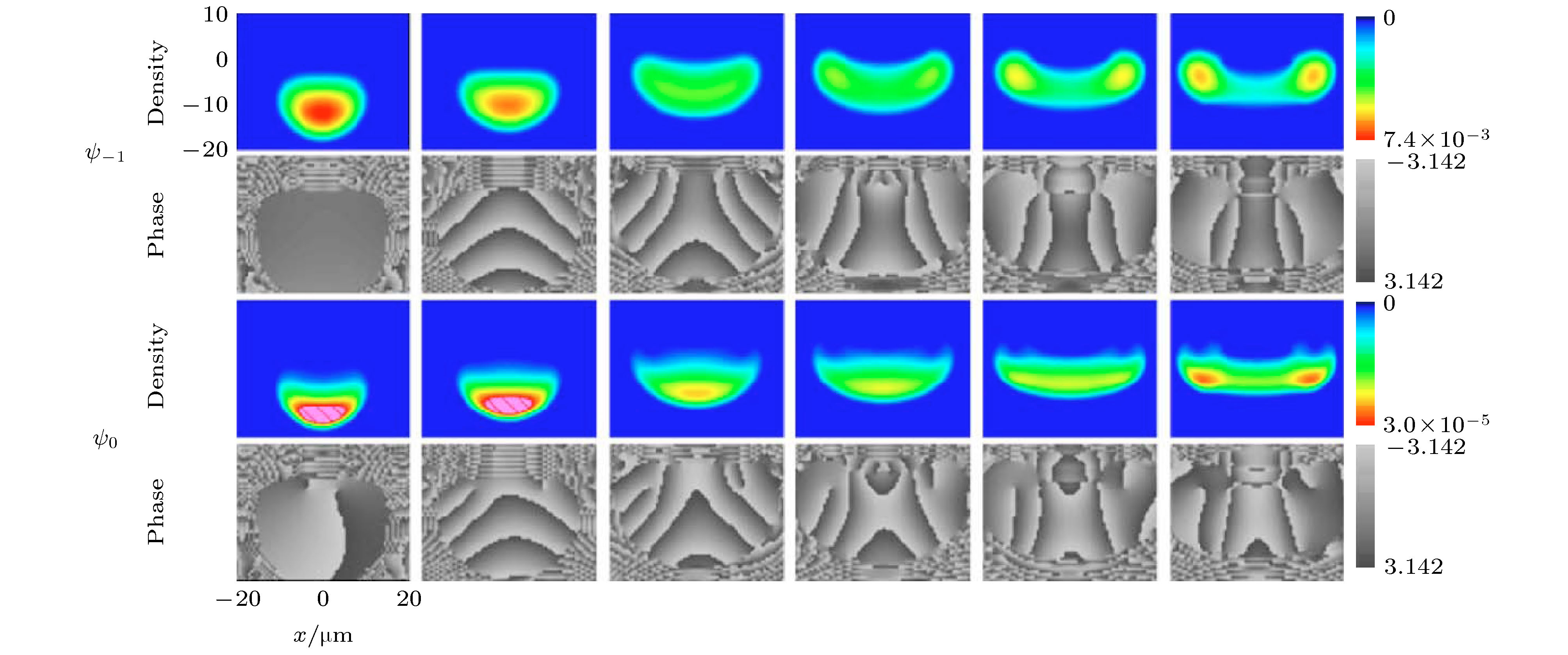
Fig. 12. Experimental creation of Dirac monopoles. Each row (a)−(f) contains images of an individual condensate. The leftmost column shows colour composite images of the column densities taken along the horizontal axis for the three spin states $\left\{ {\left| 1 \right\rangle, \left| 0 \right\rangle, \left| { - 1} \right\rangle } \right\}$; The rightmost three columns show images taken along the vertical axis^[80].

下载: 全尺寸图片幻灯片

图 13 旋转频率对²³Na旋量BEC的影响^[118], 其中${\mu _{j, 0}}\left( {j = 0, \pm 1} \right) = 3.6\;\hbar {\rm{\omega }}$, ${\text{μ}} = 25\;\hbar {\rm{\omega }}$, κ_x = κ_y = κ_z = 1, ${a_0} = 50\;{a_{\rm{B}}}$, and a₂ = 55 a_B　(a) Ω = 0; (b) Ω = 0.2 ω; (c) Ω = 0.5 ω. 第四列显示了相应的自旋纹理和涡旋的位置

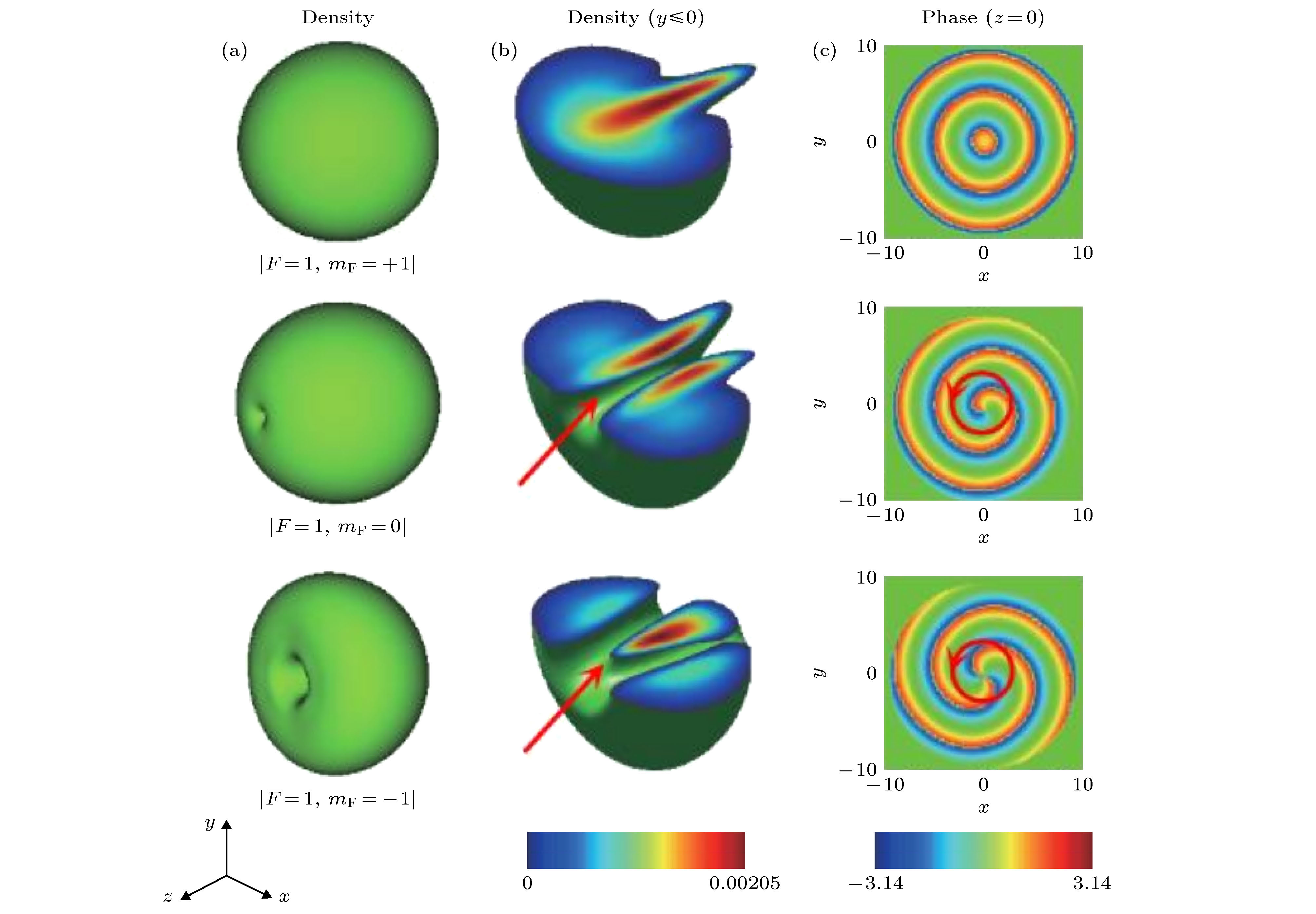
Fig. 13. The effect of rotation frequency for spinor BEC of ²³Na with ${\mu _{j, 0}}\left( {j = 0, \pm 1} \right) = 3.6\;\hbar {\rm{\omega }}$, ${\rm{\mu }} = 25\;\hbar {\rm{\omega }}$, κ_x = κ_y = κ_z = 1, ${a_0} = 50\;{a_{\rm{B}}}$, and a₂ = 55 a_B: (a) Ω = 0; (b) Ω = 0.2 ω; (c) Ω = 0.5 ω. The fourth column shows the corresponding spin textures and the positions of the vortices^[118].

下载: 全尺寸图片幻灯片

图 14 具有Mermin-Ho涡旋的磁单极子^[125]　(a)等值面的粒子数密度; (b)粒子数密度等深线段(y ≤ 0), 节点线(Dirac线)的位置用红色箭头突出显示; (c) z=0平面上的位相分布. 单涡旋(m_F = 0)和双涡旋(m_F = –1)具有相同的环流, 由红圈突出显示

Fig. 14. The monopoles with the Mermin-Ho vortex: (a) Isosurface of particle densities; (b) segments of isosurface of particle densities (y ≤ 0). the position of the nodal line (Dirac string) is highlighted by the red arrow; (c) phase distributions in the z = 0 planes. the single vortex (m_F = 0) and double vortex (m_F = –1) have the same circulations, as highlighted by the red circles^[125].

下载: 全尺寸图片幻灯片

表 1 同伦群描述的拓扑缺陷结构

Table 1. Topological defect structures described by homotopy groups.

π_n 缺陷孤子

π₀ 磁畴壁暗孤子
π₁ 涡旋非奇异磁畴壁
π₂ 磁单极二维skyrmions
π₃ skyrmions, 扭结
π₄ 瞬子

下载: 导出CSV

map

[1]	Coen S, Haelterman M 2001 Phys. Rev. Lett. 87 140401 Google Scholar
[2]	Williams J E, Holland M J 1999 Nature 401 568 Google Scholar
[3]	Abo-Shaeer J R, Raman C, Vogels J M, Ketterle W 2001 Science 292 476 Google Scholar
[4]	Leanhardt A E, Shin Y, Kielpinski D, Pritchard D E, Ketterle W 2003 Phys. Rev. Lett. 90 140403 Google Scholar
[5]	Sadler L E, Higbie J M, Leslie S R, Vengalattore M, Stamper-Kurn D M 2006 Nature 443 312 Google Scholar
[6]	Alan L M, John V P, William D P 1985 Phys. Rev. Lett. 54 2596 Google Scholar
[7]	Reichel J, Hansel W, Hansch T W 1999 Phys. Rev. Lett. 83 3398 Google Scholar
[8]	Wolfgang P, Michael H A, Jason R E 1995 Phys. Rev. Lett. 74 3352 Google Scholar
[9]	Pethick C, Smith H 2008 Bose-Einstein Condensation in Dilute Gases (UK: Cambridge Univ. Press) p569-584
[10]	Pitaevskii L, Stringari S 2002 Bose-Einstein Condensation(Oxford: Clarendon Press)p382-395
[11]	Stenger J, Stamper-Kurn D M, Andrews M R, Chikkatur A P, Inouye S, Miesner H J, Ketterle W 1998 J. Low Temp. Phys. 113 167 Google Scholar
[12]	Bloch I, Dali bard J, Zwerger W 2008 Rev. Mod. Phys. 80 885 Google Scholar
[13]	Stenger J, Inouye S, Stamper-Kurn D M, Miesner H-J, Chikkatur A P, Ketterle W 1988 Nature 396 345
[14]	Kawaguchi Y, Ueda M 2012 Phys. Rep. 520 253 Google Scholar
[15]	Weiler C N, Neely T W, Scherer D R, Bradley A S, Davis M J, Anderson B P 2008 Nature 455 948
[16]	Stamper-Kurn D M, Andrews M R, Chikkatur A P, Inouye S, Miesner H-J, Stenger J, Ketterle W 1998 Phys. Rev. Lett. 80 2027 Google Scholar
[17]	Barrett M D, Sauer J A, Chapman M S 2001 Phys. Rev. Lett. 87 010404 Google Scholar
[18]	Gustavson T L, leanhardt A E, Chikkatur A P 2003 Phys. Rev. Lett. 90 090401 Google Scholar
[19]	Chang M S, Hamley C D, Barrett M D 2004 Phys. Rev. Lett. 92 140403 Google Scholar
[20]	Schmaljohann H, Erhard M, Kronjager J 2004 Phys. Rev. Lett. 92 040402 Google Scholar
[21]	Kuwamoto T, Araki K, Eno T 2004 Phys. Rev. A 69 063604 Google Scholar
[22]	Pasquiou B, Marechal E, Vernac L 2012 Phys. Rev. Lett. 108 045307 Google Scholar
[23]	Lin Y J, Jimenez G K, Spielman I B 2011 Nature 471 83
[24]	Galitshi V, Spielman I B 2013 Nature 494 49
[25]	Dalibard J, Gerbier F, Juzeliunas G, Ohberg P 2011 Rev. Mod. Phys. 83 1523 Google Scholar
[26]	Zhai H 2012 Int. J. Mod. Phys. B 26 1230001 Google Scholar
[27]	Goldman N, Juzeliunas G, Ohberg P, Spielman I B 2014 Rep. Prog. Phys. 77 126401 Google Scholar
[28]	Zhang J Y, Ji S C, Chen Z, Zhang L, Du Z D, Yan B, Pan G S, Zhao B 2012 Phys. Rev. Lett. 109 115301 Google Scholar
[29]	Wang P J, Yu Z Q, Fu Z K, Miao J, Huang L H 2012 Phys. Rev. Lett. 109 095301 Google Scholar
[30]	Cheuk L W, Sommer A T, Hadzibabic Z, Yefsah T, Bakr W S, Zwierlein M W 2012 Phys. Rev. Lett. 109 095302 Google Scholar
[31]	Liao R, Huang Z G, Lin X M, Fialko O 2014 Phys. Rev. A 89 063614 Google Scholar
[32]	Bhat I A, Mithun T, Malomed B A, Porsezian K 2015 Phys. Rev. A 92 063606 Google Scholar
[33]	Hu F Q, Wang J J, Yu Z F, Zhang A X, Xue J K 2016 Phys. Rev. E 93 022214 Google Scholar
[34]	Li Y, Pitaevskii L P, Stringari S 2012 Phys. Rev. Lett. 108 225301 Google Scholar
[35]	Qu C, Hamner C, Gong M, Zhang C, Engels P 2013 Phys. Rev. A 88 021604 Google Scholar
[36]	Leblanc L J, Beeler M C, Garcia K J, Perry A R, Sugawa S, Williams R A, Spielman I B 2013 New J. Phys. 15 073011 Google Scholar
[37]	Beeler M C, Williams R A, Garcia K J, LeBlanc L J, Perry A R, Spielman I B 2013 Nature 498 201
[38]	Kennedy C J, Siviloglou G A, Miyake H, Burton W C, Ketterle W 2013 Phys. Rev. Lett. 111 225301 Google Scholar
[39]	Liu X J, Law K T, Ng T K 2014 Phys. Rev. Lett. 112 086401 Google Scholar
[40]	Gong M, Tewari S, Zhang C 2011 Phys. Rev. Lett. 107 195303 Google Scholar
[41]	Ho T L 1988 Phys. Rev. Lett. 81 742
[42]	Ohmi T, Machida K 1998 J. Phys. Soc. Jpn. 67 1822 Google Scholar
[43]	Law C K, Pu H, Bigelow N P 1998 Phys. Rev. Lett. 81 5257 Google Scholar
[44]	Koashi M, Ueda M 2000 Phys. Rev. Lett. 84 1066 Google Scholar
[45]	Ueda M, Koashi M 2002 Phys. Rev. A 65 063602 Google Scholar
[46]	Ciobanu C V, Yip S K, Ho T L 2000 Phys. Rev. A 61 033607 Google Scholar
[47]	Zhou F, Semenoff G W 2006 Phys. Rev. Lett. 97 180411 Google Scholar
[48]	Santos L, Pfau T 2006 Phys. Rev. Lett. 96 190404 Google Scholar
[49]	Diener R B, Ho T L 2006 Phys. Rev. Lett. 96 190405 Google Scholar
[50]	Makela H, Suominen K A 2007 Phys. Rev. A 75 033610 Google Scholar
[51]	Yip S K 2007 Phys. Rev. A 75 023625 Google Scholar
[52]	李吉 2018 博士学位论文 (北京: 中国科学院大学) Li J 2018 Ph.D. Dissertation (Beijing: Chinese Academy of Sciences) (in Chinese)
[53]	靳晶晶 2014 博士学位论文 (太原: 山西大学) Jin J J 2014 Ph. D. Dissertation (Taiyuan: Shanxi University) (in Chinese)
[54]	Modugno G, Modugno M, Riboli F, Roati G, Inguscio M 2002 Phys. Rev. Lett. 89 19040
[55]	Papp S B, Pino J M, Wieman C E 2008 Phys. Rev. Lett. 101 040402 Google Scholar
[56]	Schweikhard V, Coddington I, Engels P, Tung S, Cornell E A 2004 Phys. Rev. Lett. 93 210403 Google Scholar
[57]	Leslie L S, Hansen A, Wright K C, Deutsch B M, Bigelow N P 2009 Phys. Rev. Lett. 103 250401 Google Scholar
[58]	Matthews M R, Anderson B P, Haljan P C, Hall D S, Wieman C E, Cornell E A 1999 Phys. Rev. Lett. 83 2498 Google Scholar
[59]	Zhou F 2001 Phys. Rev. Lett. 87 080401 Google Scholar
[60]	Yip S K 1999 Phys. Rev. Lett. 83 4677 Google Scholar
[61]	Leonhardt U, Volovik G E 2000 JETP Lett. 72 46 Google Scholar
[62]	Isoshima T, Machida K, Ohmi T 2001 J. Phys. Soc. Jpn. 70 1604 Google Scholar
[63]	Makela H, Zhang Y, Suominen K A 2003 J. Phys. A: Math. Gen. 36 8555
[64]	Semeno G W, Zhou F 2007 Phys. Rev. Lett. 98 100401 Google Scholar
[65]	Kobayashi M, Kawaguchi Y, Nitta M, Ueda M 2009 Phys. Rev. Lett. 103 115301 Google Scholar
[66]	Stoof H T C, Vliegen E, Khawaja U A 2001 Phys. Rev. Lett. 87 120407 Google Scholar
[67]	Blaha S 1976 Phys. Rev. Lett. 36 874 Google Scholar
[68]	Ruostekoshi J, Anglin J R 2003 Phys. Rev. Lett. 91 190402 Google Scholar
[69]	Shankar R 1977 J. Phys. 38 1405 Google Scholar
[70]	Volovik G E, Mineev V P 1976 Pis'ma Zh. Eksp. Teor. Fiz. 23 647
[71]	Khawaja U A, Stoof H 2001 Nature 411 918 Google Scholar
[72]	Kawaguchi Y, Nitta M, Ue da 2008 Phys. Rev. Lett. 100 180403 Google Scholar
[73]	Jin J J, Zhang S Y, Han W 2011 J. Phys. B: At. Mol. Opt. Phys. 44 165302 Google Scholar
[74]	刘静思 2017 博士学位论文 (北京: 中国科学院大学) Liu J S 2017 Ph.D. Dissertation (Beijing: Chinese Academy of Sciences) (in Chinese)
[75]	Eto M, Kasamatsu K, Nitta M, Taeuchi H, Tsubota M 2011 Phys. Rev. A 83 063603 Google Scholar
[76]	Volovik G E 2000 Proc. Natl. Acad. Sci. USA 97 2431
[77]	Liu C F, Liu W M 2017 Opt. Exp. 25 32800 Google Scholar
[78]	Huhtamaki J A M, Simula T P, Kobayashi M 2009 Phys. Rev. A 80 051601
[79]	Fert A, Cros V, Sampaio J 2013 Nature Nanotech. 8 152 Google Scholar
[80]	Ray M W, Ruokokoski E, Kandel S, Möttönen M, Hall D S 2014 Nature 505 657 Google Scholar
[81]	Ray M W, Ruokokoski E, Tiurev K, Möttönen M, Hall D S 2015 Science 348 544 Google Scholar
[82]	Ruostekoski J, Anglin J R 2001 Phys. Rev. Lett. 86 3934 Google Scholar
[83]	Kawakami T, Mizushima T, Nitta M, Machida K 2012 Phys. Rev. Lett. 109 015301 Google Scholar
[84]	Stamper-Kurn D M, Ueda M 2013 Rev. Mod. Phys. 85 1191
[85]	Choi J Y, kwon W J, Shin Y I 2012 Phys. Rev. Lett. 108 035301
[86]	Hall D S, Matthews M R, Ensher J R, Wieman C E, Cornell E A 1998 Phys. Rev. Lett. 81 1539 Google Scholar
[87]	Madison K W, Chevy F, Wohlleben W, Dalibard J 2000 Phys. Rev. Lett. 84 806
[88]	Anderson B P, Haljan P C, Regal C A, Feder D L, Collins L A, Clark C W, Cornell E A 2001 Phys. Rev. Lett. 86 2926 Google Scholar
[89]	Hall D S, Ray M W, Tiurev K, Ruokokoski E, Gheorghe A H, Möttönen M 2016 Nat. Phys. 12 478 Google Scholar
[90]	Leanhardt A E, Gorlitz A, Chikkatur A P 1999 Phys. Rev. Lett. 89 190403
[91]	Battye R A, Cooper N R, Sutcliffe P M 2002 Phys. Rev. Lett. 88 080401 Google Scholar
[92]	Martikainen J P, Collin A, Suominen K A 2002 Phys. Rev. Lett. 88 090404 Google Scholar
[93]	Kasamatsu K, Tsubota M 2004 Phys. Rev. Lett. 93 100402 Google Scholar
[94]	Wang C J, Cao C, Jian C M, Zhai H 2010 Phys. Rev. Lett. 105 160403 Google Scholar
[95]	Sinha S, Nath R, Santos L 2011 Phys. Rev. Lett. 107 270401 Google Scholar
[96]	Hu H, Ramachandhran B, Pu H, Liu X J 2012 Phys. Rev. Lett. 108 010402 Google Scholar
[97]	Xu X Q, Han J H 2011 Phys. Rev. Lett. 107 200401 Google Scholar
[98]	Zhou X F, Zhou J, Wu C J 2011 Phys. Rev. A 84 063624 Google Scholar
[99]	Liu C F, Fan H, Zhang Y C, Wang D S, Liu W M 2012 Phys. Rev. A 86 053616 Google Scholar
[100]	Wang X, Tan R B, Du Z J, Zhao W Y, Zhang X F, Zhang S G 2014 Chin. Phys. B 23 070308 Google Scholar
[101]	Fetter A L 2014 Phys. Rev. A 89 023629 Google Scholar
[102]	Sakaguchi H, Umeda K 2016 J. Phys. Soc. Jpn. 85 064402 Google Scholar
[103]	Sakaguchi H 2017 Phys. Rev. A 96 043620 Google Scholar
[104]	Wang H, Wen L H, Yang H, Shi C X, Li J H 2017 J. Phys. B: At. Mol. Opt. Phys. 50 155301 Google Scholar
[105]	Kato M, Zhang X F, Saito H 2017 Phys. Rev. A 95 043605 Google Scholar
[106]	Shi C X, Wen L H, Wang Q B, Yang H, Wang H 2018 J. Phys. Soc. Jpn. 87 094003 Google Scholar
[107]	李吉, 刘伍明 2018 67 110302 Google Scholar Li J, Liu W M 2018 Acta Phys. Sin. 67 110302 Google Scholar
[108]	Pu H, Raghavan S, Bigelow N P 2001 Phys. Rev. A 63 063603 Google Scholar
[109]	Ogawa S I, Möttöen M, Nakahara M, Ohmi T, Shimada H 2002 Phys. Rev. A 66 013617 Google Scholar
[110]	Itin A P, Morishita T, Satoh M, Tolstikhin O I, Watanabe S 2006 Phys. Rev. A 73 063615 Google Scholar
[111]	Isoshima T, Machida K 2002 Phys. Rev. A 66 053610 Google Scholar
[112]	Mizushima T, Machida K, Kita T 2002 Phys. Rev. Lett. 89 030401 Google Scholar
[113]	Saito H, Kawaguchi Y, Ueda M 2006 Phys. Rev. Lett. 96 065302 Google Scholar
[114]	Saito H, Kawaguchi Y, Ueda M 2007 Phys. Rev. A 75 013621
[115]	Turner A M 2009 Phys. Rev. Lett. 103 080603 Google Scholar
[116]	Pietila V, Möttönen M, Virtanen S M 2007 Phys. Rev. A 76 023610 Google Scholar
[117]	Ji A C, Liu W M, Song J L, Zhou F 2008 Phys. Rev. Lett. 101 010402 Google Scholar
[118]	Liu C F, Liu W M 2012 Phys. Rev. A 86 033602 Google Scholar
[119]	刘超飞万文娟张赣源 2013 62 200306 Google Scholar Liu C F, Wan W J, Zhang G Y 2013 Acta Phys. Sin. 62 200306 Google Scholar
[120]	Song S W, Zhang Y C, Zhao H, Wang Xuan, Liu W M 2014 Phys. Rev. A 89 063613 Google Scholar
[121]	Lovegrove J, Borgh M O, Ruostekoski 2014 Phys. Rev. Lett. 112 075301 Google Scholar
[122]	Zhao D, Song S W, Wen L, Li Z D, Luo H G, Liu W M 2015 Phys. Rev. A 91 013619 Google Scholar
[123]	Gautam S, Adhikari S K 2016 Phys. Rev. A 93 013630 Google Scholar
[124]	刘静思李吉刘伍明 2017 66 130305 Google Scholar Liu J S, Li J, Liu W M 2017 Acta Phys. Sin. 66 130305 Google Scholar
[125]	Li J, Yu Y M, Zhuang L, Liu W M 2017 Phys. Rev. A 95 043633 Google Scholar

[1]	雍康乐, 闫家伟, 唐善发, 张蓉竹. 彗差和球差对涡旋光束斜程传输特性的影响. , 2020, 69(1): 014201. doi: 10.7498/aps.69.20191254
[2]	蒋光禹, 孙超, 李沁然. 涡旋对深海风成噪声垂直空间特性的影响. , 2020, 69(14): 144301. doi: 10.7498/aps.69.20200059
[3]	赵兴东, 张莹莹, 刘伍明. 光晶格中超冷原子系统的磁激发. , 2019, 68(4): 043703. doi: 10.7498/aps.68.20190153
[4]	李耀义, 贾金锋. 在人工拓扑超导体磁通涡旋中寻找Majorana零能模. , 2019, 68(13): 137401. doi: 10.7498/aps.68.20181698
[5]	郭成豹, 殷琦琦. 舰船磁场磁单极子阵列法建模技术. , 2019, 68(11): 114101. doi: 10.7498/aps.68.20190201
[6]	黄灿, 李小影, 朱岩, 潘燕飞, 樊济宇, 施大宁, 马春兰. 第一性原理计算Co/h-BN界面上的微弱Dzyaloshinsky-Moriya相互作用. , 2018, 67(11): 117102. doi: 10.7498/aps.67.20180337
[7]	李小影, 黄灿, 朱岩, 李晋斌, 樊济宇, 潘燕飞, 施大宁, 马春兰. -(Zn,Cr)S(111)表面上的Dzyaloshinsky-Moriya作用:第一性原理计算. , 2018, 67(13): 137101. doi: 10.7498/aps.67.20180342
[8]	轩胜杰, 柳艳. 周期性应变调控斯格明子在纳米条带中的运动. , 2018, 67(13): 137503. doi: 10.7498/aps.67.20180031
[9]	张蕾. 斯格明子相关的螺旋磁有序体系的临界行为. , 2018, 67(13): 137501. doi: 10.7498/aps.67.20180137
[10]	梁雪, 赵莉, 邱雷, 李双, 丁丽红, 丰友华, 张溪超, 周艳, 赵国平. 磁性斯格明子的赛道存储. , 2018, 67(13): 137510. doi: 10.7498/aps.67.20180764
[11]	董博闻, 张静言, 彭丽聪, 何敏, 张颖, 赵云驰, 王超, 孙阳, 蔡建旺, 王文洪, 魏红祥, 沈保根, 姜勇, 王守国. 磁性斯格明子的多场调控研究. , 2018, 67(13): 137507. doi: 10.7498/aps.67.20180931
[12]	夏静, 韩宗益, 宋怡凡, 江文婧, 林柳蓉, 张溪超, 刘小晰, 周艳. 磁斯格明子器件及其应用进展. , 2018, 67(13): 137505. doi: 10.7498/aps.67.20180894
[13]	赵巍胜, 黄阳棋, 张学莹, 康旺, 雷娜, 张有光. 斯格明子电子学的研究进展. , 2018, 67(13): 131205. doi: 10.7498/aps.67.20180554
[14]	陈光平. 简谐+四次势中自旋轨道耦合旋转玻色-爱因斯坦凝聚体的基态结构. , 2015, 64(3): 030302. doi: 10.7498/aps.64.030302
[15]	史良马, 周明健, 朱仁义. 磁场作用下超导圆环的涡旋演化. , 2014, 63(24): 247501. doi: 10.7498/aps.63.247501
[16]	周昱, 周青春, 马晓栋. 幺正极限附近费米气体反常激发模式的涡旋. , 2013, 62(14): 140301. doi: 10.7498/aps.62.140301
[17]	刘超飞, 万文娟, 张赣源. 自旋轨道耦合的23Na自旋-1玻色-爱因斯坦凝聚体中的涡旋斑图的研究. , 2013, 62(20): 200306. doi: 10.7498/aps.62.200306
[18]	黄思训, 蔡其发, 项杰, 张铭. 台风风场分解. , 2007, 56(5): 3022-3027. doi: 10.7498/aps.56.3022
[19]	王莉, 王庆峰, 王喜庆, 吕百达. 两束离轴高斯光束干涉场中的横向光涡旋. , 2007, 56(1): 201-207. doi: 10.7498/aps.56.201
[20]	李永青, 李希国, 刘紫玉, 罗培燕, 张鹏鸣. Jackiw-Pi模型的新涡旋解. , 2007, 56(11): 6178-6182. doi: 10.7498/aps.56.6178

计量

文章访问数: 14736
PDF下载量: 463
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

旋量玻色-爱因斯坦凝聚体拓扑性质的研究进展