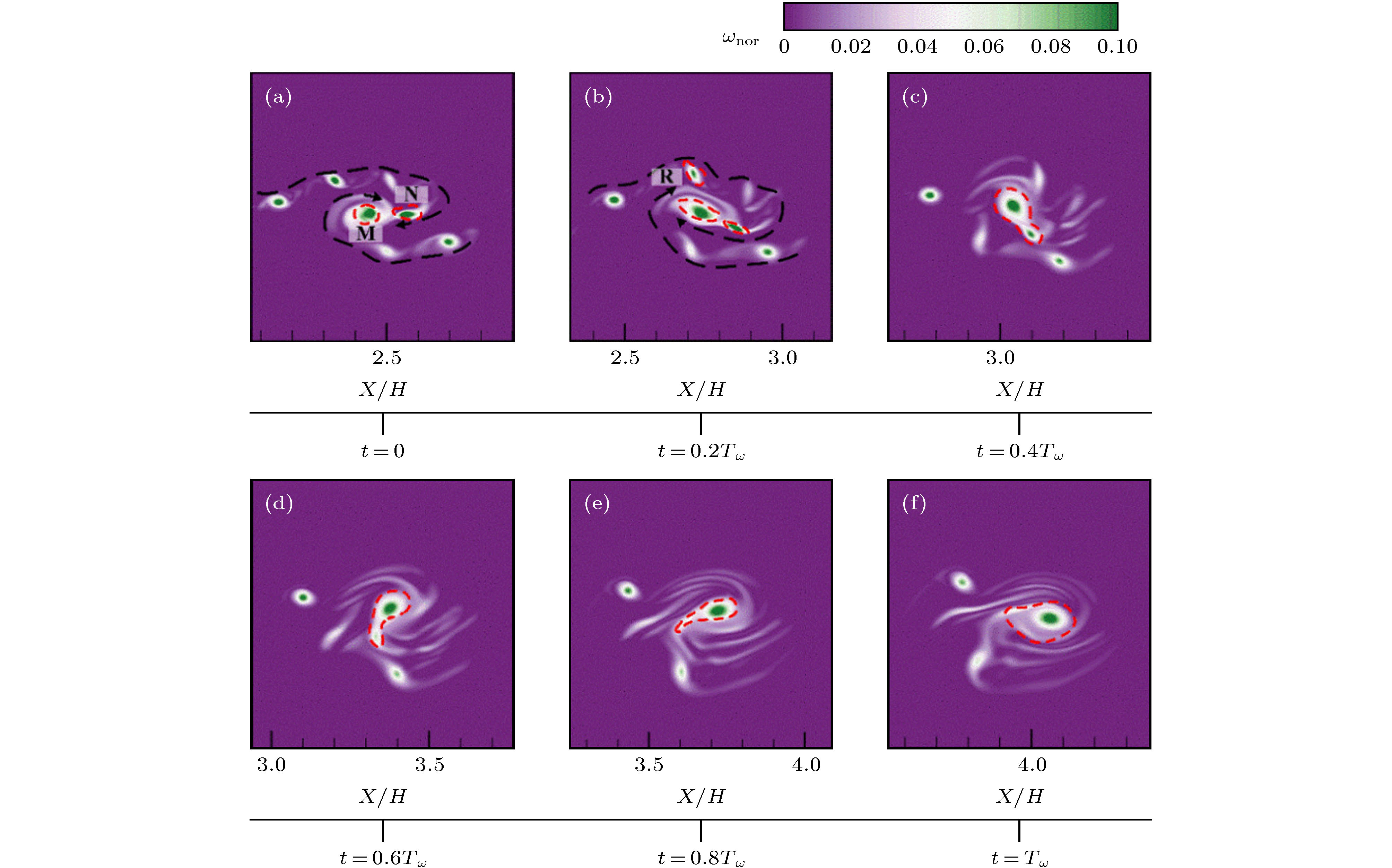
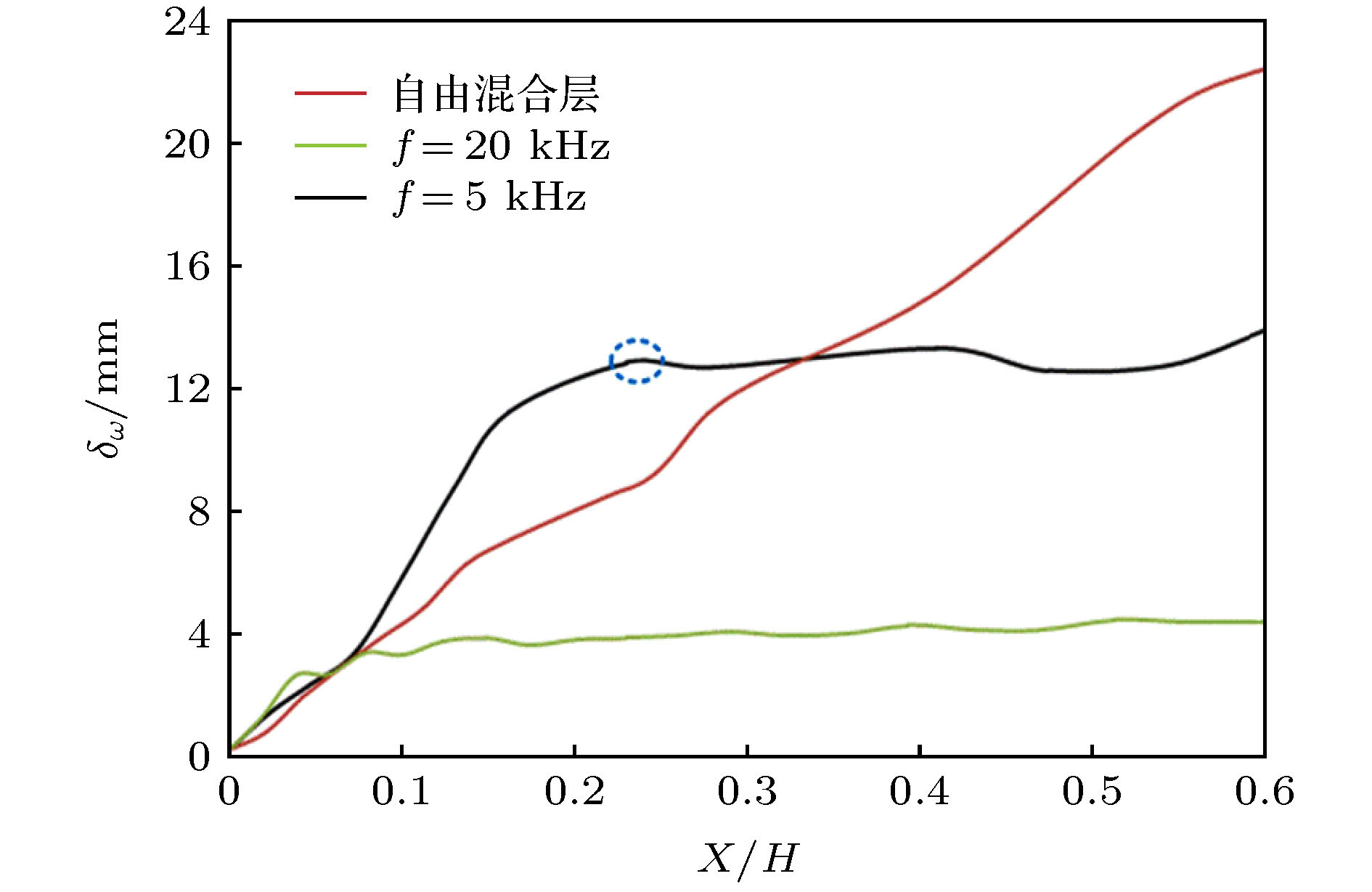
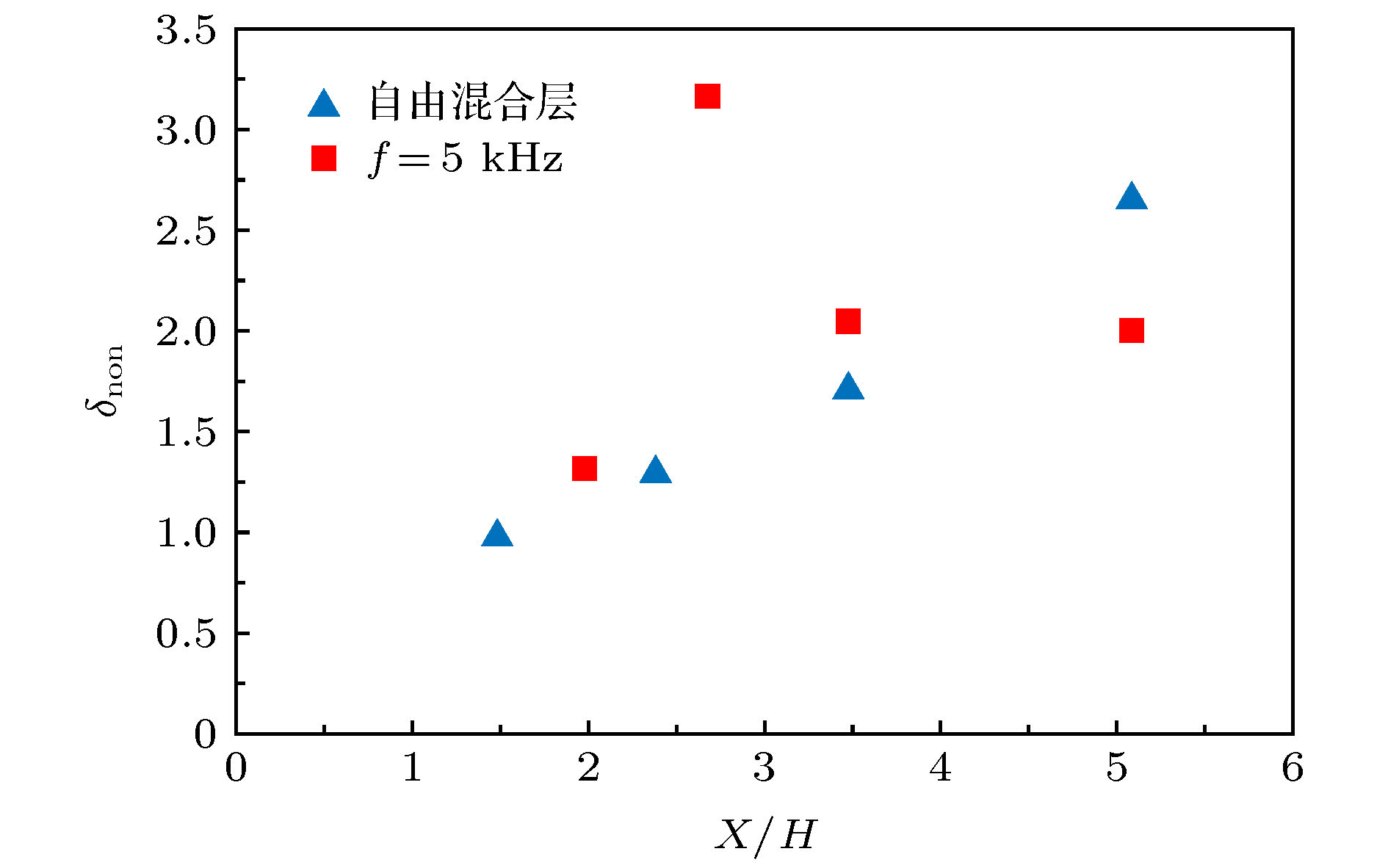
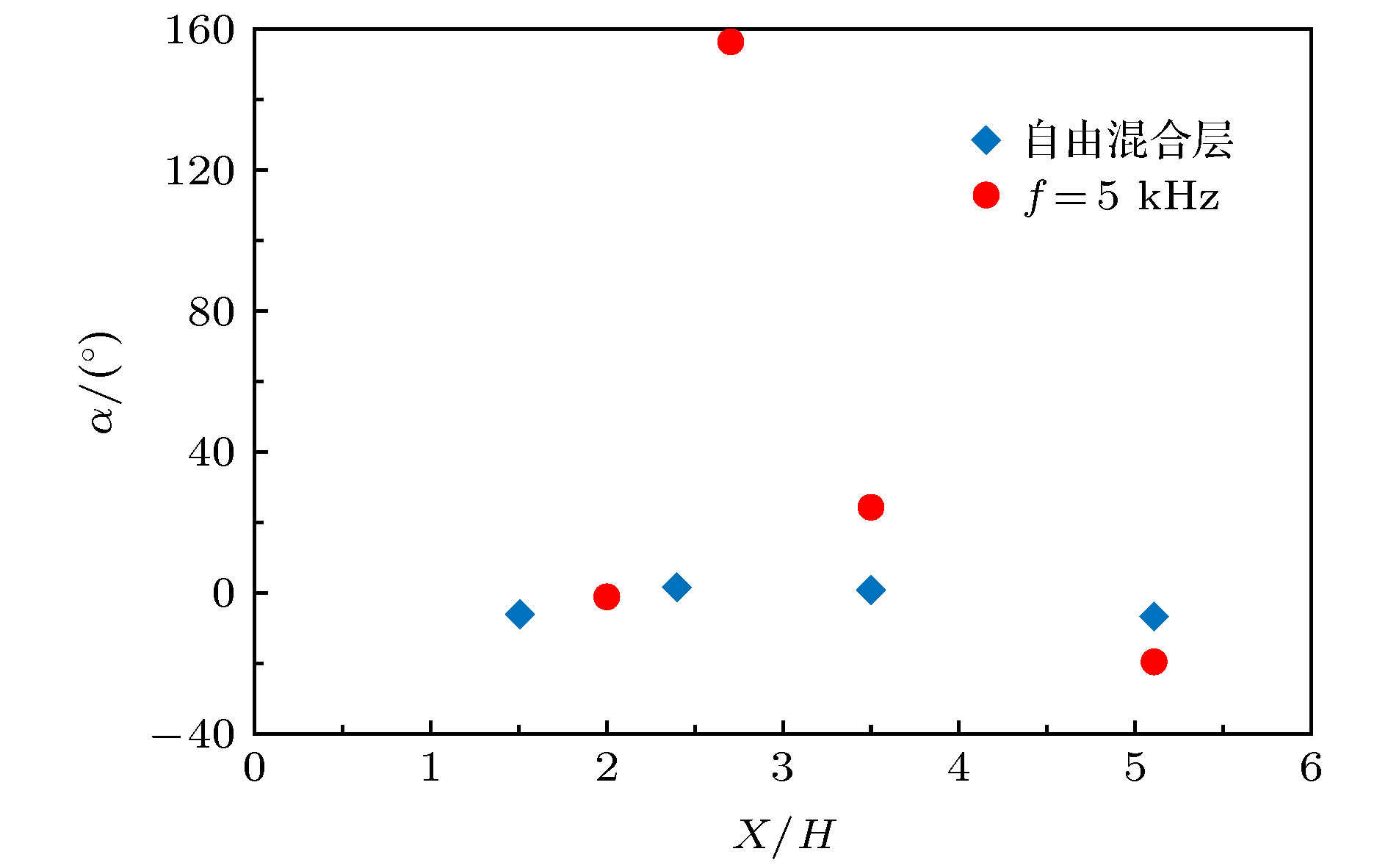
-
通过直接数值求解Navier-Stokes方程, 研究了入流激励下可压缩剪切层中Kelvin-Helmholtz (KH)涡结构的响应特性, 结果清晰地展示了KH涡的独特演化方式. 基于流动可视化数据, 采用两点相关性分析获得了流场拟序结构的空间尺寸和结构角分布. 通过分析不同激励频率下涡结构的动态特性, 揭示了入流激励下可压缩剪切层中KH涡结构的独特演化机理. 研究结果表明, 低频入流激励(f = 5 kHz)下KH涡尺寸在远场区域达到饱和后呈现锁频状态, KH涡量厚度稳定在12—14 mm之间; 与自由剪切层涡结构通过配对合并的方式实现生长的机理不同, 低频入流激励下剪切层的发展是通过中间涡核顺时针吞噬KH不稳定波诱导的一串外围小涡结构来实现生长. 此外, 针对高频激励 (f = 20 kHz)下的剪切层流动, 研究了涡结构特性和入流激励参数之间的定量关系, 发现均匀分布涡结构的尺寸近似等于对流速度与入流激励频率之比.
-
关键词:
- 可压缩剪切层 /
- 入流激励 /
- Kelvin-Helmholtz涡 /
- 生长特性
By numerically solving the Navier-Stokes equations, the response characteristics of inflow-stimulated Kelvin-Helmholtz vortex in compressible shear layer arestudied. The mixing characteristics and the unique growth mechanism of the vortex structure are clearly revealed. By employing the index of vorticity thickness, the mixing properties are quantitatively analyzed. Based on the flow visualization results, the spatial size and the structure angle of the flow coherent structure are investigated by utilizing spatial correlation analysis. The evolution mechanism of the vortex structure in supersonic mixing layer induced by inlet forcing is revealed by analyzing the dynamical performances of the flow structure under different frequency disturbances. The numerical results show that with low forcing frequency at f = 5 kHz, the mixing efficiency is remarkably increased in the near-field of the flow. Whereas, in the far-field downstream the flow, the size of the structure reaches saturation state and the vortex passage frequency is locked, which causes the vorticity thickness to stabilize from 12mm to 14mm. Meanwhile, in a free mixing layer, the pairing and merging process occur in the flow field to promote the growth of the vortex structure, while in mixing layer with inlet forcing, the growth mechanism is that the vortex core engulfs a string of vortices induced by Kelvin-Helmholtz instability. The process of engulfment contributes much to the growth of the vortex structure. The analysis of spatial correlation distribution shows that in the area where engulfment occurs, the contour line shows the property of long and narrow ellipse instead of full ellipse and the structure in the area possesses the characteristics of intense rotation and inclination. Besides, with high inlet forcing frequency at f = 20 kHz, the size of the vortices becomes full in the near-field, and the vorticity thickness stabilizes between 3mm and 4 mm downstream the flow field. Meanwhile, the size of the vortex in controlled supersonic mixing layer is dominated by the imposed high-frequency forcing. An equation describing the quantitative relationship between the vortex characteristics and the imposed forcing frequency is derived, that is, the size of the uniform distribution vortex is approximately equal to the ratio of the value of convective velocity to inlet forcing frequency.-
Keywords:
- compressible shear layer /
- inlet forcing /
- Kelvin-Helmholtz vortex /
- growth characteristics
[1] Curran E T 2001 J. Propul. Power 17 1138
Google Scholar
[2] Jumper E J, Fitzgerald E J 2001 Prog. Aerosp. Sci. 37 299
Google Scholar
[3] 张冬冬, 谭建国, 李浩, 侯聚微 2017 66 104702
Google Scholar
Zhang D D, Tan J G, Li H, Hou J W 2017 Acta Phys. Sin. 66 104702
Google Scholar
[4] Seiner J M, Dash S M, Kenzakowski D C 2001 J. Propul. Power 17 1273
Google Scholar
[5] Mason J O, Aguirre R C, Catrakis H J 2005 Inter. Assoc. Mech. Eng. Trans. 42 1973
[6] Brown G L, Roshko A 1974 J. Fluid Mech. 64 775
Google Scholar
[7] Ortwerth P J, Shine A 1977 Tech. Rep. TR-77-118
[8] Papamoschou D, Roshko A 1988 J. Fluid Mech. 197 453
Google Scholar
[9] Ho C M, Huang L S 1982 J. Fluid Mech. 119 443
Google Scholar
[10] McLaughlin D K, Martens S, Kinzie K W 1992 AIAA Paper 92−0177
[11] 郭广明, 刘洪, 张斌, 张庆兵 2017 66 084701
Google Scholar
Guo G M, Liu H, Zhang B, Zhang Q B 2017 Acta Phys. Sin. 66 084701
Google Scholar
[12] Freeman A P, Catrakis H J 2008 AIAA J. 46 2582
Google Scholar
[13] Yu K H, Gutmark E J, Smith R A 1994 AIAA Paper 94-0185
[14] 冯军红 2016 博士学位论文 (长沙: 国防科技大学)
Feng J H 2016 Ph. D. Dissertation (Changsha: National University of Defense Technology) (in Chinese)
[15] Zhang D D, Tan J G, Yao X 2019 Phys. Fluids 31 036102
Google Scholar
[16] Zhang D D, Tan J G, Lv L, Li F 2019 Acta Astronaut. 156 33
Google Scholar
[17] Jiang G S, Shu C W 1996 J. Comput. Phys. 126 202
Google Scholar
[18] Lele S 1989 AIAA Paper 89−0374
[19] Ren Z X, Wang B, Zheng L X 2018 Phys. Fluids 30 036101
Google Scholar
[20] Goebel S G, Dutton J C 1991 AIAA J. 29 538
Google Scholar
[21] Grimshaw R 1984 Annu. Rev. Fluid Mech. 16 11
Google Scholar
[22] Olsen M G, Dutton J C 2003 J. Fluid Mech. 486 51
Google Scholar
[23] Zhang D D, Tan J G, Lv L 2015 Acta Astronaut. 117 440
Google Scholar
[24] Zhang D D, Tan J G, Li H 2017 Appl. Phys. Lett. 111 114103
Google Scholar
[25] Ghoniem A F, Ng K K 1987 Phys. Fluids 30 706
Google Scholar
[26] Bourdon C J, Dutton J C 1999 Phys. Fluids 11 201
Google Scholar
[27] 武宇, 易仕和, 何霖, 全鹏程, 朱杨柱 2015 64 014703
Google Scholar
Wu Y, Yi S H, He L, Quan P C, Zhu Y Z 2015 Acta Phys. Sin. 64 014703
Google Scholar
-
图 2 数值与实验对比 (a) 时均速度; (b) 流向湍流强度
Fig. 2. Comparison between numerical and experimental results: (a) Mean velocity; (b) turbulent intensity.
图 3 不同网格条件下混合层的涡量厚度沿流向的变化
Fig. 3. Vorticity thickness variations versus stream wise direction for different mesh distributions.
图 6 高频入流激励下流场涡结构的分布 (f = 20 kHz)
Fig. 6. Vortex structures distribution under inlet forcing with high frequency (f = 20 kHz).
图 7 流场涡核之间的压力分布
Fig. 7. Pressure distribution between the vortex core in the flow field.
图 8 高频入流激励下频谱分布 (f = 20 kHz) (a) X/H = 1; (b) X/H = 4
Fig. 8. Frequency spectrum distribution under inlet forcing with high frequency (f = 20 kHz): (a) X/H = 1; (b) X/H = 4
图 9 低频入流扰动下剪切层流场涡结构分布 (f = 5 kHz)
Fig. 9. Vortex structures distribution under inlet forcing with low frequency (f = 5 kHz).
图 10 低频入流激励下频谱分布 (f = 5 kHz) (a) X/H = 1; (b) X/H = 4
Fig. 10. Frequency spectrum distribution under inlet forcing with low frequency (f = 5 kHz): (a) X/H = 1; (b) X/H = 4
图 12 剪切层涡量厚度随流向距离的分布
Fig. 12. Vorticity thickness distribution versus streamwise direction.
图 13 自由剪切层中不同流向位置处空间相关性分布 (a) (1.5, 0.6); (b) (2.4, 0.6); (c) (3.5, 0.6); (d) (5.1, 0.6)
Fig. 13. Spatial correlation distributions of free shear layers in different streamwise positions: (a) (1.5, 0.6); (b) (2.4, 0.6); (c) (3.5, 0.6); (d) (5.1, 0.6).
图 14 低频激励下不同流向位置处空间相关性分布 (a) (2.0, 0.6); (b) (2.7, 0.6); (c) (3.5, 0.6); (d) (5.1, 0.6)
Fig. 14. Spatial correlation distributions with low frequency forcing in different streamwise positions: (a) (2.0, 0.6); (b) (2.7, 0.6); (c) (3.5, 0.6); (d) (5.1, 0.6).
图 16 无量纲结构大小沿流向的分布
Fig. 16. Non-dimensional structure size distribution versus streamwise direction.
表 1 数值计算来流参数
Table 1. Inflow parameters of numerical simulation
速度
/m·s–1马赫
数密度
/kg·m–3压力
/kPa温度
/K对流马
赫数Mc上层
来流473 2.15 0.983 34 120 0.4 下层
来流284 1.12 0.737 34 160 
下载: 导出CSV
表 2 Goebel-Dutton超声速混合层实验来流参数
Table 2. Inflow parameters of supersonic mixing layer experiments conducted by Goebel and Dutton.
速度
/m·s–1马赫
数密度
/kg·m–3压力
/kPa对流马
赫数Mc上层
来流519 2.04 1.00 46 0.2 下层
来流409 1.40 0.76 46
下载: 导出CSV
-
[1] Curran E T 2001 J. Propul. Power 17 1138
Google Scholar
[2] Jumper E J, Fitzgerald E J 2001 Prog. Aerosp. Sci. 37 299
Google Scholar
[3] 张冬冬, 谭建国, 李浩, 侯聚微 2017 66 104702
Google Scholar
Zhang D D, Tan J G, Li H, Hou J W 2017 Acta Phys. Sin. 66 104702
Google Scholar
[4] Seiner J M, Dash S M, Kenzakowski D C 2001 J. Propul. Power 17 1273
Google Scholar
[5] Mason J O, Aguirre R C, Catrakis H J 2005 Inter. Assoc. Mech. Eng. Trans. 42 1973
[6] Brown G L, Roshko A 1974 J. Fluid Mech. 64 775
Google Scholar
[7] Ortwerth P J, Shine A 1977 Tech. Rep. TR-77-118
[8] Papamoschou D, Roshko A 1988 J. Fluid Mech. 197 453
Google Scholar
[9] Ho C M, Huang L S 1982 J. Fluid Mech. 119 443
Google Scholar
[10] McLaughlin D K, Martens S, Kinzie K W 1992 AIAA Paper 92−0177
[11] 郭广明, 刘洪, 张斌, 张庆兵 2017 66 084701
Google Scholar
Guo G M, Liu H, Zhang B, Zhang Q B 2017 Acta Phys. Sin. 66 084701
Google Scholar
[12] Freeman A P, Catrakis H J 2008 AIAA J. 46 2582
Google Scholar
[13] Yu K H, Gutmark E J, Smith R A 1994 AIAA Paper 94-0185
[14] 冯军红 2016 博士学位论文 (长沙: 国防科技大学)
Feng J H 2016 Ph. D. Dissertation (Changsha: National University of Defense Technology) (in Chinese)
[15] Zhang D D, Tan J G, Yao X 2019 Phys. Fluids 31 036102
Google Scholar
[16] Zhang D D, Tan J G, Lv L, Li F 2019 Acta Astronaut. 156 33
Google Scholar
[17] Jiang G S, Shu C W 1996 J. Comput. Phys. 126 202
Google Scholar
[18] Lele S 1989 AIAA Paper 89−0374
[19] Ren Z X, Wang B, Zheng L X 2018 Phys. Fluids 30 036101
Google Scholar
[20] Goebel S G, Dutton J C 1991 AIAA J. 29 538
Google Scholar
[21] Grimshaw R 1984 Annu. Rev. Fluid Mech. 16 11
Google Scholar
[22] Olsen M G, Dutton J C 2003 J. Fluid Mech. 486 51
Google Scholar
[23] Zhang D D, Tan J G, Lv L 2015 Acta Astronaut. 117 440
Google Scholar
[24] Zhang D D, Tan J G, Li H 2017 Appl. Phys. Lett. 111 114103
Google Scholar
[25] Ghoniem A F, Ng K K 1987 Phys. Fluids 30 706
Google Scholar
[26] Bourdon C J, Dutton J C 1999 Phys. Fluids 11 201
Google Scholar
[27] 武宇, 易仕和, 何霖, 全鹏程, 朱杨柱 2015 64 014703
Google Scholar
Wu Y, Yi S H, He L, Quan P C, Zhu Y Z 2015 Acta Phys. Sin. 64 014703
Google Scholar
下载:
计量
- 文章访问数: 8569
- PDF下载量: 125
- 被引次数: 0
