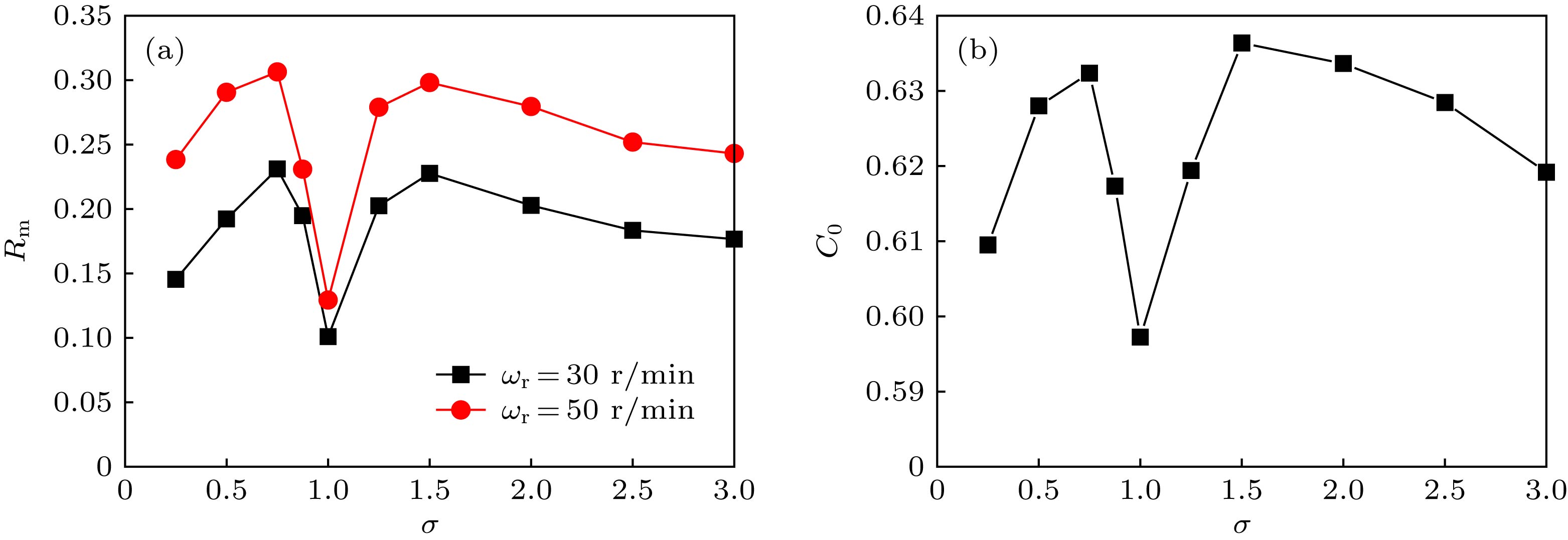
-
转筒中的颗粒流广泛存在于工业生产中, 颗粒形状是影响颗粒流动的重要因素. 本文基于超二次曲面方程描述球体和椭球颗粒的几何形态, 采用离散元方法对水平转筒中颗粒物质的流动和混合特性进行数值分析, 并与椭球混合过程的实验结果进行对比验证. 在此基础之上, 进一步研究了圆筒转速、颗粒填充分数和颗粒长宽比对混合率的影响规律. 计算结果表明: 颗粒材料的混合率随着转速的增加或填充分数的减小而增加. 在相同转速和填充分数下, 椭球颗粒的混合率高于球形颗粒. 同时, 长宽比为0.75和1.5时椭球颗粒具有最高的混合率. 当长宽比小于0.75时, 混合率随着长宽比的增加而增加; 当长宽比大于1.5时, 混合率随着长宽比的增加而减小. 此外, 椭球颗粒出现更明显的速度分层现象. 颗粒长宽比改变颗粒间的接触模式和系统的密集度, 增加了颗粒系统的平动而限制了转动, 在一定程度上提高了外部能量向颗粒系统转化的效率.
Granular flow in the drum widely appears in the fields of industrial production, and discrete element method (DEM) proves to be a critical tool for studying the flow characteristics of granular materials. Considering simple contact and efficient calculations, the three-dimensional spheres are originally adopted by the DEM. Therefore, the DEM simulations mainly focus on spherical particles, while the dynamics of non-spherical particles in rotating drums is relatively rarely studied. It is reported that particle shape significantly affects the macroscopic and microscopic properties of the granular flow. Compared with spherical particles, non-spherical particles have low fluidity and great interlock. Meanwhile, it is questionable whether conclusions drawn from spherical particle systems can be transplanted to non-spherical particle systems. In this work, super-quadric equations based on continuous function representation are used to describe the spherical and ellipsoidal particles. Considering the complex contact detection between particles, the Newton iteration algorithm is used to solve the non-linear equations. Meanwhile, a non-linear contact model considering the equivalent radius of curvature at the local contact point is used to calculate the contact force between the super-quadric elements. To examine the validity of DEM model, we compare our simulated results with the previous experimental results for mixing process of ellipsoids, and this method is verified by good agreement between the simulated results and the experimental results. According to the aforementioned method, the influences of rotating speed, fill level, and aspect ratio on the mixing rate are discussed. The results show that the granular system reaches the cascading regime and the S-shaped surface of the granular bed is observed. In addition, Lacey mixing index is used to quantify the mixing of granular systems, and the mixing rate is obtained by fitting the Lacey mixing index. The mixing rate increases as the rotating speed increases. At the same rotating speed, the mixing rate of ellipsoids is faster than that of spheres. Meanwhile, the ellipsoidal particles have the fastest mixing rate when the aspect ratio is 0.75 or 1.50. When the aspect ratio is less than 0.75, the mixing ratio increases as the aspect ratio increases; when the aspect ratio is greater than 1.50, the mixing ratio decreases as the aspect ratio increases. Moreover, more pronounced velocity stratification is observed for ellipsoids. The translational kinetic energy of ellipsoidal particles is higher than that of spherical particles, and their rotational kinetic energy is lower than that of spheres. The aspect ratio of particles can adjust the contact mode between particles and cause the interlock. It means that the relative rotation between particles is limited and the efficiency of the external energy transferring to the non-spherical system may be improved. -
Keywords:
- discrete element method /
- super-quadric element /
- ellipsoidal particle /
- mixing characteristics
[1] Gioia G, Ott-Monsivais S E, Hill K M 2006 Phys. Rev. Lett. 96 138001
Google Scholar
[2] Emilien A, Farhang R 2014 Phys. Rev. Lett. 112 078001
Google Scholar
[3] Jaeger H M, Nagel S R 1992 Science 255 1523
Google Scholar
[4] 季顺迎, 孙其诚, 严颖 2011 中国科学: 物理学 力学 天文学 41 1112
Ji S Y, Sun Q C, Yan Y 2011 Sci. Sin-Phys. Mech. Astron. 41 1112
[5] 黄德财, 孙刚, 厚美瑛, 陆坤权 2006 55 4754
Google Scholar
Huang D C, Sun G, Hou M Y, Lu K Q 2006 Acta Phys. Sin. 55 4754
Google Scholar
[6] 彭政, 蒋亦民, 刘锐, 厚美瑛 2013 62 024502
Google Scholar
Peng Z, Jiang Y M, Liu R, Hou M Y 2013 Acta Phys. Sin. 62 024502
Google Scholar
[7] 孙其诚, 王光谦 2008 57 4667
Google Scholar
Sun Q C, Wang G Q 2008 Acta Phys. Sin. 57 4667
Google Scholar
[8] 夏建新, 吉祖稳, 毛旭锋, 曹华德 2013 科学通报 58 1200
Xia J X, Ji Z W, Mao X F, Cao H D 2013 Chin. Sci. Bull. 58 1200
[9] 刘扬, 韩燕龙, 贾富国, 姚丽娜, 王会, 史宇菲 2015 64 114501
Google Scholar
Liu Y, Han Y L, Jia F G, Yao L N, Wang H, Shi Y F 2015 Acta Phys. Sin. 64 114501
Google Scholar
[10] 陈泉, 杨晖, 李然, 韩韧, 孙其诚 2019 中国科学: 物理学 力学 天文学 49 067001
Chen Q, Yang H, Li R, Han R, Sun Q C 2019 Sci. Sin.: Phys. Mech. Astron. 49 067001
[11] Cundall P A, Strack O D L 1979 Géotechnique 29 47
Google Scholar
[12] 赵子渊, 李昱君, 王富帅, 张祺, 厚美瑛, 李文辉, 马钢 2018 67 104502
Google Scholar
Zhao Z Y, Li Y J, Wang F S, Zhang Q, Hou M Y, Li W H, Ma G 2018 Acta Phys. Sin. 67 104502
Google Scholar
[13] Chand R, Khaskheli M A, Qadir A, Ge B, Shi Q 2012 Physica A 391 4590
Google Scholar
[14] 黄德财, 冯耀东, 解为梅, 陆明, 吴海平, 胡凤兰, 邓开明 2012 61 124501
Google Scholar
Huang D C, Feng Y D, Xie W M, Lu M, Wu H P, Hu F L, Deng K M 2012 Acta Phys. Sin. 61 124501
Google Scholar
[15] Gui N, Fan J 2015 Int. J. Heat Mass Tran. 84 740
Google Scholar
[16] Xiao X, Tan Y, Zhang H, Deng R, Jiang S 2017 Powder Technol. 314 182
Google Scholar
[17] Chou S H, Hu H J, Hsiau S S 2016 Adv. Powder Technol. 27 1912
Google Scholar
[18] Halidan M, Chandratilleke G R, Dong K J, Yu A B 2018 Powder Technol. 325 92
Google Scholar
[19] 高红利, 赵永志, 刘格思, 陈友川, 郑津洋 2011 60 074501
Google Scholar
Gao H L, Zhao Y Z, Liu G S, Chen Y C, Zheng J Y 2011 Acta Phys. Sin. 60 074501
Google Scholar
[20] Zhong W, Yu A, Liu X, Tong Z, Zhang H 2016 Powder Technol. 302 108
Google Scholar
[21] Lu G, Third J R, Müller C R 2015 Chem. Eng. Sci. 127 425
Google Scholar
[22] Obermayr M, Dressler K, Vrettos C, Eberhard P 2013 Comput. Geotech. 49 299
Google Scholar
[23] Govender N, Wilke D N, Kok S 2015 Appl. Math. Comput. 267 810
[24] 刘璐, 季顺迎 2019 中国科学: 物理学 力学 天文学 49 064601
Liu L, Ji S Y 2019 Sci. Sin.: Phys. Mech. Astron. 49 064601
[25] 王嗣强, 季顺迎 2018 67 094501
Google Scholar
Wang S Q, Ji S Y 2018 Acta Phys. Sin. 67 094501
Google Scholar
[26] Garboczi E J, Bullard J W 2017 Adv. Powder Technol. 28 325
Google Scholar
[27] Gui N, Yang X, Tu J, Jiang S 2017 Powder Technol. 318 248
Google Scholar
[28] Ma H, Zhao Y 2017 Chem. Eng. Sci. 172 636
Google Scholar
[29] Ma H, Zhao Y 2018 Granul. Matter 20 41
Google Scholar
[30] Kodam M, Bharadwaj R, Curtis J, Hancock B, Wassgren C 2010 Chem. Eng. Sci. 65 5863
Google Scholar
[31] You Y, Zhao Y 2018 Powder Technol. 331 179
Google Scholar
[32] Barr 1981 IEEE Comput. Graph. Appl. 1 11
Google Scholar
[33] Soltanbeigi B, Podlozhnyuk A, Papanicolopulos S A, Kloss C, Pirker S, Ooi J Y 2018 Powder Technol. 329 288
Google Scholar
[34] Houlsby G T 2009 Powder Technol. 36 953
[35] Podlozhnyuk A, Pirker S, Kloss C 2016 Comput. Part. Mech. 4 101
[36] Zhou Z Y, Zou R P, Pinson D, Yu A B 2011 Ind. Eng. Chem. Res. 50 9787
Google Scholar
[37] He S Y, Gan J Q, Pinson D, Zhou Z Y 2019 Powder Technol. 341 157
Google Scholar
[38] Goldman R 2005 Comput. Aided Geom. D 22 632
Google Scholar
[39] Lacey P M C 1954 J. Chem. Technol. Biot. 4 257
[40] Jiang M, Zhao Y, Liu G, Zheng J 2011 Particuology 9 270
Google Scholar
[41] He S, Gan J, Pinson D, Zhou Z 2017 EPJ Web of Conferences 140 06018
Google Scholar
[42] Donev A, Cisse I, Sachs D, Variano E A, Stillinger F H, Connelly R, Torquato S, Chaikin P M 2004 Science 303 990
Google Scholar
[43] Delaney G W, Cleary P W 2010 Europhys. Lett. 89 34002
Google Scholar
-
图 1 球体和椭球的超二次曲面离散元模型 (a) a = b = 2c; (b) a = b = c; (c) a = b = 0.5c
Fig. 1. Super-quadric discrete element model of spherical and ellipsoidal elements: (a) a = b = 2c; (b) a = b = c; (c) a = b = 0.5c
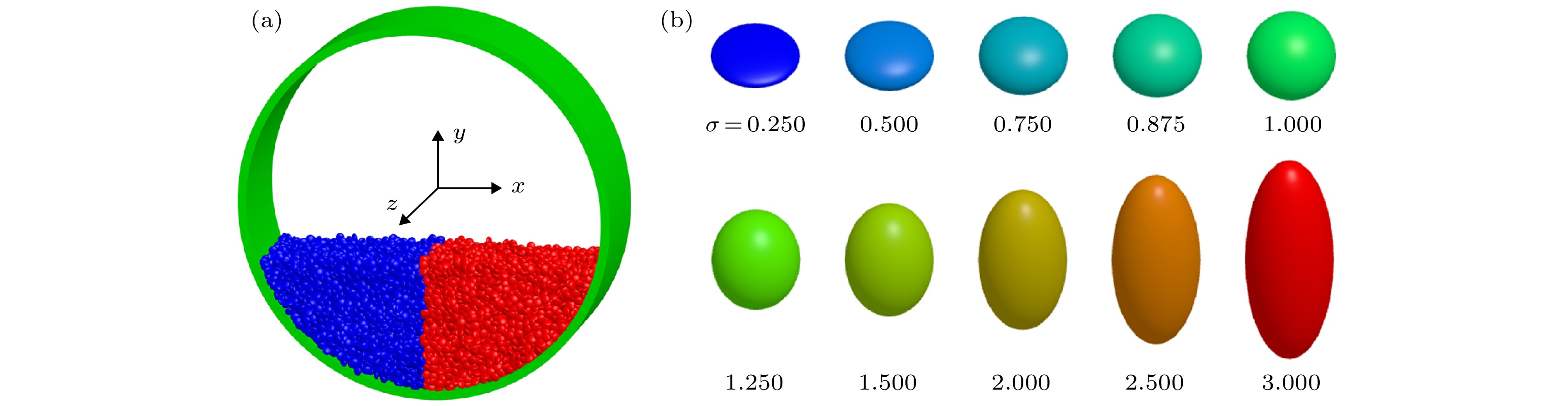
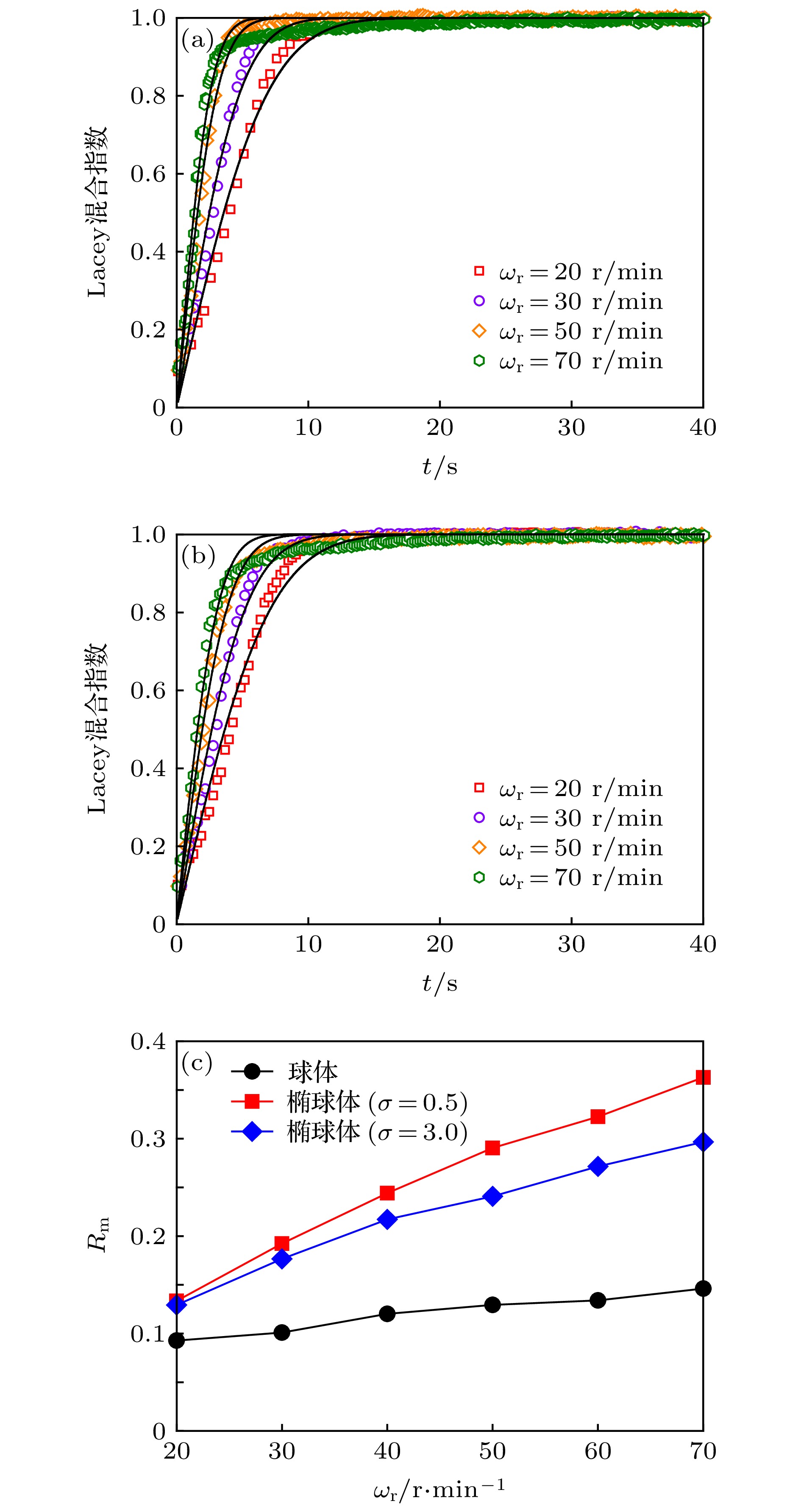
图 3 不同转动圈数下颗粒混合过程的实验结果[31]和离散元数值结果的对比 (a) ωr = 20 r/min的流动图案; (b) ωr = 20 r/min的Lacey混合指数; (c) ωr = 40 r/min的Lacey混合指数
Fig. 3. Comparison of mixing process between experiment results[31] and DEM simulation results at different rotating speeds: (a) Mixing pattern at 20 r/min; (b) Lacey mixing index at 20 r/min; (c) Lacey mixing index at 40 r/min.
图 4 三维水平圆筒的离散元数值模型(a)和不同长宽比的椭球模型(b)
Fig. 4. Schematic diagram of three-dimensional horizontal drum simulated by DEM model (a) and examples of ellipsoids with different aspect ratios (b)
图 5 旋转速度和颗粒长宽比对混合过程的影响 (a) ωr = 20 r/min的椭球颗粒(σ = 3.0); (b) ωr = 40 r/min的椭球颗粒(σ = 3.0); (c) ωr = 20 r/min的球体颗粒(σ = 1.0); (d) ωr = 20 r/min的椭球颗粒(σ = 0.5)
Fig. 5. The influence of rotating speed and aspect ratio on the mixing process: (a) Ellipsoids (σ = 3.0) at 20 r/min; (b) ellipsoids (σ = 3.0) at 40 r/min; (c) sphere (σ = 1.0) at 20 r/min; (d) ellipsoids (σ = 0.5) at 20 r/min
图 6 旋转速度和颗粒形状对Lacey混合指数和混合率的影响 (a) 椭球颗粒(σ = 0.5); (b) 椭球颗粒(σ = 3.0); (c) 混合率
Fig. 6. The influence of rotating speed and particle shape on the Lacey mixing index and mixing rate: (a) Ellipsoids (σ = 0.5); (b) ellipsoids (σ = 3.0); (c) mixing rate.
图 7 ωr = 30 r/min时椭球和球形颗粒的混合率随填充分数的变化
Fig. 7. Mixing rate of the ellipsoid and spherical particles varies with the fill level at ωr = 30 r/min
图 8 ωr = 30 r/min时椭球颗粒的混合率和初始体积分数的变化 (a)混合率; (b)初始密集度
Fig. 8. Mixing rate and initial packing fraction under various aspect ratios at 30 r/min: (a) Mixing rate; (b) initial packing fraction
图 9 不同转速下球体和椭球颗粒的速度分布 (a) 椭球(σ = 0.5); (b) 球体(σ = 1.0); (c) 椭球(σ = 3.0)
Fig. 9. The velocity profiles of granular bed for differently shaped particles: (a) Ellipsoids (σ = 0.5); (b) spheres (σ = 1.0); (c) ellip-soids (σ = 3.0)
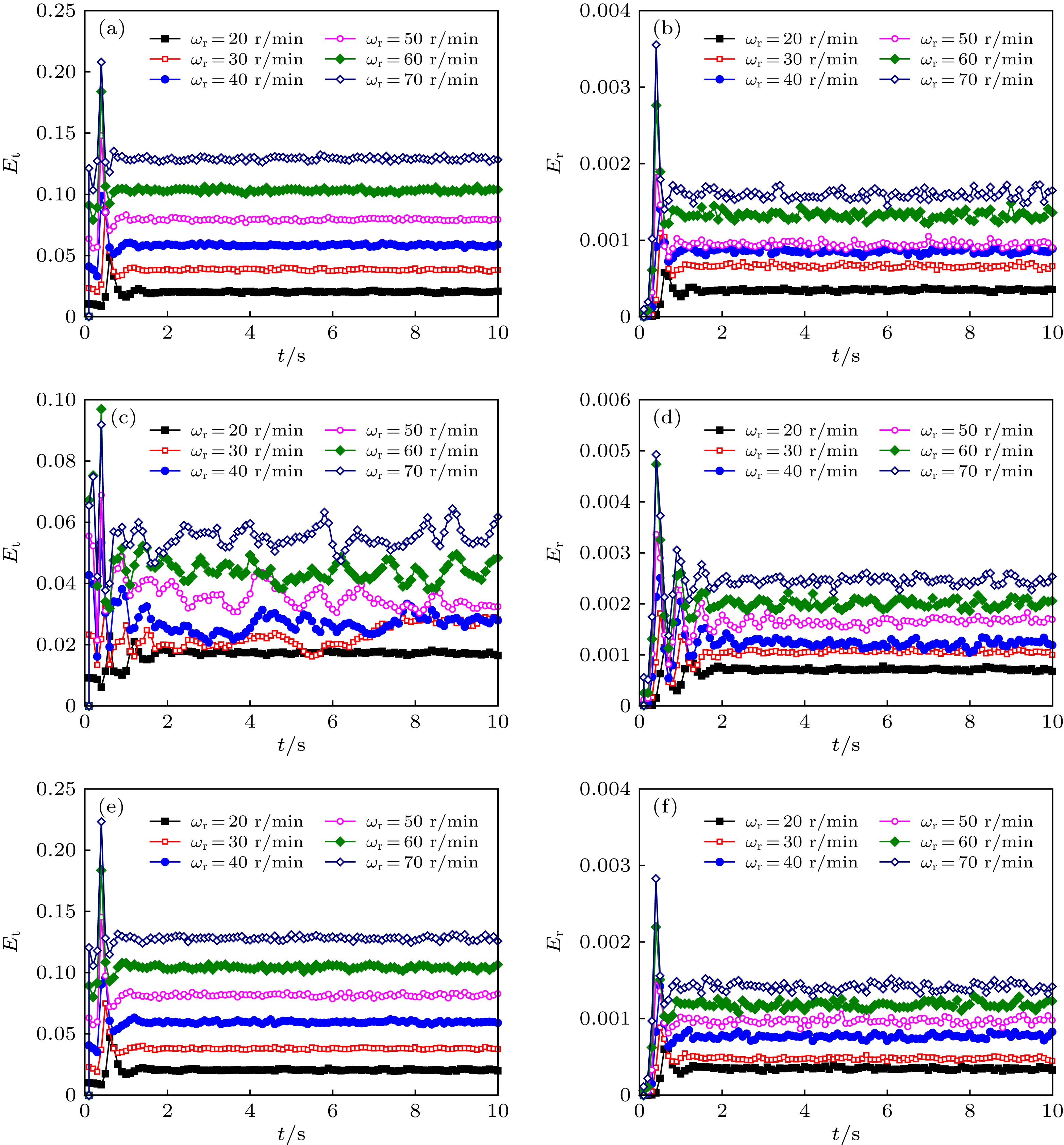
图 10 在不同转速下球体和椭球颗粒的平动动能和转动动能随时间的变化 (a), (b) 椭球(σ = 0.5); (c), (d) 球体(σ = 1.0); (e), (f) 椭球(σ = 3.0)
Fig. 10. Translational and rotational kinetic energy at different rotating speeds for differently shaped particles: (a), (b) Ellipsoids (σ = 0.5); (c), (d) spheres (σ = 1.0); (e), (f) ellipsoids (σ = 3.0)
图 11 ωr = 30和50 r/min时球体和椭球颗粒的平动和转动动能 (a)平均平动动能; (b)平均转动动能
Fig. 11. Translational and rotational kinetic energy at 30 and 50 r/min for spheres and ellipsoids: (a) Average translational kinetic energy; (b) average rotational kinetic energy
表 1 椭球颗粒离散元模拟的主要计算参数
Table 1. DEM simulation parameters of ellipsoids
参数 符号 单位 数值 参数 符号 单位 数值 弹性模量 E GPa 1.0 颗粒间摩擦系数 μs — 0.3 泊松比 ν — 0.3 法向阻尼系数 Cn — 0.05 颗粒密度 ρ kg/m3 1150.0 切向阻尼系数 Ct — 0.05 颗粒与圆筒的摩擦系数 μws — 0.9 时间步长 dt s 1 × 10–6 
下载: 导出CSV
-
[1] Gioia G, Ott-Monsivais S E, Hill K M 2006 Phys. Rev. Lett. 96 138001
Google Scholar
[2] Emilien A, Farhang R 2014 Phys. Rev. Lett. 112 078001
Google Scholar
[3] Jaeger H M, Nagel S R 1992 Science 255 1523
Google Scholar
[4] 季顺迎, 孙其诚, 严颖 2011 中国科学: 物理学 力学 天文学 41 1112
Ji S Y, Sun Q C, Yan Y 2011 Sci. Sin-Phys. Mech. Astron. 41 1112
[5] 黄德财, 孙刚, 厚美瑛, 陆坤权 2006 55 4754
Google Scholar
Huang D C, Sun G, Hou M Y, Lu K Q 2006 Acta Phys. Sin. 55 4754
Google Scholar
[6] 彭政, 蒋亦民, 刘锐, 厚美瑛 2013 62 024502
Google Scholar
Peng Z, Jiang Y M, Liu R, Hou M Y 2013 Acta Phys. Sin. 62 024502
Google Scholar
[7] 孙其诚, 王光谦 2008 57 4667
Google Scholar
Sun Q C, Wang G Q 2008 Acta Phys. Sin. 57 4667
Google Scholar
[8] 夏建新, 吉祖稳, 毛旭锋, 曹华德 2013 科学通报 58 1200
Xia J X, Ji Z W, Mao X F, Cao H D 2013 Chin. Sci. Bull. 58 1200
[9] 刘扬, 韩燕龙, 贾富国, 姚丽娜, 王会, 史宇菲 2015 64 114501
Google Scholar
Liu Y, Han Y L, Jia F G, Yao L N, Wang H, Shi Y F 2015 Acta Phys. Sin. 64 114501
Google Scholar
[10] 陈泉, 杨晖, 李然, 韩韧, 孙其诚 2019 中国科学: 物理学 力学 天文学 49 067001
Chen Q, Yang H, Li R, Han R, Sun Q C 2019 Sci. Sin.: Phys. Mech. Astron. 49 067001
[11] Cundall P A, Strack O D L 1979 Géotechnique 29 47
Google Scholar
[12] 赵子渊, 李昱君, 王富帅, 张祺, 厚美瑛, 李文辉, 马钢 2018 67 104502
Google Scholar
Zhao Z Y, Li Y J, Wang F S, Zhang Q, Hou M Y, Li W H, Ma G 2018 Acta Phys. Sin. 67 104502
Google Scholar
[13] Chand R, Khaskheli M A, Qadir A, Ge B, Shi Q 2012 Physica A 391 4590
Google Scholar
[14] 黄德财, 冯耀东, 解为梅, 陆明, 吴海平, 胡凤兰, 邓开明 2012 61 124501
Google Scholar
Huang D C, Feng Y D, Xie W M, Lu M, Wu H P, Hu F L, Deng K M 2012 Acta Phys. Sin. 61 124501
Google Scholar
[15] Gui N, Fan J 2015 Int. J. Heat Mass Tran. 84 740
Google Scholar
[16] Xiao X, Tan Y, Zhang H, Deng R, Jiang S 2017 Powder Technol. 314 182
Google Scholar
[17] Chou S H, Hu H J, Hsiau S S 2016 Adv. Powder Technol. 27 1912
Google Scholar
[18] Halidan M, Chandratilleke G R, Dong K J, Yu A B 2018 Powder Technol. 325 92
Google Scholar
[19] 高红利, 赵永志, 刘格思, 陈友川, 郑津洋 2011 60 074501
Google Scholar
Gao H L, Zhao Y Z, Liu G S, Chen Y C, Zheng J Y 2011 Acta Phys. Sin. 60 074501
Google Scholar
[20] Zhong W, Yu A, Liu X, Tong Z, Zhang H 2016 Powder Technol. 302 108
Google Scholar
[21] Lu G, Third J R, Müller C R 2015 Chem. Eng. Sci. 127 425
Google Scholar
[22] Obermayr M, Dressler K, Vrettos C, Eberhard P 2013 Comput. Geotech. 49 299
Google Scholar
[23] Govender N, Wilke D N, Kok S 2015 Appl. Math. Comput. 267 810
[24] 刘璐, 季顺迎 2019 中国科学: 物理学 力学 天文学 49 064601
Liu L, Ji S Y 2019 Sci. Sin.: Phys. Mech. Astron. 49 064601
[25] 王嗣强, 季顺迎 2018 67 094501
Google Scholar
Wang S Q, Ji S Y 2018 Acta Phys. Sin. 67 094501
Google Scholar
[26] Garboczi E J, Bullard J W 2017 Adv. Powder Technol. 28 325
Google Scholar
[27] Gui N, Yang X, Tu J, Jiang S 2017 Powder Technol. 318 248
Google Scholar
[28] Ma H, Zhao Y 2017 Chem. Eng. Sci. 172 636
Google Scholar
[29] Ma H, Zhao Y 2018 Granul. Matter 20 41
Google Scholar
[30] Kodam M, Bharadwaj R, Curtis J, Hancock B, Wassgren C 2010 Chem. Eng. Sci. 65 5863
Google Scholar
[31] You Y, Zhao Y 2018 Powder Technol. 331 179
Google Scholar
[32] Barr 1981 IEEE Comput. Graph. Appl. 1 11
Google Scholar
[33] Soltanbeigi B, Podlozhnyuk A, Papanicolopulos S A, Kloss C, Pirker S, Ooi J Y 2018 Powder Technol. 329 288
Google Scholar
[34] Houlsby G T 2009 Powder Technol. 36 953
[35] Podlozhnyuk A, Pirker S, Kloss C 2016 Comput. Part. Mech. 4 101
[36] Zhou Z Y, Zou R P, Pinson D, Yu A B 2011 Ind. Eng. Chem. Res. 50 9787
Google Scholar
[37] He S Y, Gan J Q, Pinson D, Zhou Z Y 2019 Powder Technol. 341 157
Google Scholar
[38] Goldman R 2005 Comput. Aided Geom. D 22 632
Google Scholar
[39] Lacey P M C 1954 J. Chem. Technol. Biot. 4 257
[40] Jiang M, Zhao Y, Liu G, Zheng J 2011 Particuology 9 270
Google Scholar
[41] He S, Gan J, Pinson D, Zhou Z 2017 EPJ Web of Conferences 140 06018
Google Scholar
[42] Donev A, Cisse I, Sachs D, Variano E A, Stillinger F H, Connelly R, Torquato S, Chaikin P M 2004 Science 303 990
Google Scholar
[43] Delaney G W, Cleary P W 2010 Europhys. Lett. 89 34002
Google Scholar
下载:
计量
- 文章访问数: 9933
- PDF下载量: 108
- 被引次数: 0
