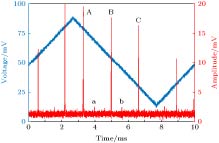
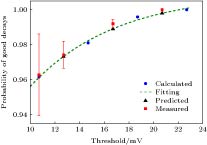
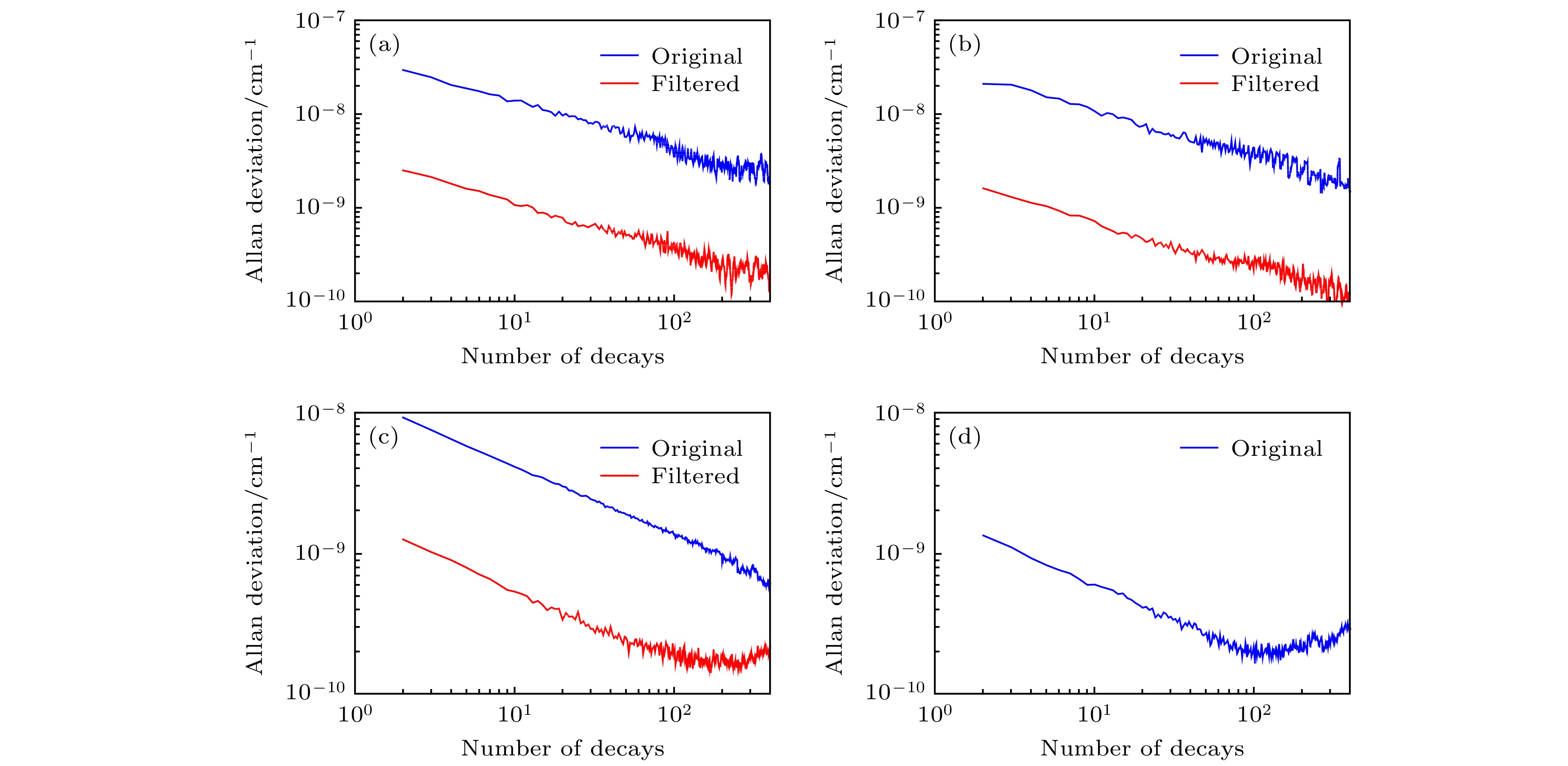
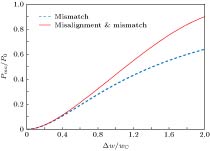
-
连续波腔衰荡光谱(CW-CRDS)测量中多模衰荡的产生是严重影响痕量气体测量灵敏度的重要因素. 本文针对衰荡腔内无光阑或光阑滤模不彻底的CRDS装置, 通过分析腔误调时的能量耦合规律以及受关断时间影响的衰荡过程, 提出阈值选择和拟合度判定两种非光阑模式筛选方法, 利用数值方法达到抑制多模衰荡及筛选基模衰荡(优衰荡)的目的. 首先对CW-CRDS实验中平均采样和单次采样模式下出现的多种衰荡类型进行了归纳分析, 发现可以通过单次采样数据预测多次采样的测量结果, 实验结果与预期一致. 解决了CRDS实验“平均”和“拟合”的先后顺序问题. 在此基础上, 利用优衰荡出现概率满足二项分布模型的特性, 建立了优衰荡出现频率随触发阈值变化的概率模型, 用于选择合适的触发阈值. 实验表明提升触发阈值可以有效地抑制多模衰荡, 使测量灵敏度提升约一个数量级. 随着触发阈值的提升, 通过优衰荡得到的Allan方差将趋于一个定值, 但是衰荡过程获取时间将逐渐延长. 因此, 在CW-CRDS检测中触发阈值应设置在保证全部衰荡过程均为优衰荡的最小阈值处. 之后, 采用拟合度判定法对实验数据进行了筛选. 最后给出了两种方法的适用范围, 拟合度判定法虽然简单但局限性较大, 阈值选择法可适用于腔误调程度不严重的情况.In continuous-wave cavity ring-down spectroscopy (CW-CRDS), the measurement sensitivity is seriously affected by the multimode excitations in the ring-down cavity. The using of an intracavity aperture is a common way to restrain the excitation of high-order modes, thus leading the laser power to additionally lose and the signal-to-noise ratio to degrade. In this paper, two numerical methods, named “trigger threshold method” and “curve fitness method”, are proposed for selecting the mode in which the decays excited by the high-order modes can be removed. The laser coupling efficiency between the incident laser and the oscillating fundamental or high-order modes is studied in a misadjusted ring-down cavity. It is found that with a misadjusted ring-down cavity, the laser energy is partially coupled into the high-order modes, and the coupling energy increases with the extent of the cavity misadjustment increasing. In this case, the ring-down decaying traces excited by these high-order modes are different from and much shorter than those excited by the fundamental mode, which are respectively called “bad decays” and “good decays” in this paper. Both the fundamental mode and the high-order modes can reach the threshold in the case of low triggering threshold selection and result in the components of both good and bad decays in the output ring-down curves. When the trigger threshold rises, the bad decays are effectively restrained by the deficient coupling into the high-order modes. Thus raising the trigger threshold is an effective method to restrain bad decays for the mode selection. Another approach is to consider the time spent on turning off the laser injection since the fitting goodness of good decays is better than that of bad decays. In this paper this characteristic is also used to separate the good decays from the bad ones. These two methods are demonstrated in the CW-CRDS experiments. The results show that the sensitivity of the CW-CRDS instrument can be greatly improved by one order of magnitude in the trigger threshold method with the minimum of Allan deviations gradually approaching to a constant, while the acquisition rate of the ring-down decays slows down with the increase of the trigger threshold. The results also explain the relationship between single sampling and averaged sampling, which presents an answer to the question about the sequence choice between averaging and fitting. A numerical model is proposed to estimate the probability of good decays versus the trigger threshold, which can be used to choose appropriate trigger threshold for CW-CRDS experiment. The applicable conditions and the limitations of these two methods in CW-CRDS for trace gas detection are also discussed in the paper.
-
Keywords:
- cavity ring-down spectroscopy /
- cavity misadjustment /
- multimode decay /
- measurement sensitivity
[1] McCarren D, Scime E 2015 Rev. Sci. Instrum. 86 103505
Google Scholar
[2] 胡纯栋, 焉镜洋, 王艳, 梁立振 2018 光谱学与光谱分析 38 346
Hu C D, Yan J Y, Wang Y, Liang L Z 2018 Spectrosc. Spect. Anal. 38 346
[3] Liu Y S, Zhou L X, Tans P P, Zang K P, Cheng S Y 2018 Sci. Total Environ. 633 1022
Google Scholar
[4] Miles N L, Martins D K, Richardson S J, Rella C W, Arata C, Lauvaux T, Davis K J, Barkley Z R, McKain K, Sweeney C 2018 Atmos. Meas. Tech. 11 1273
[5] Li Z Y, Hu R Z, Xie P H, Chen H, Wu S Y, Wang F Y, Wang Y H, Ling L Y, Liu J G, Liu W Q 2018 Opt. Express 26 A433
Google Scholar
[6] Tan Y, Wang J, Zhao X Q, Liu A W, Hu S M 2017 J. Quant. Spectosc. Radiat. Transf. 187 274
Google Scholar
[7] Chen J, Hua T P, Tao L G, Sun Y R, Liu A W, Hu S M 2018 J. Quant. Spectosc. Radiat. Transf. 205 91
Google Scholar
[8] Cui H, Li B C, Han Y L, Wang J, Gao C M, Wang Y F 2017 Chin. Opt. Lett. 15 053101
Google Scholar
[9] Cui H, Li B C, Xiao S L, Han Y L, Wang J, Gao C M, Wang Y F 2017 Opt. Express 25 5807
Google Scholar
[10] Wahl E H, Tan S M, Koulikov S, Kharlamov B, Rella C R, Crosson E R, Paldus B A 2006 Opt. Express 14 1673
Google Scholar
[11] Karpf A, Qiao Y, Rao G N 2016 Appl. Opt. 55 4497
Google Scholar
[12] Berden G, Peeters R, Meijer G 2000 Int. Rev. Phys. Chem. 19 565
Google Scholar
[13] O’Keefe A, Deacon D A G 1988 Rev. Sci. Instrum. 59 2544
Google Scholar
[14] Romanini D, Kachanov A A, Stoeckel F 1997 Chem. Phys. Lett. 270 538
Google Scholar
[15] Romanini D, Kachanov A A, Sadeghi N, Stoeckel F 1997 Chem. Phys. Lett. 264 316
Google Scholar
[16] Huang H F, Lehmann K K 2007 Opt. Express 15 8745
[17] 崔立红, 赵维宁, 颜昌翔 2015 64 224211
Cui L H, Zhao W N, Yan C X 2015 Acta Phys. Sin. 64 224211
[18] Lehmann K K, Huang H F 2009 Frontiers of Molecular Spectroscopy (Amsterdam: Elsevier Science) p638
[19] Grand Y L, Taché J P, Floch A L 1990 J. Opt. Soc. Am. B 7 1251
[20] Sayeh M R, Bilger H R, Habib T 1985 Appl. Opt. 24 3756
Google Scholar
[21] Wójtewicz S, Cygan A, Domyslawska J, Bielska K, Morzyński P, Maslowski P, Ciurylo R, Lisak D 2018 Opt. Express 26 5644
Google Scholar
[22] 谭中奇, 汪之国, 龙兴武 2007 光子学报 36 60
Tan Z Q, Wang Z G, Long X W 2007 Acta Photon. Sin. 36 60
[23] Wang J D, Yu J, Mo Z Q, He J G, Dai S J, Meng J J, Liu Y, Zhang X, Yi H 2019 Appl. Opt. 58 2773
Google Scholar
-
图 1 腔误调时高阶模功率耦合占比情况
Fig. 1. Proportion of higher-order cavity mode excitement in a misadjusted CRDS system.
图 2 受有限的关断时间影响的衰荡曲线仿真
Fig. 2. Simulation of ring-down curves affected by the finite shutdown time.
图 4 四次平均模式下的(a)衰荡曲线、(b), (c)衰荡时间及(d)拟合度分布
Fig. 4. (a) Typical decays, (b), (c) distributions of ring-down time, and (d) decay curve fitness in four times averaged mode sampling.
图 7 优衰荡出现频率随触发阈值的变化
Fig. 7. Variance of the frequency of good decays with trigger thresholds.
图 8 不同阈值单次采样下的Allan方差图 (a) 10.7 mV; (b) 14.7 mV; (c) 18.7 mV; (d) 22.7 mV
Fig. 8. Allan deviations in single sampling with different trigger thresholds: (a) 10.7 mV; (b) 14.7 mV; (c) 18.7 mV; (d) 22.7 mV
图 9 不同阈值下40个衰荡过程的Allan方差
Fig. 9. Allan deviations of 40 decays with different trigger thresholds.
图 10 不同阈值的噪声等效吸收系数及测量灵敏度
Fig. 10. Noise equivalent absorption coefficients and sensitivities under different trigger thresholds.
图 11 单次采样模式下的衰荡时间(a)及曲线调整拟合度(b)分布
Fig. 11. Distributions of ring-down times (a) and curve fitness (b) in single sampling.
-
[1] McCarren D, Scime E 2015 Rev. Sci. Instrum. 86 103505
Google Scholar
[2] 胡纯栋, 焉镜洋, 王艳, 梁立振 2018 光谱学与光谱分析 38 346
Hu C D, Yan J Y, Wang Y, Liang L Z 2018 Spectrosc. Spect. Anal. 38 346
[3] Liu Y S, Zhou L X, Tans P P, Zang K P, Cheng S Y 2018 Sci. Total Environ. 633 1022
Google Scholar
[4] Miles N L, Martins D K, Richardson S J, Rella C W, Arata C, Lauvaux T, Davis K J, Barkley Z R, McKain K, Sweeney C 2018 Atmos. Meas. Tech. 11 1273
[5] Li Z Y, Hu R Z, Xie P H, Chen H, Wu S Y, Wang F Y, Wang Y H, Ling L Y, Liu J G, Liu W Q 2018 Opt. Express 26 A433
Google Scholar
[6] Tan Y, Wang J, Zhao X Q, Liu A W, Hu S M 2017 J. Quant. Spectosc. Radiat. Transf. 187 274
Google Scholar
[7] Chen J, Hua T P, Tao L G, Sun Y R, Liu A W, Hu S M 2018 J. Quant. Spectosc. Radiat. Transf. 205 91
Google Scholar
[8] Cui H, Li B C, Han Y L, Wang J, Gao C M, Wang Y F 2017 Chin. Opt. Lett. 15 053101
Google Scholar
[9] Cui H, Li B C, Xiao S L, Han Y L, Wang J, Gao C M, Wang Y F 2017 Opt. Express 25 5807
Google Scholar
[10] Wahl E H, Tan S M, Koulikov S, Kharlamov B, Rella C R, Crosson E R, Paldus B A 2006 Opt. Express 14 1673
Google Scholar
[11] Karpf A, Qiao Y, Rao G N 2016 Appl. Opt. 55 4497
Google Scholar
[12] Berden G, Peeters R, Meijer G 2000 Int. Rev. Phys. Chem. 19 565
Google Scholar
[13] O’Keefe A, Deacon D A G 1988 Rev. Sci. Instrum. 59 2544
Google Scholar
[14] Romanini D, Kachanov A A, Stoeckel F 1997 Chem. Phys. Lett. 270 538
Google Scholar
[15] Romanini D, Kachanov A A, Sadeghi N, Stoeckel F 1997 Chem. Phys. Lett. 264 316
Google Scholar
[16] Huang H F, Lehmann K K 2007 Opt. Express 15 8745
[17] 崔立红, 赵维宁, 颜昌翔 2015 64 224211
Cui L H, Zhao W N, Yan C X 2015 Acta Phys. Sin. 64 224211
[18] Lehmann K K, Huang H F 2009 Frontiers of Molecular Spectroscopy (Amsterdam: Elsevier Science) p638
[19] Grand Y L, Taché J P, Floch A L 1990 J. Opt. Soc. Am. B 7 1251
[20] Sayeh M R, Bilger H R, Habib T 1985 Appl. Opt. 24 3756
Google Scholar
[21] Wójtewicz S, Cygan A, Domyslawska J, Bielska K, Morzyński P, Maslowski P, Ciurylo R, Lisak D 2018 Opt. Express 26 5644
Google Scholar
[22] 谭中奇, 汪之国, 龙兴武 2007 光子学报 36 60
Tan Z Q, Wang Z G, Long X W 2007 Acta Photon. Sin. 36 60
[23] Wang J D, Yu J, Mo Z Q, He J G, Dai S J, Meng J J, Liu Y, Zhang X, Yi H 2019 Appl. Opt. 58 2773
Google Scholar
下载:
计量
- 文章访问数: 7223
- PDF下载量: 107
- 被引次数: 0
