-
分子强场近似(SFA)理论虽然在描述强激光场中分子的超快动力学方面取得了巨大的成功, 但是理论本身存在关键的矛盾. 一方面SFA基本思想要求初态为无场下的系统本征态, 另一方面物理过程的空间平移不变性要求系统初态应当为激光场缀饰态, 这两个相互矛盾的要求分别对应非缀饰态和缀饰态两种形式的分子SFA理论, 两种理论的有效性和适用条件存在广泛的争议. 本文中, 我们对(椭)圆偏振激光场中N2和Ne2分子的电离过程进行了研究, 期望能对上述争议给出解答. 椭圆偏振光能有效抑制再散射过程及各种干涉效应的影响, 使得电离过程更加干净, 因此可以有效甄别缀饰态和非缀饰态的适用条件. 我们采用强场近似方法(SFA)及库仑修正强场近似方法(CCSFA)计算了缀饰态和非缀饰态下不同分子轨道对应的光电子动量分布, 并与已有的实验结果进行了对比. 我们发现, 对于Ne2这样核间距较大的分子, 必须采用缀饰态才能准确地描述其电离特征; 而对于N2这样核间距较小的分子, 缀饰态描述则不适用. 本文的结论为准确描述激光诱导分子超快过程及相应理论的进一步发展提供了参考.Despite the molecular strong-field approximation (SFA) theory has made remarkable achievements in describing the ultrafast dynamics of molecules in intense laser fields, there are basic inconsistencies in the theory itself. On the one hand, the basic principle of SFA requires that the initial state be an eigenstate of the system in the absence of the field, and on the other hand, the spatial translation invariance of the physical process requires that the initial state of the system be a laser-field-dressed state. These two conflicting requirements correspond to the two forms of molecular SFA theories, namely, the undressed state and the dressed state. The two theoretical validity and applicability conditions are widely disputed. In this paper, we investigate the ionization processes of N2 and Ne2 molecules in an elliptically polarized laser field and a circularly polarized laser field, aiming to solve the above-mentioned controversies. Elliptically polarized laser can efficiently suppress the re-scattering process and the influence of various interference effects, which makes the ionization process cleaner, and thus can effectively screen the applicable conditions for the dressed and undressed states. We calculate the photoelectron momentum distributions corresponding to different molecular orbitals in the dressed and undressed states by using the strong-field approximation (SFA) and the Coulomb-corrected strong-field approximation (CCSFA) and compare them with previous experimental results. For molecules with large nuclear spacing such as Ne2, we find that the dressed state is necessary to accurately characterise their ionization, however, for molecules with small nuclear spacing such as N2, the dressed state description is inapplicable. The conclusions of this work provide a reference for accurately describing laser-induced molecular ultrafast processes and further developing corresponding theories and molecular ultrafast imaging schemes.
-
Keywords:
- elliptically polarized laser field /
- molecule ionization /
- dressing /
- PMD
-
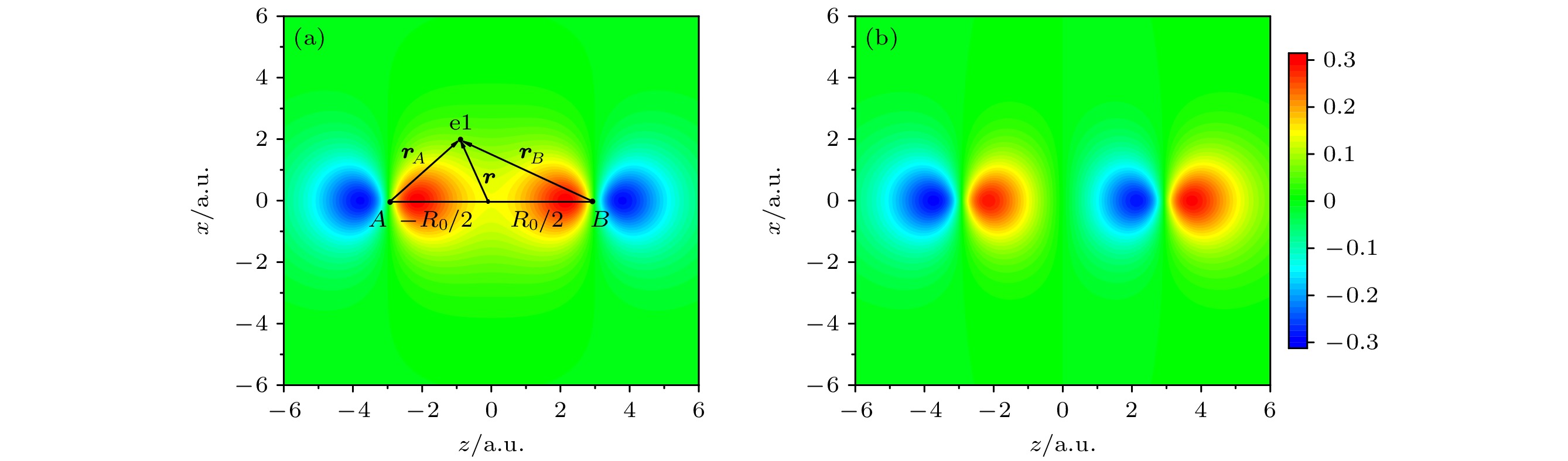
图 1 (a) 和 (b) 分别为Ne2的2p$ \sigma_g $和2p$ \sigma_u $轨道坐标空间波函数的二维分布. (a) 中的点A和点B分别表示两个原子中心的位置, 它们之间的距离标记为$ R_{0} $. 电子与原子中心A的相对位置矢量为$ {\boldsymbol{r}}_{A} $, 与原子中心B的相对位置矢量为$ {\boldsymbol{r}}_{B} $
Fig. 1. (a) and (b) represent two-dimensional distributions spatial wave functions of the 2p$ \sigma_g $ and 2p$ \sigma_u $ orbitals for Ne2 respectively. (a) shows the positions of the two atomic centres, A and B, with the distance between them marked as $ R_0 $. The electronic relative position vector to atomic centre A is denoted by $ {\boldsymbol{r}}_{A} $, and the relative position vector to atomic centre B is denoted by $ {\boldsymbol{r}}_{B} $
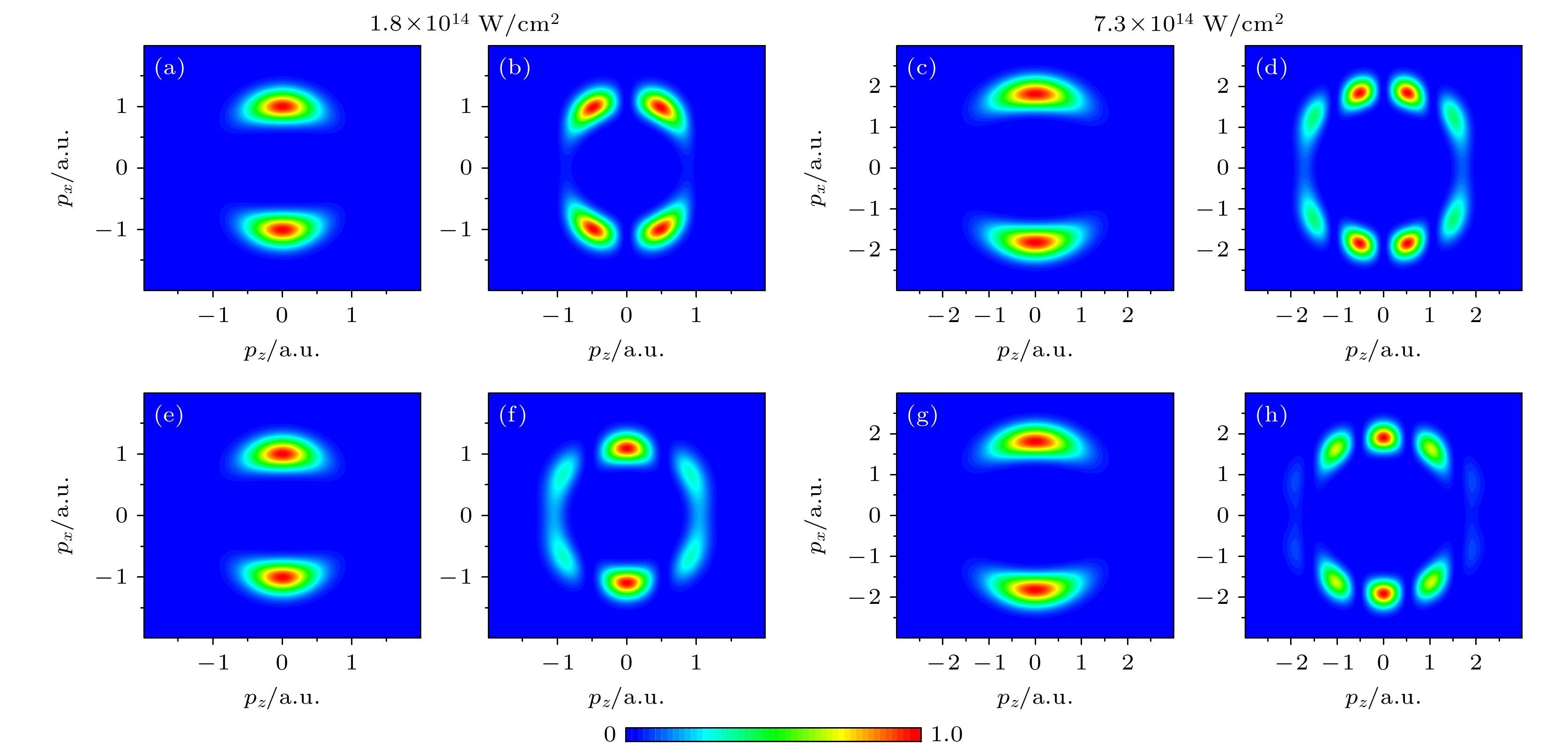
图 2 用SFA方法计算的Ne2在圆偏振光下的对称轨道2p$ \sigma_g $ (第一行)和 反对称轨道2p$ \sigma_u $ (第二行) 的光电子动量分布(PMD), 其中包括了非缀饰态(a), (c), (e), (g)和缀饰态(b), (d), (f), (h). 计算中分子轴沿着z轴方向排列, Ne-Ne键长为5.86 a.u., 左侧一组图(a), (b), (e), (f)和右侧一组图(c), (d), (g), (h)的激光峰值光强分别为$ 1.8\times10^{14}\; {\mathrm{W/cm}}^{2} $和$ 7.3\times10^{14}\; {\mathrm{W/cm}}^{2} $, 波长均为780 nm
Fig. 2. Photoelectron momentum distributions (PMD) calculated by the SFA method for the symmetric orbitals 2p$ \sigma_g $ (the first row) and antisymmetric orbitals 2p$ \sigma_u $ (the second row) in circularly polarized light for Ne2, which includes both the dressing state (a), (c), (e), (g) and the undressing state (b), (d), (f), (h). The molecular axes are aligned along the z-axis, the Ne-Ne bond length is 5.86 a.u.. The left set of panels has the peak laser intensity is $ 1.8\times10^{14}\; {\mathrm{W/cm}}^{2} $, while the right set of panels is $ 7.3\times10^{14}\; {\mathrm{W/cm}}^{2} $, the wavelength of both is 780 nm.
图 3 SFA方法中考虑不同项计算得到的Ne2在圆偏振光下对称性轨道2p$ \sigma_{\rm{g}} $ 的光电子动量分布. (a)公式(5)中不考虑$ V_{p_0} $时的PMD; (b)$ V_{p_0} $中不考虑分子干涉项时的PMD; (c)$ V_{p_0} $中只考虑非缀饰态干涉项时的PMD; (d)$ V_{p_0} $中只考虑缀饰态干涉项时的PMD. 激光峰值光强为$ 7.3\times10^{14} \;{\mathrm{W/cm}}^{2} $, 波长为780 nm. 具体细节请参考文中表述
Fig. 3. The PMD of the symmetry orbital 2p$ \sigma_{\rm{g}} $ of Ne2 in circularly polarized light calculated by considering different terms in the SFA method. (a) The PMD obtained when $ V_{p_0} $ is not considered in Eq. (5). (b) The PMD obtained when $ V_{p_0} $ is included but the molecular interference term is removed; (c) The PMD with only the undressed state interference term considered in $ V_{p_0} $; (d) The PMD with only the dressed state interference term considered in $ V_{p_0} $. The peak laser intensity is $ 7.3\times10^{14}\; {\mathrm{W/cm}}^{2} $, the wavelength is 780 nm. For details, please refer to the text.
图 4 缀饰态下的Ne2对称2p$ \sigma_{\rm{g}} $ (a)和反对称2p$ \sigma_{\rm{u}} $(b)轨道的电离率随$ p_{z} $的分布, 即将图2(d)和(h)中分布的$ p_{x} $方向动量积分
Fig. 4. The distribution of the ionization rates with $ p_{z} $ for the symmetric 2p$ \sigma_g $ (a) and antisymmetric 2p$ \sigma_u $ (b) orbitals of Ne2 in the dressed state, i.e., integrating the x-direction momentum distributions in Figs. 2(d) and (h).
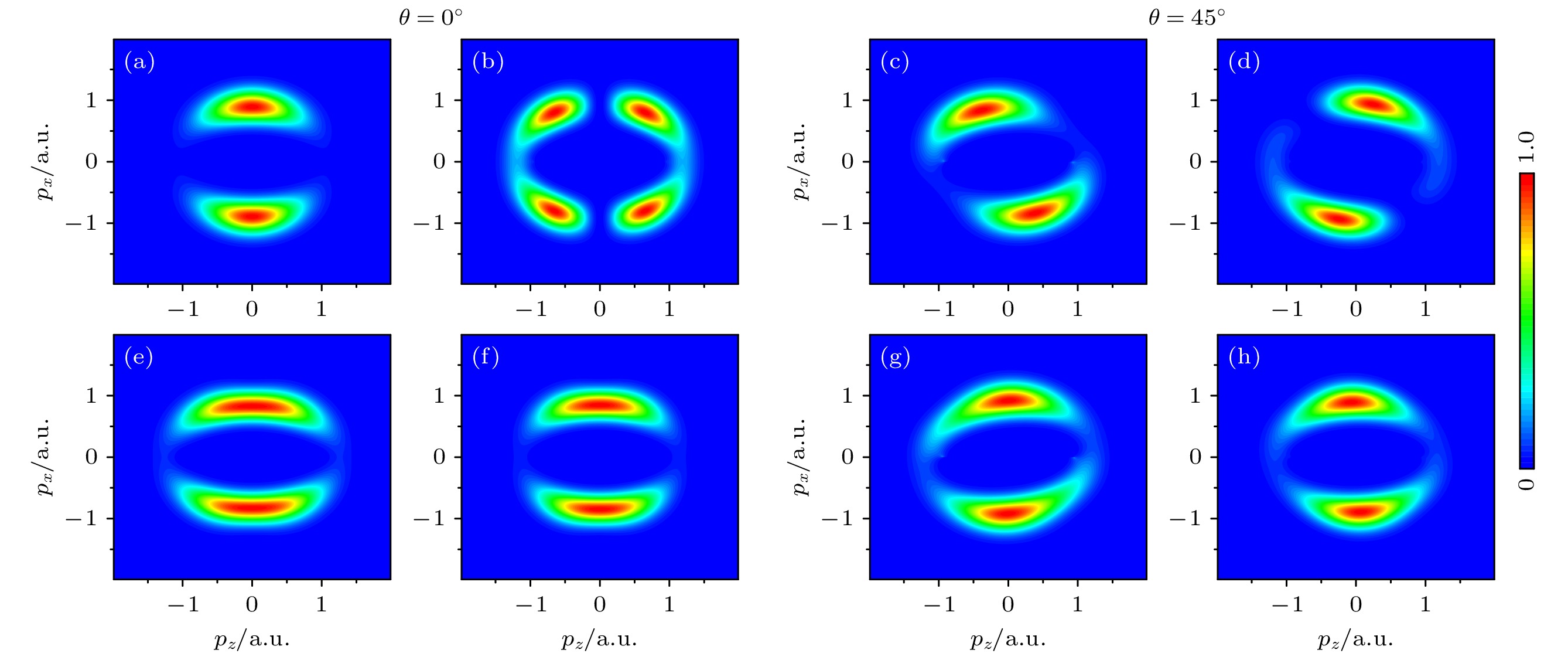
图 5 SFA方法计算的N2在椭圆偏振光下HOMO (a), (b), (c), (d) 和HOMO-1 (e), (f), (g), (h) 的光电子动量分布, 其中(a), (c), (e), (g)是非缀饰态的情况, (b), (d), (f), (h)是缀饰态的情况. 左侧一组图和右侧一组图的准直角分别为$ 0^\circ $和$ 45^\circ $. 分子轴沿着z轴方向排列, 准直角被定义为分子轴与激光场长轴方向之间的夹角. N-N键长为2.073 a.u., 激光场峰值光强是$ 1.8\times $$ 10^{14}\;{\mathrm{ W/cm}}^{2} $, 波长是800 nm, 椭偏率是0.82
Fig. 5. The PMD of N2 in elliptical laser fields for HOMO (a), (b), (c), (d) and HOMO-1 (e), (f), (g), (h) calculated by the SFA method, which (a), (c), (e), (g) are the case of the undressed state, and (b), (d), (f), (h) are the cases of the dressed state. The left set of panels and the right set of panels are aligned at angles $ 0^\circ $ and $ 45^\circ $, respectively. The molecular axes are aligned along the z-axis direction, the alignment angle is defined as the angle between the molecular axis and the main axis of the laser field. The N-N bond length is 2.073 a.u., the peak laser intensity is $ 1.8 \times10^{14}\;{\mathrm{ W/cm}}^{2} $, the wavelength is 800 nm, and the ellipticity is 0.82.
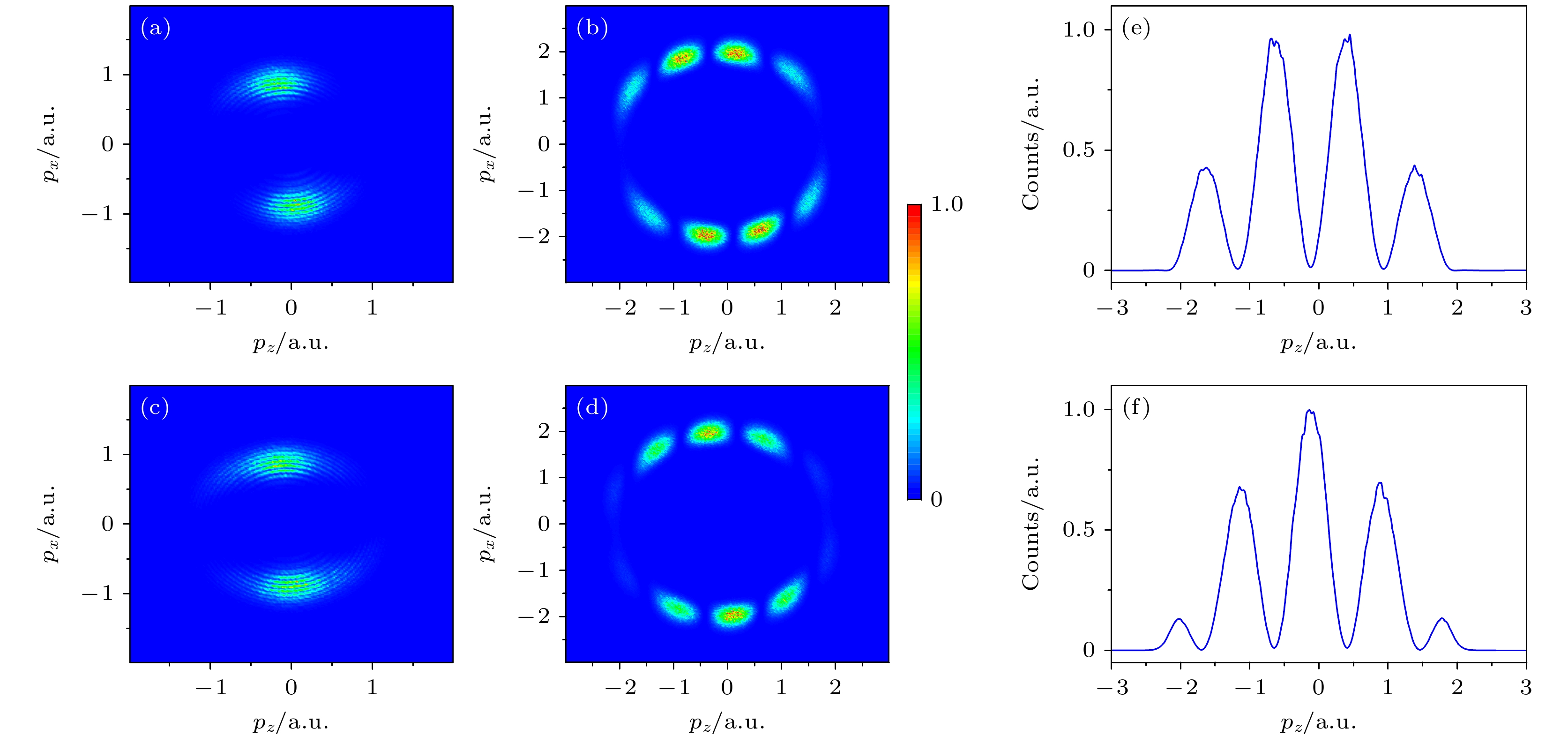
图 6 库仑修正强场近似(CCSFA)方法计算的N2和Ne2在(椭)圆偏振光 下不同轨道的光电子动量分布. (a)和(c)分别是N2在非缀饰态下的HOMO和HOMO-1的动量分布, 激光峰值光强为$ 1.8\times10^{14}\;{\rm{W}}/{\rm{cm}}^{2} $, 波长为800 nm, 椭偏率为0.82. (b)和(d)分别是Ne2在缀饰态下的对称性$ \sigma_{\rm{g}} $ 轨道和反对称性$ \sigma_{\rm{u}} $轨道的动量分布, 激光峰值光强为$ 7.3\times10^{14}\;{\rm{W}}/{\rm{cm}}^{2} $, 波长为780 nm, 椭偏率为1. (e)和(f)中分别展示了将(b) 和(d)中的分布旋转一定角度并将$ p_{x} $方向动量积分后的结果. 具体细节参考文章表述
Fig. 6. Photoelectron momentum distributions of different orbitals in (elliptical) circular polarized light for N2 and Ne2 calculated by the CCSFA. (a) and (c) are the momentum distributions of HOMO and HOMO-1 for N2 in the undressing state, the peak laser intensity is $ 1.8 \times10^{14}\; {\rm{W}}/{\rm{cm}}^{2} $, the wavelength is 800 nm, and the ellipticity is 0.82. (b) and (d) are the momentum distributions of symmetric $ \sigma_{\rm{g}} $ orbital and antisymmetric $ \sigma_{\rm{u}} $ orbital for Ne2 in the dressing state, respectively, the peak laser intensity is $ 7.3 \times10^{14}\; {\rm{W}}/{\rm{cm}}^{2} $, the wavelength is 780 nm, and the ellipticity is 1. (e) and (f) show the results after rotating the distributions in (b) and (d) by a given angle and integrating the momentum in the $ p_{x} $ direction, respectively. See the text for more details.
-
[1] Rost J M, Saalmann U 2019 Nat. Photon. 13 439
Google Scholar
[2] Blaga C I, Xu J L, DiChiara A D, Sistrunk E, Zhang K, Agostini P, Miller T A, DiMauro L F, Lin C D 2012 Nature 483 194
Google Scholar
[3] Niikura H, Légaré F, Hasbani R, Bandrauk A D, Ivanov M Y, Villeneuve D M, Corkum P B 2002 Nature 417 917
Google Scholar
[4] Niikura H, Légaré F, Hasbani R, Ivanov M Y, Villeneuve D M, Corkum P B 2003 Nature 421 826
Google Scholar
[5] Uiberacker M, Uphues T, Schultze M, Verhoef A J, Yakovlev V, Kling M F, Rauschenberger J, Kabachnik N M, Schröder H, Lezius M, Kompa K L, Muller H G, Vrakking M J J, Hendel S, Kleineberg U, Heinzmann U, Drescher M, Krausz F 2007 Nature 446 627
Google Scholar
[6] Eckle P, Smolarski M, Schlup P, Biegert J, Staudte A, Schöffler M, Muller H G, Dörner R, Keller U 2008 Nat. Phys. 4 565
Google Scholar
[7] Faisal F H 1973 J. Phys. B: Atom. Mol. Phys. 6 L89
Google Scholar
[8] Reiss H R 1980 Phys. Rev. A 22 1786
Google Scholar
[9] Lewenstein M, Balcou P, Ivanov M Y, L'huillier A, Corkum P B 1994 Phys. Rev. A 49 2117
Google Scholar
[10] Muth-Böhm J, Becker A, Faisal F 2000 Phys. Rev. Lett. 85 2280
Google Scholar
[11] Kjeldsen T K, Madsen L B 2004 J. Phys. B: At. Mol. Opt. Phys. 37 2033
Google Scholar
[12] Milošević D, Paulus G, Bauer D, Becker W 2006 J. Phys. B: At. Mol. Opt. Phys. 39 R203
Google Scholar
[13] 刘希望, 张宏丹, 贲帅, 杨士栋, 任鑫, 宋晓红, 杨玮枫 2023 72 198701
Google Scholar
Liu X W, Zhang H D, Ben S, Yang S D, Ren X, Song X H, Yang W F 2023 Acta. Phys. Sin. 72 198701
Google Scholar
[14] Xu J Y, Guo L, Qi X, Lu R H, Zhang M, Zhang J T, Chen J 2024 Chin. Phys. B 33 093301
Google Scholar
[15] Yan T M, Popruzhenko S, Vrakking M, Bauer D 2010 Phys. Rev. Lett. 105 253002
Google Scholar
[16] Wang C, Okunishi M, Hao X, Ito Y, Chen J, Yang Y, Lucchese R, Zhang M, Yan B, Li W, Ding D, Ue da, K 2016 Phys. Rev. A 93 043422
Google Scholar
[17] Yang Y Z, Ren H, Zhang M, Zhou S P, Mu X X, Li X K, Wang Z Z, Deng K, Li M X, Ma P, Li Z, Hao X L, Li W D, Chen J, Wang C C, Ding D J 2023 Nat. Commun. 14 4951
Google Scholar
[18] Milošević D 2006 Phys. Rev. A 74 063404
Google Scholar
[19] Becker W, Chen J, Chen S G, Milošević D 2007 Phys. Rev. A 76 033403
Google Scholar
[20] Pfeiffer A N, Cirelli C, Smolarski M, Dimitrovski D, Abu-Samha M, Madsen L B, Keller U 2012 Nat. Phys. 8 76
Google Scholar
[21] Shafir D, Soifer H, Bruner B D, Dagan M, Mairesse Y, Patchkovskii S, Ivanov M Y, Smirnova O, Dudovich N 2012 Nature 485 343
Google Scholar
[22] Yu M, Liu K, Li M, Yan J Q, Cao C P, Tan J, Liang J T, Guo K Y, Cao W, Lan P F, Zhang Q B, Zhou Y M, Lu P X 2022 Light: Sci. Appl. 11 215
Google Scholar
[23] Wu J, Magrakvelidze M, Schmidt L P H, Kunitski M, Pfeifer T, Schöffler M, Pitzer M, Richter M, Voss S, Sann H, Kim H, Lower J, Jahnke T, Czasch A, Thumm U, Dörner R 2013 Nat. Commun. 4 2177
Google Scholar
[24] Serov V V, Bray A W, Kheifets A S 2019 Phys. Rev. A 99 063428
Google Scholar
[25] Quan W, Serov V V, Wei M Z, Zhao M, Zhou Y, Wang Y L, Lai X Y, Kheifets A S, Liu X J 2019 Phys. Rev. Lett. 123 223204
Google Scholar
[26] Khan A, Trabert D, Eckart S, Kunitski M, Jahnke T, Dörner R 2020 Phys. Rev. A 101 023409
Google Scholar
[27] Korneev P A, Popruzhenko S, Goreslavski S, Yan T M, Bauer D, Becker W, Kübel M, Kling M F, Rödel C, Wünsche M, Paulus G G 2012 Phys. Rev. Lett. 108 223601
Google Scholar
[28] Pruzhenko S V, Korneev P A, Goreslavski S, Becker W 2002 Phys. Rev. Lett. 89 023001
Google Scholar
[29] Hao X L, Chen J, Li W D, Wang B B, Wang X D, Becker W 2014 Phys. Rev. Lett. 112 073002
Google Scholar
[30] Maxwell A S, Faria C F d M 2016 Phys. Rev. Lett. 116 143001
Google Scholar
[31] Quan W, Hao X L, Wang Y L, Chen Y J, Yu S G, Xu S P, Xiao Z L, Sun R P, Lai X Y, Hu S L, Liu M Q, Shu Z, Wang X D, Li W D, Becker W, Liu X J, Chen J 2017 Phys. Rev. A 96 032511
Google Scholar
[32] Busuladžić M, Gazibegović-Busuladžić A, Miložević D, Becker W 2008 Phys. Rev. A 78 033412
Google Scholar
[33] Busuladžić M, Milošević D 2010 Phys. Rev. A 82 015401
Google Scholar
[34] Lewenstein M, Kulander K, Schafer K, Bucksbaum P 1995 Phys. Rev. A 51 1495
Google Scholar
[35] de Morisson Faria C F, Schomerus H, Becker W 2002 Phys. Rev. A 66 043413
Google Scholar
[36] Usachenko V I, Chu S I 2005 Phys. Rev. A 71 063410
Google Scholar
[37] Kunitski M, Eicke N, Huber P, Köhler J, Zeller S, Voigtsberger J, Schlott N, Henrichs K, Sann H, Trinter F, Schmidt L P H, Kalinin A, Schöffler M S, Jahnke T, Lein M, Dörner R 2019 Nat. Commun. 10 1
Google Scholar
[38] Guo Z N, Liu Y Q 2020 J. Phys. B: At. Mol. Opt. Phys. 53 065004
Google Scholar
[39] Yan J Q, Xie W H, Li M, Liu K, Luo S Q, Cao C P, Guo K Y, Cao W, Lan P F, Zhang Q B, Zhou Y M, Lu P X 2020 Phys. Rev. A 102 013117
Google Scholar
下载:
计量
- 文章访问数: 220
- PDF下载量: 16
- 被引次数: 0
