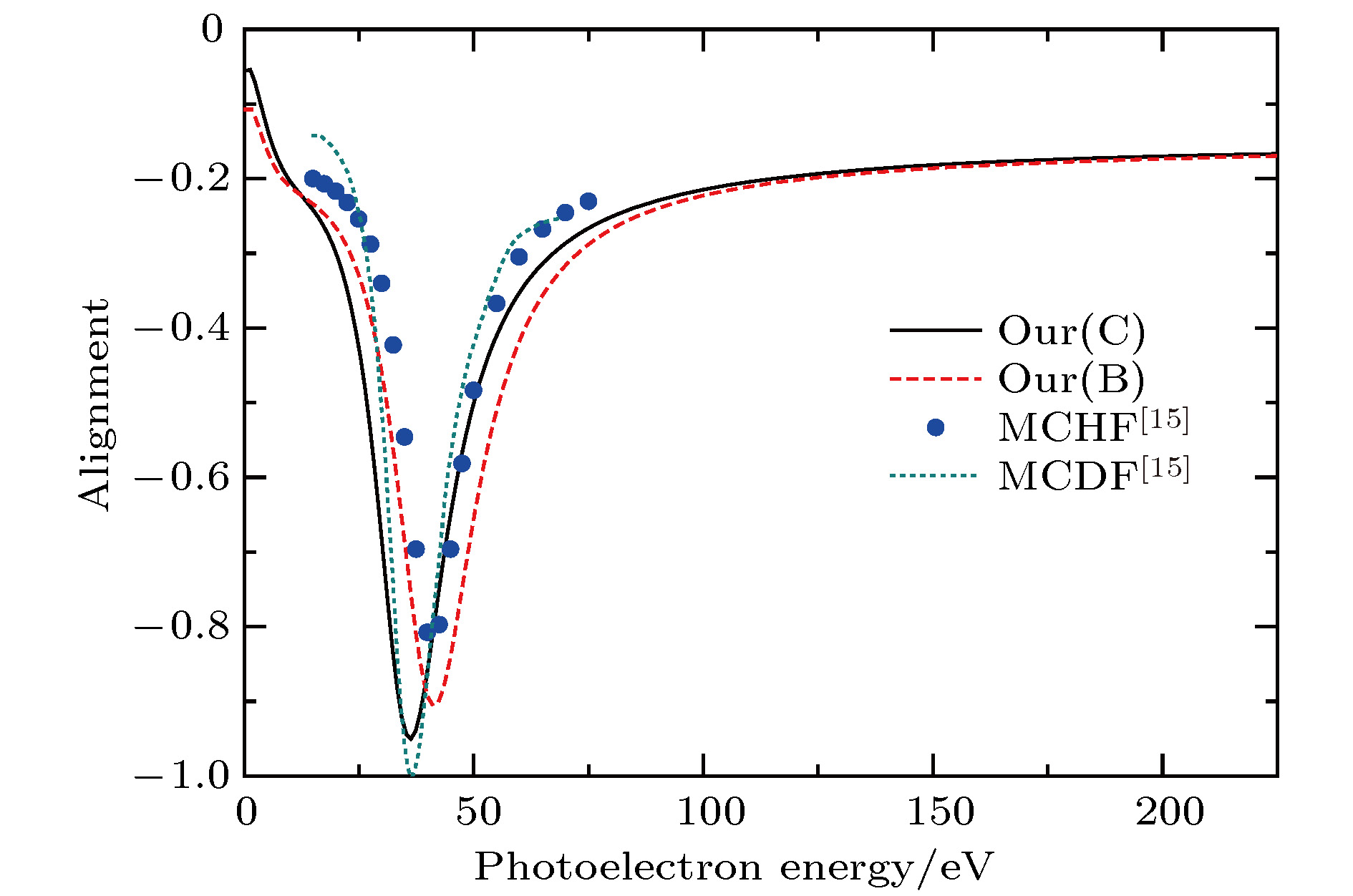
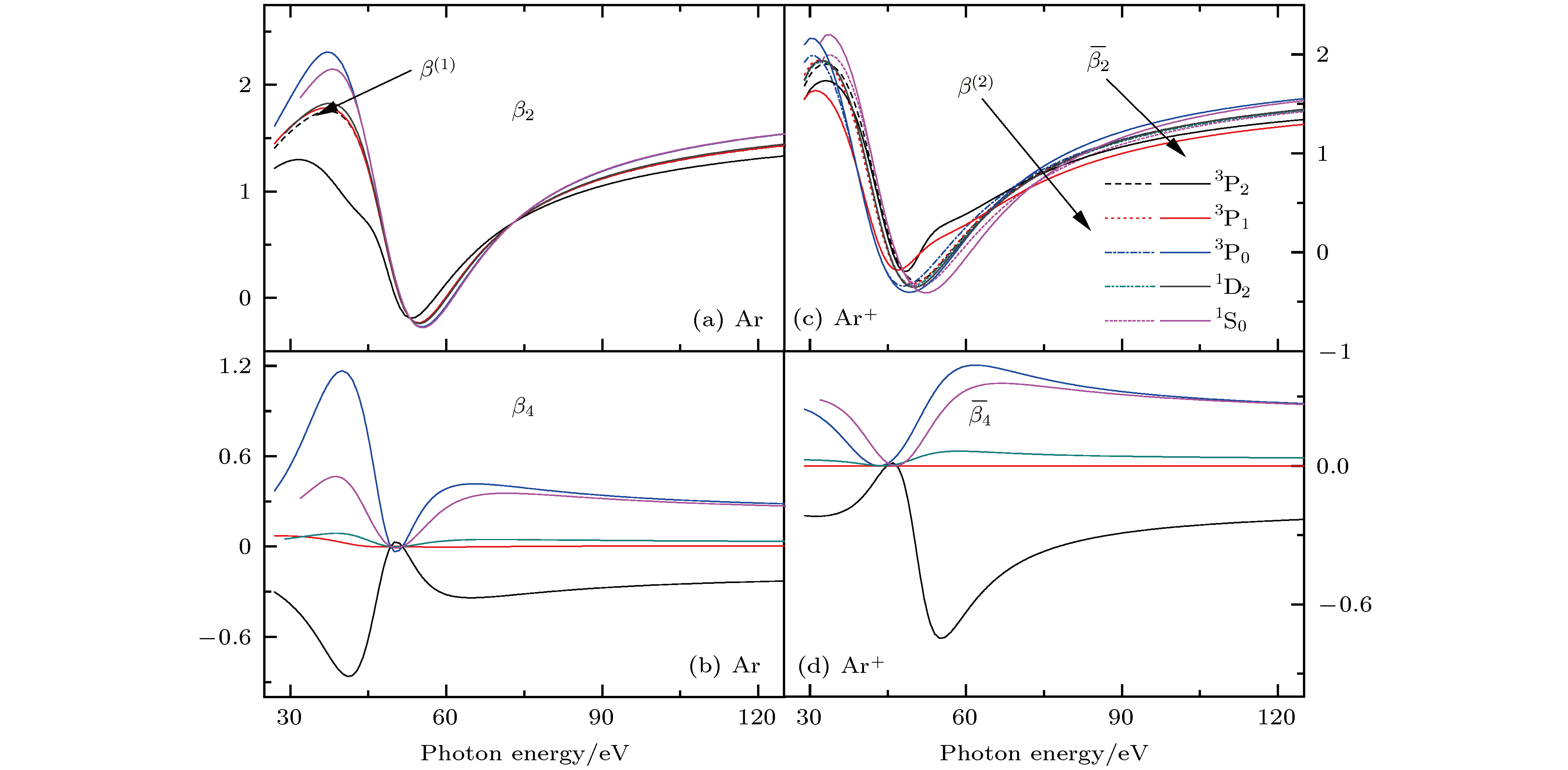
-
基于多组态Dirc-Fock方法和密度矩阵理论, 给出了原子序列双光双电离光电子角分布的计算表达式, 发展了相应的计算程序. 利用该程序对Ar原子3p壳层序列双光双电离过程进行了理论研究, 给出了光电离的总截面、磁截面、剩余离子取向以及光电子角分布的各向异性参数与入射光子能量的函数关系. 结果显示在光电离截面的Cooper极小位置附近取向参数出现极大值, 而光电子角分布的各向异性参数在该位置附近出现极小值. 进一步给出了33.94和55.34 eV光子能量下序列双光双电离过程中第一步的Ar原子和第二步的Ar+离子3p壳层光电子角分布, 分析了序列双光双电离光电子角分布与单光电离光电子角分布的差异. 将计算结果与文献已有的数据进行了比较, 具有很好的一致性. 本文的研究结果对揭示光与物质相互作用的非线性动力学机制具有重要的参考价值.With the development of the intense light source, such as free electron lasers, the experiments on the nonlinear process in atomic photo absorption in the XUV and X-ray region became more and more feasible. As one of the simplest possible nonlinear processes, the sequential two-photon double ionization, in which the first photon produces an ion which is subsequently ionized by the second photon, attracts increasing attention of theorists and experimentalists. Study on the angular distributions and angular correlations of the photoelectrons in the sequential two-photon double ionization process are especially attractive, which provides valuable information about the electronic structure of atom or molecule systems and allows the obtaining of additional information about mechanism and pathway of the two-photon double ionization. In this paper, the expression for the photoelectron angular distribution in a sequential two-photon process is given based on the multi-configuration Dirac-Fock method and the density matrix theory. And then, the relativistic calculation program for photoelectron angular distribution is further developed with the help of the program packages GRASP2K and RATIP which are based on the multi-configuration Dirac-Fock method. By using this code, the sequential two-photon double ionization of the 3p shell in atomic argon is studied theoretically. The cross section, magnetic cross section, alignment of residual ions and the asymmetry parameter of the photoelectron angular distribution, each as a function of photon energy, for the first and the second step of sequential two-photon double ionization of argon are presented. The calculations predict that the alignment has a maximum value and the asymmetry parameter has a minimum value in the region of the cooper minimum. The angular distribution of the first step ionization for Ar atom and the second step ionization for Ar+ ion are given at 33.94 eV and 55.34 eV photon energy, respectively. In addition, the difference in property between the angular distributions of the first photoelectron in sequential two-photon double ionization and in conventional one-photon single ionization is discussed. The present calculated results are compared with other available results, showing that they are in good agreement with each other. The results of this paper will be helpful in studying nonlinear processes in the XUV range.
-
Keywords:
- sequential two-photon double ionization /
- angular distribution of photoelectron /
- argon atom
[1] Bachau H, Lambropoulos P 1991 Phys. Rev. A 44 R9(R)
[2] Laulan S, Bachau H 2003 Phys. Rev. A 68 013409
Google Scholar
[3] Böhme D K 2011 Phys. Chem. Chem. Phys. 13 18253
Google Scholar
[4] Thissen R, Witasse O, Dutuit O, Wedlund C S, Gronoff G, Lilensten J 2011 Phys. Chem. Chem. Phys. 13 18264
Google Scholar
[5] Gillaspy J D, Pomeroy J M, Perrella A C, Grube H 2007 J. Phys. Conf. Ser. 58 451
Google Scholar
[6] McNeil B W J, Thompson N R 2010 Nat. Photon. 4 814
Google Scholar
[7] Wabnitz H, Bittner L, de Castro A R B, et al. 2002 Nature 420 482
Google Scholar
[8] Young L, Kanter E P, Krässig B, et al. 2010 Nature 466 56
Google Scholar
[9] Braune M, Reinköster A, Viefhaus J, et al. 2007 XXV Int. Conf. on Photonic, Electronic and Atomic Collisions (ICPEAC), Freiburg, Germany, July 25–31, 2007 p34
[10] Kurka M, Rudenko A, Foucar, et al. 2009 J. Phys. B: At. Mol. Opt. Phys. 42 141002
Google Scholar
[11] Braune M, Hartmann G, Ilchen M, et al. 2016 J. Mod. Opt. 63 324
Google Scholar
[12] Augustin S, Schulz M, Schmid G, et al. 2018 Phys. Rev. A 98 033408
Google Scholar
[13] Ilchen M, Hartmann G, Gryzlova E V, et al. 2018 Nat. Commun. 9 4659
Google Scholar
[14] Carpeggiani P A, Gryzlova E V, Reduzzi M, et al. 2019 Nat. Phys. 15 170
Google Scholar
[15] Fritzsche S, Grum-Grzhimailo A N, Gryzlova E V, Kabachnik N M 2008 J. Phys. B: At. Mol. Opt. Phys. 41 165601
Google Scholar
[16] Gryzlova E V, Grum-GrzhimailoA N, Kuzmina E I, Strakhova S I 2014 J. Phys. B: At. Mol. Opt. Phys. 47 195601
Google Scholar
[17] Grum-Grzhimailo A N, Gryzlova E V 2014 Phys. Rev. A 89 043424
Google Scholar
[18] Grum-Grzhimailo A N, Gryzlova E V, Fritzsche S, Kabachnik N M 2016 J. Mod. Opt. 63 334
Google Scholar
[19] Gryzlova E V, Grum-Grzhimailo A N, Staroselskaya E I, Strakhova S I 2015 J. Electron Spectrosc. Relat. Phenom. 204 277
Google Scholar
[20] Ma K, Xie L Y, Zhang D H, Dong C Z 2015 Chin. Phys. B 24 073402
Google Scholar
[21] Ma K, Chen Z B, Xie L Y, Dong C Z 2018 J. Phys. B: At. Mol. Opt. Phys. 51 045203
Google Scholar
[22] Ma K, Chen Z B, Xie L Y, Dong C Z, Qu Y Z 2017 J. Phys. B: At. Mol. Opt. Phys. 50 225202
Google Scholar
[23] Ma K, Chen Z B, Xie L Y, Ding X B, Zhang D H, Dong C Z 2018 J. Electron Spectrosc. Relat. Phenom. 228 1
Google Scholar
[24] Blum K 1996 Density Matrix Theory and Applications (2nd Ed.) (New York: Plenum) pp35–60
[25] Balashov V V, Grum-Grzhimailo A N, Kabachnik N M 2000 Polarization and Correlation Phenomena in Atomic Collisions (New York: Kluwer Academic/Plenum) pp76–97
[26] Jönsson P, Gaigalas G, Bieroń J, Fischer C F, Grant I P 2013 Comput. Phys. Commun. 184 2197
Google Scholar
[27] Fritzsche S 2012 Comput. Phys. Commun. 183 1525
Google Scholar
[28] Langer B 1992 ZurEnergieabhängigkeit von Photoelektronen- satelliten (Studies of Vacuum Ultraviolet and X-ray Processes (Vol. 2) (New York: AMS Press) p145
-
图 1 Ar原子序列双光双电离截面
Fig. 1. The cross section of the sequential two-photon double ionization in argon atom.
图 2 Ar原子3p3/2光电离磁截面
Fig. 2. The magnetic cross section of the 3p3/2 photoionization in argon atom.
图 3 Ar原子3p3/2光电离后剩余离子取向参数
Fig. 3. The alignment of the residual ions of 3p3/2 photoioni-zation in argon atom.
图 4 Ar原子3p壳层序列双光双电离中第一个(a)和第二个(b)光电子的角各向异性参数
Fig. 4. The asymmetry parameter of the first (a) and second (b) photoelectron angular distribution in sequential two-photon double ionization of the Ar 3p shell.
图 5 Ar原子3p壳层序列双光双电离第一个(a), (b)和第二个(c), (d)光电子角各向异性参数
Fig. 5. The asymmetry parameter of the angular distribution of the first (a), (b) and second (c), (d) photoelectrons emitted in sequential two-photon double ionization of the Ar 3p shell.
图 6 Ar原子3p壳层序列双光双电离光电离角分布
Fig. 6. Photoelectron angular distributions for the sequential two-photon double ionization of Ar 3p shell.
-
[1] Bachau H, Lambropoulos P 1991 Phys. Rev. A 44 R9(R)
[2] Laulan S, Bachau H 2003 Phys. Rev. A 68 013409
Google Scholar
[3] Böhme D K 2011 Phys. Chem. Chem. Phys. 13 18253
Google Scholar
[4] Thissen R, Witasse O, Dutuit O, Wedlund C S, Gronoff G, Lilensten J 2011 Phys. Chem. Chem. Phys. 13 18264
Google Scholar
[5] Gillaspy J D, Pomeroy J M, Perrella A C, Grube H 2007 J. Phys. Conf. Ser. 58 451
Google Scholar
[6] McNeil B W J, Thompson N R 2010 Nat. Photon. 4 814
Google Scholar
[7] Wabnitz H, Bittner L, de Castro A R B, et al. 2002 Nature 420 482
Google Scholar
[8] Young L, Kanter E P, Krässig B, et al. 2010 Nature 466 56
Google Scholar
[9] Braune M, Reinköster A, Viefhaus J, et al. 2007 XXV Int. Conf. on Photonic, Electronic and Atomic Collisions (ICPEAC), Freiburg, Germany, July 25–31, 2007 p34
[10] Kurka M, Rudenko A, Foucar, et al. 2009 J. Phys. B: At. Mol. Opt. Phys. 42 141002
Google Scholar
[11] Braune M, Hartmann G, Ilchen M, et al. 2016 J. Mod. Opt. 63 324
Google Scholar
[12] Augustin S, Schulz M, Schmid G, et al. 2018 Phys. Rev. A 98 033408
Google Scholar
[13] Ilchen M, Hartmann G, Gryzlova E V, et al. 2018 Nat. Commun. 9 4659
Google Scholar
[14] Carpeggiani P A, Gryzlova E V, Reduzzi M, et al. 2019 Nat. Phys. 15 170
Google Scholar
[15] Fritzsche S, Grum-Grzhimailo A N, Gryzlova E V, Kabachnik N M 2008 J. Phys. B: At. Mol. Opt. Phys. 41 165601
Google Scholar
[16] Gryzlova E V, Grum-GrzhimailoA N, Kuzmina E I, Strakhova S I 2014 J. Phys. B: At. Mol. Opt. Phys. 47 195601
Google Scholar
[17] Grum-Grzhimailo A N, Gryzlova E V 2014 Phys. Rev. A 89 043424
Google Scholar
[18] Grum-Grzhimailo A N, Gryzlova E V, Fritzsche S, Kabachnik N M 2016 J. Mod. Opt. 63 334
Google Scholar
[19] Gryzlova E V, Grum-Grzhimailo A N, Staroselskaya E I, Strakhova S I 2015 J. Electron Spectrosc. Relat. Phenom. 204 277
Google Scholar
[20] Ma K, Xie L Y, Zhang D H, Dong C Z 2015 Chin. Phys. B 24 073402
Google Scholar
[21] Ma K, Chen Z B, Xie L Y, Dong C Z 2018 J. Phys. B: At. Mol. Opt. Phys. 51 045203
Google Scholar
[22] Ma K, Chen Z B, Xie L Y, Dong C Z, Qu Y Z 2017 J. Phys. B: At. Mol. Opt. Phys. 50 225202
Google Scholar
[23] Ma K, Chen Z B, Xie L Y, Ding X B, Zhang D H, Dong C Z 2018 J. Electron Spectrosc. Relat. Phenom. 228 1
Google Scholar
[24] Blum K 1996 Density Matrix Theory and Applications (2nd Ed.) (New York: Plenum) pp35–60
[25] Balashov V V, Grum-Grzhimailo A N, Kabachnik N M 2000 Polarization and Correlation Phenomena in Atomic Collisions (New York: Kluwer Academic/Plenum) pp76–97
[26] Jönsson P, Gaigalas G, Bieroń J, Fischer C F, Grant I P 2013 Comput. Phys. Commun. 184 2197
Google Scholar
[27] Fritzsche S 2012 Comput. Phys. Commun. 183 1525
Google Scholar
[28] Langer B 1992 ZurEnergieabhängigkeit von Photoelektronen- satelliten (Studies of Vacuum Ultraviolet and X-ray Processes (Vol. 2) (New York: AMS Press) p145
下载:
计量
- 文章访问数: 8401
- PDF下载量: 134
- 被引次数: 0
