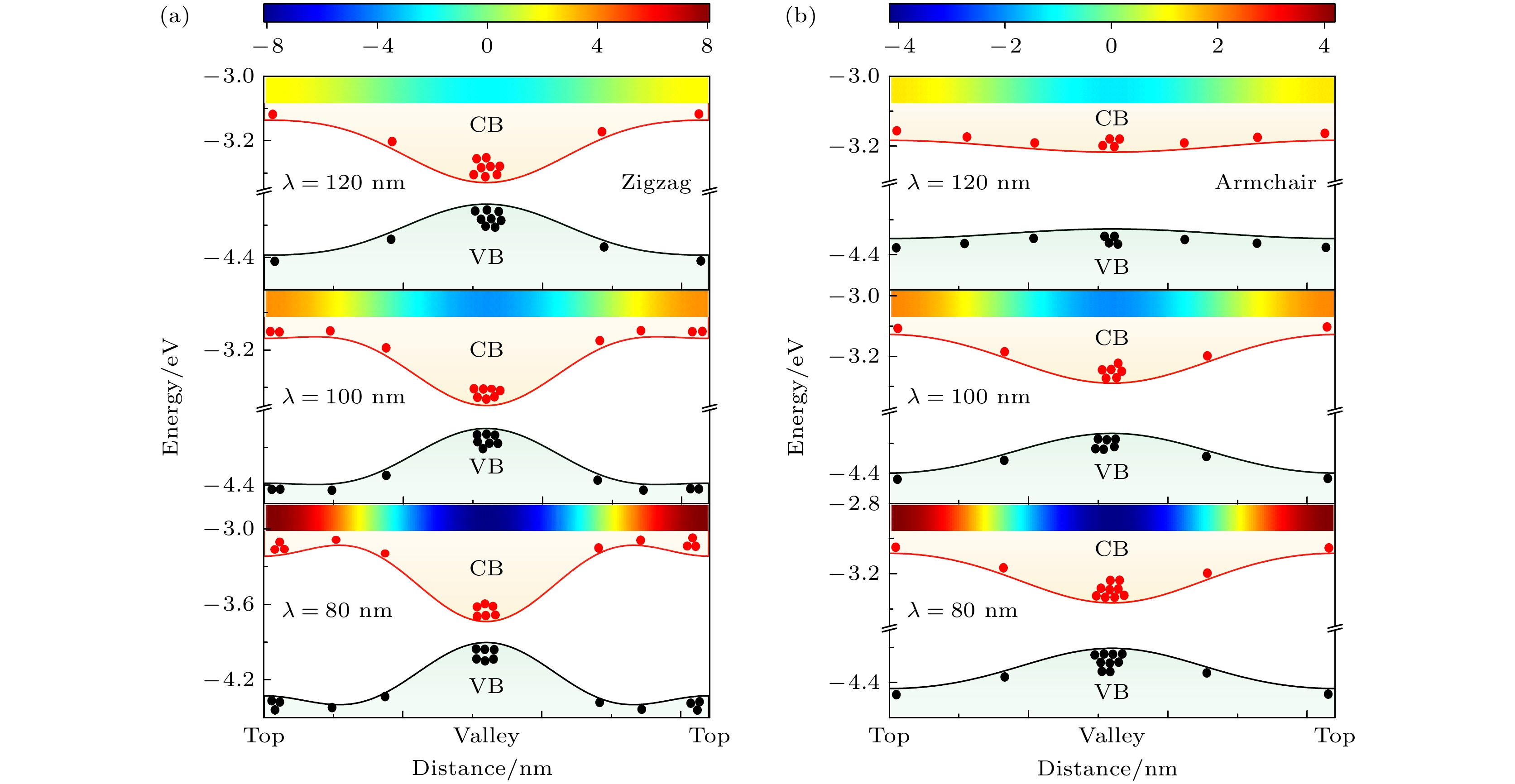
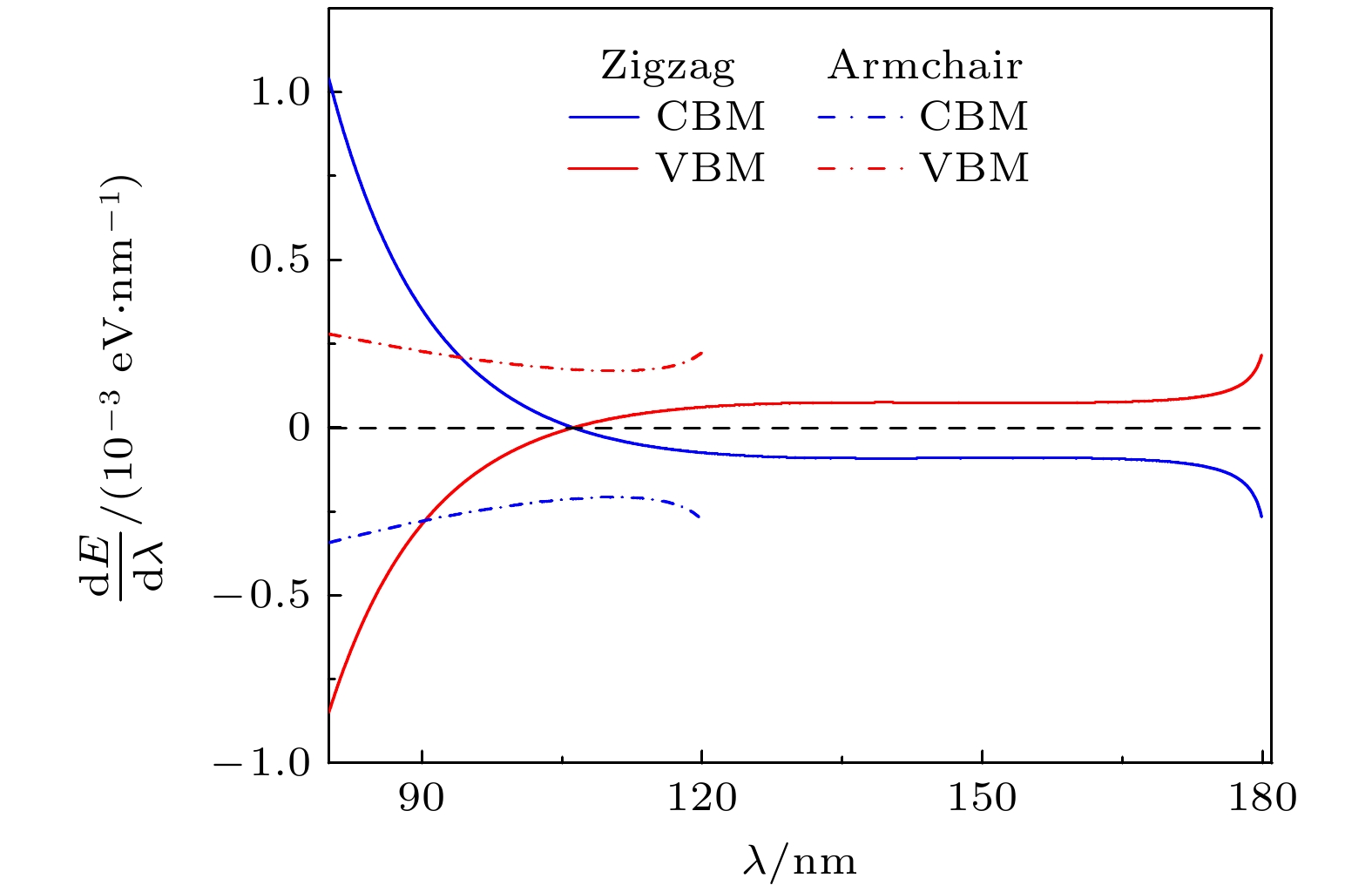
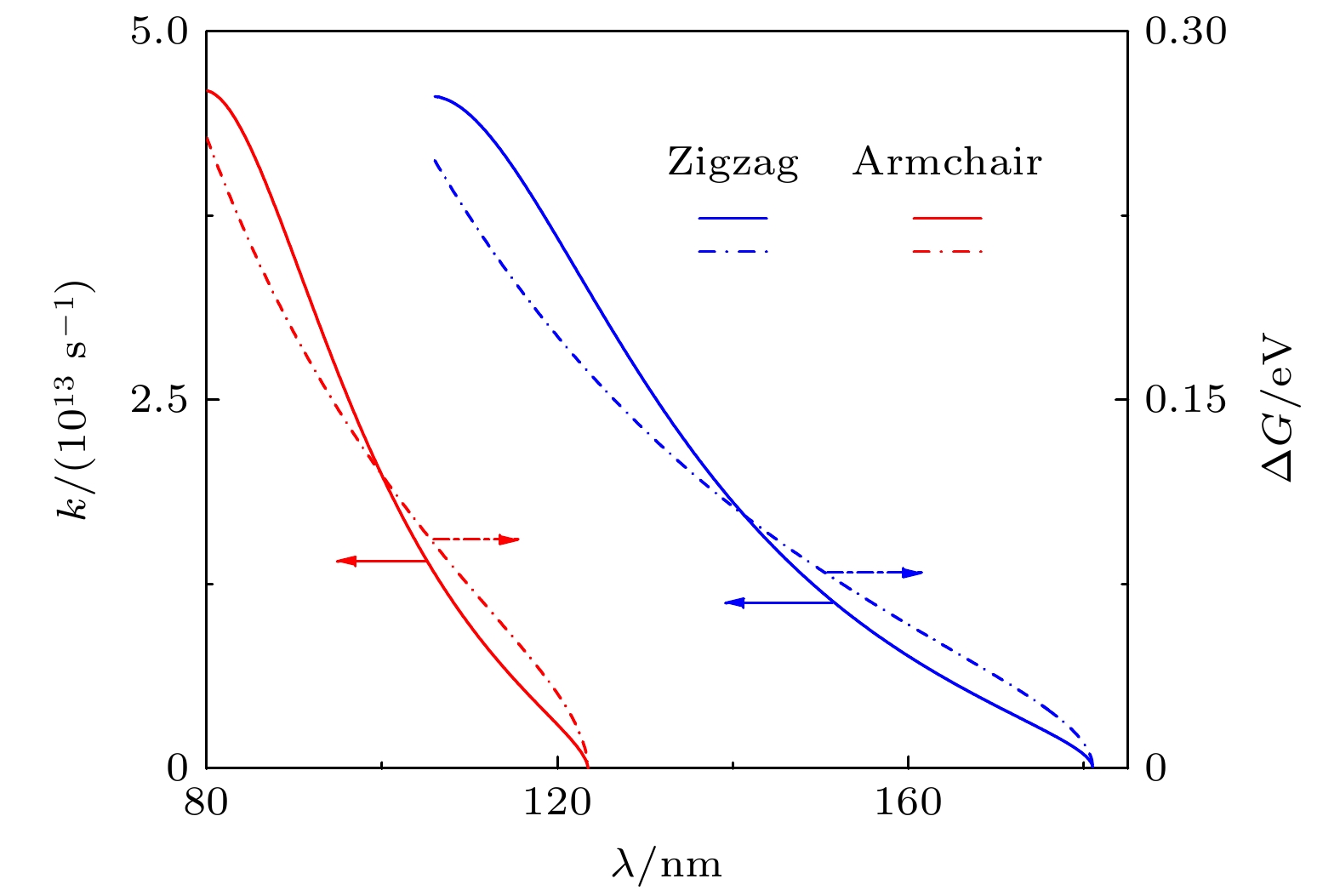
-
褶皱结构引起的周期性连续变化应变为调控二维材料电子和光电性质提供了重要手段. 然而, 目前关于褶皱状二维材料的形成机理及其对相关物性的调控研究仍缺乏理解. 本文基于原子键弛豫理论和连续介质力学方法, 系统研究了褶皱状单层GeSe能带结构随波长和位置的变化规律. 结果表明, 由于各向异性的力学性质和褶皱引起的周期性连续变化应变, 褶皱状单层GeSe表现出各向异性的能带漏斗效应, 激子会定向聚集在褶皱的谷区域, 且聚集能力随着波长的减小而增强. 此外, 当波长减小至106 nm, 锯齿型褶皱状单层GeSe的能带漏斗消失, 而扶手椅型褶皱状单层GeSe的能带漏斗依然得以保持. 这些结果为褶皱状单层GeSe在激子输运中的应用提供了理论基础, 并为设计高性能基于二维材料的光电器件提供了新策略.Two-dimensional materials with tunable wrinkled structures open up a new way to modulate their electronic and optoelectronic properties. However, the mechanisms of forming wrinkles and their influences on the band structures and associated properties are still unclear. Here, we investigate the strain distribution, bandgap, and anisotropic energy funneling effect of wrinkled monolayer GeSe and their evolution with the wrinkle wavelength based on the atomic-bond-relaxation approach and continuum medium mechanics. We find that the top region and valley region of wrinkled monolayer GeSe exhibit tensile and compressive strains, respectively, and the strain increases with wrinkle wavelength decreasing. Moreover, the periodic undulation strain in the wrinkles can lead to continuously adjustable bandgaps and band edges in wrinkled monolayer GeSe. For zigzag wrinkled monolayer GeSe, when the wrinkle wavelength is long, the conduction band minimum value (valence band maximum value) continuously decreases (increases) from the top to the valley, forming an energy funnel. As a result, the excitons accumulate in the valleys of wrinkles, and their accumulation capability increases with wrinkle wavelength decreasing. However, as the wavelength further decreases, the energy funnel will disappear, causing some excitons to t accumulate at the top of wrinkles, while the remaining excitons will accumulate in the valleys of wrinkles. The critical wavelength for the energy funnel of zigzag wrinkled GeSe to disappear is 106nm. The physical origin is that when the top strain exceeds 4%, the bandgap will decrease. Owing to the monotonic variation of bandgap with strain, the energy funneling effect of armchair wrinkled monolayer GeSe is still retained when the wavelength decreases to 80 nm, and the accumulation of excitons is further enhanced. Our results demonstrate that the energy funneling effect induced by nonuniform can realize excitons’ accumulation in one material without the need of p-n junctions, which is of great benefit to the collection of photogenerated excitons. Therefore, the proposed theory not only clarifies the physical mechanism regarding the anisotropic energy funneling effect of wrinkled monolayer GeSe, but also provides a new avenue for designing the next-generation optoelectronic devices.
-
Keywords:
- GeSe /
- wrinkle /
- energy funneling effect /
- atomic-bond-relaxation approach
-
图 1 褶皱状单层GeSe/衬底结构示意图以及单层GeSe的俯视图和侧视图
Fig. 1. Schematic illustration of wrinkled monolayer GeSe/substrate as well as the top and side views of monolayer GeSe.
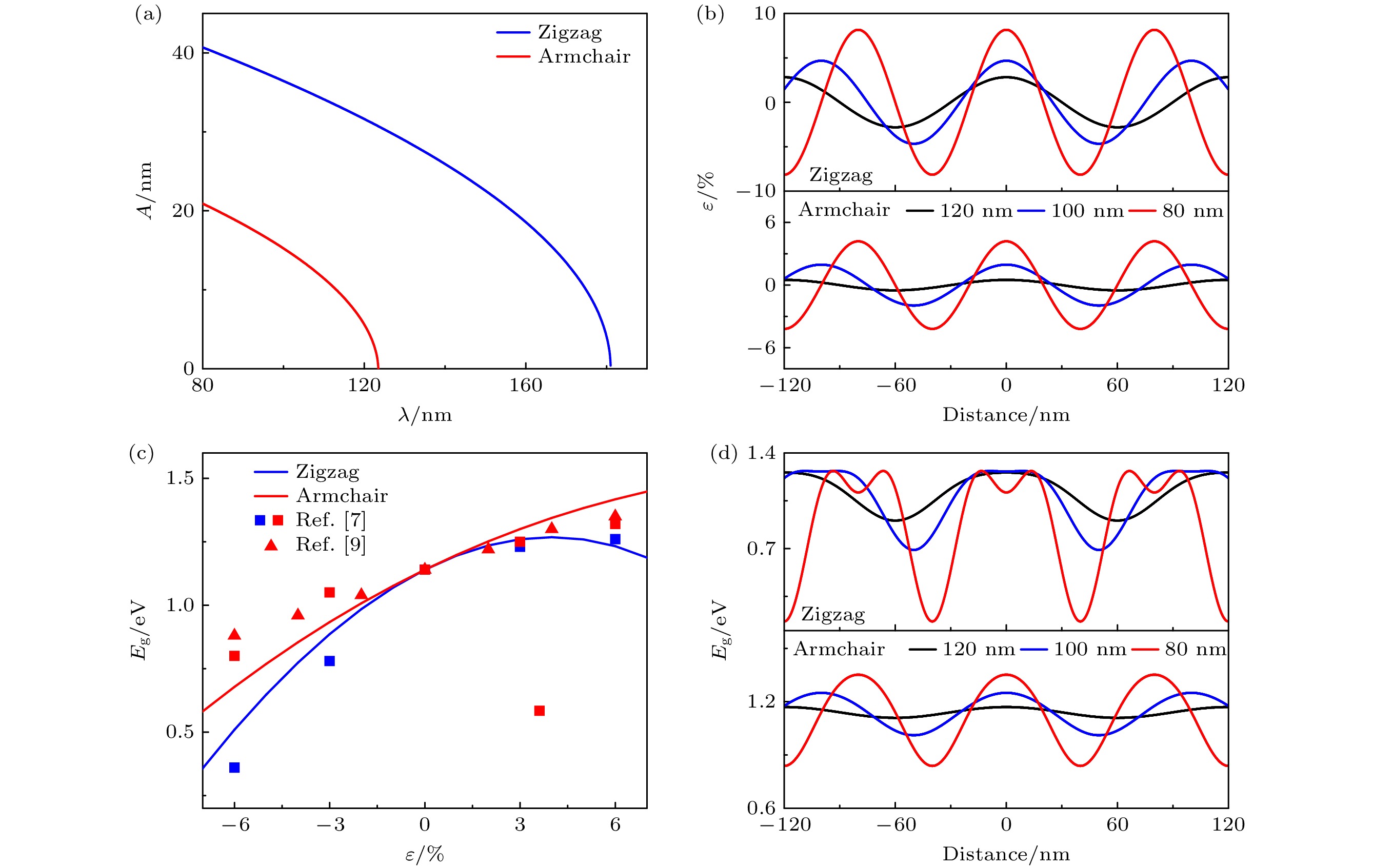
图 2 (a) 褶皱状单层GeSe振幅与波长之间的关系; (b) 不同波长下褶皱状单层GeSe应变的分布情况; (c) 单层GeSe带隙随应变的变化规律; (d) 不同波长下褶皱状单层GeSe带隙的分布情况
Fig. 2. (a) The relationship between the amplitude and wavelength of wrinkled monolayer GeSe; (b) distribution of strain of wrinkled monolayer GeSe with different wavelengths; (c) strain dependent bandgap of monolayer GeSe; (d) distribution of bandgaps of wrinkled monolayer GeSe with different wavelengths.
图 3 (a) 锯齿型褶皱状单层GeSe和 (b) 扶手椅型褶皱状单层GeSe在不同波长下单个周期内带边的分布情况
Fig. 3. Energy profiles of (a) zigzag wrinkled monolayer GeSe and (b) armchair wrinkled monolayer GeSe with different wavelengths.
图 4 褶皱状单层GeSe峰导带底和价带顶的一阶导数随波长的变化规律
Fig. 4. The first derivatives of CBM and VBM of top as a function of wavelength in zigzag wrinkled GeSe and armchair wrinkled GeSe.
图 5 褶皱状单层GeSe驱动力和激子转移速率随波长的变换规律
Fig. 5. The driving force and exciton transfer rate of wrinkled GeSe as a function of wavelength.
-
[1] Novoselov K S, Geim A K, Morozov S V, Jiang D, Zhang Y, Dubonos S V, Grigorieva I V, Firsov A A 2004 Science 306 666
Google Scholar
[2] Mak K F, Lee C, Hone J, Shan J, Heinz T F 2010 Phys. Rev. Lett. 105 136805
Google Scholar
[3] Li L K, Yu Y J, Ye G J, Ge Q Q, Ou X D, Wu H, Feng D L, Chen X H, Zhang Y B 2014 Nat. Nanotechnol. 9 372
Google Scholar
[4] Zhao H Q, Mao Y L, Mao X, Shi X, Xu C S, Wang C X, Zhang S M, Zhou D H 2018 Adv. Funct. Mater. 28 1704855
Google Scholar
[5] Zhou X, Hu X Z, Jin B, Yu J, Liu K L, Li H Q, Zhai T Y 2018 Adv. Sci. 5 1800478
Google Scholar
[6] Hu Y H, Zhang S L, Sun S F, Xie M Q, Cai B, Zeng H B 2015 Appl. Phys. Lett. 107 122107
Google Scholar
[7] Xia C X, Du J, Huang X W, Xiao W B, Xiong W Q, Wang T X, Wei Z M, Jia Y, Shi J J, Li J B 2018 Phys. Rev. B 97 115416
Google Scholar
[8] Xu Y F, Zhang H, Shao H Z, Ni G, Li J, Lu H L, Zhang R J, Peng Bo, Zhu Y Y, Zhu H Y, Soukoulis C M 2017 Phys. Rev. B 96 245421
Google Scholar
[9] Kong X, Deng J K, Li L, Liu Y L, Ding X D, Sun J, Liu J Z 2018 Phys. Rev. B 98 184104
Google Scholar
[10] Mao Y L, Xu C S, Yuan J M, Zhao H Q 2019 J. Mater. Chem. A 7 11265
Google Scholar
[11] Lu Q L, Yang W H, Xiong F B, Lin H F, Zhuang Q Q 2020 Acta Phys. Sin. 69 196801 [卢群林, 杨伟煌, 熊飞兵, 林海峰, 庄芹芹 2020 69 196801]
Google Scholar
Lu Q L, Yang W H, Xiong F B, Lin H F, Zhuang Q Q 2020 Acta Phys. Sin. 69 196801
Google Scholar
[12] Muhammad Z, Li Y L, Abbas G, Usman M, Sun Z, Zhang Y, Lv Z Y, Wang Y, Zhao W S 2022 Adv. Electron. Mater. 8 2101112
Google Scholar
[13] Huang L, Wu F G, Li J B 2016 J. Chem. Phys. 144 114708
Google Scholar
[14] Li Z B, Liu X S, Wang X, Yang Y, Liu S C, Shi W, Li Y, Xing X B, Xue D J, Hu J S 2020 Phys. Chem. Chem. Phys. 22 914
Google Scholar
[15] Zuo B Min, Yuan J M, Feng Z, Mao Y L 2019 Acta Phys. Sin. 68 113103 [左博敏, 袁健美, 冯志, 毛宇亮 2019 68 113103]
Google Scholar
Zuo B Min, Yuan J M, Feng Z, Mao Y L 2019 Acta Phys. Sin. 68 113103
Google Scholar
[16] Guo G X, Bi G 2018 Micro Nano Lett. 13 600
Google Scholar
[17] Wang J J, Zhao Y F, Zheng J D, Wang X T, Deng X, Guan Z, Ma R R Zhong Ni, Yue F Y, Wei Z M, Xiang P H, Duan C G 2021 Phys. Chem. Chem. Phys. 23 26997
Google Scholar
[18] Li Y, Ma K, Fan X, Liu F S, Li J Q, Xie H P 2020 Appl. Sur. Sci. 521 146256
Google Scholar
[19] Feng J, Qian X F, Huang C W, Li J 2012 Nat. Photonics 6 866
Google Scholar
[20] Li H, Contryman A W, Qian X F, Ardakani S Mo, Gong Y J, Wang X L, Weisse J M, Lee C H, Zhao J H, Ajayan P M, Li Ju, Manoharan H C, Zheng X L 2015 Nat. Commun. 6 7381
Google Scholar
[21] Jose P S, Parente V, Guinea F, Roldán R, Prada E 2016 Phys. Rev. X 6 031046
[22] Lam N H, Nguyen P, Cho S, Kim J 2023 Surf. Sci. 730 122251
Google Scholar
[23] Zheng J D, Zhao Y F, Bao Z Q, Shen Y H, Guan Z, Zhong N, Yue F Yu, Xiang P H, Duan C G 2022 2D Mater. 9 035005
Google Scholar
[24] Harats M G, Kirchhof J N, Qiao M X, Greben K, Bolotin K I 2020 Nat. Photonics 14 324
Google Scholar
[25] Lee J, Yun S J, Seo C, Cho K, Kim T S, An G H, Kang K, Lee H S, Kim J Y 2020 Nano Lett. 21 43
[26] Wang J W, Han M J, Wang Q, Ji Y Q, Zhang X, Shi R, Wu Z F, Zhang L, Amini A, Guo L, Wang N, Lin J H, Cheng C 2021 ACS Nano 15 6633
Google Scholar
[27] Hao S J, Hao Y L, Li J, Wang K Y, Fan C, Zhang S W, Wei Y H, Hao G L 2024 Appl. Phys. Lett. 125 072102
Google Scholar
[28] Dastgeer G, Afzal A M, Nazir G, Sarwar N 2021 Adv. Mater. Interfaces 8 2100705
Google Scholar
[29] Song Q C, An M, Chen X D, Peng Z, Zang J F, Yang N 2016 Nanoscale 8 14943
Google Scholar
[30] Ouyang G, Wang C X, Yang G W 2009 Chem. Rev. 109 4221
Google Scholar
[31] Zhu Z M, Zhang A, Ouyang G, Yang G W 2011 Appl. Phys. Lett. 98 263112
Google Scholar
[32] Dong J S, Zhao Y P, Ouyang G, Yang G W 2022 Appl. Phys. Lett. 120 080501
Google Scholar
[33] Huang R 2005 J. Mech. Phys. Solids 53 63
Google Scholar
[34] Jiang H Q, Khang D Y, Song J Z, Sun Y G, Huang Y G, Rogers J A 2007 Proc. Natl. Acad. Sci. 104 15607
Google Scholar
[35] Khang D Y, Rogers J A, Lee H H 2008 Adv. Funct. Mater. 18 1
[36] Iguiñiz N, Frisenda R, Bratschitsch R, Gomez A C 2019 Adv. Mater. 31 1807150
Google Scholar
[37] Guo Q L, Zhang M, Xue Z Y, Ye L, Wang G, Huang G S, Mei Y F, Wang X, Di Z F 2013 Appl. Phys. Lett. 103 264102
Google Scholar
[38] Vellaa D, Bicoa J, Boudaoudb A, Romana B, Reis P M 2009 Proc. Natl. Acad. Sci. 106 10901
Google Scholar
[39] Gomez A C, Roldan R, Cappelluti E, Buscema M, Guinea F, Zant H S J, Steele G A 2013 Nano Lett. 13 5361
Google Scholar
[40] Jiang J W, Zhou Y P 2017 DOI: 10.5772/intechopen.71929
[41] Sun C Q 2007 Prog. Solid State Chem. 35 1
Google Scholar
[42] Zhu Y F, Jiang Q 2016 Coordin. Chem. Rev. 326 1
Google Scholar
[43] Marcus R A 1956 J. Chem. Phys. 24 966
Google Scholar
[44] Wang J H, Ding T, Gao K M, Wang L F, Zhou P W, Wu K F 2021 Nat. Commun. 12 6333
Google Scholar
[45] Ghosh R, Papnai B, Chen Y S, Yadav K, Sankar R, Hsieh Y P, Hofmann M, Chen Y F 2023 Adv. Mater. 35 2210746
Google Scholar
[46] Garzona L V, Frisenda R, Gomez A C 2019 Nanoscale 11 12080
Google Scholar
[47] Shang H X, Liang X, Deng F, Hu S L, Shen S P 2022 Int. J. Mech. Sci. 234 107685
Google Scholar
[48] Shang H X, Dong H T, Wu Y H, Deng F, Liang X, Hu S L, Shen S P 2024 Phys. Rev. Lett. 132 116201
Google Scholar
[49] Zhang Z, Zhao Y P, Ouyang G 2017 J. Phys. Chem. C 121 19296
Google Scholar
[50] Furchi M M, Pospischil A, Libisch F, Burgdörfer J, Mueller T 2014 Nano Lett. 14 4785
Google Scholar
[51] Lee C H, Lee G H, Zande A M, Chen W C, Li Y L, Han M Y, Cui X, Arefe G, Nuckolls C, Heinz T F, Guo J, Hone J, Kim P 2014 Nat. Nanotechnol. 9 676
Google Scholar
[52] Cao G Y, Shang A X, Zhang C, Gong Y P, Li S J, Bao Q L, Li X F 2016 Nano Energy 30 260
Google Scholar
下载:
计量
- 文章访问数: 94
- PDF下载量: 5
- 被引次数: 0
