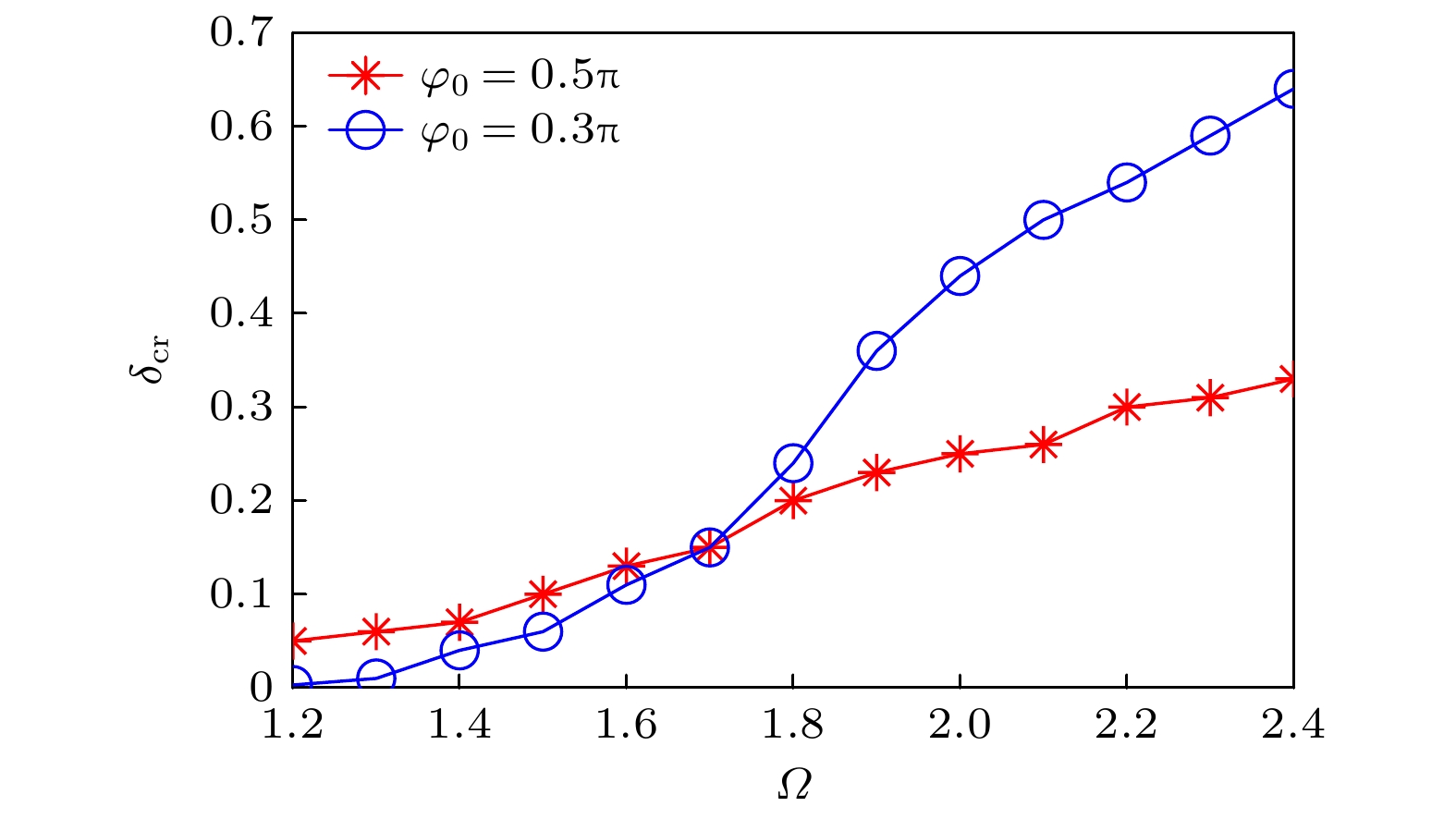
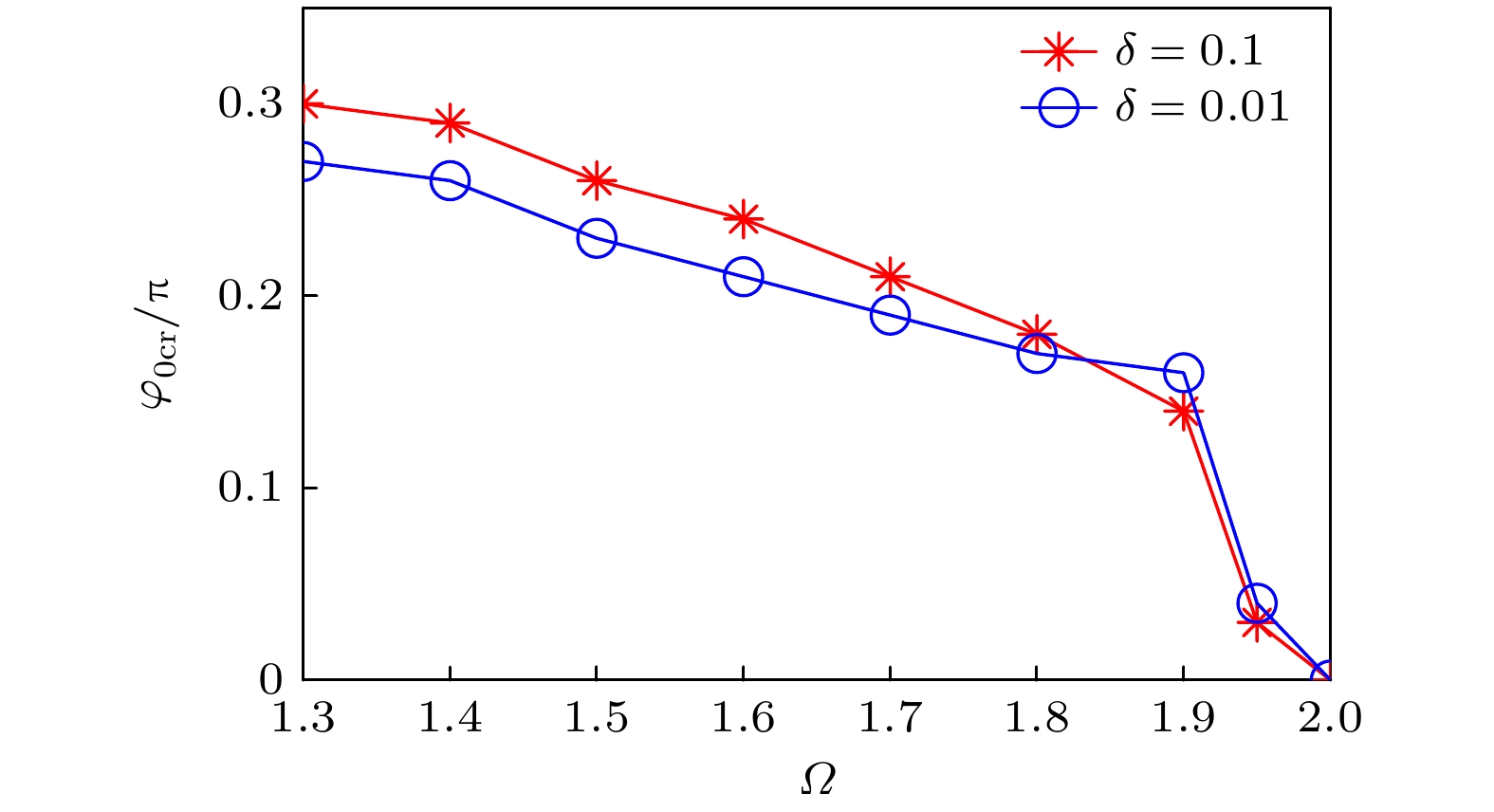
-
稳定性是影响Fermi-Pasta-Ulam-Tsingou(FPUT)现象实验观测的一个十分重要的因素, 针对文献中FPUT现象稳定性分析贫乏的问题, 本文主要通过数值模拟方法研究非线性光纤中影响FPUT现象稳定性的主要因素. 本文采用的初始条件为实验上比较容易实现的正弦扰动平面波加上白色噪声, 发现增加扰动振幅及扰动信号与泵浦光之间的相位差均可极大程度的影响FPUT现象的稳定性. 结果表明: 1) 当扰动振幅从零逐渐增加时, 所观察到的FPUT现象的稳定性逐渐增强; 当扰动振幅增加至临界值, FPUT现象的稳定性增至最强; 当扰动振幅大于该临界值, FPUT现象的稳定性开始逐渐减弱. 2) 当扰动频率位于传统调制不稳定性区域时, 随着扰动信号与泵浦光之间相位差从0增加至π/2, FPUT现象的稳定性先减弱, 当相位差增加至某临界值, FPUT现象的稳定性减至最弱, 当相位差大于临界值, FPUT现象的稳定性又出现逐步增强的规律. 当扰动频率位于非传统调制不稳定性区域时, 与传统情况不同, FPUT现象的稳定性随扰动信号与泵浦光之间的相位差的增加而增强. 以上结果表明, 在FPUT现象的实验中, 为观察到更稳定的FPUT现象, 扰动振幅的值不能太小, 且扰动信号与泵浦光之间相位差也要适宜. Stability plays a significant role in successfully observing Fermi-Pasta-Ulam-Tsingou (FPUT) phenomenon in experiment. However, there are few relevant studies in the literature. The primary object of this work is to study the stability of FPUT phenomenon in the nonlinear fibers numerically. In this study, we take the sinusoidally perturbed continuous waves (CWs) with white noise as the imposed initial condition, which can be readily realized in real experiments. We find that both the perturbation amplitude and phase difference between the perturbation and pump can drastically affect the stability of the resulting FPUT phenomenon. Firstly, as the perturbation amplitude increases, the FPUT phenomenon becomes much more stable. When the perturbation amplitude reaches a critical value, the most stable FPUT phenomenon can be observed. With the further increase of the perturbation amplitude, the stability of the resulting FPUT phenomenon weakens. Secondly, the phase difference between the perturbation and pump takes distinct effects on the stability of FPUT phenomenon for perturbation frequency located inside and outside the conventional modulation instability (MI) band. For the perturbation frequency located inside the conventional MI band, as the phase difference between the perturbation and pump increases from zero, the corresponding FPUT phenomenon first is less stable, and then becomes most instable at a critical phase difference; after that, the stability of the FPUT phenomenon is enhanced again. For the perturbation frequency located outside the conventional MI band, the stability of FPUT phenomenon is enhanced monotonically as the phase difference increases from 0 to π/2. In order to observe a much more stable FPUT phenomenon, as shown in the above results, the perturbation amplitude should be moderately large, and the phase difference between the perturbation and the pump should be appropriate to avoid the most instable FPUT phenomenon. [1] Fermi E, Pasta P, Ulam S, Tsingou M 1955 Studies of the Nonlinear Problems Los Alamos, May 1, 1955 pLA-1940
[2] Van Simaeys G, Emplit G, Haelterman M 2001 Phys. Rev. Lett. 87 033902
Google Scholar
[3] Akhmediev N N 2001 Nature 413 267
Google Scholar
[4] Devine N, Ankiewicz A, Genty G, Dudley J M, Akhmediev N 2011 Phys. Lett. A 375 4158
Google Scholar
[5] Wabnitz S, Wetzel B 2014 Phys. Lett. A 378 2750
Google Scholar
[6] Chin S A, Ashour O A, Blic M R 2015 Phys. Rev. E 92 063202
Google Scholar
[7] Bao C, Jaramillo-Villegas J A, Xuan Y, Leaird D E, Qi M, Weiner A M 2016 Phys. Rev. Lett. 117 163901
Google Scholar
[8] Kimmoun O, Hsu H C, Branger H, Li M S, Chen Y Y, Kharif C, Onorato M, Kelleher E J R, Kibler B, Akhmediev N, Chabchoub A 2016 Sci. Rep. 6 28516
Google Scholar
[9] Deng G, Li S, Biondini G, Trillo S 2017 Phys. Rev. E 96 052213
Google Scholar
[10] Pierangeli D, Flammini M, Zhang L, Marcucci G, Agranat A J, Grinevich P G, Santini P M, Conti C, DelRe E 2018 Phys. Rev. X 8 041017
Google Scholar
[11] Naveau C, Szriftgiser P, Kudlinski A, Conforti M, Trillo S, Mussot A 2019 Opt. Lett. 44 763
Google Scholar
[12] Vanderhaegen G, Szriftgiser P, Kudlinski A, Conforti M, Trillo S, Droques M, Mussot A 2020 Opt. Express 28 17773
Google Scholar
[13] Sheveleva A, Andral U, Kibler B, Colman P, Dudley J M, Finot C 2022 Optica 9 656
Google Scholar
[14] Chen S C, Liu C 2022 Physica D 438 133364
Google Scholar
[15] Sinthuja N, Rajasekar S, Senthilvelan M 2023 Nonlinear Dyn. 111 16497
Google Scholar
[16] Kraych A E, Agafontsev D, Randoux S, Suret P 2019 Phys. Rev. Lett. 123 093902
Google Scholar
[17] Chowdury A, Ankiewicz A, Akhmediev N, Chang W 2018 Chaos 28 123116
Google Scholar
[18] Copie F, Suret P, Randoux S 2022 Opt. Lett. 47 3560
Google Scholar
[19] Kimmoun O, Hsu H C, Kibler B, Chabchoub A 2017 Phys. Rev. E 96 022219
Google Scholar
[20] Yin H M, Chow K W 2021 Physica D 428 133033
Google Scholar
[21] Mussot A, Naveau C, Conforti M, Kudlinski A, Copie F, Szriftgiser P, Trillo S 2018 Nat. Photonics 12 303
Google Scholar
[22] Liu C, Wu Y H, Chen S C, Yao X, Akhmediev N 2021 Phys. Rev. Lett. 127 094102
Google Scholar
[23] Yao X K, Liu C, Yang Z Y, Yang W L 2022 Phys. Rev. Res. 4 013246
Google Scholar
[24] Conforti M, Mussot A, Kudlinski A, Trillo S, Akhmediev N 2020 Phys. Rev. A 101 023843
Google Scholar
[25] Vanderhaegen G, Naveau C, Szriftgiser P, Kudlinski A, Conforti M, Mussot A, Onorato M, Trillo S, Chabchoub A, Akhmediev N 2021 Proc. Natl. Acad. Sci. U.S.A. 118 e2019348118
Google Scholar
[26] Cheung V Y Y, Yin H M, Li J H, Chow K W 2023 Phys. Lett. A 476 128877
Google Scholar
[27] Chen S C, Liu C, Yao X, Zhao L C, Akhmediev N 2021 Phys. Rev. E 104 024215
Google Scholar
[28] Liu C, Chen S C, Yao X K, Akhmediev N 2022 Chin. Phys. Lett. 39 094201
Google Scholar
[29] Chen S C, Liu C, Akhmediev N 2023 Phys. Rev. A 107 063507
Google Scholar
[30] Hammani K, Wetzel B, Kibler B, Fatome J, Finot C, Millot G, Akhmediev N, Dudley J M 2011 Opt. Lett. 36 2140
Google Scholar
[31] Naveau C, Vanderhaegen G, Szriftgiser P, Martinelli G, Droques M, Kudlinski A, Conforti M, Trillo S, Akhmediev N, Mussot A 2021 Front. Phys. 9 637812
Google Scholar
[32] Hu X Y, Chen W, Lu Y, Yu Z J, Chen M, Meng Z 2018 IEEE Photon. Technol. Lett. 30 47
Google Scholar
[33] Vanderhaegen G, Szriftgiser P, Kudlinski A, Armaroli A, Conforti M, Mussot A, Trillo S 2023 Phys. Rev. A 108 033507
Google Scholar
[34] Goossens J W, Hafermann H, Jaouën Y 2019 Sci. Rep. 9 18467
Google Scholar
[35] Yin H M, Li J H, Zheng Z, Chiang K S, Chow K W 2024 Chaos 34 013120
Google Scholar
-
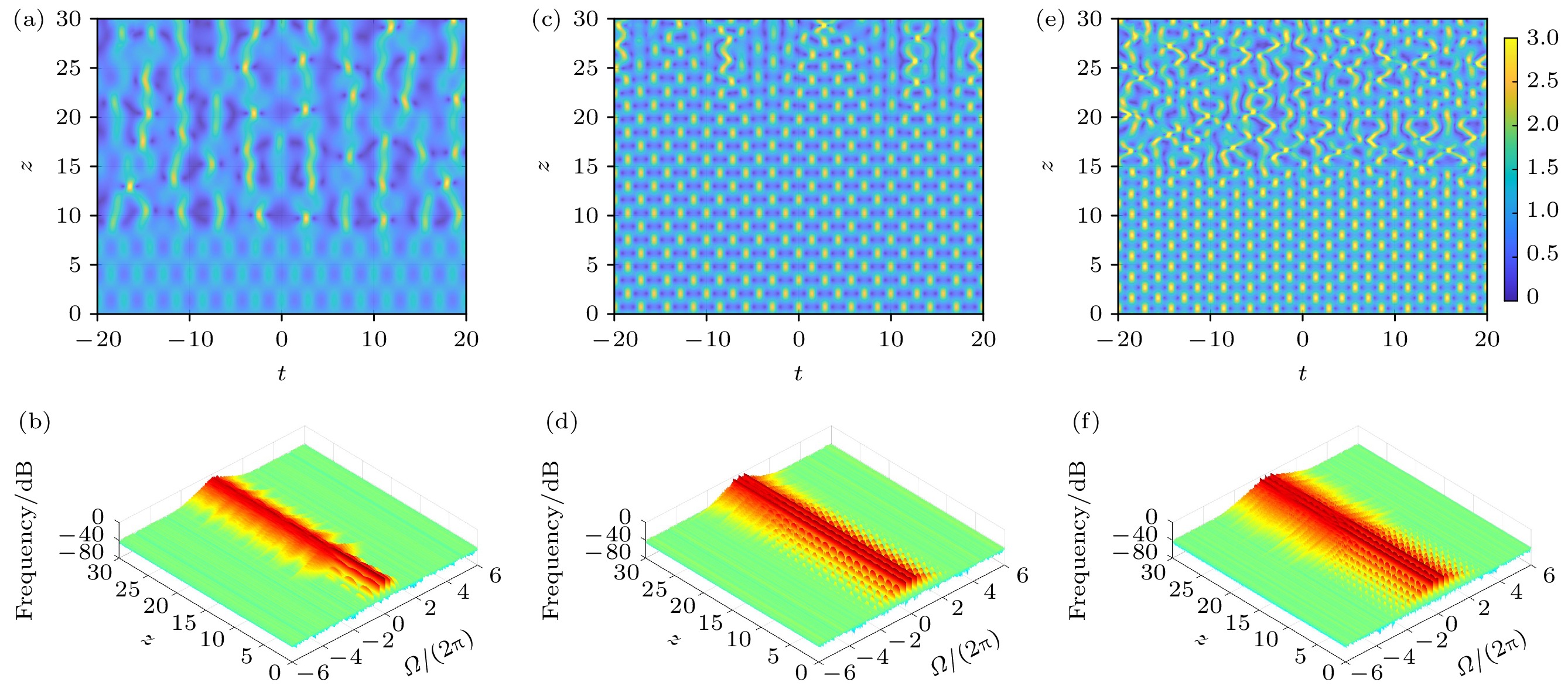
图 1 非线性光纤中扰动平面波随传输距离的时空和相应的频谱演化(1%噪声), 参数为Ω = 1.5, φ0 = 0.5π (a), (b) δ = 0.01; (c), (d) δ = 0.1; (e), (f) δ = 0.25
Fig. 1. The evolution of perturbed plane waves in nonlinear fibers with the propagation distance in the temporal and spectral domain (1% noise) with Ω = 1.5, φ0 = 0.5π: (a), (b) δ = 0.01; (c), (d) δ = 0.1; (e), (f) δ = 0.25.
图 2 非线性光纤中扰动平面波随传输距离的时空和相应的频谱演化(1%噪声), 参数为Ω = 2.2, φ0 = 0.5π (a), (b) δ = 0.01; (c), (d) δ = 0.25; (e), (f) δ = 0.5
Fig. 2. The evolution of perturbed plane waves in nonlinear fibers with the propagation distance in the temporal and spectral domain (1% noise) with Ω = 2.2, φ0 = 0.5π: (a), (b) δ = 0.01; (c), (d) δ = 0.25; (e), (f) δ = 0.5.
图 3 临界扰动振幅δcr与扰动频率的依赖关系(1%噪声)
Fig. 3. The dependence of the critical perturbation amplitude (δcr) on the perturbation frequency (1% noise).
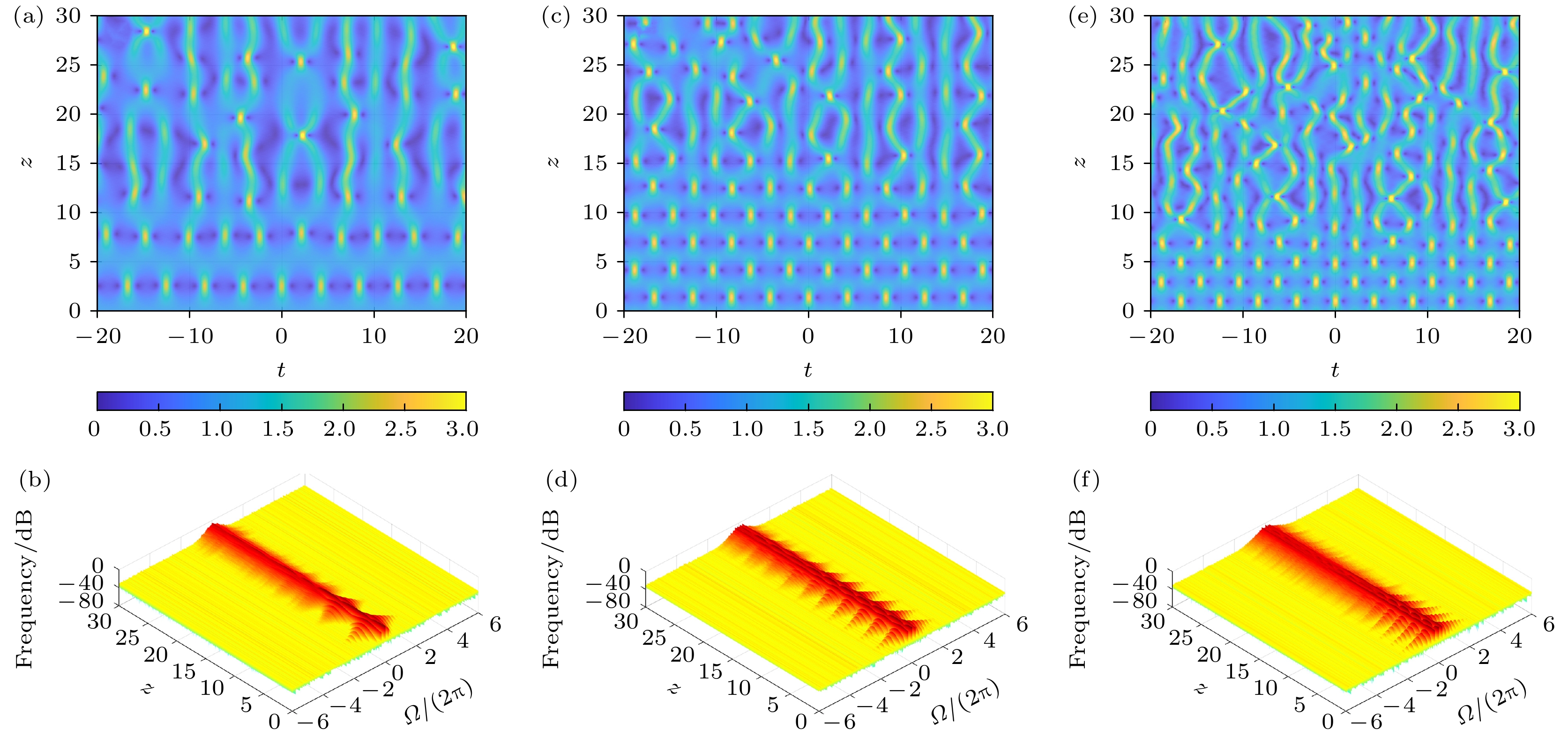
图 4 非线性光纤中扰动平面波随传输距离的时空和相应的频谱演化(1%噪声), 参数为Ω = 1.5, δ = 0.1 (a), (b) φ0 = 0.1π; (c), (d) φ0 = 0.3π; (e), (f) φ0 = 0.5π
Fig. 4. The evolution of perturbed plane waves with the propagation distance in the temporal and spectral domain (1% noise) with parameters Ω = 1.5, δ = 0.1: (a), (b) φ0 = 0.1π; (c), (d) φ0= 0.3π; (e), (f) φ0 = 0.5π.
图 5 扰动信号与泵浦光之间的临界相位差与扰动频率的依赖关系(1%噪声)
Fig. 5. The dependence of the critical phase difference between the perturbation and the pump on the perturbation frequency (1% noise).
图 6 非线性光纤中扰动平面波随传输距离的时空和相应的频谱演化(1%噪声), 参数为Ω = 2.2, δ = 0.25 (a), (b) φ0 = 0.1π; (c), (d) φ0 = 0.3π; (e), (f) φ0 = 0.5π
Fig. 6. The evolution of perturbed plane waves with the propagation distance in the temporal and spectral domain (1% noise) with parameters Ω = 2.2, δ = 0.25: (a), (b) φ0 = 0.1π; (c), (d) φ0= 0.3π; (e), (f) φ0 = 0.5π.
图 7 非线性光纤中扰动平面波随传输距离的时空和相应的频谱演化(10%噪声), 参数为Ω = 1.5, φ0 = 0.5π (a), (b) δ = 0.01; (c), (d) δ = 0.1; (e), (f) δ = 0.25
Fig. 7. The evolution of perturbed plane waves in nonlinear fibers with the propagation distance in the temporal and spectral domain (10% noise) with Ω = 1.5, φ0 = 0.5π: (a), (b) δ = 0.01; (c), (d) δ = 0.1; (e), (f) δ = 0.25.
-
[1] Fermi E, Pasta P, Ulam S, Tsingou M 1955 Studies of the Nonlinear Problems Los Alamos, May 1, 1955 pLA-1940
[2] Van Simaeys G, Emplit G, Haelterman M 2001 Phys. Rev. Lett. 87 033902
Google Scholar
[3] Akhmediev N N 2001 Nature 413 267
Google Scholar
[4] Devine N, Ankiewicz A, Genty G, Dudley J M, Akhmediev N 2011 Phys. Lett. A 375 4158
Google Scholar
[5] Wabnitz S, Wetzel B 2014 Phys. Lett. A 378 2750
Google Scholar
[6] Chin S A, Ashour O A, Blic M R 2015 Phys. Rev. E 92 063202
Google Scholar
[7] Bao C, Jaramillo-Villegas J A, Xuan Y, Leaird D E, Qi M, Weiner A M 2016 Phys. Rev. Lett. 117 163901
Google Scholar
[8] Kimmoun O, Hsu H C, Branger H, Li M S, Chen Y Y, Kharif C, Onorato M, Kelleher E J R, Kibler B, Akhmediev N, Chabchoub A 2016 Sci. Rep. 6 28516
Google Scholar
[9] Deng G, Li S, Biondini G, Trillo S 2017 Phys. Rev. E 96 052213
Google Scholar
[10] Pierangeli D, Flammini M, Zhang L, Marcucci G, Agranat A J, Grinevich P G, Santini P M, Conti C, DelRe E 2018 Phys. Rev. X 8 041017
Google Scholar
[11] Naveau C, Szriftgiser P, Kudlinski A, Conforti M, Trillo S, Mussot A 2019 Opt. Lett. 44 763
Google Scholar
[12] Vanderhaegen G, Szriftgiser P, Kudlinski A, Conforti M, Trillo S, Droques M, Mussot A 2020 Opt. Express 28 17773
Google Scholar
[13] Sheveleva A, Andral U, Kibler B, Colman P, Dudley J M, Finot C 2022 Optica 9 656
Google Scholar
[14] Chen S C, Liu C 2022 Physica D 438 133364
Google Scholar
[15] Sinthuja N, Rajasekar S, Senthilvelan M 2023 Nonlinear Dyn. 111 16497
Google Scholar
[16] Kraych A E, Agafontsev D, Randoux S, Suret P 2019 Phys. Rev. Lett. 123 093902
Google Scholar
[17] Chowdury A, Ankiewicz A, Akhmediev N, Chang W 2018 Chaos 28 123116
Google Scholar
[18] Copie F, Suret P, Randoux S 2022 Opt. Lett. 47 3560
Google Scholar
[19] Kimmoun O, Hsu H C, Kibler B, Chabchoub A 2017 Phys. Rev. E 96 022219
Google Scholar
[20] Yin H M, Chow K W 2021 Physica D 428 133033
Google Scholar
[21] Mussot A, Naveau C, Conforti M, Kudlinski A, Copie F, Szriftgiser P, Trillo S 2018 Nat. Photonics 12 303
Google Scholar
[22] Liu C, Wu Y H, Chen S C, Yao X, Akhmediev N 2021 Phys. Rev. Lett. 127 094102
Google Scholar
[23] Yao X K, Liu C, Yang Z Y, Yang W L 2022 Phys. Rev. Res. 4 013246
Google Scholar
[24] Conforti M, Mussot A, Kudlinski A, Trillo S, Akhmediev N 2020 Phys. Rev. A 101 023843
Google Scholar
[25] Vanderhaegen G, Naveau C, Szriftgiser P, Kudlinski A, Conforti M, Mussot A, Onorato M, Trillo S, Chabchoub A, Akhmediev N 2021 Proc. Natl. Acad. Sci. U.S.A. 118 e2019348118
Google Scholar
[26] Cheung V Y Y, Yin H M, Li J H, Chow K W 2023 Phys. Lett. A 476 128877
Google Scholar
[27] Chen S C, Liu C, Yao X, Zhao L C, Akhmediev N 2021 Phys. Rev. E 104 024215
Google Scholar
[28] Liu C, Chen S C, Yao X K, Akhmediev N 2022 Chin. Phys. Lett. 39 094201
Google Scholar
[29] Chen S C, Liu C, Akhmediev N 2023 Phys. Rev. A 107 063507
Google Scholar
[30] Hammani K, Wetzel B, Kibler B, Fatome J, Finot C, Millot G, Akhmediev N, Dudley J M 2011 Opt. Lett. 36 2140
Google Scholar
[31] Naveau C, Vanderhaegen G, Szriftgiser P, Martinelli G, Droques M, Kudlinski A, Conforti M, Trillo S, Akhmediev N, Mussot A 2021 Front. Phys. 9 637812
Google Scholar
[32] Hu X Y, Chen W, Lu Y, Yu Z J, Chen M, Meng Z 2018 IEEE Photon. Technol. Lett. 30 47
Google Scholar
[33] Vanderhaegen G, Szriftgiser P, Kudlinski A, Armaroli A, Conforti M, Mussot A, Trillo S 2023 Phys. Rev. A 108 033507
Google Scholar
[34] Goossens J W, Hafermann H, Jaouën Y 2019 Sci. Rep. 9 18467
Google Scholar
[35] Yin H M, Li J H, Zheng Z, Chiang K S, Chow K W 2024 Chaos 34 013120
Google Scholar
下载:
计量
- 文章访问数: 1173
- PDF下载量: 78
- 被引次数: 0
