陶瓷型复合燃料烧结过程的相场模拟研究

廖宇轩; 申文龙; 吴学志; 喇永孝; 柳文波

doi:10.7498/aps.73.20241112

摘要

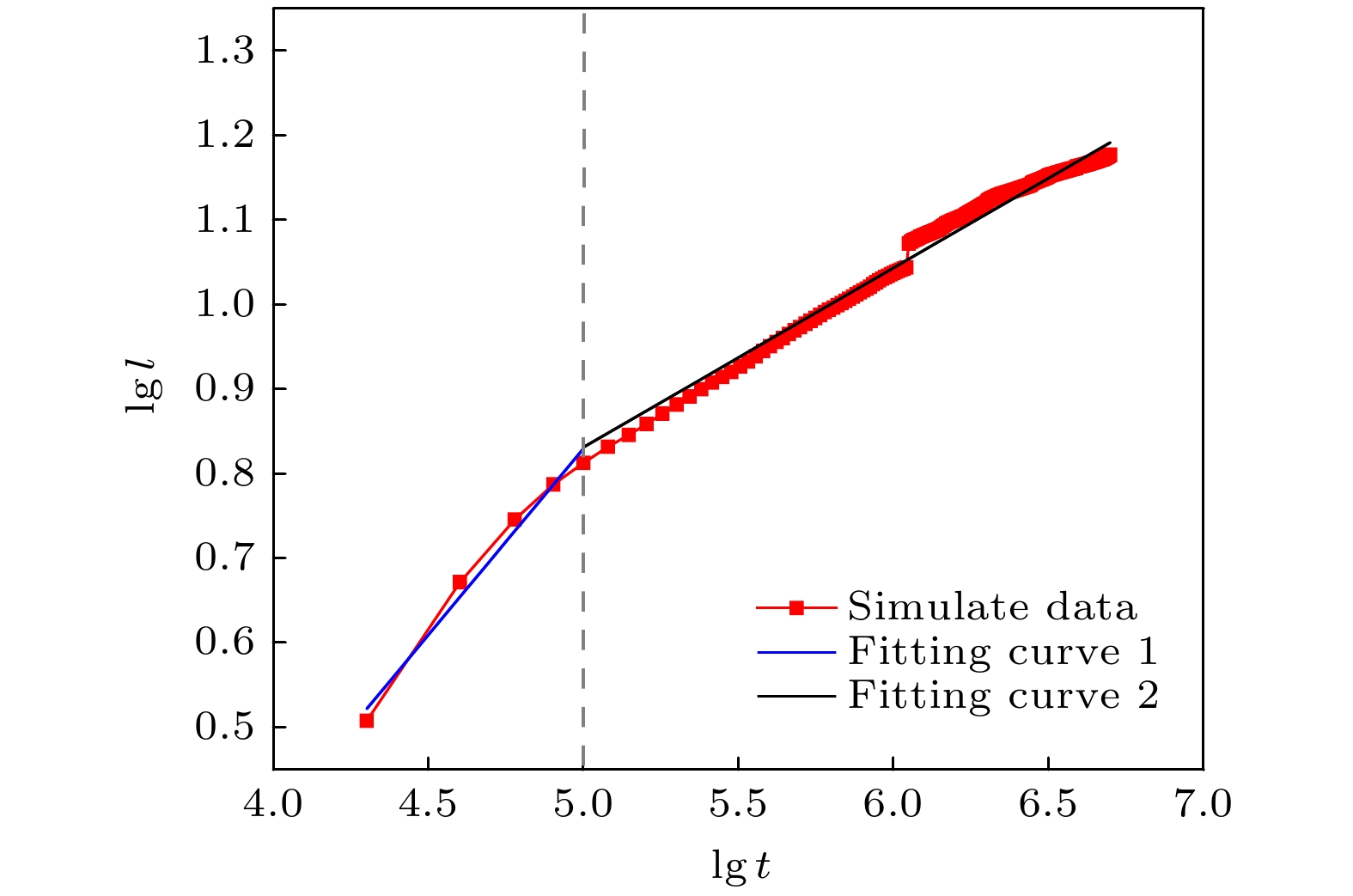
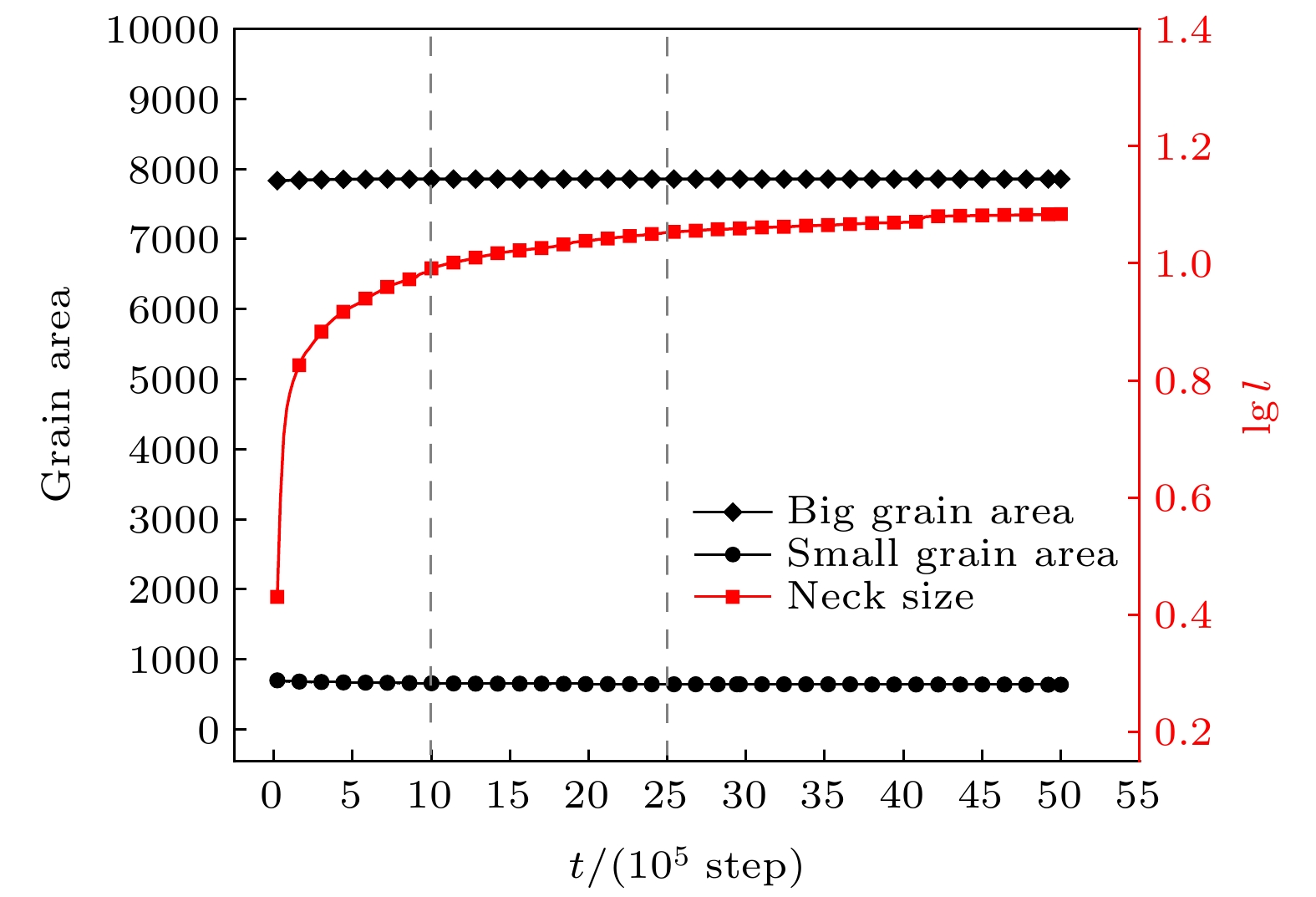
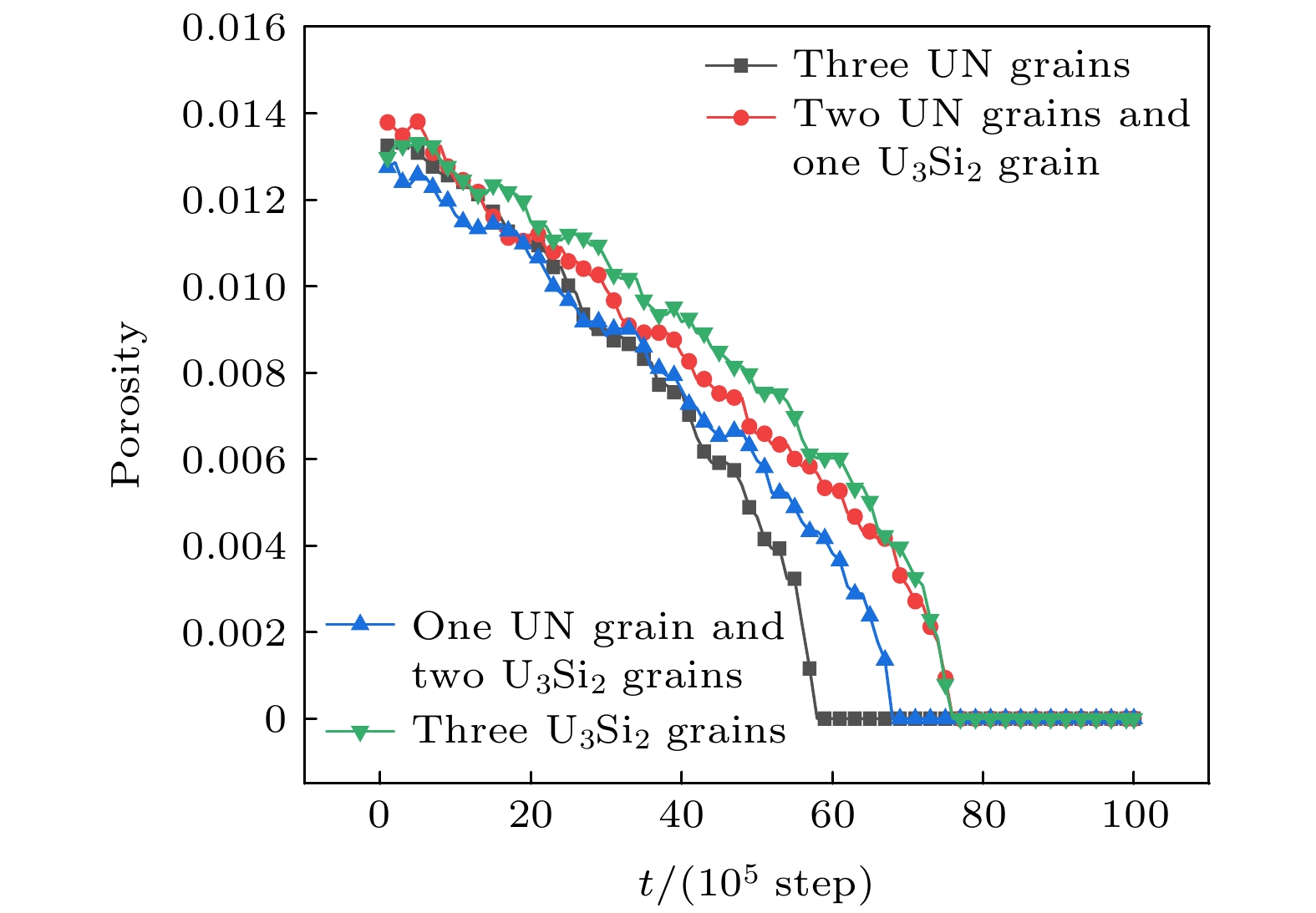
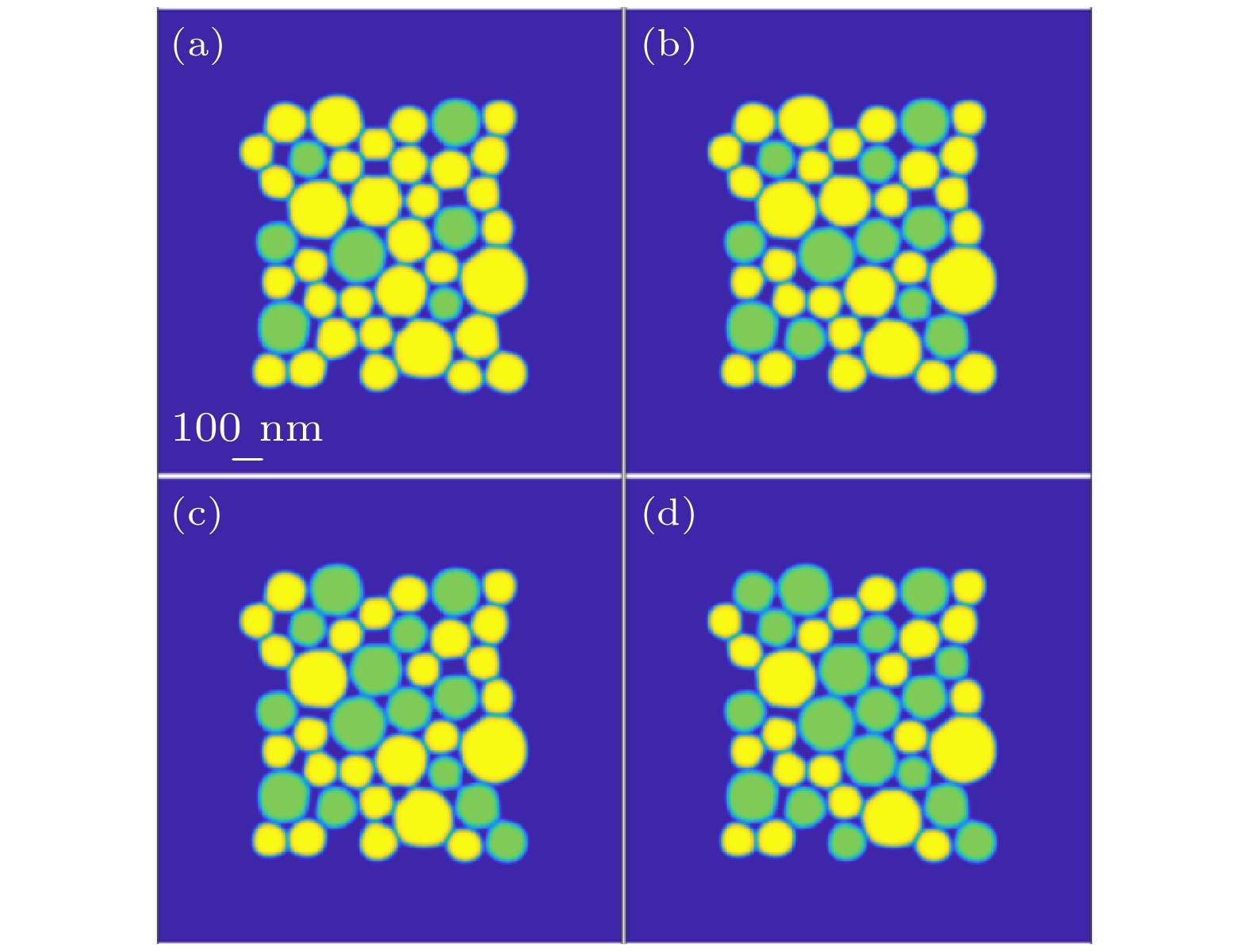
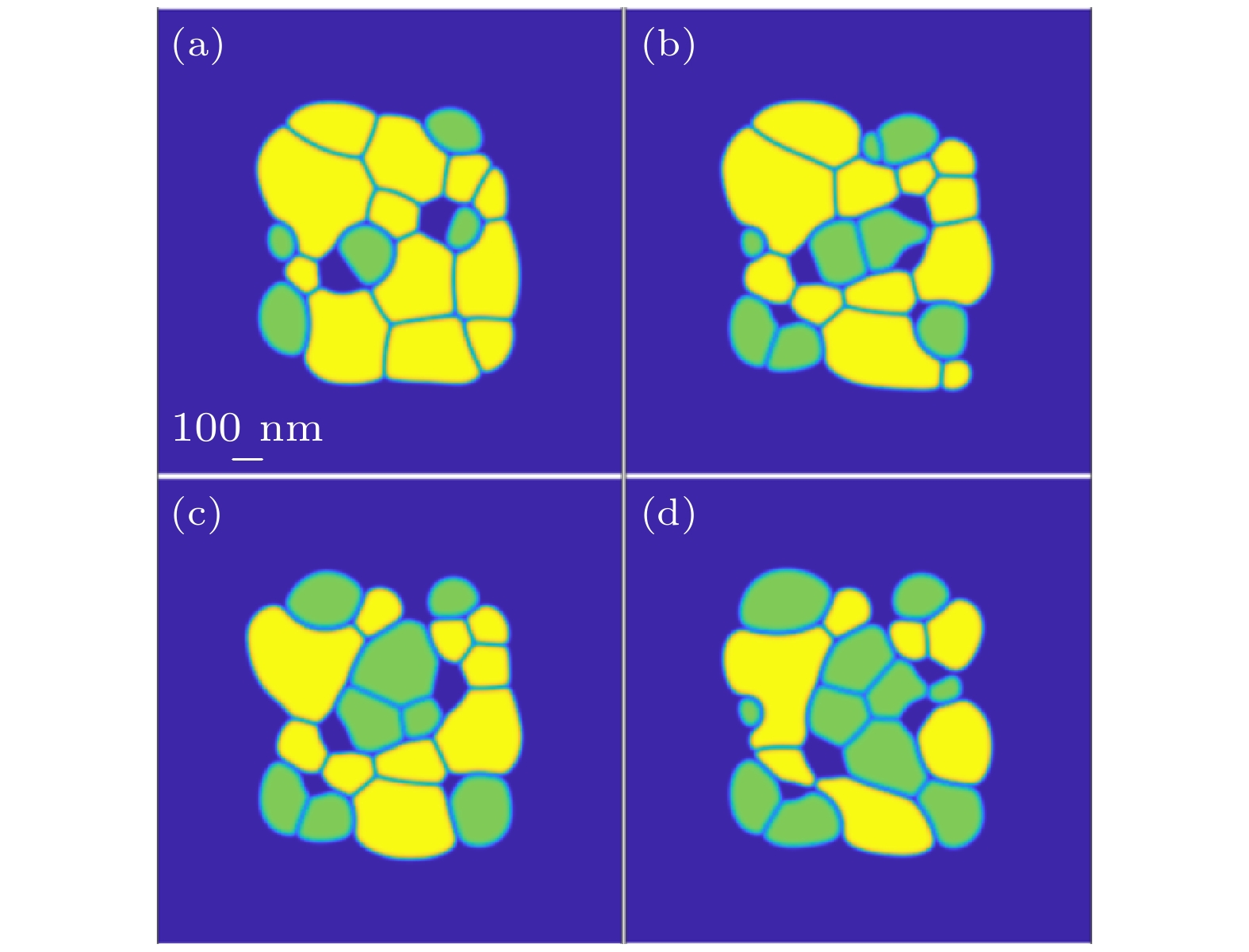
建立了陶瓷型复合燃料两相烧结过程的相场模型, 利用该模型模拟了UN-U₃Si₂复合燃料的烧结过程. 首先, 研究了两相双晶粒在烧结过程中的烧结颈的演化过程. 结果表明: 具有较高表面能的晶粒在烧结颈形成过程中的表面形变更明显; 两相双晶粒形成的平衡二面角的大小取决于两相的晶界能与表面能的比值; 两相不等大的双晶粒之间未发生大晶粒吞噬小晶粒现象. 然后, 研究了烧结过程中的两相三晶粒之间的气孔收缩和三叉晶界的演化, 以揭示符合燃料烧结过程中气孔的演变规律. 结果发现, 两相三晶粒形成的三叉晶界夹角偏离了120°, 晶界处的高能势垒阻碍了气孔的空位沿晶界的扩散, 导致三叉晶界处的气孔收缩速率减慢. 最后, 研究了两相陶瓷型复合燃料的多晶烧结过程. 不同体积分数比的两相多晶烧结组织形貌演化的模拟结果表明, 晶界扩散在两相烧结过程中起主要作用, 体积分数较大的相的晶粒生长占据主导地位, 两相晶粒之间存在阻碍晶界迁移的作用, 同相晶粒之间存在晶粒迁移现象.

关键词:

Abstract

Due to the limitation of existing experimental techniques, it is difficult to observe the evolution of microstructure in the sintering process in real time, resulting in a lack of in-depth understanding of the sintering mechanism of two-phase composite fuels. Therefore, it is greatly important to carry out theoretical simulation studies in the sintering process of composite fuels. In this work, a phase-field model of the two-phase sintering process of ceramic composite fuel is established, and the sintering process of UN-U₃Si₂ composite fuel is simulated by using this method. The simulation results show that during the formation of sintering neck, the surface deformation of the grains with higher surface energy is significant. The size of the final equilibrium dihedral angle formed by the two-phase double grains depends on the ratio of the grain boundary energy to the surface energy of the two phases. The phenomenon of large grains swallowing small grains does not occur between the two unequal double grains. Subsequently, the pore shrinkage and the properties of the trident grain boundary among the two-phase three grains are investigated in the sintering process. It is found that the angle of the trident grain boundary formed by the two-phase three grains deviates from 120°. The high-energy barrier at the grain boundary hinders the diffusion of the pore vacancies along the grain boundary, resulting in a slow shrinkage rate of the pore vacancies at the trident grain boundary. In addition, the simulation results of the microstructure evolution of two-phase polycrystalline sintered tissue with different volume fraction ratios show that the grain boundary diffusion plays a major role in the two-phase sintering process. The grain growth of the phase with a higher volume fraction is dominant, and there exists a hindrance to the migration of grain boundaries between two-phase grains. The phenomenon of grain migration exists between grains of the same phase.

Keywords:

作者及机构信息

1.
西安交通大学核科学与技术学院, 西安　710049

2.
中国原子能科学研究院, 北京　102413

3.
西安交通大学, 陕西省先进核能技术重点实验室, 西安　710049

通信作者: 柳文波, liuwenbo@xjtu.edu.cn

基金项目: 国家自然科学基金委员会与中国工程物理研究院联合基金(NSAF基金)(批准号: U2130105)和中国核工业集团有限公司领创科研项目资助的课题.

Authors and contacts

1.
Institute of Nuclear Science and Technology, Xian Jiaotong University, Xi’an 710049, China

2.
China institute of atomic energy, Beijing 102413, China

3.
Shaanxi Key Laboratory of Advanced Nuclear Energy and Technology, Xi’an Jiaotong University, Xi’an 710049, China

Corresponding author: Liu Wen-Bo, liuwenbo@xjtu.edu.cn

Funds: Project supported by the Joint Fund of the National Natural Science Foundation of China and the China Academy of Engineering Physics (NSAF Joint Fund) (Grant No. U2130105) and the Innovative Scientific Program of China National Nuclear Corporation (CNNC).

文章全文 : translate this paragraph

参考文献

[1]	Kim H G, Yang J H, Kim W J, Koo Y H 2016 Nucl. Eng. Technol. 48 1 Google Scholar
[2]	Kurata M 2016 Nucl. Eng. Technol. 48 26 Google Scholar
[3]	Leenaers A, Van den Berghe S, Koonen E, Jacquet P, Jarousse C, Guigon B, Ballagny A, Sannen L 2004 J. Nucl. Mater. 327 121 Google Scholar
[4]	Zinkle S J, Terrani K A, Gehin J C, Ott L J, Snead L L 2014 J. Nucl. Mater. 448 374 Google Scholar
[5]	Terrani K A, Wang D, Ott L J, Montgomery R O 2014 J. Nucl. Mater. 448 512 Google Scholar
[6]	Johnson K D, Raftery A M, Lopes D A, Wallenius J 2016 J. Nucl. Mater. 477 18 Google Scholar
[7]	Watkins J K, Gonzales A, Wagner A R, Sooby E S, Jaques B J 2021 J. Nucl. Mater. 553 153048 Google Scholar
[8]	Wood E S, White J T, Nelson A T 2017 J. Nucl. Mater 484 245 Google Scholar
[9]	Ortega L H, Blamer B J, Evans J A, McDeavitt S M 2016 J. Nucl. Mater. 471 116 Google Scholar
[10]	White J T, Travis A W, Dunwoody J T, Nelson A T 2017 J. Nucl. Mater. 495 463 Google Scholar
[11]	Lopes D A, Uygur S, Johnson K D 2017 J. Nucl. Sci. Technol. 54 405 Google Scholar
[12]	刘续希, 高士森, 喇永孝, 玉栋梁, 柳文波 2024 73 148201 Google Scholar Liu X X, Gao S S, La Y X, Yu D L, Liu W B 2024 Acta Phys. Sin. 73 148201 Google Scholar
[13]	刘东昆, 王庆宇, 张田, 周羽, 王翔 2024 73 066102 Google Scholar Liu D K, Wang Q Y, Zhang T, Zhou Y, Wang X 2024 Acta Phys. Sin. 73 066102 Google Scholar
[14]	王凯乐, 杨文奎, 史新成, 侯华, 赵宇宏 2023 72 076102 Google Scholar Wang K L, Yang W K, Shi X C, Hou H, Zhao Y H 2023 Acta Phys. Sin. 72 076102 Google Scholar
[15]	刘明治, 张瑞杰, 方伟, 章书周, 曲选辉 2012 金属学报 48 1207 Google Scholar Liu M Z, Zhang R J, Fang W, Zhang S Z, Qu X H 2012 Acta. Metall. Sin. 48 1207 Google Scholar
[16]	Kumar V, Fang Z Z, Fife P C 2010 Mater. Sci. Eng. A 528 254 Google Scholar
[17]	Biswas S, Schwen D, Wang H, Okuniewski M, Tomar V 2018 Comput. Mater. Sci. 148 307 Google Scholar
[18]	Du L F, Yang S M, Zhu X W, Jiang J, Hui Q, Du H L 2018 J. Mater. Sci. 53 9567 Google Scholar
[19]	Hötzer J, Seiz M, Kellner M, Rheinheimer W, Nestler B 2019 Acta Mater. 164 184 Google Scholar
[20]	Wang Y U 2006 Acta Mater. 54 953 Google Scholar
[21]	Fan D, Chen L Q 1997 Acta Mater. 45 611 Google Scholar
[22]	Moelans N, Blanpain B, Wollants P 2008 Phys. Rev. B 78 024113 Google Scholar
[23]	Ahmed K, Yablinsky C A, Schulte A, Allen T, El-Azab A 2013 Modell. Simul. Mater. Sci. Eng. 21 065005 Google Scholar
[24]	Cahn J W 1961 Acta Metall. 9 795 Google Scholar
[25]	Allen S M, Cahn J W 1979 Acta Metall. 27 1085 Google Scholar
[26]	Biner S B 2017 Programming Phase-Field Modeling (Switzerland: Springer International Publishing) p18
[27]	Holt J B, Almassy M Y 1969 J. Am. Ceram. Soc. 52 631 Google Scholar
[28]	戚晓勇, 柳文波, 何宗倍, 王一帆, 恽迪 2023 金属学报 59 1513 Google Scholar Qi X Y, Liu W B, He Z B, Wang Y F, Yun D 2023 Acta Metall. Sin. 59 1513 Google Scholar
[29]	Bocharov D, Gryaznov D, Zhukovskii Y F, Kotomin E A 2013 J. Nucl. Mater. 435 102 Google Scholar
[30]	Cooper M W D, Gamble K A, Capolungo L, Matthews C, Andersson D A, Beeler B, Stanek C R, Metzger K 2021 J. Nucl. Mater. 555 153129 Google Scholar
[31]	Beeler B, Baskes M, Andersson D, Cooper M W D, Zhang Y F 2019 J. Nucl. Mater. 514 290 Google Scholar
[32]	Cheniour A, Tonks M R, Gong B, Yao T K, He L F, Harp J M, Beeler B, Zhang Y F, Lian J 2020 J. Nucl. Mater 532 152069 Google Scholar
[33]	Chockalingam K, Kouznetsova V G, van der Sluis O, Geers M G D 2016 Comput. Methods Appl. Mech. Eng. 312 492 Google Scholar
[34]	Rahaman M N 1995 Ceramic Processing and Sintering (New York: Marcel Dekker) p446
[35]	Riedel H, Svoboda J 1993 Acta Metall. Mater. 41 1929 Google Scholar
[36]	孙正阳, 杨超, 柳文波 2020 金属学报 56 1295 Google Scholar Sun Z Y, Yang C, Liu W B 2020 Acta Metall. Sin. 56 1295 Google Scholar
[37]	Ahmed K, Allen T, El-Azab A 2016 J. Mater. Sci. 51 1261 Google Scholar
[38]	Yadav V, Vanherpe L, Moelans N 2016 Comput. Mater. Sci. 125 297 Google Scholar

施引文献

图 1 相场模型示意图 (ρ, ϕ_α, ϕ_β —浓度场变量; η₁—晶粒1的取向场变量; η₂—晶粒2的取向场变量)

Fig. 1. Schematic of phase field model (ρ, ϕ_α, ϕ_β —concentration field variable; η₁—orientation field variable of grain 1; η₂—orientation field variable of grain 2.)

下载: 全尺寸图片幻灯片

图 2 两个不同相等大圆形晶粒演化的相场模拟　(a) 6 × 10⁴ $ \Delta t $; (b) 50 × 10⁴ $ \Delta t $; (c) 200 × 10⁴ $ \Delta t $; (d) 500 × 10⁴$ \Delta t $

Fig. 2. Phase-field simulation of the evolution of two equal-sized circular grains with different phases: (a) 6 × 10⁴ $ \Delta t $; (b) 50 × 10⁴ $ \Delta t $; (c) 200 × 10⁴ $ \Delta t $; (d) 500 × 10⁴ $ \Delta t $.

下载: 全尺寸图片幻灯片

图 3 不同相晶粒的烧结颈对数增长曲线 (l — 颈部长度, t — 时间步)

Fig. 3. Logarithmic growth curves of sintering neck of different phase grains (l —neck length, t — time step).

下载: 全尺寸图片幻灯片

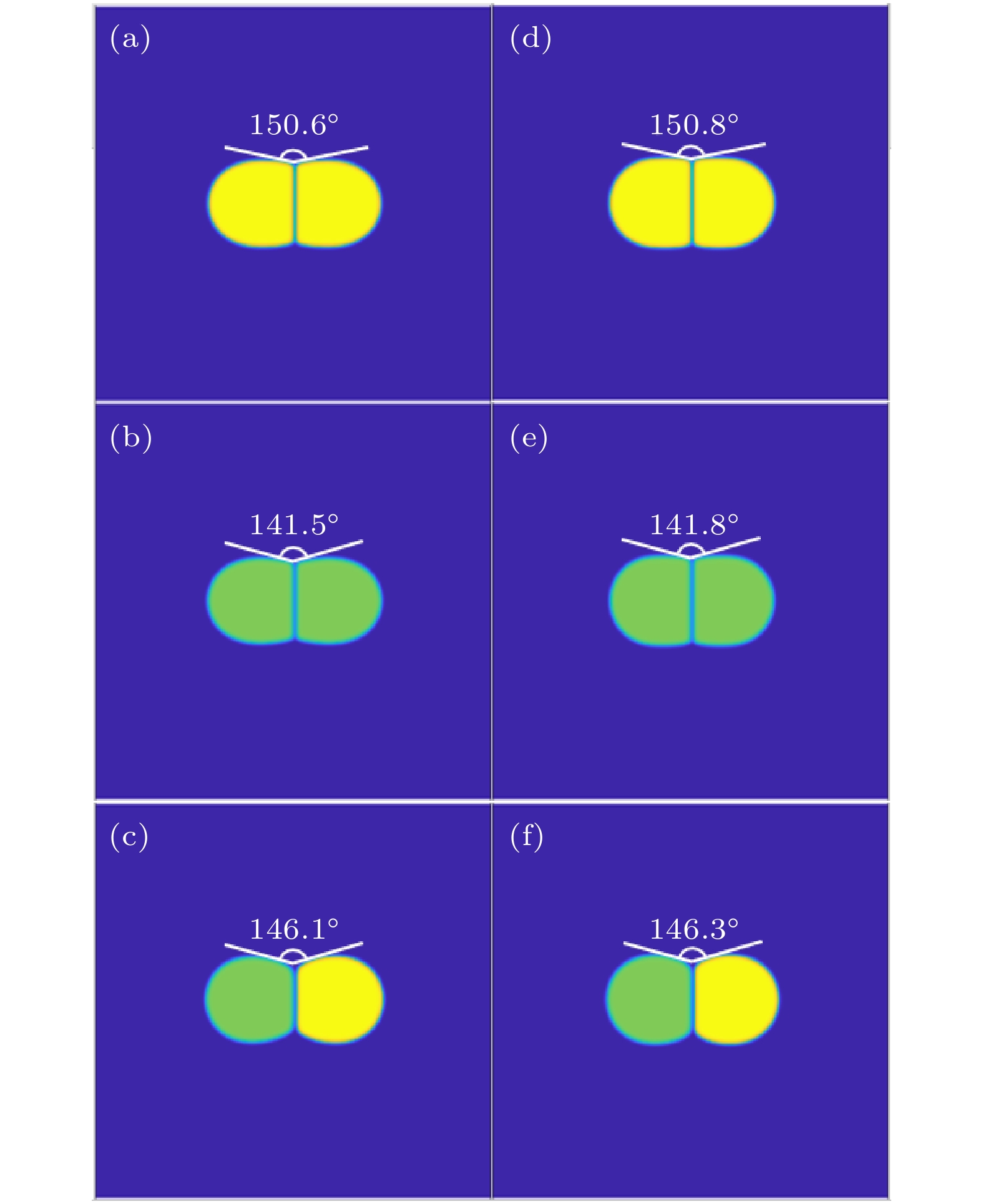
图 4 演化时间500 × 10⁴ $ \Delta t $((a)—(c))与1500 × 10⁴ $ \Delta t $((d)—(f))下的平衡二面角　(a), (d) 2个UN晶粒; (b), (e) 2个U₃Si₂晶粒; (c), (f) 1个UN晶粒和1个U₃Si₂晶粒

Fig. 4. Equilibrium dihedral angles at evolution times 500 × 10⁴ $ \Delta t $((a)–(c)) and 1500 × 10⁴ $ \Delta t $((d)–(f)): (a), (d) Two UN grains; (b), (e) two U₃Si₂ grains; (c), (f) one UN grain and one U₃Si₂ grain.

下载: 全尺寸图片幻灯片

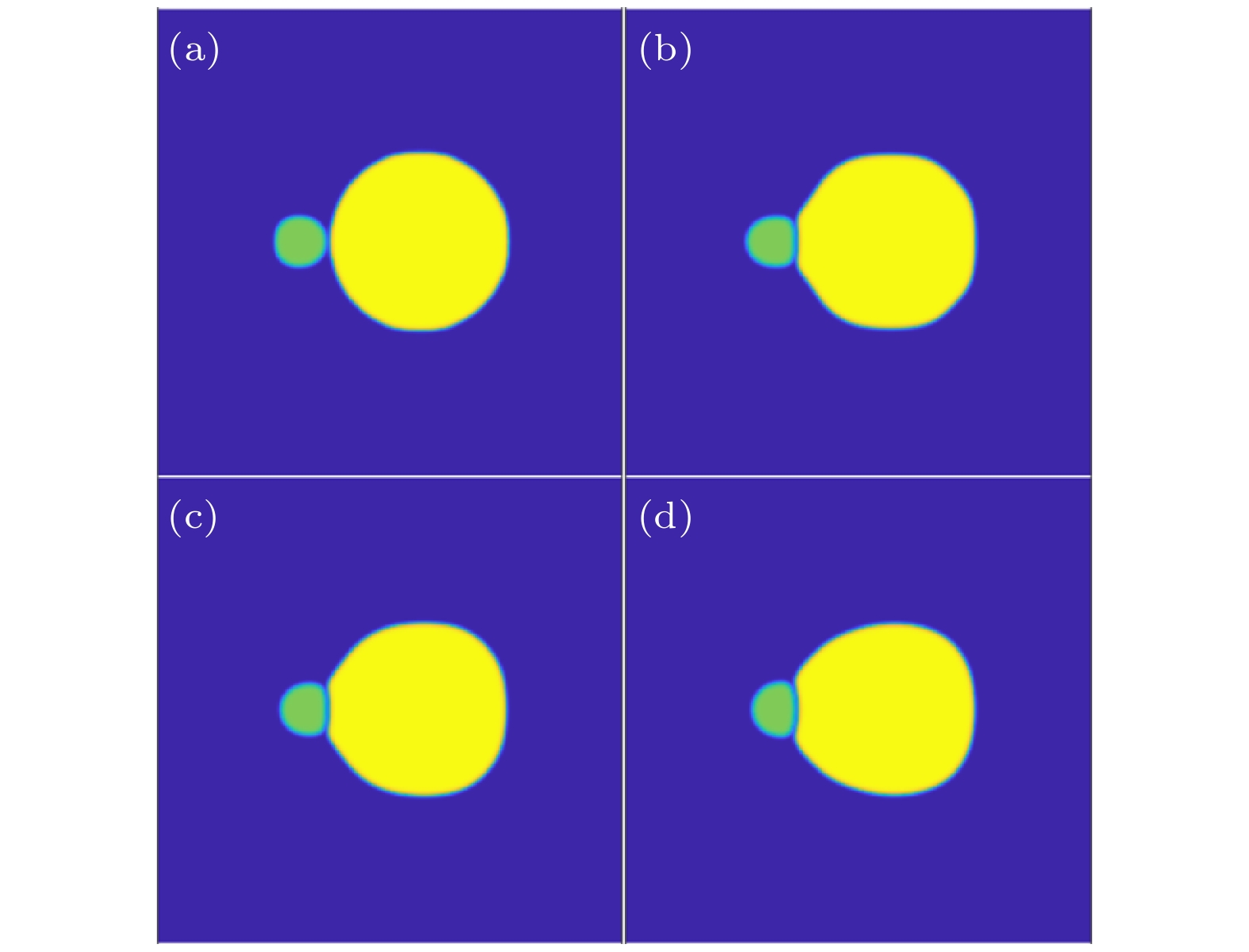
图 5 两个不同相不等大圆形晶粒演化的相场模拟　(a) 2 × 10⁴ $ \Delta t $; (b) 50 × 10⁴ $ \Delta t $; (c) 100 × 10⁴ $ \Delta t $; (d) 500 × 10⁴ $ \Delta t $.

Fig. 5. Phase-field simulation of the evolution of two unequal-sized circular grains with different phases: (a) 2 × 10⁴ $ \Delta t $; (b) 50 × 10⁴ $ \Delta t $; (c) 100 × 10⁴ $ \Delta t $; (d) 500 × 10⁴ $ \Delta t $.

下载: 全尺寸图片幻灯片

图 6 晶粒面积与烧结颈尺寸的演化曲线

Fig. 6. Evolution curves of grain area and sintering neck sizes.

下载: 全尺寸图片幻灯片

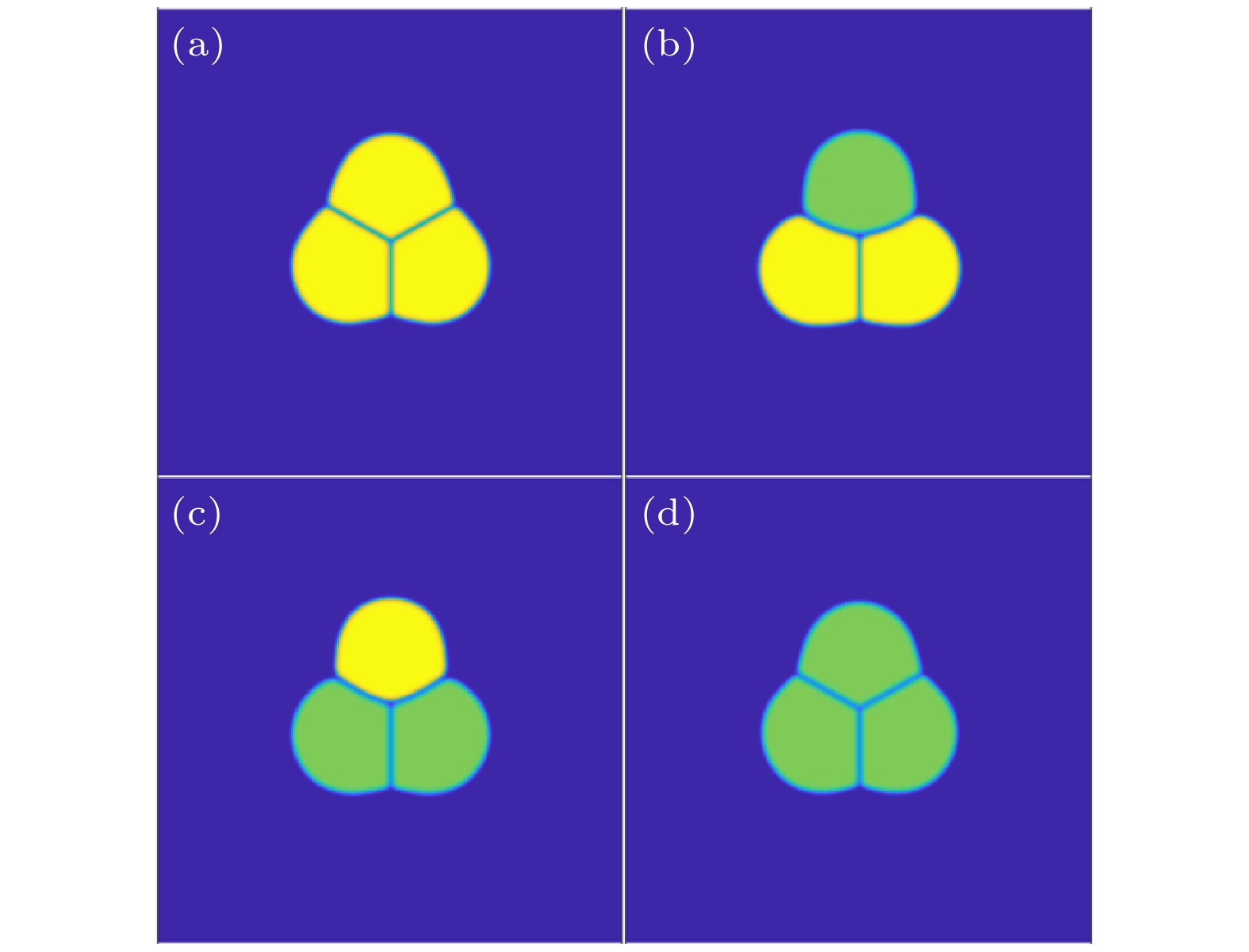
图 7 四种不同相晶粒个数比例下3个晶粒烧结的三叉晶界　(a) 3个UN晶粒; (b) 2个UN晶粒和 1个U₃Si₂晶粒; (c) 1个UN晶粒和2个U₃Si₂晶粒; (d) 3个U₃Si₂晶粒

Fig. 7. Trident grain boundaries of the sintering of three grains with four different phase grain number ratios: (a) Three UN grains; (b) two UN grains and one U₃Si₂ grain; (c) one UN grain and two U₃Si₂ grains; (d) three U₃Si₂ grains.

下载: 全尺寸图片幻灯片

图 8 三叉晶界处气孔率的演化曲线

Fig. 8. Evolution curves of the porosity at triple grain boundaries.

下载: 全尺寸图片幻灯片

图 9 不同体积分数比的两相多晶组织初始形貌　(a) 80% α-20% β; (b) 70% α-30% β; (c) 60% α-40% β; (d) 50% α-50% β

Fig. 9. Initial morphology of two-phase polycrystalline structures with different volume fraction ratios: (a) 80% α-20% β; (b) 70% α-30% β; (c) 60% α-40% β; (d) 50% α-50% β.

下载: 全尺寸图片幻灯片

图 10 不同体积分数比的两相多晶组织最终形貌　(a) 80% α-20% β; (b) 70% α-30% β; (c) 60% α-40% β; (d) 50% α-50% β

Fig. 10. Final morphology of two-phase polycrystalline structures with different volume fraction ratios: (a) 80% α-20% β; (b) 70% α-30% β; (c) 60% α-40% β; (d) 50% α-50% β.

下载: 全尺寸图片幻灯片

表 1 UN的物理参数^[27–29]

Table 1. Physical parameters of UN at 1823 K^[27–29]

Parameter	Value	Unit	Ref.
$ D_\alpha ^{\text{s}} $	7.5 × 10^–12	m²·s^–1	[27]
$ D_\alpha ^{{\text{gb}}} $	$ 0.01D_\alpha ^{\text{s}} $	m²·s^–1	[28]
$ \gamma _{\text{s}}^\alpha $	1.6	J·m^–2	[29]
$ \gamma _{{\text{gb}}}^\alpha $	0.8	J·m^–2	[29]
δ	6	nm	[29]

下载: 导出CSV

表 2 U₃Si₂的物理参数^[30–32]

Table 2. Physical parameters of U₃Si₂ at 1823 K^[30–32]

Parameter	Value	Unit	Ref.
$ D_\beta ^{\text{s}} $	100$ D_\beta ^{{\text{gb}}} $	m²·s^–1
$ D_\beta ^{{\text{gb}}} $	6.5923 × 10^–10	m²·s^–1	[30]
$ \gamma _{\text{s}}^\beta $	2.0	J·m^–2	[31]
$ \gamma _{{\text{gb}}}^\beta $	1.3	J·m^–2	[32]
δ	6	nm	[32]
Note: $ D_\alpha ^{\text{s}} $, $ D_\beta ^{\text{s}} $— surface diffusivity; $ D_\alpha ^{{\text{gb}}} $, $ D_\beta ^{{\text{gb}}} $— grain-boundary diffusivity; $ \gamma _{\text{s}}^\alpha $, $ \gamma _{\text{s}}^\beta $— surface energy; $ \gamma _{{\text{gb}}}^\alpha $, $ \gamma _{{\text{gb}}}^\beta $— grain-boundary energy; δ — diffuse interface width.

下载: 导出CSV

表 3 模拟中的无量纲参数表

Table 3. Non-dimensional parameters used in simulation.

Parameter	Value	Parameter	Value
$ \tilde A({\varphi _\alpha } = 1, {\text{ }}{\varphi _\beta } = 0) $	17	$ {\tilde \kappa _\eta } $	6.75
$ \tilde A({\varphi _\alpha } = 0, {\text{ }}{\varphi _\beta } = 1) $	11.5	$\tilde {\boldsymbol{M}}({\varphi _\alpha } = 1, {\text{ }}{\varphi _\beta } = 0)$	6817.5
$ \tilde B $	1	$\tilde {\boldsymbol{M}}({\varphi _\alpha } = 0, {\varphi _\beta } = 1)$	6817.5
$ {\tilde \kappa _\rho }({\varphi _\alpha } = 1, {\varphi _\beta } = 0) $	20.25	$ \tilde L $	1
$ {\tilde \kappa _\rho }({\varphi _\alpha } = 0, {\text{ }}{\varphi _\beta } = 1) $	14	$ \Delta x = \Delta y $	1
$ {\tilde \kappa _\phi }({\varphi _\alpha } = 1, {\text{ }}{\varphi _\beta } = 0) $	20.25	$ \Delta t $	2 × 10^–5
$ {\tilde \kappa _\phi }({\varphi _\alpha } = 0, {\text{ }}{\varphi _\beta } = 1) $	14
Note: $\tilde A$, $\tilde B$, $ {\tilde \kappa _\rho } $, $ {\tilde \kappa _\phi } $, $ {\tilde \kappa _\eta } $— non-dimensional parameters of free energy function; $ \tilde {\boldsymbol{M}} $— non-dimensional mobility; $\tilde L$—non-dimensional Allen-Cahn mobility; ∆x, ∆y — space scale; ∆t — time scale.

下载: 导出CSV

map

[1]	Kim H G, Yang J H, Kim W J, Koo Y H 2016 Nucl. Eng. Technol. 48 1 Google Scholar
[2]	Kurata M 2016 Nucl. Eng. Technol. 48 26 Google Scholar
[3]	Leenaers A, Van den Berghe S, Koonen E, Jacquet P, Jarousse C, Guigon B, Ballagny A, Sannen L 2004 J. Nucl. Mater. 327 121 Google Scholar
[4]	Zinkle S J, Terrani K A, Gehin J C, Ott L J, Snead L L 2014 J. Nucl. Mater. 448 374 Google Scholar
[5]	Terrani K A, Wang D, Ott L J, Montgomery R O 2014 J. Nucl. Mater. 448 512 Google Scholar
[6]	Johnson K D, Raftery A M, Lopes D A, Wallenius J 2016 J. Nucl. Mater. 477 18 Google Scholar
[7]	Watkins J K, Gonzales A, Wagner A R, Sooby E S, Jaques B J 2021 J. Nucl. Mater. 553 153048 Google Scholar
[8]	Wood E S, White J T, Nelson A T 2017 J. Nucl. Mater 484 245 Google Scholar
[9]	Ortega L H, Blamer B J, Evans J A, McDeavitt S M 2016 J. Nucl. Mater. 471 116 Google Scholar
[10]	White J T, Travis A W, Dunwoody J T, Nelson A T 2017 J. Nucl. Mater. 495 463 Google Scholar
[11]	Lopes D A, Uygur S, Johnson K D 2017 J. Nucl. Sci. Technol. 54 405 Google Scholar
[12]	刘续希, 高士森, 喇永孝, 玉栋梁, 柳文波 2024 73 148201 Google Scholar Liu X X, Gao S S, La Y X, Yu D L, Liu W B 2024 Acta Phys. Sin. 73 148201 Google Scholar
[13]	刘东昆, 王庆宇, 张田, 周羽, 王翔 2024 73 066102 Google Scholar Liu D K, Wang Q Y, Zhang T, Zhou Y, Wang X 2024 Acta Phys. Sin. 73 066102 Google Scholar
[14]	王凯乐, 杨文奎, 史新成, 侯华, 赵宇宏 2023 72 076102 Google Scholar Wang K L, Yang W K, Shi X C, Hou H, Zhao Y H 2023 Acta Phys. Sin. 72 076102 Google Scholar
[15]	刘明治, 张瑞杰, 方伟, 章书周, 曲选辉 2012 金属学报 48 1207 Google Scholar Liu M Z, Zhang R J, Fang W, Zhang S Z, Qu X H 2012 Acta. Metall. Sin. 48 1207 Google Scholar
[16]	Kumar V, Fang Z Z, Fife P C 2010 Mater. Sci. Eng. A 528 254 Google Scholar
[17]	Biswas S, Schwen D, Wang H, Okuniewski M, Tomar V 2018 Comput. Mater. Sci. 148 307 Google Scholar
[18]	Du L F, Yang S M, Zhu X W, Jiang J, Hui Q, Du H L 2018 J. Mater. Sci. 53 9567 Google Scholar
[19]	Hötzer J, Seiz M, Kellner M, Rheinheimer W, Nestler B 2019 Acta Mater. 164 184 Google Scholar
[20]	Wang Y U 2006 Acta Mater. 54 953 Google Scholar
[21]	Fan D, Chen L Q 1997 Acta Mater. 45 611 Google Scholar
[22]	Moelans N, Blanpain B, Wollants P 2008 Phys. Rev. B 78 024113 Google Scholar
[23]	Ahmed K, Yablinsky C A, Schulte A, Allen T, El-Azab A 2013 Modell. Simul. Mater. Sci. Eng. 21 065005 Google Scholar
[24]	Cahn J W 1961 Acta Metall. 9 795 Google Scholar
[25]	Allen S M, Cahn J W 1979 Acta Metall. 27 1085 Google Scholar
[26]	Biner S B 2017 Programming Phase-Field Modeling (Switzerland: Springer International Publishing) p18
[27]	Holt J B, Almassy M Y 1969 J. Am. Ceram. Soc. 52 631 Google Scholar
[28]	戚晓勇, 柳文波, 何宗倍, 王一帆, 恽迪 2023 金属学报 59 1513 Google Scholar Qi X Y, Liu W B, He Z B, Wang Y F, Yun D 2023 Acta Metall. Sin. 59 1513 Google Scholar
[29]	Bocharov D, Gryaznov D, Zhukovskii Y F, Kotomin E A 2013 J. Nucl. Mater. 435 102 Google Scholar
[30]	Cooper M W D, Gamble K A, Capolungo L, Matthews C, Andersson D A, Beeler B, Stanek C R, Metzger K 2021 J. Nucl. Mater. 555 153129 Google Scholar
[31]	Beeler B, Baskes M, Andersson D, Cooper M W D, Zhang Y F 2019 J. Nucl. Mater. 514 290 Google Scholar
[32]	Cheniour A, Tonks M R, Gong B, Yao T K, He L F, Harp J M, Beeler B, Zhang Y F, Lian J 2020 J. Nucl. Mater 532 152069 Google Scholar
[33]	Chockalingam K, Kouznetsova V G, van der Sluis O, Geers M G D 2016 Comput. Methods Appl. Mech. Eng. 312 492 Google Scholar
[34]	Rahaman M N 1995 Ceramic Processing and Sintering (New York: Marcel Dekker) p446
[35]	Riedel H, Svoboda J 1993 Acta Metall. Mater. 41 1929 Google Scholar
[36]	孙正阳, 杨超, 柳文波 2020 金属学报 56 1295 Google Scholar Sun Z Y, Yang C, Liu W B 2020 Acta Metall. Sin. 56 1295 Google Scholar
[37]	Ahmed K, Allen T, El-Azab A 2016 J. Mater. Sci. 51 1261 Google Scholar
[38]	Yadav V, Vanherpe L, Moelans N 2016 Comput. Mater. Sci. 125 297 Google Scholar

[1]	刘续希, 高士森, 喇永孝, 玉栋梁, 柳文波. Zr-2.5Sn合金高温腐蚀过程的相场模拟. , 2024, 73(14): 148201. doi: 10.7498/aps.73.20240393
[2]	姜彦博, 柳文波, 孙志鹏, 喇永孝, 恽迪. 外加应力作用下 UO₂ 中空洞演化过程的相场模拟. , 2022, 71(2): 026103. doi: 10.7498/aps.71.20211440
[3]	宋岩, 江鸿翔, 赵九洲, 何杰, 张丽丽, 李世欣. Al-Ti-B细化工业纯铝凝固组织演变过程数值模拟. , 2021, 70(8): 086402. doi: 10.7498/aps.70.20201431
[4]	杨朝曦, 柳文波, 张璁雨, 贺新福, 孙正阳, 贾丽霞, 师田田, 恽迪. Fe-Cr合金晶界偏析及辐照加速晶界偏析的相场模拟. , 2021, 70(11): 116101. doi: 10.7498/aps.70.20201840
[5]	刘迪, 王静, 王俊升, 黄厚兵. 相场模拟应变调控PbZr_(1–x)Ti_xO₃薄膜微观畴结构和宏观铁电性能. , 2020, 69(12): 127801. doi: 10.7498/aps.69.20200310
[6]	郝刚领, 许巧平, 李先雨, 王伟国. 金属粉末压坯烧结过程的内耗研究. , 2019, 68(12): 126101. doi: 10.7498/aps.68.20190031
[7]	张军, 陈文雄, 郑成武, 李殿中. γ-α相变中不同晶界特征下铁素体生长形貌的相场模拟. , 2017, 66(7): 070701. doi: 10.7498/aps.66.070701
[8]	段培培, 邢辉, 陈志, 郝冠华, 王碧涵, 金克新. 镁基合金自由枝晶生长的相场模拟研究. , 2015, 64(6): 060201. doi: 10.7498/aps.64.060201
[9]	杜立飞, 张蓉, 邢辉, 张利民, 张洋, 刘林. 横向限制下凝固微观组织演化的相场法模拟. , 2013, 62(10): 106401. doi: 10.7498/aps.62.106401
[10]	陈熙, 林正喆, 殷聪, 汤浩, 胡蕴成, 宁西京. 铂纳米颗粒生长和表面结构的理论预测. , 2012, 61(7): 076801. doi: 10.7498/aps.61.076801
[11]	潘诗琰, 朱鸣芳. 双边扩散枝晶生长的定量相场模型. , 2012, 61(22): 228102. doi: 10.7498/aps.61.228102
[12]	魏承炀, 李赛毅. 温度梯度对晶粒生长行为影响的相场模拟. , 2011, 60(10): 100701. doi: 10.7498/aps.60.100701
[13]	王刚, 徐东生, 杨锐. Ti-6Al-4V合金中片层组织形成的相场模拟. , 2009, 58(13): 343-S348. doi: 10.7498/aps.58.343
[14]	胡建民, 信江波, 吕强, 王月媛, 荣剑英. (Sb2Te3)0.75(1－x)(Bi2Te3)0.25(1－x)(Sb2Se3)x机械合金化粉体的制备及其冷压烧结样品的热电性能研究. , 2006, 55(9): 4843-4848. doi: 10.7498/aps.55.4843
[15]	匡安龙, 刘兴翀, 路忠林, 任尚坤, 刘存业, 张凤鸣, 都有为. 稀释磁性半导体Sn1-xMnxO2的室温铁磁性. , 2005, 54(6): 2934-2937. doi: 10.7498/aps.54.2934
[16]	李正, 何叶青, 胡伯平, 王震西. 烧结Nd-Fe-B中的热力学影响. , 2005, 54(11): 5400-5404. doi: 10.7498/aps.54.5400
[17]	段鹤, 郑毓峰, 张校刚, 孙言飞, 董有忠. 水热法合成FeS2粉晶及其生长热动力学的研究. , 2005, 54(4): 1659-1664. doi: 10.7498/aps.54.1659
[18]	黄　锐, 林璇英, 余云鹏, 林揆训, 姚若河, 黄文勇, 魏俊红, 王照奎, 余楚迎. 多晶硅薄膜低温生长中晶粒大小的控制. , 2004, 53(11): 3950-3955. doi: 10.7498/aps.53.3950
[19]	张建民, 徐可为. 银和铜膜中异常晶粒生长和织构变化的实验研究. , 2003, 52(1): 145-149. doi: 10.7498/aps.52.145
[20]	张建民, 徐可为. 面心立方多晶薄膜中应变能密度对晶粒取向的依赖. , 2002, 51(11): 2562-2566. doi: 10.7498/aps.51.2562

计量

文章访问数: 488
PDF下载量: 33
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板