-
研究了玻色-费米超流混合体系中的相互作用调制隧穿动力学特性, 其中玻色子位于对称双势阱中, 费米子位于对称双势阱中心的简谐势阱中. 采用双模近似方法得到描述双势阱玻色-爱因斯坦凝聚的动力学特性方程组, 并将其与简谐势阱中分子玻色-爱因斯坦凝聚的Gross-Pitaevskii方程进行耦合. 通过对不同参数下玻色-费米混合体系中的隧穿现象进行数值研究, 发现简谐势阱中费米子与双势阱中玻色子的相互作用使双势阱玻色-爱因斯坦凝聚的隧穿动力学特性更加丰富. 不但驱使双势阱中玻色-爱因斯坦凝聚从类约瑟夫森振荡转变为宏观量子自囚禁, 而且宏观量子自囚禁表现为三种不同的形式: 相位与时间呈负相关并随时间单调减小的自囚禁、 相位随时间演化有界的自囚禁以及相位与时间呈正相关并随时间单调增大的自囚禁.In this paper, we study the interaction-modulated tunneling dynamics of a Bose-Fermi superfluid mixture, where a Bose-Einstein condensate (BEC) with weak repulsive interaction is confined in a symmetric deep double-well potential and an equally populated two-component Fermi gas in a harmonic potential symmetrically is positioned in the center of the double-well potential. The tunneling between the two wells is modulated by fermions trapped in a harmonic potential. When the temperature is adequately low and the bosonic particle number is adequately large, we can employ the mean-field theory to describe the evolution of the BEC in the double-well potential through the time-dependent Gross-Pitaevskii equation. For the Fermi gas in the harmonic potential trap, we consider the case where the inter-fermion interaction is tuned on the deep Bose-Einstein condensate of the inter-fermion Feshbach resonance, where two fermions of spin-up and spin-down form a two-body bound state. Within the regime, the Fermi gas is well described by a condensate of these fermionic dimers, and hence can be simulated as well by a Gross-Pitaevskii equation of dimers. The inter-species interactions couple the dynamics of the two species, which results in interesting features in the tunneling oscillations. The dynamic equations of the BEC in the double-well potential is described by a two-mode approximation. Coupling it with time-dependent Gross-Pitaevskii equation of the harmonically potential trapped molecular BEC, we numerically investigate the dynamical evolution of the Boson-Fermi hybrid system under different initial conditions. It is found that the interaction among fermions in a harmonic potential leads to strong non-linearity in the oscillations of the bosons in the double-well potential and enriches the tunneling dynamics of the bosons. Especially, it strengthens macroscopic quantum self-trapping. And the macroscopic quantum self-trapping can be expressed in three forms: the phase tends to be negative and monotonically decreases with time, the phase evolves with time, and the phase tends to be positive and increases monotonically with time. This means that it is possible the tunneling dynamics of the BEC in double-well potential is adjustable. Our results can be verified experimentally in a Bose-Fermi superfluid mixture by varying different interaction parameters via Feshbach resonance and confinement-induced resonance.
-
Keywords:
- Bose-Fermi mixture /
- tunneling dynamics /
- Josephson oscillation /
- self-trapping
[1] Anderson M H, Ensher J R, Matthews M R, Wieman C E, Cornell E A 1995 Science 269 198
Google Scholar
[2] Hadzibabic Z, Stan C A, Dieckmann K, Gupta S, Ketterle W 2002 Phys. Rev. Lett. 88 160401
Google Scholar
[3] Wu C H, Santiago I, Park J W, Ahmadi P, Zwierlein M W 2011 Phys. Rev. A 84 011601
Google Scholar
[4] Stan C A, Zwierlein M W, Schunck C H, Raupach S M F, Ketterle W 2004 Phys. Rev. Lett. 93 143001
Google Scholar
[5] Deh B, Marzok C, Zimmermann C, Courteille P W 2008 Phys. Rev. A 77 010701
[6] 陈海霞 2009 博士学位论文 (太原: 山西大学)
Chen HX 2009 Ph. D. Dissertation (Taiyuan: Shanxi University) (in Chinese)
[7] 王汉权 2012 玻色-爱因斯坦凝聚中的量化涡旋及其动力学 (北京: 科学出版社)
Wang H Q 2012 Quantized Vortex States and Dynamics for Bose-Einstein Condensates (Beijing: Science Press) (in Chinese)
[8] Wang Y S, Li Z Y, Zhou Z W, Diao X F 2014 Phys. Lett. A 378 48
Google Scholar
[9] Karpiuk T, Brewczyk M, Ospelkaus-Schwarzer S, Bongs K, Rzazewski K 2004 Phys. Rev. Lett. 93 100401
Google Scholar
[10] Titvinidze I, Snoek M, Hofstetter W 2009 Phys. Rev. B 79 144506
Google Scholar
[11] Chen Q J, Wang J B, Sun L, Yu Y 2020 Chin. Phys. Lett. 37 053702
Google Scholar
[12] Yang S F, Zhou T W, Li C, Yang K X, Zhai Y Y, Yue X G, Chen X Z 2020 Chin. Phys. Lett. 37 040301
Google Scholar
[13] Cheng Y, Adhikari S K 2011 Phys. Rev. A 84 023632
Google Scholar
[14] Wang J B, Pan J S, Cui X L, Yi W 2020 Chin. Phys. Lett. 37 076701
Google Scholar
[15] Wu B, Niu Q 2000 Phys. Rev. A 61 023402
Google Scholar
[16] Ye D F, Fu L B, Jie L 2008 Phys. Rev. A 77 013402
Google Scholar
[17] Raghavan S, Smerzi A, Fantoni S, Shenoy S R 1999 Phys. Rev. A 59 620
[18] Maraj M, Wang J B, Pan J S, Yi W 2017 Eur. Phys. J. D 71 300
Google Scholar
[19] Niu Z X, Zhang X, Zhang W 2019 Eur. Phys. J. D 73 112
Google Scholar
[20] Adhikari S K, Lu H, Pu H 2009 Phys. Rev. A 80 063607
Google Scholar
[21] Qi P T, Duan W S 2011 Phys. Rev. A 84 033627
Google Scholar
[22] Milburn G J, Corney J, Wright E M, Walls D F 1997 Phys. Rev. A 55 4318
Google Scholar
[23] Xiong B, Gong J, Pu H, Bao W, Li B 2009 Phys. Rev. A 79 013626
Google Scholar
[24] 李振威 2008 量子光学学报 14 426
Google Scholar
Li Z W 2008 J. Quantum Opt. 14 426
Google Scholar
[25] Wang Y S, Long P, Zhang B, Zhang H 2017 Can. J. Phys. 95 622
Google Scholar
[26] Erdmann J, Mistakidis S I, Schmelcher P 2018 Phys. Rev. A 98 053614
Google Scholar
[27] Caballero-Benítez S F, Ostrovskaya E A, Gulácsí M, Kivshar Y S 2009 J. Phys. B: At. Mol. Opt. Phys. 42 215308
Google Scholar
-
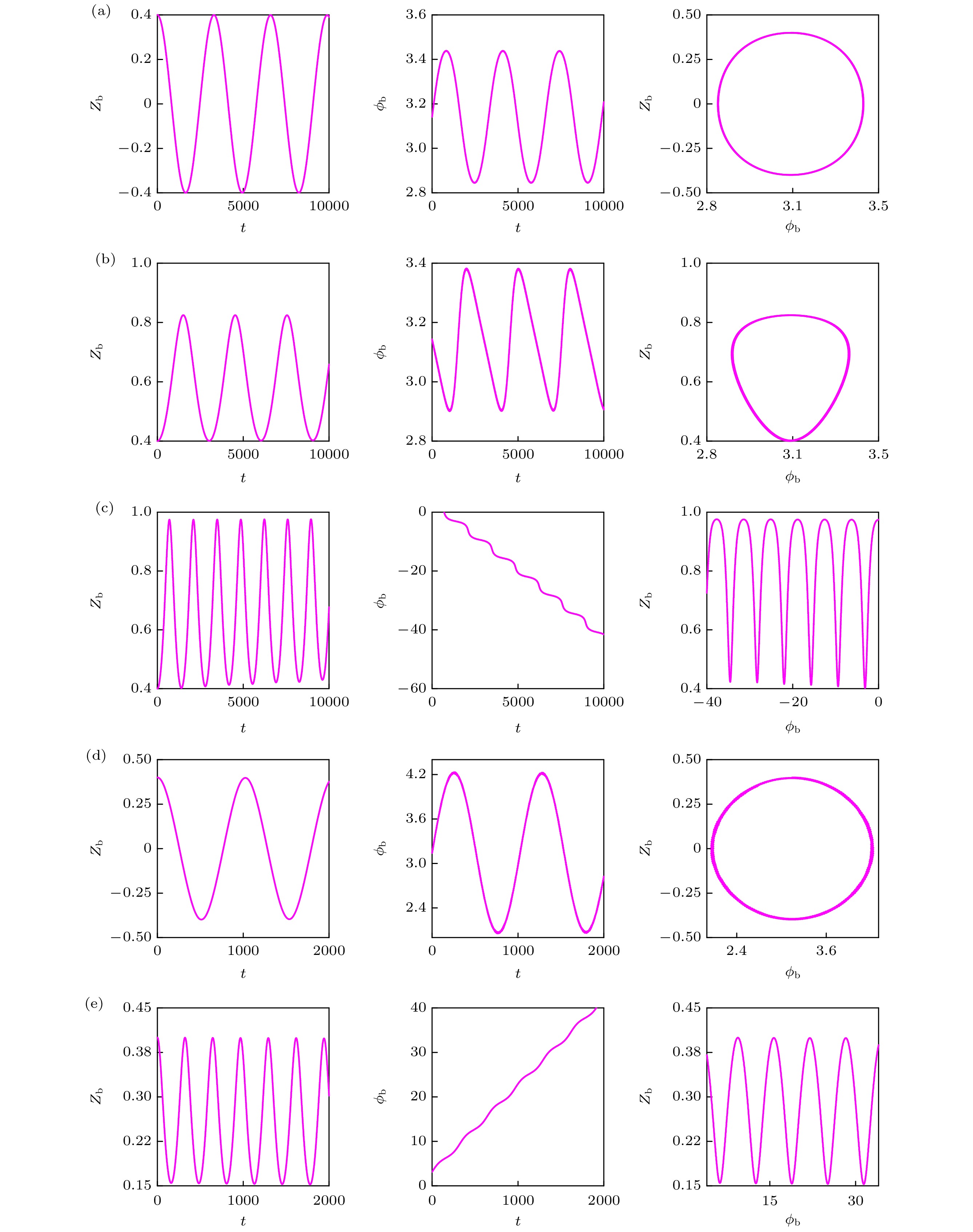
图 1 0-模时双势阱玻色子初始粒子布居数差
$ {Z_{\text{b}}}(t) $ 和相对相位$ {\phi _{\text{b}}}(t) $ 随时间$ t $ 的变化关系, 以及${Z_{\text{b}}}\text{-}{\phi _{\text{b}}}$ 相图 (a)$ {N_{\text{b}}} = 0.3{N_{\text{f}}} $ ; (b)$ {N_{\text{b}}} = {N_{\text{f}}} $ ; (c)$ {N_{\text{b}}} = 2.2{N_{\text{f}}} $ ; (d)$ {N_{\text{b}}} = 5.5{N_{\text{f}}} $ ; (e)$ {N_{\text{b}}} = 7{N_{\text{f}}} $ . (a)—(e)图中的初始条件为$ {Z_{\text{b}}}(0) = 0.6 $ ,$ {\phi _{\text{b}}}(0) = 0 $ ,$ {N_{\text{f}}} = 260 $ ,$ {g_{\text{b}}} = 2 \times {10^{ - 4}} $ ,$ {g_{\text{f}}} = 2 \times {10^{ - 4}} $ ,$ {g_{{\text{bf}}}} = 2 \times {10^{ - 2}} $ Fig. 1. For zero mode, population imbalance change with time
$ t $ , phase change with$ t $ and population imbalance change with the phase of the double-well: (a)$ {N_{\text{b}}} = 0.3{N_{\text{f}}} $ ; (b)$ {N_{\text{b}}} = {N_{\text{f}}} $ ; (c)$ {N_{\text{b}}} = 2.2{N_{\text{f}}} $ ; (d)$ {N_{\text{b}}} = 5.5{N_{\text{f}}} $ ; (e)$ {N_{\text{b}}} = 7{N_{\text{f}}} $ . For (a)–(e) figures the initial condition is set to$ {Z_{\text{b}}}(0) = 0.6 $ ,$ {\phi _{\text{b}}}(0) = 0 $ ,$ {N_{\text{f}}} = 260 $ ,$ {g_{\text{b}}} = 2 \times {10^{ - 4}} $ ,$ {g_{\text{f}}} = 2 \times {10^{ - 4}} $ ,$ {g_{{\text{bf}}}} = 2 \times {10^{ - 2}} $ .
图 2
$ {\text{π }} $ -模时双势阱玻色子初始粒子布居数差$ {Z_{\text{b}}}(t) $ 和相对相位$ {\phi _{\text{b}}}(t) $ 随时间$ t $ 的演化, 以及$ {Z_{\text{b}}}{\text{ - }}{\phi _{\text{b}}} $ 相图 (a)$ {N_{\text{b}}} = 1/30{N_{\text{f}}} $ ; (b)$ {N_{\text{b}}} = 0.1{N_{\text{f}}} $ ; (c)${{N}}_{\text{b}}={{N}}_{\text{f}}$ ; (d)$ {N_{\text{b}}} = 2{N_{\text{f}}} $ ; (e)$ {\text{N}}_{\text{b}}=\text{4}{\text{N}}_{\text{f}} $ . (a)—(e)图中的初始条件为$ {Z_{\text{b}}}(0) = 0.4 $ ,$ {\phi _{\text{b}}}(0) = {\text{π }} $ ,$ {N_{\text{f}}} = 300 $ ,${g_{\text{b}}} = $ $ 2 \times {10^{ - 4}}$ ,$ {g_{\text{f}}} = 2 \times {10^{ - 4}} $ ,$ {g_{{\text{bf}}}} = 1 \times {10^{ - 2}} $ .Fig. 2. For
$ {\text{π }} $ mode, population imbalance change with time$ t $ , phase change with$ t $ and population imbalance change with the phase of the double-well: (a)$ {N_{\text{b}}} = 1/30{N_{\text{f}}} $ ; (b)$ {N_{\text{b}}} = 0.1{N_{\text{f}}} $ ; (c)$ {N_{\text{b}}} = {N_{\text{f}}} $ ; (d)$ {N_{\text{b}}} = 2{N_{\text{f}}} $ ; (e)$ {N_{\text{b}}} = 4{N_{\text{f}}} $ . For (a)–(e) figures the initial condition is set to$ {Z_{\text{b}}}(0) = 0.4 $ ,$ {\phi _{\text{b}}}(0) = {\text{π }} $ ,$ {N_{\text{f}}} = 300 $ ,${g_{\text{b}}} = $ $ 2 \times {10^{ - 4}}$ ,$ {g_{\text{f}}} = 2 \times {10^{ - 4}} $ ,$ {g_{{\text{bf}}}} = 1 \times {10^{ - 2}} $ .
图 3
${\phi _{\text{b}}}{\text{-}}{Z_{\text{b}}}$ 平面内的相图. 费米子数$ {N_{\text{f}}} = 300 $ , 其中图(a)—(f)中,$ {N}_{\text{b}}=10, \text{ }30, \text{ }300, $ $ 600, \text{ }1800, \text{ }2100 $ . 红色线表示${\phi _{\text{b}}}(0) = $ $ 0$ 时的演化轨迹, 黑色线表示$ {\phi _{\text{b}}}(0) = {\text{π }} $ 时的演化轨迹, 蓝色的点线表示0-模时相轨迹转变的临界值, 绿色的点线表示$ \pi $ -模时相轨迹转变的临界值.Fig. 3. Phase diagram in the
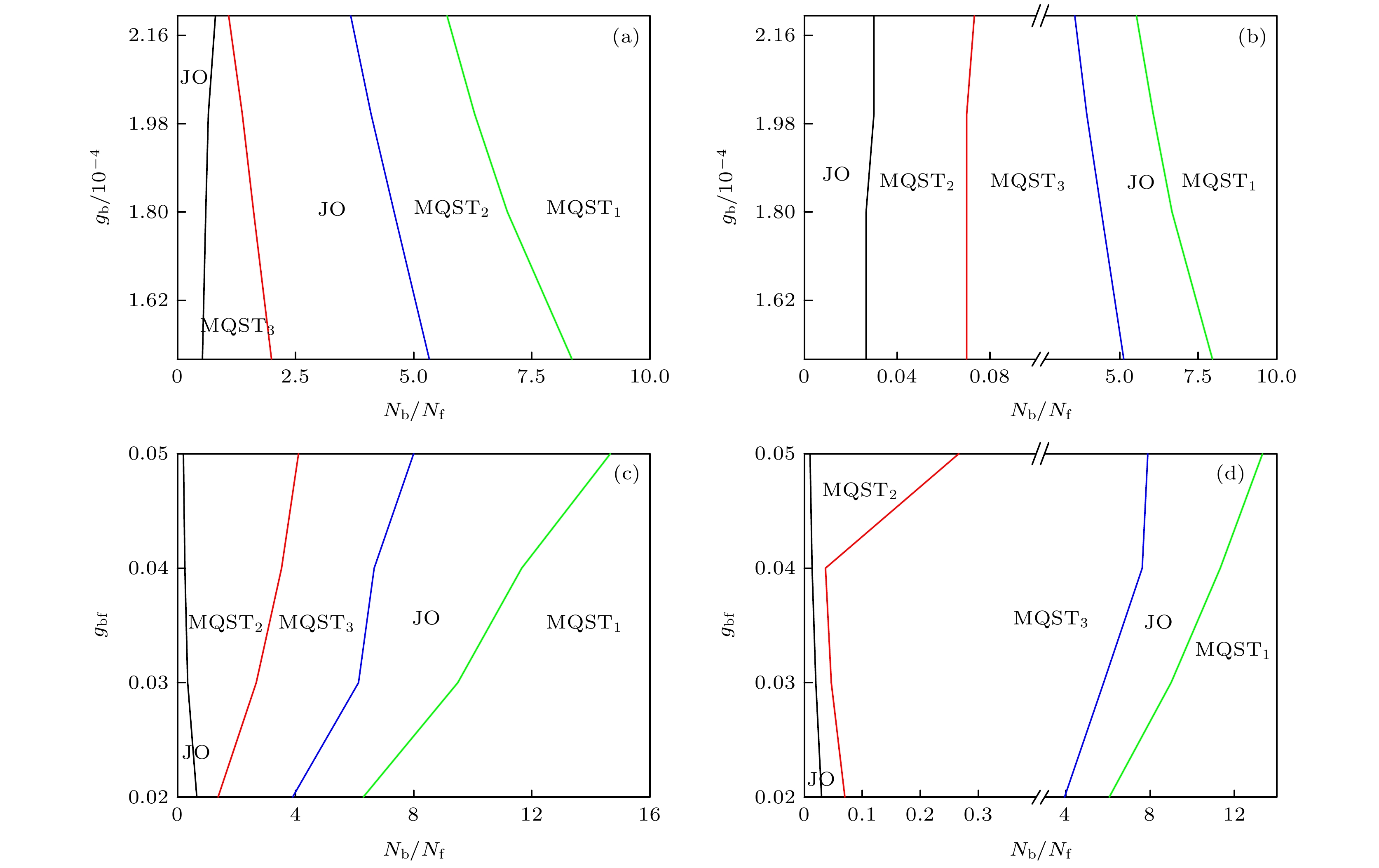
${\phi _{\text{b}}}\text{-}{Z_{\text{b}}}$ . The number of fermions is$ {N_{\text{f}}} = 300 $ with (a)–(f)$ {N_{\text{b}}} = 10, {\text{ }}30, {\text{ }}300, {\text{ }}600, {\text{ }}1800, {\text{ }}2100 $ . Trajectories with$ {\phi _{\text{b}}}(0) = 0 $ are depicted in red while those with$ {\phi _{\text{b}}}(0) = {\text{π }} $ are depicted in black. The blue dot line indicates the critical value of phase trajectory transition at 0 phase mode. The green dot line indicates the critical value of phase trajectory transition at$ {\text{π }} $ phase mode.
图 4 (a), (c) 0-模和(b), (d)
$ {\text{π }} $ -模时,${N_{\text{b}}}/{N_{\text{f}}}{\text{-}}{g_{\text{b}}}$ 和${N_{\text{b}}}/{N_{\text{f}}}{\text{-}}{g_{{\text{bf}}}}$ 平面内的相图, 其中(a), (b)参数为$ {g_{\text{f}}} = 2 \times {10^{ - 4}} $ ,${g_{{\text{bf}}}} = 2 \times $ $ {10^{ - 2}}$ ; (c), (d)参数为$ {g_{\text{b}}} = 2 \times {10^{ - 4}} $ ,$ {g_{\text{f}}} = 2 \times {10^{ - 4}} $ Fig. 4. For (a), (c) zero mode and (b), (d)
$ {\text{π }} $ mode, the phase diagram in the${N_{\text{b}}}/{N_{\text{f}}}{\text{-}}{g_{\text{b}}}$ and${N_{\text{b}}}/{N_{\text{f}}}{\text{-}}{g_{{\text{bf}}}}$ plane with (a), (b)$ {g_{\text{f}}} = 2 \times {10^{ - 4}} $ ,$ {g_{{\text{bf}}}} = 2 \times {10^{ - 2}} $ and (c), (d)$ {g_{\text{b}}} = 2 \times {10^{ - 4}} $ ,$ {g_{\text{f}}} = 2 \times {10^{ - 4}} $ . -
[1] Anderson M H, Ensher J R, Matthews M R, Wieman C E, Cornell E A 1995 Science 269 198
Google Scholar
[2] Hadzibabic Z, Stan C A, Dieckmann K, Gupta S, Ketterle W 2002 Phys. Rev. Lett. 88 160401
Google Scholar
[3] Wu C H, Santiago I, Park J W, Ahmadi P, Zwierlein M W 2011 Phys. Rev. A 84 011601
Google Scholar
[4] Stan C A, Zwierlein M W, Schunck C H, Raupach S M F, Ketterle W 2004 Phys. Rev. Lett. 93 143001
Google Scholar
[5] Deh B, Marzok C, Zimmermann C, Courteille P W 2008 Phys. Rev. A 77 010701
[6] 陈海霞 2009 博士学位论文 (太原: 山西大学)
Chen HX 2009 Ph. D. Dissertation (Taiyuan: Shanxi University) (in Chinese)
[7] 王汉权 2012 玻色-爱因斯坦凝聚中的量化涡旋及其动力学 (北京: 科学出版社)
Wang H Q 2012 Quantized Vortex States and Dynamics for Bose-Einstein Condensates (Beijing: Science Press) (in Chinese)
[8] Wang Y S, Li Z Y, Zhou Z W, Diao X F 2014 Phys. Lett. A 378 48
Google Scholar
[9] Karpiuk T, Brewczyk M, Ospelkaus-Schwarzer S, Bongs K, Rzazewski K 2004 Phys. Rev. Lett. 93 100401
Google Scholar
[10] Titvinidze I, Snoek M, Hofstetter W 2009 Phys. Rev. B 79 144506
Google Scholar
[11] Chen Q J, Wang J B, Sun L, Yu Y 2020 Chin. Phys. Lett. 37 053702
Google Scholar
[12] Yang S F, Zhou T W, Li C, Yang K X, Zhai Y Y, Yue X G, Chen X Z 2020 Chin. Phys. Lett. 37 040301
Google Scholar
[13] Cheng Y, Adhikari S K 2011 Phys. Rev. A 84 023632
Google Scholar
[14] Wang J B, Pan J S, Cui X L, Yi W 2020 Chin. Phys. Lett. 37 076701
Google Scholar
[15] Wu B, Niu Q 2000 Phys. Rev. A 61 023402
Google Scholar
[16] Ye D F, Fu L B, Jie L 2008 Phys. Rev. A 77 013402
Google Scholar
[17] Raghavan S, Smerzi A, Fantoni S, Shenoy S R 1999 Phys. Rev. A 59 620
[18] Maraj M, Wang J B, Pan J S, Yi W 2017 Eur. Phys. J. D 71 300
Google Scholar
[19] Niu Z X, Zhang X, Zhang W 2019 Eur. Phys. J. D 73 112
Google Scholar
[20] Adhikari S K, Lu H, Pu H 2009 Phys. Rev. A 80 063607
Google Scholar
[21] Qi P T, Duan W S 2011 Phys. Rev. A 84 033627
Google Scholar
[22] Milburn G J, Corney J, Wright E M, Walls D F 1997 Phys. Rev. A 55 4318
Google Scholar
[23] Xiong B, Gong J, Pu H, Bao W, Li B 2009 Phys. Rev. A 79 013626
Google Scholar
[24] 李振威 2008 量子光学学报 14 426
Google Scholar
Li Z W 2008 J. Quantum Opt. 14 426
Google Scholar
[25] Wang Y S, Long P, Zhang B, Zhang H 2017 Can. J. Phys. 95 622
Google Scholar
[26] Erdmann J, Mistakidis S I, Schmelcher P 2018 Phys. Rev. A 98 053614
Google Scholar
[27] Caballero-Benítez S F, Ostrovskaya E A, Gulácsí M, Kivshar Y S 2009 J. Phys. B: At. Mol. Opt. Phys. 42 215308
Google Scholar
下载:
计量
- 文章访问数: 4323
- PDF下载量: 73
- 被引次数: 0
