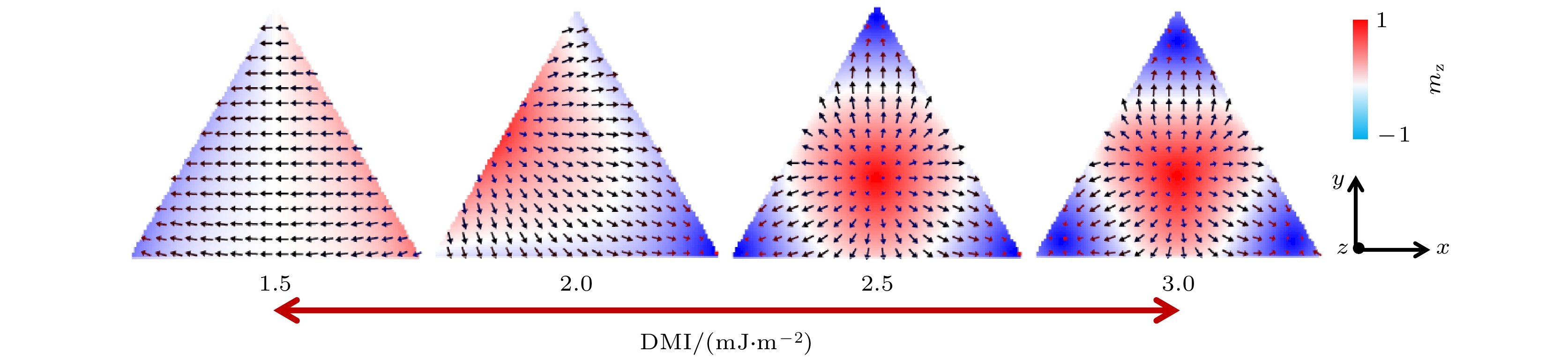
-
具有手型和极性双重属性的磁涡旋结构, 自发现以来就被视为有望成为下一代自旋电子学器件的可能载体之一. 尤其近年来随着Dzyaloshinskii–Moriya相互作用的发现, 在具有中心反演对称性破缺或强自旋轨道耦合的体系中, 使得一般的面内磁涡旋具有更加多样的动力学行为. 本文通过微磁学模拟的方法, 系统研究了在等边三角形结构中磁涡旋能够稳定存在的条件, 并在此基础上分别通过施加面内和面外两个方向的微波磁场来激励其振荡, 其中除常见的面内旋转模式和面外呼吸模式外, 还存在高频微波磁场下的分裂模式, 以及呼吸旋转同步的自旋波模式. 最后, 通过改变体系中Dzyaloshinskii–Moriya有效场的强度来改变整个三角形中的磁结构, 进而调控不同自旋波模式的本征频率. 本文结果对研究自旋波模式的多样性具有一定的借鉴意义, 并且为多类型的自旋波模式能够在相关自旋电子学器件的研发提供更多选择.As a kind of nanoscale magnetic structure, the magnetic vortex has the advantages of small size, easy integration, easy control, low driving current density, low heat loss, etc. Owing to its potential application value and research significance, it has received more and more attention since its discovery.The existence of the magnetic vortex is the result of the competition between the exchange energy and the magnetostatic energy in the system. The magnetization of magnetic vortex usually contains the in-plane part and the central region part, so it usually has dual properties of chirality and polarity. The chirality is related to the arrangement of the magnetization in the plane, which can be divided into clockwise direction and counterclockwise direction. Moreover, the polarities +1 and –1 respectively represent the magnetization in the central area of the magnetic vortex core along the +z axis and –z axis. On the one hand, the magnetic vortex can be used as an information carrier in the storage device by driving the polarity reversal, and has the advantages of fast reading and writing speed, easy erasing and rewriting. On the other hand, it is expected to be used in next-generation spintronic devices, such as spin nano-oscillators based on magnetic vortex, which can continuously output high-frequency microwave signals. To further enhance the applicability of magnetic vortex, the Dzyaloshinskii–Moriya interaction (DMI) is introduced into the system, with symmetry breaking or strong spin-orbit coupling, and its dynamic process can be regulated by changing the magnetic vortex structure. The DM effective field plays a role in forcing the adjacent magnetization to be along the perpendicular direction in the heterostructure system lacking interface inversion symmetry. Thus, the existence of DMI can make the in-plane magnetization oriented to the out-of-plane direction. In this work, the triangle-shape magnetic vortex structure is varied by changing the strength of DM effective field. The microwave magnetic fields are respectively applied along the in-plane direction and out-of-plane direction, and the eigenfrequencies are obtained by using fast Fourier transform. Next, we further explore the spin wave modes at different eigenfrequencies. Finally, we vary the intensity of DMI in the system to adjust different eigenfrequencies. These results open up possibilities for the development and application of magnetic vortex in spintronics.
-
Keywords:
- magnetic vortex /
- spin wave mode
[1] Vansteenkiste A, Chou K W, Weigand M, Curcic M, Sackmann V, Stoll H, Tyliszczak T, Woltersdorf G, Back C H, Schütz G, Waeyenberge B V 2009 Nat. Phys 5 332
Google Scholar
[2] Völkel A R, Wysin G M, Mertens F G, Bishop A R, Schnitzer H J 1994 Phys. Rev. B 50 12711
Google Scholar
[3] Thiele A A 1973 Phys. Rev. Lett 30 230
Google Scholar
[4] Choe S B, Acremann Y, Scholl A, Bauer A, Doran A, Stöhr J, Padmore H A 2004 Science 304 420
Google Scholar
[5] Kravchuk V P, Sheka D D, Gaididei Y, Mertens F G 2007 J. App. Phys 102 043908
Google Scholar
[6] Shinjo T, Okuno T, Hassdorf R, Shigeto K, Ono T 2000 Science 289 930
Google Scholar
[7] Wachowiak A, Wiebe J, Bode M, Pietzsch O, Morgenstern M, Wiesendanger R 2002 Science 298 577
Google Scholar
[8] Siracusano G, Tomasello R, Giordano A, Puliafito V, Azzerboni B, Ozatay O, Carpentieri M, Finocchio G 2016 Phys. Rev. Lett 117 087204
Google Scholar
[9] Huber D L 1982 J. Appl. Phys 53 1899
Google Scholar
[10] Xiao Q F, Rudge J, Choi B C, Hong Y K, Donohoe G 2006 Appl. Phys. Lett. 89 262507
Google Scholar
[11] Hertel R, Gliga S, Fahnle M, Schneider C M 2007 Phys. Rev. Lett. 98 117201
Google Scholar
[12] Weigand M, Waeyenberge B V, Vansteenkiste A, Curcic M, Sackmann V, Stoll H, Tyliszczak T, Kaznatcheev K, Bertwistle D, Woltersdorf G, Back C H, Schütz G 2009 Phys. Rev. Lett. 102 077201
Google Scholar
[13] Kim S K, Lee K S, Yu Y S, Choi Y S 2008 Appl. Phys. Lett. 92 022509
Google Scholar
[14] Bohlens S, Krüger B, Drews A, Bolte M, Meier G, Pfannkuche D 2008 Appl. Phys. Lett. 93 142508
Google Scholar
[15] Pigeau B, Loubens G d, Klein O, Riegler A, Lochner F, Schmidt G, Molenkamp L W, Tiberkevich V S, Slavin A N 2010 Appl. Phys. Lett. 96 132506
Google Scholar
[16] Yu X Z, Onose Y, Kanazawa N, Park J H, Han J H, Matsui Y, Nagaosa N, Tokura Y 2010 Nature 465 901
Google Scholar
[17] Huang S X, Chien C L 2012 Phys. Rev. Lett. 108 267201
Google Scholar
[18] Heinze S, Bergmann K v, Menzel M, Brede J, Kubetzka A, Wiesendanger R, Bihlmayer G, Blügel S 2011 Nat. Phys. 7 713
Google Scholar
[19] Dzyaloshinsky I 1958 J. Phys. Chem. Solids 4 241
Google Scholar
[20] Jaafar M, Yanes R, Asenjo A, Chubykalo-Fesenko O, Vázquez M, González E M, Vicent J L 2018 Nanotechnology 19 285717
[21] Jaafar M, Yanes R, Lara D P d, Chubykalo-Fesenko O, Asenjo A, Gonzalez E M, Anguita J V, Vazquez M, Vicent J L 2010 Phys. Rev. B 81 054439
Google Scholar
[22] Lin C S, Lim H S, Wang C C, Adeyeye A O, Wang Z K, Ng S C, Kuok M H 2010 J. Appl. Phys 108 114305
Google Scholar
[23] Brataas A, Kent A D, Ohno H 2012 Nat. Mater 11 372
Google Scholar
[24] Donahue M J, Porter D G 2020 New J. Phys 22 033001
Google Scholar
[25] Yoo M W, Lee J, Kim S K 2012 Appl. Phys. Lett. 100 172413
Google Scholar
[26] Zhang H, Liu Y W, Yan M, Hertel R 2010 IEEE Trans. Magn. 46 1675
Google Scholar
[27] Yan M, Leaf G, Kaper H, Camley R, Grimsditch M 2006 Phys. Rev. B 73 014425
Google Scholar
[28] Mruczkiewicz M, Gruszecki P, Krawczyk M, Guslienko K Y 2018 Phys. Rev. B 97 064418
Google Scholar
[29] Mruczkiewicz M, Krawczyk M, Guslienko K Y 2017 Phys. Rev. B 95 094414
Google Scholar
-
图 1 不同DMI有效场强度下的三角形结构中磁涡旋基态
Fig. 1. Ground state of magnetic vortex in triangular structure under different DMI constant.
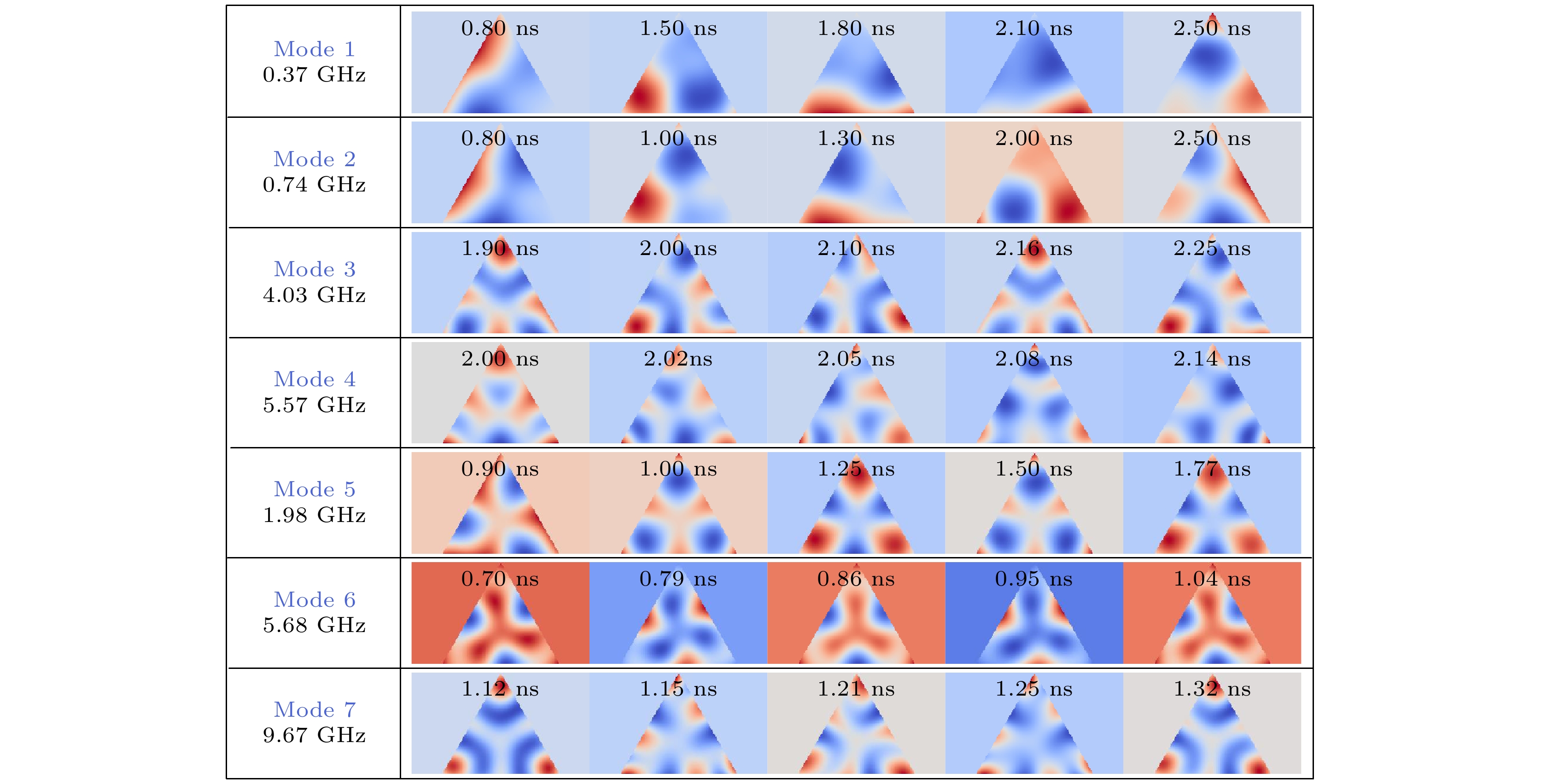
图 2 (a) sinc形式的磁场随时间变化情况; (b)和(c)分别为激励磁场在x和z方向上时, 磁涡旋的动态磁化率虚部Imχx和Imχz
Fig. 2. (a) The relationship between time and sinc-type microwave field; (b) and (c) are the imaginary part of dynamic susceptibility Imχx and Imχz when the exciting magnetic field is applied along in-plane direction and out-of-plane direction, respectively.
图 3 磁涡旋在x, y, z方向上不同自旋波模式的空间振幅及相位分布
Fig. 3. The spatial amplitude and phase distributions of the different spin wave mode of magnetic vortex in the x, y, and z directions
图 4 磁涡旋在对应本征频率的微波磁场下不同时刻的净余磁矩(
$ \delta {\mathit{m}}_{z} $ )Fig. 4. The net magnetization (
$ \delta {\mathit{m}}_{z} $ ) of the magnetic vortex at different time under the microwave magnetic field, which corresponding to the eigenfrequencies.
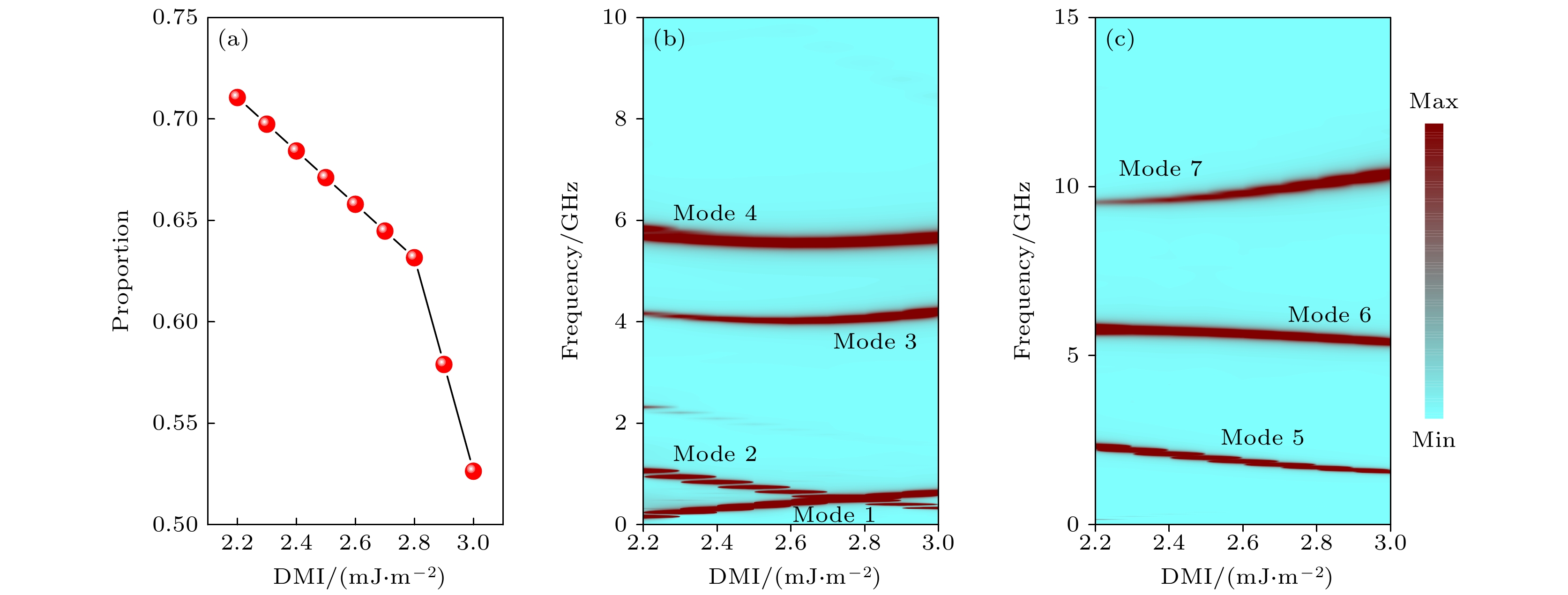
图 5 (a) 磁涡旋所在区域占三角形结构整体面积之比随DMI系数变化情况; (b)和(c)分别为激励磁场施加在面内和面外方向上时, 磁涡旋的DMI系数与共振频率的关系相图
Fig. 5. (a) The relationship between DMI constant and the ratio of the area where the magnetic vortex is located to the overall area of the triangular structure. Phase diagram of magnetic vortex as a function of DMI constant and resonance frequency, where (b) and (c) represent the excitation field applied in in-plane and out-of-plane directions, respectively.
-
[1] Vansteenkiste A, Chou K W, Weigand M, Curcic M, Sackmann V, Stoll H, Tyliszczak T, Woltersdorf G, Back C H, Schütz G, Waeyenberge B V 2009 Nat. Phys 5 332
Google Scholar
[2] Völkel A R, Wysin G M, Mertens F G, Bishop A R, Schnitzer H J 1994 Phys. Rev. B 50 12711
Google Scholar
[3] Thiele A A 1973 Phys. Rev. Lett 30 230
Google Scholar
[4] Choe S B, Acremann Y, Scholl A, Bauer A, Doran A, Stöhr J, Padmore H A 2004 Science 304 420
Google Scholar
[5] Kravchuk V P, Sheka D D, Gaididei Y, Mertens F G 2007 J. App. Phys 102 043908
Google Scholar
[6] Shinjo T, Okuno T, Hassdorf R, Shigeto K, Ono T 2000 Science 289 930
Google Scholar
[7] Wachowiak A, Wiebe J, Bode M, Pietzsch O, Morgenstern M, Wiesendanger R 2002 Science 298 577
Google Scholar
[8] Siracusano G, Tomasello R, Giordano A, Puliafito V, Azzerboni B, Ozatay O, Carpentieri M, Finocchio G 2016 Phys. Rev. Lett 117 087204
Google Scholar
[9] Huber D L 1982 J. Appl. Phys 53 1899
Google Scholar
[10] Xiao Q F, Rudge J, Choi B C, Hong Y K, Donohoe G 2006 Appl. Phys. Lett. 89 262507
Google Scholar
[11] Hertel R, Gliga S, Fahnle M, Schneider C M 2007 Phys. Rev. Lett. 98 117201
Google Scholar
[12] Weigand M, Waeyenberge B V, Vansteenkiste A, Curcic M, Sackmann V, Stoll H, Tyliszczak T, Kaznatcheev K, Bertwistle D, Woltersdorf G, Back C H, Schütz G 2009 Phys. Rev. Lett. 102 077201
Google Scholar
[13] Kim S K, Lee K S, Yu Y S, Choi Y S 2008 Appl. Phys. Lett. 92 022509
Google Scholar
[14] Bohlens S, Krüger B, Drews A, Bolte M, Meier G, Pfannkuche D 2008 Appl. Phys. Lett. 93 142508
Google Scholar
[15] Pigeau B, Loubens G d, Klein O, Riegler A, Lochner F, Schmidt G, Molenkamp L W, Tiberkevich V S, Slavin A N 2010 Appl. Phys. Lett. 96 132506
Google Scholar
[16] Yu X Z, Onose Y, Kanazawa N, Park J H, Han J H, Matsui Y, Nagaosa N, Tokura Y 2010 Nature 465 901
Google Scholar
[17] Huang S X, Chien C L 2012 Phys. Rev. Lett. 108 267201
Google Scholar
[18] Heinze S, Bergmann K v, Menzel M, Brede J, Kubetzka A, Wiesendanger R, Bihlmayer G, Blügel S 2011 Nat. Phys. 7 713
Google Scholar
[19] Dzyaloshinsky I 1958 J. Phys. Chem. Solids 4 241
Google Scholar
[20] Jaafar M, Yanes R, Asenjo A, Chubykalo-Fesenko O, Vázquez M, González E M, Vicent J L 2018 Nanotechnology 19 285717
[21] Jaafar M, Yanes R, Lara D P d, Chubykalo-Fesenko O, Asenjo A, Gonzalez E M, Anguita J V, Vazquez M, Vicent J L 2010 Phys. Rev. B 81 054439
Google Scholar
[22] Lin C S, Lim H S, Wang C C, Adeyeye A O, Wang Z K, Ng S C, Kuok M H 2010 J. Appl. Phys 108 114305
Google Scholar
[23] Brataas A, Kent A D, Ohno H 2012 Nat. Mater 11 372
Google Scholar
[24] Donahue M J, Porter D G 2020 New J. Phys 22 033001
Google Scholar
[25] Yoo M W, Lee J, Kim S K 2012 Appl. Phys. Lett. 100 172413
Google Scholar
[26] Zhang H, Liu Y W, Yan M, Hertel R 2010 IEEE Trans. Magn. 46 1675
Google Scholar
[27] Yan M, Leaf G, Kaper H, Camley R, Grimsditch M 2006 Phys. Rev. B 73 014425
Google Scholar
[28] Mruczkiewicz M, Gruszecki P, Krawczyk M, Guslienko K Y 2018 Phys. Rev. B 97 064418
Google Scholar
[29] Mruczkiewicz M, Krawczyk M, Guslienko K Y 2017 Phys. Rev. B 95 094414
Google Scholar
下载:
计量
- 文章访问数: 4014
- PDF下载量: 92
- 被引次数: 0
